A Comparison of Load Distribution Methods at the Node and Internal Force Analysis of the Lattice Beam Based on the Winkler Foundation Model
Abstract
:1. Introduction
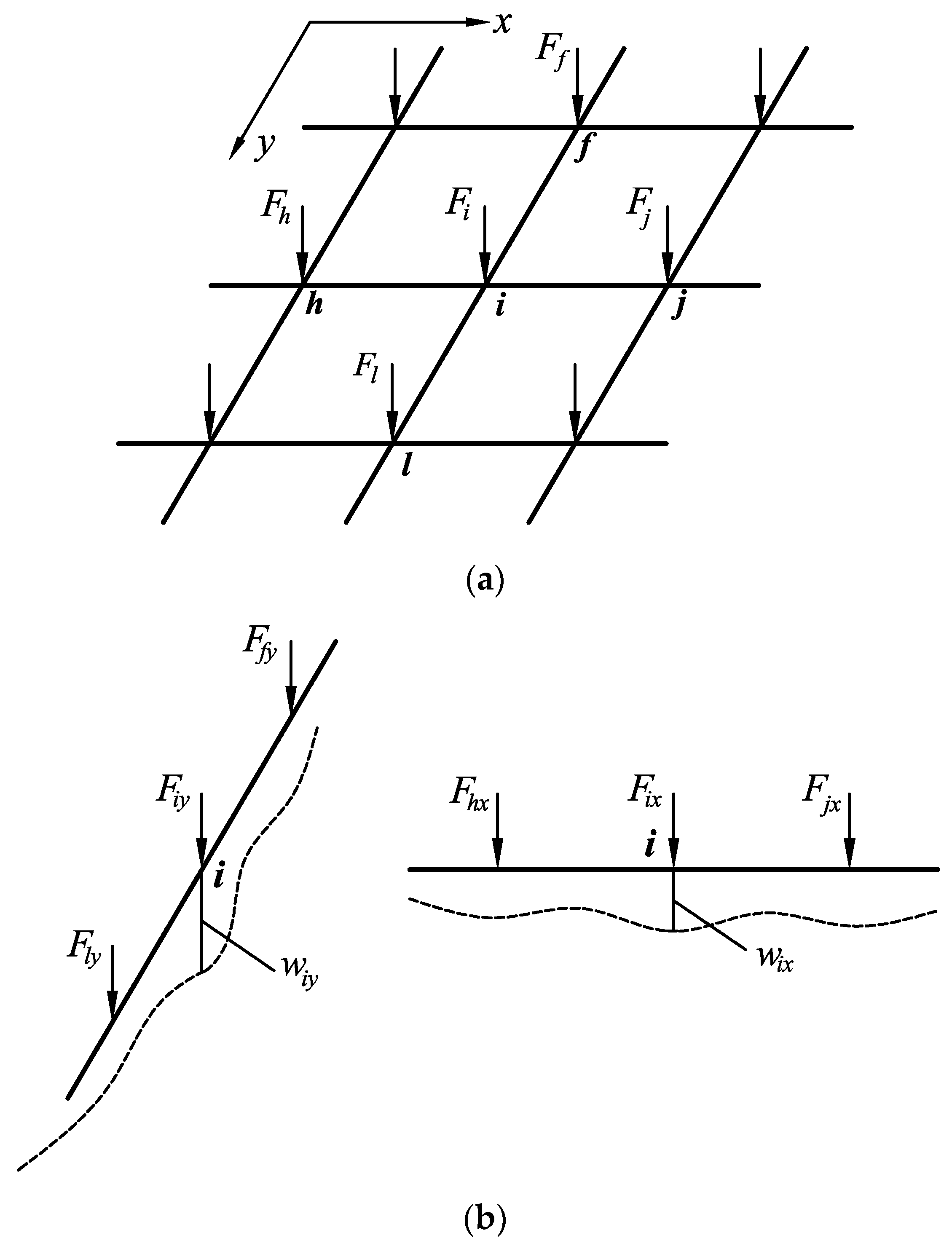
2. Load Distribution Methods at the Node for Lattice Beam
2.1. Simplified Load Distribution Method
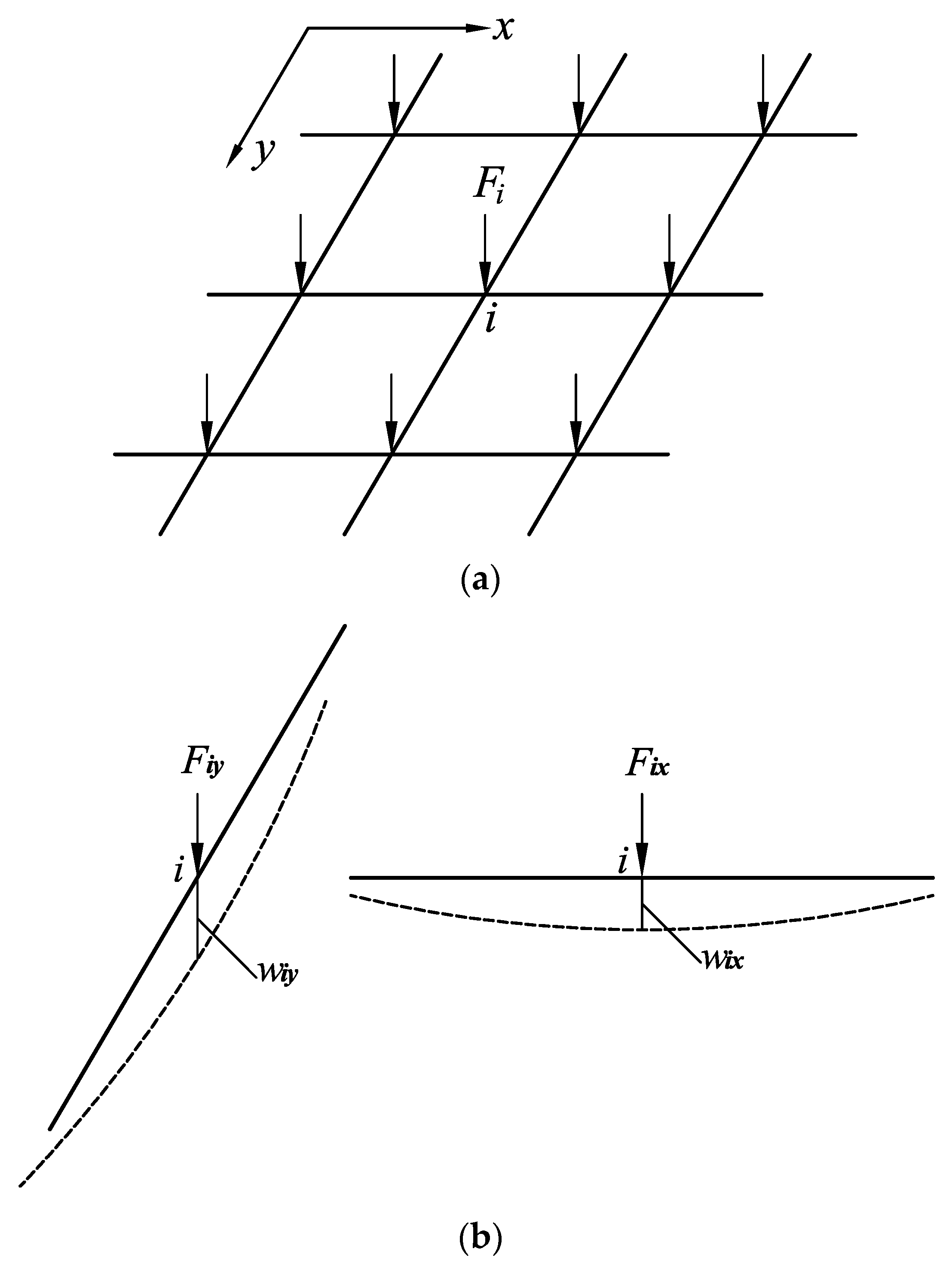
2.1.1. Load Distribution Assumptions
- (a)
- The static equilibrium condition: the sum of the loads assigned to the cross beam and the vertical beam at a node should be equal to the total load acting on the node;
- (b)
- The deformation compatibility condition: the deflection of the cross beam at a node should be equal to that of the vertical beam at the same node.
2.1.2. Theoretical Basis of SLDM
2.1.3. Node Classification and Load Distribution Equations
The Corner Node
The Edge Node
The Inner Node
2.1.4. Evaluation of the SLDM
2.2. Introduction to the NLDM
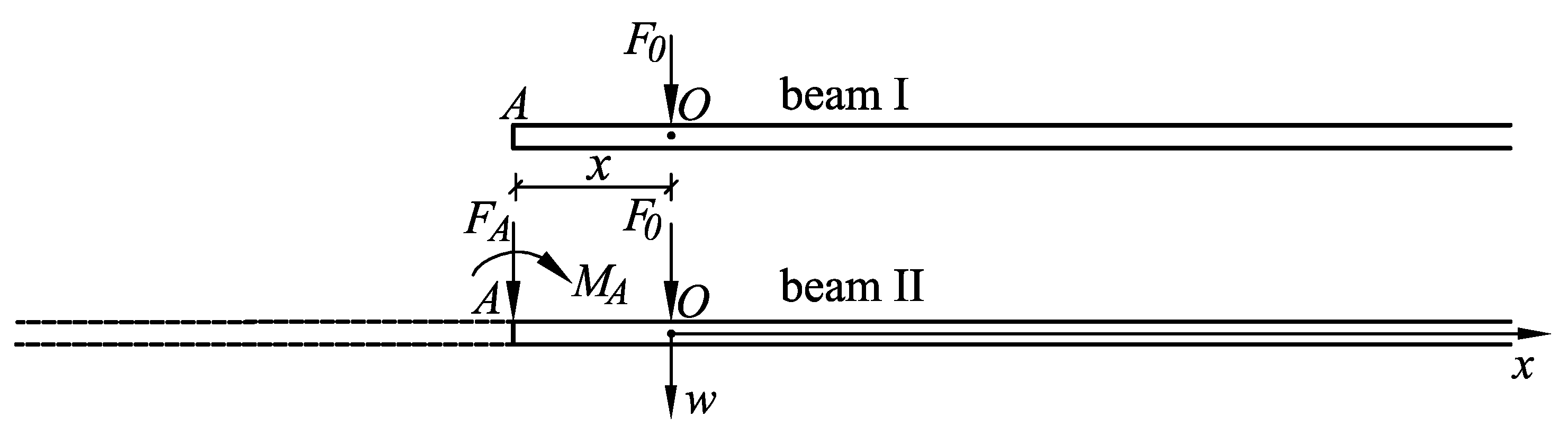
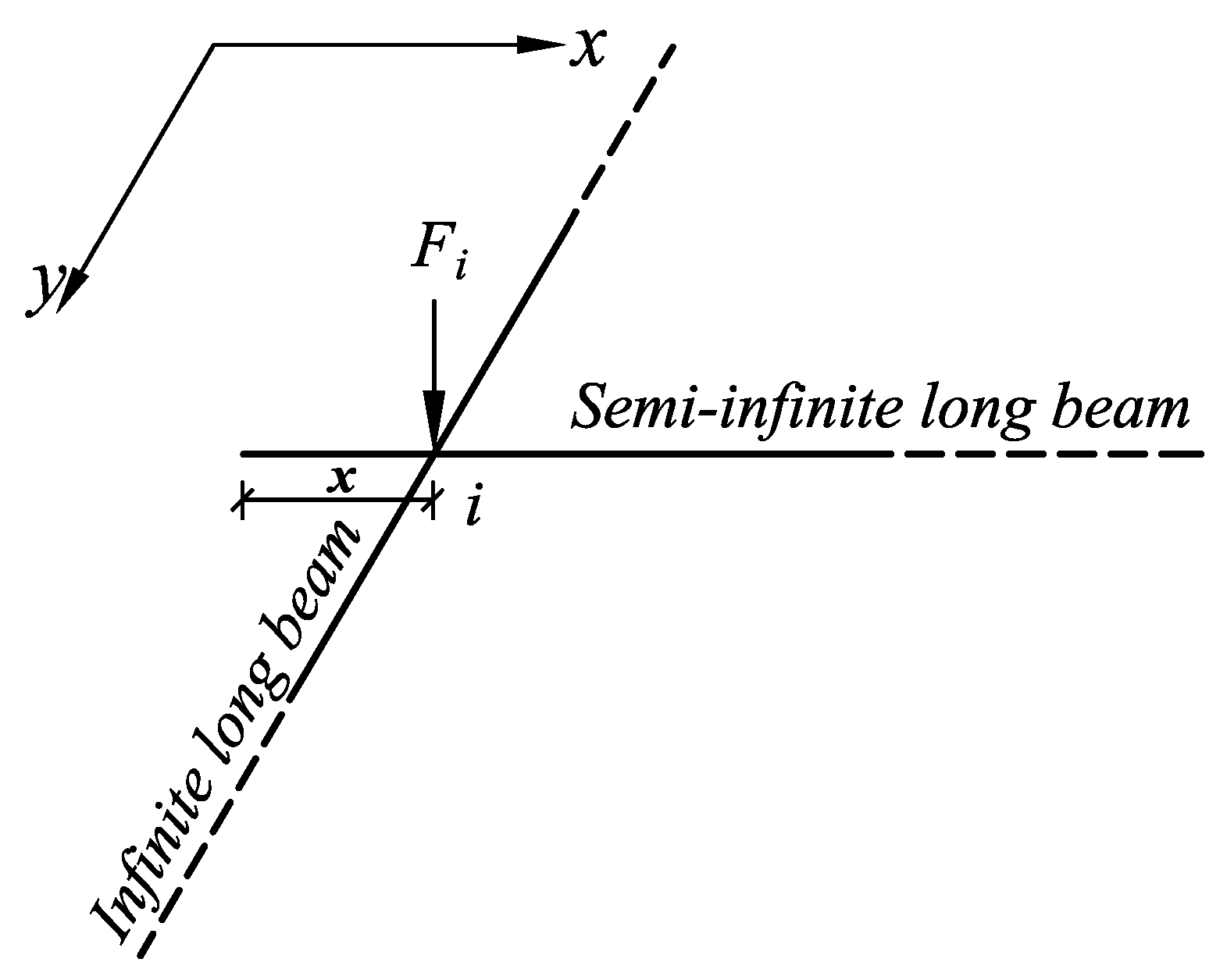
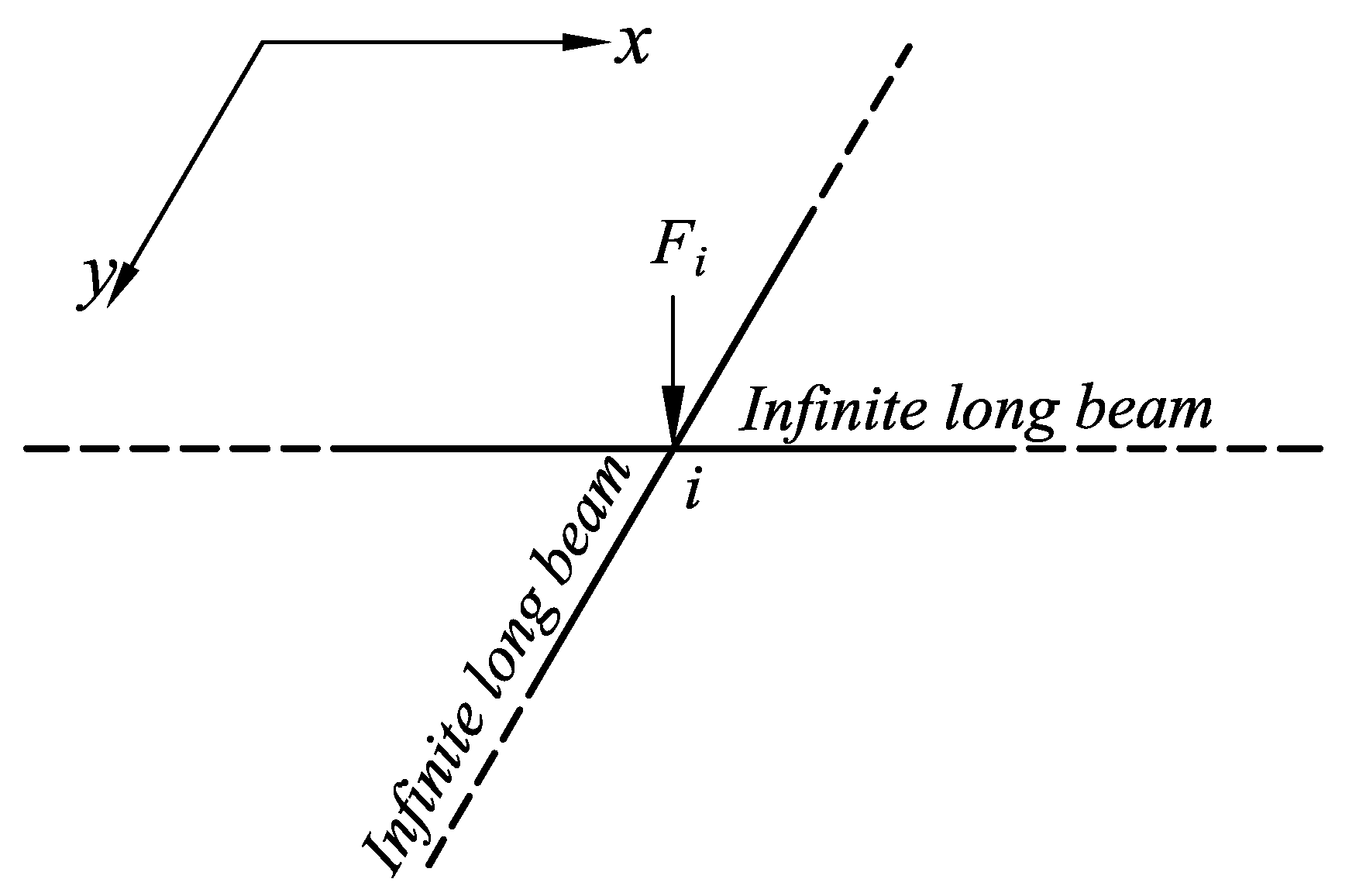
2.2.1. The New Load Distribution Model Based on the Force Method
2.2.2. Analysis of the Deflection at the Nodes and Formation of a System of Linear Equations
3. A Case Study
4. Results and Discussion
4.1. Results under the Design Condition
4.1.1. Load Distributions under the Design Condition
4.1.2. Verification of NLDM
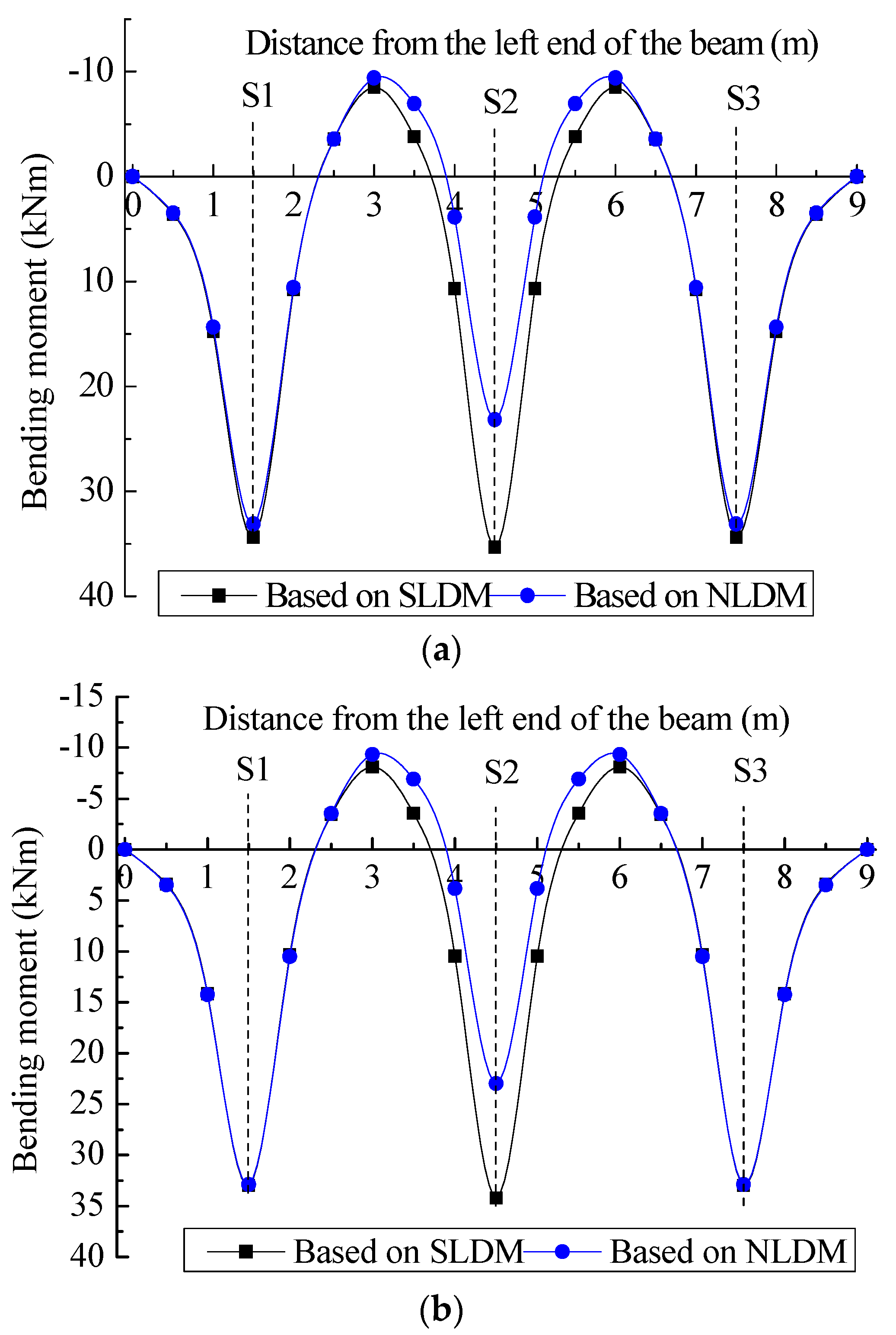
4.1.3. Bending Moment under the Design Condition
4.2. Results under the Actual Working Condition
4.2.1. Load Distributions under the Actual Working Condition
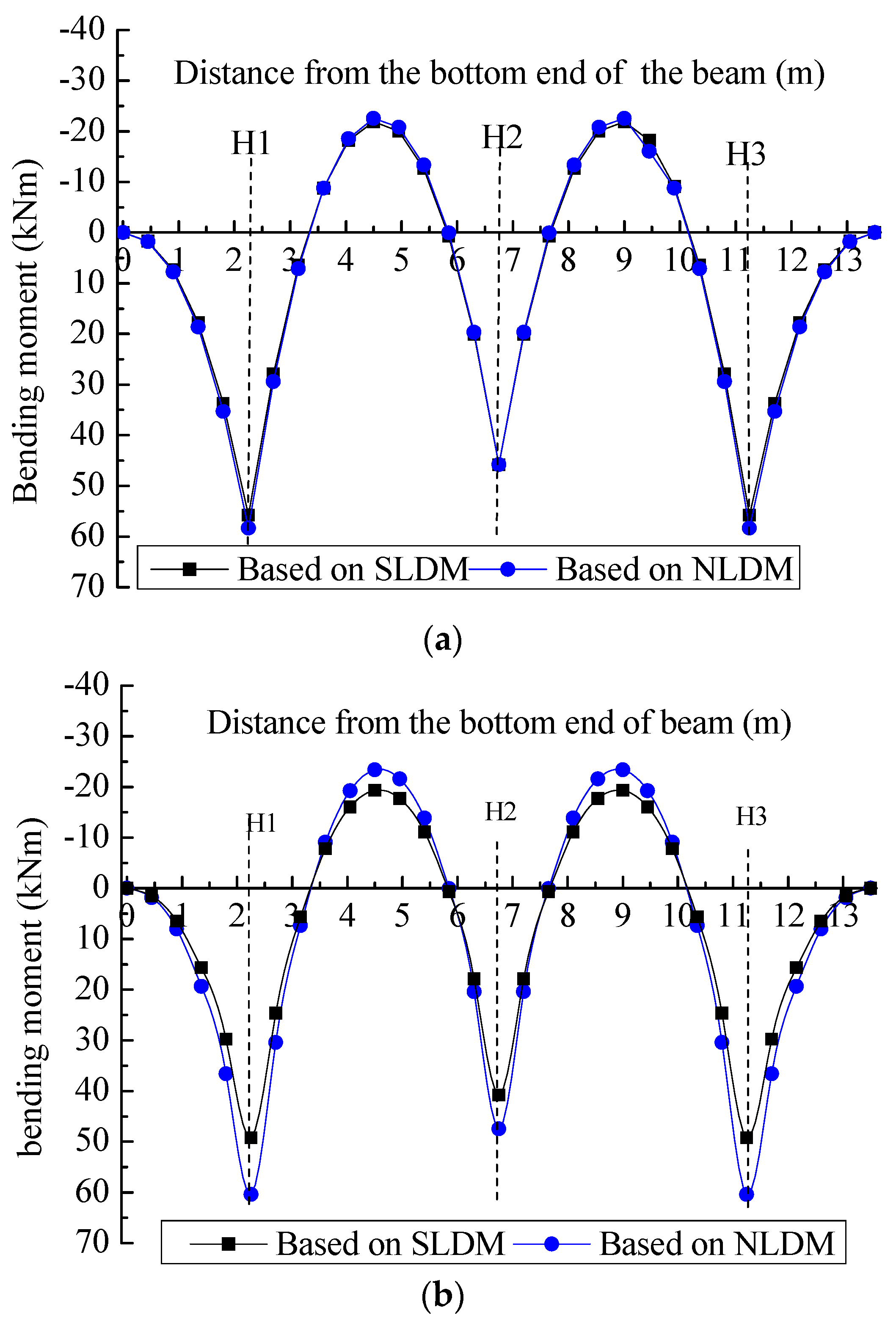
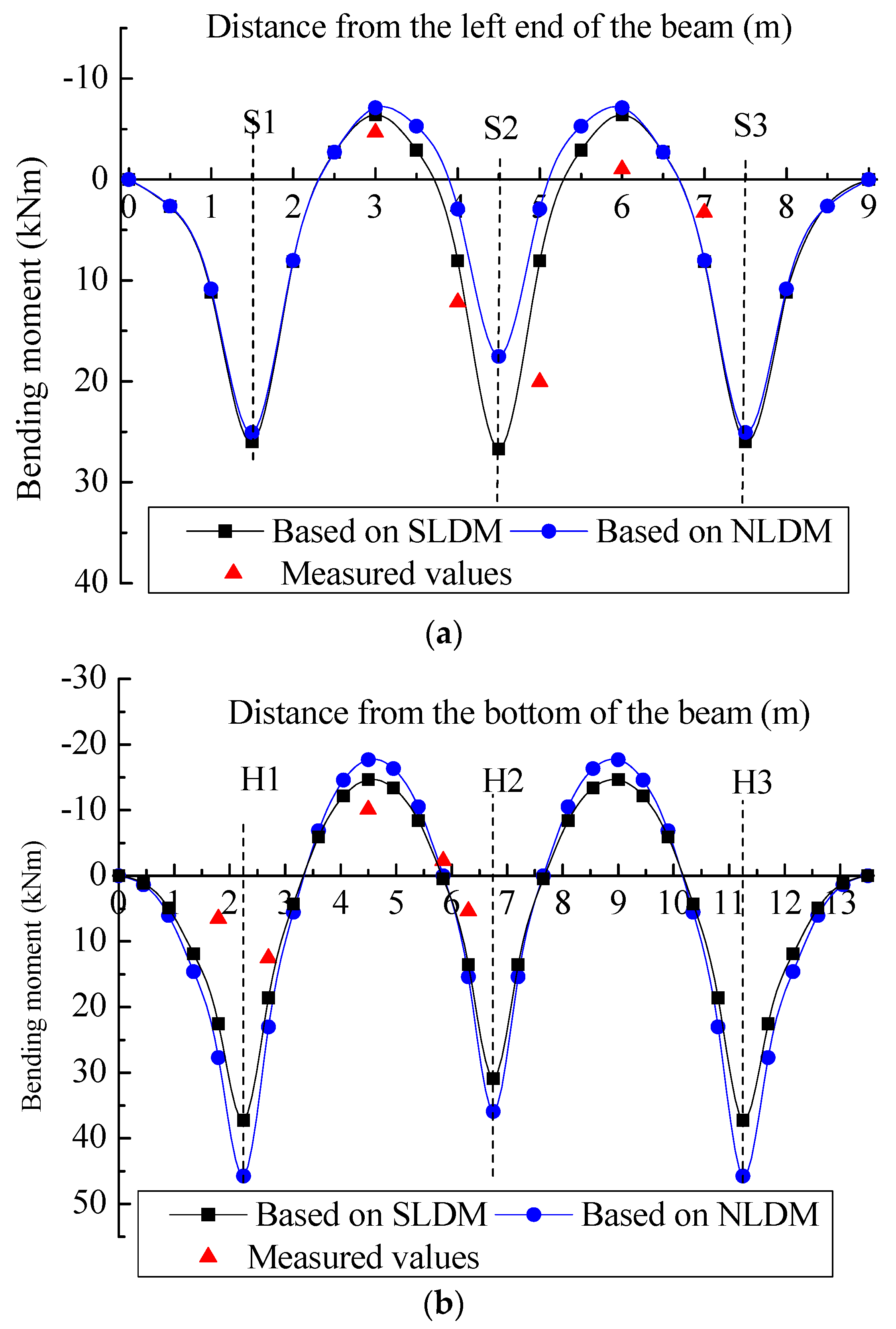
4.2.2. Bending Moment under the Actual Working Condition
5. Conclusion and Recommendations
- (1)
- According to the static equilibrium condition and the deformation compatibility condition at the nodes of the lattice beam, the load distribution results under the design condition show that NLDM can distinguish the differences in stiffness of the beams in both directions relative to the ground, and evenly distribute the larger loads to the vertical beams with the larger spans, but evenly distribute the smaller loads to the cross beams with the smaller spans;
- (2)
- The deflections at the nodes of the lattice beam loaded with the known loads under the design condition are estimated separately, and the results of the deformation analysis show that the deflections of the cross beams and vertical beams are almost the same at the same nodes according to NLDM, thus demonstrating the superiority of the NLDM compared to the SLDM in terms of the deformation compatibility condition;
- (3)
- The results of the theoretical bending moment analysis based on the two load distribution methods show that the bending moments of the cross beam differ significantly in the middle third of the beam length, while the bending moments of the vertical beams differ significantly at the beam sections where the maximum bending moments are located;
- (4)
- Under the actual condition, the theoretical bending moments of the lattice beam based on both load distribution methods agree well with the measured values, but the measured values cannot sufficiently prove the superiority of NLDM due to the inaccuracy of the modulus of subgrade reaction k and the errors during the testing process;
- (5)
- Considering the fact that the deflections at the nodes are sensitive to the modulus of subgrade reaction k, based on NLDM, it is necessary to estimate the modulus of subgrade reaction k reasonably in order to obtain accurate results of the load distribution at the nodes and further accurate internal forces of the lattice beam.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| Ax | the first function of influence |
| Bx | the second function of influence |
| bx | the bottom width of the beam in the x direction |
| by | the bottom width of the beam in the y direction |
| Cx | the third function of influence |
| Dx | the forth function of influence |
| E | elastic modulus of the reinforced concrete |
| Fi | the component of the total anchoring force perpendicular to the slope surface |
| Fix | the load assigned by Fi acting on the node i to the beam running in x direction |
| Fiy | he load assigned by Fi acting on the node i to the beam running in y direction |
| I | moment of inertia of the beam section |
| k | modulus of subgrade reaction |
| Pi | the total anchoring force of the anchor cable acting on the node i of the lattice beam |
| S | characteristic length of the beam on Winkler |
| Sx | characteristic length of the beam in x direction |
| Sy | characteristic length of the beam in y direction |
| wix | the deflection of the beam in x direction at the node i |
| wiy | the deflection of the beam in y direction at the node i |
| Z | comprehensive function of influence of the beam |
| Zx | comprehensive function of influence of the beam in x direction |
| Zy | comprehensive function of influence of the beam in y direction |
| α | the angle of the design slope |
| δij | deflection at the node i caused by unit load at the node j of the beam on Winkler foundaton |
| θ | the dip of the anchor cable to the horizontal plane |
References
- Zhang, H.; Lu, Y.; Cheng, Q. Numerical simulation of reinforcement for rock slope with rock bolt (anchor cable) Frame Beam. J. Highw. Transp. Res. Dev. 2008, 3, 65–71. [Google Scholar] [CrossRef]
- Choi, K.; Cheung, R. Landslide disaster prevention and mitigation through works in Hong Kong. J. Rock Mech. Geotech. Eng. 2013, 5, 354–365. [Google Scholar] [CrossRef] [Green Version]
- Chen, Z.; Wang, Z.; Xi, H.; Yang, Z.; Zou, L.; Zhou, Z.; Zhou, C. Recent advances in high slope reinforcement in China: Case studies. J. Rock Mech. Geotech. Eng. 2016, 8, 775–788. [Google Scholar] [CrossRef]
- Deng, D.; Zhao, L.; Li, L. Limit-Equilibrium Analysis on Stability of a Reinforced Slope with a Grid Beam Anchored by Cables. Int. J. Geomech. 2017, 17, 06017013. [Google Scholar] [CrossRef]
- Shi, K.; Wu, X.; Liu, Z.; Dai, S. Coupled calculation model for anchoring force loss in a slope reinforced by a frame beam and anchor cables. Eng. Geol. 2019, 260, 105245. [Google Scholar] [CrossRef]
- Ye, S.; Fang, G.; Ma, X. Reliability Analysis of Grillage Flexible Slope Supporting Structure with Anchors Considering Fuzzy Transitional Interval and Fuzzy Randomness of Soil Parameters. Arab. J. Sci. Eng. 2019, 44, 8849–8857. [Google Scholar] [CrossRef]
- Zhang, J.J.; Niu, J.Y.; Fu, X.; Cao, L.C.; Yan, S.J. Failure modes of slope stabilized by frame beam with prestressed anchors. Eur. J. Environ. Civ. Eng. 2022, 26, 2120–2142. [Google Scholar] [CrossRef]
- Bao, X.; Liao, W.; Dong, Z.; Wang, S.; Tang, W. Development of Vegetation-Pervious Concrete in Grid Beam System for Soil Slope Protection. Materials 2017, 10, 96. [Google Scholar] [CrossRef]
- Zhu, X.; Chen, Z.; Ren, Y. The Application of Ecological Treatment Technology of Bolt and Lattice Beam in Highway Mountain Slope Support. Geotech. Geol. Eng. 2019, 37, 1891–1896. [Google Scholar] [CrossRef]
- Fan, J.; Yang, S.; Deng, B.; Sun, B.; Liu, T. A New Technique of Lattice Beam Construction with Pre-Anchoring for Strengthening Cut Slope: A Numerical Analysis of Temporary Stability during Excavation. Buildings 2022, 12, 1930. [Google Scholar] [CrossRef]
- Lin, Y.; Lu, L.; Yang, G. Seismic behavior of a single-form lattice anchoring structure and a combined retaining structure supporting soil slope: A comparison. Environ. Earth Sci. 2020, 79, 78. [Google Scholar] [CrossRef]
- Ai, X.; Sheng, M.; Su, X.; Ai, S.; Jiang, X.; Yang, S.; Huang, Z.; Ai, Y. Effects of frame beam on structural characteristics of artificial soil on railway cut-slopes in southwestern China. Land Degrad. Dev. 2021, 32, 482–493. [Google Scholar] [CrossRef]
- Huang, A.; Zhu, Y.; Wang, L.; Ye, S.; Fang, G. Three-Dimensional Stability of Unsaturated Soil Slopes Reinforced by Frame Beam Anchor Plates. Int. J. Geomech. 2023, 23, 04023068. [Google Scholar] [CrossRef]
- Luo, G.; Zhong, Y.; Yang, Y. Failure Mechanism and Mitigation Measures of the G1002 Electricity Pylon Landslide at the Jinping I Hydropower Station. Adv. Civ. Eng. 2020, 2020, 8820315. [Google Scholar] [CrossRef]
- Tao, H.-C.; Zhang, Y.; Dong, J.-X.; Zhou, Z.-Q.; Gong, X.-Y.; Zhang, S.-W. Study on Deformation Mechanism and Control Measures of Tanziyan Landslide. Geofluids 2022, 2022, 8237954. [Google Scholar] [CrossRef]
- Du, C.; Chen, J.; Chen, S.; Peng, M.; Shi, Z. Numerical analysis of a slope stabilized with piles and anchor cable frame beams. Environ. Earth Sci. 2023, 82, 100. [Google Scholar] [CrossRef]
- Lin, Y.-L.; Yang, G.-L.; Yang, X.; Zhao, L.-H.; Shen, Q.; Qiu, M.-M. Response of gravity retaining wall with anchoring frame beam supporting a steep rock slope subjected to earthquake loading. Soil Dyn. Earthq. Eng. 2017, 92, 633–649. [Google Scholar] [CrossRef]
- Lin, Y.-L.; Li, Y.-X.; Yang, G.-L.; Li, Y. Experimental and numerical study on the seismic behavior of anchoring frame beam supporting soil slope on rock mass. Soil Dyn. Earthq. Eng. 2017, 98, 12–23. [Google Scholar] [CrossRef]
- Lei, X.; Wang, B.; Li, N.; Hao, J. Study on Dynamic Response of Pressure Type Anchor and Lattice Beam System Under Seismic Loadings. Geotech. Geol. Eng. 2020, 38, 6057–6067. [Google Scholar] [CrossRef]
- Li, N.; Wang, B.; Yuan, L.; Men, Y.; Li, J.; Liu, X. Seismic response of soil slope reinforced by compression anchor and frame beam based on shaking table test. Arab. J. Geosci. 2020, 13, 261. [Google Scholar] [CrossRef]
- Maleki, M.; Nabizadeh, A. Seismic performance of deep excavation restrained by guardian truss structures system using quasi-static approach. Sn Appl. Sci. 2021, 3, 417. [Google Scholar] [CrossRef]
- Maleki, M.; Mohammad, H. Assessment of the Pseudo-static seismic behavior in the soil nail walls using numerical analysis. Innov. Infrastruct. Solut. 2022, 7, 262. [Google Scholar] [CrossRef]
- Maleki, M.; Khezri, A.; Nosrati, M.; Hosseini, S.M.M.M. Seismic amplification factor and dynamic response of soil-nailed walls. Model. Earth Syst. Environ. 2023, 9, 1181–1198. [Google Scholar] [CrossRef]
- Tang, H.; Xu, Y.; Cheng, X. Research on design theory of lattice frame anchor structure in landslide control engineering. Rock Soil Mech. 2014, 25, 1683–1687. (In Chinese) [Google Scholar] [CrossRef]
- Zhu, D.P.; Xu, Y.Z.; Yan, E.C.; Xiao, W. In-situ test and study of the internal force features of prestress anchor lattice beam. In Boundaries of Rock Mechanics; CRC Press: Boca Raton, FL, USA, 2008; pp. 609–614. [Google Scholar]
- GB/T 38509-2020; Code for the Design of Landslide Stabilization. China Geological Environmental Monitoring Institute, China Standard Press: Beijing, China, 2020. (In Chinese)
- Wu, X. Building Foundation; South China University of Technology Press: Guangzhou, China, 1997. (In Chinese) [Google Scholar]
- Li, Q.; Zhang, G.; Tang, H.; Wang, L. Analysis on internal force of lattice beam and optimization design. Coal Geol. Explor. 2006, 34, 50–53. (In Chinese) [Google Scholar]
- Chen, G.; Wang, J.; Lin, Z. Simplified calculation method of slope frame beam on winkler assumption and its engineering application. J. Geomech. 2016, 24, 822–827. (In Chinese) [Google Scholar] [CrossRef]
- Arthur, A.; Richard, J. Advanced Mechanics of Materials, 6th ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2002. [Google Scholar]
- Hetenyi, M. Beams on Elastic Foundation; University of Michigan Press: Ann Arbor, MI, USA, 1946. [Google Scholar]
- Shen, J. Design Manual of Foundation; Shanghai Science and Technology Press: Shanghai, China, 1988. (In Chinese) [Google Scholar]
- Lin, T. Foundation Design; Huazhong University of Science and Technology: Wuhan, China, 1996. (In Chinese) [Google Scholar]
- Li, J.; Zhu, Y.; Ye, S.; Ma, X. Internal force analysis and field test of lattice beam based on Winkler theory for elastic foundation beam. Math. Probl. Eng. 2019, 2019, 5130654. [Google Scholar] [CrossRef]
- Dinev, D. Analytical solution of beam on elastic foundation by singularity functions. Eng. Mech. 2012, 19, 381–392. [Google Scholar] [CrossRef]




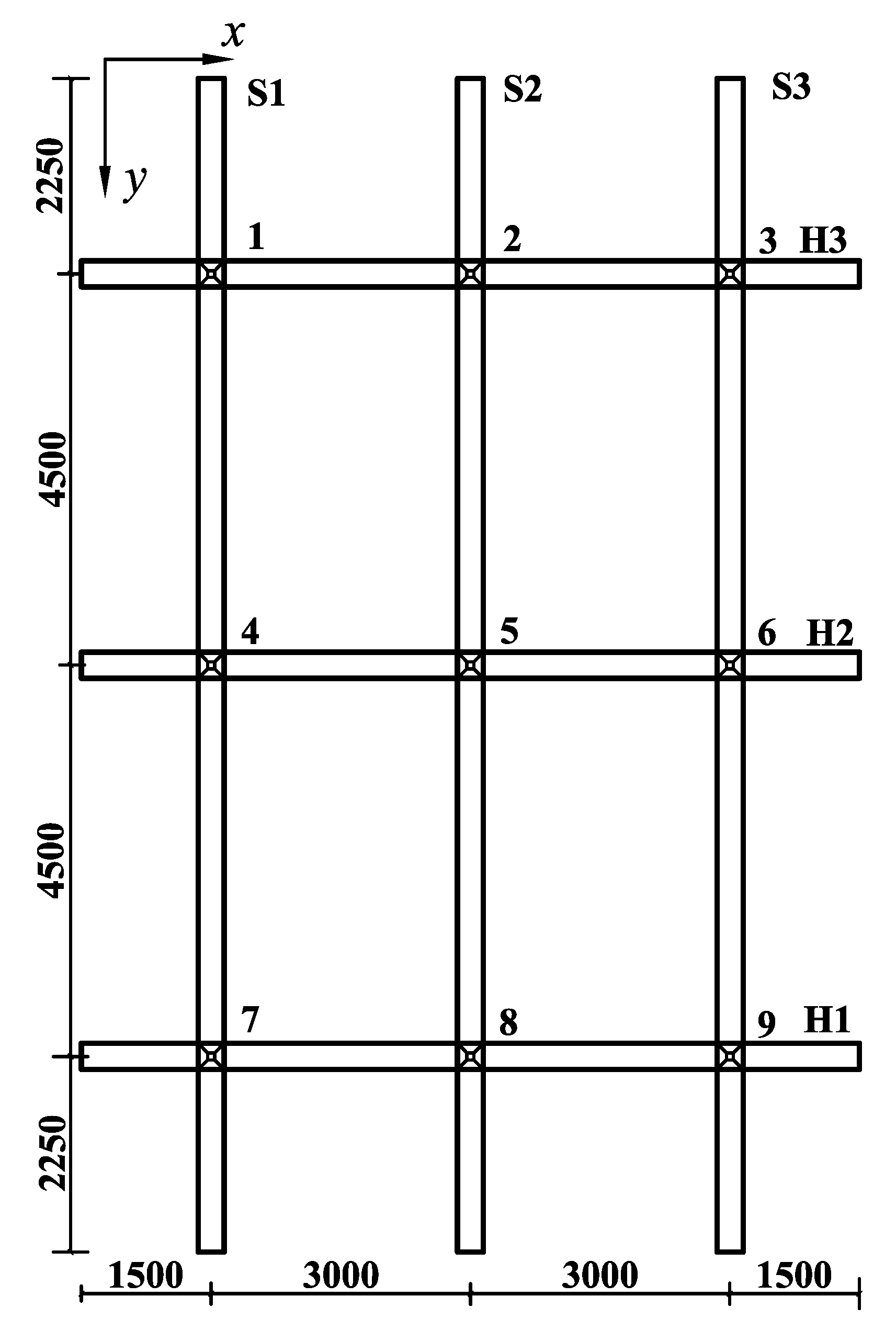

| Parameter Type | Elastic Modulus of Reinforced Concrete E (kPa) | Coefficient of Subgrade Reaction k (kN/m3) |
|---|---|---|
| Values | 2.8 × 107 | 12,000 |
| Node Type | Based on SLDM | Based on NLDM | ||
|---|---|---|---|---|
| Fix (kN) | Fiy (kN) | Fix (kN) | Fiy (kN) | |
| The corner nodes (1, 3, 7, 9) | 104.38 | 125.14 | 99.14 | 130.38 |
| The edge nodes (2, 8) | 119.02 | 110.50 | 94.44 | 135.08 |
| The edge nodes (4, 6) | 100.17 | 129.35 | 98.46 | 131.06 |
| The inner node (5) | 114.76 | 114.76 | 93.76 | 135.76 |
| Node Type | Based on SLDM | Based on NLDM | ||
|---|---|---|---|---|
| wix (m) | wiy (m) | wix (m) | wiy (m) | |
| The corner nodes (1, 3, 7, 9) | 0.0100085 | 0.00886305 | 0.00922644 | 0.00922611 |
| The edge nodes (2, 8) | 0.0114290 | 0.00782735 | 0.00955923 | 0.00955864 |
| The edge nodes (4, 6) | 0.0104004 | 0.00902771 | 0.00916267 | 0.00916265 |
| The inner node (5) | 0.0113063 | 0.00800715 | 0.00949142 | 0.00949134 |
| Node Type | Based on SLDM | Based on NLDM | ||
|---|---|---|---|---|
| Fix (kN) | Fiy (kN) | Fix (kN) | Fiy (kN) | |
| The corner nodes (1, 3, 7, 9) | 78.97 | 94.67 | 75.00 | 98.64 |
| The edge nodes (2, 8) | 90.04 | 83.60 | 71.44 | 102.20 |
| The edge nodes (4, 6) | 75.78 | 97.86 | 74.49 | 99.15 |
| The inner node (5) | 86.82 | 86.82 | 70.93 | 102.71 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fan, J.; Yang, S.; Deng, B.; Sun, B.; Liu, T. A Comparison of Load Distribution Methods at the Node and Internal Force Analysis of the Lattice Beam Based on the Winkler Foundation Model. Buildings 2023, 13, 1731. https://doi.org/10.3390/buildings13071731
Fan J, Yang S, Deng B, Sun B, Liu T. A Comparison of Load Distribution Methods at the Node and Internal Force Analysis of the Lattice Beam Based on the Winkler Foundation Model. Buildings. 2023; 13(7):1731. https://doi.org/10.3390/buildings13071731
Chicago/Turabian StyleFan, Junwei, Shijiao Yang, Bo Deng, Bing Sun, and Taoying Liu. 2023. "A Comparison of Load Distribution Methods at the Node and Internal Force Analysis of the Lattice Beam Based on the Winkler Foundation Model" Buildings 13, no. 7: 1731. https://doi.org/10.3390/buildings13071731





