Influence Law of Axis Deflection on the Mechanical Properties of Steel Stranded Short Slings in Arch Bridges
Abstract
:1. Introduction
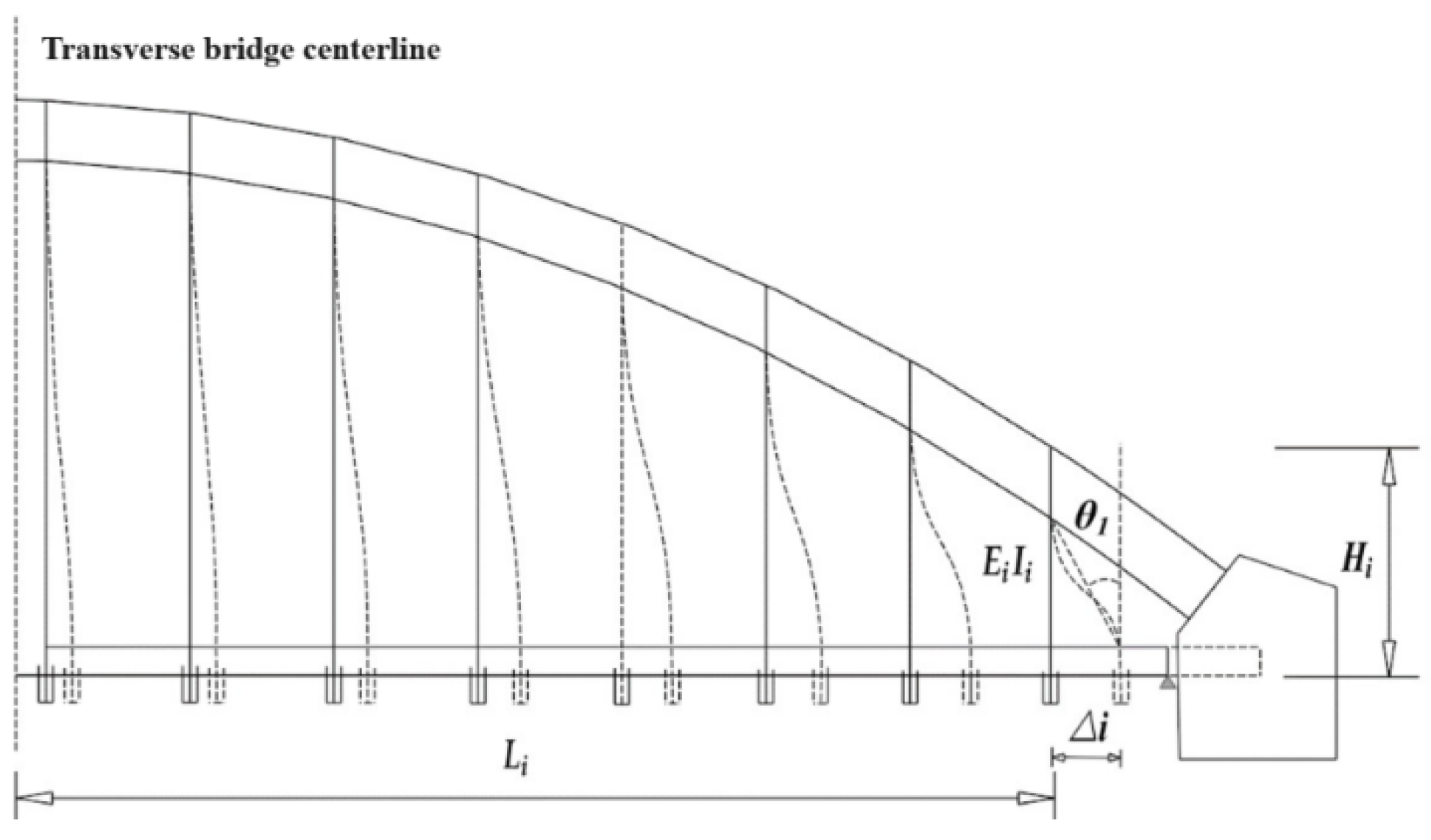
2. Deflection Angle of Engineering Arch Bridge Slings
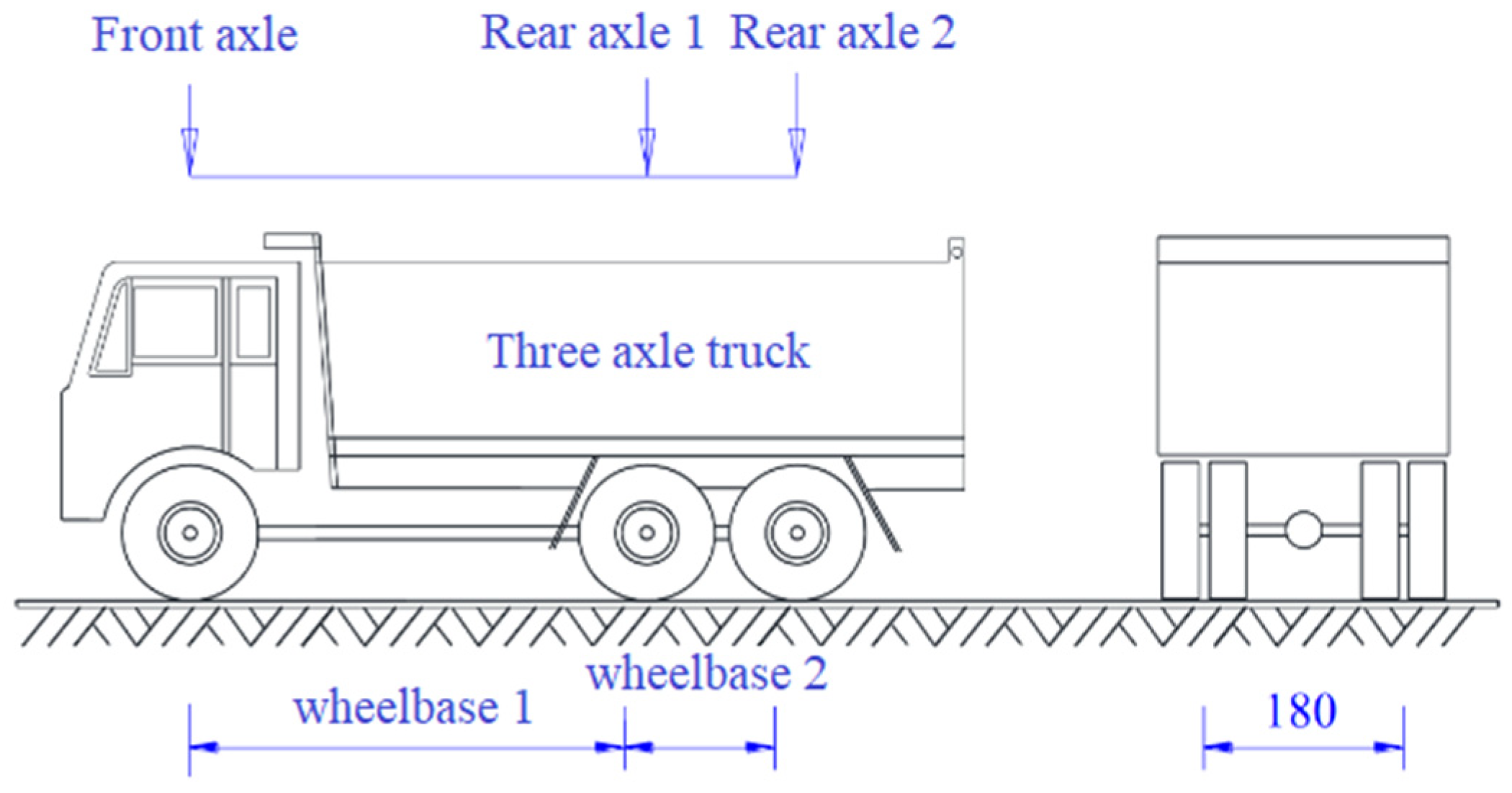
2.1. Distribution Law of the Deflection Angle of Slings under a Full Bridge Load and Deflected Load
2.2. Distribution Law of the Deflection Angle of Slings under Temperature Loading
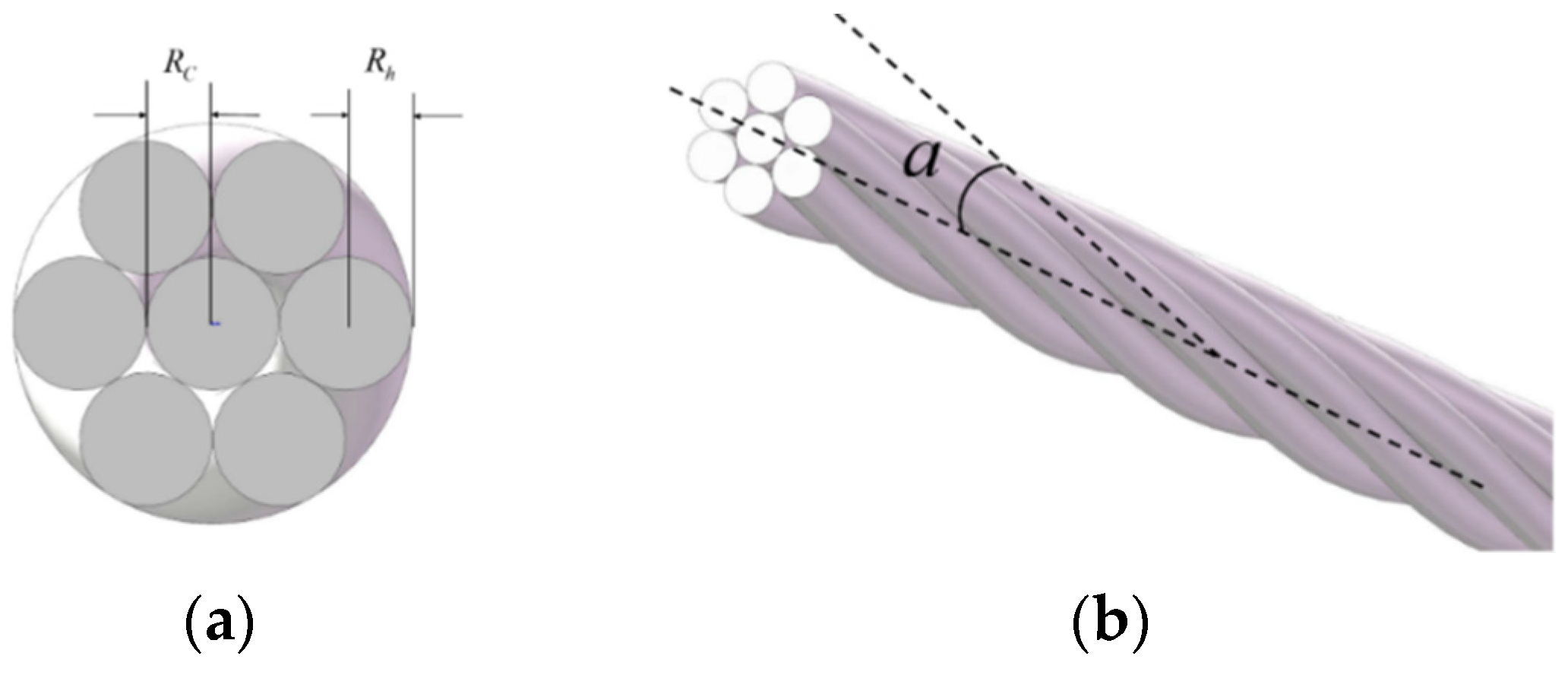
3. Finite Element Analysis of the Static Performance of Steel Stranded Slings with Different Axial Deflection Angles
4. Finite Element Analysis of the Fatigue Performance of Steel Stranded Wire Slings under Different Axial Deflections
5. Conclusions
- (1)
- Whether under full bridge load or deflected load, the distribution patterns of deflection angles of slings are the same, and the angles of deflection are also similar. The deflection angle of the short slings under the deflected load is slightly larger than that under the full bridge load. From the short sling to the mid-span sling, the distribution pattern of deflection angles under the action of different temperatures is almost the same. The deflection angles of the short sling and second shortest sling increase linearly with increasing temperature. The deflection angle of the sling decreases as the length of the sling increases.
- (2)
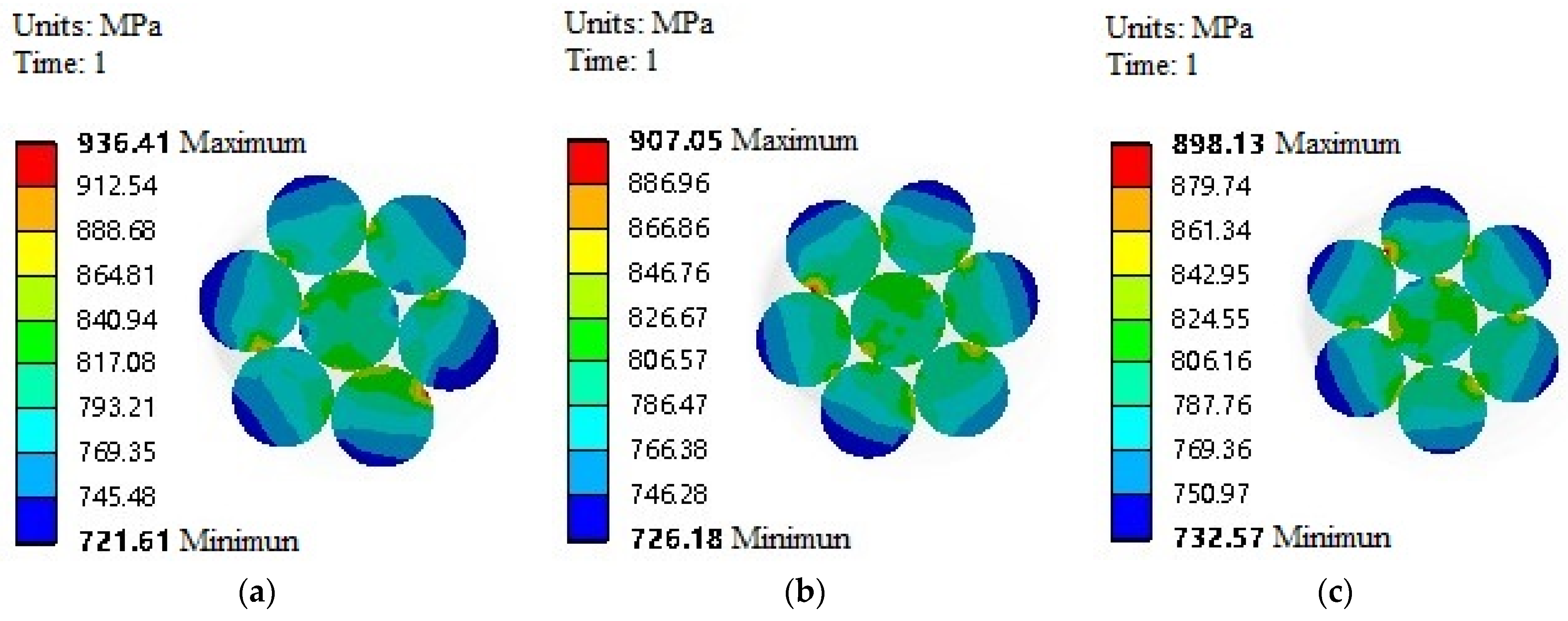
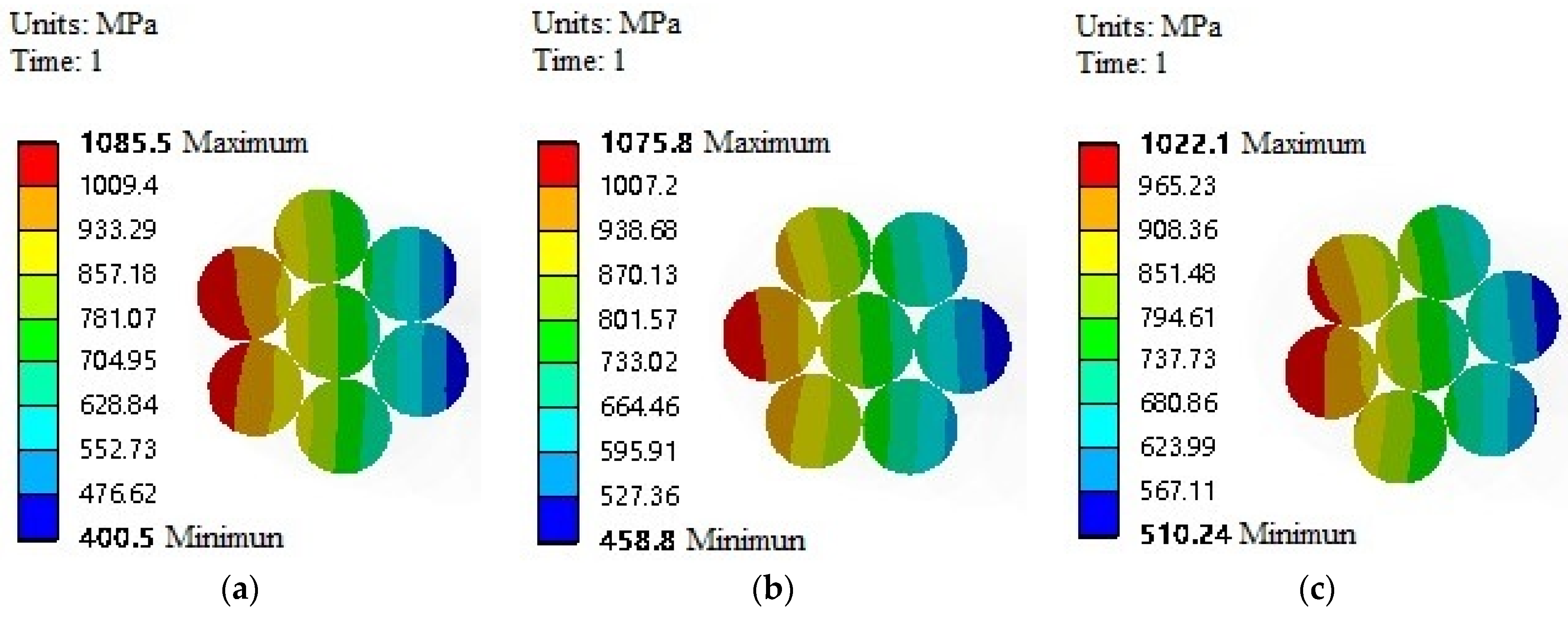
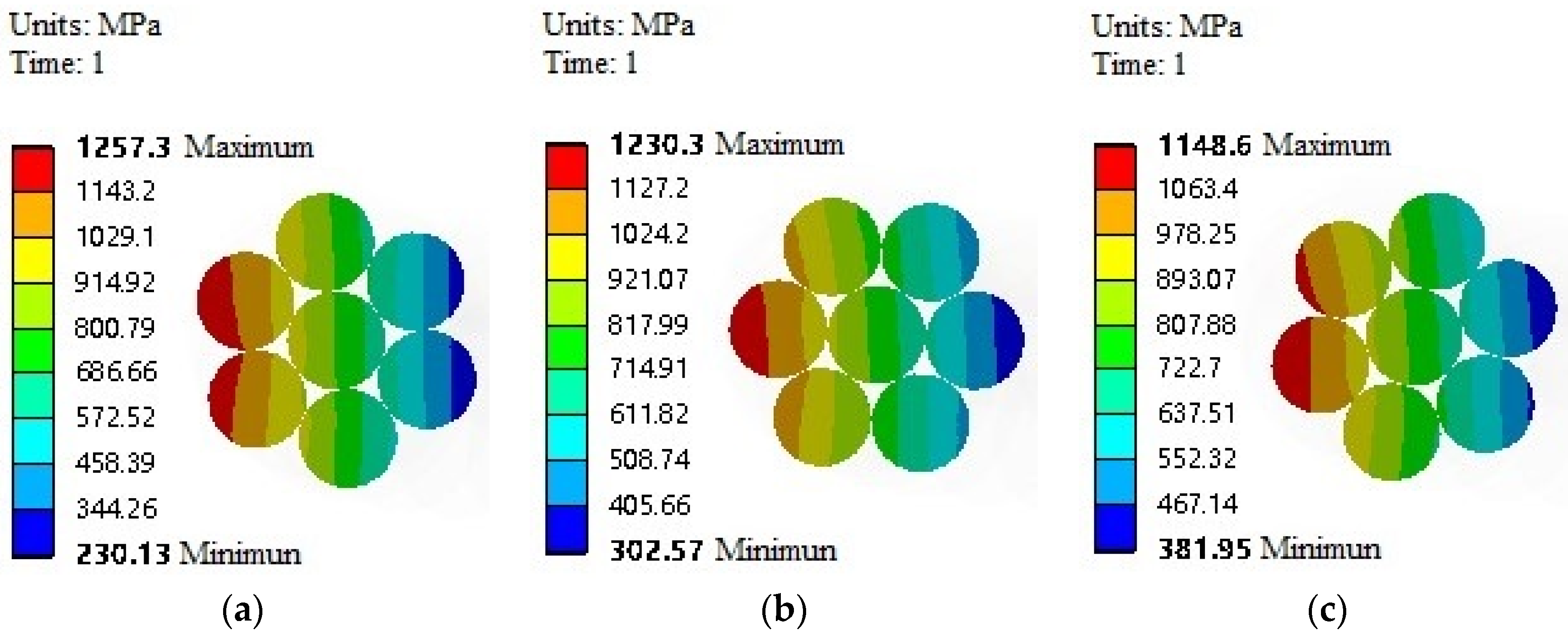
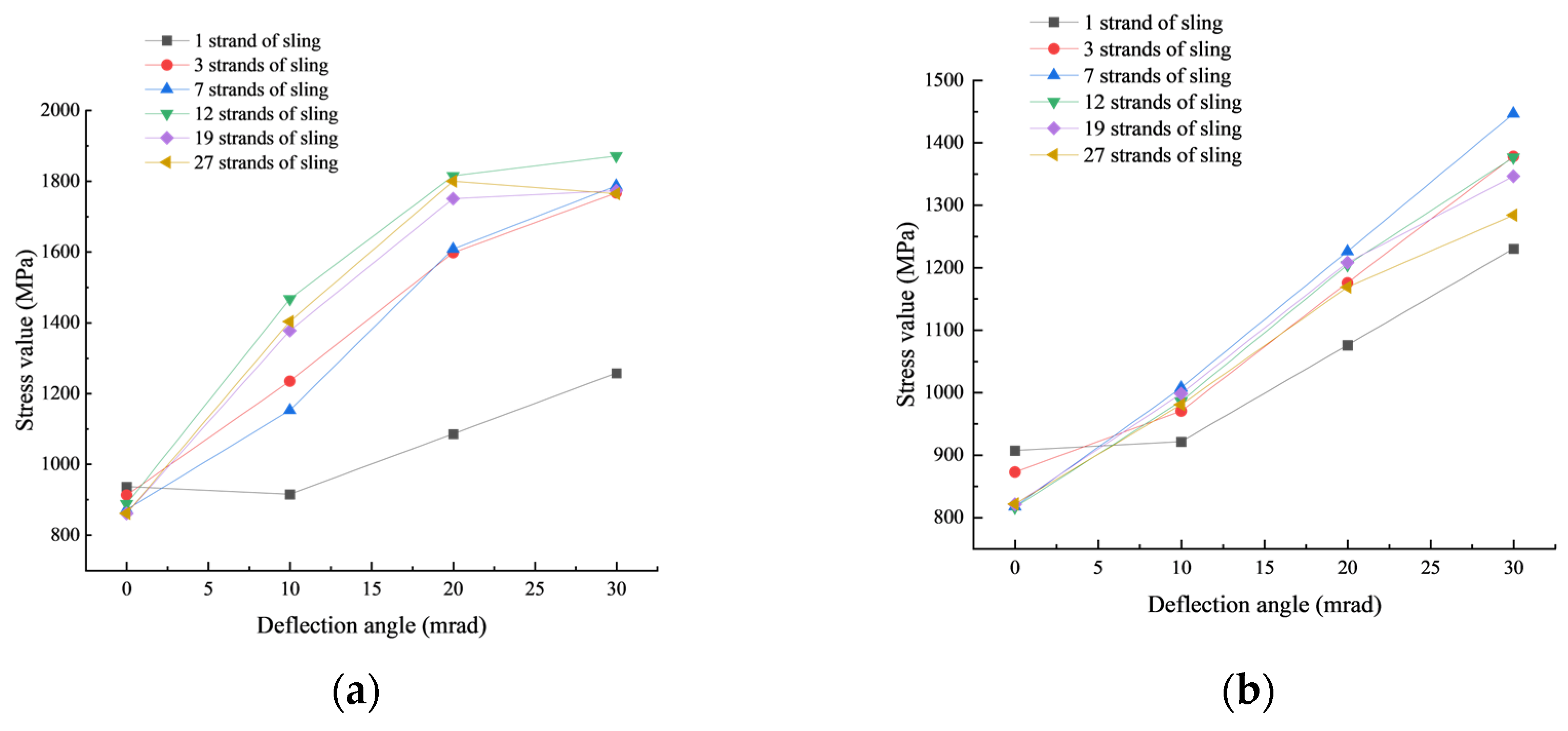
- The performances of 1, 3, 7, 9, 12, 19, and 27 strands of 1860 MPa whole bunch extruded strand slings were simulated and analyzed at different axial deflection angles at force values. At 1 cm from the anchorage end, the maximum stress of the single-strand sling is 936.4 MPa at a deflection angle of 0 mrad, and the maximum stress is 1257.3 MPa at a deflection angle of 30 mrad, which increases by 0.34 fold. The maximum stress increases by 0.93 fold for the 3-strand sling from 0 mrad to 30 mrad, 1.05 fold for the 7-strand sling, 1.10 fold for the 12-strand sling, 1.06 fold for the 19-strand sling, and 1.05 fold for the 27-strand sling. The ultimate tensile properties decreased with increasing shaft deflection angle.
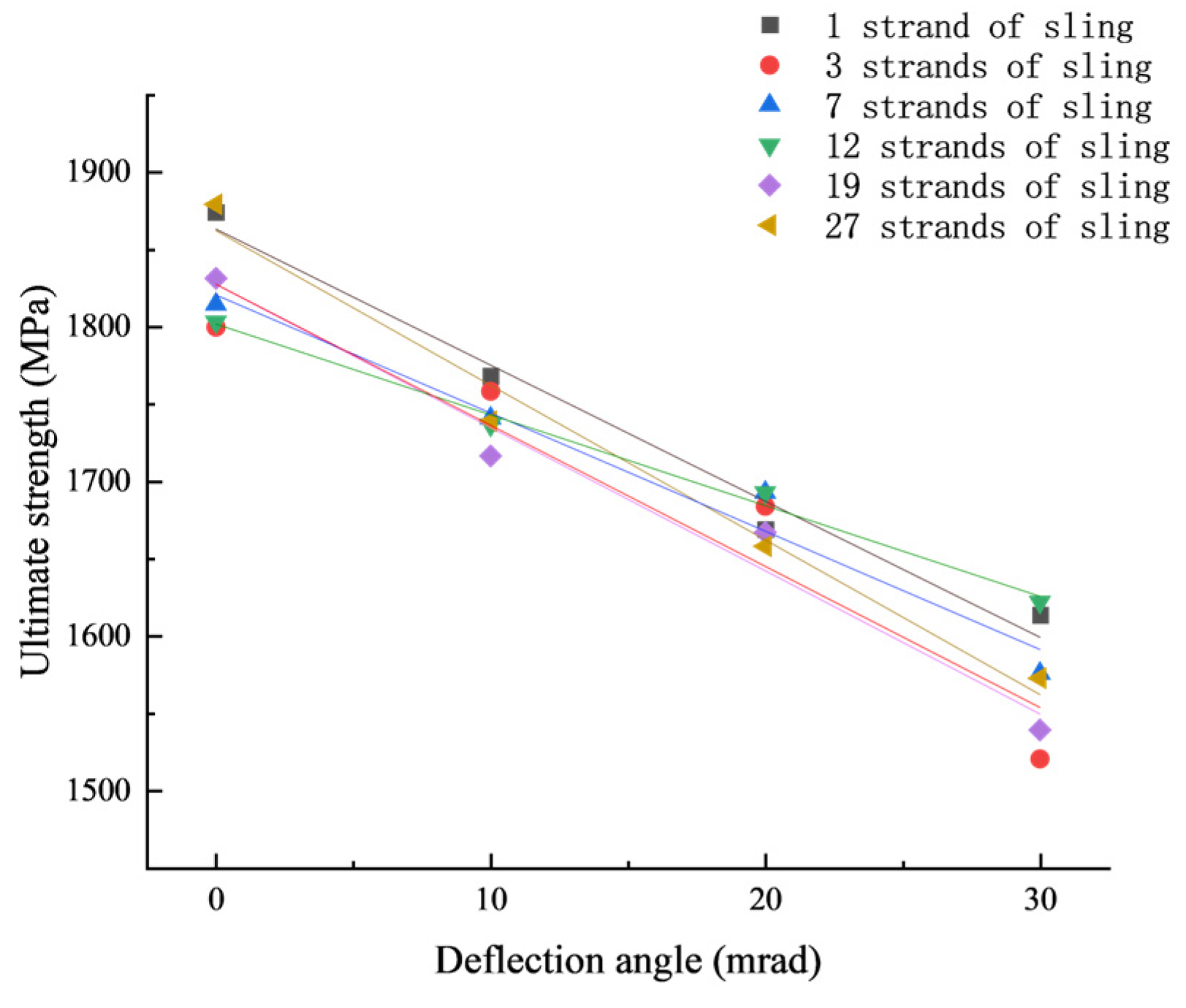
- (3)
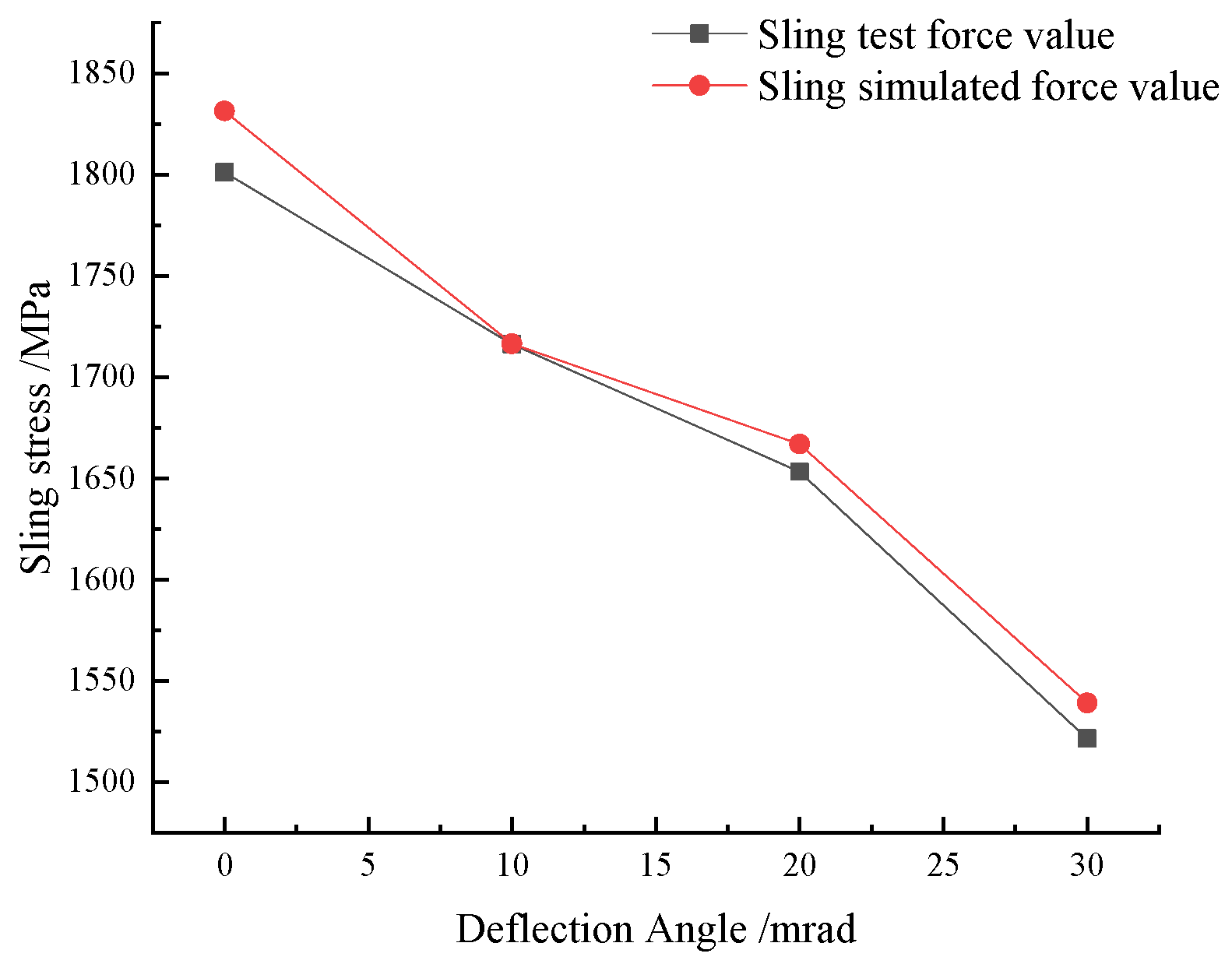
- The breaking strength of the stranded wire sling decreases with increasing shaft deflection angle. At the same axis deflection angle, the broken strength of the sling has a certain degree of decline with an increasing number of strands. The broken strength decreases by 13.8% from 0 mrad to 30 mrad for a single strand of steel stranded sling, 15.5% for the 3-strand sling, 13.1% for the 7-strand sling, 10.0% for the 12-strand sling, 15.9% for the 19-strand sling, and 16.3% for the 27-strand sling. The test results of the ultimate tensile properties of 19 strands of Φ15.2 mm strand slings at different axial deflection agree with the simulation results.
- (4)
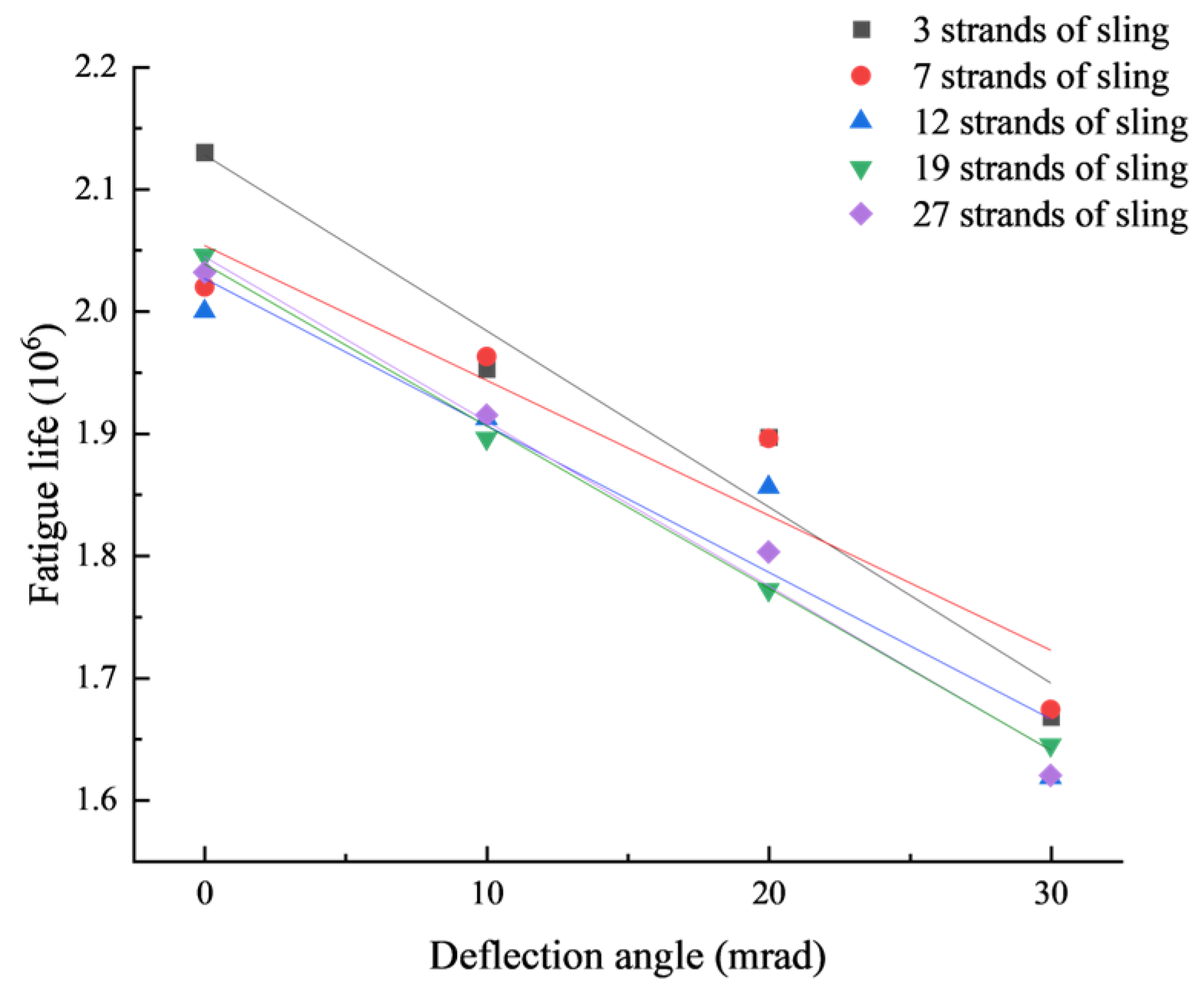
- In the normal course of service, the fatigue life of the stranded wire sling reaches 2 million cycles when there is no shaft deflection, which is in line with national standards. With the increase in the shaft deflection angle, the fatigue life of the steel stranded wire sling has a certain degree of decline. The decline rate of the fatigue life of the sling increases rapidly with increasing shaft deflection angle. From 0 mrad to 30 mrad, the fatigue life of the 3-strand sling decreases by 27.8%, while that of the 7-strand sling decreases by 20.6%, 12-strand sling decreases by 23.6%, 19-strand sling decreases by 24.3%, and 27-strand sling decreases by 25.4%.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chen, B.C.; Liu, J.P. Review of construction and technology development of arch bridges in the world. J. Traffic Transp. Eng. 2020, 20, 27–41. [Google Scholar]
- Liu, J.P.; Chen, B.C.; Li, C.; Zhang, M.J.; Tabatabai, H. Recent Application of and Research on Concrete Arch Bridges in China. Struct. Eng. Int. 2023, 33, 394–398. [Google Scholar] [CrossRef]
- Zhang, X.C.; Xie, K.; Ren, Y.Y. Analying the reason and renovating project of “little south door Jinsha River bridge of Yibin City” collapsing. J. Henan Univ. Urban Constr. 2002, 11, 15–17. [Google Scholar]
- Chen, X.H. Wuyishan Mansion Bridge collapsed life rescue. J. Saf. Health 2011, 39, 9. [Google Scholar]
- Gu, A.B.; Xu, J.L. Structural analysis of short slings of half through or through arch bridge. J. Chongqing Jiaotong Univ. Nat. Sci. 2002, 21, 1–3. [Google Scholar]
- Vermass, G. Corrosion and Embrittlement of High-Strength Steel Bridge Wires; Columbia University: New York, NY, USA, 2001. [Google Scholar]
- Nakamura, S.; Suzumura, K.; Tarui, T. Mechanical properties and remaining strength of corroded bridge wires. Struct. Eng. Int. 2004, 14, 50–54. [Google Scholar] [CrossRef]
- Yang, J.X.; Chen, W.Z.; Gu, R. Analysis of Dynamic Characteristics of Short Hangers of Arch Bridge. Bridge Constr. 2014, 44, 13–18. [Google Scholar]
- Gimsing, N.J. Cable Supported Bridges; China Communication Press: Beijing, China, 2002. [Google Scholar]
- Lu, J.G. Research on the Damage of Hangers of Concrete-Filled Steel Tubular Arch Bridges; Tianjin University: Tianjin, China, 2005. [Google Scholar]
- Liu, E.D.; Liao, G.M. The Arch Bridge Sling Breaking Analysis. North Commun. 2013, 11, 61–64. [Google Scholar]
- Yu, Y.J.; Chen, Z.H.; Liu, H.B.; Wang, X.D. Finite element study of behavior and interface force conditions of seven-wire strand under axial and lateral loading. Constr. Build. Mater. 2014, 66, 10–18. [Google Scholar] [CrossRef]
- Erena, D.; Valeo, J.; Navarro, C.; Domínguez, J. Fatigue and fracture analysis of a seven-wire stainless steel strand under axial and bending loads. Fatigue Fract. Eng. Mater. Struct. 2020, 43, 149–161. [Google Scholar] [CrossRef]
- Zhou, Y.M.; Deng, N.C.; Yang, T. A Study on the Strength and Fatigue Properties of Seven-Wire Strands in Hangers under Lateral Bending. Appl. Sci. 2020, 10, 2160. [Google Scholar] [CrossRef]
- Zhu, G.C.; Deng, N.C.; Xu, J.; Xie, Q.Q. Effect of Deflection Angle on Mechanical Properties and Pit Stress of Strands with Different Crorrosion Degree. Joural Shijiazhuang Tiedao Univ. (Nat. Sci. Ed.) 2022, 35, 14–20. [Google Scholar]
- Jiang, J.W.; Deng, N.C.; Guo, X.; Liu, L.H.; Li, C.S. Influence of short slings’ bending deflection on mechanical properties of arch bridges. China Sci. 2022, 17, 167–173. [Google Scholar]
- Deng, N.C.; Xu, J.; Zhu, G.C.; Han, Z.Q. Study on the Mechanical Properties of Corroded Steel Strands at Deflection Angles. Buildings 2023, 13, 795. [Google Scholar] [CrossRef]
- Gang, S.; John, M.; Harry, C. Bending Fatigue Life Evaluation of Bridge Stay Cables. J. Eng. Mech. 2022, 148, 04021168. [Google Scholar]
- Li, C.C.; Liu, K.; Shen, Y. Common diseases and treatment measures of pulling sling. J. Highw. Transp. Res. Dev. 2013, 9, 4. [Google Scholar]
- García-Guerrero, J.M.; Jorquera-Lucerga, J.J. Effect of stiff hangers on the longitudinal structural behavior of tied-arch bridges. Appl. Sci. 2018, 8, 258. [Google Scholar] [CrossRef]
- JTG/T J21-01; People’s Republic of China Industry Recommended standards: Load Test Methods for Highway Bridge. Ministry of Transport: Beijing, China, 2015.
- Ma, J.; Ge, S.R.; Zhang, D.K. Distribution of wire deformation within strands of wire ropes. J. China Univ. Min. Technol. 2008, 18, 475–478. [Google Scholar] [CrossRef]
- Chen, M.C.; Fang, W.; Yang, C.; Xie, L. Bayesian prediction and probabilistic model of fatigue cracks in steel structures. Eng. Fail. Anal. 2019, 103, 335–346. [Google Scholar] [CrossRef]
- Qian, H.M.; Zong, Z.H.; Xie, G.Y.; Liao, Y.C.; Du, M.L. Refined simulation of elastic-plastic static behavior of grade 1860 strand wires. J. Southeast Univ. (Nat. Sci. Ed.) 2019, 49, 624–630. [Google Scholar]
- Wu, S.B. Fatigue Safety and Design Study of Through and Half-through Arch Bridges’ Sling; Chongqing University: Chongqing, China, 2008. [Google Scholar]
- Kong, Q.K. Structural Behavior Study on Short Slings of Half through Arch Bridges with Large Span; Southwest Jiaotong University: Chengdu, China, 2003. [Google Scholar]
- Nakamura, S.; Suzumura, K. Experimental study on fatigue strength of corroded bridge wires. J. Bridge Eng. 2013, 18, 200–209. [Google Scholar] [CrossRef]
- Zhao, D.; Liu, S.; Xu, Q.; Shi, F.; Sun, W.; Chai, L. Fatigue life prediction of wire rope based on stress field intensity method. Eng. Fail. Anal. 2017, 81, 1–9. [Google Scholar] [CrossRef]
- Gaur, V.; Enoki, M.; Okada, T.; Yomogida, S. A study on fatigue behavior of MIG-welded Al-Mg alloy with different filler-wire materials under mean stress. Int. J. Fatigue 2018, 107, 119–129. [Google Scholar] [CrossRef]
- Li, X.Z.; Xie, X.; Pan, X.Y.; Sun, W.; Zhu, H. Experimental study on fatigue performance of corroded high tensile steel wires of arch bridge hangers. China Civ. Eng. J. 2015, 48, 68–76. [Google Scholar]
- Ma, L. Study on fatigue properties of domestic 1860 grade low-relaxation prestressed steel strand. Railw. Stand. Des. 2000, 20, 21–23. [Google Scholar]











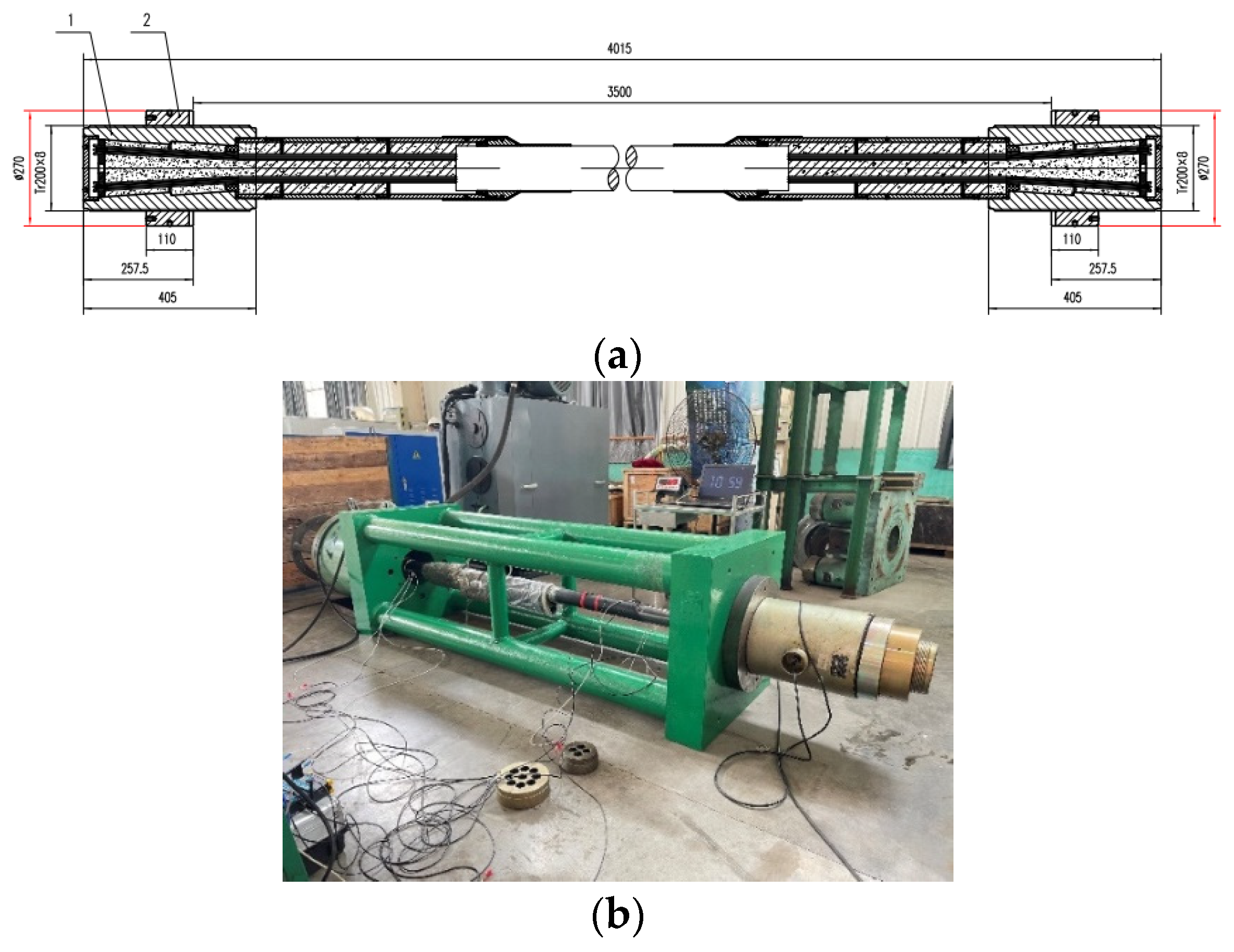



| Item | Tensile Strength/MPa | Compressive Strength/MPa | Yield Strength/MPa | Poisson’s Ratio | Modulus of Elasticity /MPa |
|---|---|---|---|---|---|
| Steel strand | 1860 | - | - | 0.15 | 1.95 × 105 |
| Q420qD steel | 540 | - | 420 | 0.31 | 2.06 × 105 |
| C70 concrete | 2.99 | 44.5 | - | 0.23 | 3.70 × 104 |
| C40 concrete | 2.39 | 26.8 | - | 0.20 | 3.25 × 104 |
| Temperature/°C | Simulated Values/mrad | Theoretical Calculated Value/mrad | ||
|---|---|---|---|---|
| No. 1 Sling | No. 2 Sling | No. 1 Sling | No. 2 Sling | |
| 5 | 2.5 | 0.6 | 2.7 | 0.7 |
| 10 | 5.0 | 1.2 | 5.4 | 1.4 |
| 15 | 7.5 | 1.9 | 8.1 | 2.1 |
| 20 | 10.0 | 2.5 | 10.9 | 2.8 |
| 25 | 12.5 | 3.1 | 13.6 | 3.5 |
| 30 | 15.0 | 3.7 | 16.3 | 4.3 |
| 35 | 17.5 | 4.4 | 19.1 | 4.9 |
| 40 | 20.0 | 5.0 | 21.8 | 5.7 |
| 45 | 22.5 | 5.6 | 24.5 | 6.4 |
| Name of Sling | Deflection Angle 0 mrad/MPa | Deflection Angle 10 mrad/MPa | Deflection Angle 20 mrad/MPa | Deflection Angle 30 mrad/MPa |
|---|---|---|---|---|
| 1 strand of sling 1 cm from anchorage end | 936.4 | 914.8 | 1085.5 | 1257.3 |
| 1 strand of sling 4 cm from anchorage end | 907.0 | 921.2 | 1075.8 | 1230.3 |
| 1 strand of sling 7 cm from anchorage end | 898.1 | 895.6 | 1022.1 | 1148.6 |
| 3 strands of sling 1 cm from anchorage end | 912.8 | 1234.6 | 1597.6 | 1767.2 |
| 3 strands of sling 4 cm from anchorage end | 872.4 | 970.1 | 1175.7 | 1378.3 |
| 7 strands of sling 1 cm from anchorage end | 871.4 | 1152.0 | 1608.3 | 1786.9 |
| 7 strands of sling 4 cm from anchorage end | 817.1 | 1007.5 | 1255.9 | 1446.8 |
| 12 strands of sling 1 cm from anchorage end | 887.7 | 1467.0 | 1814.8 | 1871.8 |
| 12 strands of sling 4 cm from anchorage end | 816.1 | 985.9 | 1204.3 | 1377.0 |
| 19 strands of sling 1 cm from anchorage end | 860.4 | 1377.3 | 1751.00 | 1773.4 |
| 19 strands of sling 4 cm from anchorage end | 820.5 | 998.0 | 1207.9 | 1346.3 |
| 27 strands of sling 1 cm from anchorage end | 860.9 | 1403.3 | 1799.9 | 1764.9 |
| 27 strands of sling 4 cm from anchorage end | 821.0 | 980.4 | 1168.8 | 1284.0 |
| Number of Strands | Breaking Strength at Different Shaft Deflection Angles (MPa) | |||
|---|---|---|---|---|
| 0 mrad | 10 mrad | 20 mrad | 30 mrad | |
| 1 | 1874.0 | 1768.1 | 1669.1 | 1613.6 |
| 3 | 1799.9 | 1758.4 | 1683.9 | 1520.5 |
| 7 | 1814.6 | 1740.8 | 1692.8 | 1575.7 |
| 12 | 1803.3 | 1736.6 | 1692.9 | 1622 |
| 19 | 1831.4 | 1716.5 | 1667.0 | 1539.1 |
| 27 | 1879.3 | 1738.8 | 1658.1 | 1572.7 |
| Shaft Deflection Angle/mrad | Test Force Value/kN | Test Stress Value/MPa | Simulated Value of Force/kN | Simulated Stress Value/MPa |
|---|---|---|---|---|
| 0 | 4791.8 | 1801.2 | 4871.5 | 1831.4 |
| 10 | 4565.3 | 1716.3 | 4565.8 | 1716.5 |
| 20 | 4397.7 | 1653.3 | 4434.2 | 1667.0 |
| 30 | 4047.1 | 1521.5 | 4094.0 | 1539.1 |
| Material | Modulus of Elasticity/GPa | Poisson’s Ratio | Tensile Strength/MPa | Elongation Rate |
|---|---|---|---|---|
| 1860 grade strand | 195 | 0.3 | 1860 | ≥4% |
| Number of Strands | Fatigue Life at Different Shaft Deflection Angles/106 | |||
|---|---|---|---|---|
| 0 mrad | 10 mrad | 20 mrad | 30 mrad | |
| 3 | 2.130 | 1.953 | 1.897 | 1.668 |
| 7 | 2.020 | 1.963 | 1.896 | 1.674 |
| 12 | 2.000 | 1.912 | 1.856 | 1.618 |
| 19 | 2.046 | 1.896 | 1.772 | 1.645 |
| 27 | 2.032 | 1.915 | 1.803 | 1.620 |
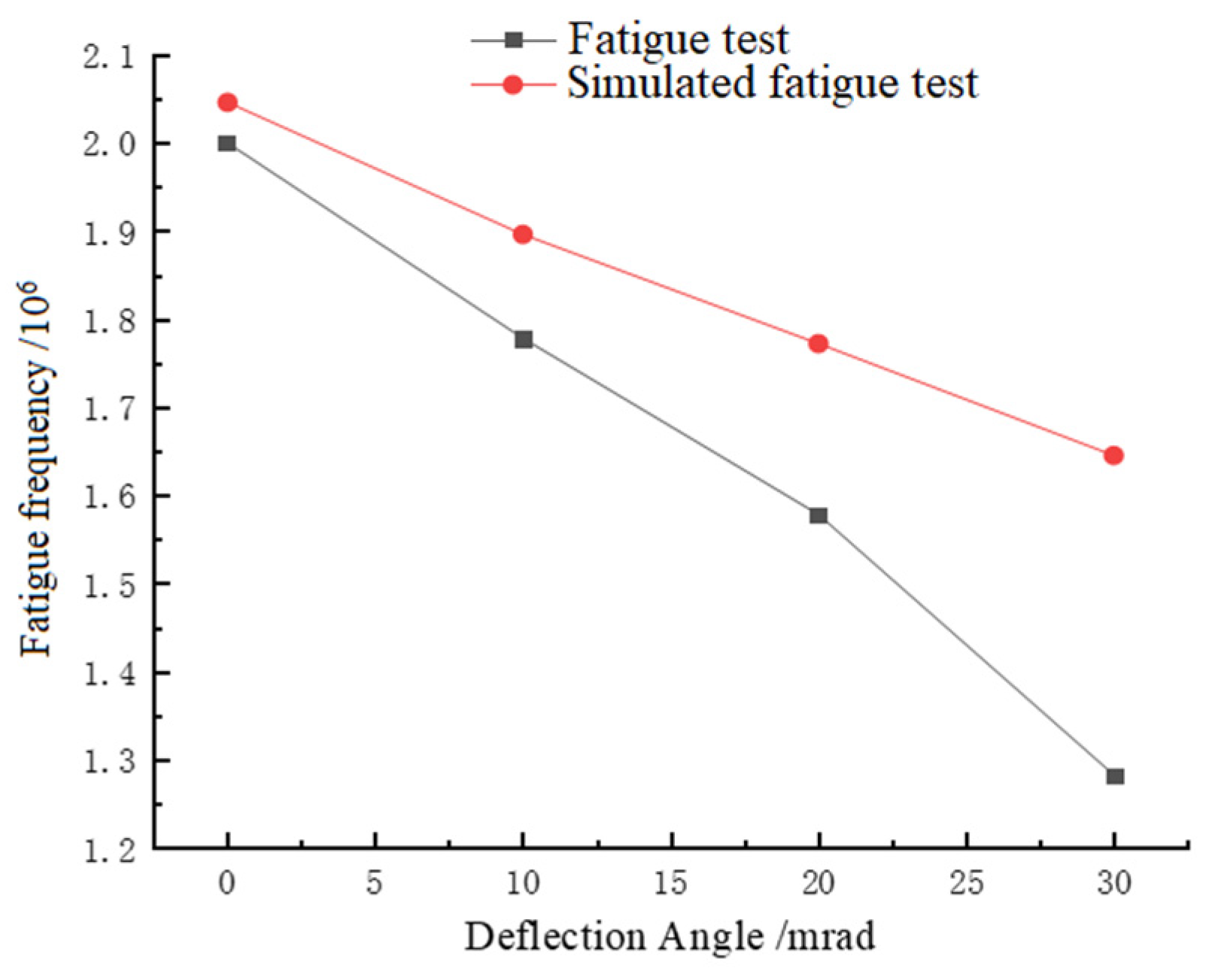
| - | Stress Amplitude/MPa | Upper Stress Limit/MPa | Fatigue Life at Different Axial Deflection Angles/106 | |||
|---|---|---|---|---|---|---|
| 0 mrad | 10 mrad | 20 mrad | 30 mrad | |||
| Test fatigue value | 200 | 837 | 2.000 | 1.778 | 1.578 | 1.282 |
| Simulated fatigue value | 200 | 837 | 2.046 | 1.896 | 1.772 | 1.645 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Deng, N.; Liang, H.; Zhu, G.; Xu, J.; Tang, Z. Influence Law of Axis Deflection on the Mechanical Properties of Steel Stranded Short Slings in Arch Bridges. Buildings 2024, 14, 223. https://doi.org/10.3390/buildings14010223
Deng N, Liang H, Zhu G, Xu J, Tang Z. Influence Law of Axis Deflection on the Mechanical Properties of Steel Stranded Short Slings in Arch Bridges. Buildings. 2024; 14(1):223. https://doi.org/10.3390/buildings14010223
Chicago/Turabian StyleDeng, Nianchun, Hongjie Liang, Guochao Zhu, Jie Xu, and Zhiyu Tang. 2024. "Influence Law of Axis Deflection on the Mechanical Properties of Steel Stranded Short Slings in Arch Bridges" Buildings 14, no. 1: 223. https://doi.org/10.3390/buildings14010223
APA StyleDeng, N., Liang, H., Zhu, G., Xu, J., & Tang, Z. (2024). Influence Law of Axis Deflection on the Mechanical Properties of Steel Stranded Short Slings in Arch Bridges. Buildings, 14(1), 223. https://doi.org/10.3390/buildings14010223






