A Theoretical and Numerical Approach to Ensure Ductile Failure in Strengthened Reinforced Concrete Slabs with Fiber-Reinforced Polymer Sheets

Abstract

While fiber-reinforced polymer (FRP) sheets effectively enhance the flexural strength of reinforced concrete (RC) slabs, excessive flexural strengthening can reduce ductility and lead to brittle failure. This study provides an overview of the failure limits for the end spans of continuous RC slabs, considering the relationship between moment and shear capacities. A design approach for maximizing the strength contribution and amount of carbon FRP (CFRP) while ensuring ductile failure in strengthened slabs was developed and refined based on ACI standard recommendations. The failure mode of the strengthened slab was validated through numerical analysis using Abaqus software by further investigating the stress distribution of flexural members. Analytical results indicated that a 0.15 mm thick CFRP layer could enhance the nominal failure load by 148% while preserving desirable ductile failure behavior, demonstrating the effectiveness and feasibility of the proposed approach.

1. Introduction

The need for strengthening and rehabilitating infrastructure is increasingly critical due to factors such as aging, corrosion, and unexpected loads [1,2,3]. Furthermore, addressing design or construction deficiencies and enhancing the load-bearing capacity of existing reinforced concrete (RC) structures are crucial to ensure their continued serviceability [4,5]. Reconstruction and rehabilitation are the primary options for improving structural resistance [6]. Strengthening existing infrastructure is a more sustainable approach, offering reduced costs and minimizing environmental impact compared to demolition and reconstruction [7,8,9].

Various techniques have been developed for rehabilitating existing RC structures, which can be categorized into material replacement and external strengthening methods [10]. Material replacement involves casting or replacing a part of the structure with high-strength materials such as high-performance concrete, fiber-reinforced concrete, or nanofiber cement [11,12,13]. On the other hand, external strengthening includes techniques such as concrete or steel jacketing [14,15], fiber-reinforced polymer (FRP) wrapping [16,17], and near-surface mounted reinforcement [18,19,20], all aimed at improving the flexure, shear, and durability of RC structures. Among these, FRP materials (in the forms of pultruded sections, grids, sheets, and rebars), including basalt FRP (BFRP), glass FRP (GFRP), and carbon FRP (CFRP), have gained significant attention for flexural strengthening [21]. These materials offer several advantages, including high tensile strength, lightweight properties, exceptional durability in harsh environments, and remarkable versatility [22,23,24,25,26,27]. While CFRP is widely used thanks to its superior strength, GFRP and BFRP provide cost-effective alternatives with slightly lower mechanical properties [28,29]. Nevertheless, a critical factor in FRP-strengthened RC structures is their increased susceptibility to brittle failure [30,31], contrasted with the more ductile behavior of steel-reinforced concrete. This brittleness stems from the bond characteristics at the FRP–concrete interface and the linear-elastic, brittle tensile properties of FRP composites [32,33,34,35].

Additionally, an excessive increase in flexural strength relative to shear strength can compromise the ductility of the member. Ensuring structural ductility is crucial for forewarning potential failures and preventing sudden collapse [36,37,38]. Previous research has established design limits to prevent brittle shear failure and induce ductile behavior in strengthened continuous RC slabs. Nguyen et al. [39] defined these failure limits for end spans, while Kim et al. [40] focused on interior spans. A design approach to prevent brittle failure in strengthened slabs using retrofit systems was also proposed [41]. However, these theoretical analyses are based on linear-elastic assumptions, while reinforced concrete exhibits nonlinear behavior. To accurately assess the behavior of the strengthened slab and validate the design approach, the further investigation of stress and strain distributions in flexural members, along with the nonlinear response of constitutive materials, is essential. Finite element method (FEM) analysis [42,43] offers a suitable and cost-effective approach to capture this nonlinear behavior and improve the reliability and feasibility of the proposed design solution. FEM analysis using Abaqus software (v2024) [44] is a powerful tool for predicting the behavior of strengthened RC structures, providing the accurate simulation of nonlinear stress–strain response, stress distribution, and the interaction of the constituent materials (concrete, steel, and FRP). Its proven reliability in structural engineering research makes it ideal for evaluating performance and validating results. Additionally, a case study for the end-span is designed with configuration and material properties similar to the FEM model for verification and comparison purposes. This integrated approach, combining numerical analysis with theoretical analysis based on ACI 440.2R guidelines [45], improves the accuracy of failure mode evaluation and maximizes the load-carrying capacity of strengthened RC structures while ensuring ductile failure behavior.

This study provides an overview of the failure limits for the end spans of continuous RC slabs based on their moment and shear capacities. It evaluates the failure modes of strengthened slabs using a combination of theoretical and numerical analyses. A case study is presented to demonstrate the effectiveness of the proposed approach in optimizing the strengthening efficiency of slabs with FRP sheets, following ACI 440.2R recommendations.

2. Theoretical Overview

2.1. Overview of Failure Limits

Continuous concrete structures must be designed to resist the maximum factored loads determined through elastic analysis. This study investigates the failure limits of the end span of a continuous slab under a uniform load—the flexural rigidities of members and columns control bending moment distribution. The moment at the support and mid-span is obtained by $\mathrm{M}=\mathrm{C}\mathrm{w}{l}^2,$ where C is a coefficient based on rigidities, and l is the span length. For members with uniformly distributed loads and more than two spans, where the longer span does not exceed 1.2 times the shorter span, the ACI 318 committee [46] recommends specific load patterns to identify critical shears and moments, as shown in Figure 1. The moments and shears for flexural members subjected to uniformly distributed loads can be estimated based on the applied load, clear span length, and approximate coefficients, as outlined below.

$${\mathrm{M}}_{\mathrm{u}}={\mathrm{C}}_{\mathrm{m}}\left({\mathrm{w}}_{\mathrm{u}}{l}_{\mathrm{n}}^2\right)$$

(1)

$${\mathrm{V}}_{\mathrm{u}}={\mathrm{C}}_{\mathrm{v}}\left({\displaystyle \frac{{\mathrm{w}}_{\mathrm{u}}{l}_{\mathrm{n}}}{2}}\right)$$

(2)

Nguyen et al. [39] defined failure limits based on moment and shear capacities for the end spans of frames to ensure ductile failure, applying the principles of elastic analysis, as illustrated in Figure 2.

Table 1. Classification of failure modes for end spans based on plastic hinge formation sequence.

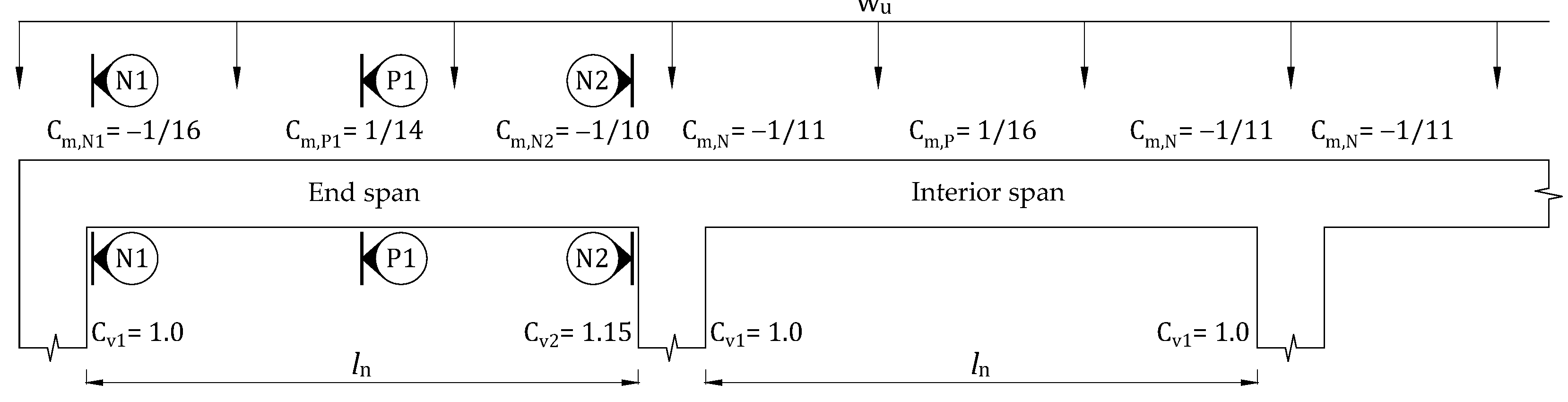
Failure Region	1st Plastic Hinge	2nd Plastic Hinge	3rd Plastic Hinge	Shear Failure	Failure Mode
D-1	N2	N1	P1	-	Ductile
D-2	N2	P1	N1	-	Ductile
D-3	P1	N2	N1	-	Ductile
DB-1	N2	N1	-	N2	Brittle
DB-2	N2	P1	-	N2	Brittle
DB-3a	P1	-	-	N2	Brittle
DB-3b	P1	N2	-	N2	Brittle
B-1	N2	-	-	N2	Brittle
B-2	-	-	-	N2	Brittle

Notes: P1: mid-span section; N1: the interior face of the exterior support; and N2: the exterior face of the first interior support, as shown in Figure 1.

summarizes different failure modes based on the sequence of plastic hinge formation for the end spans of continuous RC slabs. If plastic hinges form at all critical sections, including supports and mid-span, the slab’s failure mode can be classified as a ductile failure. Conversely, the failure mode is considered brittle. Failure modes D-1, D-2, and D-3 represent desirable ductile failures, while failure modes DB-1, DB-2, DB-3a, DB-3b, B-1, and B-2 are undesirable brittle failures. The nominal failure load of the strengthened RC slab can be determined using the superposition method considering plastic redistribution and calculated using the expressions provided in

Table 2. Calculating nominal failure loads of continuous RC slabs.

Failure Region	Nominal Failure Load
D-1	${\mathrm{w}}_{\mathrm{fn}}={\displaystyle \frac{8}{l_{\mathrm{n}}^2}}\left({\mathrm{M}}_{\mathrm{n},\mathrm{P}}+{\mathrm{M}}_{\mathrm{n},\mathrm{N}}{\displaystyle \frac{\left(1/8-{\mathrm{C}}_{\mathrm{m},\mathrm{P}1}\right)}{{\mathrm{C}}_{\mathrm{m},\mathrm{N}2}}}\right)$	(12)
D-2	${\mathrm{w}}_{\mathrm{fn}}={\displaystyle \frac{4}{l_{\mathrm{n}}^2}}\left({\mathrm{M}}_{\mathrm{n},\mathrm{P}}+{\mathrm{M}}_{\mathrm{n},\mathrm{N}}{\displaystyle \frac{\left(1/4+{\mathrm{C}}_{\mathrm{m},\mathrm{N}2}-{\mathrm{C}}_{\mathrm{m},\mathrm{N}1}-{\mathrm{C}}_{\mathrm{m},\mathrm{P}1}\right)}{{\mathrm{C}}_{\mathrm{m},\mathrm{N}2}}}\right)$	(13)
D-3	${\mathrm{w}}_{\mathrm{fn}}={\displaystyle \frac{4}{l_{\mathrm{n}}^2}}\left({\mathrm{M}}_{\mathrm{n},\mathrm{P}}{\displaystyle \frac{\left(1/4-{\mathrm{C}}_{\mathrm{m},\mathrm{N}1}\right)}{{\mathrm{C}}_{\mathrm{m},\mathrm{P}1}}}+{\mathrm{M}}_{\mathrm{n},\mathrm{N}}\right)$	(14)
Other regions	${\mathrm{w}}_{\mathrm{fn}}={\displaystyle \frac{2{\mathrm{V}}_{\mathrm{n}}}{{\mathrm{C}}_{\mathrm{v}2}{l}_{\mathrm{n}}}}$	(15)

. The approach simplifies the challenge of preventing sudden failure in strengthened continuous structures reinforced with externally bonded FRP materials. The failure limits for each region of the end span can be derived from the following expressions:

$${\mathrm{M}}_{\mathrm{n},\mathrm{N}}={\displaystyle \frac{{2\mathrm{C}}_{\mathrm{m},\mathrm{N}2}}{{\mathrm{C}}_{\mathrm{v}2}}}{\mathrm{V}}_{\mathrm{n}}{l}_{\mathrm{n}}$$

(3)

$${\mathrm{M}}_{\mathrm{n},\mathrm{P}}={\displaystyle \frac{{2\mathrm{C}}_{\mathrm{m},\mathrm{P}1}}{{\mathrm{C}}_{\mathrm{v}2}}}{\mathrm{V}}_{\mathrm{n}}{l}_{\mathrm{n}}$$

(4)

$${\mathrm{M}}_{\mathrm{N}1}={\displaystyle \frac{{2\mathrm{C}}_{\mathrm{m},\mathrm{N}1}}{{\mathrm{C}}_{\mathrm{v}2}}}{\mathrm{V}}_{\mathrm{n}}{l}_{\mathrm{n}}$$

(5)

$${\displaystyle \frac{{\mathrm{M}}_{\mathrm{N}1}}{{\mathrm{M}}_{\mathrm{n},\mathrm{P}}}}={\displaystyle \frac{{\mathrm{C}}_{\mathrm{m},\mathrm{N}1}}{{\mathrm{C}}_{\mathrm{m},\mathrm{P}1}}}$$

(6)

$${\displaystyle \frac{{\mathrm{M}}_{\mathrm{n},\mathrm{N}}}{{\mathrm{M}}_{\mathrm{n},\mathrm{P}}}}={\displaystyle \frac{{\mathrm{C}}_{\mathrm{m},\mathrm{N}2}}{{\mathrm{C}}_{\mathrm{m},\mathrm{P}1}}}$$

(7)

$${\mathrm{M}}_{\mathrm{n},\mathrm{P}}+{\mathrm{M}}_{\mathrm{n},\mathrm{N}}\left({\displaystyle \frac{{\mathrm{C}}_{\mathrm{v}2}/8+{\mathrm{C}}_{\mathrm{m},\mathrm{N}1}-{\mathrm{C}}_{\mathrm{m},\mathrm{P}1}-{\mathrm{C}}_{\mathrm{v}2}{\mathrm{C}}_{\mathrm{m},\mathrm{N}1}}{{\mathrm{C}}_{\mathrm{m},\mathrm{N}2}}}+{\mathrm{C}}_{\mathrm{v}2}-1\right)={\displaystyle \frac{1}{4}}{\mathrm{V}}_{\mathrm{n}}{l}_{\mathrm{n}}$$

(8)

$${\mathrm{M}}_{\mathrm{n},\mathrm{P}}\left(2{\mathrm{C}}_{\mathrm{v}2}-1\right)+{\mathrm{M}}_{\mathrm{n},\mathrm{N}}\left({\displaystyle \frac{{\mathrm{C}}_{\mathrm{v}2}/4+{\mathrm{C}}_{\mathrm{m},\mathrm{P}1}-{\mathrm{C}}_{\mathrm{m},\mathrm{N}1}-2{\mathrm{C}}_{\mathrm{v}2}{\mathrm{C}}_{\mathrm{m},\mathrm{P}1}}{{\mathrm{C}}_{\mathrm{m},\mathrm{N}2}}}+1\right)={\displaystyle \frac{1}{2}}{\mathrm{V}}_{\mathrm{n}}{l}_{\mathrm{n}}$$

(9)

$${\mathrm{M}}_{\mathrm{n},\mathrm{P}}\left({\displaystyle \frac{{\mathrm{C}}_{\mathrm{v}2}/8-{\mathrm{C}}_{\mathrm{m},\mathrm{N}2}}{{\mathrm{C}}_{\mathrm{m},\mathrm{P}1}}}\right)+{\mathrm{M}}_{\mathrm{n},\mathrm{N}}={\displaystyle \frac{1}{4}}{\mathrm{V}}_{\mathrm{n}}{l}_{\mathrm{n}}$$

(10)

$${\mathrm{M}}_{\mathrm{n},\mathrm{P}}\left({\displaystyle \frac{{\mathrm{C}}_{\mathrm{v}2}/4+{\mathrm{C}}_{\mathrm{m},\mathrm{N}2}-{\mathrm{C}}_{\mathrm{m},\mathrm{N}1}-2{\mathrm{C}}_{\mathrm{v}2}{\mathrm{C}}_{\mathrm{m},\mathrm{N}2}}{{\mathrm{C}}_{\mathrm{m},\mathrm{P}1}}}\right)+2{\mathrm{C}}_{\mathrm{v}2}{\mathrm{M}}_{\mathrm{n},\mathrm{N}}={\displaystyle \frac{1}{2}}{\mathrm{V}}_{\mathrm{n}}{l}_{\mathrm{n}}$$

(11)

2.2. Design Example

In this case, a calculation is performed on a three-span continuous slab, with and without CFRP strengthening, each having a clear span length of 2.65 m. Figure 1 illustrates the moment and shear coefficients for the end span in the column support case, where ${\mathrm{C}}_{\mathrm{m},\mathrm{N}1}=1/16,{\mathrm{C}}_{\mathrm{m},\mathrm{N}2}=1/10,{\mathrm{C}}_{\mathrm{m},\mathrm{P}}=1/14,{\mathrm{C}}_{\mathrm{v}1}=1,$ and ${\mathrm{C}}_{\mathrm{v}2}=1.15$. In Figure 3, the RC slab has a rectangular section (b × h) of 850 × 155 mm², reinforced with longitudinal and transverse No. 10 (ϕ 9.5 mm) bars, uniformly spaced on both the top and bottom sides. The CFRP thickness (t_F) is a variable in the analysis, with an initial value of 1 mm, and is applied to the tensile regions of the RC slab, covering the entire slab width, as shown in Figure 4.

Table 3. Key dimensions and material properties of the slab.

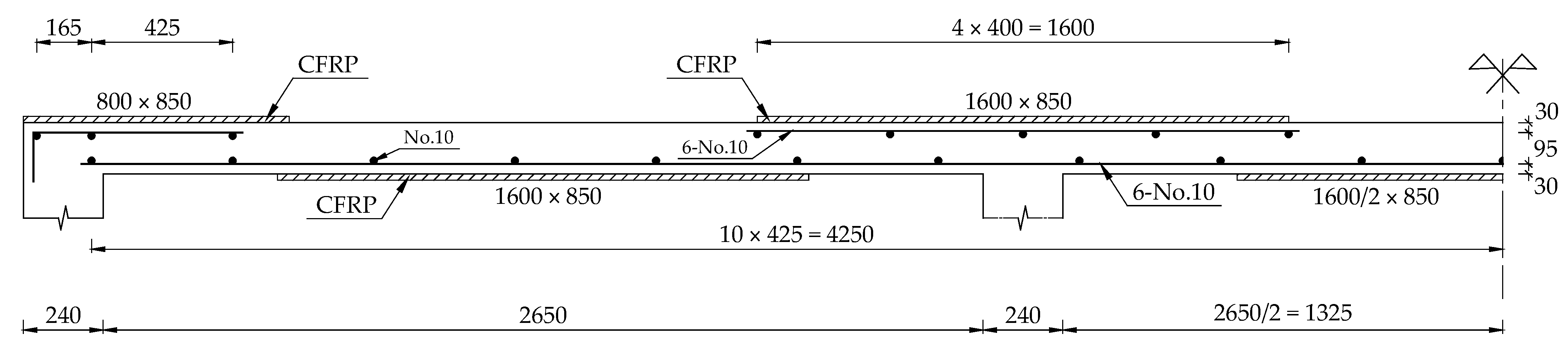
Section	RC Slab							CFRP
	f′c (MPa)	As (mm2)	d (mm)	fy (MPa)	Es (GPa)	γc (kg/m3)		f*fu (MPa)	Ef (GPa)	γF (kg/m3)
Mid-span	28	428	125	400	200	2400		600	40	1200
Supports

provides key dimensions and material properties. Preliminary calculations for the control and strengthened slabs are shown in

Table 4. Preliminary analysis of the slab.

Analysis	Control Slab
Nominal resistances	Mn,N = Mn,P = 20.67 kNm; Vn = 93.7 kN
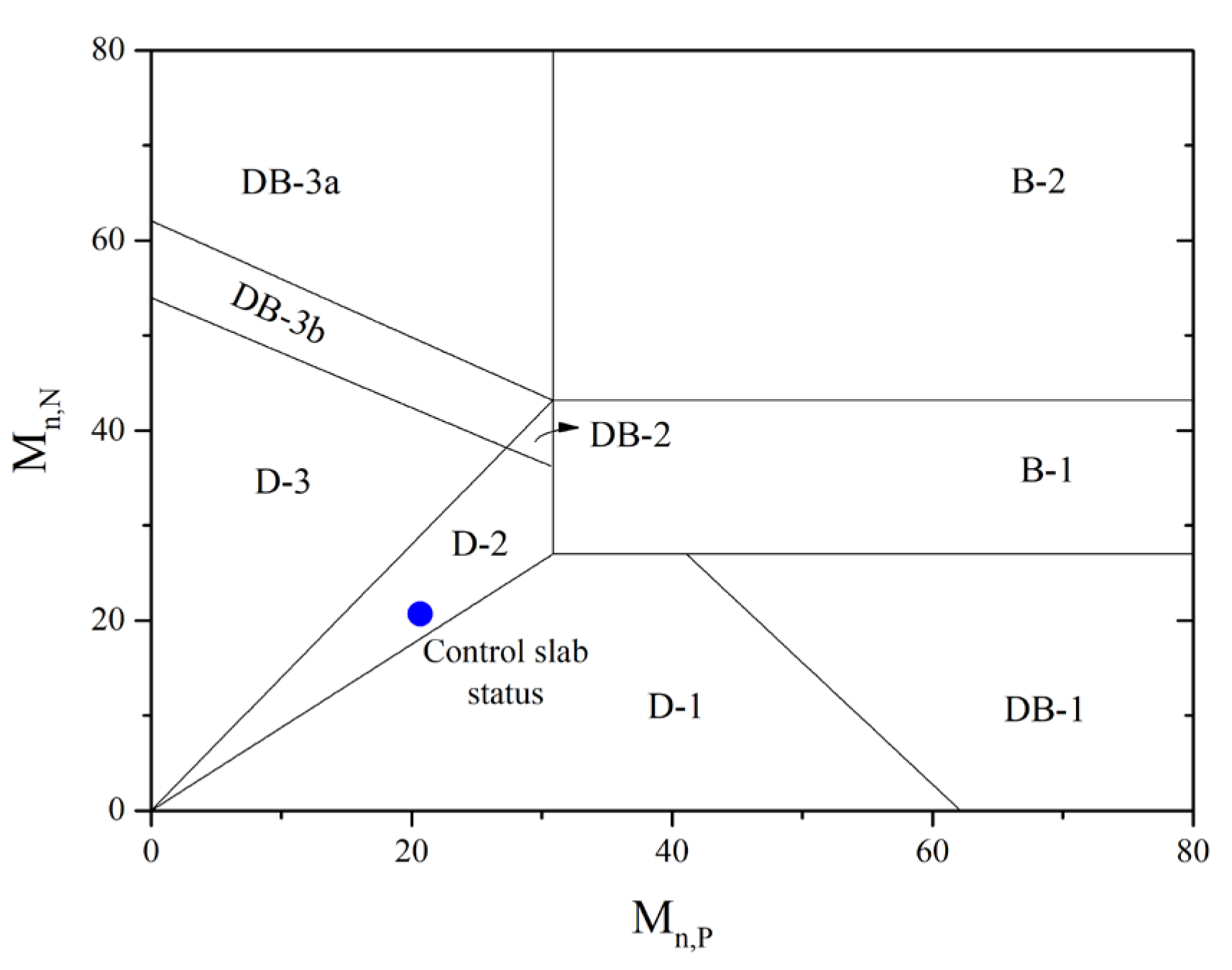
Failure region	D-2 according to Figure 5
Nominal failure load	wfn = 37.2 kN/m according to Equation (13)
Self-weight	${\mathrm{w}}_{\mathrm{c}}={\mathsf{\gamma }}_{\mathrm{c}}\mathrm{bh}$= 24(0.85)(0.155) = 3.16 kN/m
Young modulus	${\mathrm{E}}_{\mathrm{c}}=4700\sqrt{{\mathrm{f}}_{\mathrm{c}}^{\prime }}$= $4700\sqrt{28}$= 24,900 MPa
Support, kd	kd = 28 mm
Support, cracking moment	Icr,N = $3.86\times {10}^7{\mathrm{mm}}^4$
The ultimate strength and strain of CFRP (CE = 1)	${\mathrm{f}}_{\mathrm{fu}}={\mathrm{C}}_{\mathrm{E}}{\mathrm{f}}_{\mathrm{fu}}^{\ast }$ = 600 MPa; ${\mathsf{\epsilon }}_{\mathrm{fu}}={\displaystyle \frac{{\mathrm{f}}_{\mathrm{fu}}}{{\mathrm{E}}_{\mathrm{fe}}}}$ = 0.015
Moment due to dead load ${\mathrm{M}}_{\mathrm{D}}={\mathrm{C}}_{\mathrm{m}}\left({\mathrm{w}}_{\mathrm{c}}{l}_{\mathrm{n}}^2\right)$	at N2 section: ${\mathrm{M}}_{\mathrm{D},\mathrm{N}2}$ = ${\displaystyle \frac{1}{10}}\left(3.16\right)\left({2.65}^2\right)$= 2.22 kNm at mid-span section: ${\mathrm{M}}_{\mathrm{D},\mathrm{P}}$ = ${\displaystyle \frac{1}{14}}\left(3.16\right)\left({2.65}^2\right)$ = 1.59 kNm
Existing state of strain ${\mathsf{\epsilon }}_{\mathrm{bi}}={\displaystyle \frac{{\mathrm{M}}_{\mathrm{D}}\left({\mathrm{d}}_{\mathrm{f}}-\mathrm{kd}\right)}{{\mathrm{I}}_{\mathrm{cr}}{\mathrm{E}}_{\mathrm{c}}}}$	at N2 section: ${\mathsf{\epsilon }}_{\mathrm{bi},\mathrm{N}}$= ${\displaystyle \frac{2.22\times {10}^6\left(155-28\right)}{3.86\times {10}^7\left(\mathrm{24,900}\right)}}$= 0.000295 at mid-span section: ${\mathsf{\epsilon }}_{\mathrm{bi},\mathrm{P}}$= ${\displaystyle \frac{2.22\times {10}^6\left(155-28\right)}{3.86\times {10}^7\left(\mathrm{24,900}\right)}}$ = 0.000211

. The sectional resistances were evaluated to predict the slab’s failure mode based on established failure criteria. Accordingly, the CFRP sheet thickness was optimized to achieve the target flexural resistance and the desired failure mode. In this analysis, it is assumed that there is a perfect bond between CFRP laminates and concrete interface up to the failure load. The failure mode and status of the control slab are illustrated in Figure 5.

3. Numerical Simulation

3.1. Boundary Conditions, Element Type, and Mesh

The nonlinear behavior of the structural components was simulated using Abaqus/CAE (2024). In Figure 6, a quarter model of the full slab was built, leveraging its symmetry to minimize computational effort while maintaining accuracy. The symmetric plane was constrained in the x- and z-axes to simulate the boundary condition. The slab’s response, subjected to a uniform distributed load, was further investigated. The FE model was constructed using 8-noded solid elements (C3D8R) for the concrete slab, 2-noded linear truss elements (T3D2) for the steel bars, and 4-noded shell elements (S4R) for the CFRP. A convergence study was carried out to determine the optimal mesh density. Based on the results of preliminary analyses with element sizes ranging from 25 mm to 40 mm, a 30 mm element size was selected to ensure an acceptable balance between accuracy and computational efficiency, as shown in Figure 7. Abaqus’ embedded region constraint was used to model the concrete–steel bond, effectively simulating the interaction between the two materials. For the CFRP–concrete interface, a tie constraint was employed, consistent with the perfect bond assumption of the theoretical analysis. The models were subsequently analyzed under static loading conditions using the Abaqus/CAE software.

3.2. Material Model

The concrete damage plasticity (CDP) model is a widely accepted approach for simulating the behavior of plain and reinforced concrete, offering advanced stress–strain curves with significant advantages [47,48,49]. In this study, the CDP model was employed to analyze the static load behavior of concrete, proving highly effective for evaluating damage using the ABAQUS software [50]. Material properties, including tensile strength and Young’s modulus, were determined according to ACI 318M [46]. Figure 8 depicts the equivalent uniaxial stress–strain curve, accurately representing concrete’s nonlinear behavior in compression and tension based on the FIB model code [51,52]. Additionally, assuming axial force transmission within the steel reinforcement, a linear elastic material model was employed. Poisson’s ratio of steel was assumed to be 0.3. Material properties of the steel and CFRP are provided in Table 3.

4. Results and Discussion

4.1. Theoretical Analysis

Table 4 shows that the nominal failure load for the end span of the control slab was 37.2 kN/m, and Figure 5 identifies the failure region as D-2. Prior to initiating the design procedure, a preliminary analysis was performed for the control and strengthened slabs, as summarized in Table 4. In

Table 5. Calculation of strengthened slab using CFRP.

	At Support (N2 Section)	At Mid-Span
1. Assume CFRP thicknesses	tF,N = 1 mm	tF,P = 1 mm
2. Debonding strain of CFRP ${\mathsf{\epsilon }}_{\mathrm{fd}}=0.41\sqrt{{\displaystyle \frac{{\mathrm{f}}_{\mathrm{c}}^{\prime }}{{\mathrm{nE}}_{\mathrm{F}}{\mathrm{t}}_{\mathrm{F}}}}}\le 0.9{\mathsf{\epsilon }}_{\mathrm{fu}}$	${\mathsf{\epsilon }}_{\mathrm{fd}}=0.41\sqrt{{\displaystyle \frac{28}{1\left(\mathrm{40,000}\right)\left(1\right)}}}$ = 0.0108 $\le 0.9{\mathsf{\epsilon }}_{\mathrm{fu}}$ = 0.0135
3. Assume neutral axis depth	cN = 30.36 mm	cP = 30.38 mm
4. Calculate CFRP strain (εfe), CFRP stress (ffe), and concrete strain (εc) ${\mathsf{\epsilon }}_{\mathrm{fe}}=0.003\left({\displaystyle \frac{{\mathrm{d}}_{\mathrm{f}}-\mathrm{c}}{\mathrm{c}}}\right)-{\mathsf{\epsilon }}_{\mathrm{bi}}\le {\mathsf{\epsilon }}_{\mathrm{fd}}$ ${\mathrm{f}}_{\mathrm{fe}}={\mathsf{\epsilon }}_{\mathrm{fe}}{\mathrm{E}}_{\mathrm{F}}\le {\mathrm{f}}_{\mathrm{fu}}$ ${\mathsf{\epsilon }}_{\mathrm{c}}=({\mathsf{\epsilon }}_{\mathrm{fe}}+{\mathsf{\epsilon }}_{\mathrm{bi}})\left({\displaystyle \frac{\mathrm{c}}{{\mathrm{d}}_{\mathrm{f}}-\mathrm{c}}}\right)$	$\begin{array}{ll}{\mathsf{\epsilon }}_{\mathrm{fe},\mathrm{N}}& =0.003\left({\displaystyle \frac{155.5-30.36}{30.36}}\right)-0.000295\\ & =0.0108{ < \mathsf{\epsilon }}_{\mathrm{fd}}\end{array}$ ${\mathrm{f}}_{\mathrm{fe},\mathrm{N}}$ = 0.0108(40,000) = 433.9 MPa ${\mathsf{\epsilon }}_{\mathrm{c},\mathrm{N}}$ = $\left(0.0108+0.000295\right)\left({\displaystyle \frac{30.36}{155.5-30.36}}\right)$ = 0.0027	$\begin{array}{ll}{\mathsf{\epsilon }}_{\mathrm{fe},\mathrm{P}}& =0.003\left({\displaystyle \frac{155.5-30.38}{30.38}}\right)-0.000211\\ & =0.0108{ < \mathsf{\epsilon }}_{\mathrm{fd}}\end{array}$ ${\mathrm{f}}_{\mathrm{fe},\mathrm{P}}$ = 0.0108(40,000) = 433.9 MPa ${\mathsf{\epsilon }}_{\mathrm{c},\mathrm{P}}$ = $\left(0.0108+0.000211\right)\left({\displaystyle \frac{30.38}{155.5-30.38}}\right)$ = 0.00269
5. Calculate strain (εs) and stress (fs) in the reinforcing steel ${\mathsf{\epsilon }}_{\mathrm{s}}=({\mathsf{\epsilon }}_{\mathrm{fe}}+{\mathsf{\epsilon }}_{\mathrm{bi}})\left({\displaystyle \frac{\mathrm{d}-\mathrm{c}}{{\mathrm{d}}_{\mathrm{f}}-\mathrm{c}}}\right)$ ${\mathrm{f}}_{\mathrm{s}}={\mathsf{\epsilon }}_{\mathrm{s}}{\mathrm{E}}_{\mathrm{s}}\le {\mathrm{f}}_{\mathrm{y}}$	${\mathsf{\epsilon }}_{\mathrm{s},\mathrm{N}}$ = $\left(0.0108+0.000295\right)\left({\displaystyle \frac{125-30.36}{155.5-30.36}}\right)$ = 0.00843 ${\mathrm{f}}_{\mathrm{s},\mathrm{N}}$ = $0.00843\left(\mathrm{200,000}\right)$ = 1686 MPa $>{\mathrm{f}}_{\mathrm{y}}$ $\to {\mathrm{f}}_{\mathrm{s},\mathrm{N}}={\mathrm{f}}_{\mathrm{y}}=400 \mathrm{MPa}$	${\mathsf{\epsilon }}_{\mathrm{s},\mathrm{P}}$ = $\left(0.0108+0.000211\right)\left({\displaystyle \frac{125-30.38}{155.5-30.38}}\right)$ = 0.00836 ${\mathrm{f}}_{\mathrm{s},\mathrm{P}}$ = $0.00836\left(\mathrm{200,000}\right)$ = 1672 MPa $>{\mathrm{f}}_{\mathrm{y}}$ $\to {\mathrm{f}}_{\mathrm{s},\mathrm{N}}={\mathrm{f}}_{\mathrm{y}}=400 \mathrm{MPa}$
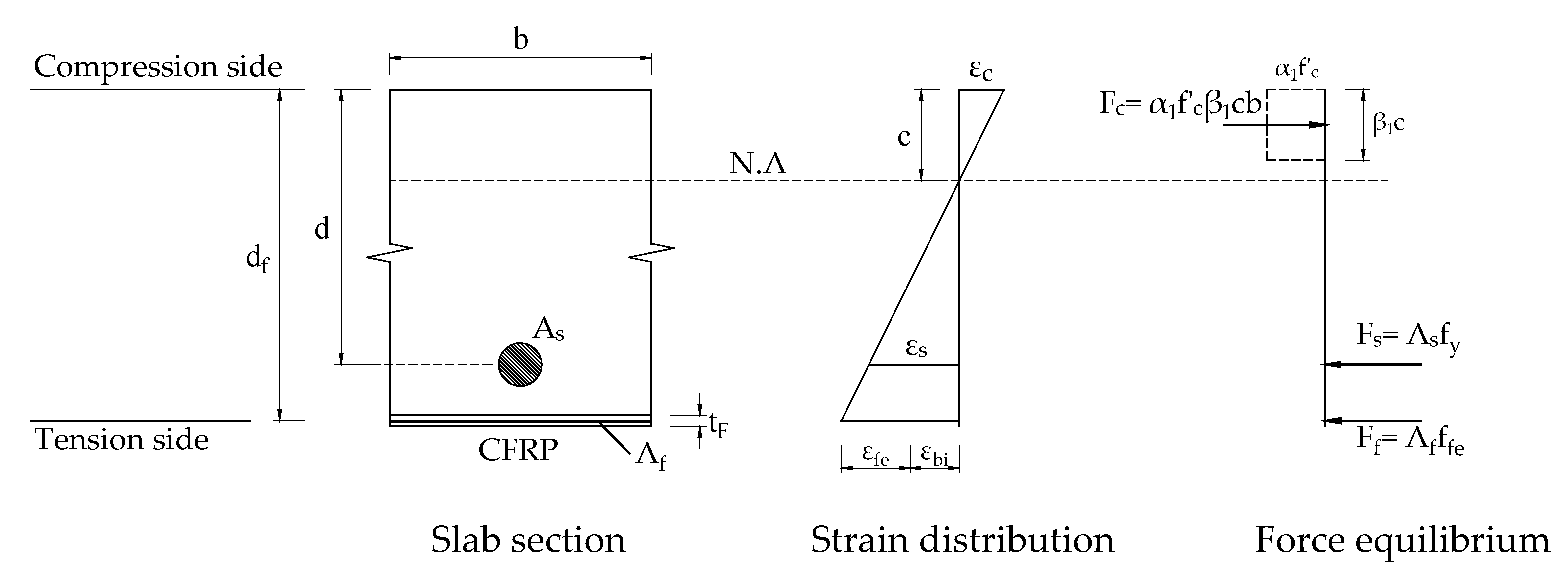
6. Check for force equilibrium ${\mathsf{\beta }}_1={\displaystyle \frac{4{\mathsf{\epsilon }}_{\mathrm{c}}^{\prime }-{\mathsf{\epsilon }}_{\mathrm{c}}}{6{\mathsf{\epsilon }}_{\mathrm{c}}^{\prime }-2{\mathsf{\epsilon }}_{\mathrm{c}}}}$; ${\mathsf{\alpha }}_1={\displaystyle \frac{3{\mathsf{\epsilon }}_{\mathrm{c}}^{\prime }{\mathsf{\epsilon }}_{\mathrm{c}}-{\mathsf{\epsilon }}_{\mathrm{c}}^2}{3{\mathsf{\beta }}_1{{\mathsf{\epsilon }}_{\mathrm{c}}^{\prime }}^2}}$ ${\mathsf{\epsilon }}_{\mathrm{c}}^{\prime }$ is the strain relative to ${\mathrm{f}}_{\mathrm{c}}^{\prime }$ ${\mathsf{\epsilon }}_{\mathrm{c}}^{\prime }={\displaystyle \frac{1.7{\mathrm{f}}_{\mathrm{c}}^{\prime }}{{\mathrm{E}}_{\mathrm{c}}}}$ Check the neutral axis depth $\mathrm{c}={\displaystyle \frac{{\mathrm{A}}_{\mathrm{s}}{\mathrm{f}}_{\mathrm{s}}+{\mathrm{t}}_{\mathrm{F}}{\mathrm{b}\mathsf{\epsilon }}_{\mathrm{fe}}{\mathrm{E}}_{\mathrm{F}}}{{\mathsf{\alpha }}_1{\mathrm{f}}_{\mathrm{c}}^{\prime }{ \mathsf{\beta }}_1\mathrm{b}}}$	${\mathsf{\epsilon }}_{\mathrm{c}}^{\prime }={\displaystyle \frac{1.7(28)}{\mathrm{24,900}}}=0.0019$ ${\mathsf{\beta }}_{1,\mathrm{N}}={\displaystyle \frac{4(0.0019)-0.0027}{6(0.0019)-2(0.0027)}}=0.815$ ${\mathsf{\alpha }}_{1,\mathrm{N}}={\displaystyle \frac{3(0.0019)(0.0027)-{0.0027}^2}{3(0.77)({0.0019}^2)}}=0.917$ $\begin{array}{ll}{\mathrm{c}}_{\mathrm{N}}& ={\displaystyle \frac{(428)(400)+(1)(850)(0.0108)(\mathrm{40,000})}{(0.917)(28)(0.815)(850)}}\\ & =30.36 \mathrm{mm} \left(\mathrm{OK}\right)\end{array}$	${\mathsf{\beta }}_{1,\mathrm{P}}={\displaystyle \frac{4(0.0019)-0.00269}{6(0.0019)-2(0.00269)}}=0.813$ ${\mathsf{\alpha }}_{1,\mathrm{P}}={\displaystyle \frac{3(0.0019)(0.00269)-{0.00269}^2}{3(0.813)({0.0019}^2)}}=0.919$ $\begin{array}{ll}{\mathrm{c}}_{\mathrm{P}}& ={\displaystyle \frac{(428)(400)+(1)(850)(0.0108)(\mathrm{40,000})}{(0.919)(28)(0.813)(850)}}\\ & =30.38 \mathrm{mm} \left(\mathrm{OK}\right)\end{array}$
7. Calculate nominal flexural strength ${\mathrm{M}}_{\mathrm{n}}={\mathrm{M}}_{\mathrm{ns}}+{\mathsf{\psi }}_{\mathrm{f}}{\mathrm{M}}_{\mathrm{nf}}$ (${\mathsf{\psi }}_{\mathrm{f}}=0.85$) Steel contribution: ${\mathrm{M}}_{\mathrm{ns}}={\mathrm{A}}_{\mathrm{s}}{\mathrm{f}}_{\mathrm{s}}(\mathrm{d}-{\displaystyle \frac{{\mathsf{\beta }}_1\mathrm{c}}{2}})$ CFRP contribution: ${\mathrm{M}}_{\mathrm{nf}}={\mathrm{A}}_{\mathrm{f}}{\mathrm{f}}_{\mathrm{fe}}\left({\mathrm{d}}_{\mathrm{f}}-{\displaystyle \frac{{\mathsf{\beta }}_1\mathrm{c}}{2}}\right)$	$\begin{array}{ll}{\mathrm{M}}_{\mathrm{ns},\mathrm{N}}& =(428\left)\right(400)\left(125-{\displaystyle \frac{(0.815\left)\right(30.36)}{2}}\right)\\ & =19.28 \mathrm{kNm}\end{array}$ $\begin{array}{ll}{\mathrm{M}}_{\mathrm{nf},\mathrm{N}}& =(1\left)\right(433.9)\left(155.5-{\displaystyle \frac{(0.815\left)\right(30.36)}{2}}\right)\\ & =52.79 \mathrm{kNm}\end{array}$ ${\mathrm{M}}_{\mathrm{n},\mathrm{N}}=19.28+(0.85\left)\right(52.8)=72.07 \mathrm{kNm}$	$\begin{array}{ll}{\mathrm{M}}_{\mathrm{ns},\mathrm{P}}& =(428\left)\right(400)\left(125-{\displaystyle \frac{(0.813\left)\right(30.38)}{2}}\right)\\ & =19.3 \mathrm{kNm}\end{array}$ $\begin{array}{ll}{\mathrm{M}}_{\mathrm{nf},\mathrm{P}}& =(1\left)\right(433.9)\left(155.5-{\displaystyle \frac{(0.813\left)\right(30.38)}{2}}\right)\\ & =52.8 \mathrm{kNm}\end{array}$ ${\mathrm{M}}_{\mathrm{n},\mathrm{P}}=19.3+(0.85\left)\right(52.8)=72.08 \mathrm{kNm}$
8. Calculate nominal shear strength ${\mathrm{V}}_{\mathrm{n}}=\left(\mathrm{d}\sqrt{{\mathrm{f}}_{\mathrm{c}}^{\prime }}\right){\displaystyle \frac{\mathrm{b}}{6}}$	${\mathrm{V}}_{\mathrm{n}}=(125\sqrt{28}){\displaystyle \frac{850}{6}}=93.7 \mathrm{kN}$
9. Determine failure region and nominal failure load ${\mathrm{w}}_{\mathrm{fn}}={\displaystyle \frac{{2\mathrm{V}}_{\mathrm{n}}}{{\mathrm{C}}_{\mathrm{v}2}{l}_{\mathrm{n}}}}$	B-2, as shown in Figure 9a; Equation (15), ${\mathrm{w}}_{\mathrm{fn}}={\displaystyle \frac{2(93.7)}{(1.15)(2.65)}}=61.5 \mathrm{kN}/\mathrm{m}$
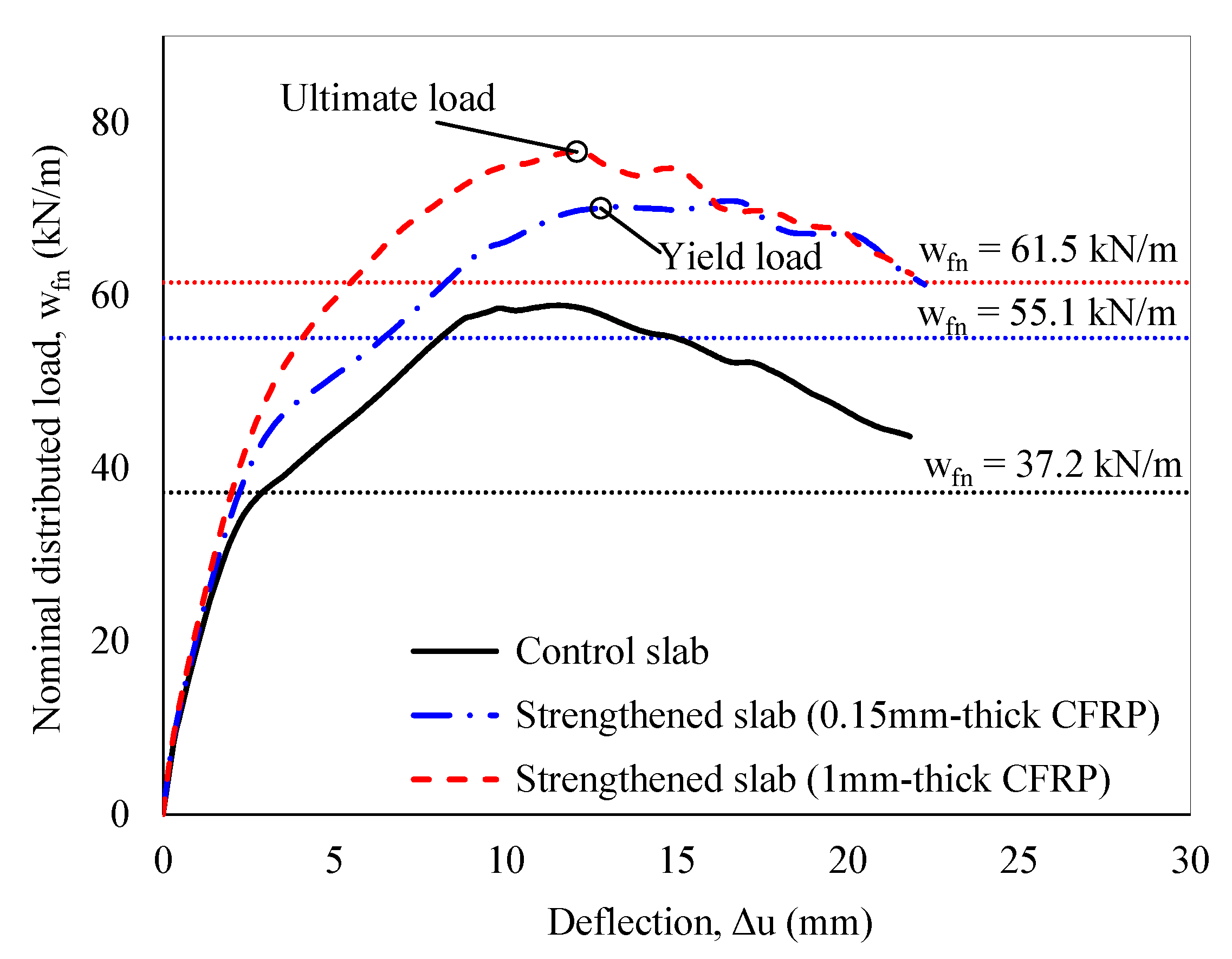
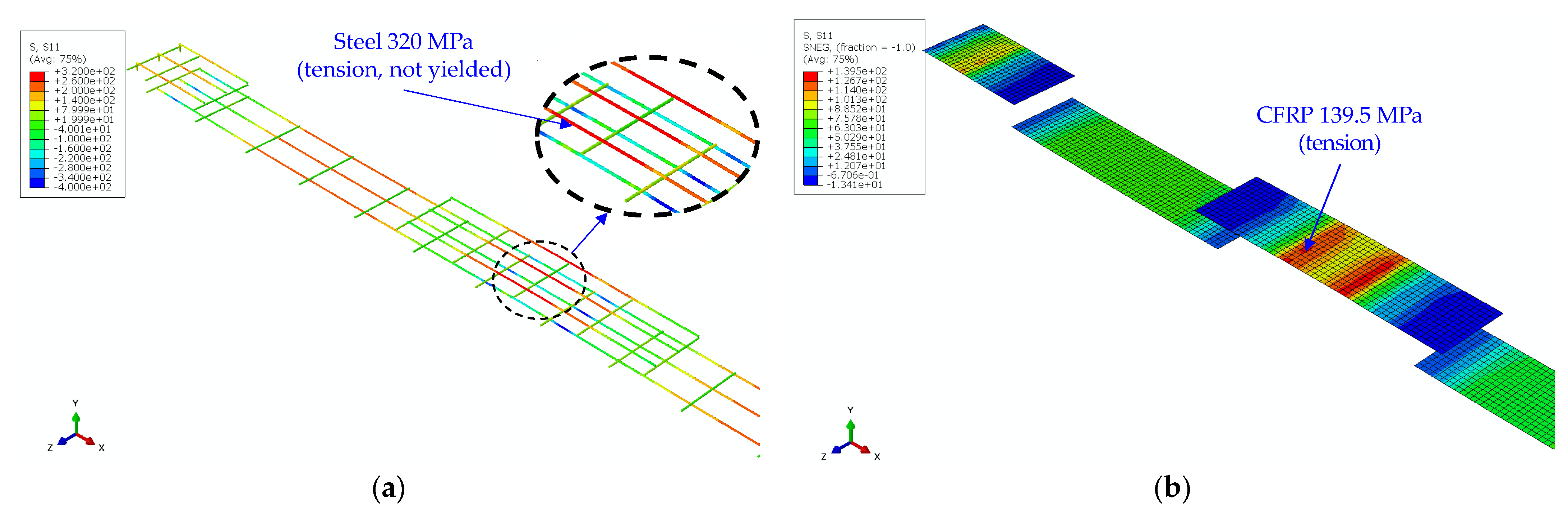
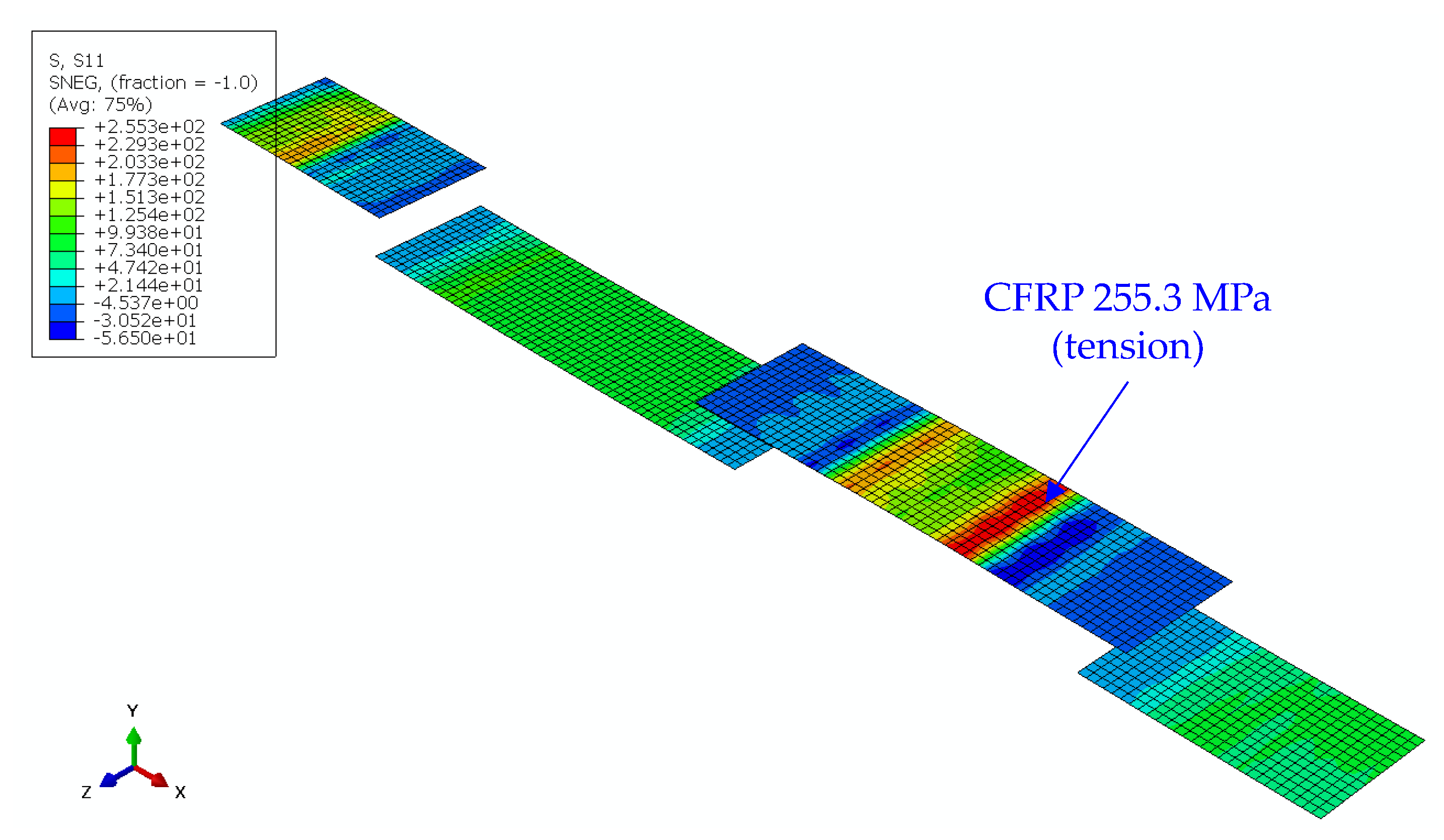
Iteratively adjust the CFRP thickness to ensure ductile failure	This can be achieved with tF = 0.15 mm; Failure region D-2, as shown in Figure 9b; Failure load wfn = 55.1 kN/m

, the calculation for the strengthened slab with an initial CFRP thickness of 1 mm was detailed, as recommended by the ACI 440.2R [45]. In step 2, the debonding strain of CFRP was determined. In step 3, the neutral axis depth was assumed and applied in steps 4 and 5. An iterative procedure was then employed in step 6 to achieve force equilibrium before determining nominal flexural and shear strengths in steps 7 and 8. Finally, the nominal failure load was calculated in step 9. The 1 mm CFRP-strengthened slab exhibited brittle failure in region B-2 (Figure 9a) at a nominal failure load of 61.5 kN/m. While the nominal failure load is increased by 165% compared to the control slab, it results in undesirable brittle failure.

As previously mentioned, established failure limits based on moment and shear capacities are essential for determining the appropriate CFRP amount, preventing excessive flexural strength enhancement over shear strength that could lead to brittle shear failure. CFRP sheet strengthening can be flexibly adjusted in terms of thickness, width, and strengthening schemes, allowing the slab to attain the desired ductile failure in regions D-1, D-2, or D-3. Specifically, a similar analysis using a CFRP thickness of 0.15 mm revealed ductile failures in the failure region D-2 (Figure 9b), with a corresponding failure load of 55.1 kN/m.

Table 6. Summary of the result analysis for the control and strengthened slabs.

Slab	Failure Region	wfn (kN/m)		tF (mm)	Failure Mode
Control slab	D-2	37.2	[100%]	-	Ductile
Strengthened slab	D-2	55.1	[148%]	0.15	Ductile
	B-2	61.5	[165%]	1.00	Brittle

indicates a substantial increase in failure load for the strengthened slab with a 0.15 mm thick CFRP sheet, achieving a remarkable 148% enhancement compared to the control slab. Importantly, this enhancement is accompanied by a desirable ductile failure mode, significantly mitigating concerns about sudden failure.

4.2. Numerical Analysis

FE models for both strengthened and unstrengthened continuous slabs were developed to evaluate their global responses under quasi-static loading conditions. The interaction between the CFRP and concrete in the strengthened slab was simulated using a perfect bond assumption. Figure 10 presents a comparison of the nominal uniformly distributed load–deflection relationships for both strengthened and unstrengthened slabs, emphasizing the strong agreement between numerical and theoretical predictions. Figure 11 presents the strain distribution in CFRP sheets for two different strengthening scenarios. The measured strains in the CFRP sheets for the 1 mm thick and 0.15 mm thick CFRP layers at ultimate failure were 0.003 and 0.006, respectively. These values are significantly lower than the respective debonding strains of 0.0108 and 0.0135, as recommended by ACI 440.2R [45]. These findings supported the assumption of a perfect bond of CFRP-to-concrete interface, which was considered in the analysis.

The numerical results suggested the theoretical analysis may be conservative in its load-bearing capacity predictions. For instance, the strengthened slab with a 1 mm thick CFRP sheet reached an ultimate distributed failure load of 76.6 kN/m at a deflection of 12.1 mm, exceeding the theoretical prediction of 61.5 kN/m by 125%, as shown in Figure 10. Figure 12 shows the stress distribution at the ultimate load, with maximum tensile stresses of 320 MPa in the steel reinforcement and 139.5 MPa in the CFRP sheet. Notably, the slab reached its ultimate load before any yielding of the steel reinforcement, confirming the brittle failure mode predicted by the theoretical analysis.

The 0.15 mm CFRP-strengthened slab demonstrated a yield load of 70.3 kN/m at a deflection of 13.3 mm, surpassing the theoretical prediction (55.1 kN/m) by 128%. Figure 13 depicts the stress distribution at the yielding load, with tensile stresses of 400 MPa in the steel reinforcement and 240 MPa in the CFRP sheet. It indicates that the steel reinforcement reached its yield limit, confirming the occurrence of ductile failure in the slab strengthened with a 0.15 mm thick CFRP sheet. Additionally, Figure 14 shows that the tensile stress in the CFRP sheet at ultimate failure reached 255.3 MPa, significantly higher than that observed in the 1 mm thick CFRP case. It demonstrates the effectiveness of the proposed approach in achieving the more efficient utilization of the material’s load-bearing capacity.

5. Conclusions

The reliability of the proposed design approach was enhanced through a comprehensive evaluation of failure modes, involving a combination of theoretical analysis based on ACI 440.2R guidelines and numerical simulations conducted using Abaqus/CAE software. The methodology establishes strengthening limits based on moment and shear capacities, enabling the optimization of the CFRP amount to improve bearing performance and ensure ductile failure. The refined optimization process demonstrated that a 0.15 mm thick CFRP layer could increase the nominal failure load by 148% while maintaining desirable ductile failure behavior. Additionally, numerical analysis confirmed the proposed approach’s effectiveness, showing that the strengthened slab with 0.15 mm thick CFRP exhibited ductile failure—evidenced by yielded steel reinforcement—while the slab with 1 mm thick CFRP experienced brittle failure. Nevertheless, further experimental investigations, including large-scale testing under varying environmental conditions and long-term performance evaluations, are recommended to validate the proposed approach for practical applications.