A Study on the Longitudinal Deformation of the Underlying Oblique Crossing Tunnel Induced by Foundation Excavation
Abstract
:1. Introduction
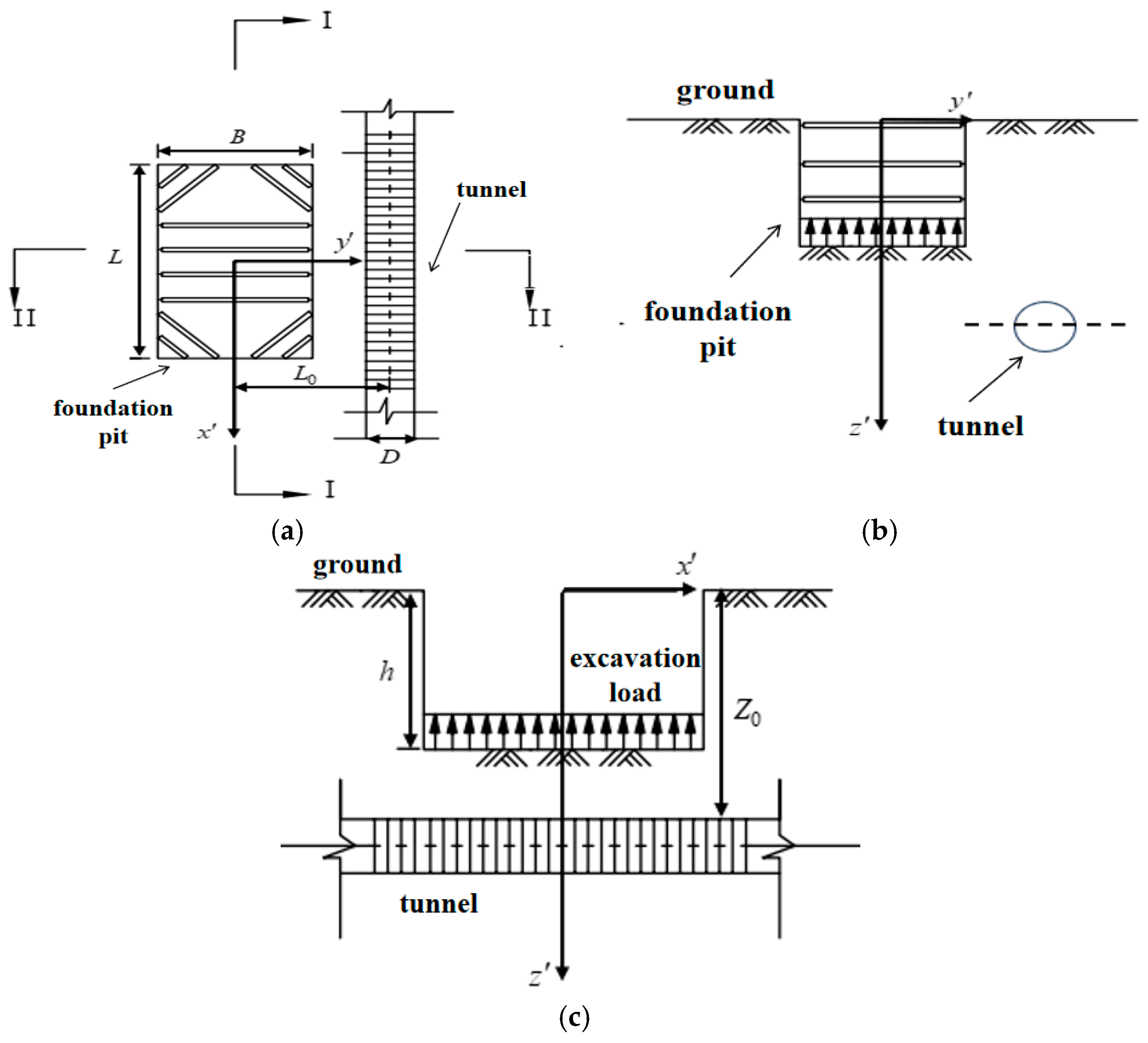
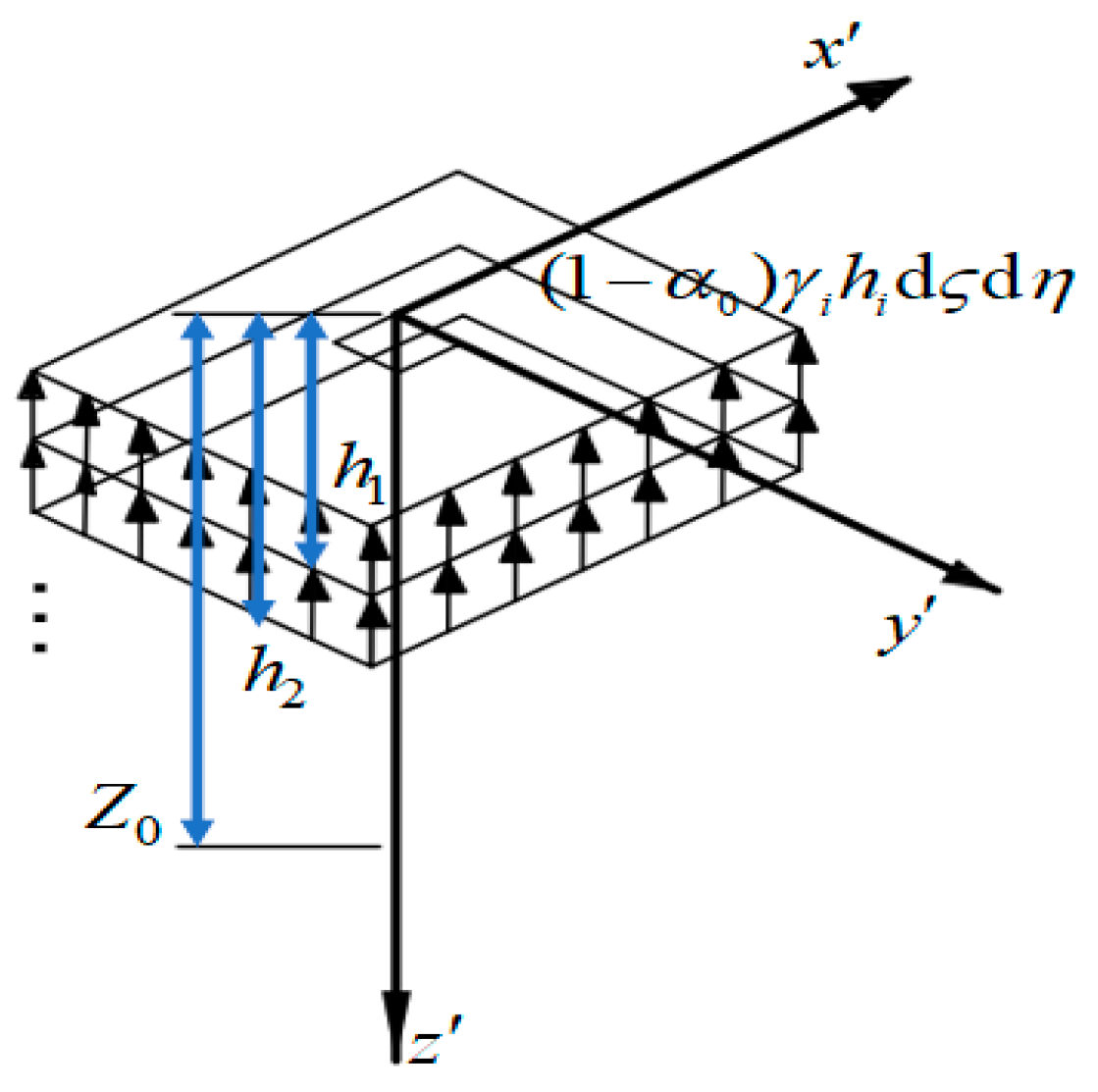
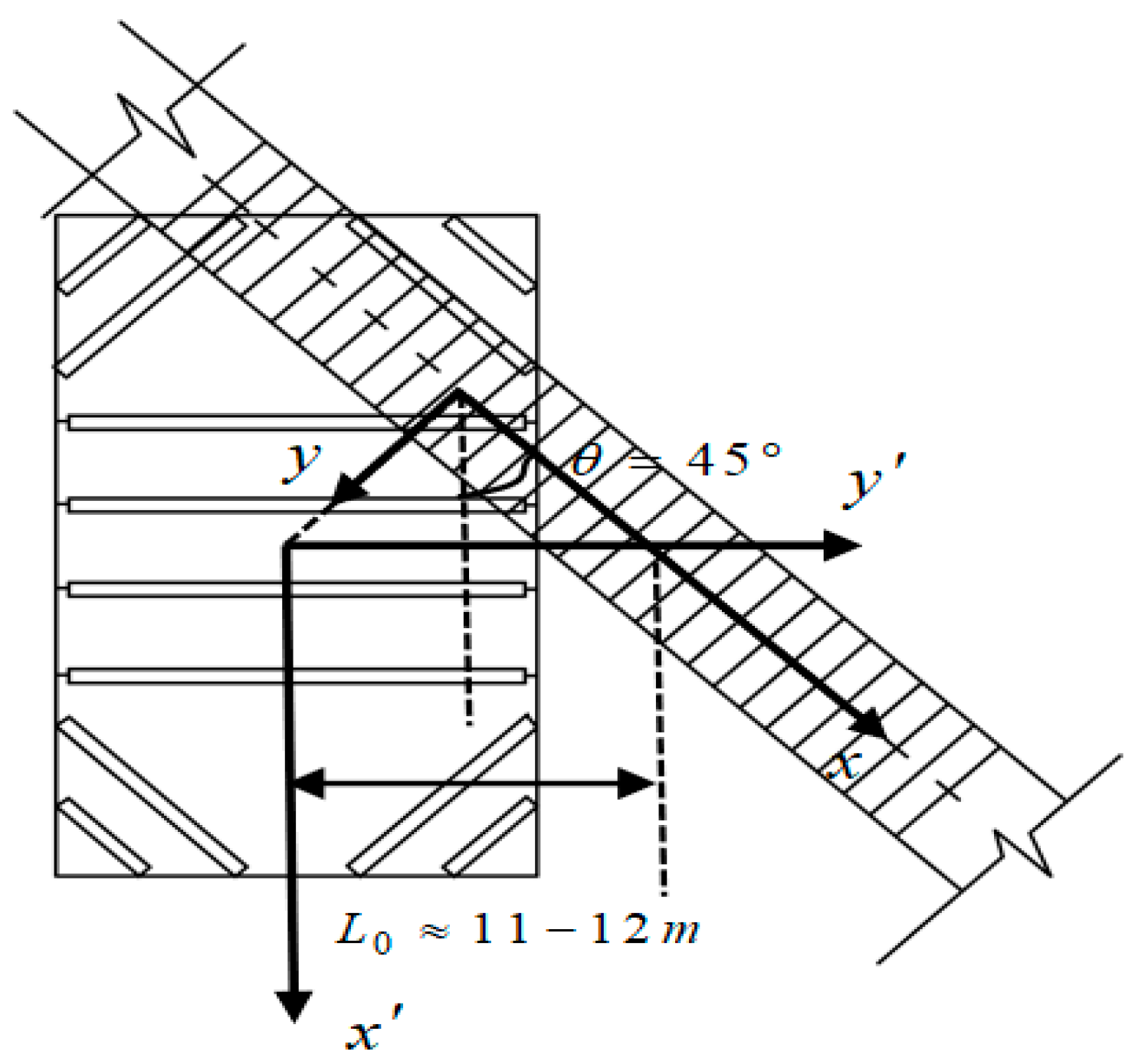
2. Foundation Pit Excavation Induces Additional Tunnel Stress
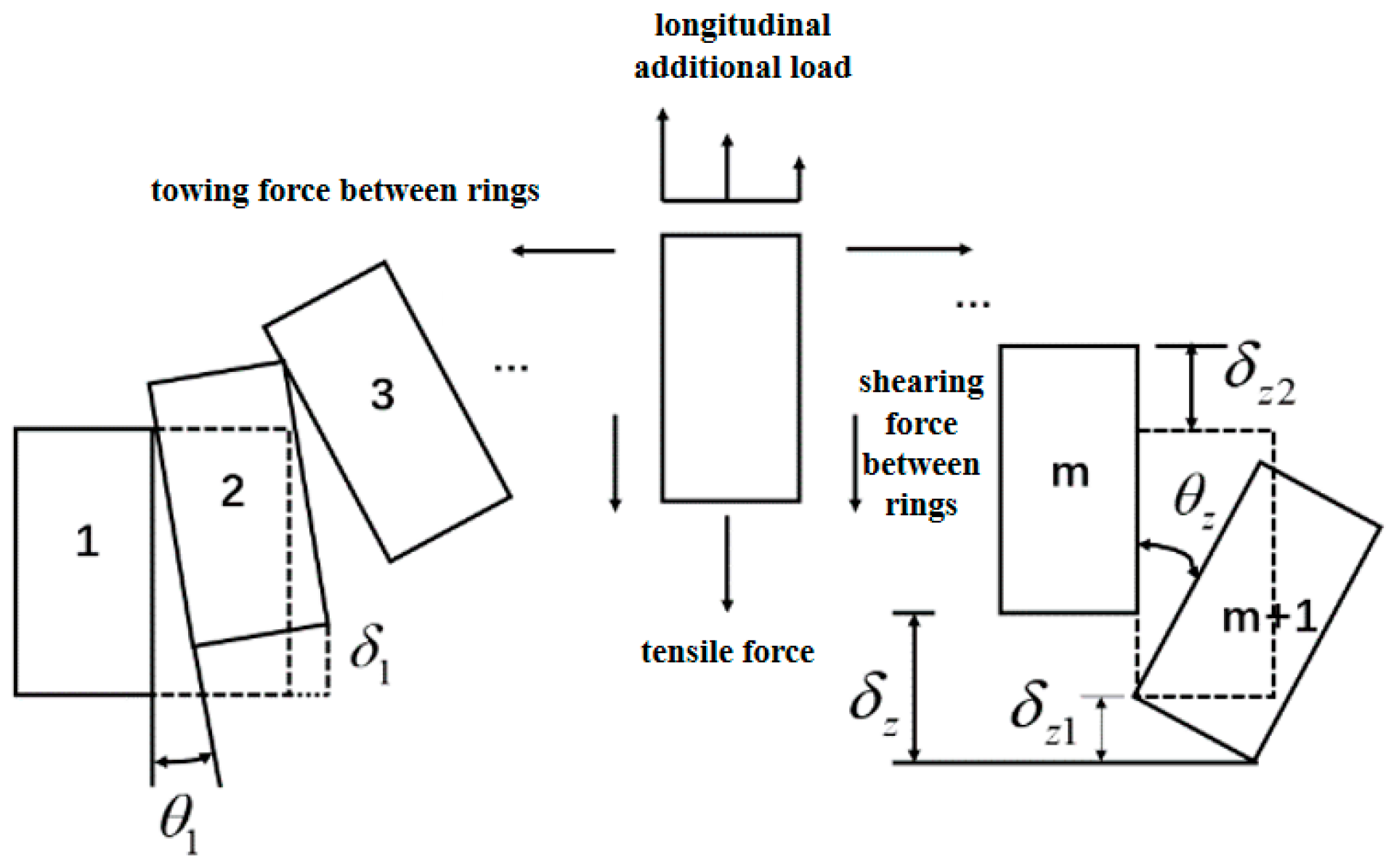
3. The Calculation of Longitudinal Deformation of Tunnels Considering the Rotation and Dislocation Between Segment Rings
3.1. The Deformation Model of the Tunnel Under the Synergy of Rotation and Dislocation
3.2. Total Potential Energy of Shield Tunnel Deformation
3.3. The Longitudinal Displacement of the Shield Tunnel Is Expanded in Series
3.4. Solving Variational Control Equations
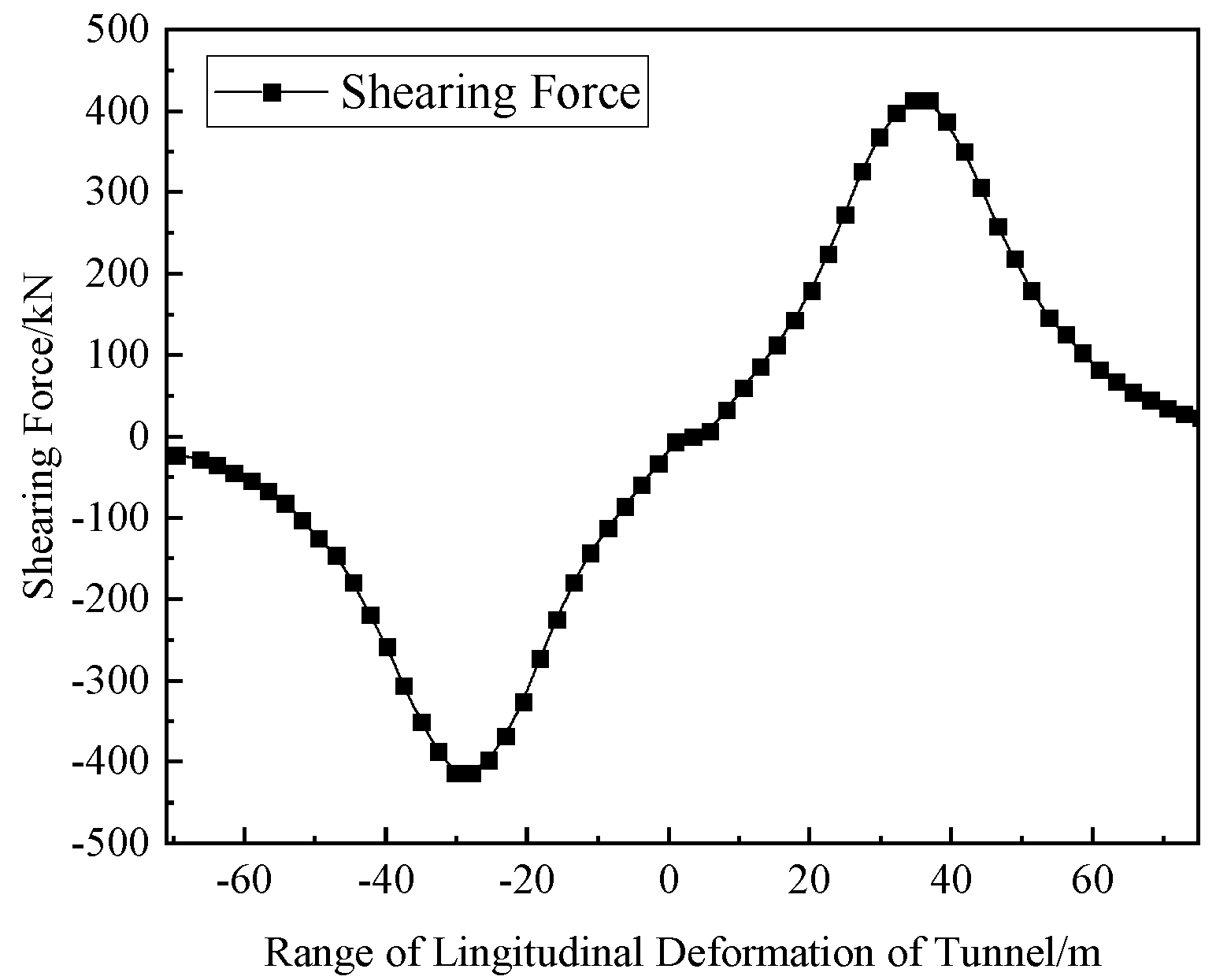
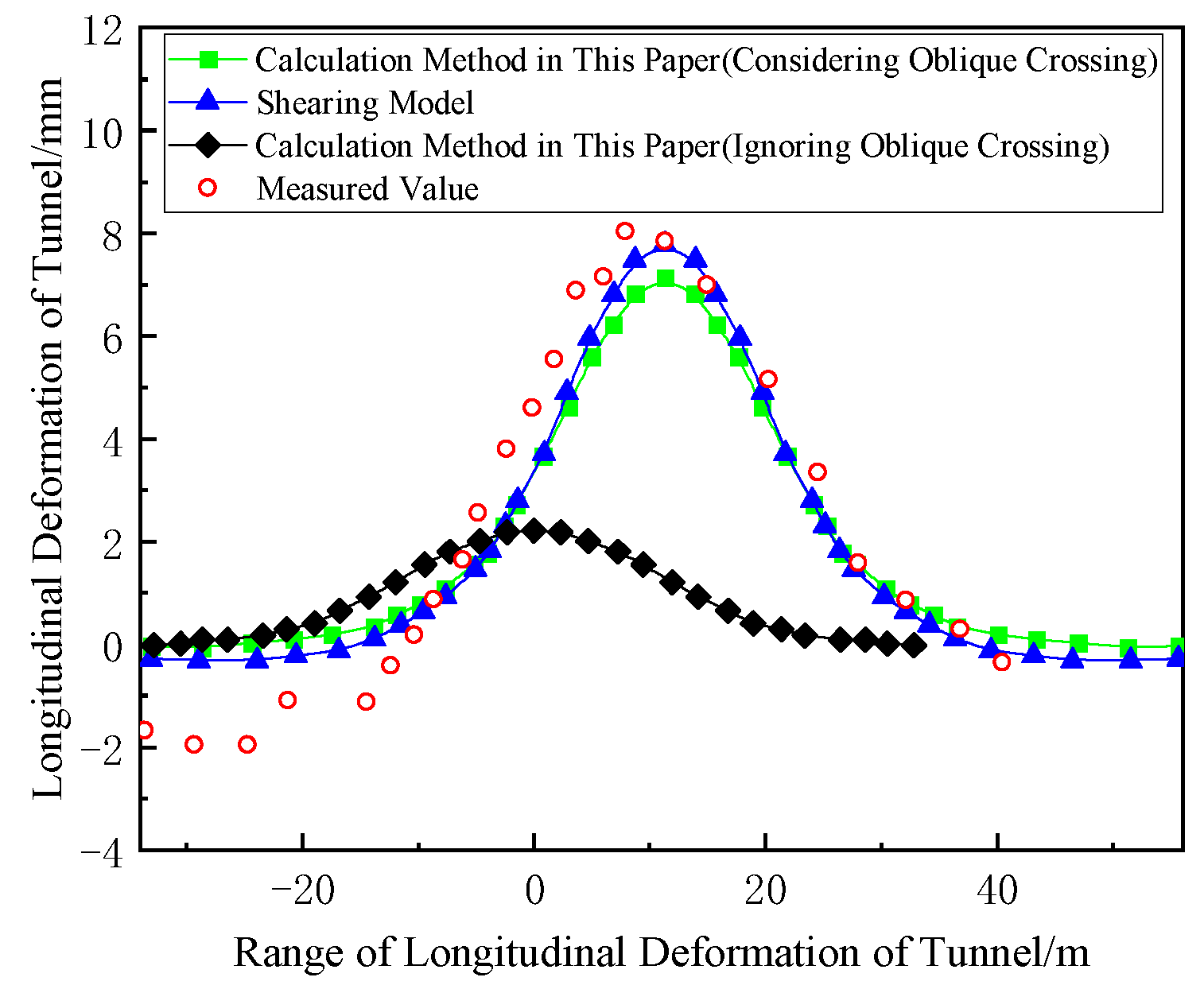
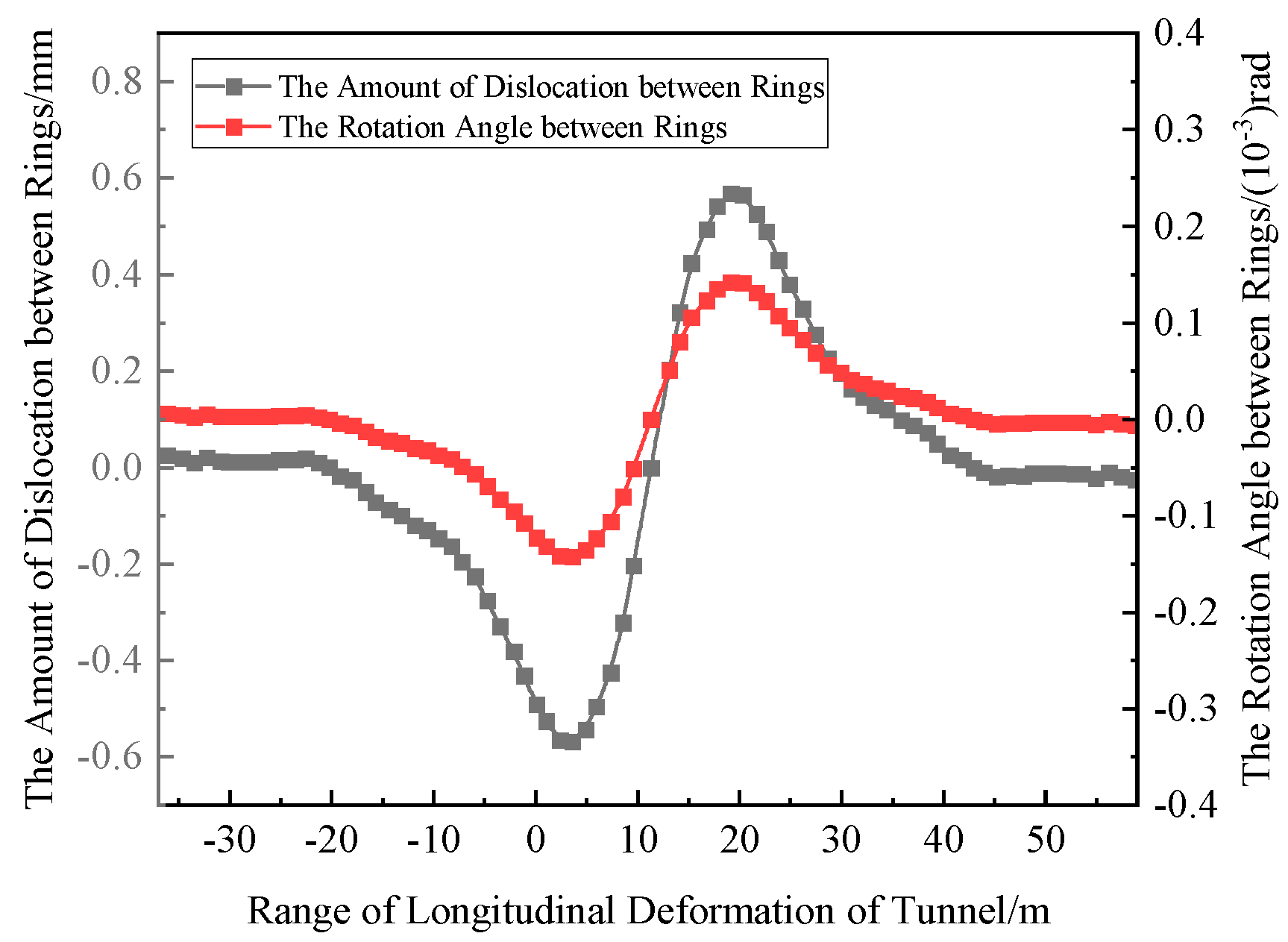
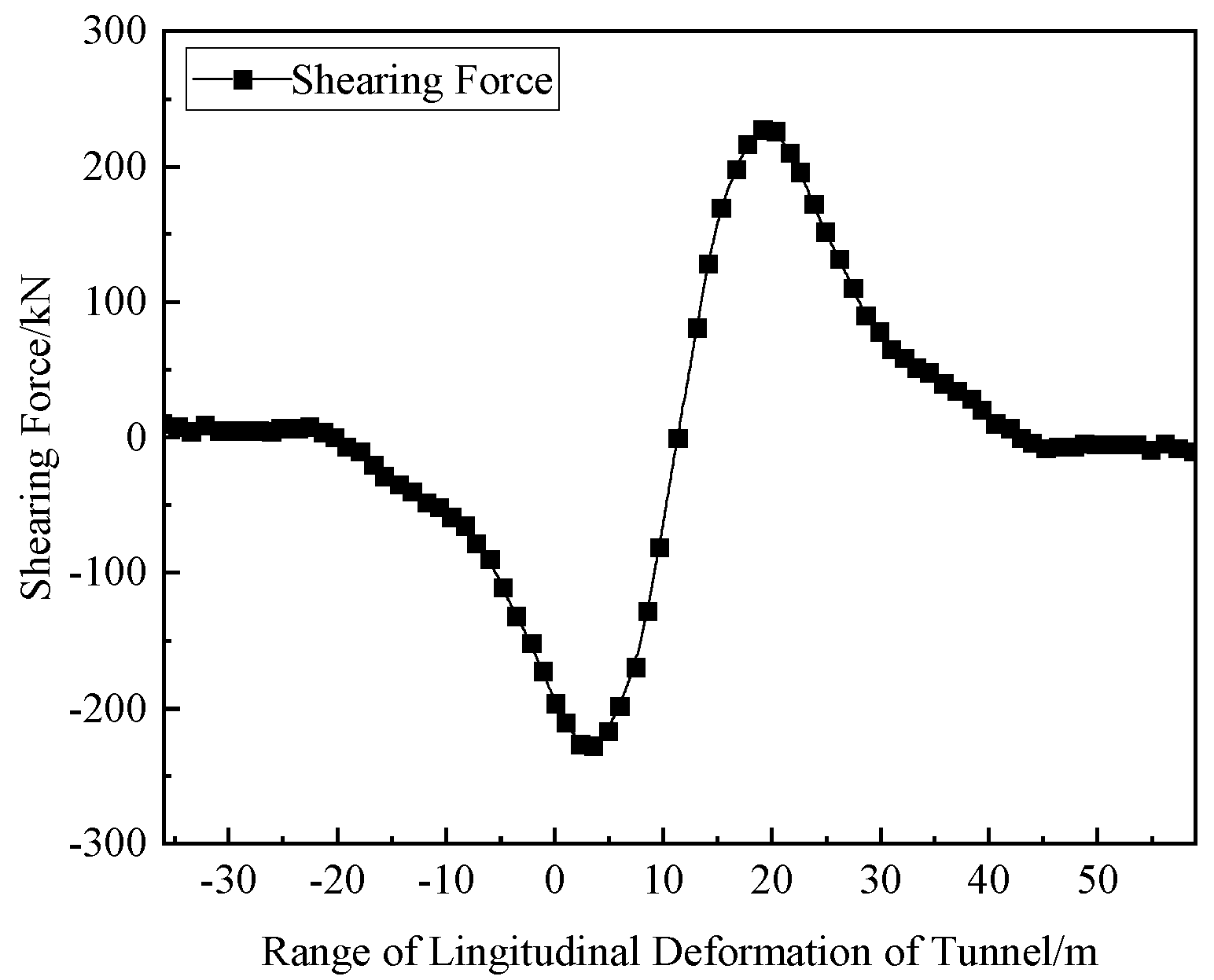
4. Example Verification
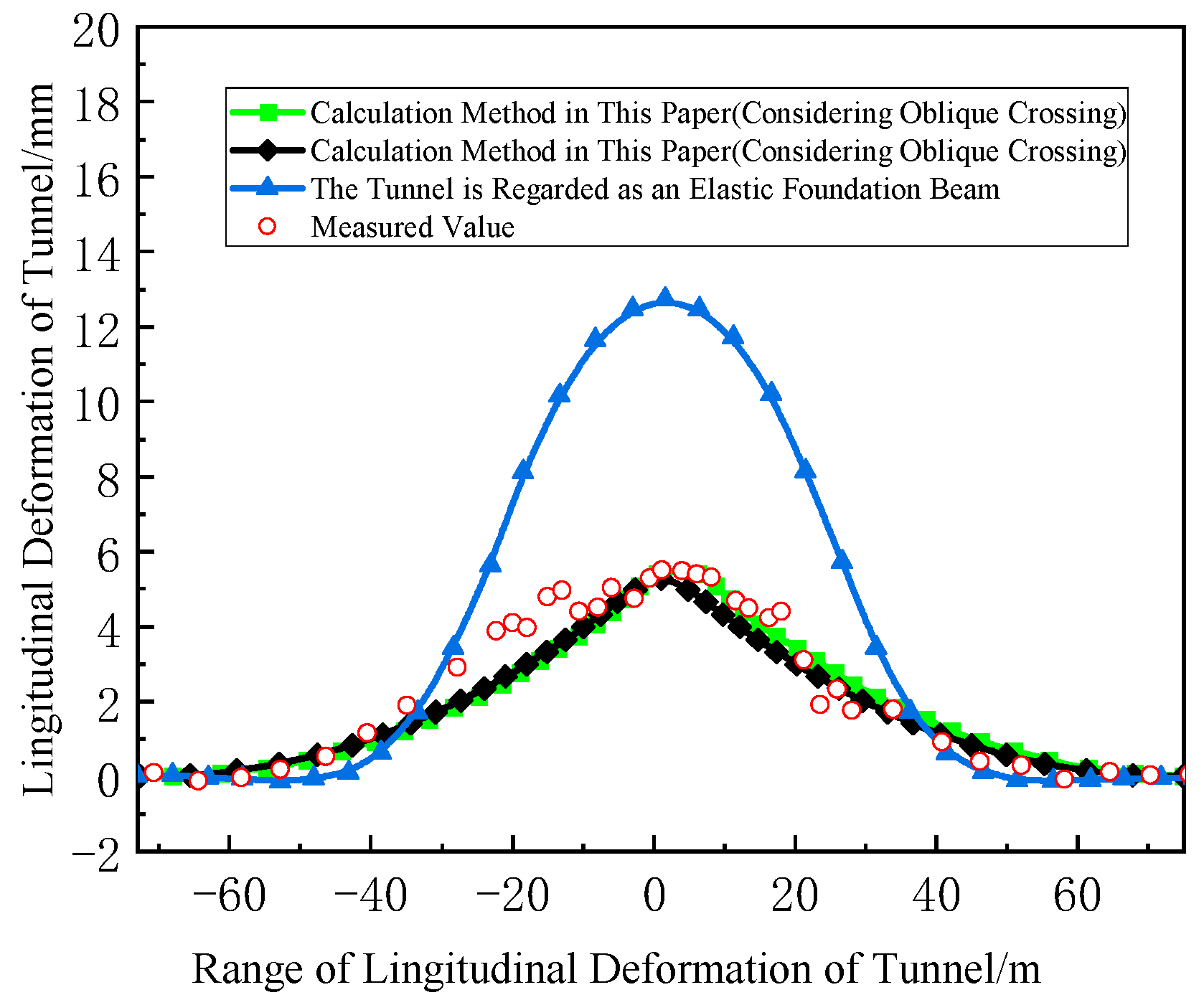
4.1. Case 1 (Hangzhou Jiusha River Foundation Pit Project)
4.2. Case 2 (Shanghai Metro Line 2 Is Adjacent to the Foundation Pit)
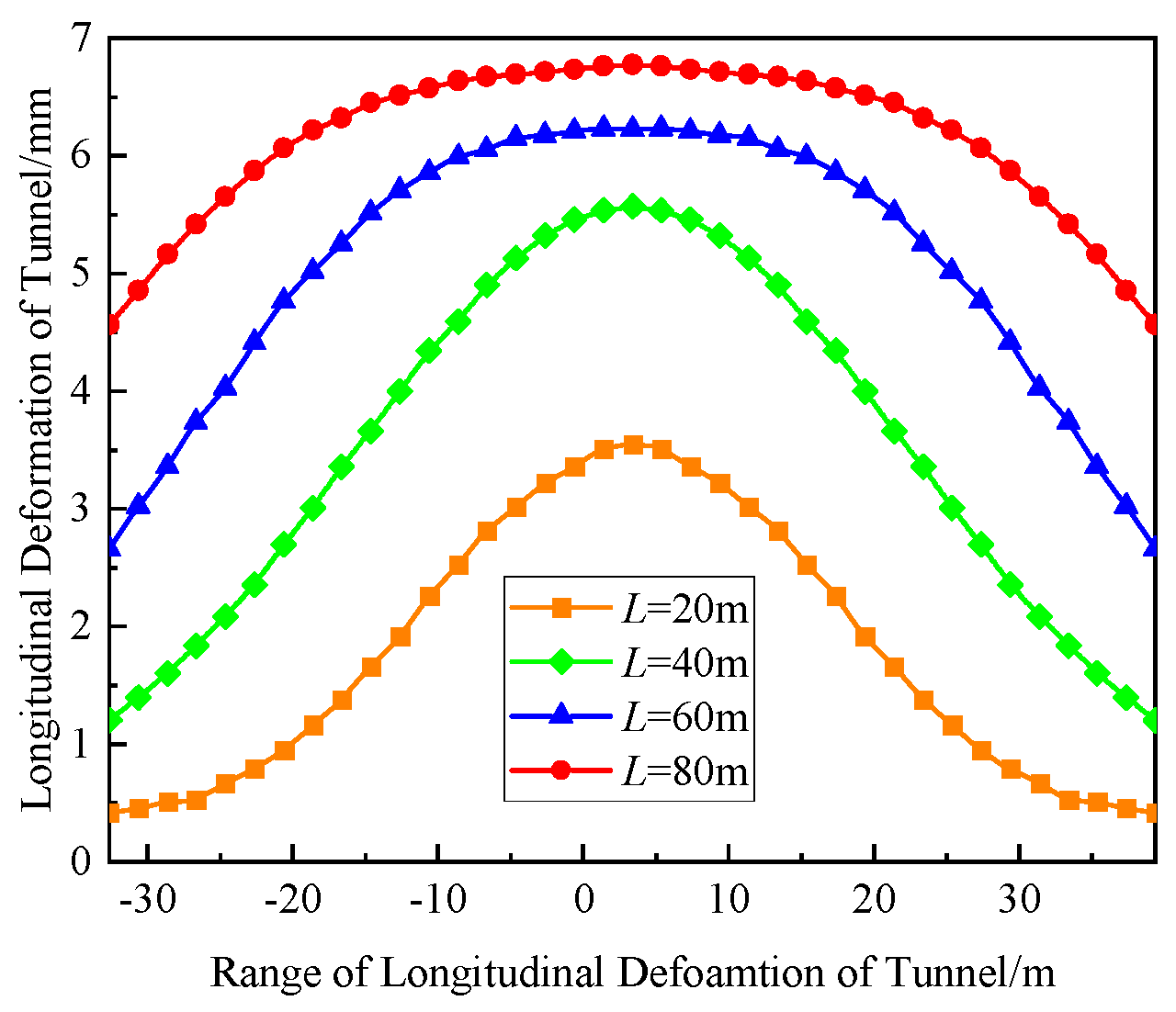
5. Parameter Analysis
5.1. Proportion Coefficient of the Rigid Body Rotation Effect of Segment Rings
5.2. Number of Rings for Tunnel Segment Calculation
5.3. Excavation Length
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Li, J.Y.; Pan, L.D.; Hu, M.Y.; Peng, K.S. A modified calculation method of heave deformation of underlying tunnel caused by excavation. Chin. J. Ground Improv. 2019, 1, 10–19. [Google Scholar]
- Wei, G.; Hu, L.W.; Chen, K.L. Numerical Simulation of Effect of Foundation Pit Excavation on Underlain Shield Tunnel. In Proceedings of the 4th International Conference on Civil Engineering, Architecture and Building Materials (CEABM), Haikou, China, 24–25 May 2014; pp. 1001–1007. [Google Scholar]
- Xu, L.; Huang, H.W. Effect of foundation pit excavation on underlying metro tunnels. Chin. J. Geotech. Eng. 2008, 30 (Suppl. S1), 164–166. [Google Scholar]
- Huang, J.Z.; Liu, J.Z.X.; Guo, K.; Wu, C.; Yang, S.; Luo, M.X.; Lu, Y.N. Numerical Simulation Study on the Impact of Deep Foundation Pit Excavation on Adjacent Rail Transit Structures—A Case Study. Buildings 2024, 14, 1853. [Google Scholar] [CrossRef]
- Ding, Z.; Zhang, X.; Yin, X.S.; Jiang, J.Q. Analysis of the influence of soft soil grouting on the metro tunnel based on field measurement. Eng. Comput. 2019, 36, 1522–1541. [Google Scholar]
- Sun, H.S.; Chen, Y.D.; Zhang, J.H.; Kuang, T.S. Analytical investigation of tunnel deformation caused by circular foundation pit excavation. Comput. Geotech. 2019, 106, 193–198. [Google Scholar]
- Liang, R.Z.; Wu, W.B.; Yu, F.; Jiang, G.S.; Liu, J.W. Simplified method for evaluating shield tunnel deformation due to adjacent excavation. Tunn. Undergr. Space Technol. 2018, 71, 94–105. [Google Scholar]
- Liu, J.W.; Shi, C.H.; Lei, M.F.; Cao, C.Y.; Lin, Y.X. Improved analytical method for evaluating the responses of a shield tunnel to adjacent excavations and its application. Tunn. Undergr. Space Technol. 2020, 98, 103339. [Google Scholar]
- Wang, L.; Zheng, G.; Ou, R.N. Effect of jet grouting and dewatering on an adjacent tunnel in a Shanghai deep excavation. Proc. Inst. Civ. Eng.-Ground Improv. 2019, 172, 274–284. [Google Scholar]
- Wang, L.; Xu, P.; Ma, Z.S.; Yang, R.S.; Liu, P.F. Analysis of the Impact of Foundation Pit Construction on the Deformation of Underlying Metro Tunnels. Transp. Res. Rec. 2024. [Google Scholar] [CrossRef]
- Ding, H.B.; Wang, X.P.; Tong, L.H.; Lu, Q.; Xu, C.J.; Guang, L.X. Performance of isolation piles in protecting subway tunnels adjacent to foundation pits: Experimental and numerical investigations. Tunn. Undergr. Space Technol. 2025, 156, 106221. [Google Scholar]
- He, J.R.; Cheng, X.S.; Zhang, G.W. Model test of foundation pit excavation adjacent to the existing double-lane tunnel. Eng. Res. Express 2024, 6, 045110. [Google Scholar]
- Xu, T.; Zhao, D.Y.; Tao, H.W.; Lei, P. Extended CFD models for numerical simulation of tunnel fire under natural ventilation: Comparative analysis and experimental verification. Case Stud. Therm. Eng. 2022, 31, 101815. [Google Scholar]
- Liu, Z.; Wang, X.L.; Cheng, Z.F.; Sun, R.R.; Zharig, A.L. Simulation of construction ventilation in deep diversion tunnels using Euler-Lagrange method. Comput. Fluids 2014, 105, 28–38. [Google Scholar]
- Zhang, Z.G.; Huang, M.S.; Wang, W.D. Evaluation of deformation response for adjacent tunnels due to soil unloading in excavation engineering. Tunn. Undergr. Space Technol. 2013, 38, 244–253. [Google Scholar]
- Liang, R.Z. Simplified analytical method for evaluating the effects of overcrossing tunnelling on existing shield tunnels using the nonlinear Pasternak foundation model. Soils Found. 2019, 59, 1711–1727. [Google Scholar]
- Liang, R.Z.; Xia, T.D.; Huang, M.S.; Lin, C.G. Simplified analytical method for evaluating the effects of adjacent excavation on shield tunnel considering the shearing effect. Comput. Geotech. 2017, 81, 167–187. [Google Scholar]
- Timoshenko, S.P. On the correction for shear of the differential equation for transverse vibrations of prismatic bars. Philos. Mag. 1921, 41, 744–746. [Google Scholar]
- Wei, X.J.; Ma, J.H.; Wang, X.; Yan, Z.H.; Yan, J.J.; Wei, G. Evaluation of the Additional Stress on Adjacent Tunnel Shafts Induced by Foundation Pit Excavation. Adv. Civ. Eng. 2024, 2024, 890483. [Google Scholar]
- Zhang, X.H.; Wei, G.; Lin, X.B.; Xia, C.; Wei, X.J. Transverse Force Analysis of Adjacent Shield Tunnel Caused by Foundation Pit Excavation Considering Deformation of Retaining Structures. Symmetry 2021, 13, 1478. [Google Scholar] [CrossRef]
- Liu, X.Q.; Liang, F.Y.; Zhang, H.; Chu, F. Energy variational solution for settlement of buried pipeline induced by tunneling. Rock Soil Mech. 2014, 35 (Suppl. S2), 217–222+231. [Google Scholar]
- Shen, S.L.; Wu, H.N.; Cui, Y.J.; Yin, Z.Y. Long-term settlement behaviour of metro tunnels in the soft deposits of Shanghai. Tunn. Undergr. Space Technol. 2014, 40, 309–323. [Google Scholar]
- Zhang, J.F.; Chen, J.J.; Wang, J.H.; Zhu, Y.F. Prediction of tunnel displacement induced by adjacent excavation in soft soil. Tunn. Undergr. Space Technol. 2013, 36, 24–33. [Google Scholar]
- Liu, G.B.; Hou, X.Y. Residual stress analysis of uplift deformation due to soft soil excavation. Undergr. Eng. Tunn. 1996, (02), 2–7. [Google Scholar]
- Mindlin, R.D. Force at a Point in the Interior of a Semi-Infinite Solid. Physics 1936, 7, 195–202. [Google Scholar] [CrossRef]
- Tong, X.; Yuan, J.; Jiang, Y.X.; Liu, X.W.; Li, Y. Calculation of layered unloading additional stress of foundation pit based on Mindlin solution and the analysis of multiple factors influencing the rebound deformation. Rock Soil Mech. 2020, 41, 2432–2440. [Google Scholar]
- Zhang, X.H. Study on the Influence of Foundation Pit Excavation on the Stress and Deformation of Shield Tunnel Structure. Master’s Thesis, Zhejiang University, Hangzhou, China, 2022. [Google Scholar]
- Zhou, S.H.; He, C.; Xiao, J. Energy Method for Calculating Deformation of Adjacent Shield Tunnels due to Foundation Pit Excavation Considering Step between Rings. China Railw. Sci. 2016, 37, 53–60. [Google Scholar]
- Wang, R.L. Factors influencing deformation of Shanghai soft soil metro tunnel and deformation analysis. Undergr. Eng. Tunn. 2009, 1–6+52. [Google Scholar]
- Guo, L.; Yang, X.A.; Qiu, Y. Longitudinal heterogeneous equivalent continuous model for stagger joint segmental lining. Urban Mass Transit 2017, 20, 17–22. [Google Scholar]
- Vesic, A.B. Bending of beams resting on isotropic elastic solids. J. Eng. Mech. 1961, 87, 35–53. [Google Scholar]
- Li, H.R.; Ye, F.; Han, X.B.; Han, X. Comparative Analysis of the Numerical Simulation and Measured Data of an Existing Tunnel Subjected to Multiple Disturbances: A Case Study. Appl. Sci. 2024, 14, 4717. [Google Scholar] [CrossRef]
- Zhang, Z.G.; Cheng, Z.X.; Zhang, M.X. Dislocation deformation for existing tunnel longitudinal structure induced by shield tunneling by under-crossing type considering influence of lining permeability. China J. Highw. Transp. 2022, 35, 180–194. [Google Scholar]
- Ying, H.W.; Cheng, K.; Yu, J.L.; Xu, R.Q.; Qiu, Z.J.; Zhan, X.B.; Qing, J.S.; Lou, C.H. Prediction of shield tunnel displacement due to adjacent basement excavation considering continues deformation of ground. J. Zhejiang Univ. 2021, 55, 318–329. [Google Scholar]






| Name of Soil Layer | Depth of Soil Layer/m | Unit Weight/kN·m−3 | Constrained Modulus/MPa |
|---|---|---|---|
| fill | 6 | ||
| silty clay | 2.5 | 18.4 | 6.04 |
| clay and silty soil | 4.5 | 17.7 | 3.44 |
| muddy clay | 9 | 16.9 | 2.41 |
| clay | 10 | 17.8 | 3.85 |
| silty clay | 16 | 17.9 | 4.94 |
| sandy silt | 5 | 18.7 | 10.30 |
| Name of Soil Layer | Depth of Layer/m | Unit Weight/kN·m−3 | Constrained Modulus/MPa |
|---|---|---|---|
| fill | 1.82 | 18.5 | 3 |
| brownish yellow silty clay | 1.13 | 18.4 | 6.43 |
| grayish yellow silty soil | 0.82 | 17.7 | 3.71 |
| gray mucky silty clay | 1.08 | 17.7 | 4.43 |
| gray sandy silt | 2.28 | 17.2 | 9.72 |
| gray mucky silty clay | 2.46 | 16.6 | 3.36 |
| gray muddy clay | 8.70 | 17.9 | 2.27 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, Y.; Wu, D.; Cheng, P.; Chen, M. A Study on the Longitudinal Deformation of the Underlying Oblique Crossing Tunnel Induced by Foundation Excavation. Buildings 2025, 15, 1194. https://doi.org/10.3390/buildings15071194
Tang Y, Wu D, Cheng P, Chen M. A Study on the Longitudinal Deformation of the Underlying Oblique Crossing Tunnel Induced by Foundation Excavation. Buildings. 2025; 15(7):1194. https://doi.org/10.3390/buildings15071194
Chicago/Turabian StyleTang, Yuhang, Dazhi Wu, Peirui Cheng, and Meiling Chen. 2025. "A Study on the Longitudinal Deformation of the Underlying Oblique Crossing Tunnel Induced by Foundation Excavation" Buildings 15, no. 7: 1194. https://doi.org/10.3390/buildings15071194
APA StyleTang, Y., Wu, D., Cheng, P., & Chen, M. (2025). A Study on the Longitudinal Deformation of the Underlying Oblique Crossing Tunnel Induced by Foundation Excavation. Buildings, 15(7), 1194. https://doi.org/10.3390/buildings15071194






