Research on the Reinforcement Design of Concrete Deep Beams with Openings Based on the Strut-and-Tie Model
Abstract
:1. Introduction
2. Construction of the STM
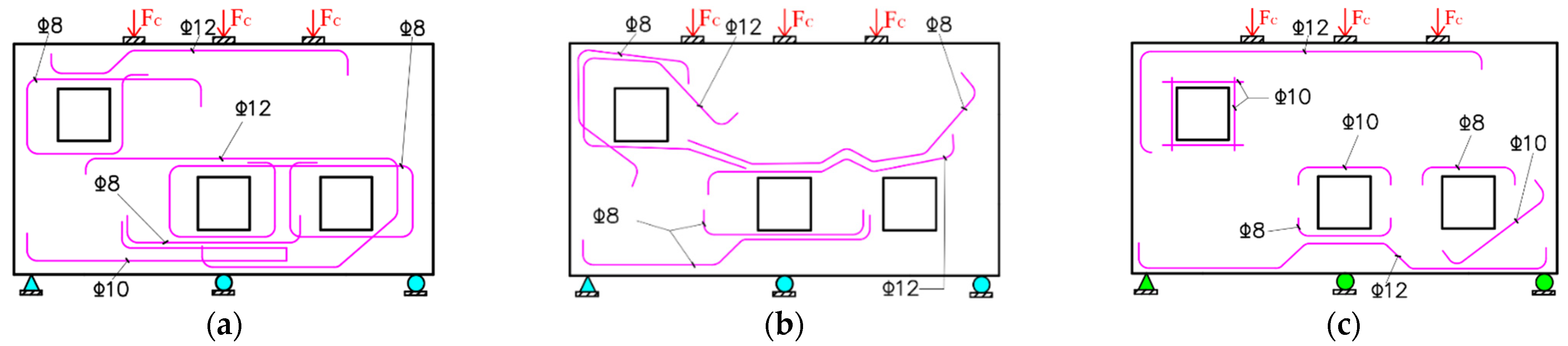
2.1. Specimen Design
2.2. Topology Optimization and Initial STM
2.3. Crack Propagation Simulation and Optimization of the STM
2.3.1. Concrete Crack Propagation Simulation
2.3.2. Optimization of STM
2.4. The STMs in the Existing Literature
3. Experimental Program
3.1. Specimen Production and Loading Scheme
3.2. Material Performance Test
4. Test Results and Analysis
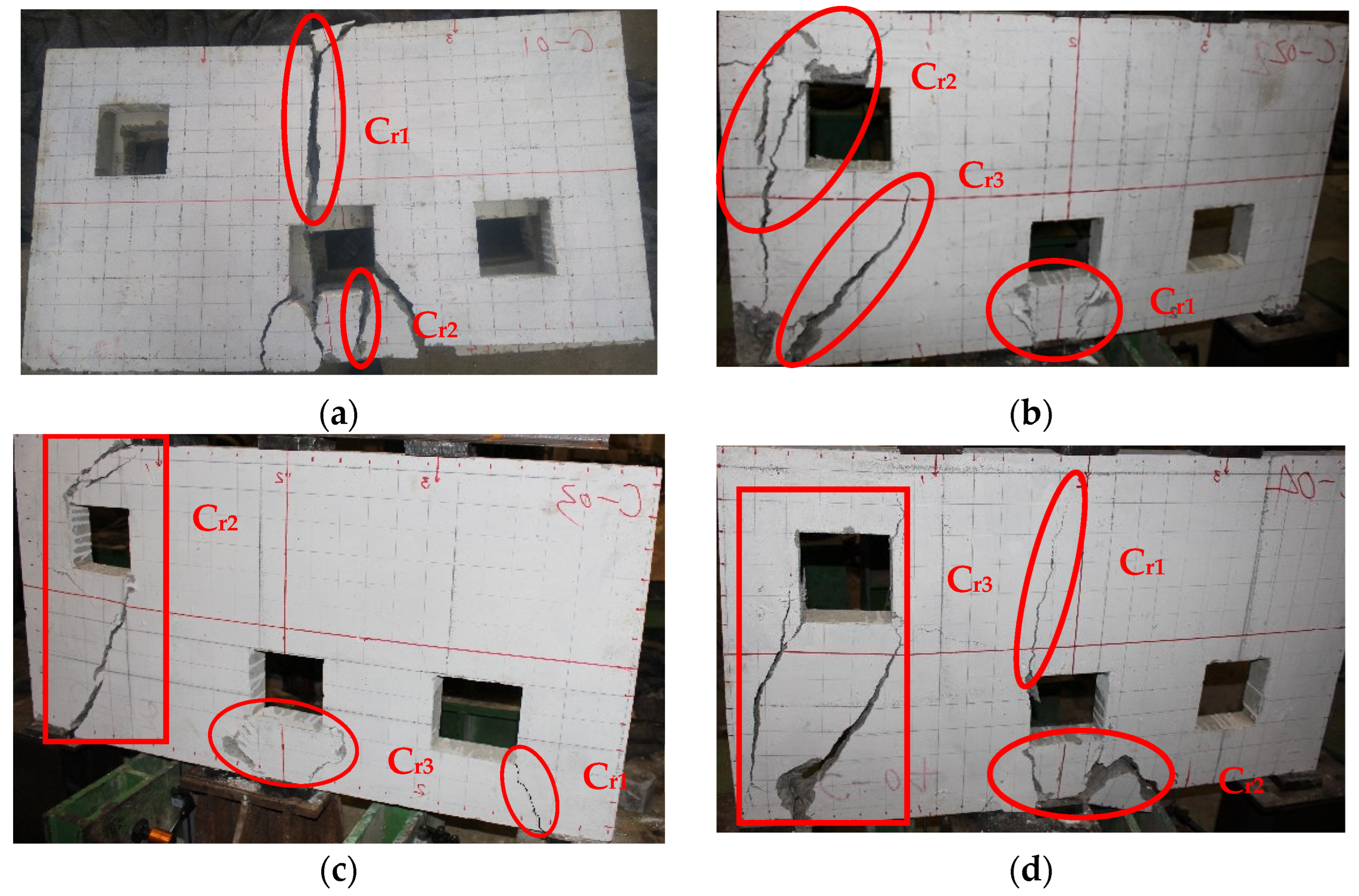
4.1. Failure Morphology
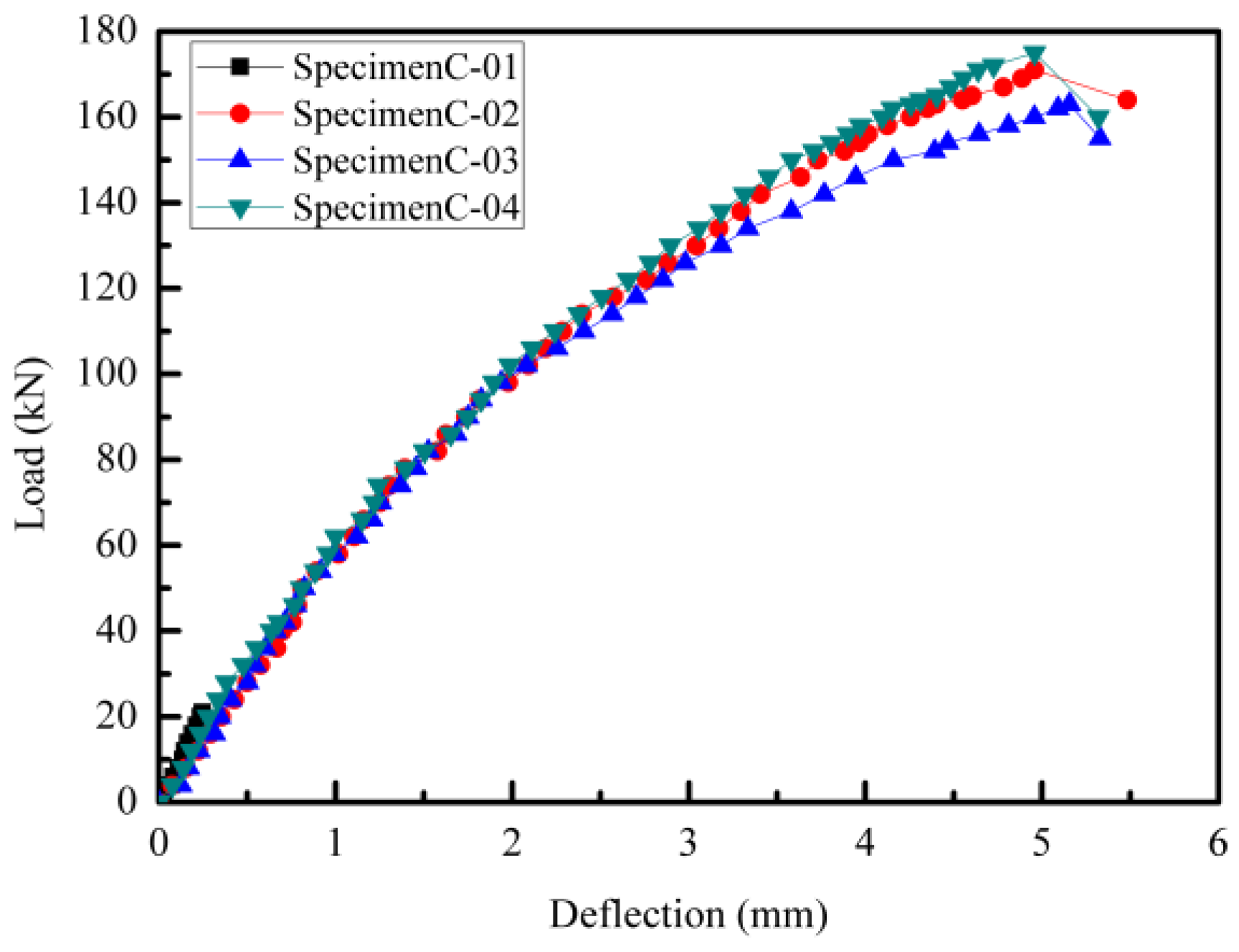
4.2. Performance of the Specimens
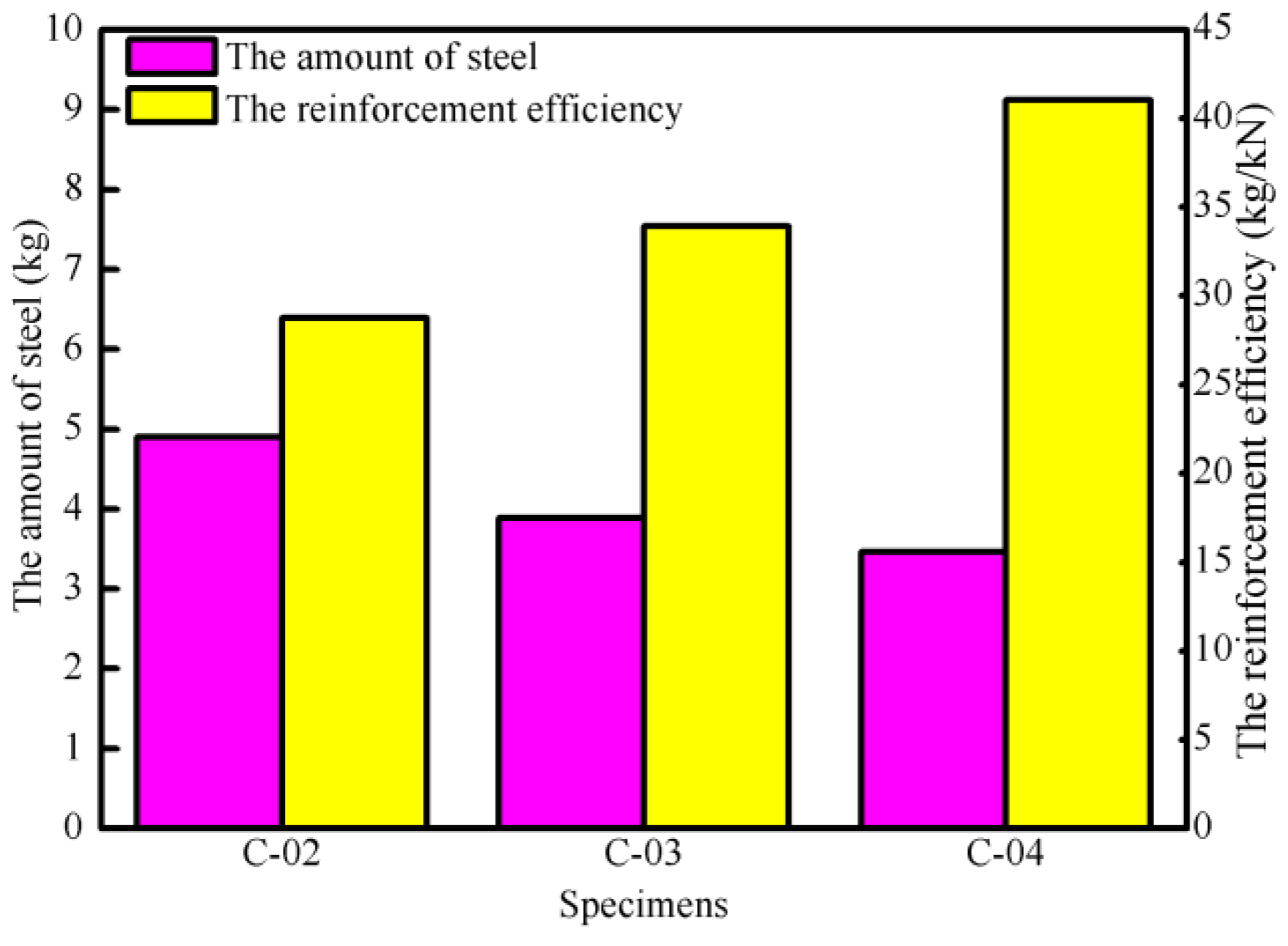
4.3. Steel Consumption and Reinforcement Efficiency
5. Conclusions
- (1)
- The design method based on the optimized STM is suitable for the reinforcement design of the concrete deep beam with openings and has good reliability in terms of determining the bearing capacity. This concrete deep beam designed by STM C-04 has certain advantages in delaying concrete cracking, improving its bearing capacity and reducing steel consumption.
- (2)
- Compared to the STMs in the existing literature, the concrete deep beam designed with STM C-04 can increase the cracking load by up to 6.8% and the bearing capacity by up to 7.4%, and the corresponding steel consumption can be saved up to 29.4%.
- (3)
- The perforated concrete deep beam designed using the strut-and-tie model (STM-04) developed in this study exhibited superior deformation capacity but faster stiffness degradation prior to concrete cracking. After cracking, its deformation performance and stiffness degradation behavior were essentially identical to those of the perforated deep beams designed with STM-02 and STM-03.
- (4)
- The concrete deep beam designed using the design method of STM C-04 has the highest reinforcement efficiency, which can be up to 42.6% higher than the concrete deep beams designed by the other two STMs. That is to say, the economic rationality of the reinforcement design guided by the STM C-04 is better.
- (5)
- The concrete deep beam with openings using this STM design method is subject to shear failure, which should be considered during subsequent reinforcement design.
- (6)
- This study has limitations regarding specimen size effects, boundary conditions, the shape of the openings, and parametric finite element analysis. Future research will focus on addressing these aspects.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Rabi, M.; Shamass, R.; Cashell, K. Structural performance of stainless steel reinforced concrete members: A review. Constr. Build. Mater. 2022, 325, 126673. [Google Scholar] [CrossRef]
- Aksoylu, C.; Ozkilic, Y.O.; Hakeem, I.Y.; Kalkan, I. Effects of the location and size of web openings on shear behavior of clamped-clamped reinforced concrete beams. Comput. Concr. 2024, 33, 251–264. [Google Scholar] [CrossRef]
- Ring, J.D.; Tanner, J.E. Evaluation of Masonry Beams with Openings and Validation Using a Strut-and-Tie Model. J. Struct. Eng. 2021, 147, 04021195. [Google Scholar] [CrossRef]
- Özkılıç, Y.O.; Aksoylu, C.; Hakeem, I.Y.; Özdöner, N.; Kalkan, I.; Karalar, M.; Stel’makh, S.A.; Shcherban, E.M.; Beskopylny, A.N. Shear and Bending Performances of Reinforced Concrete Beams with Different Sizes of Circular Openings. Buildings 2023, 13, 1989. [Google Scholar] [CrossRef]
- Zaborac, J.; Choi, J.; Bayrak, O. Assessment of Deep Beams with Inadequate Web Reinforcement Using Strut-and-Tie Models. Eng. Struct. 2020, 218, 110832. [Google Scholar] [CrossRef]
- Aksoylu, C.; Özkılıç, Y.O.; Çeledir, E.; Başaran, B.; Arslan, M.H. Experimental and numerical investigation of bending performance of prestressed purlins having different longitudinal web opening. Structures 2024, 60, 105839. [Google Scholar] [CrossRef]
- Aksoylu, C.; Özkılıç, Y.O.; Çeledir, E.; Arslan, M.H. Bending performance of dapped-end beams having web opening: Experimental and numerical investigation. Structures 2023, 48, 736–753. [Google Scholar] [CrossRef]
- Meng, C.; Li, K.; Zhou, Z.; Zhang, H. Simulation study on reinforced concrete deep beams with openings designed using topology optimization—STM method. J. Shaoyang Univ. (Nat. Sci. Ed.) 2024, 21, 38–47. [Google Scholar]
- GB 50010-2010; Ministry of Housing and Urban-Rural Development of the People’s Republic of China. Code for Design of Concrete Structures. China Architecture & Building Press: Beijing, China, 2011.
- Mozaffari, S.; Akbarzadeh, M.; Vogel, T. Graphic statics in a continuum: Strut-and-tie models for reinforced concrete. Comput. Struct. 2020, 240, 106335. [Google Scholar] [CrossRef]
- Chetchotisak, P.; Teerawong, J.; Yindeesuk, S. Modified interactive strut-and-tie modeling of reinforced concrete deep beams and corbels. Structures 2022, 45, 284–298. [Google Scholar] [CrossRef]
- Abbood, I.S. Strut-and-tie model and its applications in reinforced concrete deep beams: A comprehensive review. Case Stud. Constr. Mater. 2023, 19, e02643. [Google Scholar] [CrossRef]
- Jing, Z.-N.; Liu, R.-G.; Xie, G.-H.; Liu, D. Shear Strengthening of Deep T-Section RC Beams with CFRP Bars. Materials 2021, 14, 6103. [Google Scholar] [CrossRef] [PubMed]
- Xia, Y.; Langelaar, M.; Hendriks, M.A. Automated optimization-based generation and quantitative evaluation of Strut-and-Tie models. Comput. Struct. 2020, 238, 106297. [Google Scholar] [CrossRef]
- Yang, X.Y.; Xie, Y.M.; Steven, G.P.; Querin, O.M. Bidirectional Evolutionary Method for Stiffness Optimization. AIAA J. 1999, 37, 1483–1488. [Google Scholar] [CrossRef]
- Xia, Y.; Langelaar, M.; Hendriks, M.A. A critical evaluation of topology optimization results for strut-and-tie modeling of reinforced concrete. Comput. Civ. Infrastruct. Eng. 2020, 35, 850–869. [Google Scholar] [CrossRef]
- El-Zoughiby, M.E.; Amasha, R.E.; Ghaleb, A.A. A unifying strut-and-tie model for conventionally reinforced link beams. Eng. Struct. 2023, 299, 117109. [Google Scholar] [CrossRef]
- Nie, Y.; Zhang, J.; Liu, D.; Lan, T.; Xiao, D.; Xiong, M.; Dong, Z. Strut-and-tie model and reinforcement design method for gusset in the containment vessel. Structures 2024, 65, 106693. [Google Scholar] [CrossRef]
- Abarkan, I.; Rabi, M.; Ferreira, F.P.V.; Shamass, R.; Limbachiya, V.; Jweihan, Y.S.; Santos, L.F.P. Machine learning for optimal design of circular hollow section stainless steel stub columns: A comparative analysis with Eurocode 3 predictions. Eng. Appl. Artif. Intell. 2024, 132, 107952. [Google Scholar] [CrossRef]
- Rabi, M.; Shamass, R.; Cashell, K. Description of the constitutive behaviour of stainless steel reinforcement. Case Stud. Constr. Mater. 2024, 20, e03013. [Google Scholar] [CrossRef]
- Chen, H.; Wang, L.; Zhong, J. Study on an Optimal Strut-And-Tie Model for Concrete Deep Beams. Appl. Sci. 2019, 9, 3637. [Google Scholar] [CrossRef]
- Resmy, V.R.; Rajasekaran, C. Evolutionary Topology Optimization of Structural Concrete Under Various Load Cases. In Advances in Civil Engineering; Singh, R.M., Sudheer, K.P., Kurian, B., Eds.; Lecture Notes in Civil Engineering; Springer: Singapore, 2021; Volume 83, pp. 369–380. ISBN 978-981-15-5643-2. [Google Scholar]
- Rabi, M.; Cashell, K.; Shamass, R. Ultimate behaviour and serviceability analysis of stainless steel reinforced concrete beams. Eng. Struct. 2021, 248, 113259. [Google Scholar] [CrossRef]
- Rabi, M.; Cashell, K.; Shamass, R. Flexural analysis and design of stainless steel reinforced concrete beams. Eng. Struct. 2019, 198, 109432. [Google Scholar] [CrossRef]
- Kumar, R.; Nayak, G.C. Numerical Modeling of Tensile Crack Propagation in Concrete Dams. J. Struct. Eng. 1994, 120, 1053–1074. [Google Scholar] [CrossRef]
- Walraven, J.C.; Reinhardt, H.W. Theory and experiments on the mechanical behavior of cracks in plain and reinforced concrete subjected to shear loading. Heron 1981, 26, 68. [Google Scholar]
- Jayasuriya, A.; Bandelt, M.J.; Adams, M.P. Stochastic Mesoscopic Modeling of Concrete Systems Containing Recycled Concrete Aggregates Using Monte Carlo Methods. ACI Mater. J. 2022, 119, 3–18. [Google Scholar] [CrossRef]
- van Mier, J.G.; van Vliet, M.R.; Wang, T.K. Fracture mechanisms in particle composites: Statistical aspects in lattice type analysis. Mech. Mater. 2002, 34, 705–724. [Google Scholar] [CrossRef]
- Al-Kheetan, M.J.; Jweihan, Y.S.; Rabi, M.; Ghaffar, S.H. Durability Enhancement of Concrete with Recycled Concrete Aggregate: The Role of Nano-ZnO. Buildings 2024, 14, 353. [Google Scholar] [CrossRef]
- Shamass, R.; Limbachiya, V.; Ajibade, O.; Rabi, M.; Lopez, H.U.L.; Zhou, X. Carbonated Aggregates and Basalt Fiber-Reinforced Polymers: Advancing Sustainable Concrete for Structural Use. Buildings 2025, 15, 775. [Google Scholar] [CrossRef]
- Garber, D.B.; Gallardo, J.M.; Huaco, G.D.; Samaras, V.A.; Breen, J.E. Experimental Evaluation of Strut-and-Tie Model of Indeterminate Deep Beam. ACI Struct. J. 2014, 111, 51686738. [Google Scholar] [CrossRef]
- Wight, J.K.; American Concrete Institute (Eds.) Building Code Requirements for Structural Concrete (ACI 318-08) and Commentary (ACI 318R-08): An ACI Standard; ACI: Farmington Hills, MI, USA, 2008; ISBN 978-0-87031-264-9. [Google Scholar]
- Kuchma, D.A.; Tjhin, T.N. CAST (Computer Aided Strut-and-Tie) Design Tool. In Proceedings of the Structures 2001, Washington, DC, USA, 21–23 May 2001; American Society of Civil Engineers: Washington, DC, USA, 2001; pp. 1–7. [Google Scholar]
- GB/T 228.1-2010; Metallic Materials—Tensile Testing—Part 1: Method of Test at Room Temperature. China Architecture & Building Press: Beijing, China, 2010.
- GB/T 50081-2019; Standard for Test Methods of Concrete Physical and Mechanical Properties. China Architecture & Building Press: Beijing, China, 2019.

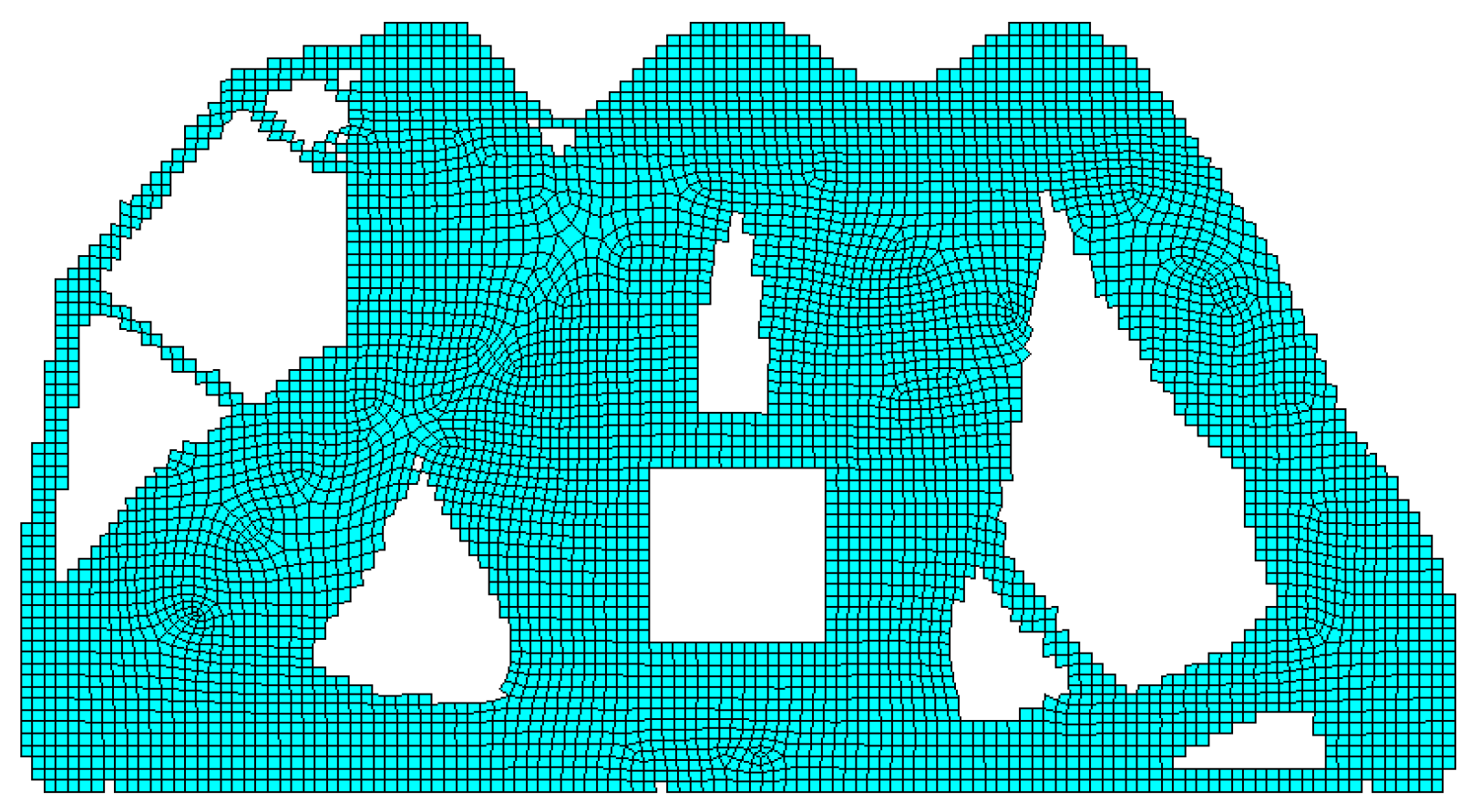

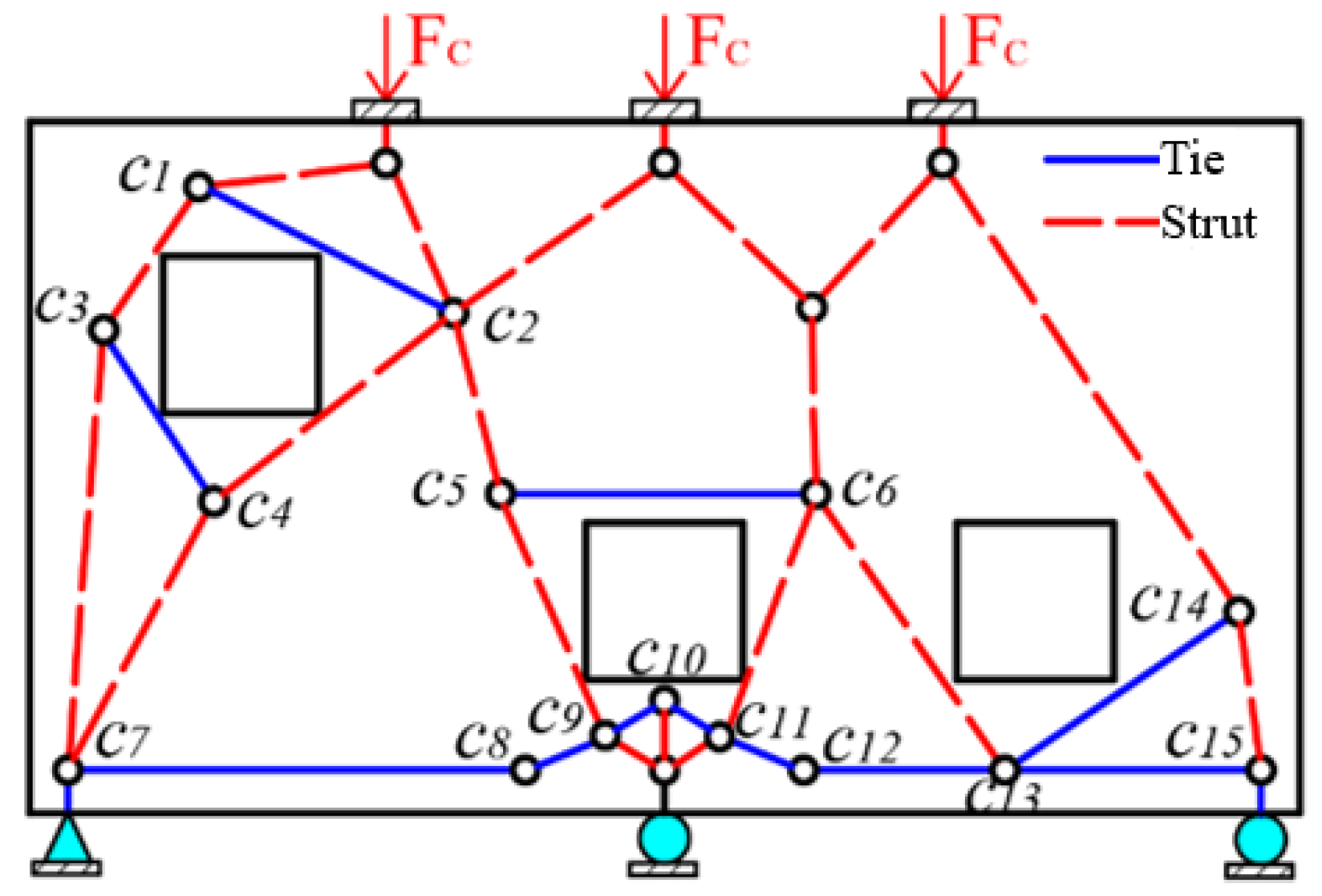



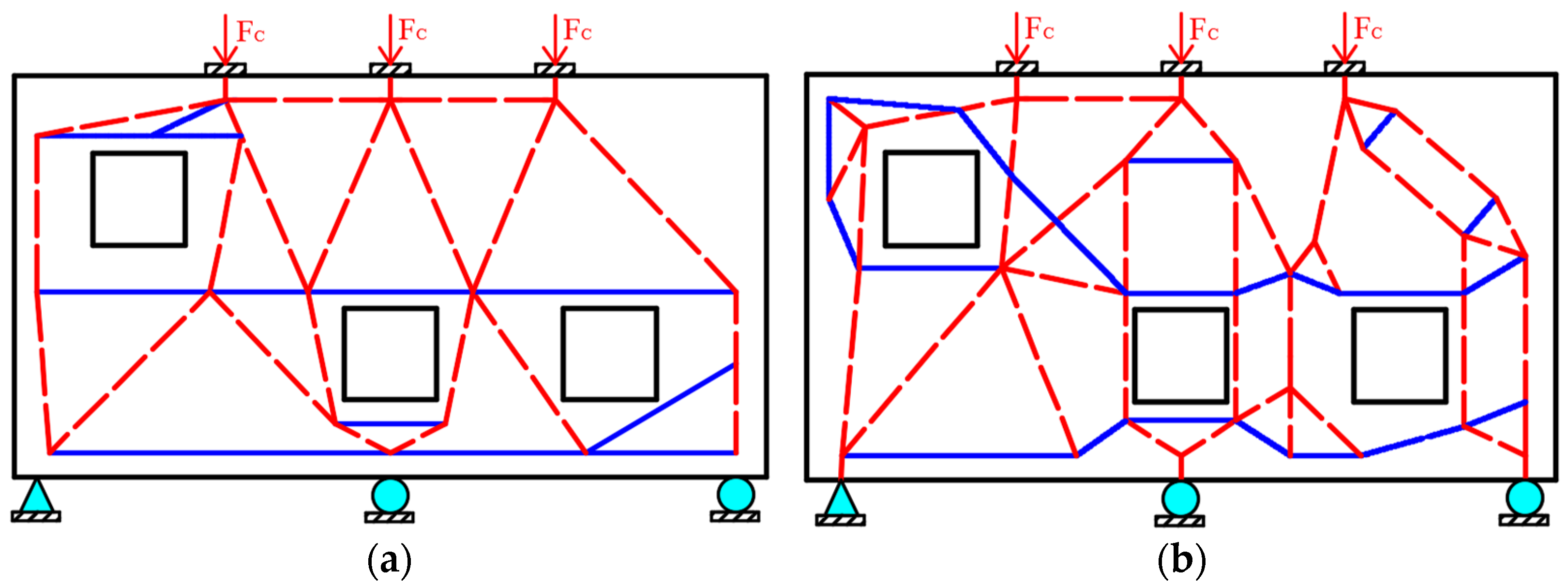


| Filter Radius | Volume Fraction | Evolutionary Rate (ER) | Convergence Tolerance |
|---|---|---|---|
| 3 mm | 0.3 | 1% | 0.1% |
| Diameter (mm) | Yield Strength/fy,m (MPa) | Ultimate Strength/fu,m (MPa) | Yield Strain/εy,m (10−6) |
|---|---|---|---|
| 8 | 482 | 603 | 2101 |
| 10 | 438 | 551 | 1965 |
| 12 | 407 | 512 | 1915 |
| Specimen | Pc (kN) | Pd (kN) | Pu (kN) | Δc (mm) | Δu (mm) | Δu/Δc | Δu/l0 | Pu/Pd |
|---|---|---|---|---|---|---|---|---|
| C-01 | - | 150 | 21 | - | - | - | - | 0.14 |
| C-02 | 105 | 150 | 171 | 2.14 | 4.96 | 2.32 | 0.0043 | 1.14 |
| C-03 | 103 | 150 | 163 | 2.12 | 5.16 | 2.43 | 0.0045 | 1.09 |
| C-04 | 110 | 150 | 175 | 2.24 | 4.96 | 2.21 | 0.0043 | 1.17 |
| Specimen | k0 | Kc/K0 | Ku/K0 |
|---|---|---|---|
| C-02 | 1.00 | 0.67 | 0.36 |
| C-03 | 1.00 | 0.60 | 0.32 |
| C-04 | 1.00 | 0.62 | 0.39 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, H.; Sun, Y.; Deng, M. Research on the Reinforcement Design of Concrete Deep Beams with Openings Based on the Strut-and-Tie Model. Buildings 2025, 15, 1382. https://doi.org/10.3390/buildings15081382
Chen H, Sun Y, Deng M. Research on the Reinforcement Design of Concrete Deep Beams with Openings Based on the Strut-and-Tie Model. Buildings. 2025; 15(8):1382. https://doi.org/10.3390/buildings15081382
Chicago/Turabian StyleChen, Haitao, Yanze Sun, and Meixu Deng. 2025. "Research on the Reinforcement Design of Concrete Deep Beams with Openings Based on the Strut-and-Tie Model" Buildings 15, no. 8: 1382. https://doi.org/10.3390/buildings15081382
APA StyleChen, H., Sun, Y., & Deng, M. (2025). Research on the Reinforcement Design of Concrete Deep Beams with Openings Based on the Strut-and-Tie Model. Buildings, 15(8), 1382. https://doi.org/10.3390/buildings15081382






