A Simple Dynamic Characterization Method for Thin Stacked Dielectric Elastomer Actuators by Suspending a Weight in Air and Electrical Excitation
Abstract
:1. Introduction
2. Thin Stacked Dielectric Elastomer Actuator and Measurement of the Deformation
2.1. Thin Stacked Dielectric Elastomer Actuator
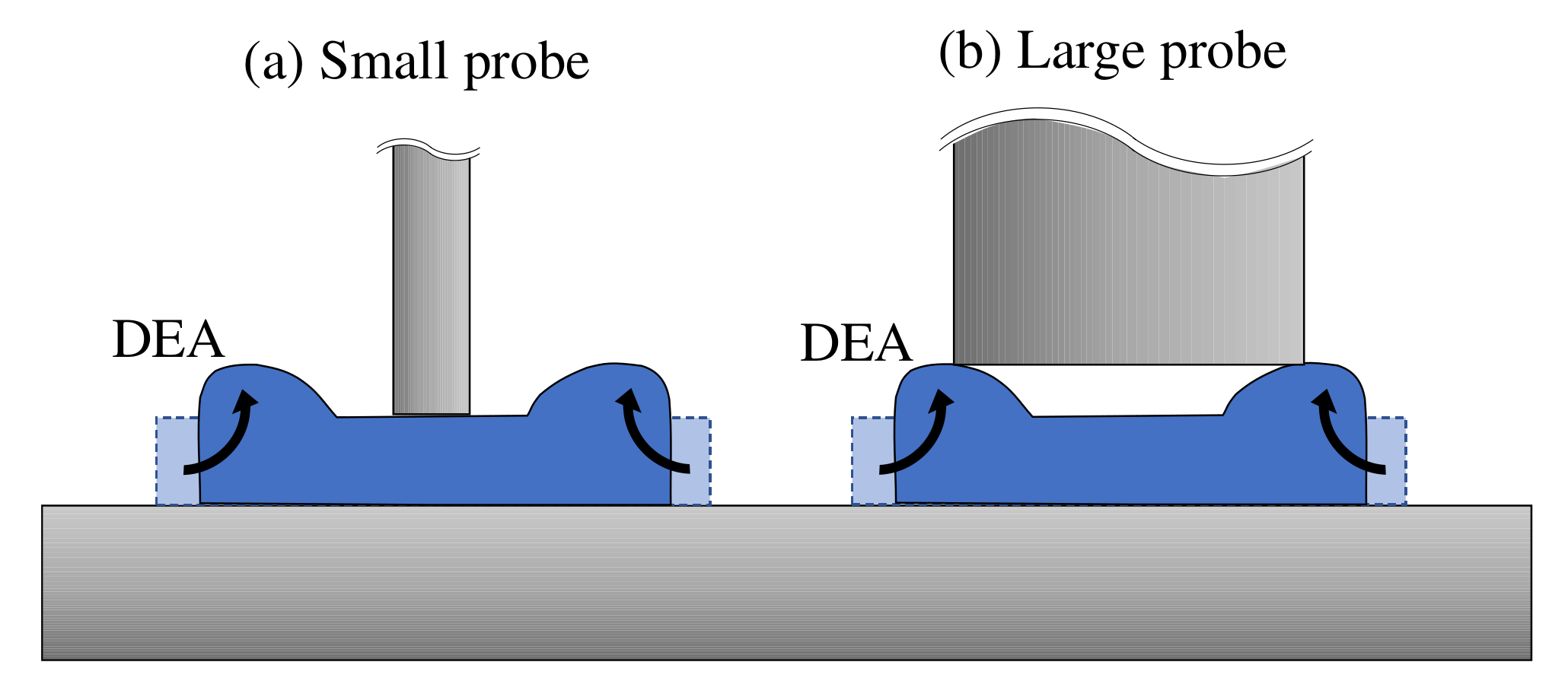
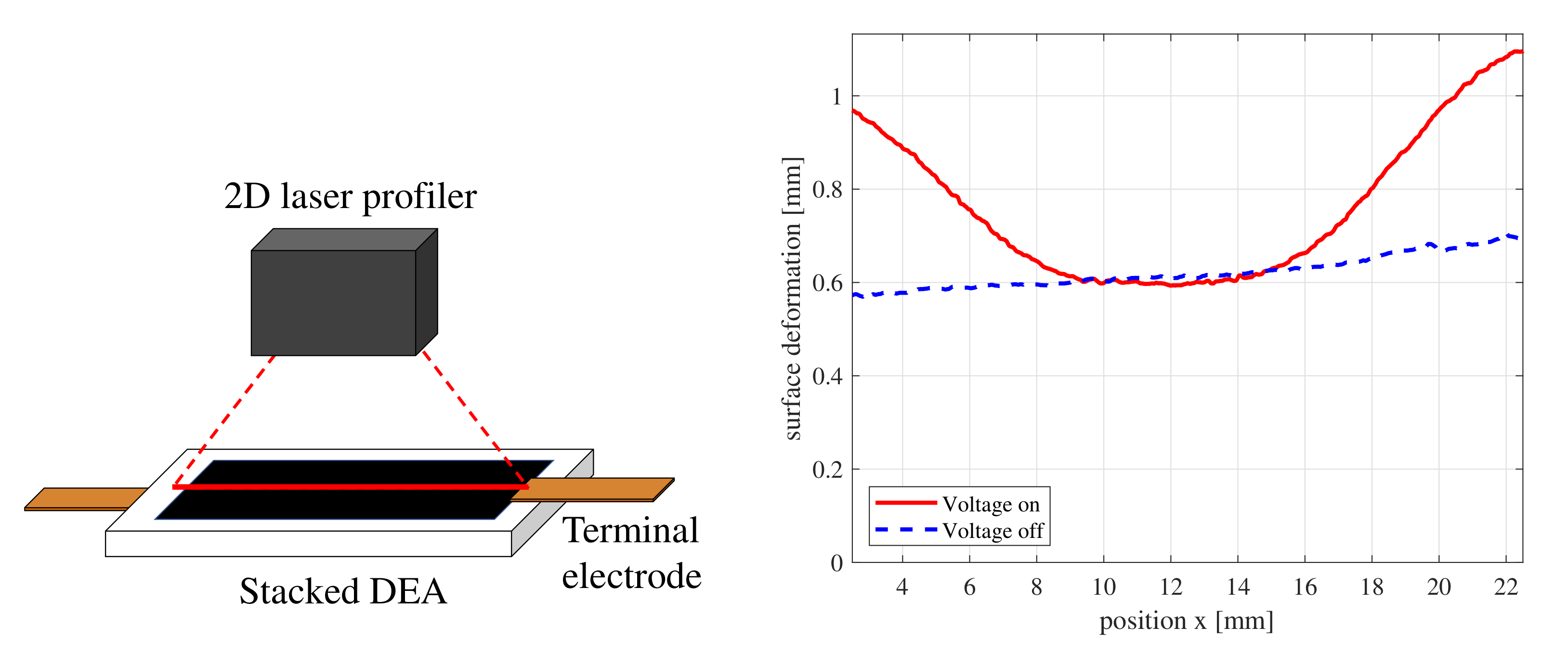
2.2. Preliminary Experiment with a DEA Placed Flat
- A DEA is placed flat on a smooth insulated table (phenol formaldehyde resin, 10 mm thick).
- A voltage of 750 V is applied to the DEA.
- The deformed shape is measured using a two-dimensional laser displacement sensor (Keyence, LJ-X8000).
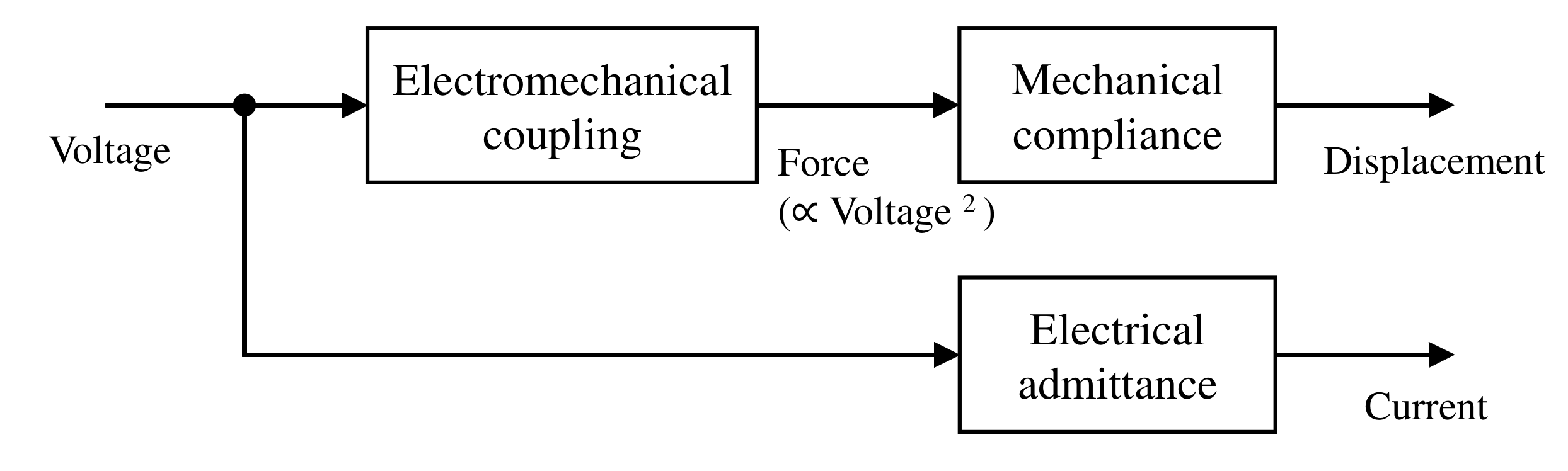
2.3. Proposal of Mechanical Impedance Measurement Using a Weight Suspension
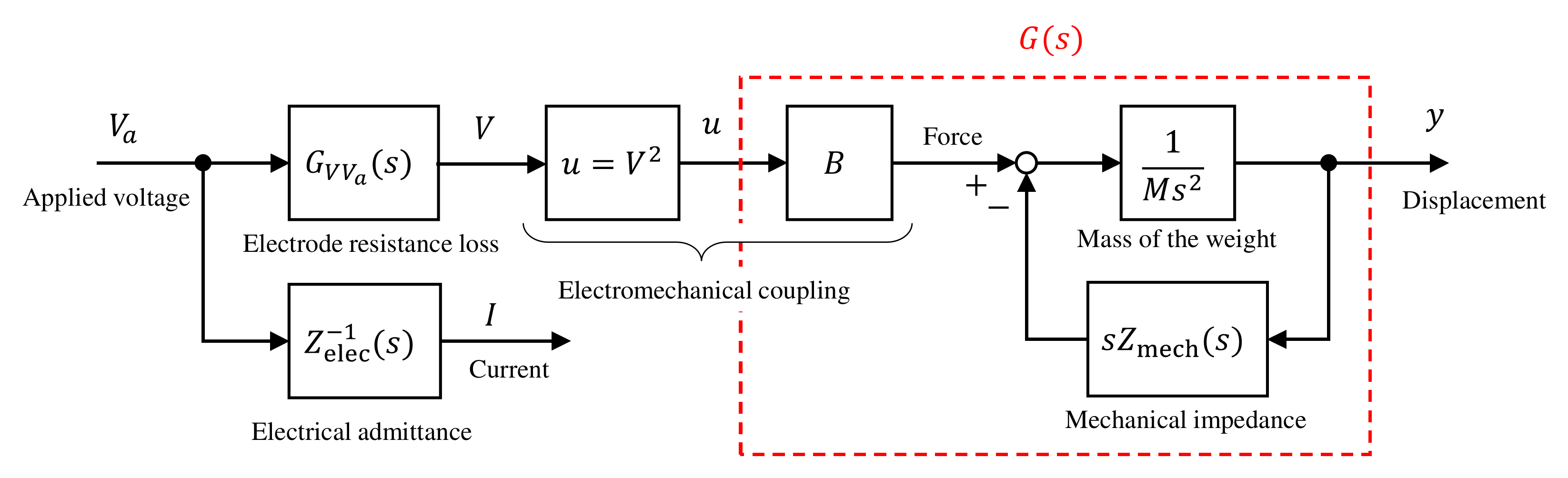
3. Modeling
3.1. Model Structure of a Dielectric Elastomer Actuator
3.2. Mechanical Impedance Model
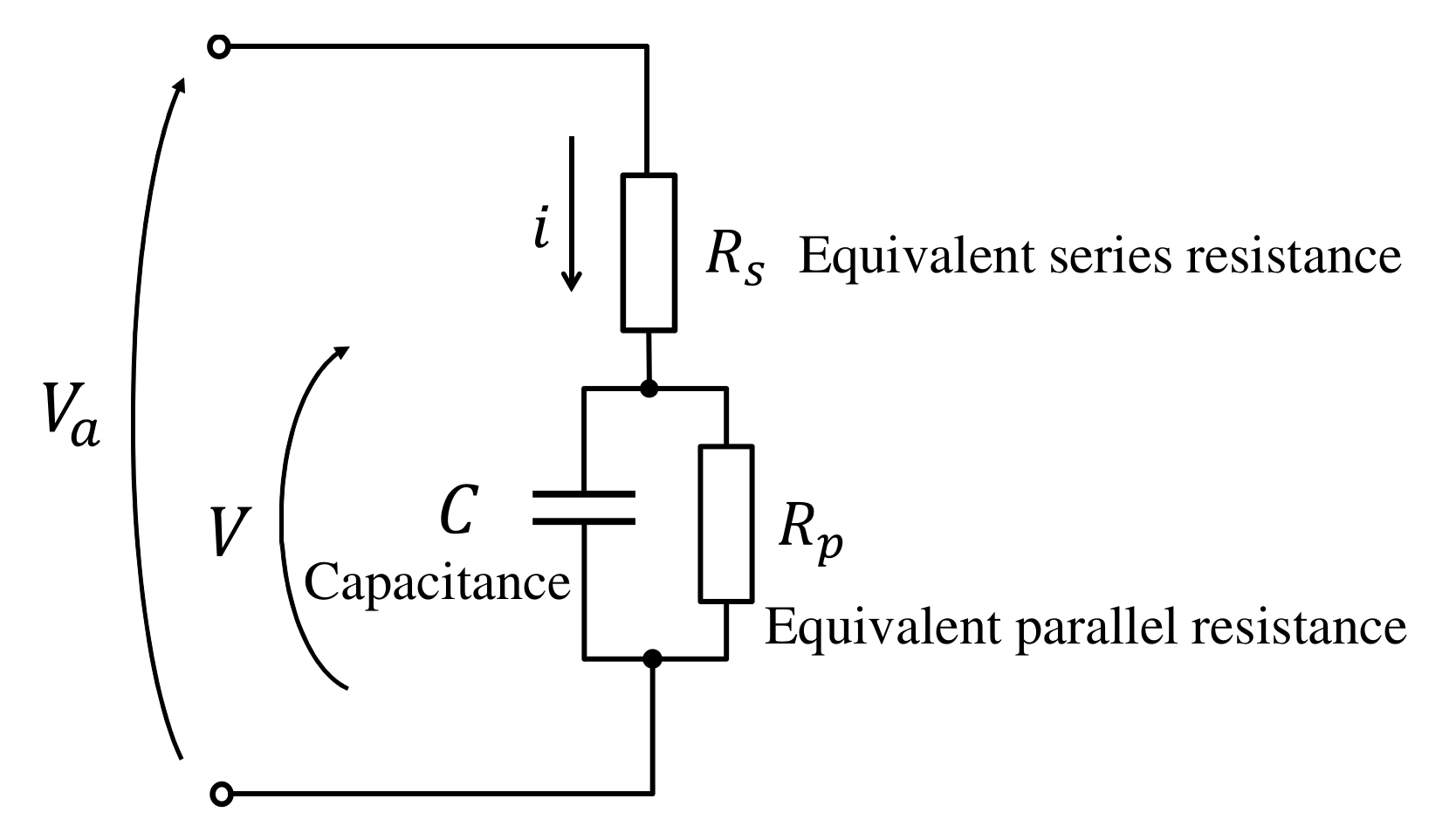
3.3. Electrical Impedance Model
3.4. Electromechanical Coupling Coefficient
3.5. Overall Model
4. Experiment
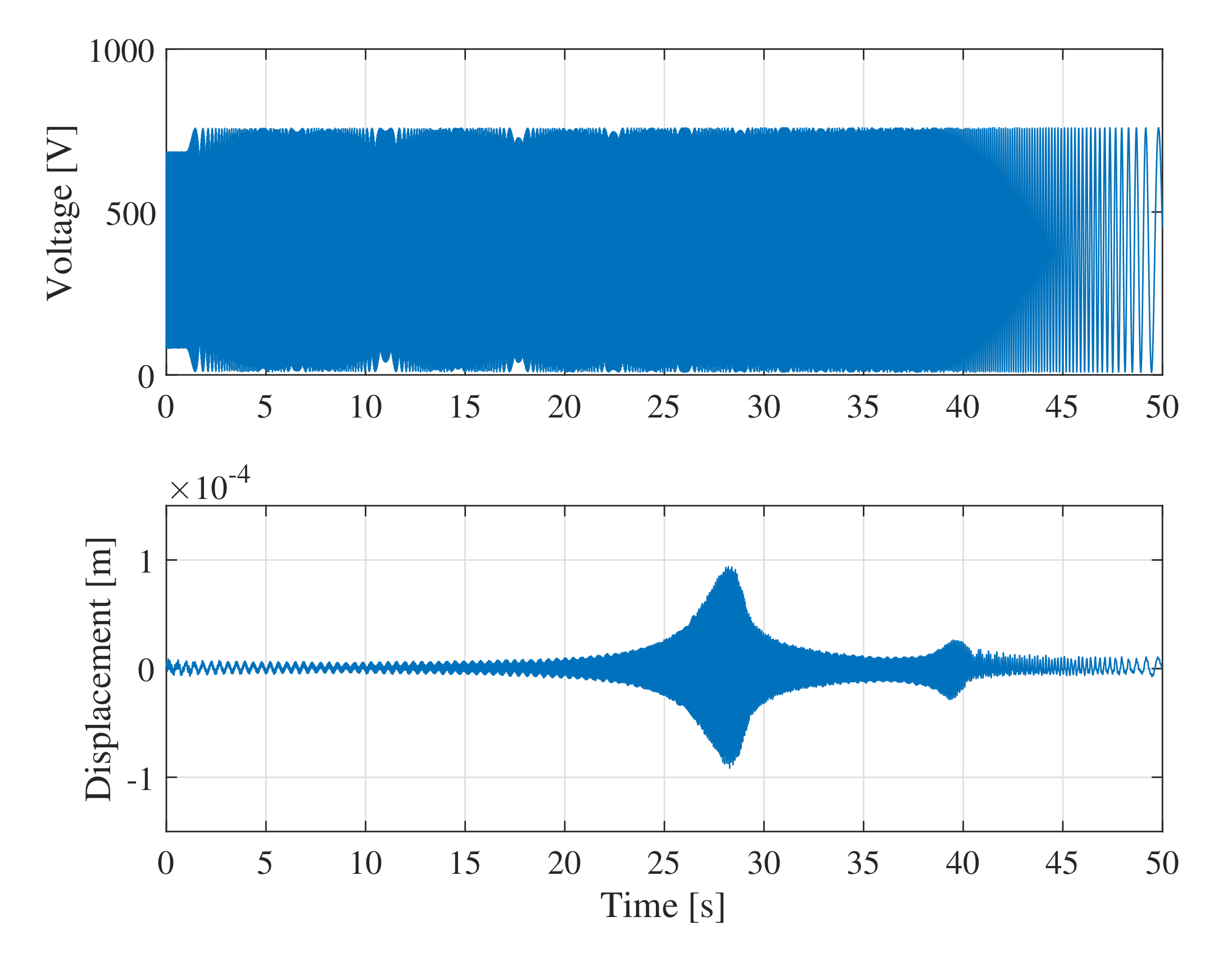
4.1. Experimental Setup and Method
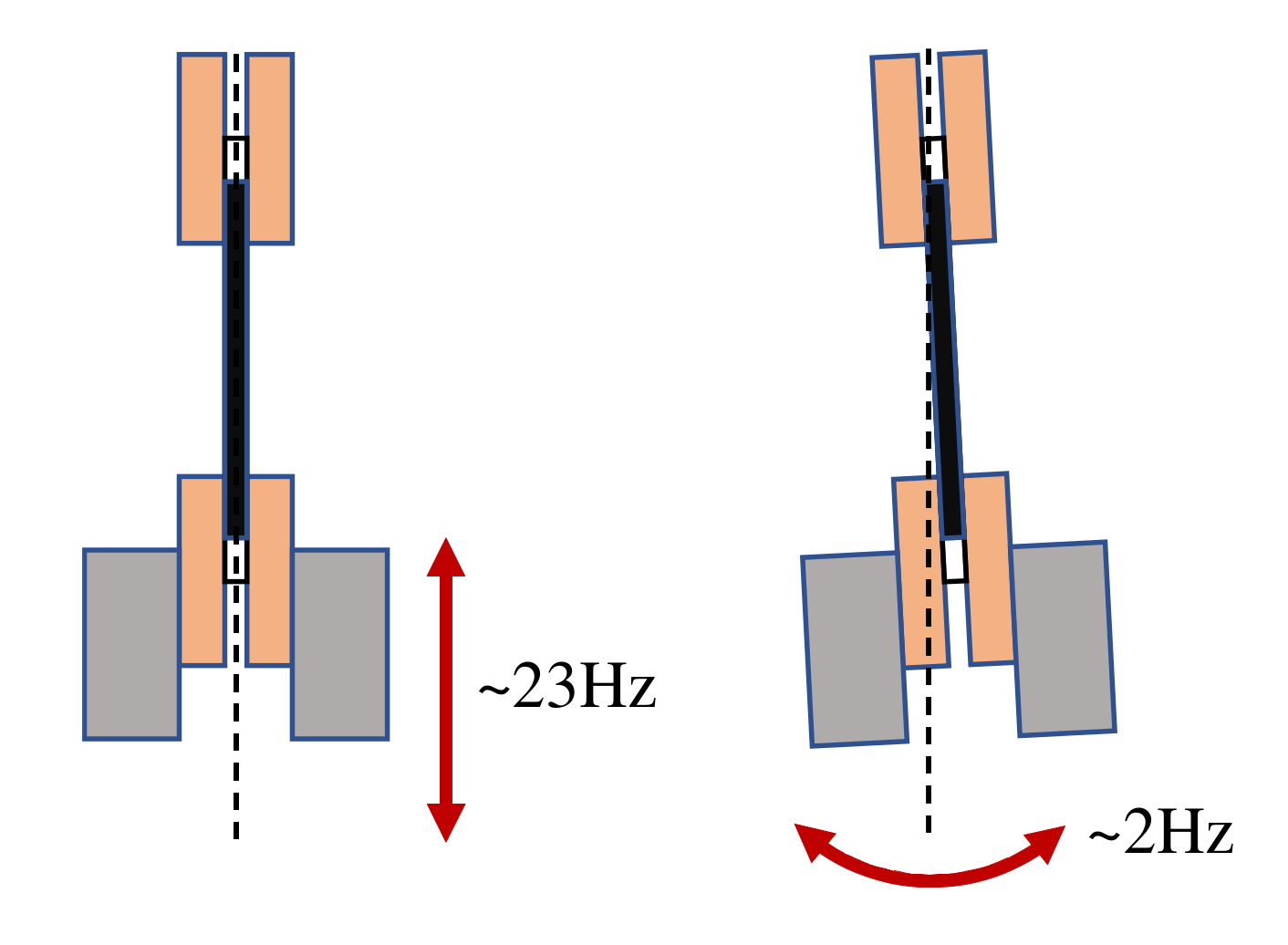
- A weight is attached to the DEA and let hang. Two types of weights ( g or g) are used to investigate the effect of different prestress.
- Swept sinusoidal voltage is applied to the DEA. The applied voltage is 0 V to 750 V, the frequency range is 0.02 to 5000 Hz divided into five, and the swept sine wave is output from a function generator. The divided ranges are shown in Table 2.
- The displacement of the weight is measured and recorded in a data logger together with the voltage and current. Experiments are performed three times for averaging.
- The frequency response is calculated based on the recorded voltage, current, and displacement. The tfestimate function (MATLAB, MathWorks) can estimate the frequency response based on the averaged spectra, and the influence of noise can be reduced. Besides, estimation is performed for each frequency range shown in Table 2, and the estimated frequency responses are concatenated together.
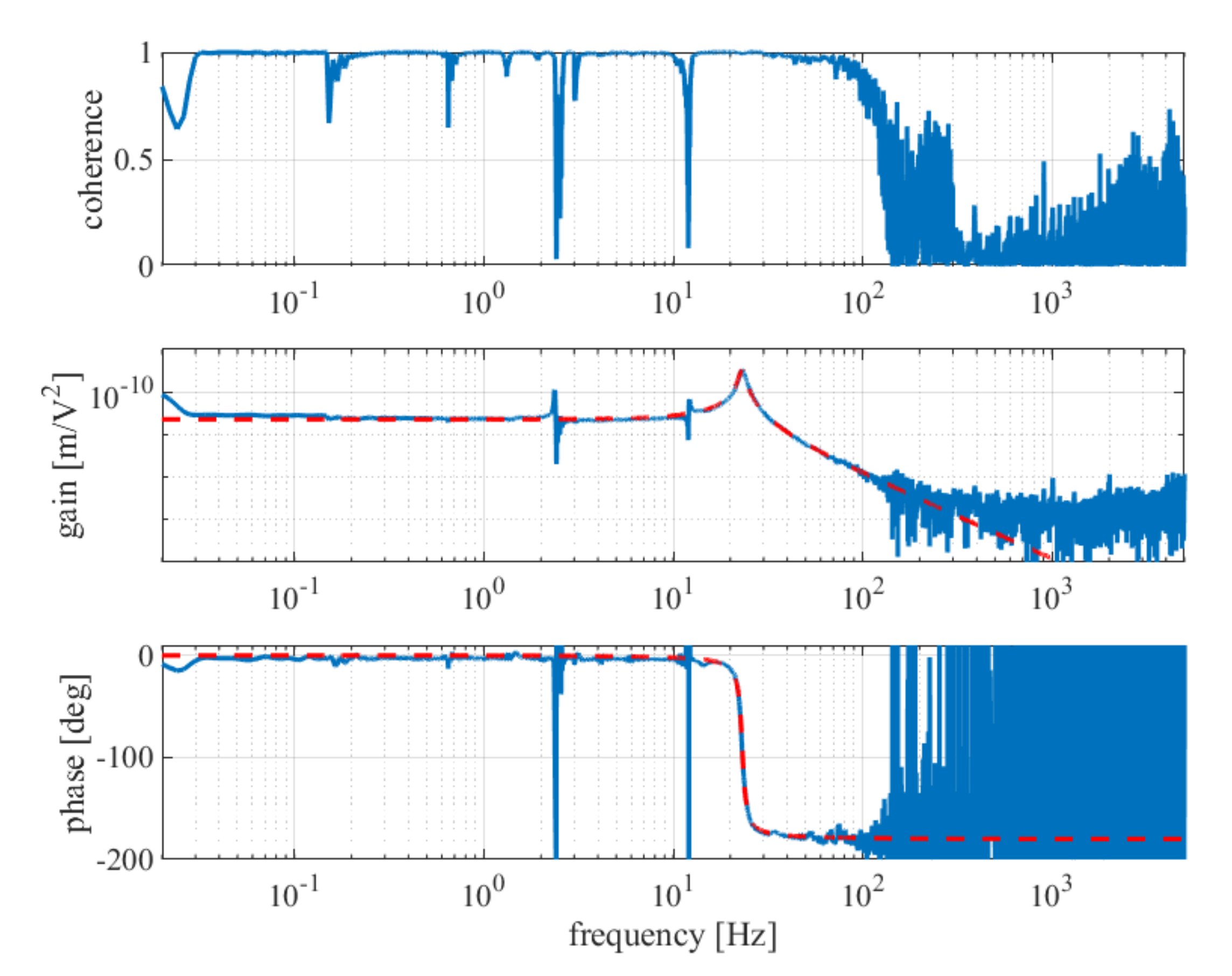
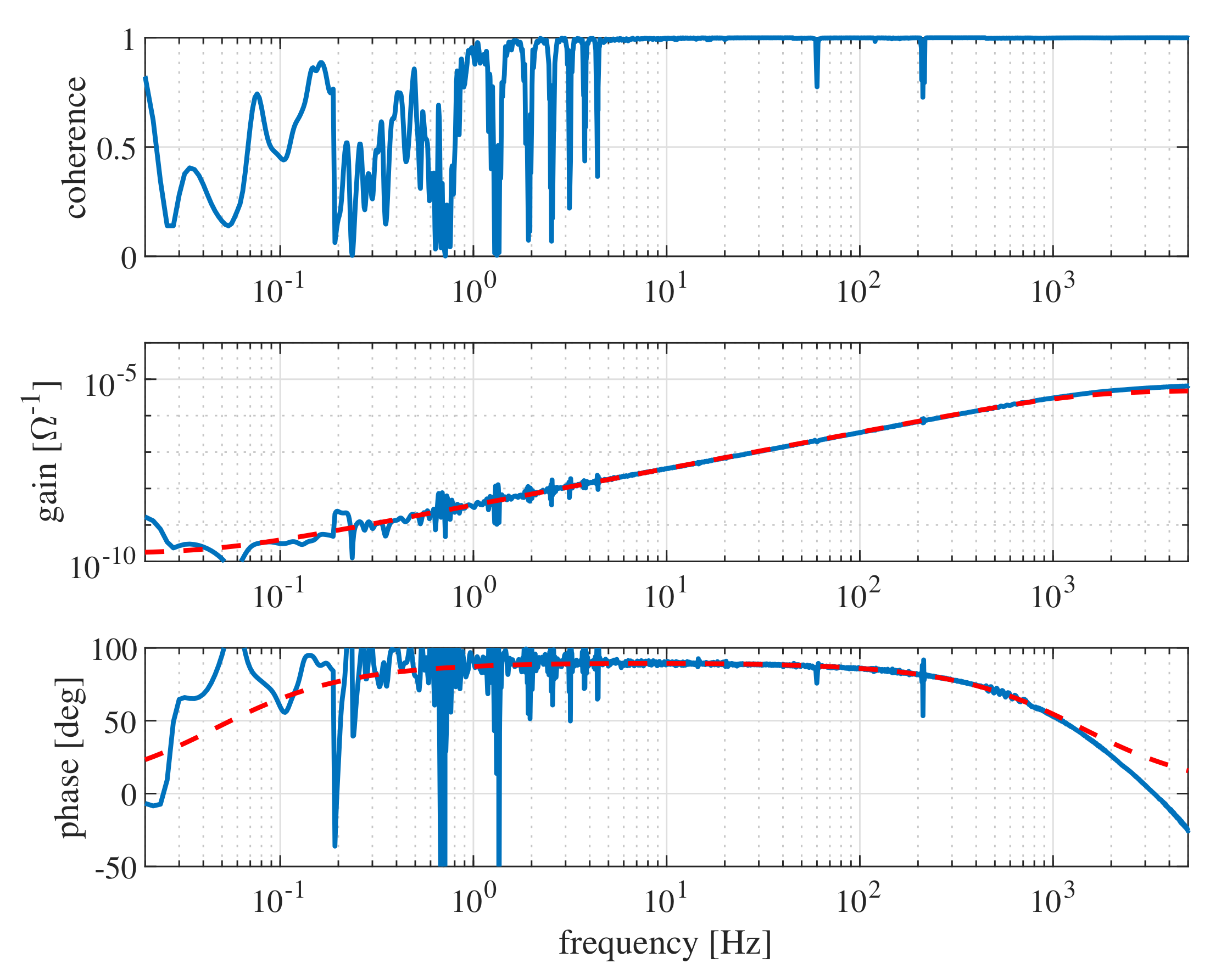
4.2. Results and Discussions: Identification of the Mechanical Model
4.3. Results and Discussions: Identification of the Electrical Model
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| DE | Dielectric elastomer |
| DEA | Dielectric elastomer actuator |
References
- Hirai, T.; Sadatoh, H.; Ueda, T.; Kasazaki, T.; Kurita, Y.; Hirai, M.; Hayashi, S. Polyurethane-elastomer-actuator. Die Angew. Makromol. Chem. Appl. Macromol. Chem. Phys. 1996, 240, 221–229. [Google Scholar] [CrossRef]
- Pelrine, R.E.; Kornbluh, R.D.; Joseph, J.P. Electrostriction of polymer dielectrics with compliant electrodes as a means of actuation. Sens. Actuators A Phys. 1998, 64, 77–85. [Google Scholar] [CrossRef]
- Pelrine, R.; Kornbluh, R.; Pei, Q.; Joseph, J. High-speed electrically actuated elastomers with strain greater than 100%. Science 2000, 287, 836–839. [Google Scholar] [CrossRef]
- Carpi, F. Dielectric Elastomers as Electromechanical Transducers: Fundamentals, Materials, Devices, Models and Applications of an Emerging Electroactive Polymer Technology; Elsevier: Amsterdam, The Netherlands, 2008. [Google Scholar]
- Wingert, A.; Lichter, M.D.; Dubowsky, S.; Hafez, M. Hyper-redundant robot manipulators actuated by optimized binary-dielectric polymers. In Proceedings of the SPIE’s 9th Annual International Symposium on Smart Structures and Materials, San Diego, CA, USA, 17–21 March 2002; Volume 4695, pp. 415–423. [Google Scholar]
- Carpi, F.; Bauer, S.; De Rossi, D. Stretching dielectric elastomer performance. Science 2010, 330, 1759–1761. [Google Scholar] [CrossRef]
- Carpi, F.; Frediani, G.; Turco, S.; De Rossi, D. Bioinspired tunable lens with muscle-like electroactive elastomers. Adv. Funct. Mater. 2011, 21, 4152–4158. [Google Scholar] [CrossRef]
- Koo, I.M.; Jung, K.; Koo, J.C.; Nam, J.D.; Lee, Y.K.; Choi, H.R. Development of soft-actuator-based wearable tactile display. IEEE Trans. Robot. 2008, 24, 549–558. [Google Scholar] [CrossRef]
- Bolzmacher, C.; Hafez, M.; Khoudja, M.B.; Bernardoni, P.; Dubowsky, S. Polymer-based actuators for virtual reality devices. In Proceedings of the Smart Structures and Materials, San Diego, CA, USA, 14–18 March 2004; Volume 5385, pp. 281–289. [Google Scholar]
- Ozsecen, M.Y.; Sivak, M.; Mavroidis, C. Haptic interfaces using dielectric electroactive polymers. In Proceedings of the SPIE Smart Structures and Materials + Nondestructive Evaluation and Health Monitoring, San Diego, CA, USA, 7–11 March 2010; Volume 7647, p. 764737. [Google Scholar]
- Frediani, G.; Mazzei, D.; De Rossi, D.E.; Carpi, F. Wearable wireless tactile display for virtual interactions with soft bodies. Front. Bioeng. Biotechnol. 2014, 2, 31. [Google Scholar] [CrossRef] [Green Version]
- Boys, H.; Frediani, G.; Poslad, S.; Busfield, J.; Carpi, F. A dielectric elastomer actuator-based tactile display for multiple fingertip interaction with virtual soft bodies. In Proceedings of the SPIE Smart Structures and Materials + Nondestructive Evaluation and Health Monitoring, Portland, OR, USA, 25–29 March 2017; Volume 10163, p. 101632D. [Google Scholar]
- Ma, J.; Cheng, X.; Wang, P.; Jiao, Z.; Yu, Y.; Yu, M.; Luo, B.; Yang, W. A Haptic Feedback Actuator Suitable for the Soft Wearable Device. Appl. Sci. 2020, 10, 8827. [Google Scholar] [CrossRef]
- Pyo, D.; Ryu, S.; Kyung, K.U.; Yun, S.; Kwon, D.S. High-pressure endurable flexible tactile actuator based on microstructured dielectric elastomer. Appl. Phys. Lett. 2018, 112, 061902. [Google Scholar] [CrossRef] [Green Version]
- Phung, H.; Nguyen, C.T.; Jung, H.; Nguyen, T.D.; Choi, H.R. Bidirectional tactile display driven by electrostatic dielectric elastomer actuator. Smart Mater. Struct. 2020, 29, 035007. [Google Scholar] [CrossRef]
- Zhao, H.; Hussain, A.M.; Israr, A.; Vogt, D.M.; Duduta, M.; Clarke, D.R.; Wood, R.J. A Wearable Soft Haptic Communicator Based on Dielectric Elastomer Actuators. Soft Robot. 2020, 7, 451–461. [Google Scholar] [CrossRef] [PubMed]
- Ji, X.; Liu, X.; Cacucciolo, V.; Civet, Y.; El Haitami, A.; Cantin, S.; Perriard, Y.; Shea, H. Untethered Feel-Through Haptics Using 18-μm Thick Dielectric Elastomer Actuators. Adv. Funct. Mater. 2020. [Google Scholar] [CrossRef]
- Kovacs, G.; Düring, L.; Michel, S.; Terrasi, G. Stacked dielectric elastomer actuator for tensile force transmission. Sens. Actuators A Phys. 2009, 155, 299–307. [Google Scholar] [CrossRef]
- Biggs, J.; Danielmeier, K.; Hitzbleck, J.; Krause, J.; Kridl, T.; Nowak, S.; Orselli, E.; Quan, X.; Schapeler, D.; Sutherland, W.; et al. Electroactive polymers: Developments of and perspectives for dielectric elastomers. Angew. Chem. Int. Ed. 2013, 52, 9409–9421. [Google Scholar] [CrossRef] [PubMed]
- Matysek, M.; Lotz, P.; Flittner, K.; Schlaak, H.F. High-precision characterization of dielectric elastomer stack actuators and their material parameters. In Proceedings of the SPIE Smart Structures and Materials + Nondestructive Evaluation and Health Monitoring, San Diego, CA, USA, 9–13 March 2008; Volume 6927, p. 692722. [Google Scholar]
- Lotz, P.; Matysek, M.; Schlaak, H.F. Fabrication and application of miniaturized dielectric elastomer stack actuators. IEEE/ASME Trans. Mechatron. 2010, 16, 58–66. [Google Scholar] [CrossRef]
- Kaal, W.; Bartel, T.; Herold, S. Active vibration isolation with a dielectric elastomer stack actuator. Smart Mater. Struct. 2017, 26, 055016. [Google Scholar] [CrossRef]
- Carpi, F.; Anderson, I.; Bauer, S.; Frediani, G.; Gallone, G.; Gei, M.; Graaf, C.; Jean-Mistral, C.; Kaal, W.; Kofod, G.; et al. Standards for dielectric elastomer transducers. Smart Mater. Struct. 2015, 24, 105025. [Google Scholar] [CrossRef] [Green Version]
- Suo, Z. Theory of dielectric elastomers. Acta Mech. Solida Sin. 2010, 23, 549–578. [Google Scholar] [CrossRef]
- Kofod, G.; Sommer-Larsen, P.; Kornbluh, R.; Pelrine, R. Actuation response of polyacrylate dielectric elastomers. J. Intell. Mater. Syst. Struct. 2003, 14, 787–793. [Google Scholar] [CrossRef]
- Lochmatter, P.; Michel, S.; Kovacs, G. Electromechanical model for static and dynamic activation of elementary dielectric elastomer actuators. In Proceedings of the SPIE Smart Structures and Materials + Nondestructive Evaluation and Health Monitoring, San Diego, CA, USA, 26 February–2 March 2006; Volume 6168. [Google Scholar] [CrossRef]
- Schmidt, A.; Bergamini, A.; Jordi, C.; Kovacs, G.; Mazza, E. Electro-mechanical modeling of dielectric elastomer transducers with micro-structured electrodes. In Proceedings of the SPIE Smart Structures and Materials + Nondestructive Evaluation and Health Monitoring, San Diego, CA, USA, 6–10 March 2011; Volume 7976, p. 79760L. [Google Scholar] [CrossRef]
- Sarban, R.; Lassen, B.; Willatzen, M. Dynamic electromechanical modeling of dielectric elastomer actuators with metallic electrodes. IEEE/ASME Trans. Mechatron. 2012, 17, 960–967. [Google Scholar] [CrossRef]
- Haus, H.; Matysek, M.; Mößinger, H.; Schlaak, H.F. Modelling and characterization of dielectric elastomer stack actuators. Smart Mater. Struct. 2013. [Google Scholar] [CrossRef]
- Rizzello, G.; Naso, D.; York, A.; Seelecke, S. Modeling, Identification, and Control of a Dielectric Electro-Active Polymer Positioning System. IEEE Trans. Control. Syst. Technol. 2015, 23, 632–643. [Google Scholar] [CrossRef]
- Hackl, C.M.; Tang, H.Y.; Lorenz, R.D.; Turng, L.S.; Schroder, D. A multidomain model of planar electro-active polymer actuators. IEEE Trans. Ind. Appl. 2005, 41, 1142–1148. [Google Scholar] [CrossRef]
- Choi, H.R.; Jung, K.; Ryew, S.; Nam, J.D.; Jeon, J.; Koo, J.C.; Tanie, K. Biomimetic soft actuator: Design, modeling, control, and applications. IEEE/ASME Trans. Mechatron. 2005, 10, 581–593. [Google Scholar] [CrossRef]
- Wissler, M.; Mazza, E. Electromechanical coupling in dielectric elastomer actuators. Sens. Actuators A Phys. 2007, 138, 384–393. [Google Scholar] [CrossRef]
- Kaal, W.; Herold, S. Electroactive polymer actuators in dynamic applications. IEEE/ASME Trans. Mechatron. 2010, 16, 24–32. [Google Scholar] [CrossRef]
- York, A.; Dunn, J.; Seelecke, S. Experimental characterization of the hysteretic and rate-dependent electromechanical behavior of dielectric electro-active polymer actuators. Smart Mater. Struct. 2010, 19, 094014. [Google Scholar] [CrossRef]
- Chiang Foo, C.; Cai, S.; Jin Adrian Koh, S.; Bauer, S.; Suo, Z. Model of dissipative dielectric elastomers. J. Appl. Phys. 2012, 111, 034102. [Google Scholar] [CrossRef]
- Rizzello, G.; Naso, D.; York, A.; Seelecke, S. A nonlinear electro-mechanical model for an annular dielectric elastomer actuator with a biasing mass. In Proceedings of the VDI Tagung Mechatronik, Aachen, Germany, 6–8 March 2013; pp. 117–122. [Google Scholar]
- Hoffstadt, T.; Maas, J. Analytical modeling and optimization of DEAP-based multilayer stack-transducers. Smart Mater. Struct. 2015, 24, 094001. [Google Scholar] [CrossRef]
- Rizzello, G.; Hodgins, M.; Naso, D.; York, A.; Seelecke, S. Modeling of the effects of the electrical dynamics on the electromechanical response of a DEAP circular actuator with a mass–spring load. Smart Mater. Struct. 2015, 24, 094003. [Google Scholar] [CrossRef]
- Liu, L.; Sun, W.; Sheng, J.; Chang, L.; Li, D.; Chen, H. Effect of temperature on the electromechanical actuation of viscoelastic dielectric elastomers. EPL 2015, 112, 27006. [Google Scholar] [CrossRef]
- Gu, G.Y.; Gupta, U.; Zhu, J.; Zhu, L.M.; Zhu, X.Y. Feedforward deformation control of a dielectric elastomer actuator based on a nonlinear dynamic model. Appl. Phys. Lett. 2015, 107, 042907. [Google Scholar] [CrossRef] [Green Version]
- Rizzello, G.; Naso, D.; Turchiano, B.; Seelecke, S. Robust position control of dielectric elastomer actuators based on LMI optimization. IEEE Trans. Control. Syst. Technol. 2016, 24, 1909–1921. [Google Scholar] [CrossRef]
- Gu, G.Y.; Gupta, U.; Zhu, J.; Zhu, L.M.; Zhu, X. Modeling of viscoelastic electromechanical behavior in a soft dielectric elastomer actuator. IEEE Trans. Robot. 2017, 33, 1263–1271. [Google Scholar] [CrossRef]
- Choi, H.R.; Ryew, S.; Jung, K.M.; Kim, H.; Jeon, J.W.; Nam, J.; Maeda, R.; Tanie, K. Soft actuator for robotic applications based on dielectric elastomer: Quasi-static analysis. In Proceedings of the 2002 IEEE International Conference on Robotics and Automation (ICRA), Washington, DC, USA, 11–15 May 2002; Volume 3, pp. 3212–3217. [Google Scholar]
- Berselli, G.; Vertechy, R.; Babič, M.; Parenti Castelli, V. Dynamic modeling and experimental evaluation of a constant-force dielectric elastomer actuator. J. Intell. Mater. Syst. Struct. 2013, 24, 779–791. [Google Scholar] [CrossRef]
- Wacker Chemie AG. ELASTOSIL® Film 2030 250/50. Available online: https://www.wacker.com/h/en-us/silicone-rubber/silicone-films/elastosil-film-2030/p/000038005 (accessed on 8 December 2020).
- Carpi, F.; Chiarelli, P.; Mazzoldi, A.; De Rossi, D. Electromechanical characterisation of dielectric elastomer planar actuators: Comparative evaluation of different electrode materials and different counterloads. Sens. Actuators A Phys. 2003, 107, 85–95. [Google Scholar] [CrossRef]
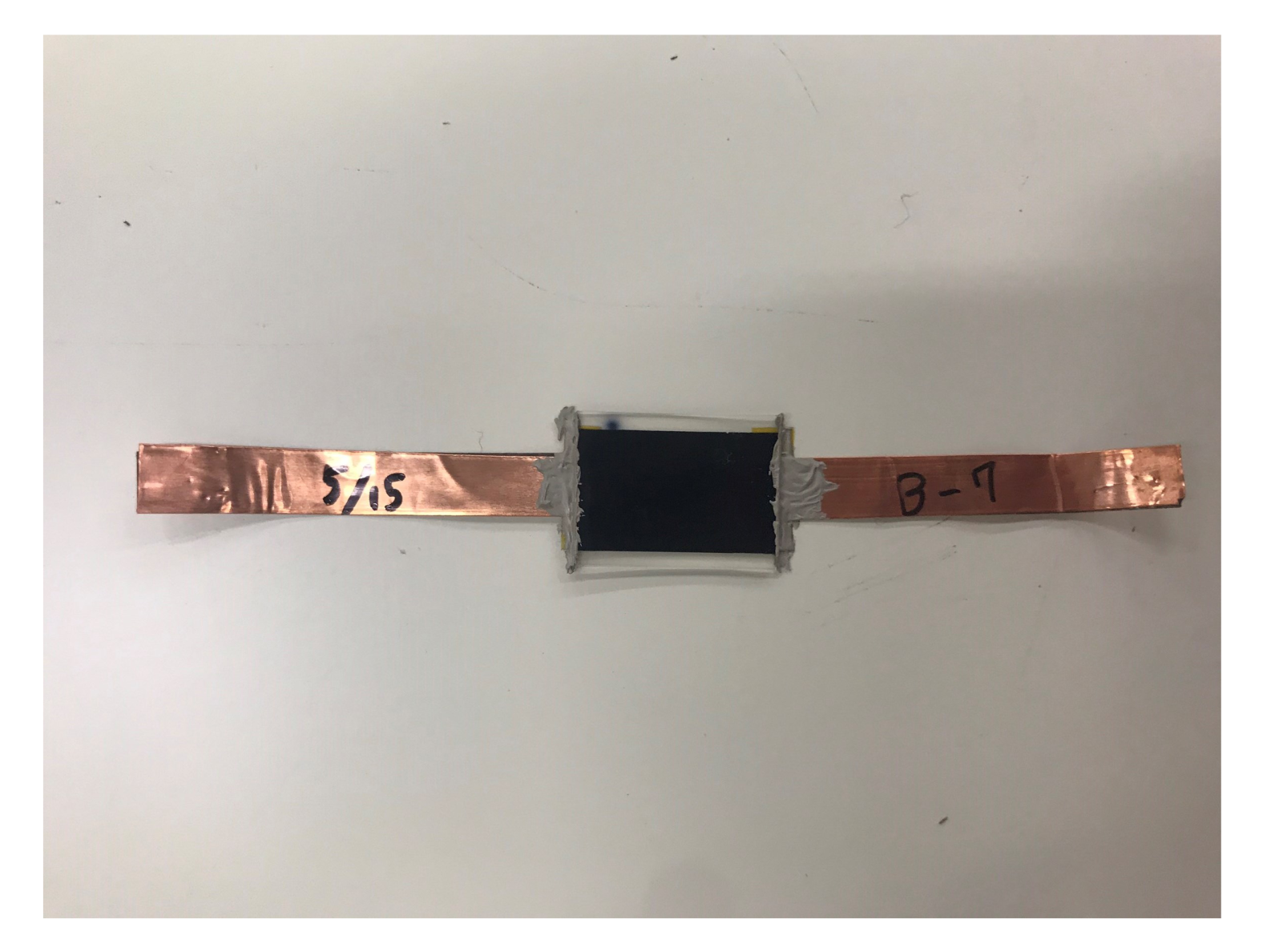
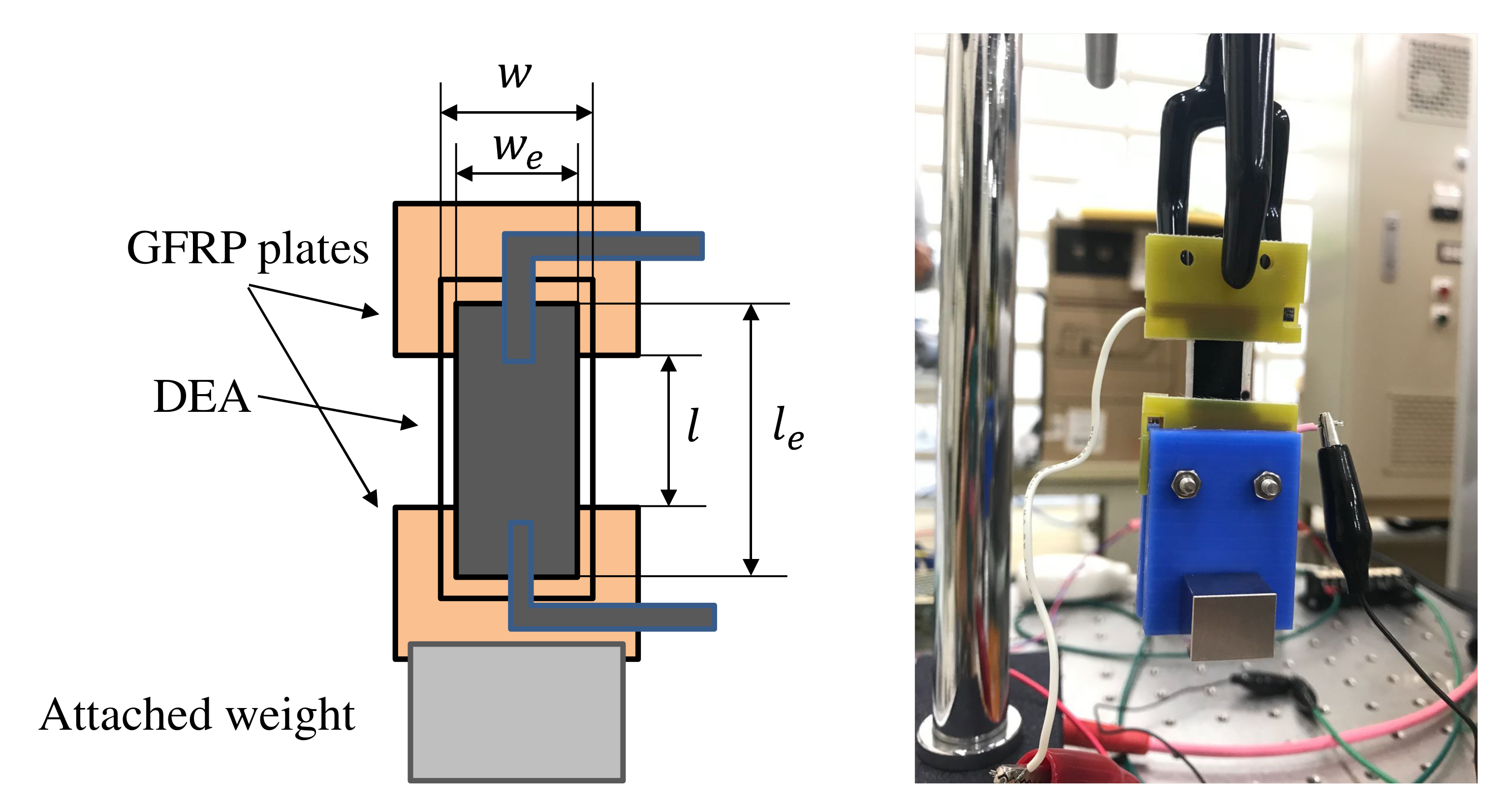


| Deformable length | l | [m] | |
|---|---|---|---|
| Length of the electrode | [m] | ||
| Width | w | [m] | |
| Width of the electrode | [m] | ||
| Total thickness | h | [m] | |
| Thickness of the dielectric | [m] | ||
| Number of the layers | N | - | 10 |
| Input Frequency [Hz] | Sampling Frequency [Hz] | Length of the Record T [s] | |
|---|---|---|---|
| (1) | 0.02 to 0.2 | 20 | 500 |
| (2) | 0.1 to 5 | 20 | 500 |
| (3) | 1 to 50 | 200 | 50 |
| (4) | 10 to 500 | 2000 | 5 |
| (5) | 100 to 5000 | 20,000 | 0.5 |
| Attached mass | M | kg | ||
| (Equivalent prestress) | Pa | |||
| Spring constant | K | [N/m] | ||
| Damping coefficient | [Ns/m] | |||
| Electromechanical coupling coefficient | B | [N/V] | ||
| Young’s modulus | E | Pa | ||
| Damping time constant | [s] | |||
| Electromechanical coupling ratio | - |
| Attached mass | M | kg | ||
| (Equivalent prestress) | Pa | |||
| Capacitance | C | F | ||
| Equivalent parallel resistance | > | > | ||
| Equivalent series resistance | < | |||
| Relative permittivity | - | |||
| Resistivity | m | > | > |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Takagi, K.; Kitazaki, Y.; Kondo, K. A Simple Dynamic Characterization Method for Thin Stacked Dielectric Elastomer Actuators by Suspending a Weight in Air and Electrical Excitation. Actuators 2021, 10, 40. https://doi.org/10.3390/act10030040
Takagi K, Kitazaki Y, Kondo K. A Simple Dynamic Characterization Method for Thin Stacked Dielectric Elastomer Actuators by Suspending a Weight in Air and Electrical Excitation. Actuators. 2021; 10(3):40. https://doi.org/10.3390/act10030040
Chicago/Turabian StyleTakagi, Kentaro, Yuya Kitazaki, and Kota Kondo. 2021. "A Simple Dynamic Characterization Method for Thin Stacked Dielectric Elastomer Actuators by Suspending a Weight in Air and Electrical Excitation" Actuators 10, no. 3: 40. https://doi.org/10.3390/act10030040
APA StyleTakagi, K., Kitazaki, Y., & Kondo, K. (2021). A Simple Dynamic Characterization Method for Thin Stacked Dielectric Elastomer Actuators by Suspending a Weight in Air and Electrical Excitation. Actuators, 10(3), 40. https://doi.org/10.3390/act10030040






