A Large Force Haptic Interface with Modular Linear Actuators
Abstract
1. Introduction
2. Mechanism Design and Analysis
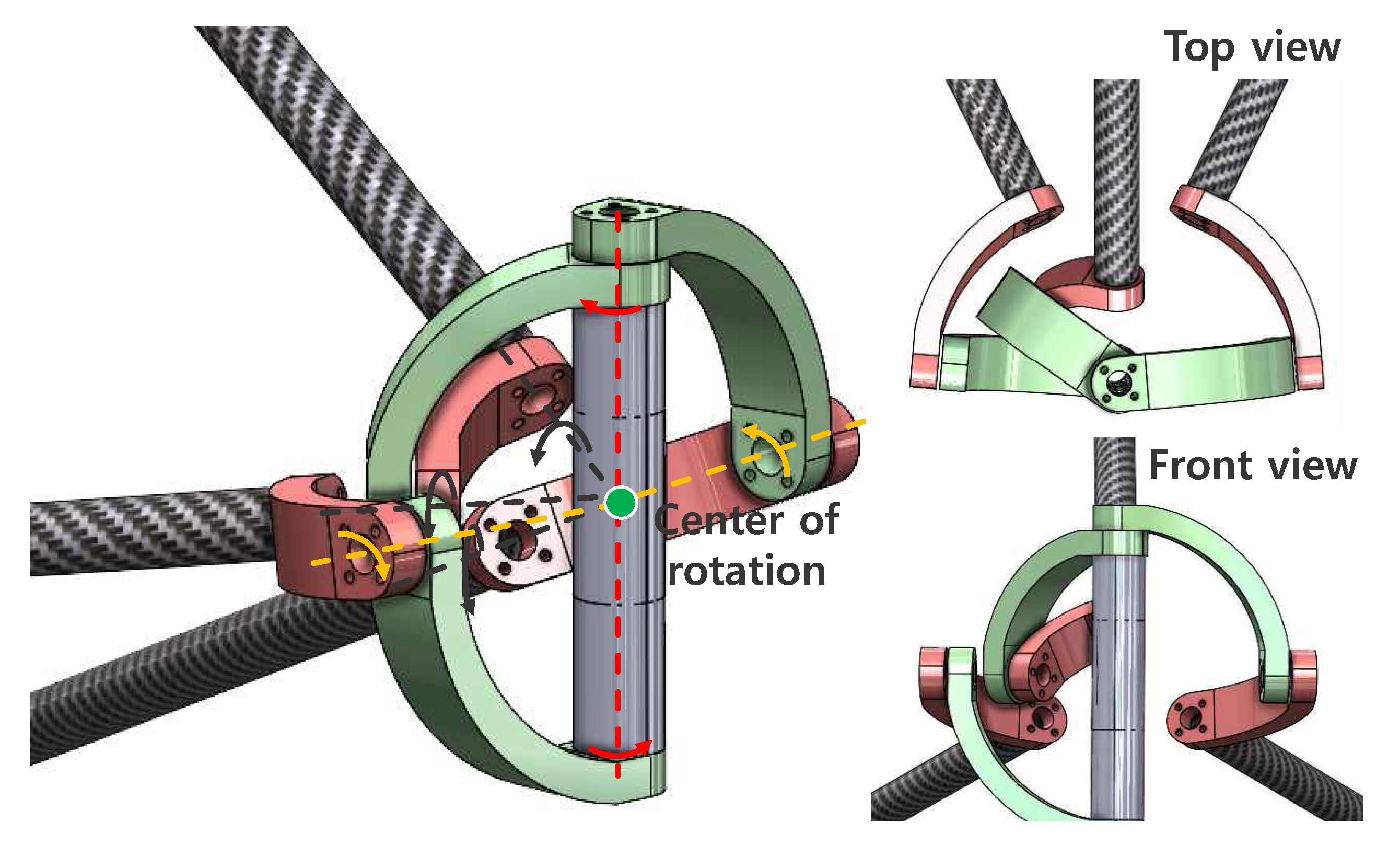
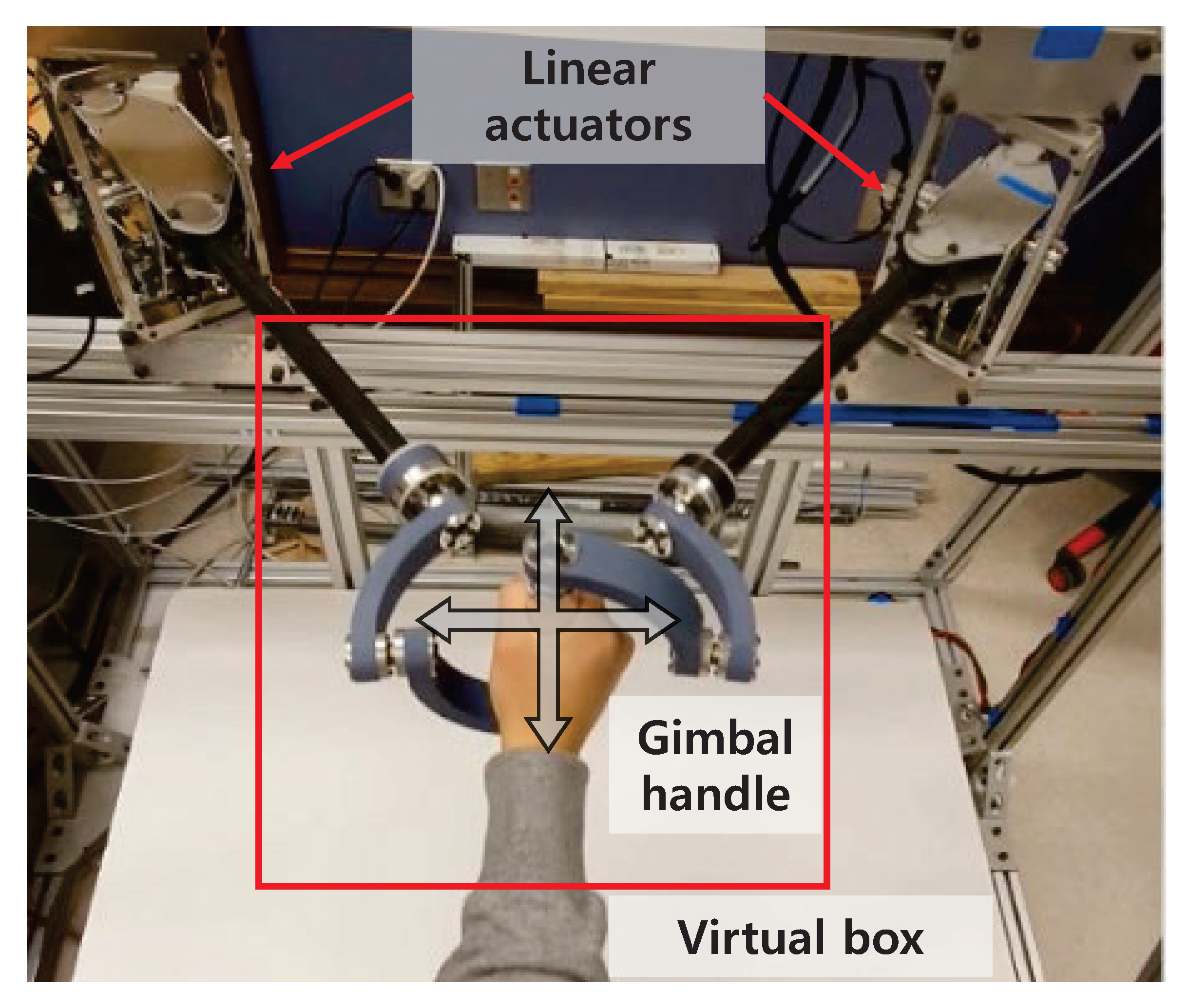
2.1. Design
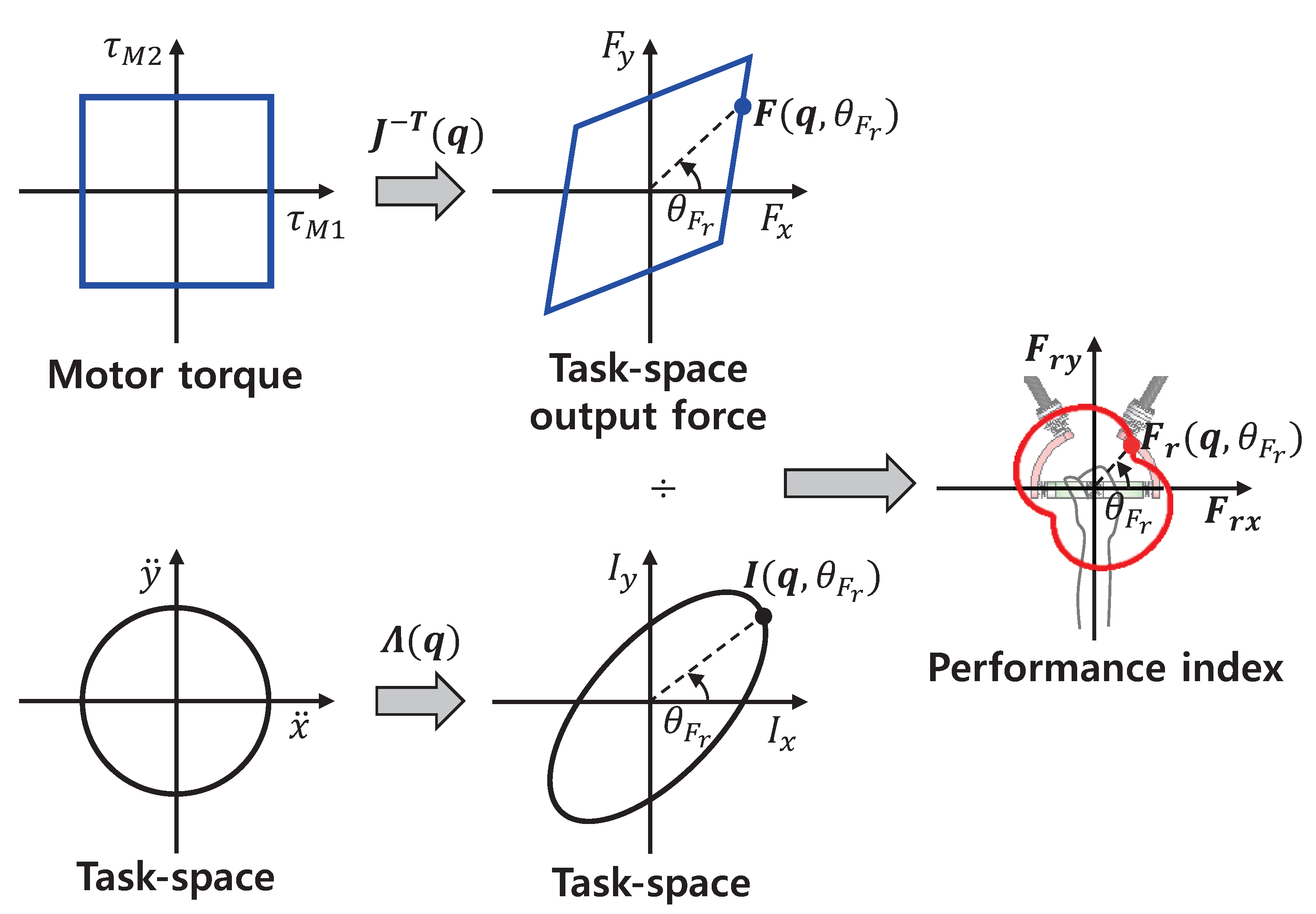
2.2. Analysis
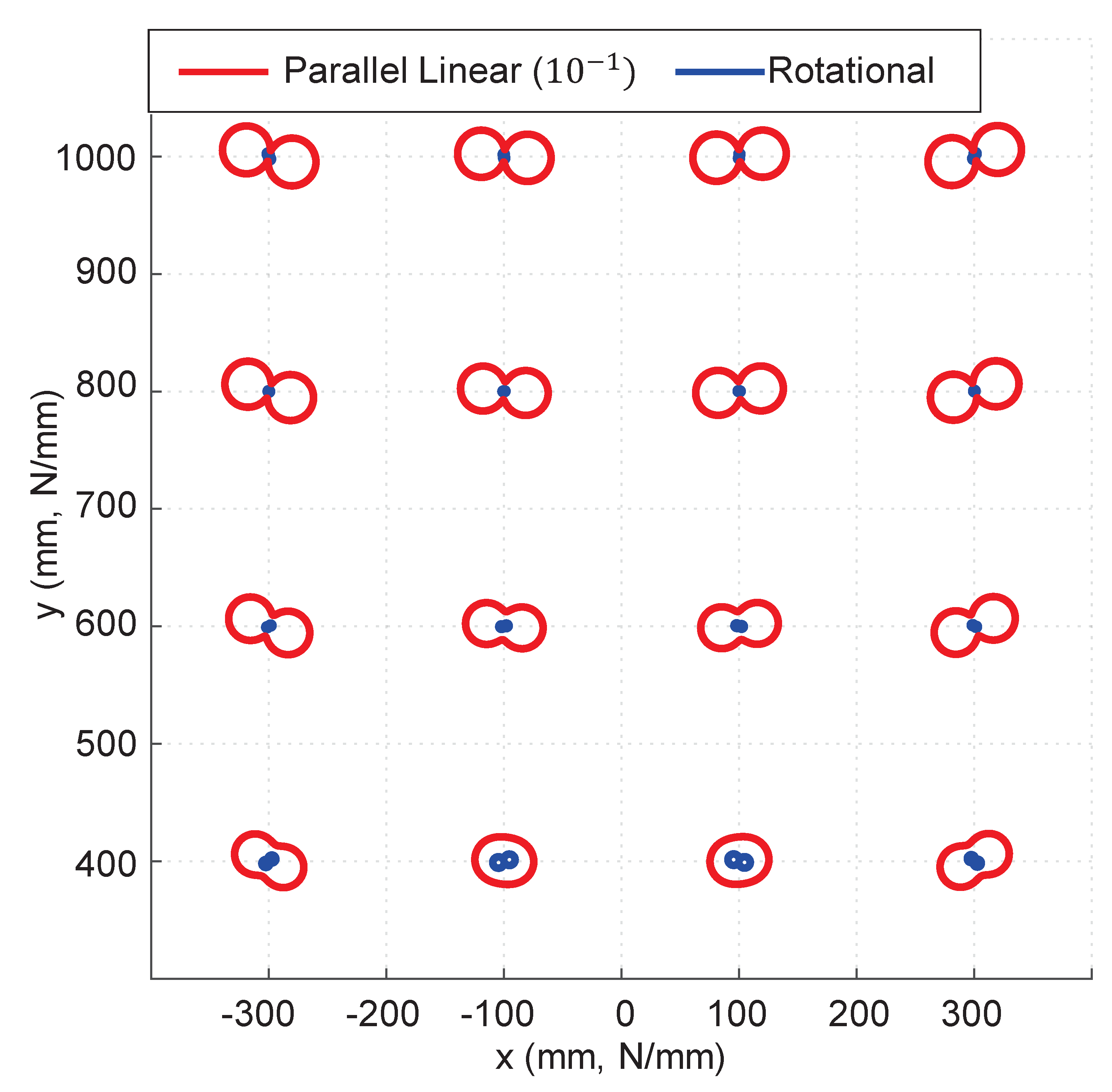
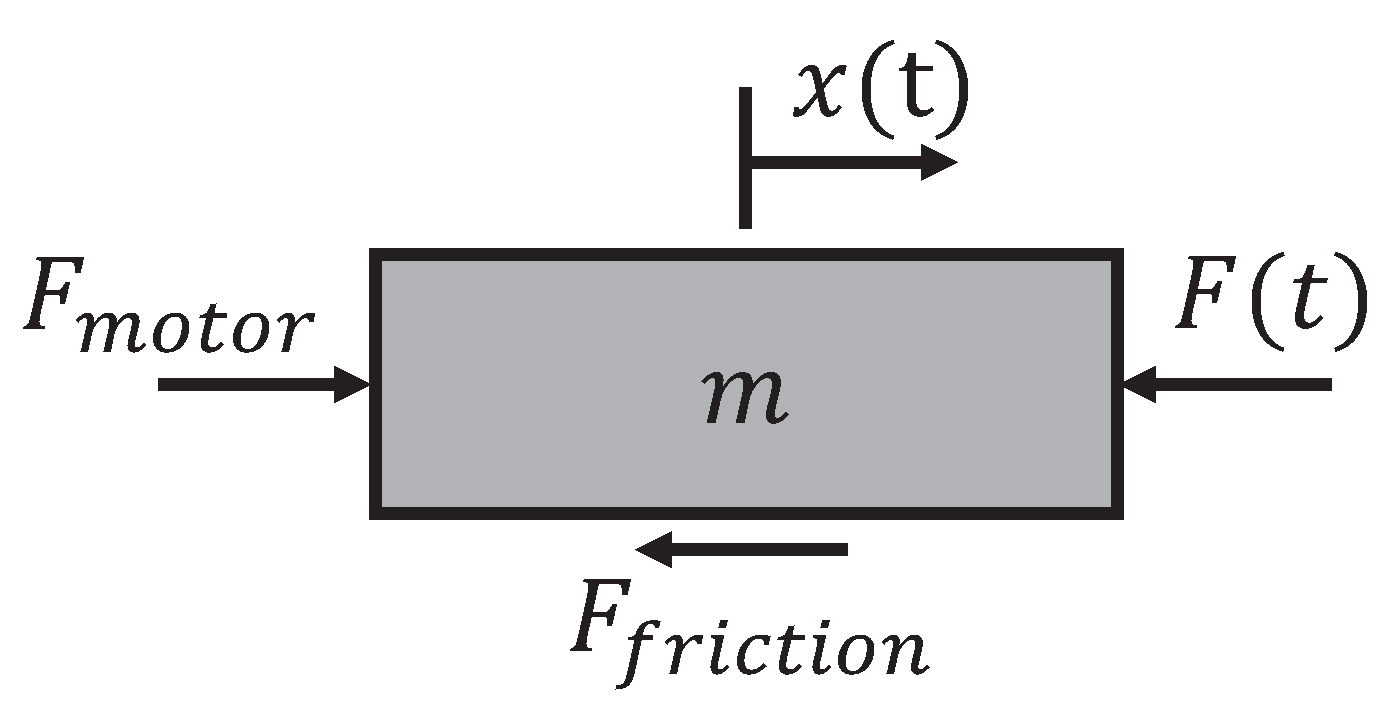
3. Analysis of the Modular Linear Actuator
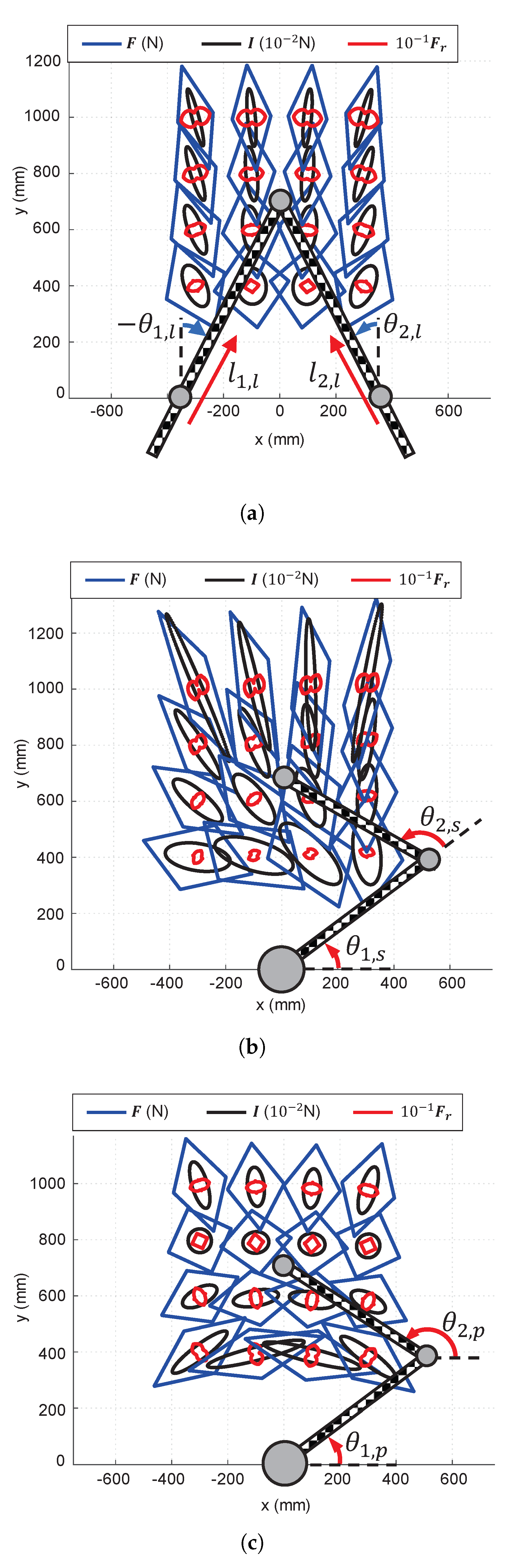
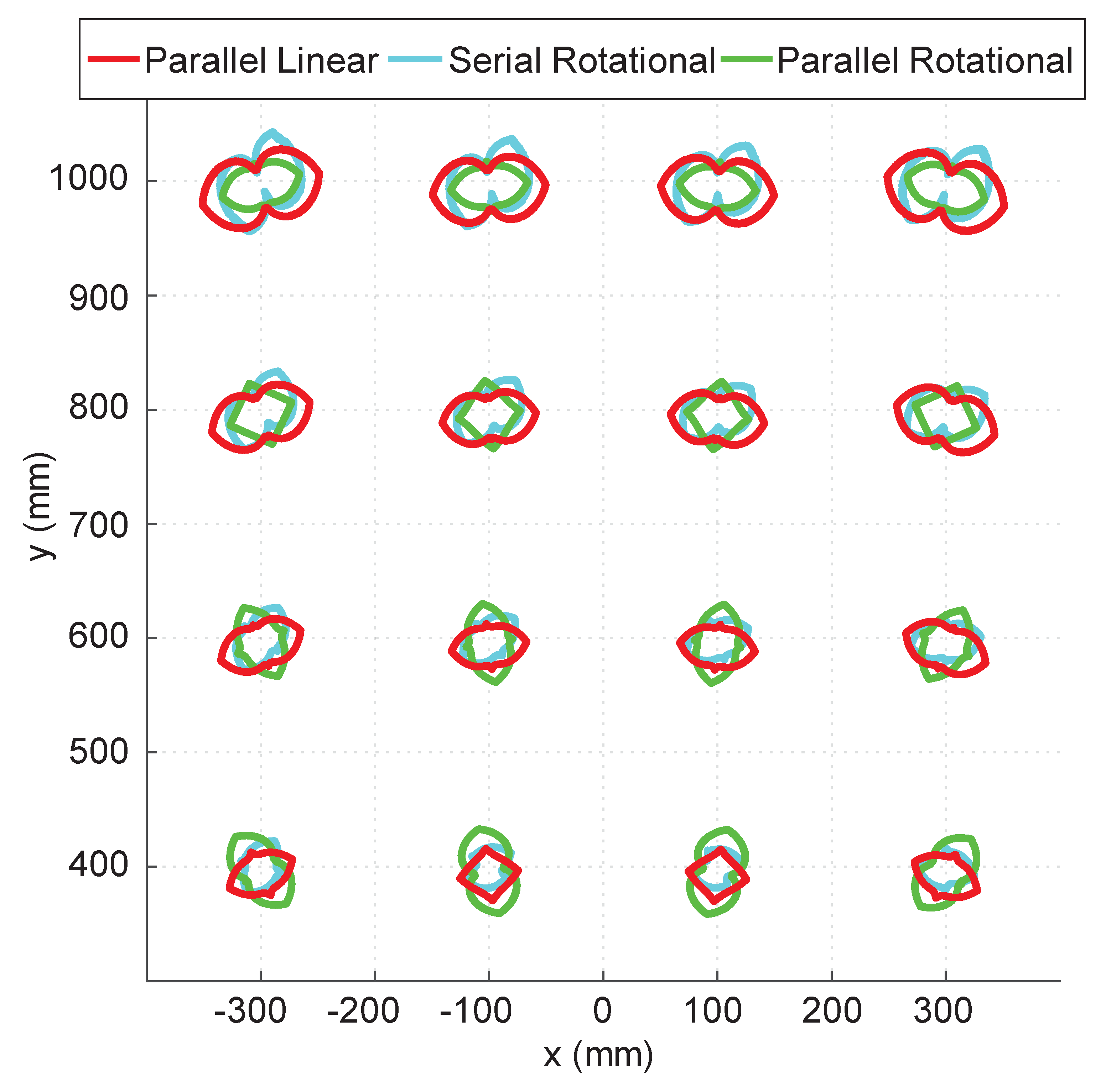
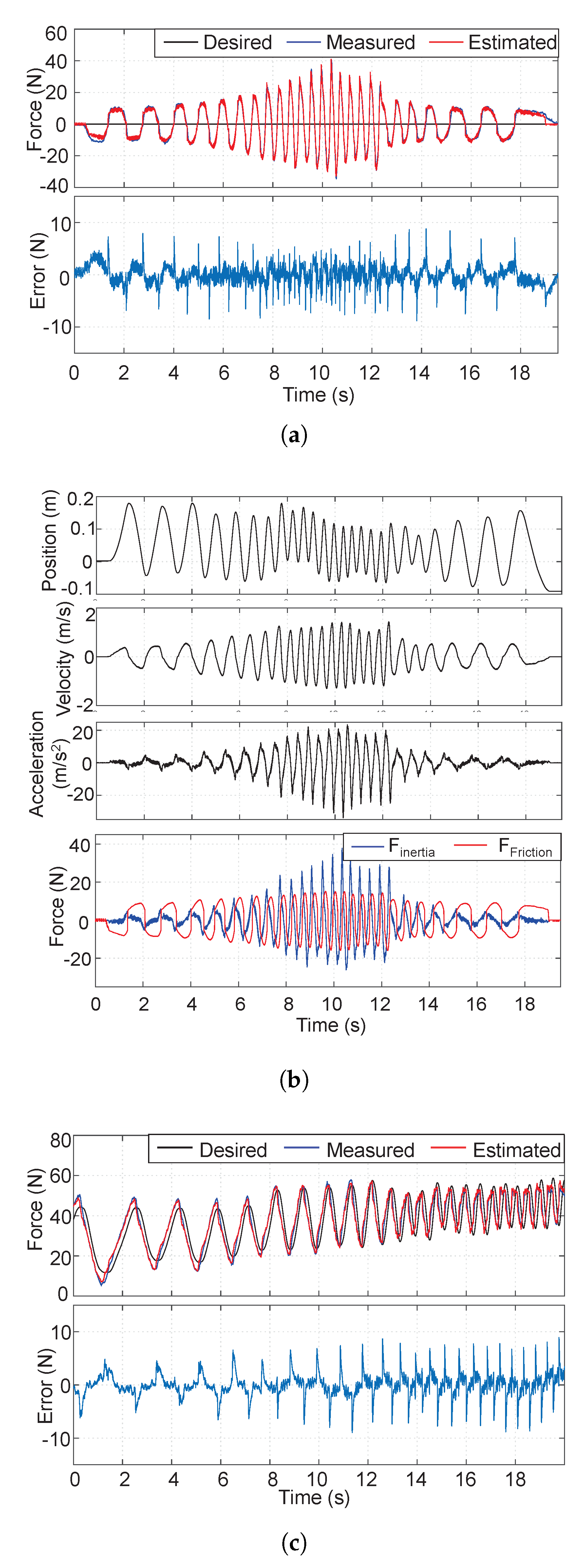
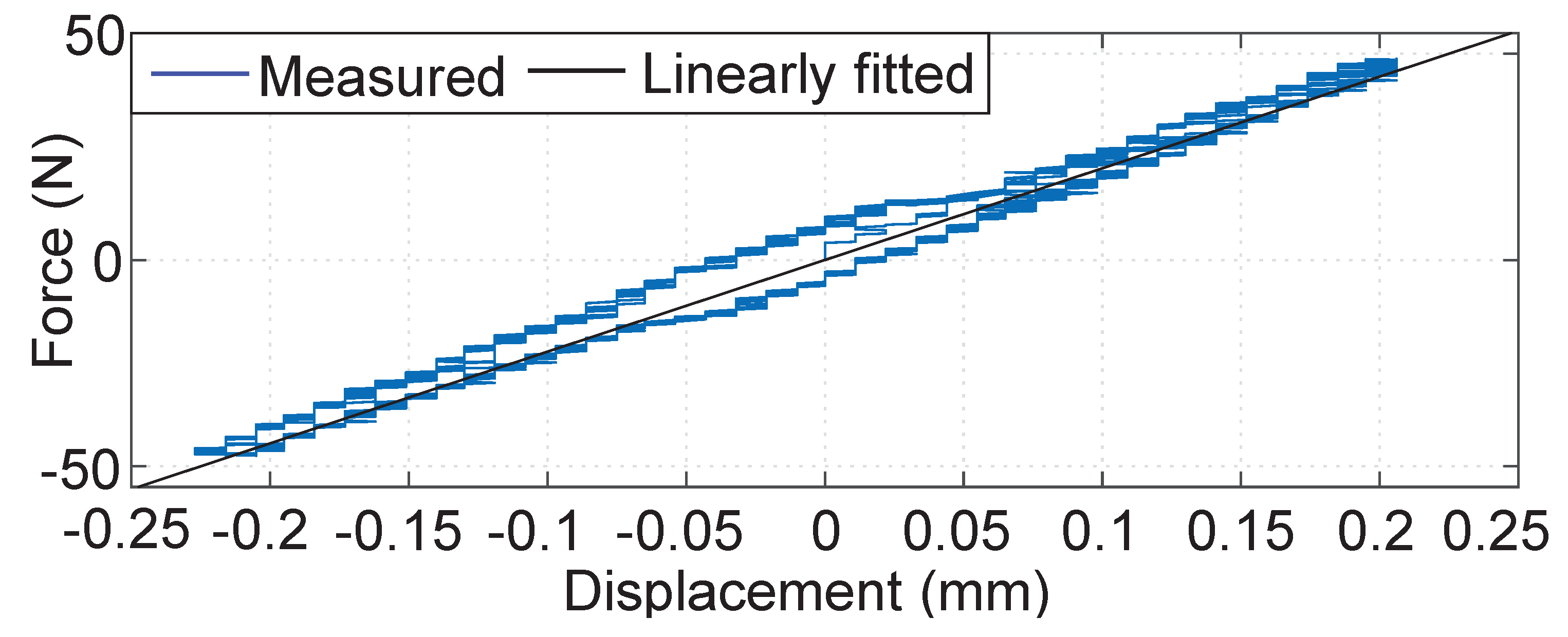
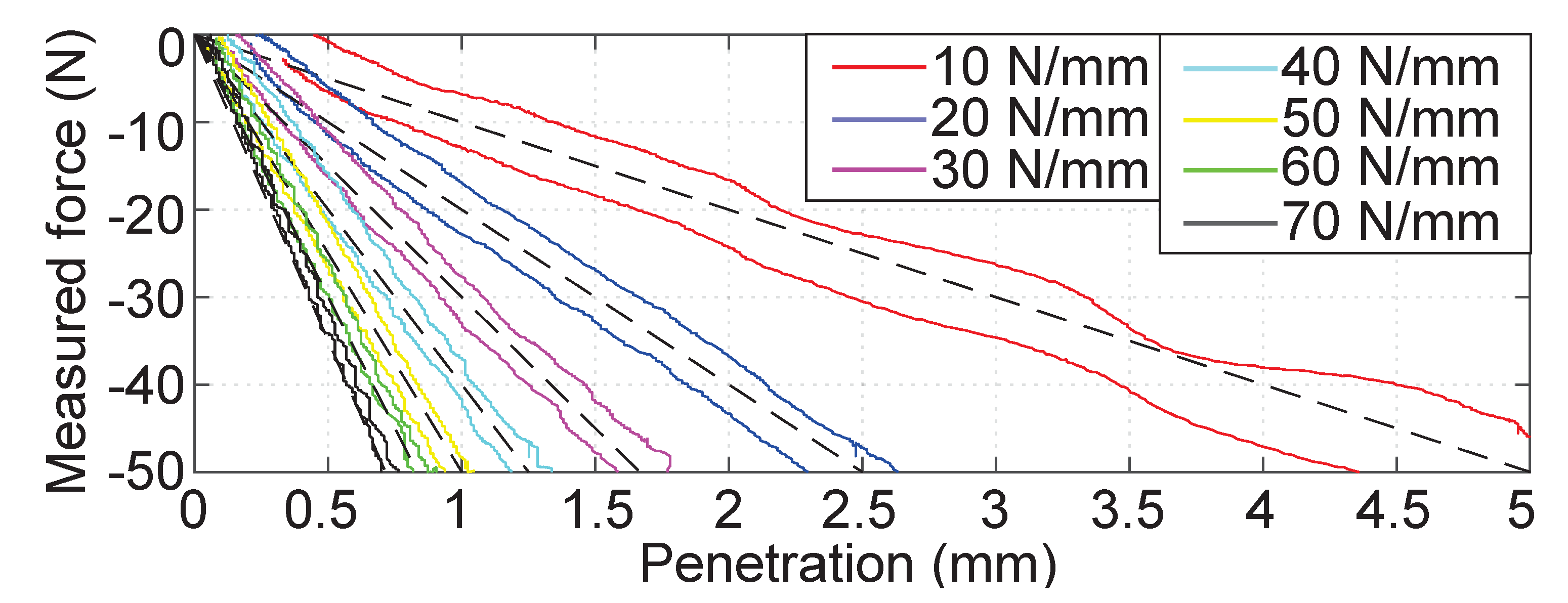
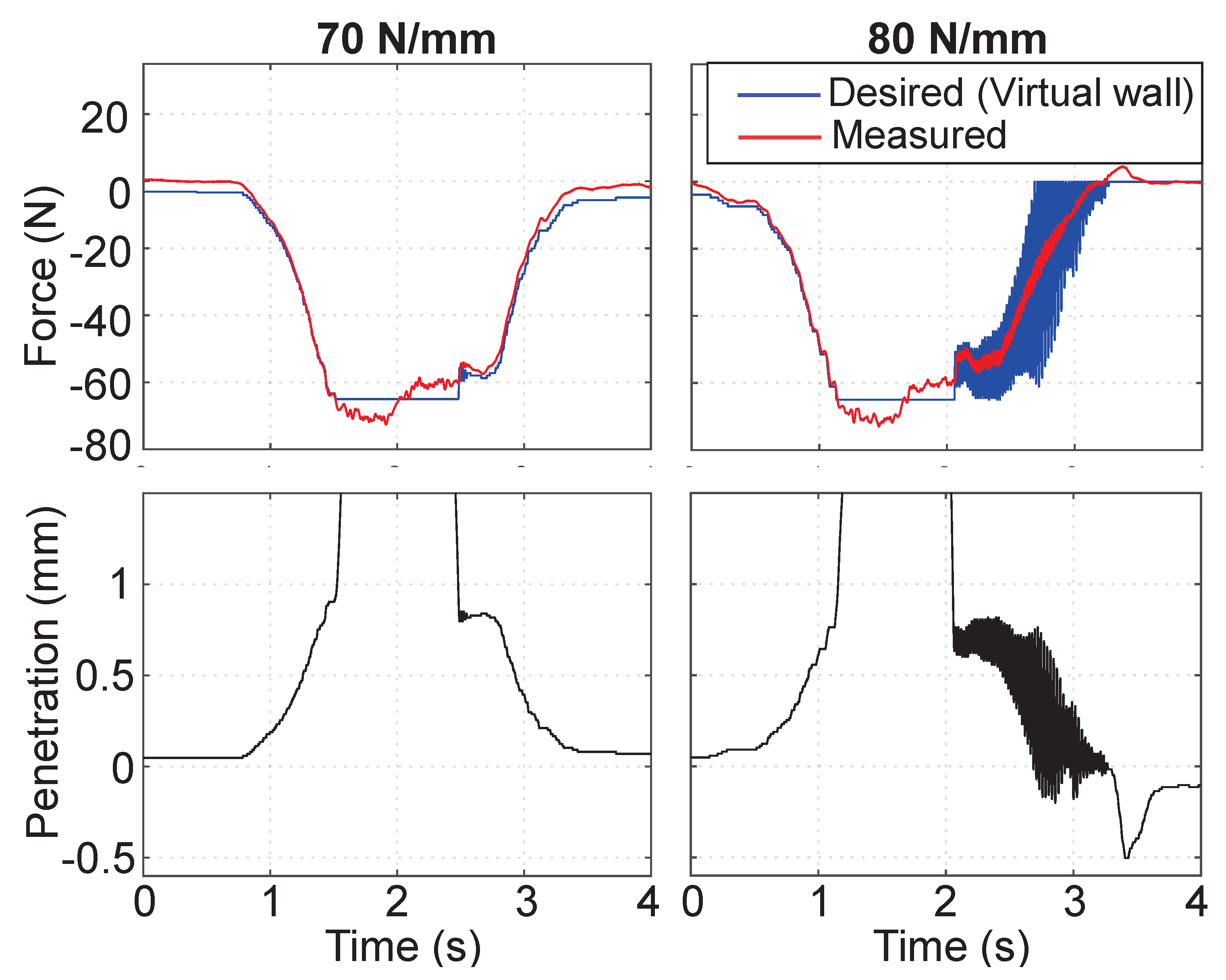
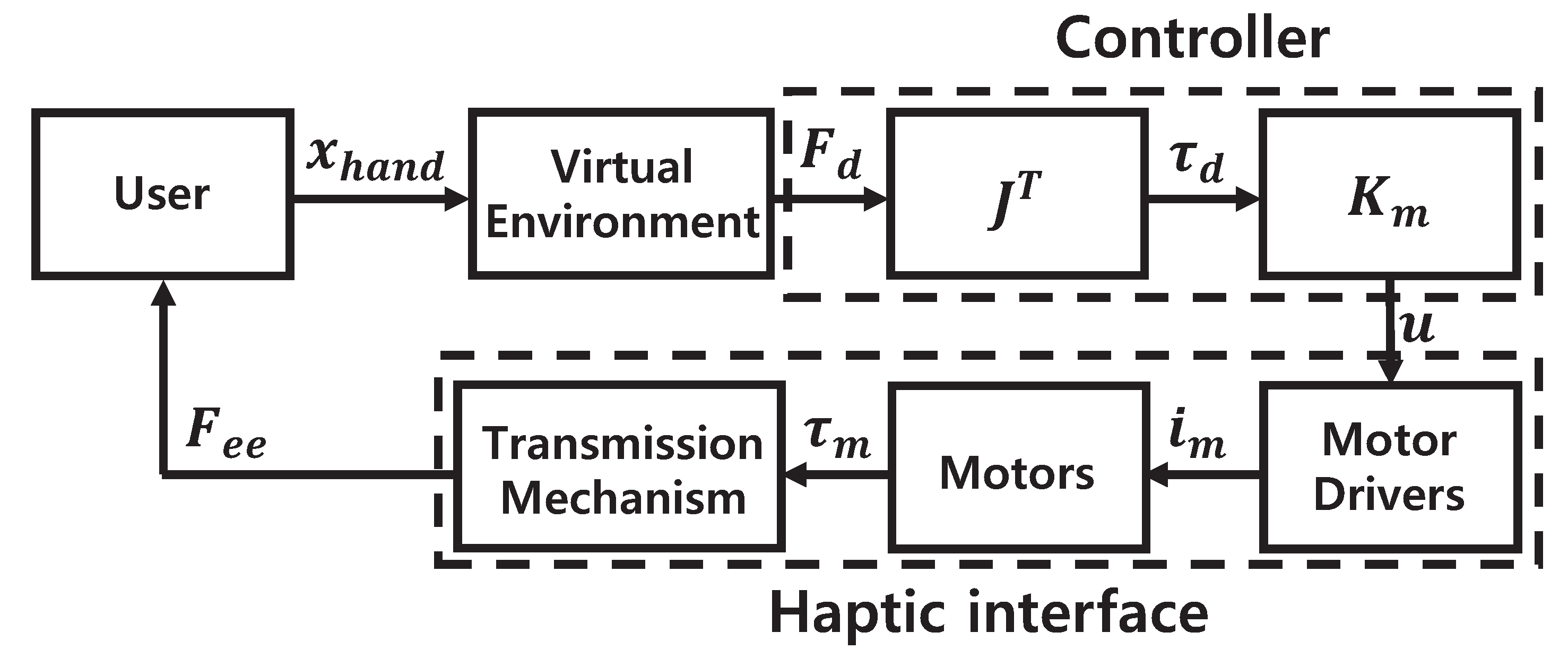
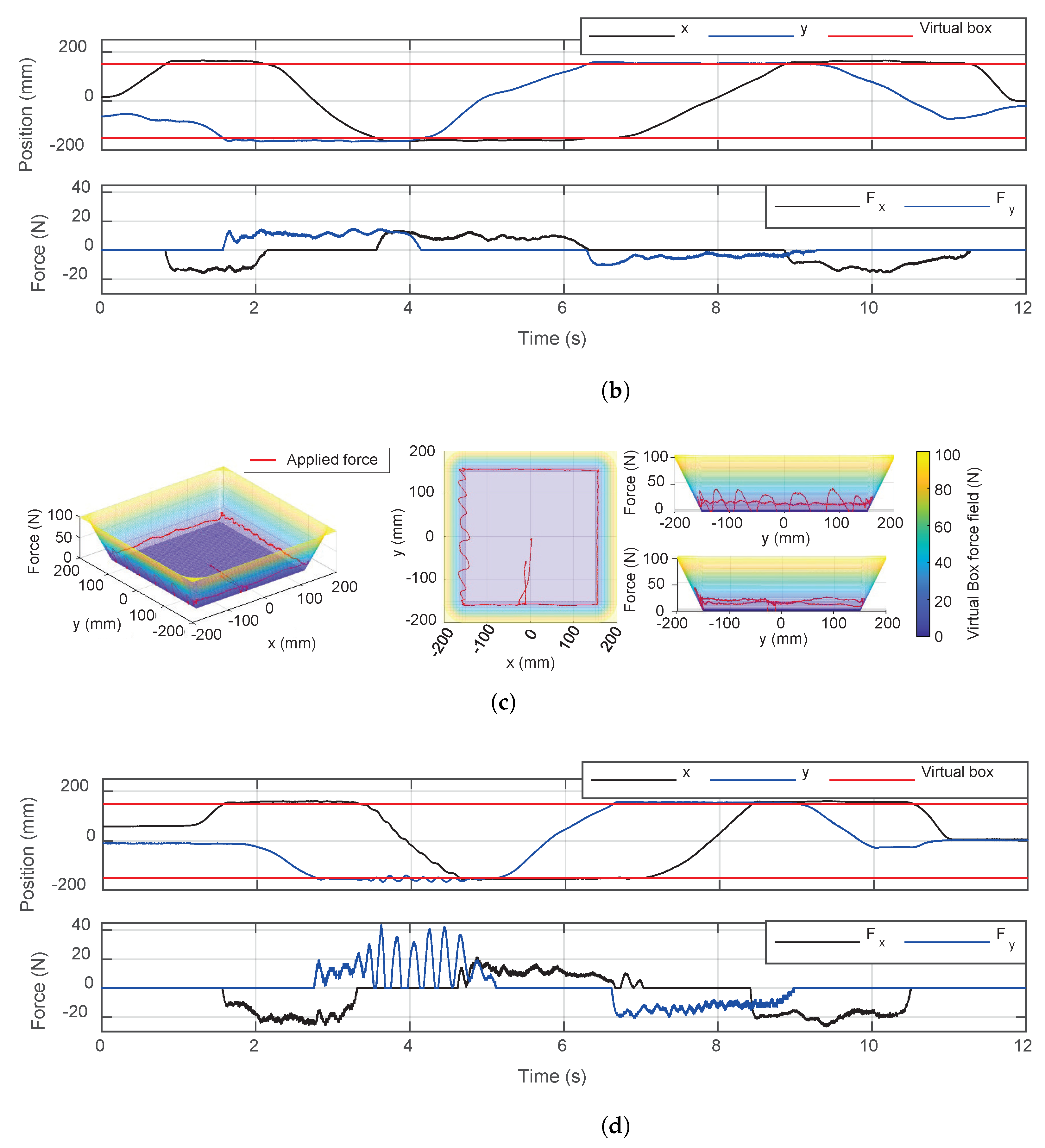
4. Experimental Results and Discussion
5. Conclusions
- Force, inertia, and stiffness analyses showed that the proposed mechanism has advantages over conventional haptic interface mechanisms because of its lightweight and high-stiffness parallel structure. A 2-DOF haptic interface was evaluated as an example. Its scalability and modular design with a linear actuator enable expanding the ROM and DOFs without sacrificing performance.
- Our experiments showed that the actuators were highly backdrivable without any feedback and can impart very high force and stiffness compared to existing devices. The timing belt transmission eliminates the need for a high-quality gearbox, resulting in reduced cost and a simplified design by changing the pulley radius. An accurate system identification was achieved with the Coulomb and viscous friction model, although it showed less accurate results near zero velocity.
- Our analysis and experiments with the prototype haptic interface imply that the proposed mechanism can provide high force and stiffness along with a wide ROM. The proposed haptic interface mechanism allows the free rotation of human hands with the gimbal handle while providing interaction with the virtual environment.
- A fully developed 3D haptic interface with a gimbal design is introduced in Section 2, and its application in dynamic teleoperation will be discussed in future research. More advanced friction models could be used in future works for friction compensation control.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Hannaford, B.; Ryu, J. Time-Domain Passivity Control of Haptic Interfaces. IEEE Trans. Robot. Autom. 2002, 18, 1–10. [Google Scholar] [CrossRef]
- Abdossalami, A.; Sirouspour, S. Adaptive Control for Improved Transparency in Haptic Simulations. IEEE Trans. Haptics 2009, 2, 2–14. [Google Scholar] [CrossRef] [PubMed]
- Forbrigger, S.; Pan, Y. Improving Haptic Transparency for Uncertain Virtual Environments Using Adaptive Control and Gain-Scheduled Prediction. IEEE Trans. Haptics 2018, 11, 543–554. [Google Scholar] [CrossRef] [PubMed]
- Park, J.; Khatib, O. A Haptic Teleoperation Approach Based on Contact Force Control. Int. J. Robot. Res. 2006, 25, 575–591. [Google Scholar] [CrossRef]
- Lawrence, D.A. Stability and Transparency in Bilateral Teleoperation. IEEE Trans. Robot. Autom. 1993, 9, 624–637. [Google Scholar] [CrossRef]
- Sim, Y.; Ramos, J. The dynamic effect of mechanical losses of transmissions on the equation of motion of legged robots. In Proceedings of the IEEE International Conference on Robotics and Automation (ICRA), Xi’an, China, 30 May–5 June 2021; pp. 2056–2062. [Google Scholar]
- Hulin, T.; Hertkorn, K.; Kermer, P.; Schatzle, S.; Artigas, J.; Sagardia, M.; Zacharias, F.; Preusche, C. The dlr bimanual haptic device with optimized workspace. In Proceedings of the IEEE International Conference on Robots and Automation (ICRA), Shanghai, China, 9–13 May 2011; pp. 3441–3442. [Google Scholar]
- Frisoli, A.; Salsedo, F.; Bergamasco, M.; Rossi, B.; Carboncini, M.C. A Force-Feedback Exoskeleton for Upper-Limb Rehabilitation in Virtual Reality. Appl. Bionics Biomech. 2009, 6, 115–126. [Google Scholar] [CrossRef]
- Endo, T.; Kawasaki, H.; Mouri, T.; Ishigure, Y.; Shimomura, H.; Matsumura, M.; Koketsu, K. Five-fingered haptic interface robot: Hiro III. IEEE Trans. Haptics 2011, 4, 14–27. [Google Scholar] [CrossRef] [PubMed]
- Force Dimension. Delta.3. Available online: https://www.forcedimension.com/products/delta (accessed on 25 April 2023).
- Quanser. HD2 High Definition Hpatic Device. Available online: https://www.quanser.com/products/ (accessed on 25 April 2023).
- Cyber Glove Systems. CyberForce. Available online: http://www.cyberglovesystems.com/cyberforce (accessed on 25 April 2023).
- 3D Systems. Phantom Premium 1.5 HF. Available online: https://www.3dsystems.com/ (accessed on 25 April 2023).
- Gosselin, G.; Kong, X.; Focault, S.; Bonev, I. A fully-decoupled 3-dof translational parallel mechanism. In Proceedings of the 4th Chemnitz Parallel Kinematics Seminar Parallel Kinematic Machines in Research and Practice, Chemnitz, Germany, 20–21 April 2004; pp. 595–610. [Google Scholar]
- Zinn, M.; Khatib, O.; Roth, B.; Salisbury, J.K. Large Workspace Haptic Devices—A New Actuation Approach. In Proceedings of the Symposium on Haptic Interfaces for Virtual Environments and Teleoperator Systems, Reno, NV, USA, 13–14 March 2008; pp. 185–192. [Google Scholar]
- Perret, J.; Dominjon, L. The INCA 6D: A Commercial Stringed Haptic System Suitable for Industrial Applications. In Proceedings of the SPIDAR Anniversary Symposium, Lyon, France, 9 December 2009. [Google Scholar]
- Proteus Motion. Proteus Motion. Available online: https://proteusmotion.com/ (accessed on 25 April 2023).
- Maxwell, S. A Modulated-Energy-Dissipation Manipulator and Application to Suppressing Human Arm Tremor. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 1990. [Google Scholar]
- Billette, G.; Gosselin, C. Producing rigid contacts in cable-driven haptic interfaces using impact generating reels. In Proceedings of the IEEE International Conference on Robots and Automation (ICRA), Kobe, Japan, 12–17 May 2009; pp. 307–312. [Google Scholar]
- Lee, G.; Hur, S.; Oh, Y. High-Force Display Capability and Wide Workspace With a Novel Haptic Interface. IEEE/ASME Trans. Mechatron. 2017, 22, 138–148. [Google Scholar] [CrossRef]
- Barnaby, G.; Roudaut, A. Mantis: A Scalable, Lightweight and Accessible Architecture to Build Multiform Force Feedback Systems. In Proceedings of the 32nd Annual ACM Symposium on User Interface Software and Technology, New Orleans, LA, USA, 20–23 October 2019; pp. 937–948. [Google Scholar]
- Wang, S.; Ramos, J. Dynamic Locomotion Teleoperation of a Reduced Model of a Wheeled Humanoid Robot Using a Whole-Body Human-Machine Interface. IEEE Robot. Autom. Lett. 2022, 7, 1872–1879. [Google Scholar] [CrossRef]
- Wang, S.; Murphy, K.; Kenney, D.; Ramos, J. A comparison between joint space and task space mappings for dynamic teleoperation of an anthropomorphic robotic arm in reaction tests. In Proceedings of the IEEE International Conference on Robots and Automation (ICRA), Xi’an, China, 30 May–5 June 2021; pp. 2846–2852. [Google Scholar]
- Purushottam, A.; Jung, Y.; Murphy, K.; Baek, D.; Ramos, J. Hands-free Telelocomotion of a Wheeled Humanoid. In Proceedings of the IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Kyoto, Japan, 23–27 October 2022; pp. 8313–8320. [Google Scholar]
- Rancourt, D.; Hogan, N. Dynamics of Pushing. J. Mot. Behav. 2001, 33, 351–362. [Google Scholar] [CrossRef] [PubMed]
- Gosselin, C.M.; Pierre, E.S.; Gagne, M. On the development of the Agile Eye. IEEE Robot. Autom. Mag. 1996, 3, 29–37. [Google Scholar] [CrossRef]
- Stewart, D. A platform with six degrees of freedom. Proc. Inst. Mech. Eng. 1965, 180, 371–386. [Google Scholar] [CrossRef]
- Lynch, K.M.; Park, F.C. Modern Robotics; Cambridge University Press: Cambridge, UK, 2017. [Google Scholar]
- Adams, R.J.; Hannaford, B. Stable haptic interaction with virtual environments. IEEE Trans. Robot. Autom. 1999, 15, 465–474. [Google Scholar] [CrossRef]
- Sim, Y.; Ramos, J. Tello Leg: The Study of Design Principles and Metrics for Dynamic Humanoid Robots. IEEE Robot. Autom. Lett. 2022, 7, 9318–9325. [Google Scholar] [CrossRef]
- Ramos, J.; Kim, S. Dynamic locomotion synchronization of bipedal robot and human operator via bilateral feedback teleoperation. Sci. Robot. 2019, 4, eaav4282. [Google Scholar] [CrossRef] [PubMed]





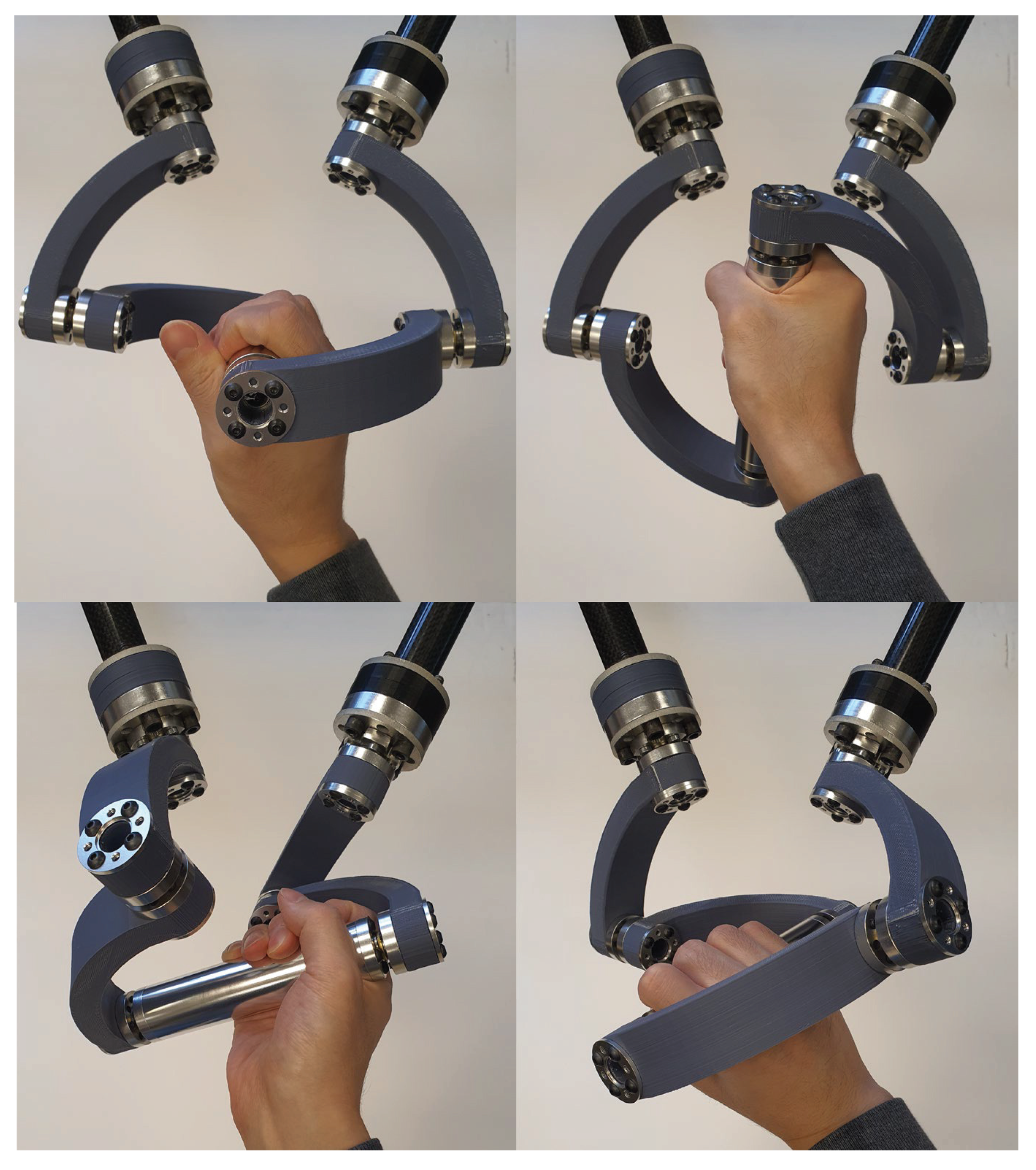



| Specification | |
|---|---|
| Min. available force | 83 N (Continuous) |
| at home position | 166 N (Peak) |
| Motor | Turnigy 9235–100 KV |
| Pulley radius: 28.245 mm | |
| Nominal torque: 2.825 Nm | |
| Sensor | Motor encoder: |
| 14 bit incremental | |
| Actuator gimbal encoder: | |
| 12 bit absolute | |
| Controller | NI cRIO-9082 |
| Control rate: 1 kHz | |
| FPGA clock: 40 MHz | |
| Linear motion rod | Material: CFRP |
| OD: 25.4 mm | |
| ID: 22.86 mm | |
| Length: 1219 mm | |
| Weight: 158 g | |
| Timing belt | Gates PowerGrip |
| GT3 5MGT | |
| Glass fiber reinforced | |
| Gimbal handle | Material: ABS and steel |
| Weight: 562 g |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jung, Y.; Ramos, J. A Large Force Haptic Interface with Modular Linear Actuators. Actuators 2023, 12, 293. https://doi.org/10.3390/act12070293
Jung Y, Ramos J. A Large Force Haptic Interface with Modular Linear Actuators. Actuators. 2023; 12(7):293. https://doi.org/10.3390/act12070293
Chicago/Turabian StyleJung, Yeongtae, and Joao Ramos. 2023. "A Large Force Haptic Interface with Modular Linear Actuators" Actuators 12, no. 7: 293. https://doi.org/10.3390/act12070293
APA StyleJung, Y., & Ramos, J. (2023). A Large Force Haptic Interface with Modular Linear Actuators. Actuators, 12(7), 293. https://doi.org/10.3390/act12070293






