1. Introduction
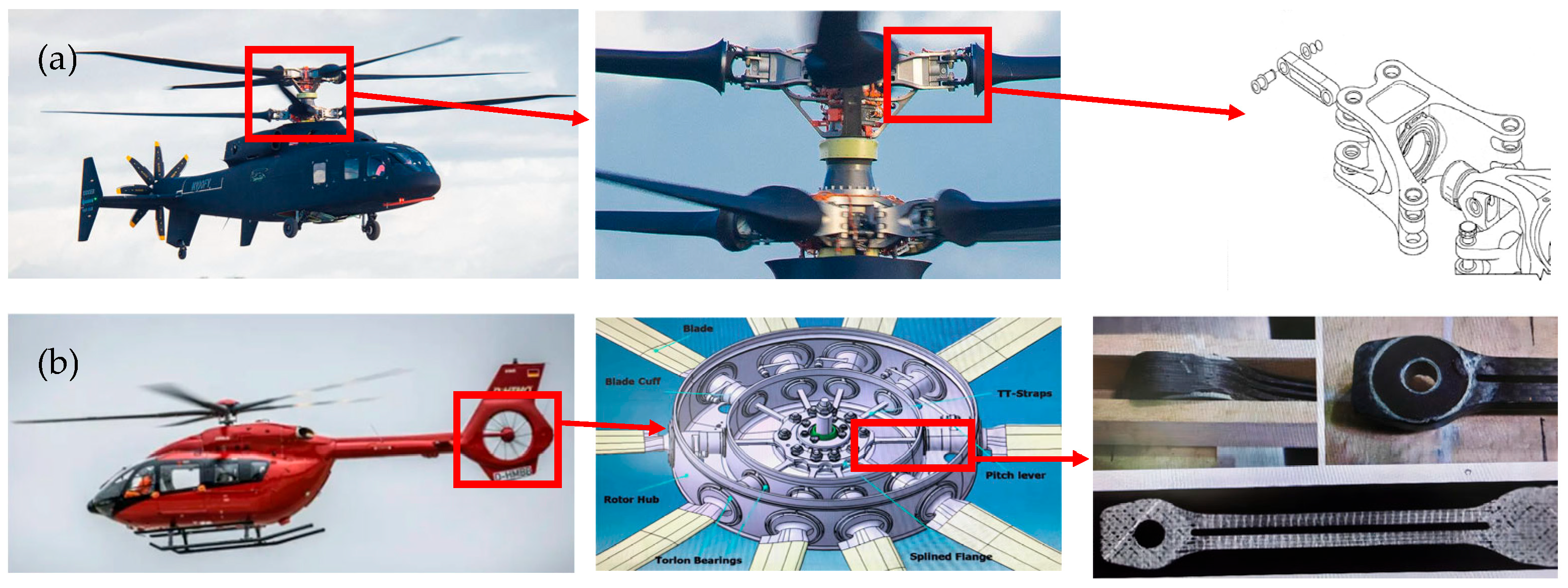
TT straps have been extensively applied in the rotor systems of various helicopters, such as the heavy-lift Mi-26, the Sikorsky compound coaxial helicopter SB > 1 (
Figure 1a), the Bell XV-15 tilt rotor research aircraft, and the Airbus H120 and H145 [
1,
2]. The TT strap predominantly connects the rotor blade to the hub, allowing blade feathering while reacting to the centrifugal load [
3]. Moreover, the TT strap improves the load handling capacity of the axial hinges in rotor hubs, simplifying the hub structure.
All prominent companies engaged in the research and development of high-performance TT straps, such as LORD, Airbus, and Mil Moscow Helicopter Plant, have decades of development experience and have released multiple generations of products. The fourth generation of a carbon fiber TT strap currently applied to the H145 tail rotor was developed by Airbus, and it is well known that this is the most advanced TT strap product [
4]. The TT strap of the Mi-26 helicopter rotor is known as the largest product and can transmit the largest centrifugal force. It is worth noting that the TT strap has become a subject of intellectual property protection for major helicopter companies [
5,
6,
7].
The mechanical performance of TT straps is a key aspect of their functionality. Over the past two decades, there has been relatively limited research in the literature focusing on the key performance metrics of the TT strap, such as its torsional stiffness, deformation, and load-bearing capacity. Elif Ahci-Ezg [
8] explored structural design methodologies for these components in a study of the H145 Fenestron tail rotor TT strip (
Figure 1b). In this research, the dimensions of the connection area were determined using a set of specific formulas. For the torsional deformation segment, parameters such as the number of layers, width, and thickness were optimized via Hyperstudy. However, during the TT strap design process, the slotting at the torsional deformation segment still depended on experience. Hongjin Xiong [
9] created a simulation model using finite element software. The stiffness and stress distribution of a composite material TT strap were analyzed. The accuracy of the established model was confirmed through experimental testing.
Previous studies on the key performance characteristics of TT straps have relied on software for sensitivity analysis or the direct application of optimization software for deriving structural parameters. However, these methods have often demonstrated limited efficiency in assessing the performance of TT straps and have not enabled the comprehensive analysis of the correlation between key performance characteristics and critical design parameters, such as the materials, dimensions, and load-bearing capacities of the connection segment in relation to torsional stiffness and stress. In this work, a theoretical and experimental investigation of the key performance of TT straps was conducted. The torsional response of a TT strap under a specified torque was calculated, the relationship between key design parameters and performance was illustrated, a rapid evaluation method for the performance of the torsional deformation segment was established, and an analytical investigation into the key parameters influencing the TT strap performance was performed. Finally, further experimental research was carried out to examine the impacts of critical parameters such as the length, material, and structure of the connector segments through four design cases.
2. Rapid Evaluation Method for Key Performance Characteristics
This study focused on a typical TT strap using filament winding composites, aiming to establish a method for rapidly evaluating key aspects of their performance. These TT straps feature two rigid connecting segments and a flexible torsional deformation segment, composed of either a single layer or multiple layers of strap elements [
10,
11]. At the torsional deformation segment, strap elements with more than two layers are not interconnected, while the connecting segments utilize glass fiber fabric layers for bonding.
The structural characteristics of the strap elements include unidirectional composite laminates wound around resin or rubber filler blocks, which are then fitted within metal bushings (
Figure 2).
This research was centered on the design requirements for the mechanical performance of TT straps, with the specific aim of ensuring that, under centrifugal force Fc, their torsional stiffness remains below a designated threshold, while also allowing for the maximum pitch angle alteration of the rotor blades. The mechanical performance of these straps is critically influenced by the parameters of the torsional deformation segment, which consists of multiple cuboids. A primary objective of this section is the development of a computational model to evaluate the torsional stiffness and stress distribution within these cuboids. The connection segment is responsible for bearing the loads generated by the torsional deformation segment, with its load-bearing capacity being one of the key parameters. However, other design parameters, such as the dimensions, have a relatively minor impact on the mechanical performance. Given the complexity of stresses and the diversity of configurations within the connecting segments, an accurate assessment of the load-bearing capacity using purely parametric methods presents challenges, necessitating targeted experimental research.
This section presents the detailed construction process of the rapid evaluation model for the mechanical performance of the torsional deformation segment of TT straps. Initially, in two coordinate systems, the displacement method is utilized to decompose the complex deformation of each rectangular section in the torsional deformation segment into a superposition of multiple simpler deformations: (1) free torsion; (2) warping-constrained torsion; (3) eccentric bending; and (4) additional deformation due to centrifugal forces. Subsequently, relationships between each simple deformation and the applied torque are established.
Section 2.2 specifically focuses on the formulation process for the expression of additional torque due to eccentric bending in rectangles, taking into account the centrifugal force. Following this, by superimposing these expressions for simple deformations, an overall torque balance equation is derived. Finally, by solving this equation, a set of expressions is presented in
Section 2.3, which intuitively demonstrates how the materials, structural parameters, and centrifugal force parameters influence the stiffness and stress distribution of TT straps.
Based on the derived expressions, this study enables the performance of the specific design parameters to be evaluated, leading to the identification of key parameters. In
Section 3, analytical calculations regarding the design of critical parameters for TT straps are presented, enabling the relationship between the performance of TT straps and these parameters to be examined. The goal is to offer a more detailed theoretical foundation for the design of essential parameters of TT straps. The research approach adopted in this study is illustrated in
Figure 3.
2.1. Coordinate Systems
The method is described within the following two coordinate systems:
The torsional center coordinate system: Its origin, denoted as Ot, is located at the starting end face of the torsional deformation segment. The xt-axis coincides with the torsional center axis, and the zt-axis is parallel to the axis of the bushing hole.
The centroid coordinate system of any cuboid: Its origin, O
ci, is at the starting end face of the torsional deformation segment, with its
xci-axis aligning with the centroid axis of the cuboid. It is important to note that the centroid coordinate system can be obtained by translating the torsional center coordinate system (
Figure 4).
2.2. Additional Torque Caused by Centrifugal Forces
This section provides a detailed analysis of the additional torque due to centrifugal forces under two states: free torsion and eccentrically constrained torsion. Here, the constraint refers to the restriction of warping and bending deformations at both ends of the torsional deformation segment, assuming that the connecting segments are rigid. The deformations under free torsion and constrained torsion are depicted in
Figure 5. A segment of length d
x on the torsional deformation segment was selected for in-depth analysis. To simplify the analysis, a small-angle assumption was employed. That is, sin(d
θ) is approximately equal to d
θ, where d
θ represents the relative torsional deformation angle between two cross-sections of the infinitesimal element.
We analyzed the deviation angle between the centrifugal tensile stress σ
xFc and the coordinates (
y,
z) of a microelement, which possesses an area of d
yd
z, within the torsional center coordinate system following torsional deformation. In the initial state, without torsional deformation, the microelement’s edges are aligned with the centrifugal force direction. However, when torsional deformation is introduced, shear-induced deformation prompts a deviation of the microelement’s edges from their original orientation, characterized by an angle of d
θ. This phenomenon results in a relative displacement, denoted as d
l1, of the microelement’s opposing end surfaces in the
yt–
zt plane. Subsequently, the normal stress σ
xFc is bifurcated into two distinct components: one aligned with the deformation direction and the other, σ
1, aligned along d
l1’s direction (
Figure 5a). The analysis proceeds to compute the torque M(σ
1) engendered by σ
1. Integrating this torque across the microelement’s entire cross-section enables the determination of the supplementary torque necessary to counteract the centrifugal force during torsional deformation.
Furthermore, under eccentrically constrained torsion conditions, a cuboid not only undergoes torsion around its centroid but also experiences bending and deflection deformations along its centroid axis. The exertion of centrifugal force notably modifies the cuboid’s bending stiffness and then increases the additional torsional stiffness.
2.3. Analytical Calculation Formulas
- (1)
Torque expression
The first part of this section elucidates the torque calculation for a cuboid during torsional deformation. Neglecting the influence of body forces ensures that the total torque along the xt-axis of the cuboid remains constant. The displacement method was employed to model the deformation distribution of the cuboid, dividing it into four parts: free torsion, warping-constrained deformation, eccentric bending deformation, and additional deformation due to centrifugal force. Given the deformation θ(L) at both ends of the cuboid around the xt-axis, the following four torques were solved: the torque Mθ due to free torsion, the additional torque Mtφ caused by the torsional warping constraint, the additional torque Mtlz and Mtly resulting from eccentric bending, and the additional torque MFc generated by centrifugal force.
The free torsion torque M
θ of the cuboid was determined using a formula derived from fitting a table [
12] based on different width-to-thickness ratios. The computation for M
θ is given below:
where
x is the spatial coordinate along the
xt direction.
denotes the derivative of
with respect to
x, where
is the distribution of the torsional angle for the analyzed cuboid. G represents the shear modulus of the material, and
and
are the width and thickness of the cuboid, respectively.
The warping constraint torque M
tφ for a rectangular cross-section is an approximate solution obtained by neglecting the n > 1 terms of the warping function [
12]. M
tφ can be calculated as follows:
where E denotes the tensile modulus in the fiber direction, and
represents the third derivative of
with respect to
x.
The additional torque resulting from eccentric bending and denoted by M
tlz can be expressed as follows:
where
represents the deviation of the centroid of the analyzed cuboid from the torsional center along the
zt-axis direction.
The additional torque resulting from eccentric bending and denoted by M
tly can be calculated as follows:
where
denotes the deviation of the centroid of the analyzed cuboid from the torsional center along the
yt-axis direction.
The formula of M
Fc is outlined below:
where
symbolizes the centrifugal force, while
signifies the total cross-sectional area of the torsional deformation segment.
Through the superposition of five torque equations, the equilibrium expression for the total torque M
t is derived.
The equation governing the total torque M
t is formulated as a second-order differential equation with constant coefficients with respect to
. By meticulously addressing the boundary conditions at both extremities of the torsional deformation segment, an analytical solution for
can be derived for any prescribed M
t. The general solution is as follows:
where
where
and
represent the hyperbolic sine and hyperbolic cosine functions, respectively.
- (2)
Calculation formulas for torsional stiffness
The second part of this section describes the calculation formulas for various torsional stiffnesses, which serve as part of the rapid evaluation method.
D
θ denotes the free torsional stiffness of the cross-section of the cuboid, and it can be calculated as follows:
D
Fc refers to the additional torsional stiffness induced by the centrifugal force in the cross-section of the cuboid, and it can be calculated as follows:
DL
θ represents the overall free torsional stiffness of the analyzed cuboid. The expression proceeds as follows:
where L denotes the length of the torsional deformation segment.
D
LΣ denotes the overall torsional stiffness of the analyzed cuboid. The expression proceeds as follows:
- (3)
Stress and shear force calculation formulas.
The third part of this section presents the calculation formulas for determining the distribution of tensile stress , shear stresses and , and shear force at the end surface of the cuboid due to eccentric torsional bending. Additionally, it provides the formula for calculating the shear stress induced by the shear force . These equations constitute an integral part of the rapid evaluation method.
The calculation formula for the normal stress
induced by the centrifugal force is as follows:
The formula for calculating the shear stress distribution is derived by omitting the terms with n > 1 from the warping function [
12] when dealing with the torsion of a rectangular cross-sectioned bar.
The tensile stress distribution
can be calculated as follows:
The shear force
can be calculated as follows:
where
θ(L) represents the relative torsional angle at both ends of the torsional deformation segment.
The shear stress
can be calculated as follows:
The rapid evaluation method for assessing the performance of TT straps in this article comprises the three aforementioned sets of calculation formulas. These equations form the foundation for the subsequent analytical investigation of key parameter design aspects.
3. Analytical Study of the Key Performance
This section presents an analytical study of the key design parameters of TT straps based on the rapid performance evaluation method described in
Section 2.3 and the structural parameters of the TT strap presented in [
9]. The structural parameters of the TT strap in [
9] are as follows: three-layer strap elements, 16.2 mm wide, 8.8 mm thick, with 276 mm long torsional deformation segments made of 3232A/S4C10-800 glass-fiber-reinforced unidirectional resin composite. This material has a tensile modulus E of 50 GPa and a shear modulus G of 4.9 GPa.
Central to the design requirements of the TT straps is their mechanical performance. Taking the TT straps of tail rotors, tail propellers, and tilt-rotor aircraft as examples, this section analyzes the key design parameters and their related mechanical performance design requirements.
- (1)
Under prescribed centrifugal forces , the torsional stiffness should remain within predefined limits .
- (2)
The torsional deformation must prevent structural failure at the maximum torsional angle. It should keep the maximal stress below the tensile stress threshold of the material under the specified centrifugal force and maximum torsion. Additionally, the shear stress and should stay within the shear strength limit of the material. The shear force should not exceed the structural load-bearing capacity of the connecting segments.
- (3)
The dimensional parameters should comply with the specified spatial constraints.
The parameters , , , , , and , pivotal in these design requirements, can be efficiently calculated using Formulas (12), (15), (13), (14), (11), and (16), respectively. These calculations facilitate the formulation of tailored adjustment plans to meet the specific design criteria.
Based on the parameters outlined in [
9], this section presents an analytical study of the key parameter design of TT straps, utilizing relevant equations:
- (1)
Equation (12) reveals the key design parameters for tensile stress . When the tensile stress generated by centrifugal force becomes excessive, it can be mitigated by enhancing the cross-sectional area of the torsional deformation segment. Additionally, materials with a higher tensile stress limit can be employed to augment the load-bearing capacity.
- (2)
Equation (15) reveals that the key design parameters affecting the maximum additional tensile stress due to torsion include E, , , , , , and L. To reduce excessive stress induced by torsion, the following measures can be adopted:
Firstly, materials with a lower modulus of elasticity in the direction of tension can be selected.
Secondly, the number of cuboids can be increased to reduce the width
and thickness
.
are also the maximum values of 2
and 2
, respectively.
Figure 6a illustrates the variations in
with
, where the total cross-sectional area
of the torsional deformation segment is kept constant. This approach is exemplified in the TT straps by Lord Corporation and the use of metal wire winding in the main rotor TT straps of the Mi-26, resulting in the torsional deformation segment consisting of numerous blocks with a very small width
and thickness
.
Thirdly, all cuboids in the torsional deformation segment can be concentrated towards the center of torsion, reducing the distance from the centroid (
,
) of all cuboids to the center of torsion.
Figure 6b illustrates the variation in
with changes in
and
. This approach is exemplified in the carbon fiber torsion–tension straps of the H145 and the main rotor TT straps of the Mi-26.
Fourthly, the length of the torsional deformation segment (L) can be increased to reduce the maximum value of
, as depicted in
Figure 6c.
Lastly, a gradient reinforcement approach can be employed to alter the distribution of torsional
rigidity, reducing the maximum value at both ends of the torsional deformation segment.
Figure 6d demonstrates the distribution pattern of
. This strategy is implemented in the H145, where a gradually thickening transition segment is introduced between the torsional deformation and the connecting segments.
- (3)
Equations (13) and (14) elucidate the essential design parameters for shear stress and , represented as G, a, b, and L. These formulas are straightforward and do not necessitate illustrative analytical calculations demonstrations or charts. In instances where the additional shear stress due to torsion is significant, several strategies can be employed to mitigate this stress:
Firstly, the selection of materials with a lower shear modulus , such as rubber-based composite materials, can effectively decrease the torsional additional shear stress.
Secondly, increasing the number of segments in the design can effectively reduce the shear stress values for each segment. This method is exemplified by the tail rotor TT straps in the H145.
Another effective strategy is to increase the length of the torsional deformation segment L, which contributes to a reduction in the torsional rate .
Equation (11) identifies the key design parameters influencing the overall torsional stiffness , including E, G, , , , , L, , and n. To address instances of excessive overall torsional stiffness, the following strategies can be implemented:
Firstly, opting for materials with a lower shear modulus G can effectively decrease the free torsional stiffness.
Secondly, employing materials with a lower tensile modulus E can aid in minimizing the torsional bending stiffness.
Thirdly, increasing the number of cuboids can lead to a reduction in both the width and thickness of each cuboid, thereby diminishing the free torsional stiffness and torsional bending stiffness.
Figure 7a depicts the variations in torsional stiffness with changes in the width and thickness.
Fourthly, designing the cross-section to converge towards the torsional center can decrease both the moment of inertia and the radius of gyration, subsequently reducing both the torsional bending stiffness and the centrifugal additional stiffness.
Figure 7b shows the variation in torsional stiffness
with
and
.
Fifthly, elongating the torsional deformation section (L) serves to minimize the torsional angle per unit length, thereby reducing the overall torsional stiffness.
Figure 7c demonstrates how torsional stiffness
varies with changes in L.
- (5)
Equation (16) delineates the critical design parameters for the shear force transmitted to the connection segment, encompassing E, G, , , , , L, , n, and the structure of the connecting segment. To mitigate large shear forces at both ends of the torsional deformation segment, several strategies can be employed:
Firstly, the overall torsional stiffness can be reduced as described previously.
Secondly, the load-bearing capacity of the connecting segment structure can be enhanced by selecting materials with superior shear resistance.
Thirdly, modifying the connecting segment structure can improve its load-bearing ability.
In summary, the tension–torsion bar’s key design parameters include E, G,
,
,
,
, L,
,
,
, n, and the structure of the connecting segment. Both the length of the torsional deformation segment (L) and the material properties are of paramount importance. Accordingly, the authors of this study embarked on experimental research to examine the impact of varying lengths of the deformation segment (L) and material properties. While each key performance metric can be quantitatively assessed using the formulas outlined in
Section 2.3, quantitatively describing the load-bearing capacity of the connecting segment structure remains challenging. As a result, this study incorporates experimental research to evaluate the load-bearing capacity of different connecting segment structures.
4. Experimental Design and Procedure
The experimental research presented in this work includes the following components:
- (1)
Stiffness and stress tests on TT straps were conducted to validate the accuracy of the rapid performance evaluation method. Specifically, strain tests were performed on the TT strap in Case 2.
- (2)
Aiming to examine the effect of the critical parameter, the length of the torsional deformation segment L, TT straps with varying lengths of this segment were designed and tested for stiffness comparison, covering Cases 1, 2, and 3. Additionally, TT straps made from two different materials were compared to assess the influence of material on the overall torsional stiffness, involving Cases 3 and 4. Lastly, because it is challenging to parametrically quantify the load-bearing capacity of the connecting segment structure, the experimental analysis of the load-bearing capacities of various connecting segment structures was explored. The connecting segments in Cases 1, 2, and 3 were sequentially reinforced to enhance the resistance to shear forces
. The framework of this chapter is illustrated in
Figure 8.
4.1. The Design Process of Key Parameters for the Test Specimen
An experimental design was implemented based on a TT strap configured to undergo a 35° twist under a centrifugal force of 400,000 N. In this design, the length of the TT strap at the torsional deformation segment is limited to 300 mm, with a maximum height of 35 mm. The overall torsional stiffness of the TT strap is kept within a defined threshold of 35 Nm/°.
- (1)
The design inputs are specified as:
The centrifugal force is 400,000 N;
The maximum torsional angle of the blades is 35°.
- (2)
The design requirements are mathematically expressed as follows:
- (3)
The initial structural input parameters required for the test specimen are as follows:
E: Material tensile modulus;
G: Material shear modulus;
: Distance from the centroid of the cuboid to the torsion center along the zt-direction;
: Distance from the centroid of the cuboid to the torsion center along the yt-direction;
: Width of the cross-section of the cuboids;
: Thickness of the cross-section of the cuboids;
L: Length of the torsional deformation segment;
: Total cross-sectional area of the torsional deformation segment;
: Material tensile strength limit;
: Material shear strength limit;
n: Number of subdivisions in the cross-section of the torsional deformation segment.
- (4)
Initial structural parameter determination:
The internal diameter of the bushing was determined to be 28 mm, with a wall thickness of 2 mm, based on centrifugal force and the metal’s shear resistance. Materials with a high tensile strain limit, such as glass-fiber-reinforced composite materials and Kevlar-reinforced rubber composite materials, were selected based on Equation (13). For Cases 1 to 3, the TT straps were made out of 3232A/S4C10-800 glass-fiber-reinforced resin-based composite material without a weft band, featuring a tensile modulus E of 50 GPa, a shear modulus G of 4.9 GPa, a tensile limit of 1500 MPa, and a shear strength limit of 90 MPa. Case 4 used Kevlar-fiber-reinforced rubber composite material with a modulus E of 70 GPa and a shear modulus of 0.05 GPa.
Considering a safety margin of 1× as per Equation (12), the typical sectional area was ensured to be no less than 533 mm2, with a preliminary set value of 600 mm. Given the outer diameter of the bushing and the height constraints of the TT strap, the initial width of the cuboids was set to 10 mm. The number of subdivisions in the typical section’s cross-section was initially set to 2, and the thickness of cuboids was 30 mm. Consequently, the distances from the centroid of the cuboid to the torsion center and were preliminarily determined to be 0 and 25 mm, respectively. The length of the TT strap’s typical segment L was preliminarily set to 300 mm, considering the length constraints, the dimensions of the bushing, and the width of the strap element.
Using these preliminary parameters and following the key performance design method outlined in
Section 3, the key parameters were adjusted to meet design requirements, excluding the load-bearing capacity of the connecting segment, which is typically assessed through experimentation due to the complexity and diversity of stresses and configurations in the connecting segment.
4.2. Design Results for the Test Specimens
During the experimental process, different configurations of the length of the torsional deformation segment L, different materials, and different connecting segment structures were planned on four test specimens to evaluate their influence on the key performances.
- (1)
Design results of the torsional deformation segment
The parameter design results of the torsional deformation segment of the four schemes are shown in
Table 1.
Here, Lbh is the distance between two bushing holes.
The calculation results for the four schemes are presented in
Table 2. These results indicate that all four schemes generally meet the design requirements. However, it is noted that the tensile stress in Case 3 slightly exceeded the material’s tensile strength limit.
- (2)
Design of the connecting segment
During the experimental process, different structures of the connecting segment were planned on Cases 1 to 3 to evaluate their capacity to withstand shear forces induced by eccentric torsional bending. Specifically, this involved the following:
- (1)
The load-bearing capacity was enhanced in Case 2 compared to Case 1 by increasing the length of the glass fabric layers in the connection segment by 10 mm and adding two additional layers.
- (2)
Compared to Case 2, the length of the glass fabric layers was increased by an additional 10 mm, and the number of layers was increased by 12. Furthermore, an I-shaped bushing was utilized in Case 3, which converts part of the shear force in the connection segment into compressive stress, thereby further enhancing its load-bearing capacity.
The key detailed structures of the connection segment of the three schemes are presented in
Table 3. Here, D
ib is the internal diameter of the bushing, and D
ob is the external diameter of the bushing.
4.3. Experimental Procedure and Equipment
The clamping method for the test specimens is as follows:
- (1)
One connecting segment was fixed to the load-bearing wall.
- (2)
The other connecting segment was connected to the centrifugal force and torque-loading end.
The experimental setup is illustrated in
Figure 9. During the experiment, an angle measurement sensor and a torque sensor were both installed at the loading end. The loading system utilized the MTS Flextest 100 coordinated load control system, which is capable of outputting both the torque and torsional angle.
Eight tensile test strain sensors (labeled S01–S08) were arranged on the TT strap, as shown in
Figure 10.
The tests for stiffness and torsional capacity were conducted separately. First, the stiffness tests were performed for Cases 1 to 4. After the stiffness tests were complete, the torsional capacity tests were carried out for Cases 1 to 3.
The stiffness test was conducted as follows:
- (1)
Initially, torsional displacement was applied in the absence of centrifugal force, commencing from 0° and incrementing in one direction by 1° up to 12°. Subsequently, the displacement was reversed, decreasing by 1° back to 0°.
- (2)
The centrifugal force was applied, starting from 0 and increasing by increments of 10,000 N up to a maximum of 170,000 N. At this centrifugal force, torsional displacement was again applied as in Step 1, followed by unloading.
- (3)
The final step involved progressively increasing the centrifugal force from 0 in 10,000 N increments until reaching up to 270,000 N. Under this force, torsional displacement was applied as per Step 1 and then unloaded.
Considering the potential impact of the loading speed and frequency on the occurrence of jumping resonance in experimental testing, as noted in [
13], in this experiment, we adopted a slow loading approach. Data collection was initiated only after ensuring that the measurement points required for data acquisition remained stable for a sufficient period. Each of these steps was replicated three times. The choice of the maximum angle in stiffness testing aimed to mitigate the influence of the installation gaps and to avert potential damage to the TT straps during testing.
The torsional capability testing comprised the following steps:
- (1)
The centrifugal force was incrementally increased from 0 by 10,000 N intervals until it reached up to 400,000 N, and this load was sustained for 3 s.
- (2)
With the centrifugal force constant, torsional angular displacement was then applied, escalating in 1° increments until either specimen failure or a torsional angle of 50° was achieved.
- (3)
In the event of no failure in the second step, the torsional angle was maintained at 50°, and the centrifugal force was further increased in 10,000 N increments until specimen failure occurred.
6. Conclusions
This study carried out an analytical and experimental investigation into the key performance design of TT straps. The initial phase involved deriving a general solution for the torsional response of the TT strap under specified torque, leading to the development of calculation formulas that clearly link key parameters with performance. Consequently, a rapid evaluation method for the performance of the torsional deformation segment was established. Further, this study provides an in-depth analysis of the crucial design parameters for the TT strap, coupled with a practical case study showcasing the method’s application in design. To assess the influence of variables such as the length, material, and connecting segment structure on the TT strap’s performance, four torsion bars were meticulously designed and rigorously tested. The conclusions drawn are as follows:
The experimental findings robustly corroborate the precision of the rapid assessment method introduced in this research, particularly concerning stiffness and stress, thereby validating its efficacy in capturing key parameters.
An experimental investigation into the load-bearing capacities of the connecting segments was conducted. This study elucidated the failure mechanisms in three distinct connecting segment designs of TT straps and their correlations with the shear force F1 as assessed by the rapid evaluation method. This method significantly contributes to enhancing the optimization of connecting segment configurations.
The outcomes of this research provide a practical, rapid evaluation method for key performance evaluation and valuable experimental insights for the expedited design of TT straps in engineering applications. This approach not only fosters enhanced design efficiency and cost-effectiveness but also offers a pertinent reference for the performance evaluation and optimization of various structural components.