On the Relative Kinematics and Control of Dual-Arm Cutting Robots for a Coal Mine
Abstract
:1. Introduction
- An advanced dual-arm tunneling robotic system for coal mines is developed in this research. This system can achieve the synchronous operation of excavation and the permanent support of laneways to efficiently complete excavation tasks for large-section coal/rock laneways.
- The proposed relative kinematic model of the DACR counteracts the influence of shared motion by integrating the kinematics of both arms into a unified framework. It can simultaneously describe the motion states of the two cutting arms. Additionally, a closed-loop kinematic controller for the robot is developed based on this relative kinematics, enabling control of both cutting arms through a single parameter.
- The AW for the DACR is presented, and this can provide a reference for cutting trajectory planning of the two cutting arms.
2. Proposed Dual-Arm Tunneling Robotic System
3. Relative Kinematics of the DACR
3.1. Relative Kinematics Model of the DACR
3.2. AW Generation Algorithm
- According to the actual size of the DACR, the variable range of the kth joint of the DACR is specified as (qmink, qmaxk).
- N random values in the interval (0, 1) can be generated by using the function rand(N, 1). The random step size generated by each joint is denoted as .
- The end-effectors of the DACR are plotted in Cartesian space at random positions by substituting into and in Equation (3). The contour of the AW is generated when the number of random samples N is sufficiently large. It should be noted that the larger the value of N is, the more accurate the depiction of the contour of the AW for the DACR.
| Algorithm 1. AW generation algorithm of the DACR. |
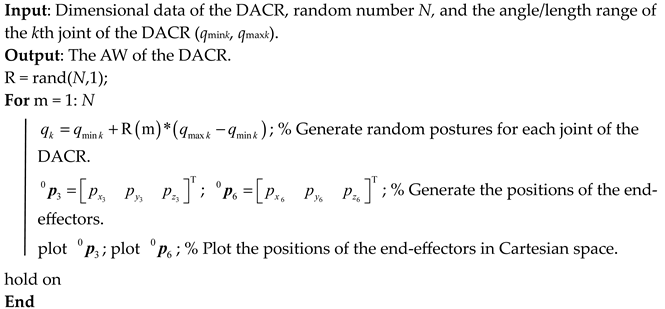 |
4. Proposed Kinematics Controller
5. Simulation Analysis and Discussion
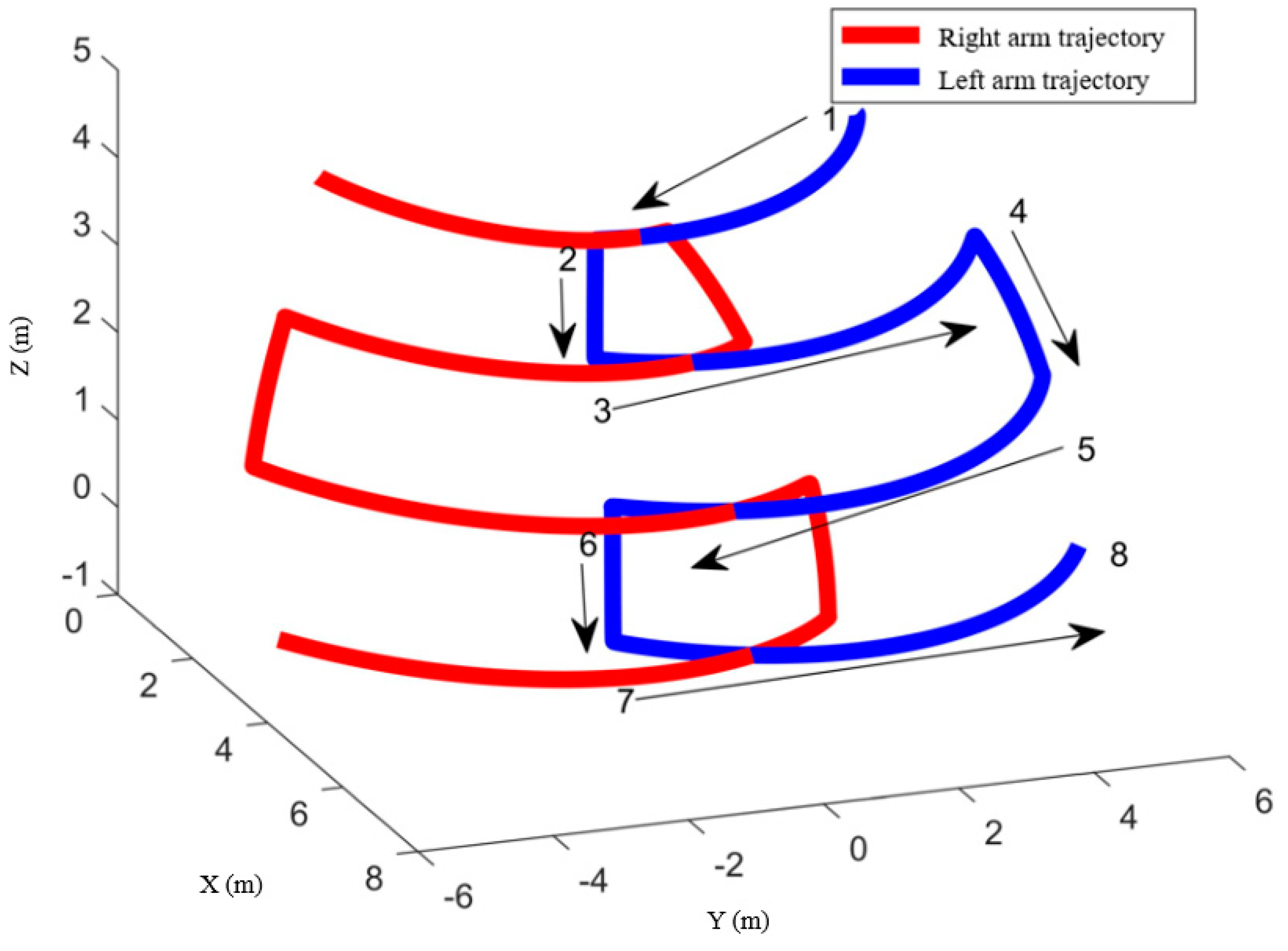
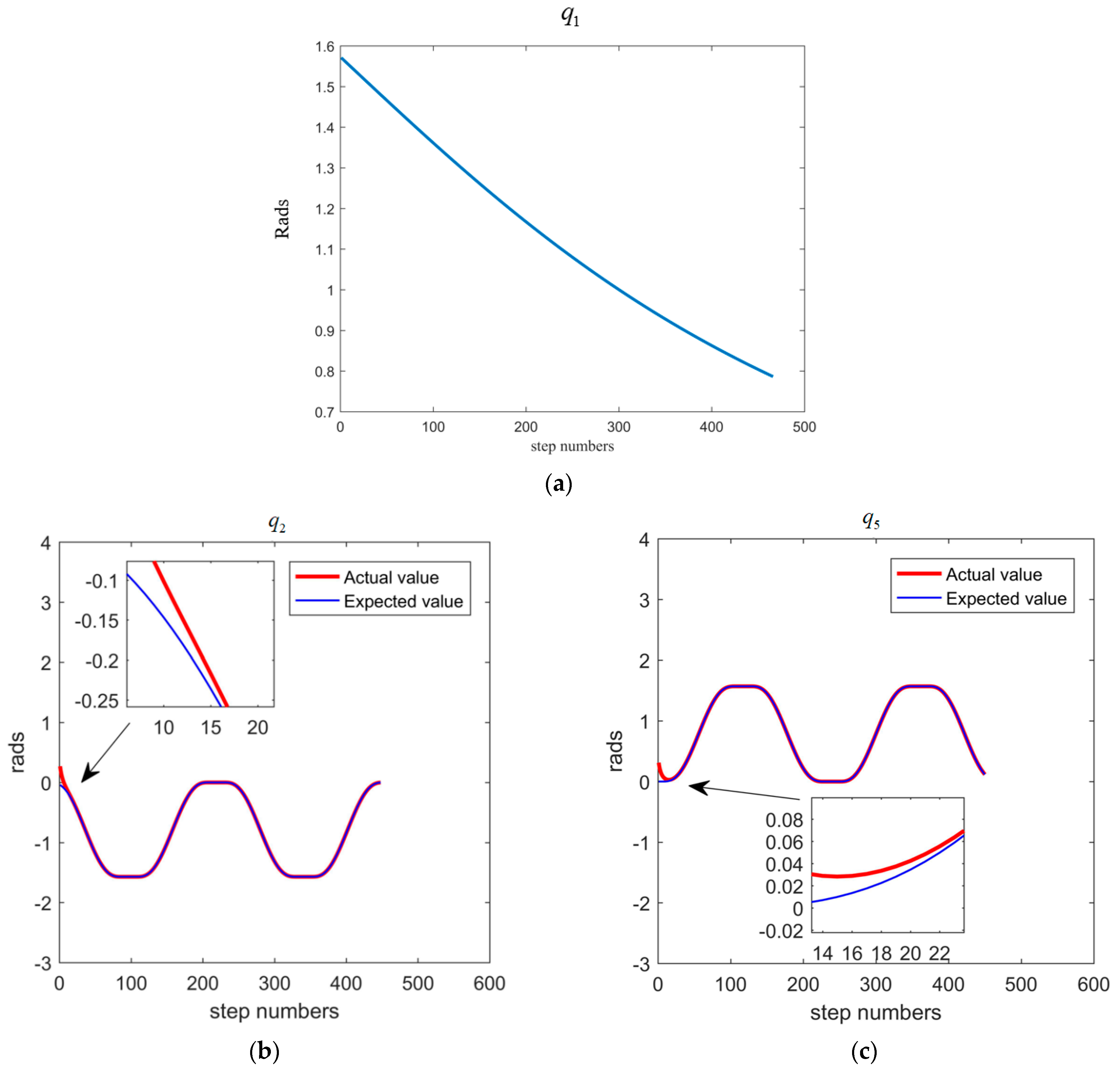
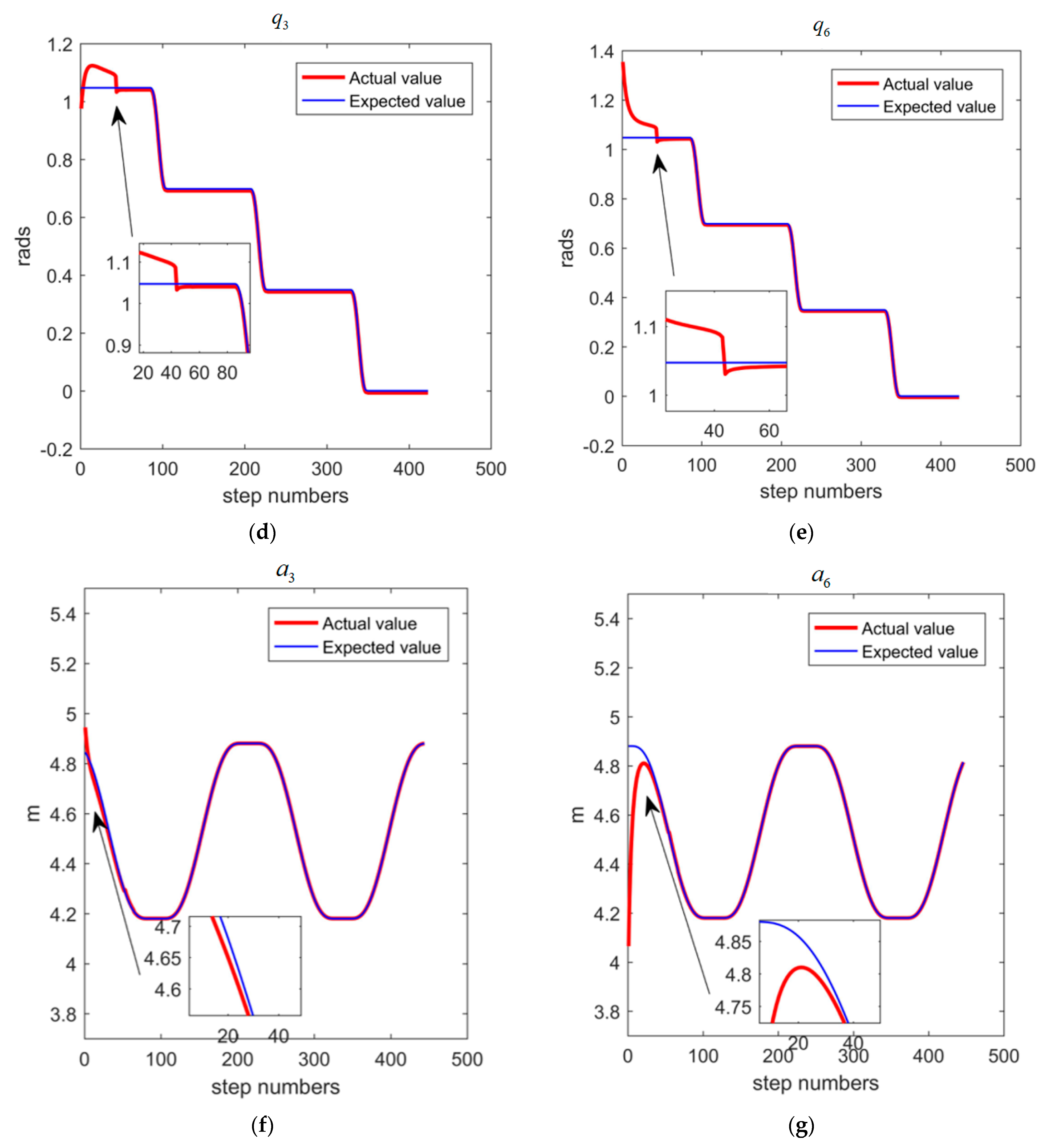
5.1. Motion Continuity of the DACR
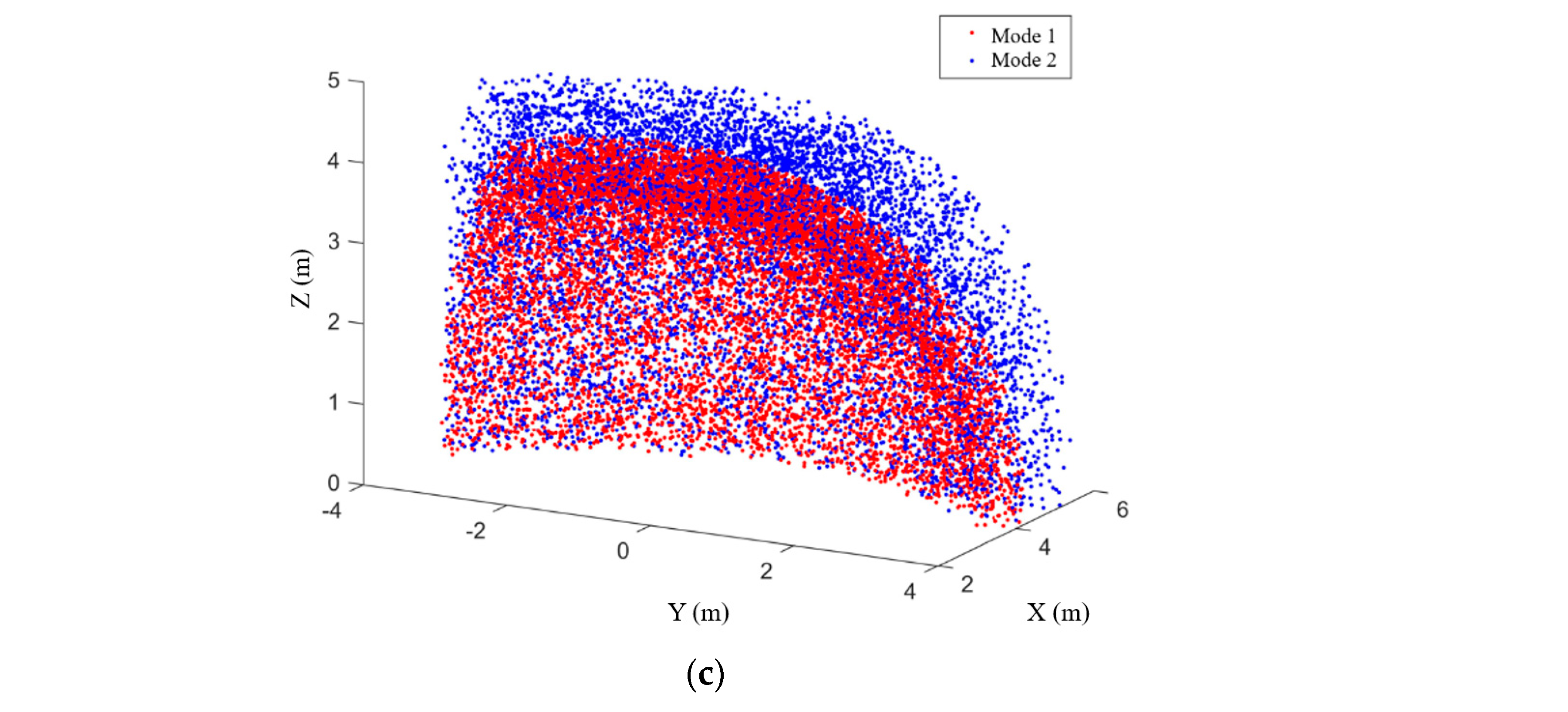
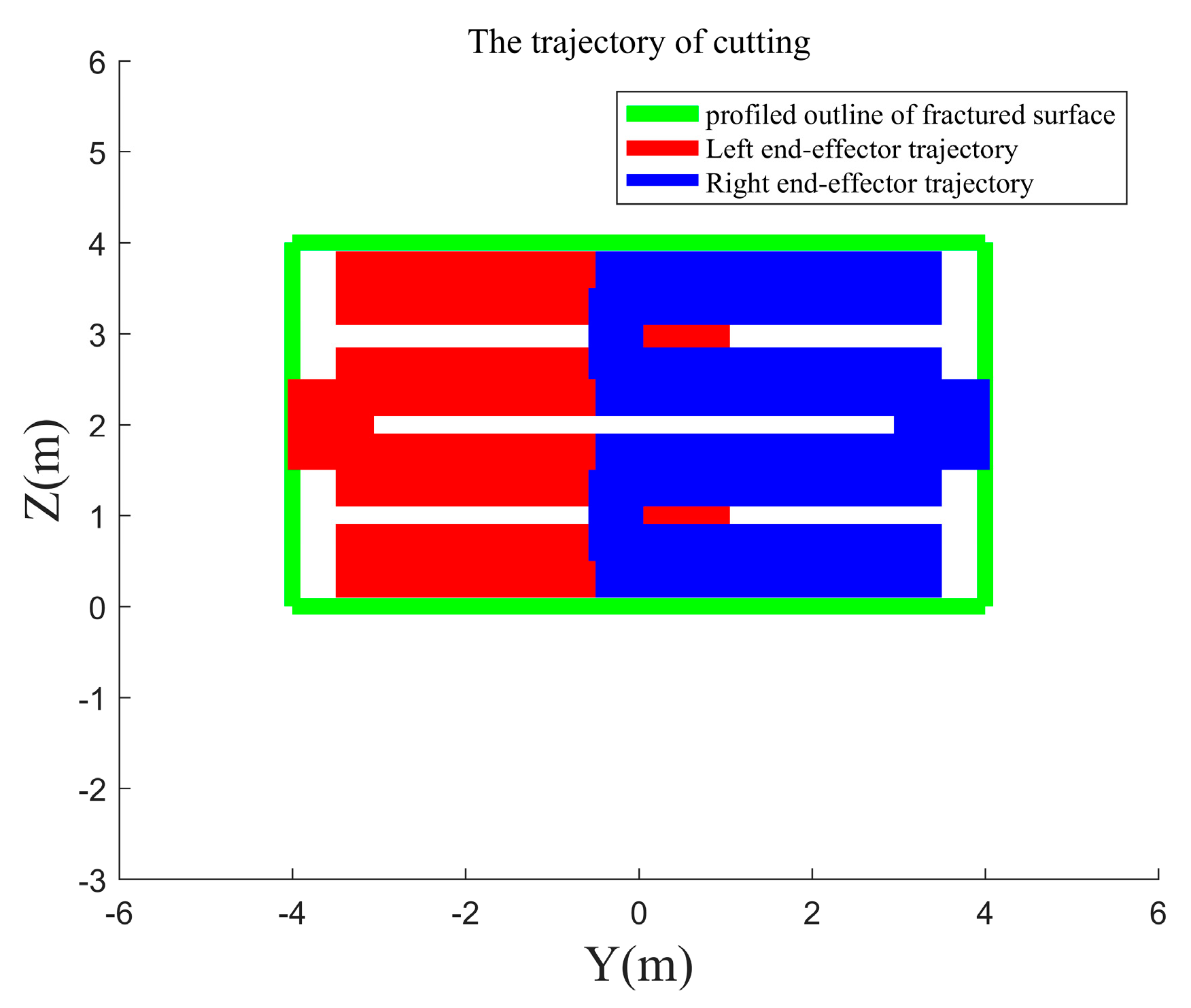
5.2. AW of the DACR
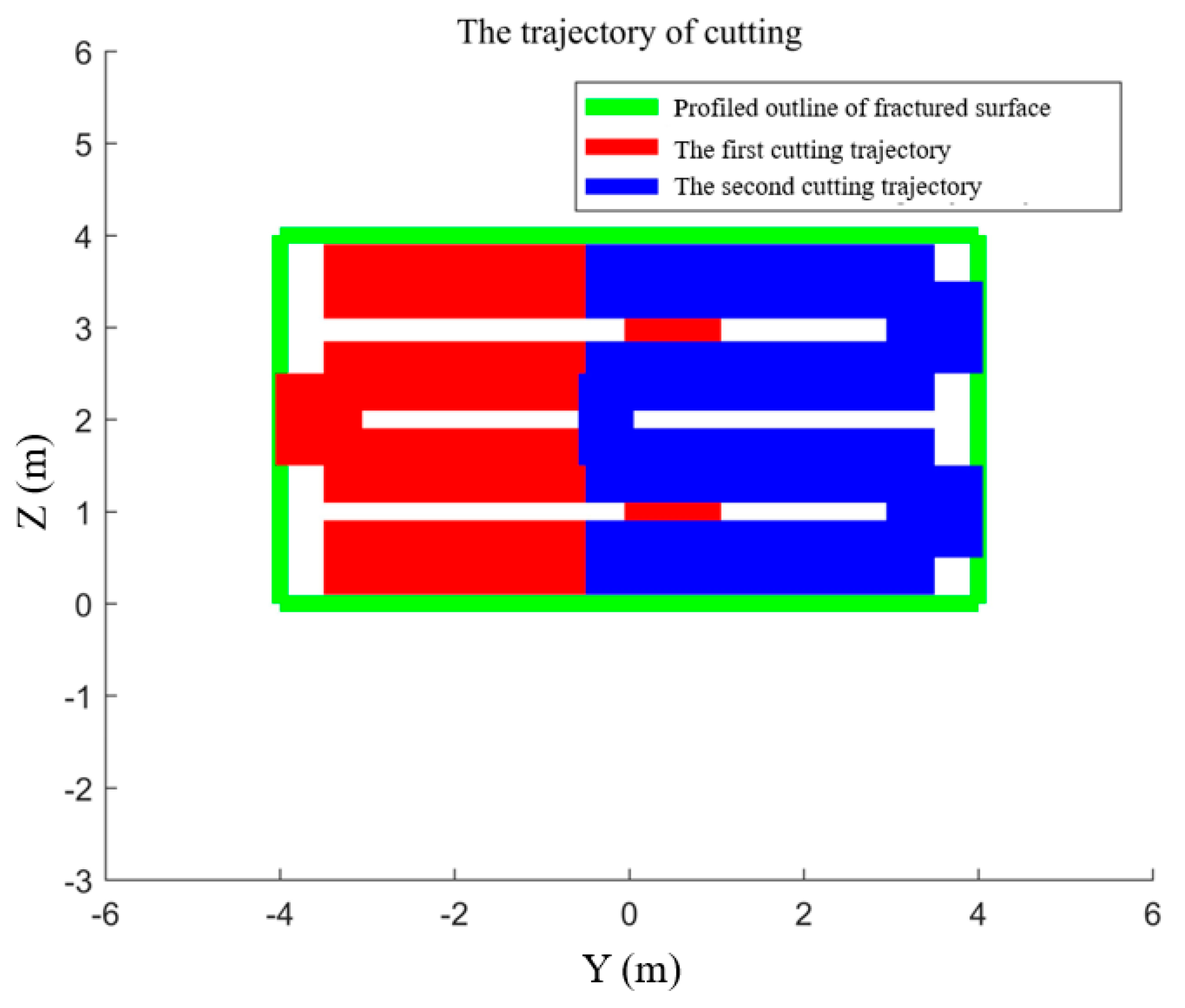
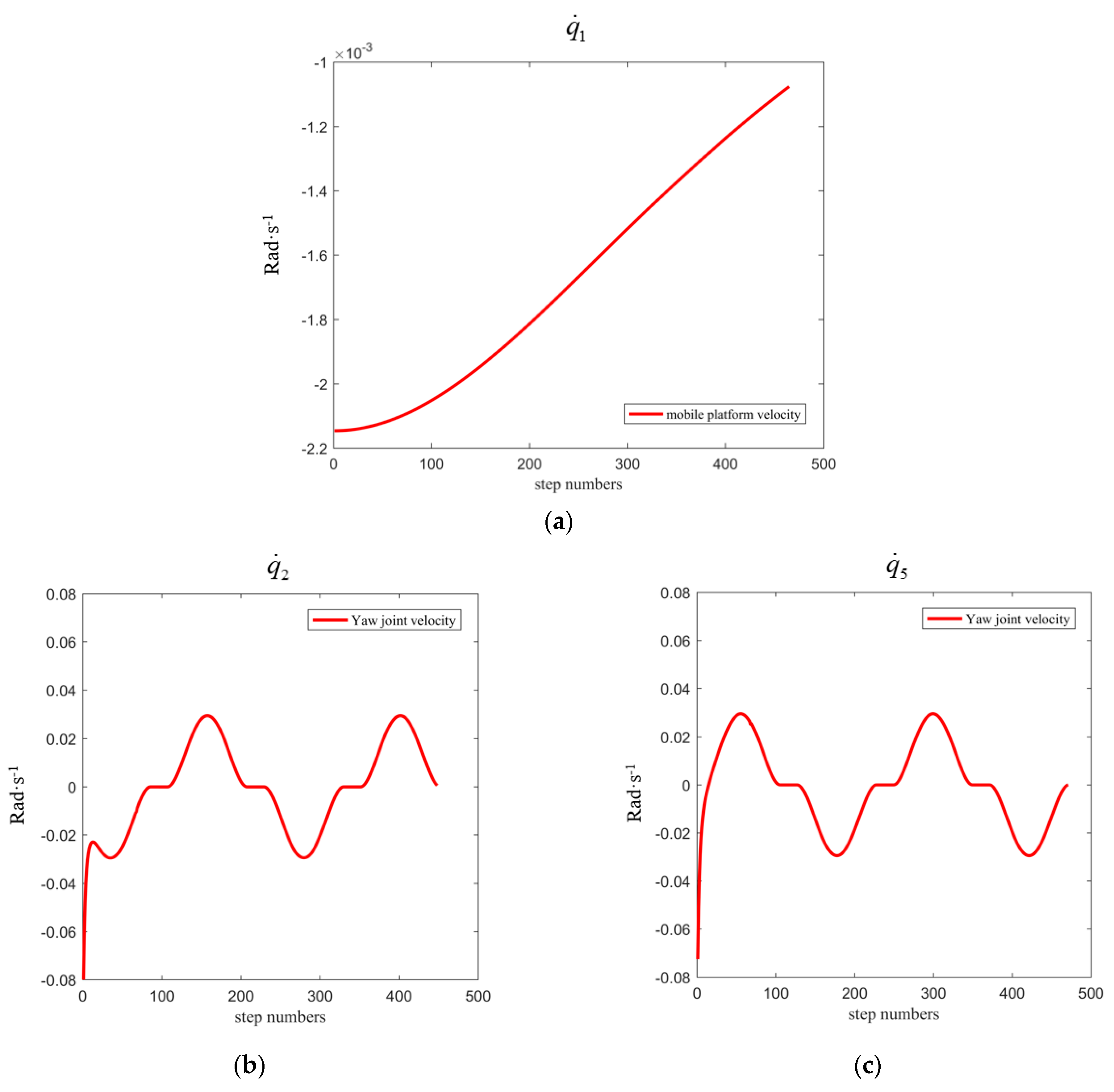
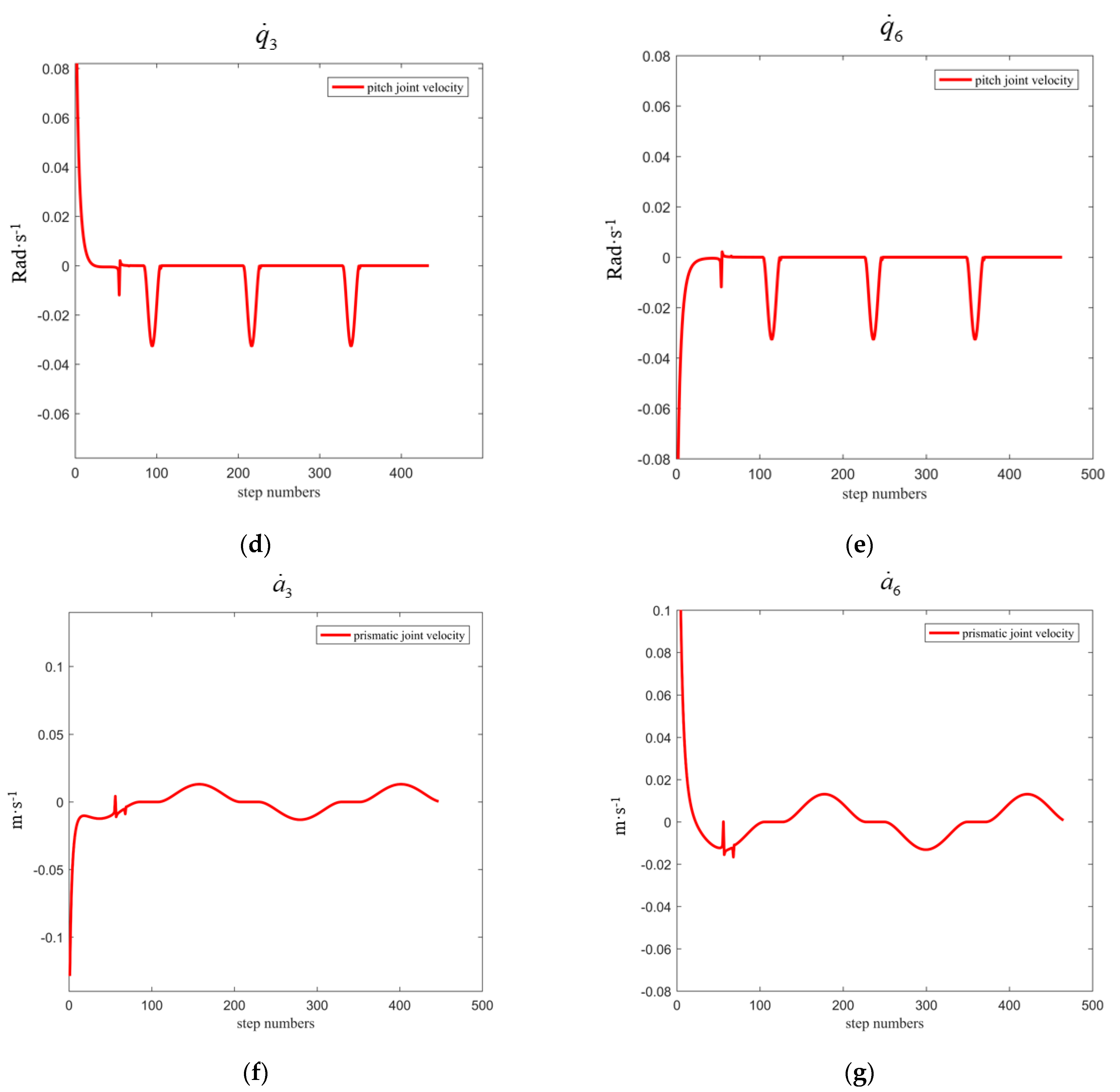
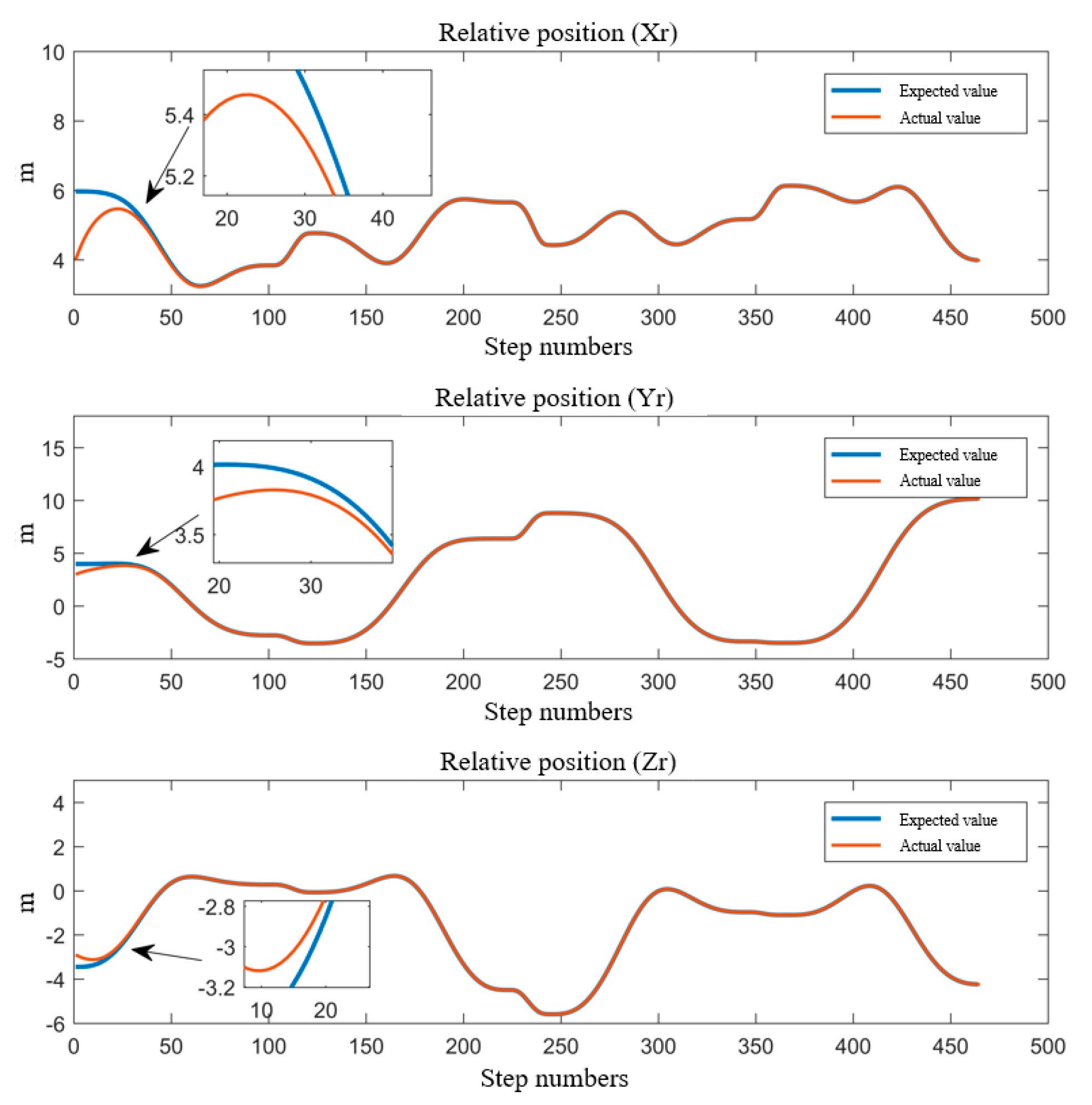
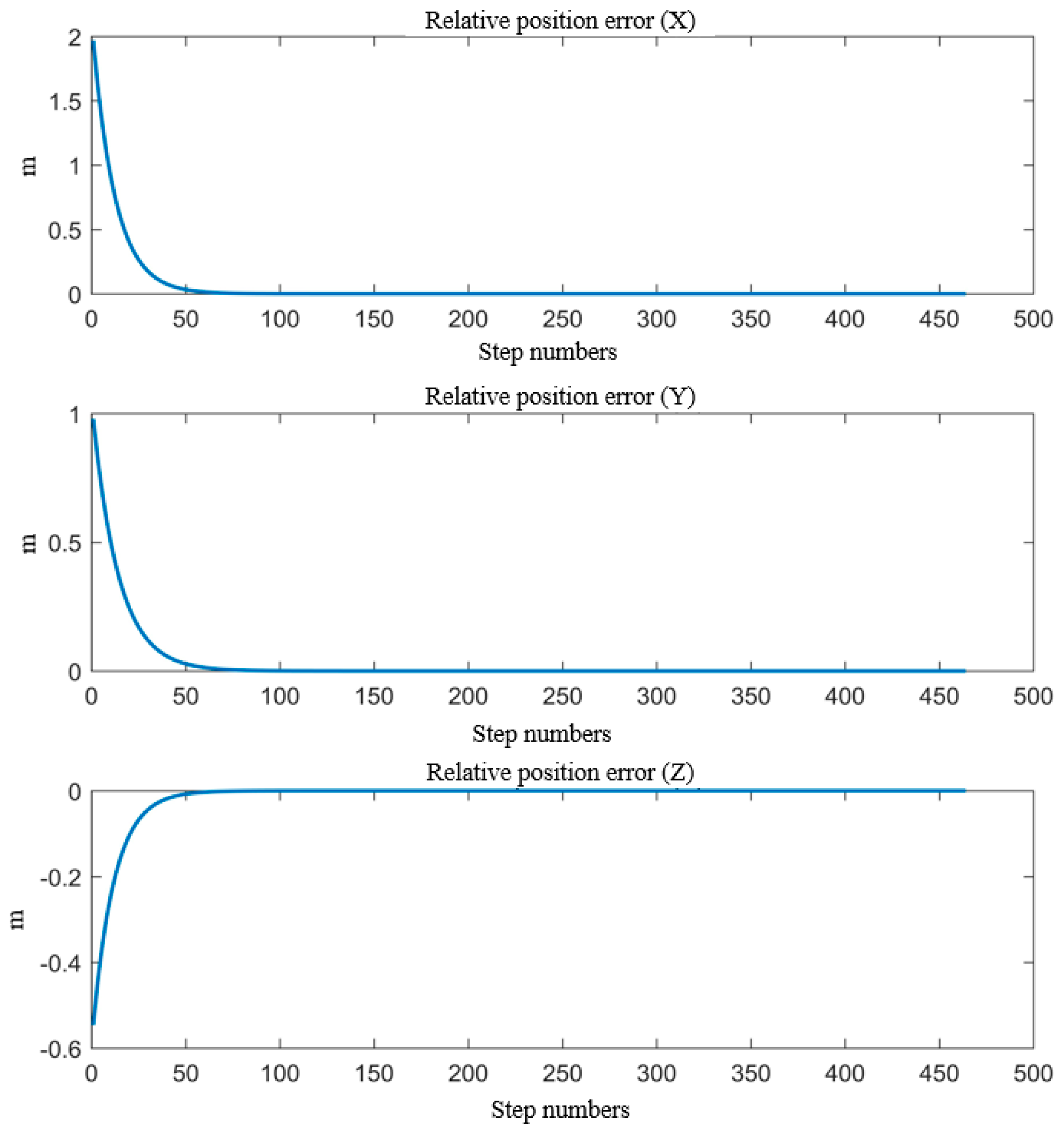
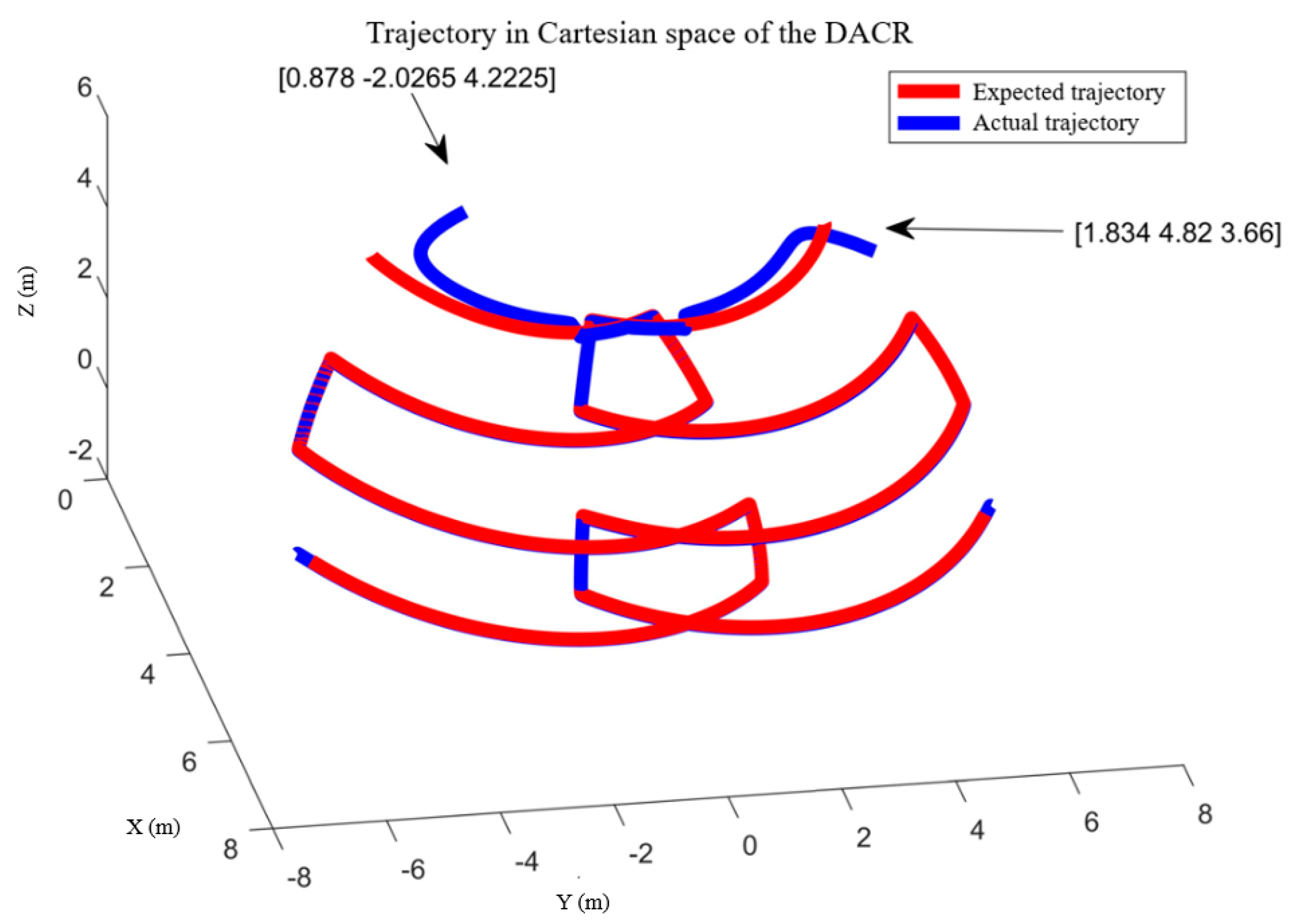
5.3. Trajectory Tracking Control System
6. Conclusions
- An advanced dual-arm tunneling robotic system for a coal mine is developed, and the main components and characteristics of the robotic system are presented in this paper. The major benefit of this type of robotic system is the achievement of the synchronous operation of excavation and permanent support of laneways.
- The relative kinematic model of the DACR is established. This model integrates the independent kinematics of both arms into a unified framework, allowing the simultaneous description of the motion states of both arms using only one variable. Additionally, a control strategy is proposed based on relative kinematics, enabling simultaneous control of both cutting arms by using a single variable. Furthermore, the AW of the DACR is generated and proposed by a Monte Carlo algorithm.
- The simulation of the motion continuity of the DACR validates the correctness of its relative kinematics model. The status of the DACR is studied for two typical working modes, and the simulation of the AW verifies that the DACR has a larger workspace and reduces the excavation process of large-sized cross-section laneways, thereby improving mining efficiency. Finally, the simulation of relative kinematic control selects the more suitable mode 2 and demonstrates the effectiveness of the proposed control strategy. The DACR converges to the desired trajectory within 50 steps, with an absolute error in the relative positions between the two arms and the desired relative positions of less than 0.01837 m, and the root mean square error of the angle/length of each joint is maintained at a small value. This indicates that the DACR can achieve precise feedback control with good error correction effect and response speed, with minimal fluctuations after trajectory tracking stabilization. This provides a theoretical foundation for the design, analysis, and future implementation of dynamic control for the proposed DACR.
- Compared to other dual-arm robots, the advantage of the DACR’s control strategy lies in the proposed relative position controller’s ability to track changes in the relative positions of the end-effectors. In contrast, conventional dual-arm robots primarily focus on operations such as transporting, gripping, and lifting objects, where the relative positions of the two end-effectors remain constant. Therefore, the proposed controller exhibits greater versatility and can serve as a reference for future dual-arm robot control systems.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tang, Y.; Tang, J.; Wang, S.; Wang, S. Analysis of rock cuttability based on excavation parameters of TBM. J. Geomech. Geophys. Geo-Energy Geo-Resour. 2023, 9, 93. [Google Scholar] [CrossRef]
- Zheng, Y.; He, L. TBM tunneling in extremely hard and abrasive rocks: Problems, solutions and assisting methods. J. Cent. South Univ. 2021, 28, 454–480. [Google Scholar] [CrossRef]
- Zhang, X.; Zheng, X.; Yang, W.; Li, Y.; Ma, B.; Dong, Z.; Chen, X. Research on path planning method of underground mining robot. J. Coal Geol. Explor. 2024, 52, 1–13. [Google Scholar] [CrossRef]
- Zhang, Y.; Huang, Q.; Liao, Q.; Yang, X.; Wei, S. Research on the novel method for inverse kinematics analysis of spatial 6R serial manipulators. J. Mech. Eng. 2022, 58, 1–11. [Google Scholar] [CrossRef]
- Vavro, J.; Vavro, J.; Marček, L.; Taraba, M.; Klimek, L. Kinematic and dynamic analysis and distribution of stress for six-item mechanism by means of the SolidWorks program. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1199, 012047. [Google Scholar] [CrossRef]
- Kheylo, S.V.; Garin, O.A.; Terekhova, A.N.; Prokhorovich, V.E.; Dukhov, A.V. Solving problems of the dynamics of a manipulator with six degrees of freedom. J. Mach. Manuf. Reliab. 2022, 51, 30–36. [Google Scholar] [CrossRef]
- Sun, X.; Wang, H.; Guo, X.; He, X. Kinematics study on redundant manipulator with serial-parallel hybrid mechanism of bolter-drilling rig. Mech. Sci. Technol. Aerosp. Eng. 2023, 42, 493–503. [Google Scholar] [CrossRef]
- Wang, X.; Shi, L.; Katupitiya, J. Robust control of a dual-arm space robot for in-orbit screw-driving operation. Acta Astronaut. 2022, 200, 139–148. [Google Scholar] [CrossRef]
- Ouyang, Y.; Sun, C.; Dong, L. Actor–critic learning based coordinated control for a dual-arm robot with prescribed performance and unknown backlash-like hysteresis. ISA Trans. 2022, 126, 1–13. [Google Scholar] [CrossRef]
- Shen, Y.; Fan, G.; Tang, X.; Dong, Y.; Deng, L. Gaussian process based differential kinematics control of the soft robot. Control Eng. China 2021, 28, 1360–1365. [Google Scholar] [CrossRef]
- Guo, J.; Liu, J. Workspace analysis and structure optimization of parallel robot. Manuf. Technol. Mach. Tool 2022, 11, 20–26. [Google Scholar] [CrossRef]
- Wang, J.; Xu, J. Kinematic modeling and simulation of dual-arm robot. J. Robot. Netw. Artif. Life 2021, 8, 56–59. [Google Scholar] [CrossRef]
- Lee, S.H.; Lee, Y.J.; Kim, D.H. Extension of inverse kinematic solution for a robot to cope with joint angle constraints. Int. J. Control Autom. Syst. 2023, 21, 1899–1909. [Google Scholar] [CrossRef]
- Yang, S.; Wen, H.; Hu, Y.; Jin, D. Coordinated motion control of a dual-arm space robot for assembling modular parts. Acta Astronaut. 2020, 177, 627–638. [Google Scholar] [CrossRef]
- Lei, M.; Wang, T.; Yao, C.; Liu, H.; Wang, Z.; Deng, Y. Real-time kinematics-based self-collision avoidance algorithm for dual-arm robots. Appl. Sci. 2020, 10, 5893. [Google Scholar] [CrossRef]
- Du, H.; Hu, N. Kinematics and dynamics analysis of lower limb robot based on human motion data. J. Mech. Transm. 2022, 46, 128–134. [Google Scholar] [CrossRef]
- Ahmed, A.; Ju, H.; Yang, Y.; Xu, H. An improved unit quaternion for attitude alignment and inverse kinematic solution of the robot arm wrist. Machines 2023, 11, 669. [Google Scholar] [CrossRef]
- Jiang, Z.; Yu, Z.; Zhang, X. Research on the inverse kinematic algorithm of the robot arm of the minecart. J. Phys. Conf. Ser. 2023, 2483, 012012. [Google Scholar] [CrossRef]
- Wan, Y.; Li, H.; Chen, X. Kinematics analysis and visualization simulation of 6-DOF intelligent cooperative robot. J. Phys. Conf. Ser. 2022, 2283, 012013. [Google Scholar] [CrossRef]
- Wei, Y. Kinematics analysis and modelling based on 4 DOF industrial robot. J. Phys. Conf. Ser. 2023, 2562, 012076. [Google Scholar] [CrossRef]
- Meng, Z.; Cao, W.; Ding, H.; Chen, Z. A new six degree-of-freedom parallel robot with three limbs for high-speed operations. Mech. Mach. Theory 2022, 173, 104875. [Google Scholar] [CrossRef]
- Huang, Y.; Li, Z.; Xing, K.; Gong, H. A manipulator size optimization method based on dexterous workspace volume. Cobot 2022, 1, 3. [Google Scholar] [CrossRef]
- Bader, A.M.; Maciejewski, A.A. The kinematic design of redundant robots for maximizing failure-tolerant workspace size. Mech. Mach. Theory 2022, 173, 104850. [Google Scholar] [CrossRef]
- Meng, Q.; Fei, C.; Jiao, Z.; Xie, Q.; Dai, Y.; Fan, Y.; Shen, Z.; Yu, H. Design and kinematical performance analysis of the 7-DOF upper-limb exoskeleton toward improving human-robot interface in active and passive movement training. Technol. Health Care 2022, 30, 1167–1182. [Google Scholar] [CrossRef]
- Liu, C.; Gao, B.; Yu, C.; Tapus, A. Self-protective motion planning for mobile manipulators in a dynamic door-closing workspace. Ind. Rob. 2021, 48, 803–811. [Google Scholar] [CrossRef]
- Wang, X.-Y.; Ding, Y.-M. Method for workspace calculation of 6R serial manipulator based on surface enveloping and overlaying. J. Shanghai Jiaotong Univ. Sci. 2010, 15, 556–562. [Google Scholar] [CrossRef]
- Zeng, Q.; Zhou, G.; Shi, J.; Li, Y.; Liu, Y.; Chen, T.; Wang, L. Robot workspace optimization based on Monte Carlo method and multi island genetic algorithm. Mechanics 2022, 28, 308–316. [Google Scholar] [CrossRef]
- Li, J.; Zhao, Z.; Zhang, S.; Su, C. Dynamics and workspace analysis of a multi-robot collaborative towing system with floating base. J. Mech. Sci. Technol. 2021, 35, 4727–4735. [Google Scholar] [CrossRef]
- Boanta, C.; Brișan, C. Estimation of the kinematics and workspace of a robot using artificial neural networks. Sensors 2022, 22, 8356. [Google Scholar] [CrossRef]
- Xu, Z.; Zhao, Z.; He, S.; He, J.; Wu, Q. Improvement of Monte Carlo method for robot workspace solution and volume calculation. Opt. Precis. Eng. 2018, 26, 2703–2713. [Google Scholar] [CrossRef]
- Su, C.; Li, J.; Ding, W.; Zhao, Z. Static workspace analysis of a multi-robot collaborative towing system with floating base. Int. J. Model. Simul. Sci. Comput. 2021, 12, 2150009. [Google Scholar] [CrossRef]
- Li, T.; Jia, Q.; Chen, G.; Sun, H. Motion reliability assessment of robot for trajectory tracking task. Syst. Eng. Electron. 2014, 36, 2556–2561. [Google Scholar] [CrossRef]




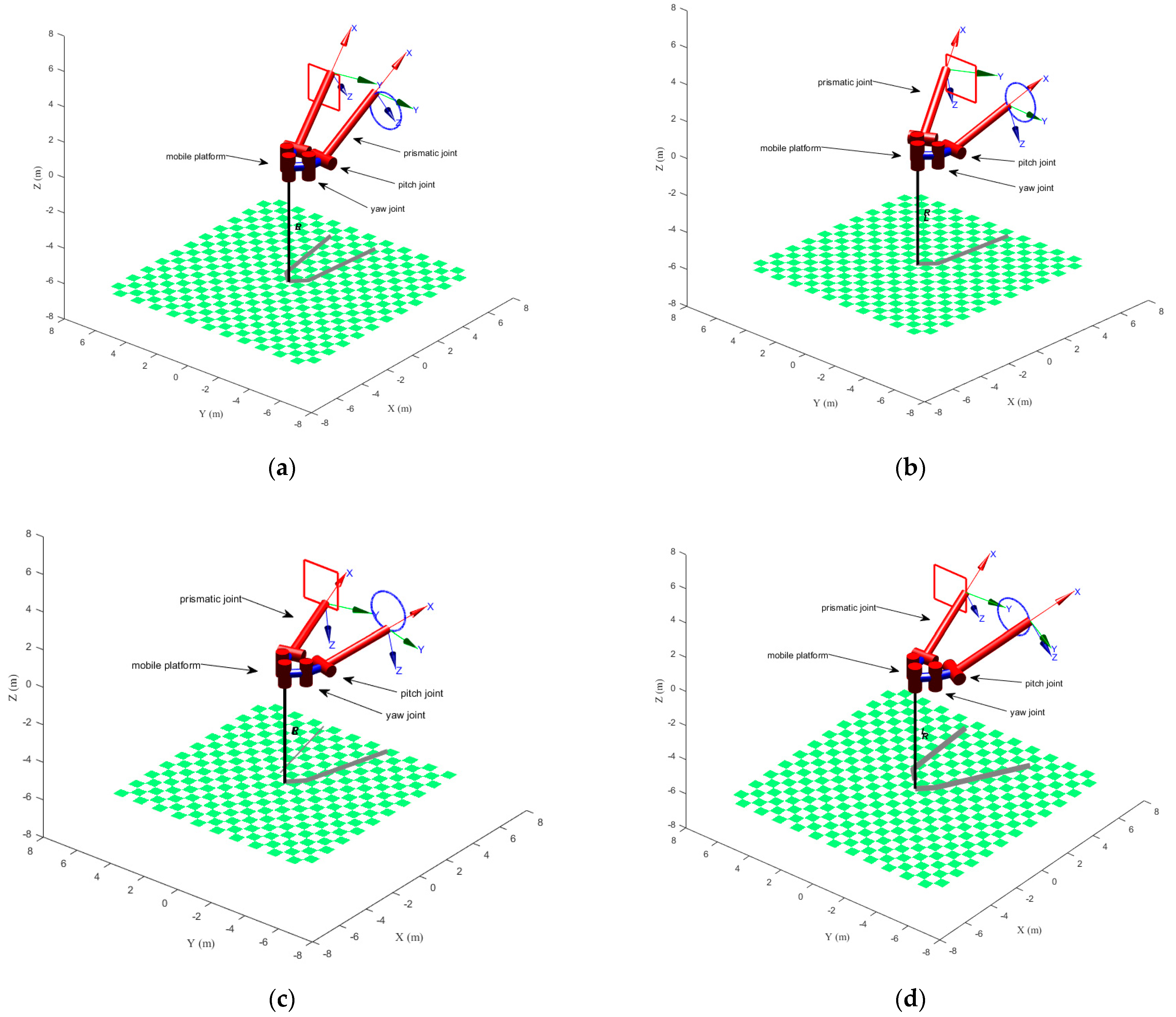



| Link | qi/rads | di/m | ai/m | αi/rads |
|---|---|---|---|---|
| i = 1 | q1 | 0 | a1 | 0 |
| i = 2 | q2 | 0 | a2 | π/2 |
| i = 3 | q3 | 0 | a3 | 0 |
| i = 4 | q4 | 0 | a4 | 0 |
| i = 5 | q5 | 0 | a5 | π/2 |
| i = 6 | q6 | 0 | a6 | 0 |
| Point | Base/m | q2/rads | q3/rads | a3/m | q5/rads | q6/rads | a6/m |
|---|---|---|---|---|---|---|---|
| 1 | 0 | 0 | π/3 | 4.88 | 0 | π/3 | 4.88 |
| 2 | 0.2174 | −π/2 | π/3 | 4.08 | π/2 | π/3 | 4.08 |
| 3 | 0.2609 | −π/2 | 2π/9 | 4.08 | π/2 | 2π/9 | 4.08 |
| 4 | 0.4783 | 0 | 2π/9 | 4.88 | 0 | 2π/9 | 4.88 |
| 5 | 0.5217 | 0 | π/9 | 4.88 | 0 | π/9 | 4.88 |
| 6 | 0.7391 | −π/2 | π/9 | 4.08 | π/2 | π/9 | 4.08 |
| 7 | 0.7826 | −π/2 | 0 | 4.08 | π/2 | 0 | 4.08 |
| 8 | 1 | 0 | 0 | 4.88 | 0 | 0 | 4.88 |
| q2/rads | q3/rads | a3/m | q5/rads | q6/rads | a6/m | |
|---|---|---|---|---|---|---|
| RMSE | 0.0032 | 0.0149 | 0.0136 | 0.0053 | 0.0127 | 0.0125 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, P.; Zhou, H.; Qiao, X.; Zhu, Y. On the Relative Kinematics and Control of Dual-Arm Cutting Robots for a Coal Mine. Actuators 2024, 13, 157. https://doi.org/10.3390/act13050157
Liu P, Zhou H, Qiao X, Zhu Y. On the Relative Kinematics and Control of Dual-Arm Cutting Robots for a Coal Mine. Actuators. 2024; 13(5):157. https://doi.org/10.3390/act13050157
Chicago/Turabian StyleLiu, Peng, Haochen Zhou, Xinzhou Qiao, and Yan Zhu. 2024. "On the Relative Kinematics and Control of Dual-Arm Cutting Robots for a Coal Mine" Actuators 13, no. 5: 157. https://doi.org/10.3390/act13050157
APA StyleLiu, P., Zhou, H., Qiao, X., & Zhu, Y. (2024). On the Relative Kinematics and Control of Dual-Arm Cutting Robots for a Coal Mine. Actuators, 13(5), 157. https://doi.org/10.3390/act13050157







