A New Active Disturbance Rejection Control Tuning Method for High-Order Electro-Hydraulic Servo Systems

Abstract

In our industry, active disturbance rejection control already has been used to enhance the performance of the electro-hydraulic servo systems, despite the fact that electro-hydraulic servo systems are usually reduced to first-order and second-order systems. The aim of this paper is to extend the application of active disturbance rejection control to high-order electro-hydraulic servo systems by introducing a new tuning method. Active disturbance rejection control is transformed into two separate parts in the frequency domain: a pre-filter $H\left(s\right)$ and a controller $T\left(s\right)$. The parameters of the pre-filter and controller can be tuned to satisfy the performance requirements of high-order electro-hydraulic servo systems using quantitative feedback theory. To assess the efficacy of the proposed tuning approach, simulations and an application of a third-order electro-hydraulic servo system have been carried out and the stability of the application with an improved active disturbance rejection controller is analyzed. The results of simulations and experiments reveal that the new tuning method for high-order electro-hydraulic servo systems can obtain a better performance than the bandwidth tuning method and other methods.

1. Introduction

Electro-hydraulic servo systems (EHSSs) occupy a very important position in our industry, due to their advantages, such as large outputs (force/torque), high power-to-weight ratios, quick response, large stiffness, and less space occupation [1]. EHSSs are widely used in various fields, such as vehicle active suspensions [2], manipulators [3], aircraft actuators [4], steel manufacturing equipment [5], load simulators [6], engineering machineries [7], and some recent applications also can be seen in [8,9,10]. However, EHSSs, particularly high-order EHSSs, are highly nonlinear systems that exhibit many uncertainties, including uncertain fluid parameters, unmodeled dynamics, and unknown external disturbances [11]. And the impact of external mechanical vibration on a hydraulic valve also is an important issue that needs to be solved [12]. Over the past few decades, numerous advanced technologies, particularly nonlinear methods, have been investigated to enhance the dynamic performance of EHSSs in high-precision motion control field [13,14]. These nonlinear approaches usually offer a better performance compared to the linear methods [15,16]. If the mathematical model of the plant is precisely known, the adaptive control method is regarded as the optimal solution for mitigating the adverse impacts of uncertain parameters [17,18,19]. However, adaptive control may be ineffective at handling uncertain nonlinearities of EHSSs, which pose significant challenges in achieving high-precision motion control [20,21]. Detailed analyses of EHSS with a proportional distributor are presented in [22], where simulations and experimental studies were carried out for control signals of different shapes and for different feedbacks from the hydraulic system. To enhance the robustness of the adaptive controller, various robust adaptive control (RAC) approaches have been put forward [11,23,24]. One robust nonlinear controller was developed in [25], which can handle the uncertain nonlinear systems with modelling uncertainties without explicit upper bound information. However, the robust nonlinear controller presented in this paper is limited to dealing with a specific class of control systems with a bounded error. Additionally, a robust integral of the sign of the error (RISE) based on adaptive control was developed in [26,27,28]. These methods display excellent performances even when dealing with uncertain nonlinearities and continuous input signals. However, high feedback gains are the main obstacle to utilizing the RISE method due to issues such as annoying measurement noise, high-frequency dynamics, and the finite sampling frequency of the control systems [29,30]. Furthermore, sliding mode control (SMC), its derivatives, and other approaches, have been proposed to deal with the problems of EHSSs in [31,32,33,34]. All of the aforementioned control methods rely on accurate mathematical models of control systems, which are particularly being difficult to obtain for researchers, especially high-order EHSSs. Hence, achieving precise motion control of high-order EHSSs remains a significant challenge for theoreticians and researchers that needs plenty of time and effort to overcome.

The control methods can be categorized into three distinct groups based on their reliance on the mathematical models of the plants: model-free black-box control (MFBB), model-based white-box control (MBWB), and grey-box control (GBC) [35]. MFBB primarily comprises PID, fuzzy control, fuzzy PID control, and their respective derivatives [36,37,38]. MFBB requires an extensive amount of practical experiences, which necessitates a considerable period of time to accumulate [39]. MBWB primarily includes adaptive control, SMC, RAC, and their respective derivatives [40]. MBWB can solve the time varying parameters and uncertainties of the control systems. However, it relies on precise mathematical models of these systems, which can be extremely challenging for applicants to obtain. The aforementioned two points demonstrate why the PID controller, despite its limited performance, remains popular in engineering technology today [41]. Active disturbance rejection control (ADRC) stands as an outstanding representative of GBC, has been proposed by Chinese researcher JQ Han. ADRC requires less information about the control systems and is a practical controller for achieving precise motion control in high-order EHSSs [42]. A linear ADRC method was developed by doctor Z.Q. Gao to address the issue of having too many parameters to tune in traditional ADRC.Since then, there has been significant progress by researchers in theoretical analysis and many engineering applications [1,43,44]. The ADRC possesses considerable potential and competitive abilities, particularly in the realm of high-precision control for high-order EHSSs [33].

ADRC is well known for its simplicity, robustness, and excellent anti-interference capabilities [34]. Given the unknown external disturbances and inherent uncertainties of high-order EHSSs, utilizing ADRC as a solution to enhance control performance is an optimal choice. In addition, ADRC possesses another noteworthy advantage by utilizing the total disturbance to effectively deal with the unknown parameters and uncertainties of the control system [45]. Using frequency domain analysis as the primary method to handle the problems of control systems is a common method, and the second-order ADRC controller has been extensively studied and exploited through frequency domain analysis [46]. In the literature [47,48,49], Z.Q. Gao and his colleagues conducted an analysis of the control performance of the second-order linear ADRC controller, aiming to solve the problems in nonlinear control systems encompassing parameter uncertainties, disturbance estimations, stability, and measurement noise. A transfer function description was introduced for the ADRC-based control system by G Tian and Dr. Gao in [46]. A equivalent conventional feedback structure for generalized ADRC was also proposed in [50]. In [51], a novel control method based on linear ADRC was proposed to solve the problem of secondary frequency control in the isolated operation mode of an AC microgrid. A feedback control configuration based on ADRC was proposed by Dr. Gao in [31] for tuning the parameters of ADRC.

This paper introduces a new ADRC tuning method for high-order EHSSs that relies on frequency domain analysis and solves the problems of tuning the parameters of ADRC for high-order EHSSs effectively. Finally, the simulations and experimental results demonstrate that the improved ADRC controller, designed using the new tuning method, has a better performance compared with the bandwidth method and other methods. The remaining contents of this paper are organized as follows. Section 2 presents a mathematical model for a single-rod EHSS and the issues of EHSS are pointed out. A brief introduction to the basic concept of the ADRC is presented and the new ADRC tuning method using quantitative feedback theory for high-order EHSSs is proposed in Section 3. In Section 4, simulations and the application of a third-order EHSS are conducted, and the stability of the application is also analyzed. Then, the validity of the new tuning method is verified, and the results show that the improved ADRC controller tuned by the proposed method has a better performance than the ADRC controller tuned by the bandwidth method and other methods. Finally, some conclusions are drawn in Section 5.

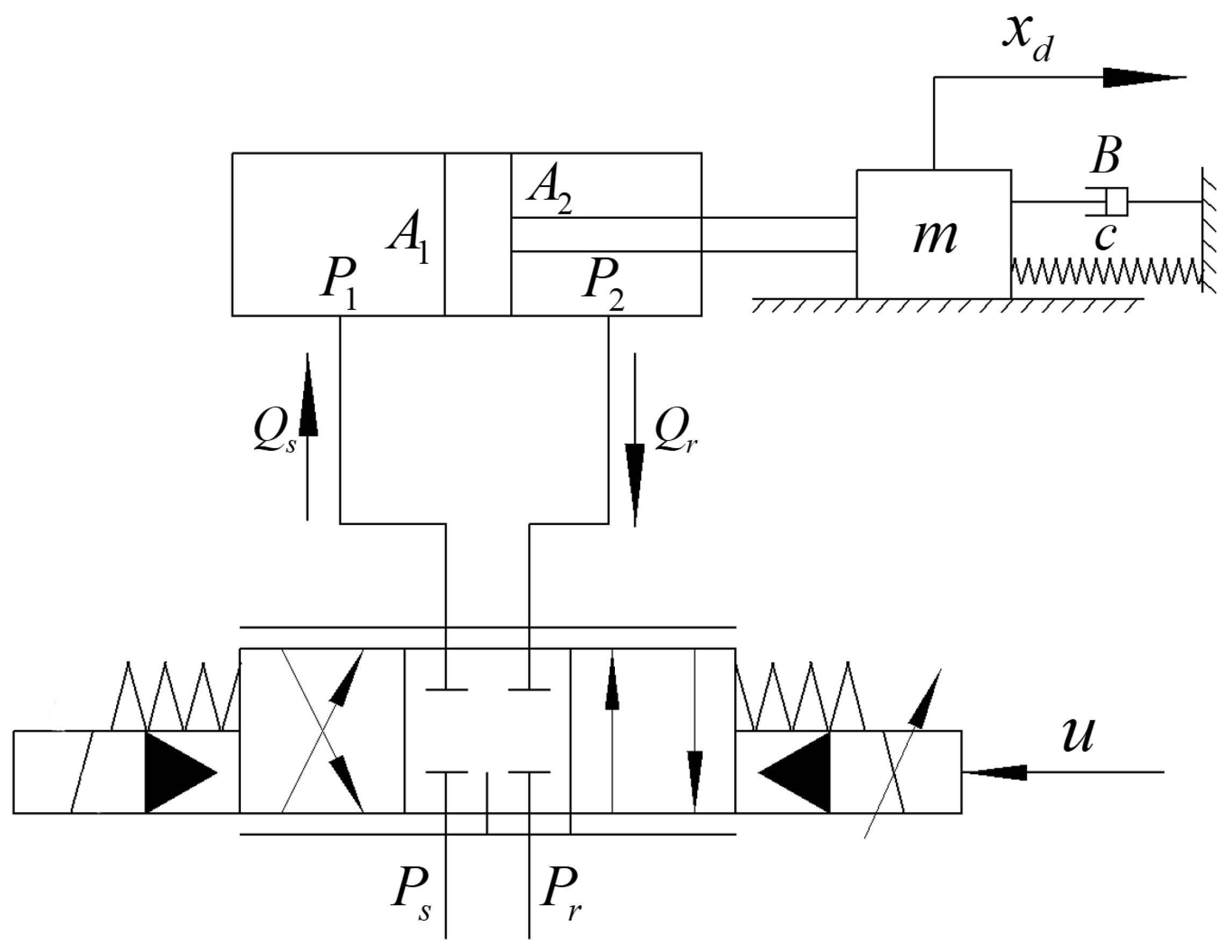
2. The Mathematical Model of Electro-Hydraulic Servo System

The schematic diagram of EHSS is depicted in Figure 1 and the single-rod EHSS primarily comprises a 4/3-way electro-hydraulic servo valve, a single-rod hydraulic cylinder, a displacement sensor, an inertial load, and some other attachments. As depicted in Figure 1, the inertial load is actuated by a single-rod hydraulic cylinder, which is controlled by an electro-hydraulic servo valve. Assuming that the pressure of the supply oil $P_s$ remains constant, and the pressure of the return oil $P_r$ is negligible, it can also be disregarded. The dynamics of the single-rod EHSS can be represented as Equation (1).

$$m{\ddot{x}}_d=P_1{A}_1-P_2{A}_2-B{\dot{x}}_d-c{x}_d-d$$

(1)

where m denotes the equivalent mass, including both the inertial load and the piston of the single-rod cylinder; the velocity and acceleration of the inertial load are represented by ${\dot{x}}_d$ and ${\ddot{x}}_d$, respectively, while $x_d$ signifies the displacement of the same load; $P_1$ and $P_2$ represent the pressures of the rodless chamber and the rod chamber applied to the single-rod hydraulic cylinder, respectively; $A_1$ and $A_2$ represent the effective areas of the rodless chamber and the rod chamber, respectively; the kinematic viscous damping coefficient of the cylinder is denoted by B, while the load stiffness is represented by c; and d represents the total disturbance including the unknown external load and uncertainties of mechanical dynamics such as the unmodeled friction effects and unconsidered effects of parameter deviations.

Neglecting the internal and external leakage of the single-rod cylinder depicted in Figure 1, the pressure dynamic equations of the single-rod cylinder can be expressed as [52]

$$\left\{\begin{array}{cc}\dot{P_1}\hfill & ={\displaystyle \frac{{\beta }_{e_1}}{V_1}}\left[Q_s-A_1{\dot{x}}_d-c_t(P_1-P_2)\right]\hfill \\ \dot{P_2}\hfill & ={\displaystyle \frac{-{\beta }_{e_2}}{V_2}}\left[Q_r-A_2{\dot{x}}_d-c_t(P_1-P_2)\right]\hfill \end{array}\right.$$

(2)

The flow rate entering the rodless chamber is denoted by $Q_s$, where the flow rate returning from the rod chamber of the single-rod cylinder is represented by $Q_r$; $V_1$ represents the total volume of the rodless chamber, while $V_2$ denotes the total volume of the rod chamber; and $V_{01}$ and $V_{02}$ represent the initial volumes of the two chambers, respectively, with $V_1=V_{01}+A_1{x}_d$ and $V_2=V_{02}-A_2{x}_d$. Furthermore, ${\beta }_{e_1}$ and ${\beta }_{e_2}$ represent the effective oil bulk modulus of the hydraulic oil in the chambers, respectively. Generally, there is ${\beta }_{e_1}={\beta }_{e_2}={\beta }_e$, because the servo value is symmetrical and matched. $c_t$ represents the coefficient of the internal oil leakage within the single-rod hydraulic cylinder.

As a high response servo value is selected, its dynamic response speed significantly exceeds the operational frequency of EHSS, and the dynamics of the electro-hydraulic servo valve can be neglected. The control input signal u exhibits a direct proportionality with a spool displacement valve of $x_v$, e.g., $x_v=k_{x}u,\left(\left|u\le 10\right|\right)$. $k_x$ represents a positive proportional coefficient, where u denotes the voltage of the electro-hydraulic servo valve. The oil flow equation of the servo value can be expressed as

$$\left\{\begin{array}{cc}{Q}_s\hfill & =k_{q_1}{k}_{x}u[s\left(u\right)\sqrt{P_s-P_1}+s(-u)\sqrt{P_1-P_r}]\hfill \\ Q_r\hfill & =k_{q_2}{k}_{x}u[s\left(u\right)\sqrt{P_2-P_r}+s(-u)\sqrt{P_s-P_2}]\hfill \end{array}\right.$$

(3)

The value gains at the left and right ends of the spool displacement of the electro-hydraulic servo valve are denoted as $k_{q_1}$ and $k_{q_2}$, respectively. Generally, there is $k_{q_1}=k_{q_2}=k_q$ and $k_q=c_d\omega \sqrt{2/\rho }$. The flow coefficient and the area gradient of the servo value are denoted by $c_d$ and $\omega$, respectively. The density of the hydraulic oil is denoted by the symbol $\rho$. The switching function $s(\ast )$ is defined as

$$s(\ast )=\left\{\begin{array}{c}1,if\ast >0,\hfill \\ 0,if\ast \le 0.\hfill \end{array}\right.$$

(4)

The oil flow equation of the electro-hydraulic servo valve can be reformulated as

$$\left\{\begin{array}{cc}{Q}_s\hfill & =g{T}_{1}u\hfill \\ Q_r\hfill & =g{T}_{2}u\hfill \end{array}\right.$$

(5)

with

$$\begin{array}{cc}\hfill g& =k_q{k}_x\hfill \\ \hfill T_1& =s\left(u\right)\sqrt{P_s-P_1}+s(-u)\sqrt{P_1-P_r}\hfill \\ \hfill T_2& =s\left(u\right)\sqrt{P_2-P_r}+s(-u)\sqrt{P_s-P_2}\hfill \end{array}$$

Substituting Equation (5) into Equation (2), we obtain Equation (6).

$$\left\{\begin{array}{cc}g{T}_{1}u\hfill & =A_1{\dot{x}}_d+c_t(P_1-P_2)+{\beta }_{e_1}^{-1}{V}_1{\dot{P}}_1\hfill \\ g{T}_{2}u\hfill & =A_2{\dot{x}}_d+c_t(P_1-P_2)-{\beta }_{e_2}^{-1}{V}_2{\dot{P}}_2\hfill \end{array}\right.$$

(6)

From Equation (1) to Equation (6), we define the state vector of the plant as $x={[x_1,x_2,x_3]}^T={[x_d,{\dot{x}}_d,{\ddot{x}}_d]}^T$. The state-space equation of the single-rod EHSS is described as

$$\left\{\begin{array}{cc}\dot{x}\hfill & =Ax+Bu+F{a}_0\hfill \\ y\hfill & =Cx\hfill \end{array}\right.$$

(7)

with

$$\begin{array}{cc}\hfill A& =\left[\begin{array}{ccc}0& 1& 0\\ 0& 0& 1\\ a_1& a_2& a_3\end{array}\right]\hfill \\ \hfill B& ={\left[\begin{array}{ccc}0& 0& b_1\end{array}\right]}^T\hfill \\ \hfill F& ={\left[\begin{array}{ccc}0& 0& 1\end{array}\right]}^T\hfill \\ \hfill C& =\left[\begin{array}{ccc}1& 0& 0\end{array}\right]\hfill \\ \hfill a_0& =-{\displaystyle \frac{{\beta }_e{c}_t(A_1{V}_1^{-1}+A_2{V}_2^{-1})(P_1-P_2)+\dot{d}}{m}}\hfill \\ \hfill a_1& =0\hfill \\ \hfill a_2& =-{\displaystyle \frac{{\beta }_e{A}_1{A}_2{V}_1^{-1}+{\beta }_e{A}_2^2{V}_2^{-1}+c}{m}}\hfill \\ \hfill a_3& =-{\displaystyle \frac{B}{m}}\hfill \\ \hfill b_1& =-{\displaystyle \frac{A_1{\beta }_{e}g{T}_1{V}_1^{-1}+A_2{\beta }_{e}g{T}_2{V}_2^{-1}}{m}}\hfill \end{array}$$

Depending on the mathematical model of the single-rod EHSS, it is evident that numerous parameters are time-varying and difficult to obtain. Under varying operating conditions, with the position change in the inertial load, the equivalent mass of the single-rod EHSS is also changed. Parameters $c_t$ and ${\beta }_e$ also vary with the fluctuation in temperature and pressure of the oil, respectively [53]. In addition, the exact values of the control system parameters B, c, and ${\beta }_e$ are also very difficult to obtain, which are defined as parameter uncertainties. Unmodeled dynamics and external disturbances of EHSS are the important issues for improving the control performance of high-order EHSS [54]. Thus, it is obvious that the parameter uncertainties, unmodeled dynamics, and external disturbances of EHSS are the main obstacles to improving its performance, and they’re the main limitations of the performance in the high-order EHSSs control field. The bandwidth tuning method has the issue of oversimplification and also restricts the performance of the ADRC controller [55]. This paper proposes a tuning method to solve the above problems. The application of this work is a third-order EHSS. The external disturbances of EHSS are random and uncertain, for example, the dynamic friction of a piston is a time-varying function of the displacement and velocity. To sum up, a controller that is not based on an accurate mathematical model should be adopted because the precise model of EHSS is notoriously difficult to obtain.This paper illustrates a new ADRC tuning method for high-order EHSSs that can deal with the problems of EHSSs, and allow them to track any position trajectory as smoothly, and as soon and closely as possible.

3. Active Disturbance Rejection Control for High-Order Electro-Hydraulic Servo Systems

In this section, a brief introduction to the schematic diagram of ADRC is presented, as depicted in Figure 2. Subsequently, a tuning method is introduced that employs quantitative feedback theory, based on the frequency domain analysis.

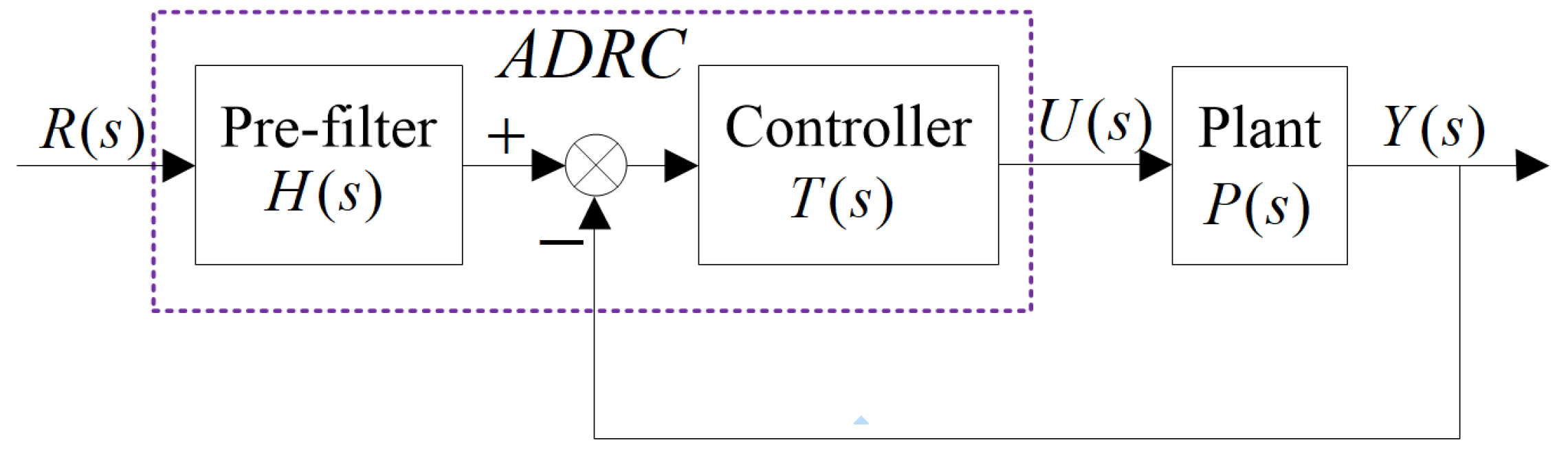
3.1. Active Disturbance Rejection Control

The basic idea of an ADRC controller is to estimate the internal dynamics and external disturbances of control systems using an extended state observer (ESO) in real-time, and then to actively compensate them with a unique control law. In general, ADRC encompasses three primary components: the tracking differentiator (TD), the ESO, and the tracking error feedback control law (TEFC) [56]. The schematic diagram of ADRC is illustrated in Figure 2—where ESO and TEFC jointly form a control structure with 2-DOF. The transfer function of the control system is denoted by $G_p\left(s\right)$, while $F_u\left(s\right)$ and $F_y\left(s\right)$ represent the transfer functions from the input signal u and the output response y to the augment state ${\overline{x}}_{(n+1)}$, respectively [45]. For the sake of simplicity, we consider a, nth-order dynamic system, which can be represented by

$$y^{\left(n\right)}=g(y^{(n-1)},y^{(n-2)},\dots ,\dot{y},y,\omega ,u)+bu$$

(8)

where $\omega$ signifies the external disturbances acting upon the control system; $g(y^{(n-1)},y^{(n-2)},\dots ,\dot{y},y,\omega ,u)$ denotes a multi-variable function of the internal dynamics and the total disturbance consists of unmodeled dynamics, parametric uncertainties, and some other disturbances. Lastly, b denotes the control signal gain, which regulates the performance of the controller.

Assuming that the augment state $x_{n+1}=g(y^{(n-1)},\dots ,\dot{y},y,\omega ,u)$ is differentiable, and let $h={\dot{x}}_{n+1}=\dot{g}(y^{(n-1)},y^{(n-2)},\dots ,\dot{y},y,\omega ,u)$, the control system is extended to a $(n+1)$th order control system [57]. The state-space equation of the $(n+1)$th order control system is formulated as

$$\left\{\begin{array}{c}\dot{x}=Ax+Bu+Eh\hfill \\ y=Cx\hfill \end{array}\right.$$

(9)

with

$$\begin{array}{cc}\hfill A& ={\left[\begin{array}{ccccc}0& 1& 0& \cdots & 0\\ 0& 0& 1& \cdots & 0\\ ⋮& ⋮& ⋮& \ddots & ⋮\\ 0& 0& 0& \cdots & 1\\ 0& 0& 0& \cdots & 0\end{array}\right]}_{(n+1)\times (n+1)}\hfill \end{array}$$

$$\begin{array}{cc}\hfill B& ={\left[\begin{array}{ccccc}0& 0& \cdots & b& 0\end{array}\right]}_{(n+1)\times 1}^T\hfill \\ \hfill C& ={\left[\begin{array}{ccccc}1& 0& \cdots & 0& 0\end{array}\right]}_{1\times (n+1)}\hfill \\ \hfill E& ={\left[\begin{array}{ccccc}0& 0& \cdots & 0& 1\end{array}\right]}_{(n+1)\times 1}^T\hfill \end{array}$$

In Equation (9), the state vector of the extended $(n+1)$th order control system is represented by $x={[x_1,x_2,x_3,\cdots ,x_n,x_{n+1}]}^T\in \mathbb{R}$, where $x_{n+1}$ represents the augment state, which encompasses the total disturbance of the extended control system. The total disturbance comprises unmodeled dynamics, parametric uncertainties, and unknown external disturbances.

The ESO for the extended control system (9) can be constructed as [58]

$$\left\{\begin{array}{c}\dot{\overline{x}}=A\overline{x}+Bu+L(y-\overline{y})\hfill \\ \overline{y}=C\overline{x}\hfill \end{array}\right.$$

(10)

In Equation (10), the state vector of ESO is denoted by $\overline{x}={[{\overline{x}}_1,{\overline{x}}_2,\cdots ,{\overline{x}}_n,{\overline{x}}_{n+1}]}^T$, and the gain vector of the ESO is $L=[l_1,l_2,\cdots ,l_n,l_{n+1}]$, which needs to be tuned. The objective of tuning the gain vector of ESO L is to ensure that the states of the ESO accurately track the states of the control system, as outlined in Equation (9).

A general feedback control law is introduced to mitigate the negative impact of the augmented state $g(y^{(n-1)},y^{(n-2)},\dots ,\dot{y},y,\omega ,u)$ on the performance of the control system. The augmented state is monitored by the augment state ${\overline{x}}_{n+1}$. The specific form of the general feedback control law is outlined in Equation (11).

$$\begin{array}{cc}\hfill u& ={\displaystyle \frac{k_1(R-{\overline{x}}_1)+k_2(\dot{R}-{\overline{x}}_2)+\cdots +k_n(R^{(n-1)}-{\overline{x}}_n)-{\overline{x}}_{n+1}}{b}}\hfill \\ & =K(\overline{R}-\overline{x})\hfill \end{array}$$

(11)

with

$$\begin{array}{cc}\hfill K& ={\displaystyle \frac{[k_1,k_2,\cdots ,k_n,1]}{b}}\hfill \\ \hfill \overline{R}& =[R,\dot{R},\cdots ,R^{(n-1)},0]\hfill \end{array}$$

where $[k_1,k_2,\cdots ,k_n]$ is the gain vector of the designed controller and R denotes the reference input signal.

The control system can be represented as

$$y^{\left(n\right)}=g-{\overline{x}}_{n+1}+k_1(R-{\overline{x}}_1)+k_2(\dot{R}-{\overline{x}}_2)+\cdots +k_n(R^{(n-1)}-{\overline{x}}_n)$$

(12)

If the ESO is tuned properly, the response of $g-{\overline{x}}_{n+1}$ becomes small and can be negligible, the right side of Equation (12) can be simplified to a PD controller [59].

In the above tuning process of the ADRC controller, the number of parameters requiring tuning is $2n+1$ in a nth order control system, which is the sum of the observer gains and the controller gains. Notably, the number of parameters that need to be tuned for the ADRC controller is directly proportional to the order of the control system. Hence, tuning the various parameters of the ADRC controller for high-order EHSS is a significant challenge. The current tuning method, which employs bandwidth to reduce the number of parameters required for ADRC controller design, appears straightforward and convenient. However, this approach significantly compromises the performance of the control system. Tuning the parameters through trial and error is also challenging. To broaden the utilization of ADRC to high-order EHSSs, this study introduces a convenient method for adjusting the ADRC controller, aiming to enhance the performance of high-order EHSSs.

3.2. The New Tuning Method for Active Disturbance Rejection Control

In this section, a new tuning approach is introduced, leveraging quantitative feedback theory [60] to tune the parameters of ADRC for high-order EHSSs. As depicted in Figure 3, ADRC undergoes a transformation from the state space to the frequency domain, resulting in its division into two distinct components: a controller $T\left(s\right)$ and a pre-filter $H\left(s\right)$. Subsequently, the components are restructured into a 2-DOF feedback control structure. The proposed tuning method in this paper facilitates the adjustment of the controller parameters $T\left(s\right)$ and pre-filter parameters $H\left(s\right)$ in accordance with the specific performance demands of the control systems.

By applying the Laplace transform to both ESO and TEFC, we obtain

$$s\overline{X}\left(s\right)=(A-LC)\overline{X}\left(s\right)+BU\left(s\right)+LY\left(s\right)$$

(13)

$$U\left(s\right)=K(\overline{R}\left(s\right)-\overline{X}\left(s\right))$$

(14)

with

$$\overline{R}\left(s\right)=R\left(s\right){[1,s,\cdots ,s^{(n-1)},0]}^T$$

where $\overline{X}\left(s\right),U\left(s\right),Y\left(s\right)$, and $\overline{R}\left(s\right)$ are the Laplace transform of $\overline{x},u,y$ and R, respectively.

Rewrite Equation (13) for $\overline{X}\left(s\right)$, we obtain Equation (15).

$$\overline{X}\left(s\right)=\mathsf{\Phi }(BU\left(s\right)+LY\left(s\right))$$

(15)

with

$$\mathsf{\Phi }={(sI-A+LC)}^{-1}$$

Substitute Equation (15) into Equation (14), we obtain Equation (16).

$$U\left(s\right)=K(\overline{R}\left(s\right)-\mathsf{\Phi }(BU\left(s\right)+LY\left(s\right)))$$

(16)

Rewrite Equation (16)

$$(1+K\mathsf{\Phi }B)U\left(s\right)=K\overline{R}\left(s\right)-K\mathsf{\Phi }LY\left(s\right)$$

(17)

and it can be rewritten as

$$\begin{array}{cc}\hfill U\left(s\right)=& {\displaystyle \frac{D_2\left(s\right)}{D_1\left(s\right)}}({\displaystyle \frac{D_3\left(s\right)}{D_2\left(s\right)}}R\left(s\right)-Y\left(s\right))\hfill \\ \hfill =& T\left(s\right)\left(H\right(s\left)R\right(s)-Y(s\left)\right)\hfill \end{array}$$

(18)

with

$$T\left(s\right)={\displaystyle \frac{D_2\left(s\right)}{D_1\left(s\right)}}={\displaystyle \frac{K\mathsf{\Phi }L}{1+K\mathsf{\Phi }B}}$$

(19)

$$H\left(s\right)={\displaystyle \frac{D_3\left(s\right)}{D_2\left(s\right)}}={\displaystyle \frac{K{[1,s,\cdots ,s^{(n-1)},0]}^T}{K\mathsf{\Phi }L}}$$

(20)

Consider that a high-order EHSS is described as $P\left(s\right)=Y\left(s\right)/U\left(s\right)$, from Equation (18) we can find a feedback control system with 2-DOF is constructed by the ADRC controller and the control system $P\left(s\right)$, as shown in Figure 3, and the ADRC controller is divided into a pre-filter $H\left(s\right)$ and controller $T\left(s\right)$. It is also shown that the structures of the controller $T\left(s\right)$ and the pre-filter $H\left(s\right)$ are determined by Equation (19) and Equation (20), respectively, as long as the order of $P\left(s\right)$ is known. For example, considering that $P\left(s\right)$ is a second-order EHSS, the structure of the controller $T\left(s\right)$ and the pre-filter $H\left(s\right)$ determined by Equation (19) and Equation (20), respectively.

$$T\left(s\right)={\displaystyle \frac{g_1}{s}}{\displaystyle \frac{s^2+g_{2}s+g_3}{s^2+g_{4}s+g_5}}$$

(21)

$$H\left(s\right)={\displaystyle \frac{h_1{s}^4+h_2{s}^3+h_3{s}^2+h_{4}s+h_5}{b{g}_1{s}^2+g_{2}s+g_3}}$$

(22)

Shown below are the coefficients of the controller $T\left(s\right)$ and the pre-filter $H\left(s\right)$.

$$\begin{array}{c}\hfill \left\{\begin{array}{c}{g}_1=(l_3+l_1{k}_1+l_2{k}_2)/b\hfill \\ g_2=(l_2{k}_1+l_3{k}_2)/(l_3+l_1{k}_1+l_2{k}_2)\hfill \\ g_3=l_3{k}_1/(l_3+l_1{k}_1+l_2{k}_2)\hfill \\ g_4=l_1+k_2\hfill \\ g_5=l_2+k_1+l_1{k}_2\hfill \end{array}\right.\end{array}$$

$$\begin{array}{c}\hfill \left\{\begin{array}{c}{h}_1=k_2\hfill \\ h_2=k_1+l_1{k}_2\hfill \\ h_3=l_1{k}_1+l_2{k}_2\hfill \\ h_4=l_2{k}_1+l_3{k}_2\hfill \\ h_5=l_3{k}_1\hfill \end{array}\right.\end{array}$$

The objective of the tuning procedure of the ADRC controller lies in determining the coefficients of the controller $T\left(s\right)$ and the pre-filter $H\left(s\right)$, as depicted in Figure 3. Once the coefficients of the controller $T\left(s\right)$ are determined, the coefficients of the pre-filter $H\left(s\right)$ can be derived by estimating the control signal gain b and solving the coefficient Equation (19). It is evident that the pre-filter $H\left(s\right)$ designed using this approach is solely reliant on the estimation of the control signal gain b. However, due to the parametric uncertainties present in high-order EHSSs, the control signal gain b is not a precise value. Consequently, accurately estimating the value of b is crucial for ensuring the optimal performance of the ADRC controller. In simpler terms, the primary challenges in designing the ADRC controller utilizing this approach lie in the design of the controller $T\left(s\right)$ and the determination of the control signal gain b. Furthermore, given that the degree of the numerator in the pre-filter $H\left(s\right)$ exceeds the degree of its denominator, the frequency response of $H\left(s\right)$ indicates that the pre-filter has the potential to amplify high-frequency components within the reference signal. Owing to this, the sole necessity of the pre-filter $H\left(s\right)$ is to produce a smooth signal for the control system to follow, and a basic one can suffice in its place, functioning as a low-pass filter.

The quantitative feedback theory, as referenced in [61], can effectively be utilized for tuning the parameters of both the controller $T\left(s\right)$ and the pre-filter $H\left(s\right)$, as demonstrated in Figure 3. Once a high-order EHSS $P\left(s\right)$ is validated, the tuning procedure for the improved ADRC controller specific to the system typically encompasses the following four sequential steps.

Firstly, generate the Nichols charts for the high-order EHSS across the selected frequencies. Each chosen frequency’s designated template encompasses a compilation of frequency responses derived from the high-order EHSS, taking into consideration the potential parametric uncertainties.

Secondly, establish the closed-loop performance specifications for the higher-order EHSS and then convert them into constraints on the nominal open-loop transfer function $L_n\left(s\right)=P_n\left(s\right)T\left(s\right)$, where $P_n\left(s\right)$ signifies the nominal form of $P\left(s\right)$.

Thirdly, following the sequence of the high-order EHSS, we employ Equation (19) to determine the parameters of the controller $T\left(s\right)$ and perform loop shaping of $L_n\left(s\right)$ to satisfy the established boundaries. Once a satisfactory $L_n\left(s\right)$ is achieved, we can leverage $T\left(s\right)=L_n\left(s\right)/P_n\left(s\right)$ to determine the positions of the poles and zeroes of the controller $T\left(s\right)$.

Finally, the pre-filter $H\left(s\right)$ is designed by modifying the closed-loop frequency response to make sure that it falls within the prescribed tracking bounds.

The straightforward and concise process of designing the pre-filter $H\left(s\right)$ as a low-pass filter can be achieved through just three simple steps.

Initially, assume the pre-filter $H\left(s\right)=1$, and then proceed to plot the Bode diagram of the selected frequency responses of the high-order EHSS.

Secondly, overlay the reference tracking specification on the same Bode diagram, ensuring it terminates at identical upper and lower bounds.

Lastly, modify the frequency responses of the high-order EHSS within the defined tracking bounds by shifting the poles and zeros, thereby reconstructing the transfer function of the pre-filter $H\left(s\right)$.

4. Application of Active Disturbance Rejection Control to High-Order Electro-Hydraulic Servo Systems

In this section, a third-order ADRC controller is designed using the tuning method proposed in this paper for an application. Then, the stability of the application with the improved controller is analyzed. The simulations and experiments are conducted to verify the control performance of the application. The results indicate that the improved ADRC controller of the application has a better performance than the ADRC controller tuned by the bandwidth method and other methods.

4.1. The Tuning Process of The Third-Order Application

To maintain brevity in this paper, the proposed tuning approach will be used to tune the parameters of the ADRC controller for a third-order EHSS. The model of EHSS is represented by Equation (7).

In the third-order EHSS application, the state-space model of the third-order EHSS $P\left(s\right)$ is considered as Equation (7), and a third-order improved ADRC controller is designed to control the system. The transfer function of the third-order EHSS is depicted as

$$P\left(s\right)={\displaystyle \frac{b_1}{s^3+a_3{s}^2+a_{2}s+a_1}}$$

(23)

with

$$\begin{array}{cc}\hfill a_1& =0\hfill \\ \hfill a_2& ={\displaystyle \frac{{\beta }_e{A}_1{A}_2{V}_1^{-1}+{\beta }_e{A}_2^2{V}_2^{-1}+c}{m}}\hfill \\ \hfill a_3& ={\displaystyle \frac{B}{m}}\hfill \\ \hfill b_1& =-{\displaystyle \frac{A_1{\beta }_{e}g{T}_1{V}_1^{-1}+A_2{\beta }_{e}g{T}_2{V}_2^{-1}}{m}}\hfill \end{array}$$

And the transfer function of the closed-loop control system is show as Equation (24).

$$G\left(s\right)=H\left(s\right){\displaystyle \frac{T\left(s\right)P\left(s\right)}{1+T\left(s\right)P\left(s\right)}}$$

(24)

Table 1. The parameters of the third-order EHSS.

Symbol	Nominal	Range
$m\left(\mathrm{kg}\right)$	$9.877\times {10}^3$	-
${\beta }_e\left(\mathrm{Pa}\right)$	$6.89\times {10}^8$	$5.56\times {10}^8\sim 8.90\times {10}^8$
$A_1\left({\mathrm{m}}^2\right)$	0.89	-
$A_2\left({\mathrm{m}}^2\right)$	0.45	-
$V_1\left({\mathrm{m}}^3\right)$	0.7	-
$V_2\left({\mathrm{m}}^3\right)$	0.4	-
$B(\mathrm{N}/(\mathrm{m}/\mathrm{s}\left)\right)$	$2\times {10}^3$	$1.5\times {10}^3\sim 2.5\times {10}^3$
$c({\mathrm{m}}^3/\mathrm{s}/\mathrm{Pa})$	$4\times {10}^{-13}$	$2.5\times {10}^{-13}\sim 7.0\times {10}^{-13}$
$k_q{k}_x({\mathrm{m}}^3/\mathrm{s}/\mathrm{V}/{\mathrm{Pa}}^{1/2})$	$8.92\times {10}^{-8}$	-
$P_s\left(\mathrm{MPa}\right)$	30	-
$P_r\left(\mathrm{MPa}\right)$	0	-
$P_1\left(\mathrm{MPa}\right)$	29.84	-
$P_2\left(\mathrm{MPa}\right)$	0.23	-

introduces the nominal values alongside the ranges of the parameters of the third-order EHSS. The control signal gain b and the parameters c and ${\beta }_e$ are the uncertain parameters of the third-order EHSS. With the purpose of preventing any potential conflicts, the symbols are exclusively valid within this application. In order to enhance the tracking performance of the third-order EHSS, an improved ADRC controller has been designed using the proposed tuning method.

Figure 4 depicts the templates corresponding to the selected frequencies of the aforementioned third-order EHSS inclusive of uncertainties. The third-order improved ADRC controller should be designed according to the specifications of the closed-loop stability and the reference tracking.

$$\left\{\begin{array}{c}\left|{\displaystyle \frac{G\left(jw\right)P\left(jw\right)}{1+G\left(jw\right)P\left(jw\right)}}\right|\le \delta =1.06,\hfill \\ \left|T_L\left(jw\right)\right|\le \left|T\left(jw\right)\right|\le \left|T_U\left(jw\right)\right|,\hfill \end{array}\forall w\in [0,\infty )\right..$$

(25)

with

$$\begin{array}{cc}\hfill & T_L\left(j\omega \right)={\displaystyle \frac{4\times {10}^6}{(j\omega +5)(j\omega +20){(j\omega +200)}^2}}\hfill \\ \hfill & T_U\left(j\omega \right)={\displaystyle \frac{7.7\times (j\omega +5.6)(j\omega +125)}{(j\omega +6){(j\omega +30)}^2}}\hfill \end{array}$$

The 2% settling time of the lower bound $T_L\left(j\omega \right)$ is 0.85 s and there is no overshoot. And the upper bound, $T_U\left(j\omega \right)$ has 5% overshoot and the 2% settling time, which is 0.4 s. The stability specifications guarantee a gain margin of 5.77 dB and a phase margin of 56.29° for the control system. Subsequently, the stability configurations undergo a transformation into nominal open-loop transfer function constraints, which are given in boundaries as depicted in Figure 5. Loop shaping has been carried out, and Figure 5 illustrates an acceptable nominal transfer function $L_n\left(s\right)$. The controller $T\left(s\right)$ is determined as

$$T\left(s\right)={\displaystyle \frac{360}{s}}·{\displaystyle \frac{s^2/{372}^2+0.2s/242+1}{s^2/{817}^2+1.45s/977+1}}·{\displaystyle \frac{(s/3.75+1)}{(s/937+1)}}$$

(26)

Figure 6 demonstrates a successful implementation of the pre-filter $H\left(s\right)$, which ensures that the frequency responses of the closed-loop system adhere to the tracking bounds requirements. It is expressed as

$$H\left(s\right)={\displaystyle \frac{(s/22+1)}{(s/12+1)(s/25+1)(s/110+1)}}$$

(27)

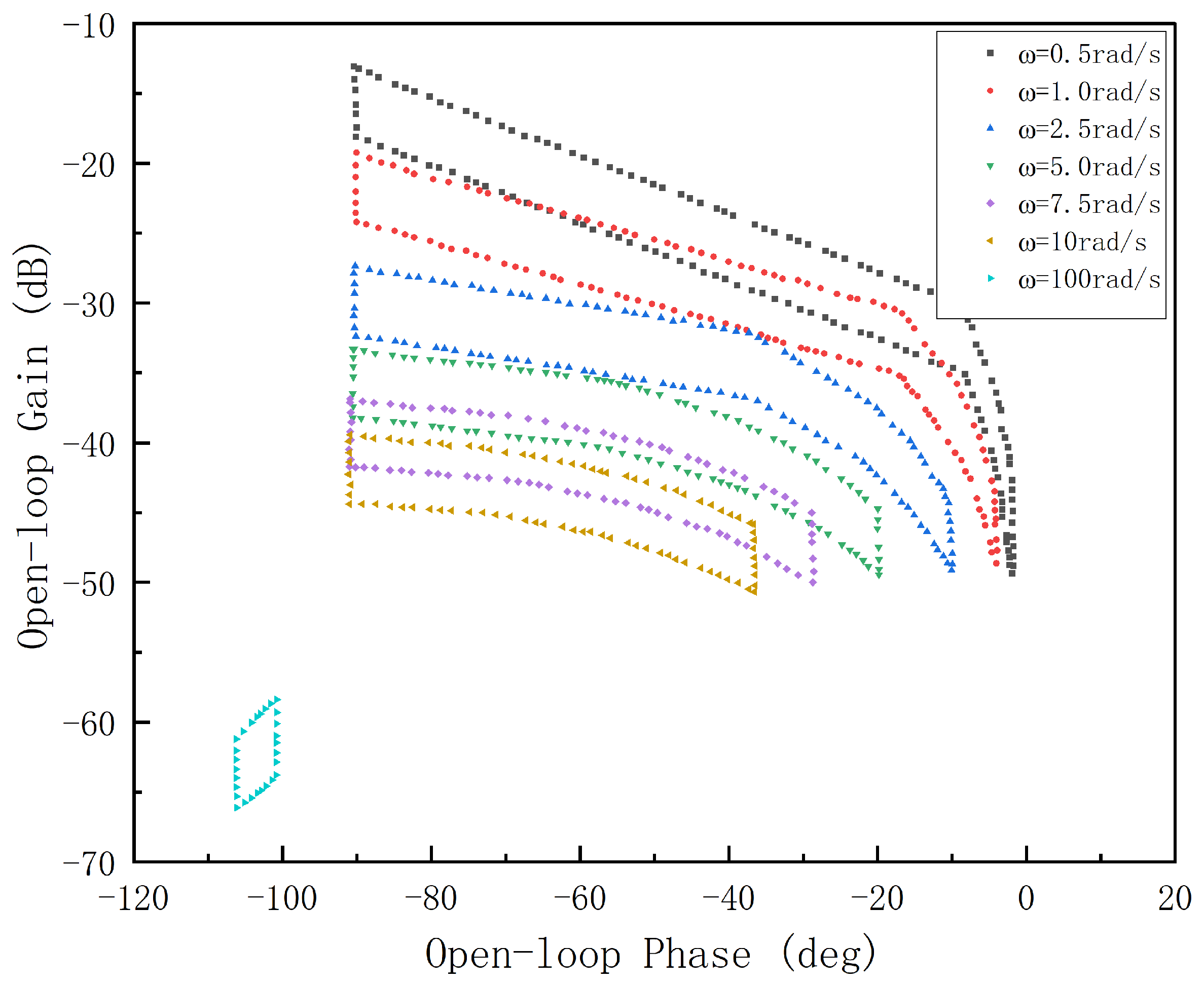
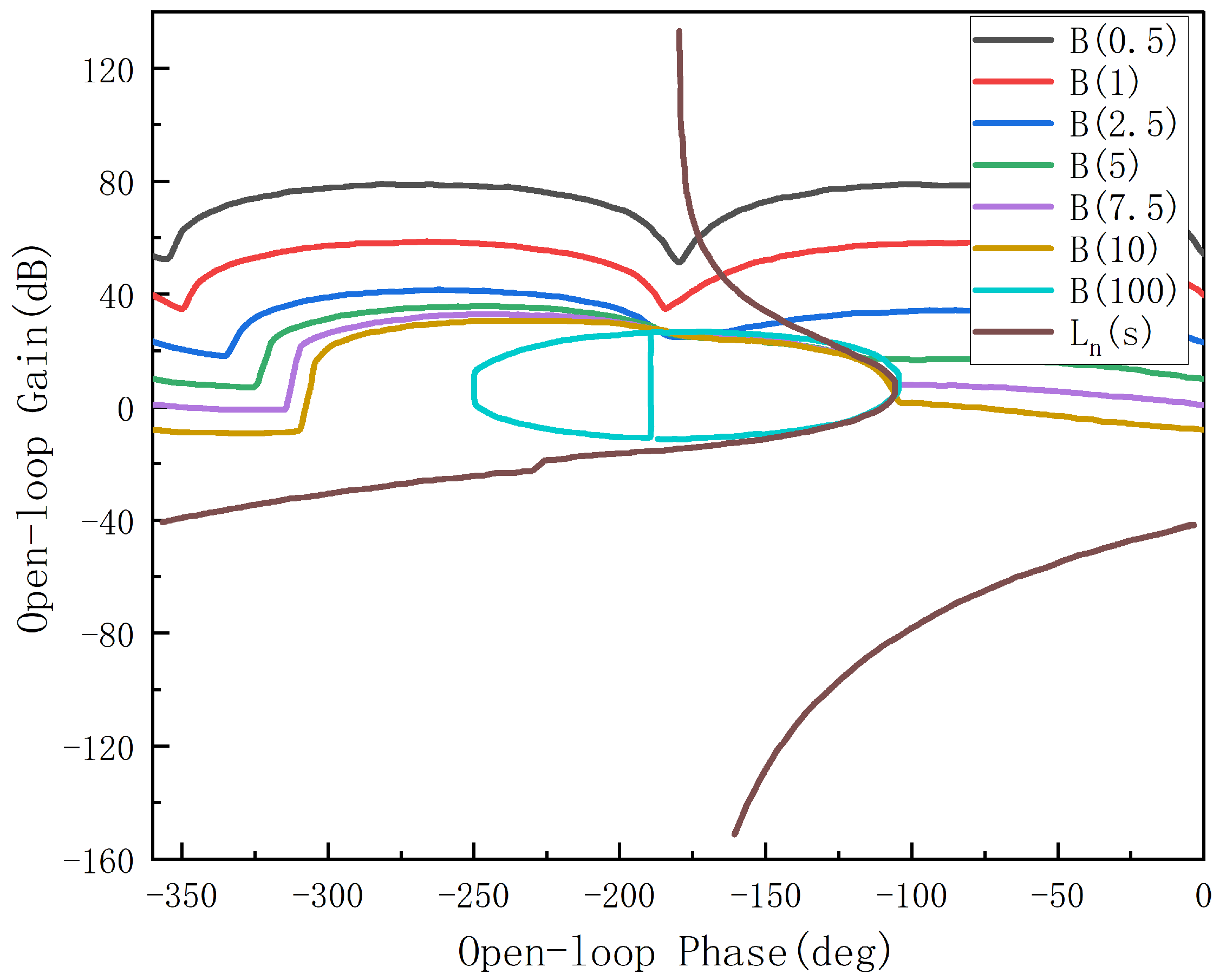
4.2. The Stability Analysis of The Application with Improved ADRC Controller

The stability of the bounded-input bounded-output EHSS is analyzed in this work. The third-order application is given as

$$\ddot{y}=F(y^{\left(3\right)},y^{\left(2\right)},\dot{y},y)+bu\left(t\right)$$

(28)

Assuming that $x_1=y,x_2=\dot{y},x_3=F$ and $h=\dot{F}$. Then, convert Equation (28) to its state space form, the state space form of the application is expressed as

$$\begin{array}{c}\hfill \left\{\begin{array}{c}{\dot{x}}_1=x_2\hfill \\ {\dot{x}}_2=x_3\hfill \\ {\dot{x}}_3=h\hfill \\ y=x_1\hfill \end{array}\right.\end{array}$$

(29)

The ESO of the third-order EHSS is designed as

$$\begin{array}{c}\hfill \left\{\begin{array}{c}{\dot{\overline{x}}}_1={\overline{x}}_2+l_1(x_1-{\overline{x}}_1)\hfill \\ {\dot{\overline{x}}}_2={\overline{x}}_3+l_2(x_1-{\overline{x}}_1)+bu\hfill \\ {\dot{\overline{x}}}_3=l_3(x_1-{\overline{x}}_1)\hfill \end{array}\right.\end{array}$$

(30)

where $\overline{x}={[{\overline{x}}_1,{\overline{x}}_2,{\overline{x}}_3]}^T$ represents the state vector of ESO; $L={[l_1,l_2,l_3]}^T$ denotes the gain vector of ESO.

The estimation errors between the third-order EHSS and ESO is expressed as

$$\begin{array}{c}\hfill \left\{\begin{array}{c}{\dot{\widehat{x}}}_1={\dot{x}}_1-{\dot{\overline{x}}}_1\hfill \\ {\dot{\widehat{x}}}_2={\dot{x}}_2-{\dot{\overline{x}}}_2\hfill \\ {\dot{\widehat{x}}}_3={\dot{x}}_3-{\dot{\overline{x}}}_3\hfill \end{array}\right.\end{array}$$

(31)

Define the estimation error vector as $\widehat{x}={[{\widehat{x}}_1,{\widehat{x}}_2,{\widehat{x}}_3]}^T$, and then solve the Equations (29) to (31); Equation (31) can be rewritten as

$$\dot{\widehat{x}}=A\widehat{x}+Bh$$

(32)

with

$$\begin{array}{cc}\hfill A& =\left[\begin{array}{ccc}-l_1& 1& 0\\ -l_2& 0& 1\\ -l_3& 0& 0\end{array}\right]\hfill \\ \hfill B& ={\left[\begin{array}{ccc}0& 0& 1\end{array}\right]}^T\hfill \end{array}$$

Considering that h is bounded, and the gain vector of ESO is tuned such that the matrix A is Hurwitz, the estimation errors between the third-order EHSS and ESO are bounded-input bounded-output stable [30].

Then, let $R_1=R$, $R_2=\dot{R}$, $R_3=\ddot{R}$, the error equation can be obtained as

$$\begin{array}{c}\hfill \left\{\begin{array}{c}{e}_1=R_1-x_1\hfill \\ e_2=R_2-x_2\hfill \\ e_3=R_3-x_3\hfill \end{array}\right.\end{array}$$

(33)

The controller is designed as

$$\begin{array}{cc}\hfill u& ={\displaystyle \frac{k_1(R_1-{\overline{x}}_1)+k_2(R_2-{\overline{x}}_2)-{\overline{x}}_3}{b}}\hfill \\ \hfill & ={\displaystyle \frac{k_1(e_1+{\widehat{x}}_1)+k_2(e_2+{\widehat{x}}_2)-(x_3-{\widehat{x}}_3)}{b}}\hfill \end{array}$$

(34)

And the tracking error of the reference and the states of the third-order EHSS is expressed as

$$\begin{array}{c}\hfill \left\{\begin{array}{c}{\dot{e}}_1={\dot{R}}_1-{\dot{x}}_1\hfill \\ {\dot{e}}_2={\dot{R}}_2-{\dot{x}}_2=R_3-k_1(e_1+{\widehat{x}}_1)-k_2(e_2+{\widehat{x}}_2)-{\widehat{x}}_3\hfill \end{array}\right.\end{array}$$

(35)

Define the error vector as $e={[e_1,e_2]}^T$, Equation (35) can be rewritten as

$$\dot{e}=A_{e}e+B_e\widehat{x}+R_3$$

(36)

with

$$\begin{array}{cc}\hfill A_e& =\left[\begin{array}{cc}0& 1\\ -k_1& -k_2\end{array}\right]\hfill \\ \hfill B_e& =\left[\begin{array}{ccc}0& 0& 0\\ -k_1& -k_2& -1\end{array}\right]\hfill \end{array}$$

Begin with the premise that both $\widehat{x}$ and $R_3$ are bounded, the gains of the controller are tuned to make the matrix $A_e$ Hurwitz; thus, the tracking error vector is bounded-input bounded-output stable.

4.3. Simulation and Experimental Verification

To assess the efficacy of the improved ADRC controller, which is comprised of a controller $T\left(s\right)$ and a pre-filter $H\left(s\right)$ tuned using the tuning method proposed in this paper, a series of simulations and experiments have been carried out. For comparison, in the following simulation analysis, we use MATLAB/Simulink and its version is 9.2.0.538062, and the performance of the improved ADRC controller, PID, and ADRC controller tuned with the bandwidth method are investigated, respectively. The parameters of the third-order EHSS are given in Table 1.

The step responses of the closed-loop control system controlled by the improved ADRC controller are described in Figure 7a. The values of uncertain parameters gradually increase from small to large within the specified ranges outlined in Table 1. It is quite obvious that the step responses of the closed-loop control system controlled by the modified ADRC controller are all located between the lower and upper tracking bounds. The step responses of the closed-loop control system, controlled by an ADRC controller tuned using the bandwidth method, are illustrated in Figure 7b. To ensure all responses fall within the tracking bounds, the observer bandwidth is tuned to ${\omega }_o=700$, the controller bandwidth is tuned to ${\omega }_c=130$, and the parameter $b_0$ is set as $b_0=70$. Compared with the step responses of the closed-loop control system controlled by the improved ADRC controller and the ADRC controller tuned by the bandwidth tuning method, it is not difficult to notice that the response of the closed-loop control system tuned using the bandwidth method almost have oscillations and a certain amount of overshoot.

With the purpose of comparing the two tuning methods clearly, simulation results with specified parameter values are shown in Figure 8. The step response of the closed-loop control system is amplified shown in Figure 8, the step response of the closed-loop control system controlled by the improved ADRC controller is smoother than the step response of the closed-loop control system tuned by the bandwidth method. The step response of the closed-loop control system tuned by the bandwidth method, the observer bandwidth is tuned to ${\omega }_o=700$, the parameter $b_0$ is set as $b_0=70$, and the controller bandwidth is tuned to ${\omega }_c=130$ and ${\omega }_c=140$, also shown in Figure 8. Obviously, the step responses of the ADRC controller tuned by the bandwidth method have obvious oscillations, and the larger the bandwidth of the controller, the better the performance of the ADRC controller.

Figure 9 and Figure 10 show the tracking performance and the tracking error in displacement tracking under a sinusoidal signal $r=sin\left(0.5\pi t\right)\mathrm{mm}$, comparing both the PID controller and the improved ADRC controller. From the results of the simulation, the tracking error of the improved ADRC controller is smaller than the PID controller, and the tracking performance of the improved ADRC controller also is better than PID controller. So, the improved ADRC controller exhibits a superior control efficacy compared with the PID controller, and can achieve a stable state faster. The simulation results show that the modified ADRC controller has a better performance and it can enable the third-order EHSS to track the target signal smoothly without experiencing overshoot.

The results of above simulations show that:

(1)

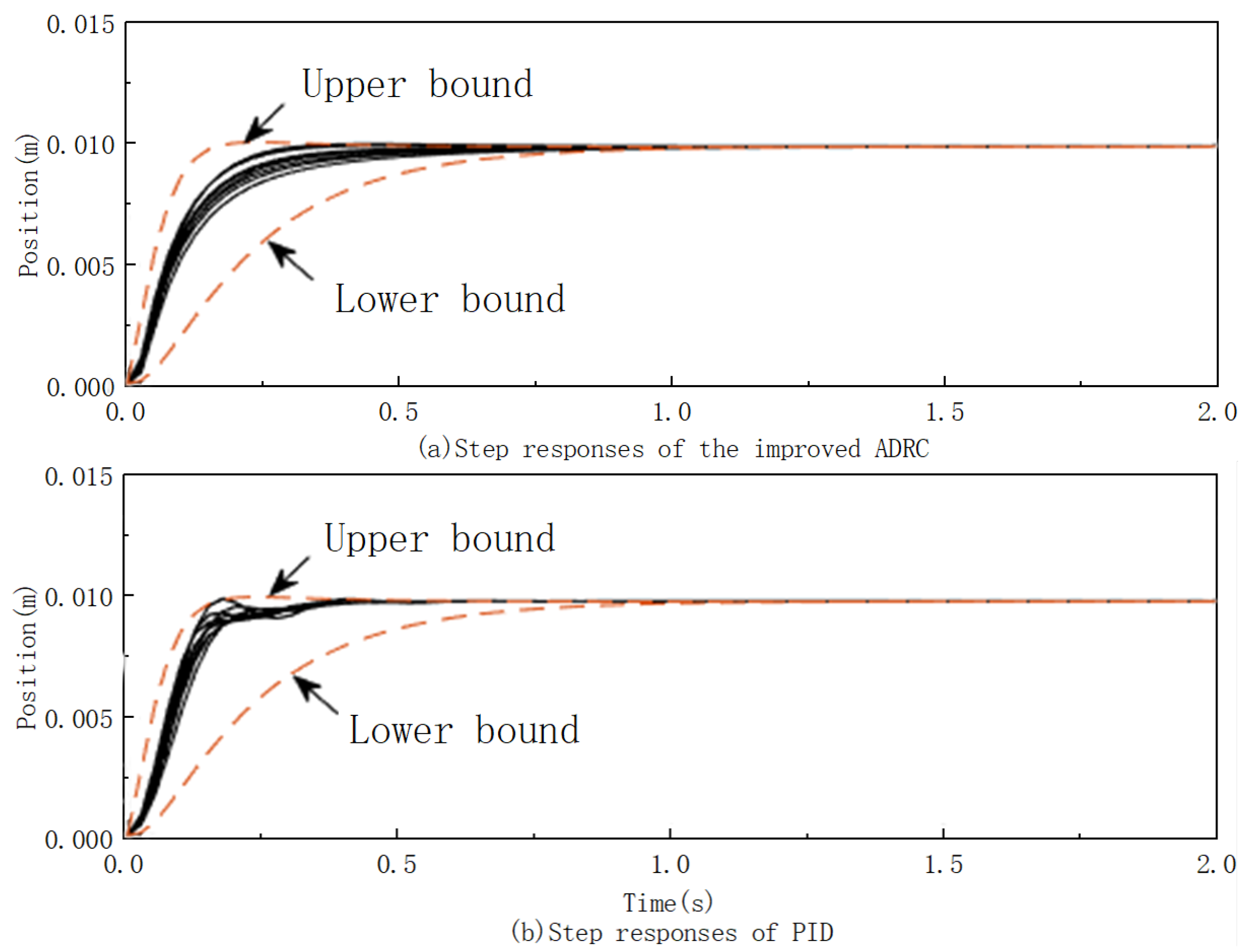
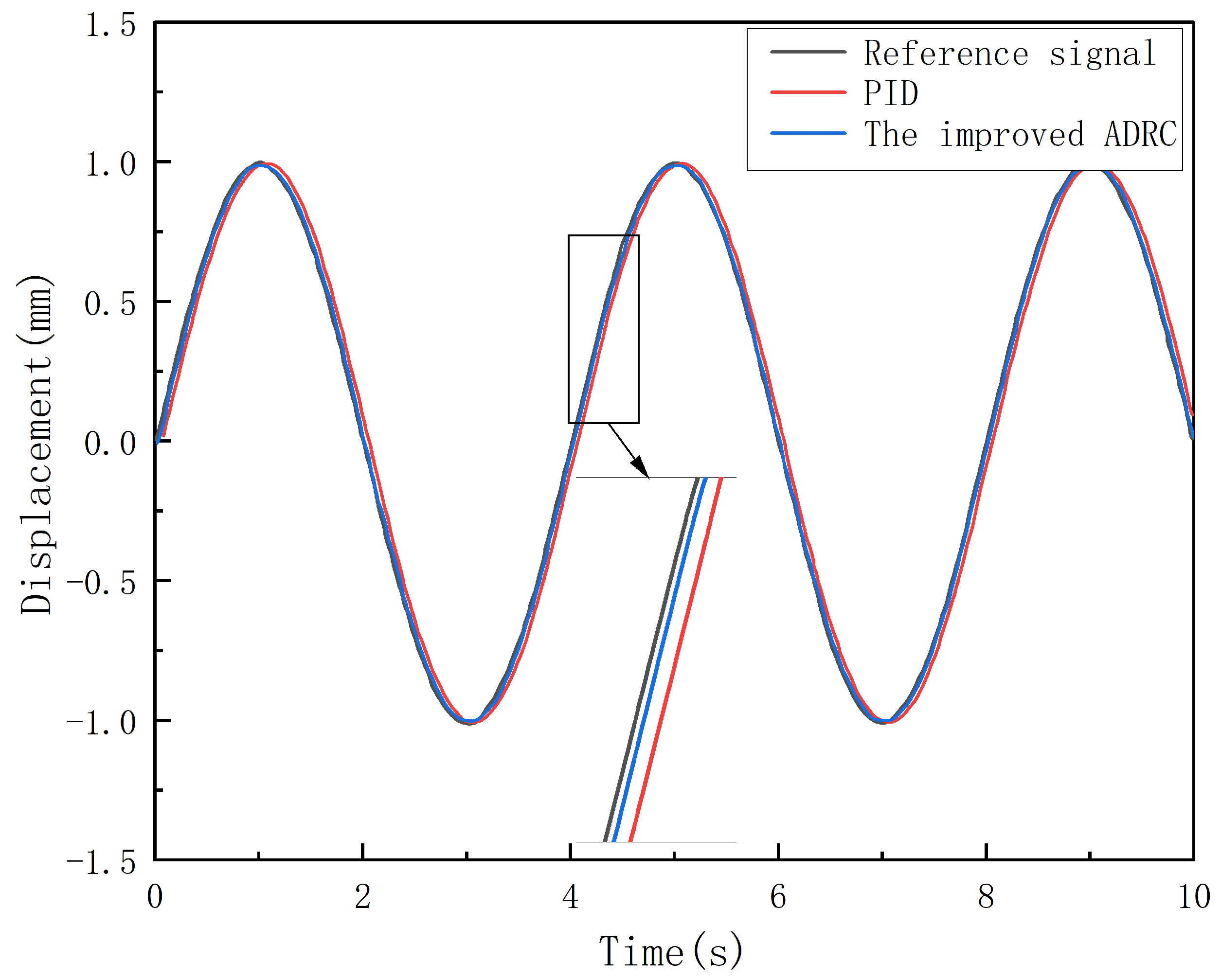
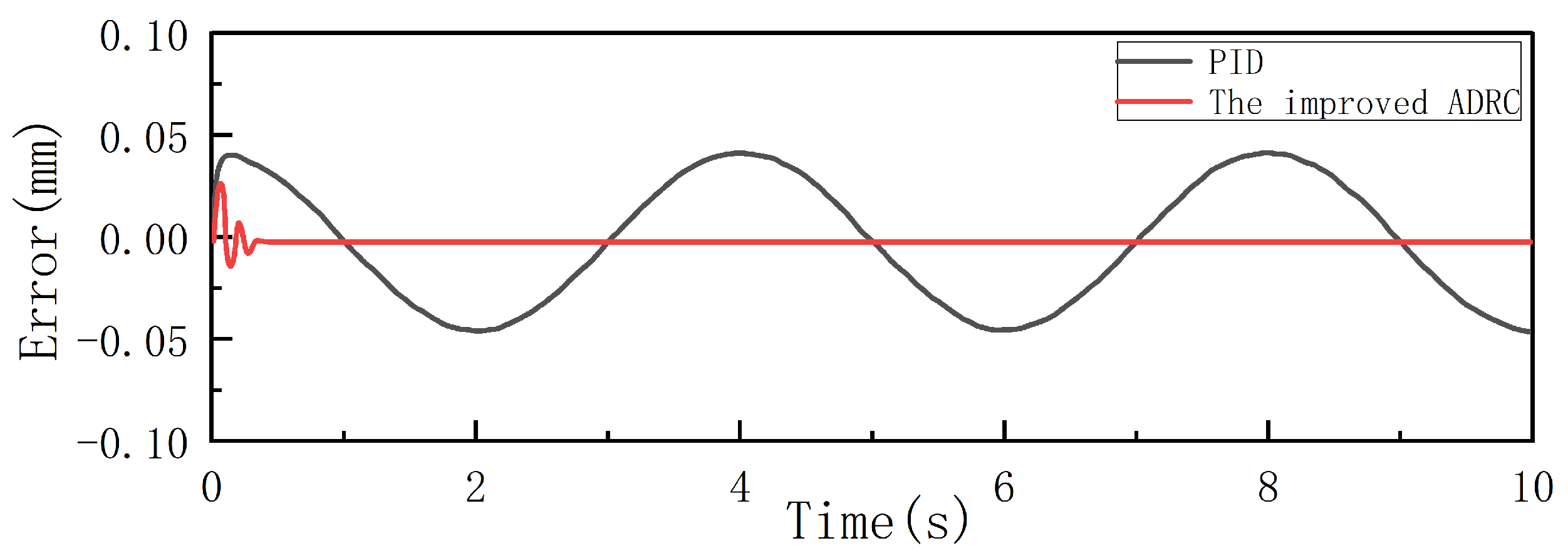
Compared with the bandwidth tuning method and the PID controller, the proposed method enables the improved ADRC controller to have a better tracking performance.

(2)

The bandwidth tuning method exhibits significant oscillations and overshoot in the step response and also has a hardware limitation, which often leads to undesired responses in practical applications.

(3)

The bandwidth tuning method need large observer bandwidth to enable the ADRC controller to have a better performance, but the amplitude of the oscillations becomes larger.

The experimental platform of the closed-loop control system is illustrated in Figure 11. The experimental platform mainly consists of a bench case; servo valves; some other attachments; and an industrial computer with a counter card, an A/D converter, and a D/A converter. In addition, the experimental platform also includes relief valves, oil filters, and other accumulators to ensure the normal operation of the experimental platform. The bench case consists of three hydraulic cylinders, a circle-shape frame, four connecting rods, two bases, and some stainless steel oil pipes. The main parameters of the components of the experiment platform are shown in Table 1 and the main components of the experiment platform are shown in

Table 2. Main components of the experimental platform.

Element	Type	Marks
Computer	Vostro 3460	Dell, Austin, TX, USA
Servo value	4WRPH10C3B100L	Rexroth, Stuttgart, Germany
Pressure sensor	US175-C00002-200BG	MEAS, Richmond, VA, USA
Displacement sensor	LS628C	Heidenhain, Bavaria, Germany
Counter card	IK-220	Heidenhain, Bavaria, Germany
A/D card	PCI-1716	Advantech, Taiwan, China
D/A card	PCI-1723	Advantech, Taiwan, China

.

The PID controller is used in our industry widely [62], and the improved ADRC controller proposed in this paper is compared with the PID controller. The improved ADRC controller was tested on the experimental platform depicted in Figure 11. The single-rod hydraulic cylinder is designed to follow a square wave with an amplitude of 0.01 meters precisely, and the response of the closed-loop control system is shown in Figure 12. It is obvious that the improved ADRC controller can track the target signal closely. The results indicate that the response of the closed-loop control system falls within the lower and upper bounds, and the performance of the improved ADRC controller tuned by the tuning method proposed in this paper is satisfactory to deal with the uncertainties of the third-order EHSS. Additionally, Figure 12 also presents the square-wave response of a PID controller for comparative purposes. It is obvious that the improved ADRC controller can track the reference signal quickly and it has less settling time and oscillations. The performance of the ADRC controller is better than the PID controller.

5. Conclusions

This paper proposes a new tuning method for an ADRC controller for high-order electro-hydraulic servo systems in the presence of uncertain parameters, unmodeled mechanical dynamics, and external disturbance. The justification and effectiveness of the new tuning method have been verified through theoretical analysis, simulations, and experiments. Based on the simulation and experimental results, we find that the proposed new tuning method of ADRC controller for high-order EHSSs has a better performance than the ADRC controller tuned by the bandwidth method and PID controller. Furthermore, the new tuning method results in the ADRC controller easily being used for high-order electro-hydraulic servo systems. In the future, using the tuning method on multi-input multi-output high-order control systems that are frequently employed in our industry may become a new popular research point.