Records of Ground Deformation in Northern Kefalonia Inferred from Cosmogenic 36Cl Geochronology
Abstract
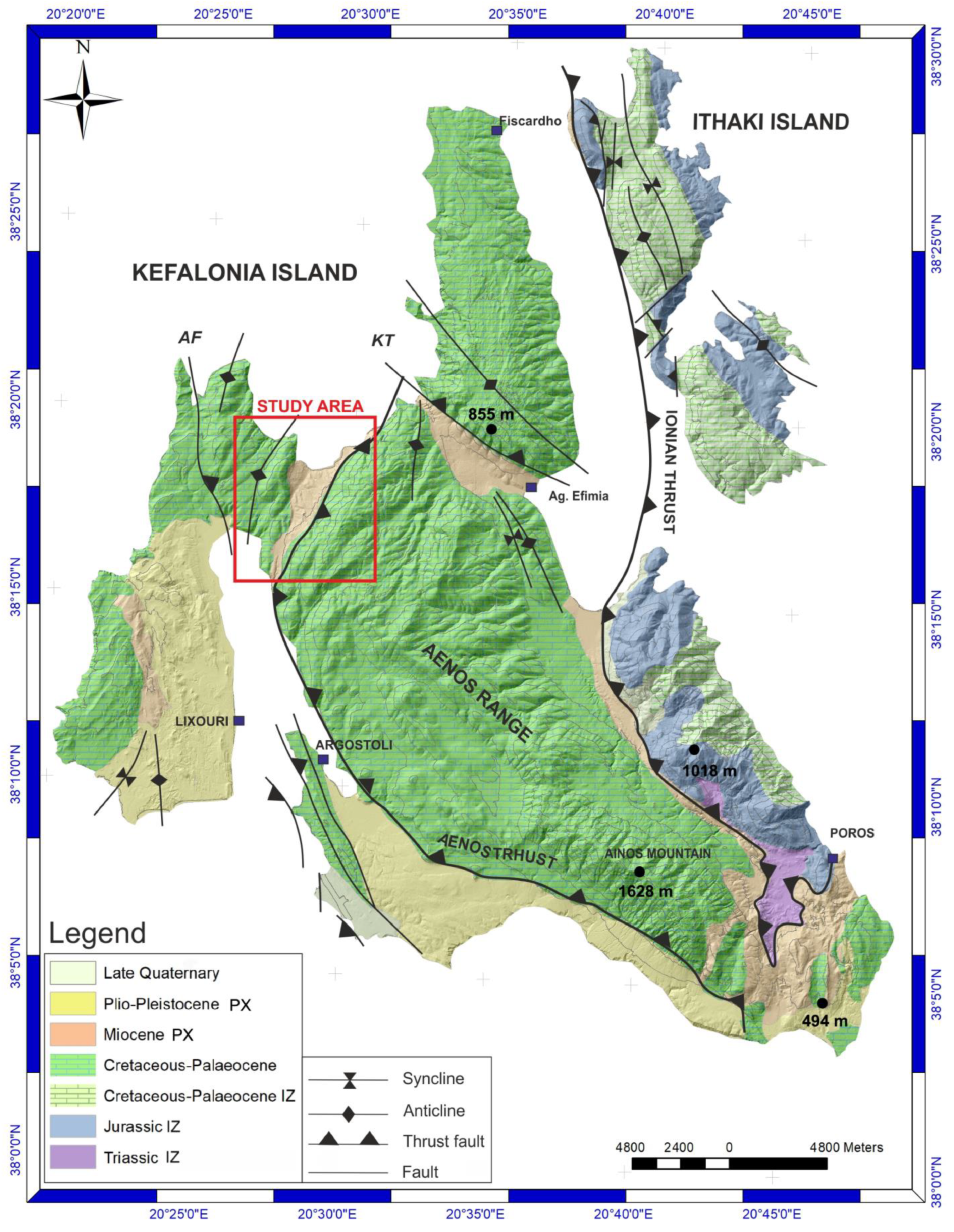
1. Introduction
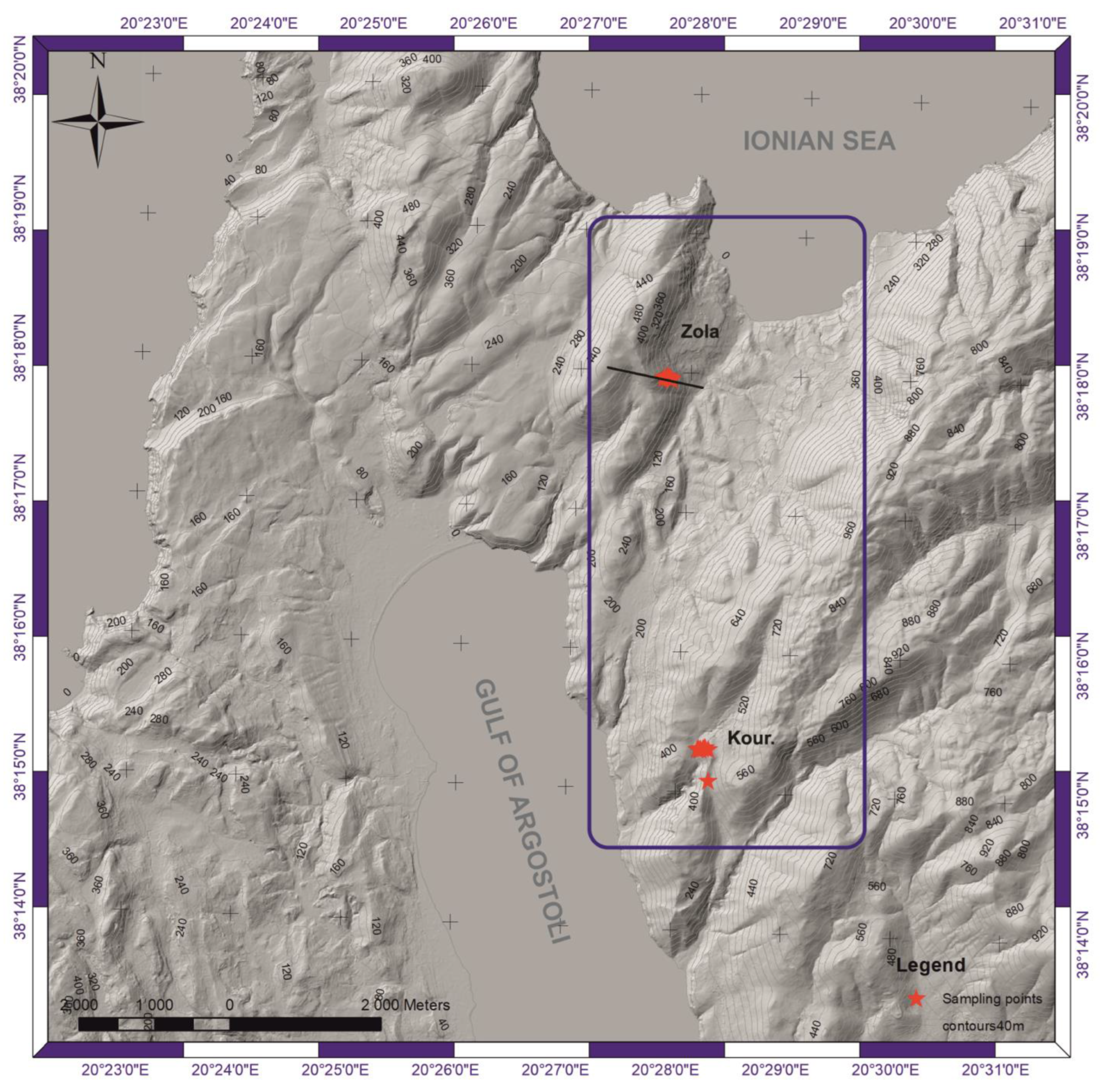
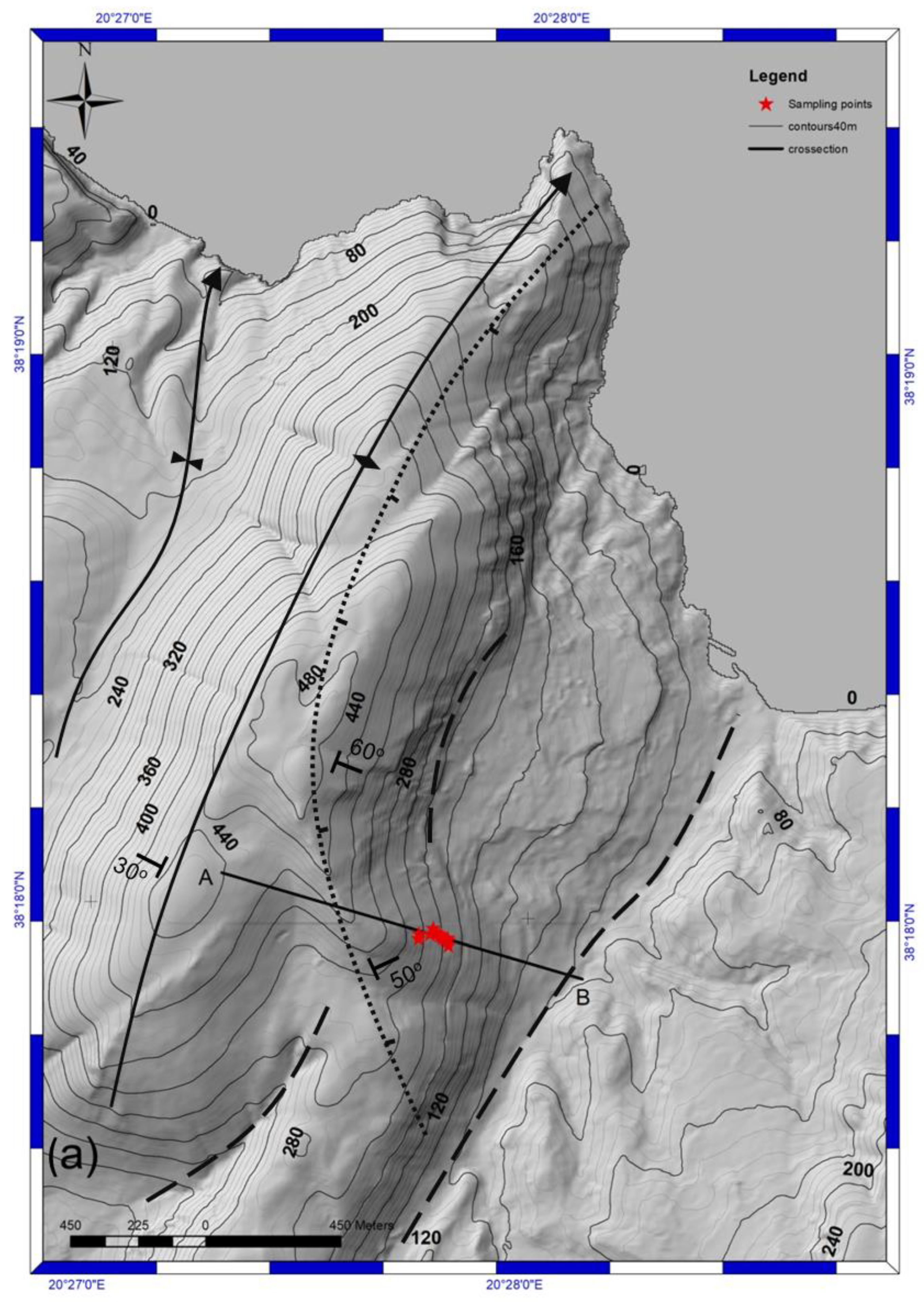
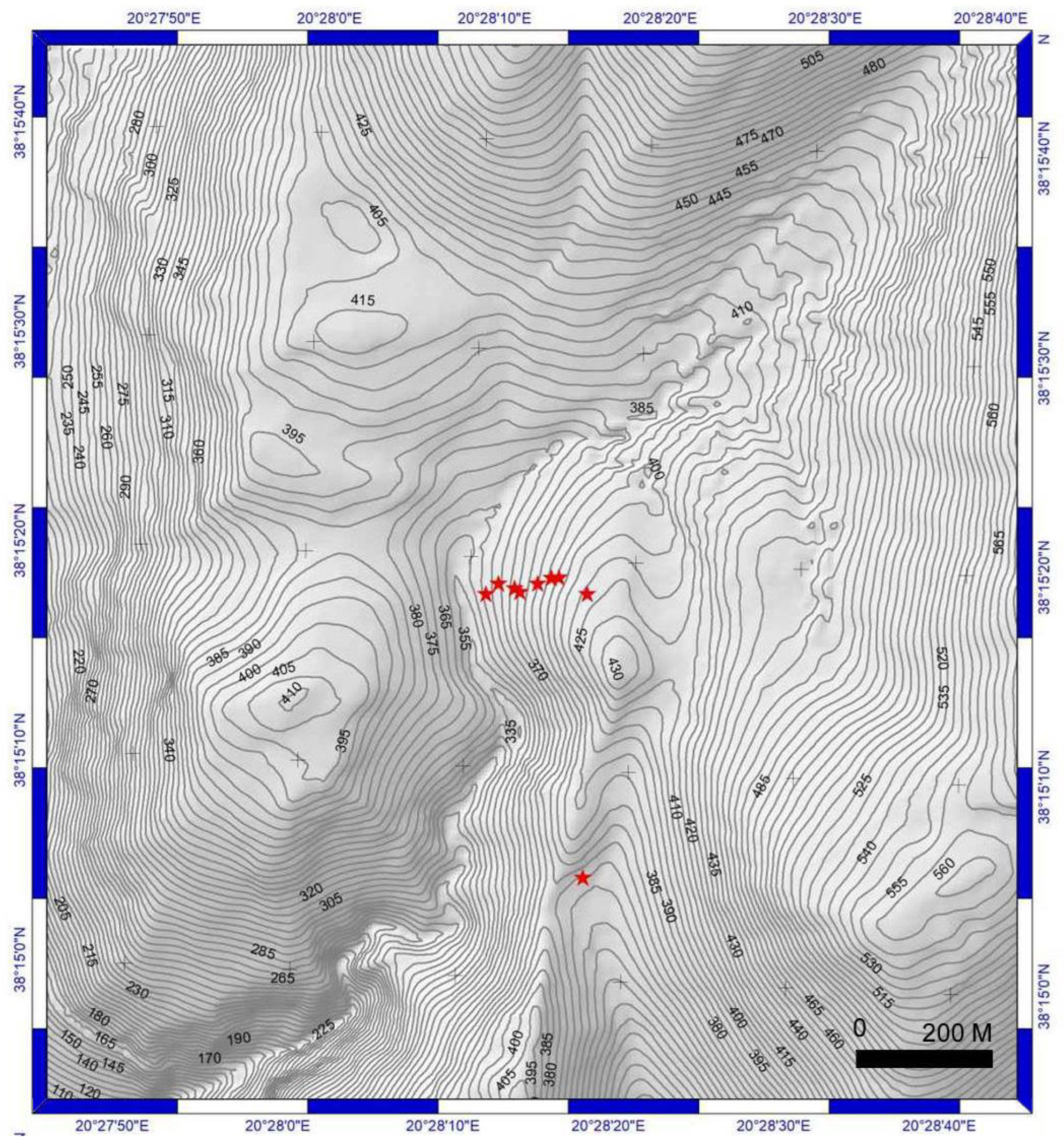
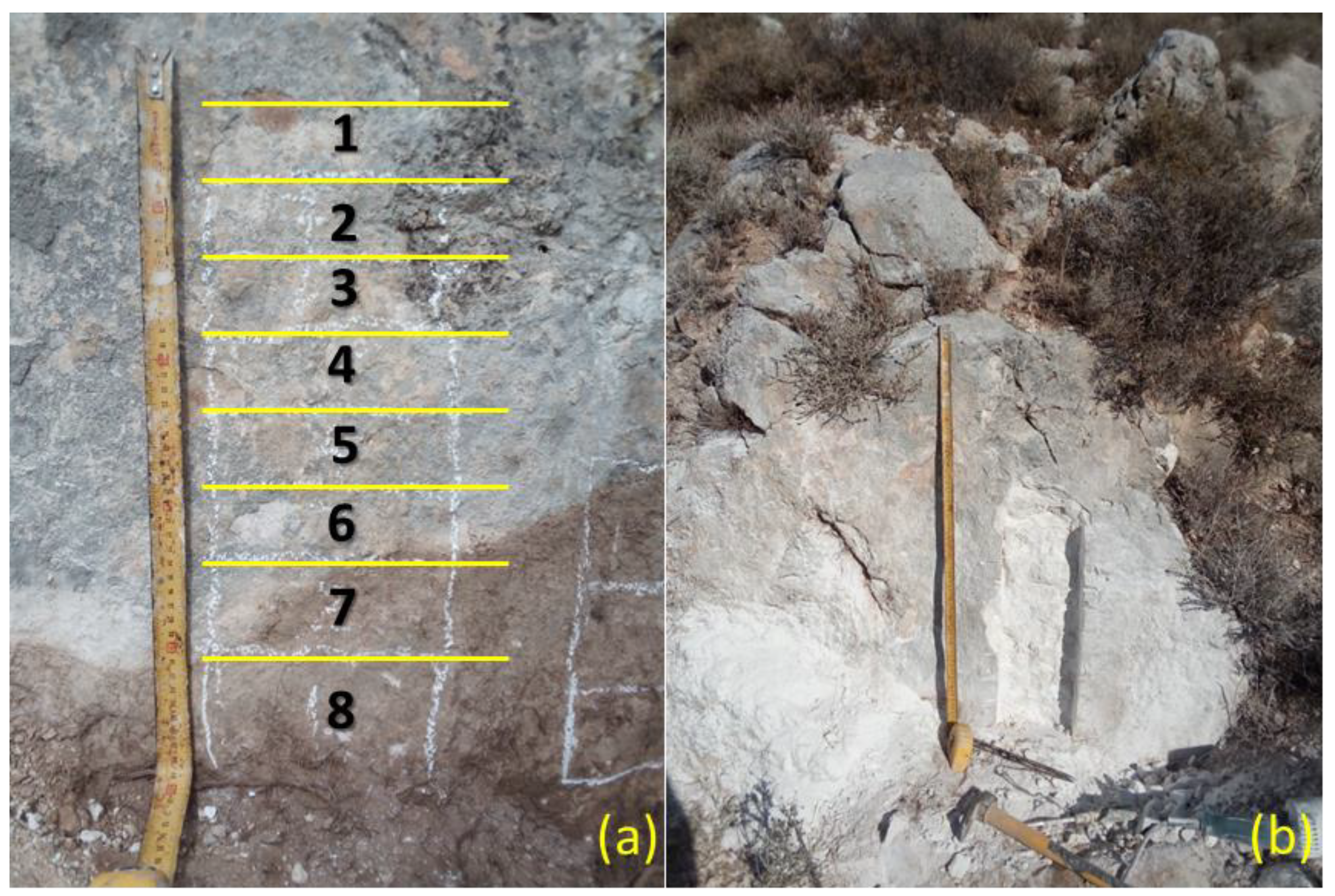
2. Geological Setting and Samples
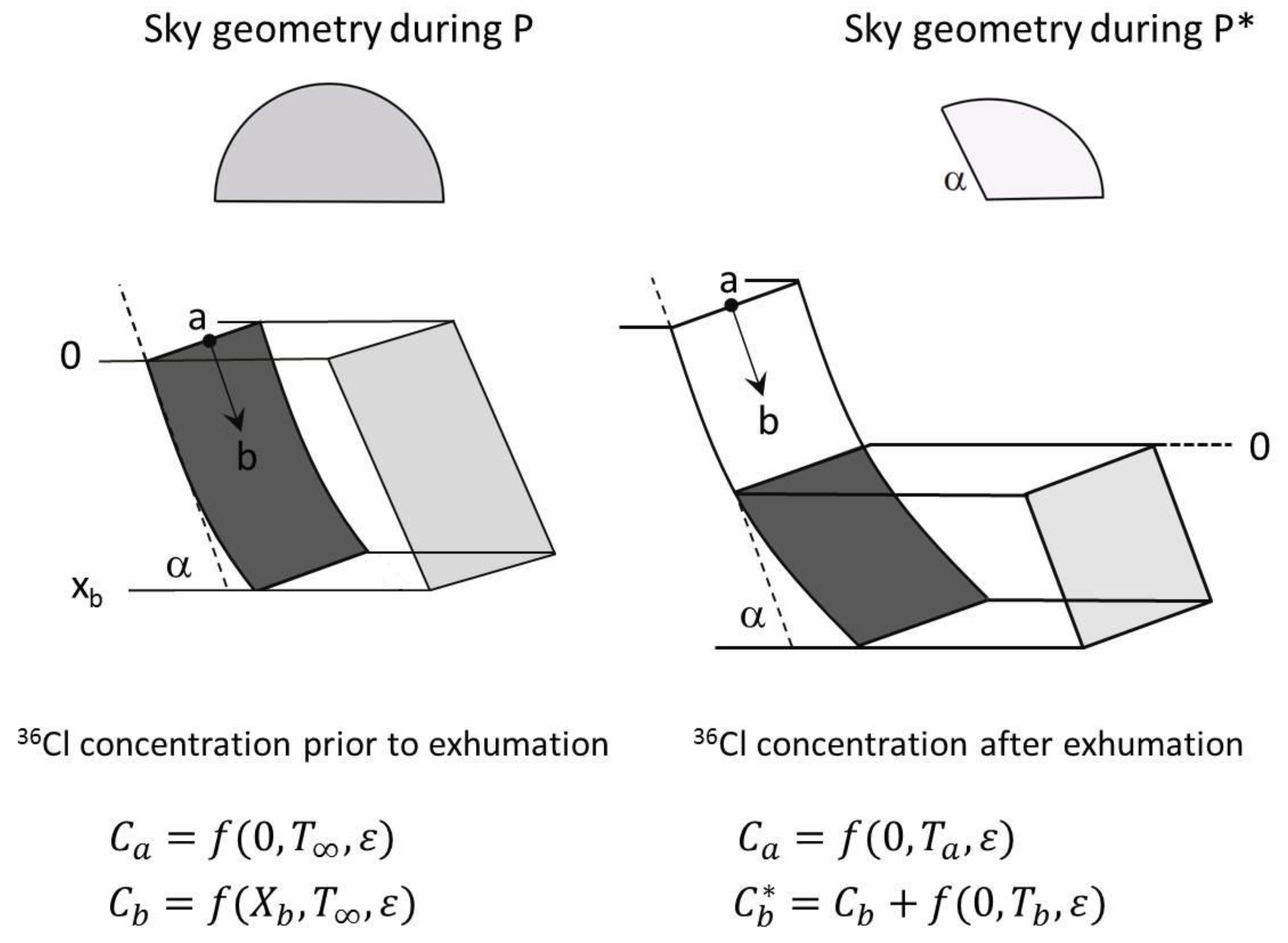
3. Methodology
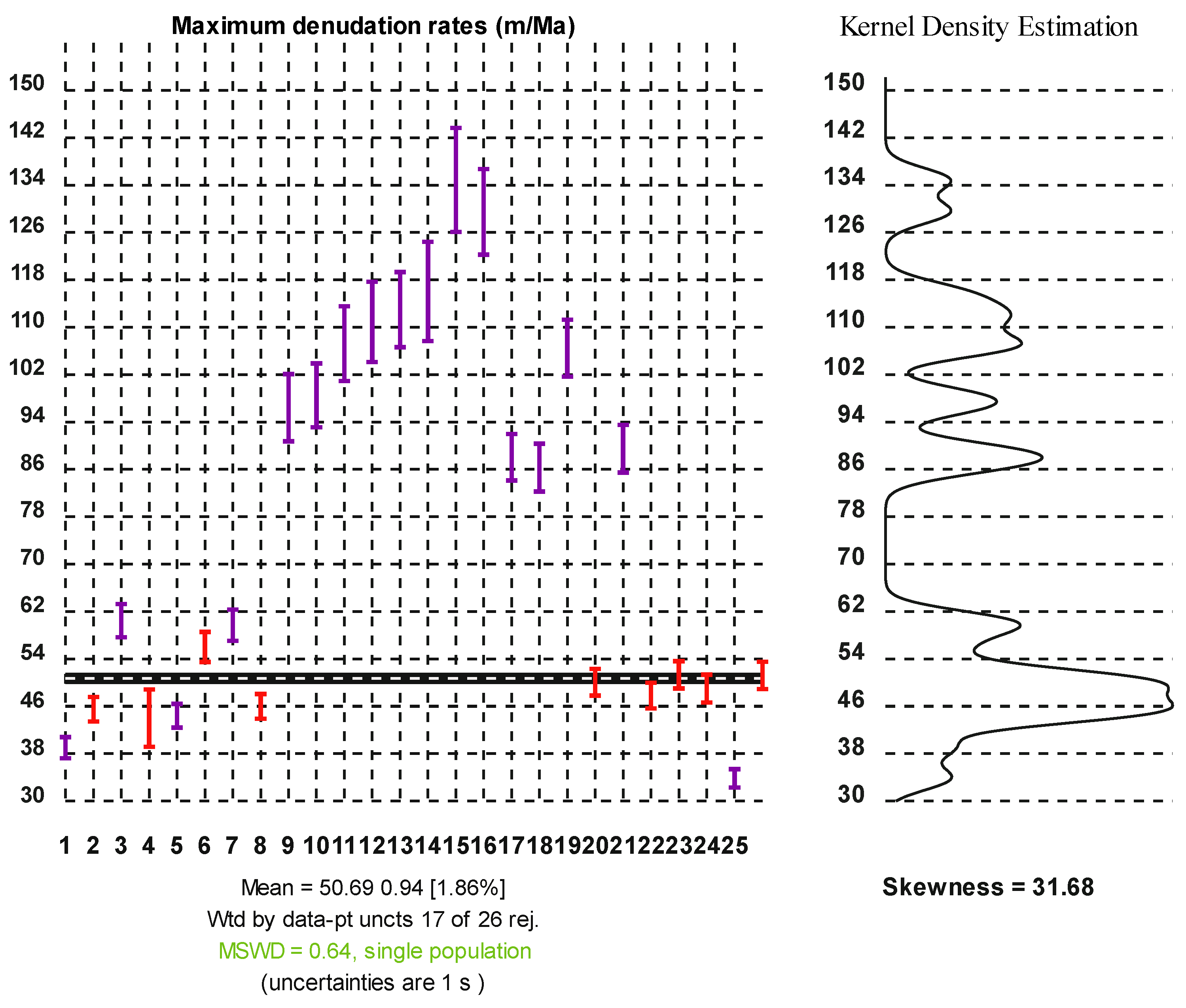
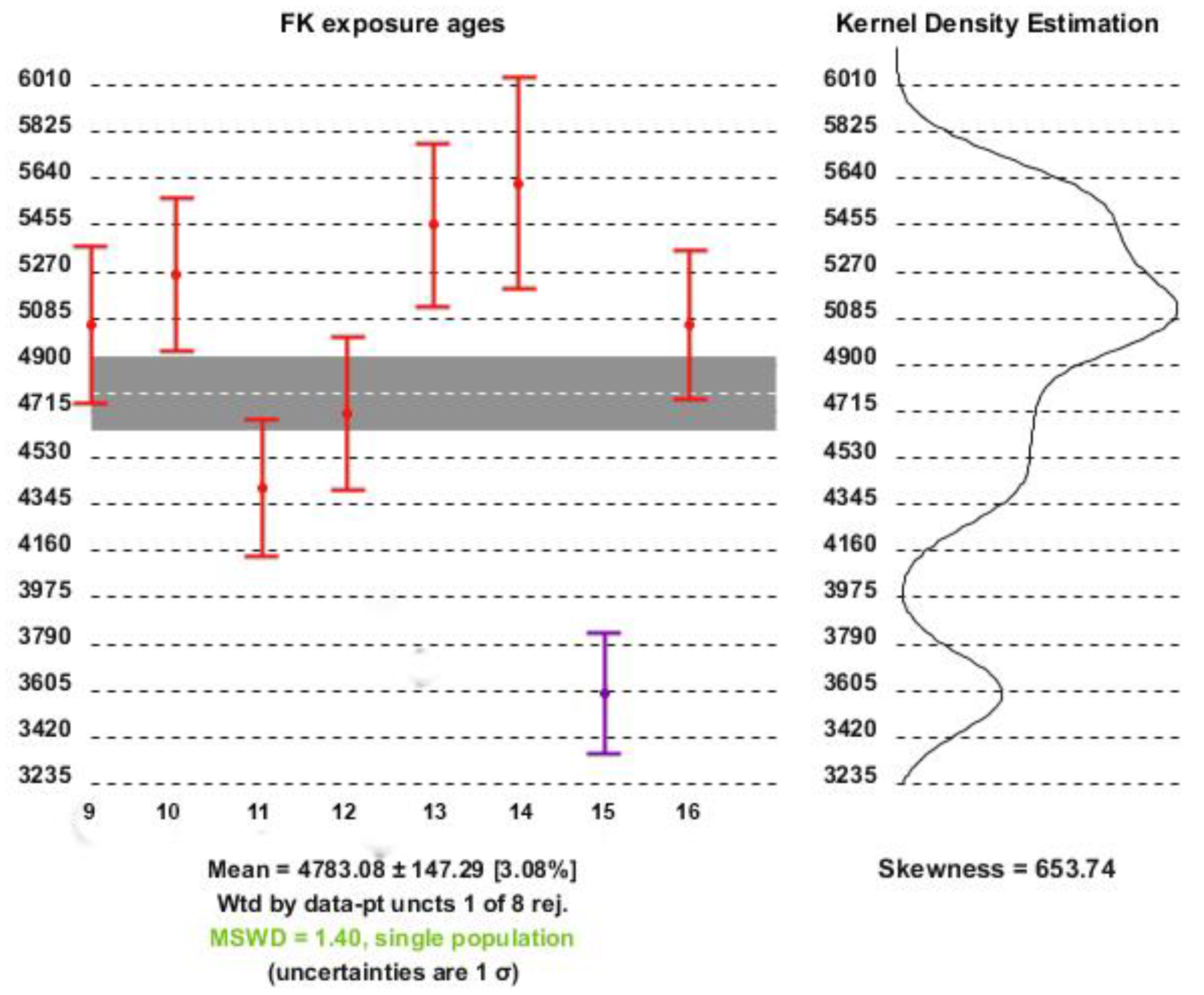
4. Results
| Sample | Lat. | Long. | Elevation | Shielding | Depth | 36Cl | 35Cl | %Ca |
|---|---|---|---|---|---|---|---|---|
| (m a.s.l.) | (m) | (at/g) | (ppm) | |||||
| SK01 | 38.25777 | 20.47303 | 404 | 0.86 | 0 | 735,717 ± 33,685 | 2 | 39.359 |
| SK02 | 38.25797 | 20.47255 | 389 | 0.86 | 0 | 715,670 ± 32,515 | 10 | 40.090 |
| SK03 | 38.25797 | 20.47243 | 386 | 0.86 | 0 | 549,114 ± 25,545 | 8 | 40.139 |
| SK04 | 38.25788 | 20.4722 | 378 | 0.86 | 0 | 741,515 ± 81,627 | 44 | 40.784 |
| SK05 | 38.25776 | 20.4719 | 371 | 0.86 | 0 | 738,992 ± 33517 | 29 | 40.022 |
| SK06 | 38.25781 | 20.47181 | 367 | 0.86 | 0 | 576,172 ± 26,005 | 42 | 39.722 |
| SK07 | 38.25786 | 20.47154 | 358 | 0.86 | 0 | 513,814 ± 22,757 | 22 | 39.825 |
| SK08 | 38.25772 | 20.47133 | 351 | 0.86 | 0 | 658,402 ± 29,933 | 20 | 40.013 |
| FK01 | 38.25772 | 20.47133 | 366 | 0.43 | 0.50 | 306,710 ± 18,086 | 103 | 25.111 |
| FK02 | 38.25409 | 20.4732 | 366 | 0.43 | 0.55 | 295,629 ± 16,159 | 89 | 26.075 |
| FK03 | 38.25409 | 20.4732 | 366 | 0.43 | 0.65 | 265,267 ± 15,606 | 94 | 24.765 |
| FK04 | 38.25409 | 20.4732 | 366 | 0.43 | 0.75 | 268,455 ± 16,396 | 94 | 25.808 |
| FK05 | 38.25409 | 20.4732 | 366 | 0.43 | 0.85 | 262,391 ± 14,733 | 92 | 25.833 |
| FK06 | 38.25409 | 20.4732 | 366 | 0.43 | 0.95 | 260,559 ± 18,793 | 101 | 25.257 |
| FK07 | 38.25409 | 20.4732 | 366 | 0.43 | 1.05 | 222,756 ± 14,504 | 81 | 26.201 |
| FK08 | 38.25409 | 20.4732 | 366 | 0.43 | 1.15 | 238,940 ± 13,327 | 77 | 27.776 |
| Z01 | 38.30198 | 20.46523 | 216 | 0.9 | 0 | 363,183 ± 16,172 | 11 | 39.757 |
| Z02 | 38.30196 | 20.46513 | 218 | 0.9 | 82 | 355,935 ± 16,680 | 32 | 39.818 |
| Z03 | 38.30198 | 20.46504 | 233 | 0.9 | 67 | 293,212 ± 13,239 | 14 | 39.934 |
| Z04 | 38.30203 | 20.4650 | 237 | 0.9 | 63 | 630,575 ± 28,345 | 28 | 39.774 |
| Z05 | 38.30206 | 20.46494 | 238 | 0.9 | 62 | 341,582 ± 15,298 | 24 | 39.791 |
| Z06 | 38.30209 | 20.46483 | 244 | 0.9 | 56 | 628,376 ± 28,353 | 27 | 39.911 |
| Z07 | 38.30225 | 20.46468 | 252 | 0.9 | 48 | 522,870 ± 23,515 | 26 | 39.034 |
| Z08 | 38.30208 | 20.46456 | 255 | 0.9 | 45 | 535,861 ± 25,708 | 15 | 39.508 |
| Z09A | 38.30207 | 20.46414 | 282 | 0.9 | 0 | 775,022 ± 35,348 | 27 | 39.855 |
| Z09B | 38.30193 | 20.46413 | 281 | 0.5 | 1 | 428,512 ± 19,142 | 13 | 40.040 |
| Sample | Νο | Min. Age | Inheritance | Age | Comment | |
|---|---|---|---|---|---|---|
| (ka) | (m/Ma) | (at/g) | ||||
| SK01 | 1 | 28.94 ± 1.33 | 39 ± 1.79 | 663,903 | 1.43 ± 0.07 | |
| SK02 | 2 | 24.19 ± 1.1 | 45.48 ± 2.07 | 737,006 | N.A. | Exces. inher. |
| SK03 | 3 | 18.76 ± 0.87 | 60.45 ± 2.81 | 727,097 | N.A. | Exces. inher. |
| SK04 | 4 | 28.11 ± 3.09 | 43.98 ± 4.84 | 741,515 | N.A. | Ref. sample |
| SK05 | 5 | 25.89 ± 1.17 | 44.4 ± 2.01 | 745,148 | N.A. | Exces. inher. |
| SK06 | 6 | 22.75 ± 1.03 | 56.01 ± 2.53 | 711,452 | N.A. | Exces. inher. |
| SK07 | 7 | 21.05 ± 0.93 | 59.67 ± 2.64 | 670,693 | N.A. | Exces. inher. |
| SK08 | 8 | 25.93 ± 1.18 | 45.97 ± 2.09 | 684,125 | N.A. | Exces. inher. |
| FK01 | 9 | 23.91 ± 1.41 | 96.41 ± 5.68 | 212,878 | 5.06 ± 0.3 | - |
| FK02 | 10 | 25.19 ± 1.38 | 98.5 ± 5.38 | 203,219 | 5.26 ± 0.29 | - |
| FK03 | 11 | 26.47 ± 1.56 | 107.22 ± 6.31 | 186,126 | 4.41 ± 0.26 | - |
| FK04 | 12 | 28.94 ± 1.77 | 110.89 ± 6.77 | 182,608 | 4.71 ± 0.29 | - |
| FK05 | 13 | 29.74 ± 1.67 | 112.97 ± 6.34 | 169,856 | 5.46 ± 0.31 | - |
| FK06 | 14 | 32.65 ± 2.35 | 116.03 ± 8.37 | 162,879 | 5.62 ± 0.41 | - |
| FK07 | 15 | 34.04 ± 2.22 | 134.87 ± 8.78 | 155,170 | 3.6 ± 0.23 | - |
| FK08 | 16 | 37.81 ± 2.11 | 129.48 ± 7.22 | 150,590 | 5.06 ± 0.28 | - |
| Z01 | 17 | 13.21 ± 0.59 | 88.04 ± 3.92 | 616,190 | N.A. | Exces. inher. |
| Z02 | 18 | 15.1 ± 0.71 | 86.3 ± 4.04 | 0 | 33.51 ± 1.57 | - |
| Z03 | 19 | 11.65 ± 0.53 | 106.46 ± 4.81 | 5 | 20.63 ± 0.93 | - |
| Z04 | 20 | 22.89 ± 1.03 | 50.06 ± 2.25 | 9 | 1930.71 ± 86.79 | Steady state |
| Z05 | 21 | 14.4 ± 0.65 | 89.48 ± 4.01 | 11 | 30.84 ± 1.38 | - |
| Z06 | 22 | 24.84 ± 1.12 | 47.8 ± 2.16 | 29 | 2540.84 ± 114.64 | Steady state |
| Z07 | 23 | 25.13 ± 1.13 | 51.31 ± 2.31 | 106 | 194.9 ± 8.76 | Steady state |
| Z08 | 24 | 25.84 ± 1.24 | 49.00 ± 2.35 | 173 | 2367.83 ± 113.6 | Ref. sample |
| Z09A | 25 | 36.07 ± 1.65 | 33.83 ± 1.54 | 775,025 | N.A. | Ref for Z09B |
| Z09B | 26 | 32.18 ± 1.44 | 51.21 ± 2.29 | 412,871 | 1.26 ± 0.06 | 1.26 ± 0.06 |
5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- McKenzie, D.P. Active tectonics of the Alpine-Himalayan belt: The Aegean Sea and surrounding regions. Geophys. J. R. Astr. Soc. 1978, 55, 217–254. [Google Scholar] [CrossRef]
- Le Pichon, X.; Lyberis, N.; Angelier, J.; Renards, V. Tectonics of the hellenic trench: A synthesis of sea-beam and submersible observations. Tectonophysics 1982, 86, 243–274. [Google Scholar] [CrossRef]
- Underhill, J.R. Late Cenozoic Deformation of the Hellenic Foreland 1982, Western Greece. Geol. Soc. Am. Bull. 1989, 101, 613–634. [Google Scholar] [CrossRef]
- Royden, L.H. The tectonic expression slab pull at continental convergent boundaries. Tectonics 1993, 12, 303–325. [Google Scholar] [CrossRef]
- Louvari, E.; Kiratzi, A.A.; Papazachos, B.C. The Cephalonia transform fault and its extension to western Lefkada Island (Greece). Tectonophysics 1999, 308, 223–236. [Google Scholar] [CrossRef]
- Özbakir, A.D.; Govers, R.; Fichtner, A. The Kefalonia Transform Fault: A STEP fault in the making. Tectonophysics 2020, 787, 228471. [Google Scholar] [CrossRef]
- Baker, C.; Hatzfeld, D.; Lyon-Caen, H.; Papadimitriou, E.; Rigo, A. Earthquake mechanisms of the Adriatic Sea and Western Greece: Implications for the oceanic subduction-continental collision transition. Geophys. J. Int. 1997, 131, 559–594. [Google Scholar] [CrossRef]
- Papadimitriou, E.E. Mode of strong earthquake recurrence in the central Ionian Islands (Greece): Possible triggering due to Coulomb stress changes generated by the occurrence of previous strong shocks. Bull. Seismol. Soc. Am. 2002, 92, 3293–3308. [Google Scholar] [CrossRef]
- Papazachos, C.B.; Kiratzi, A.A. A detailed study of the active crustal deformation in the Aegean and surrounding area. Tectonophysics 1996, 253, 129–153. [Google Scholar] [CrossRef]
- Kahle, H.G.; Müller, M.V.; Geiger, A.; Danuser, G.; Mueller, S.; Veis, G.; Billiris, H.; Paradissis, D. The strain field in northwestern Greece and the Ionian Islands: Results inferred from GPS measurements. Tectonophysics 1995, 249, 41–52. [Google Scholar] [CrossRef]
- Cocard, M.; Kahle, H.G.; Peter, Y.; Geiger, A.; Veis, G.; Felekis, S.; Paradissis, D.; Billiris, H. New constraints on the rapid crustal motion of the Aegean region: Recent results inferred from GPS measurements (1993–1998) across the West Hellenic Arc, Greece. Earth Planet. Sci. Lett. 1999, 172, 39–47. [Google Scholar] [CrossRef]
- Kourouklas, C.; Papadimitriou, E.; Karakostas, V. Long-Term Recurrence Pattern and Stress Transfer along the Kefalonia Transform Fault Zone (KTFZ), Greece: Implications in Seismic Hazard Evaluation. Geosciences 2023, 13, 295. [Google Scholar] [CrossRef]
- Scordilis, E.; Karakaisis, G.F.; Karakostas, V.; Panagiotopoulos, D.G.; Comninakis, P.E.; Papazachos, B.C. Evidence for Transform Faulting in the Ionian Sea: The Cephalonia Island earthquake sequence of 1983. Pure Appl. Geophys. 1985, 123, 388–397. [Google Scholar] [CrossRef]
- Kiratzi, A.; Langston, C. Moment tensor inversion of the 1983 January 17 Kefallinia event of Ionian Island (Greece). Geophys. J. Int. 1991, 105, 529–538. [Google Scholar] [CrossRef]
- Papadimitriou, E. Focal mechanisms along the convex side of the Hellenic Arc. Boll. Geof. Teor. Appl. 1993, 140, 401–426. [Google Scholar]
- Triantafyllou, I.; Papadopoulos, G.A. Earthquakes in the Ionian Sea, Greece, Documented from Little-Known Historical Sources: AD 1513–1900. Geosciences 2023, 13, 285. [Google Scholar] [CrossRef]
- Pirazzoli, P.; Stiros, S.; Laborel, J.; Laborel-Deguen, F.; Arnold, M.; Papageorgiou, S.; Morhangel, C. Late-Holocene shoreline changes related to palaeoseismic events in the Ionian Islands, Greece. Holocene 1994, 4, 397–405. [Google Scholar] [CrossRef]
- Stiros, S.; Pirazzoli, P.A.; Laborel, J.; Laborel-Doguen, F. The 1953 earthquake in Cephalonia (Western Hellenic Arc): Coastal uplift and halotectonic faulting. Geophys. J. Int. 1994, 117, 834–849. [Google Scholar] [CrossRef]
- Rondoyianni, T.; Sakellariou, M.; Baskoutas, J.; Christodoulou, N. Evaluation of active faulting and earthquake secondary effects in Lefkada Island, Ionian Sea, Greece: An overview. Nat. Hazards 1994, 61, 843–850. [Google Scholar] [CrossRef]
- Papadopoulos, G.A.; Karastathis, V.K.; Koukouvelas, I.; Sachpazi, M.; Baskoutas, G.; Agalos, A.; Daskalaki, E.; Minadakis, G.; Moshou, A.; Mouzakiotis, A.; et al. The Cephalonia, Ionian Sea (Greece), sequence of strong earthquakes of January–February 2014: A first report. Res. Geophys. 2014, 4, 5441. [Google Scholar] [CrossRef]
- Lekkas, E.L.; Mavroulis, S.D. Earthquake environmental effects and ESI 2007 seismic intensities of the early 2014 Cephalonia (Ionian Sea, Western Greece) earthquakes (January 26 and February 3, Mw 6.0). Nat. Hazards 2014, 78, 1517–1544. [Google Scholar] [CrossRef]
- Valkaniotis, S.; Ganas, A.; Papathanasiou, G.; Papanikolaou, M. Field observations of geological effects triggered by the January–February 2014 Cephalonia (Ionian Sea, Greece) earthquakes. Tectonophysics 2014, 630, 150–157. [Google Scholar] [CrossRef]
- Mavroulis, S.; Lekkas, E. Revisiting the Most Destructive Earthquake Sequence in the Recent History of Greece: Environmental Effects Induced by the 9, 11 and 12 August 1953 Ionian Sea Earthquakes. Appl. Sci. 2021, 11, 8429. [Google Scholar] [CrossRef]
- Mavroulis, S.; Diakakis, M.; Kranis, H.; Vassilakis, E.; Kapetanidis, V.; Spingos, I.; Kaviris, G.; Skourtsos, E.; Voulgaris, N.; Lekkas, E. Inventory of Historical and Recent Earthquake-Triggered Landslides and Assessment of Related Susceptibility by GIS-Based Analytic Hierarchy Process: The Case of Cephalonia (Ionian Islands, Western Greece). Appl. Sci. 2022, 12, 2895. [Google Scholar] [CrossRef]
- Jenkins, D.A.L. Structural development of Western Greece. AAPG Bull. 1972, 56, 128–149. [Google Scholar]
- Underhill, J.R. Triassic Evaporites and Plio-Quaternary Diapirism in Western Greece. J. Geol. Soc. Lond. 1988, 145, 209–282. [Google Scholar] [CrossRef]
- Le Pichon, X.; Angelier, J. The hellenic arc and trench system: A key to the neotectonic evolution of the eastern mediterranean area. Tectonophysics 1979, 60, 1–42. [Google Scholar] [CrossRef]
- Karakostas, V.G.; Papadimitriou, E.E.; Papazachos, C.B. Properties of the 2003 Lefkada, Ionian Islands, Greece, earthquake seismic sequence and seismicity triggering. Bull. Seismol. Soc. Am. 2004, 94, 1976–1981. [Google Scholar] [CrossRef]
- Ganas, A.; Elias, P.; Bozionelos, G.; Papathanassiou, G.; Avallone, A.; Papastergios, A.; Valkaniotis, S.; Parcharidis, I.; Briole, P. Coseismic deformation, field observations and seismic fault of the 17 November 2015 M = 6.5, Lefkada Island, Greece earthquake. Tectonophysics 2016, 687, 210–222. [Google Scholar] [CrossRef]
- Saltogianni, V.; Moschas, F.; Stiros, S. The 2014 Cephalonia Earthquakes: Finite Fault Modeling, Fault Segmentation, Shear and Thrusting at the NW Aegean Arc (Greece). Pure Appl. Geophys. 2018, 175, 4145–4164. [Google Scholar] [CrossRef]
- Svigkas, N.; Atzori, S.; Kiratzi, A.; Tolomei, C.; Antonioli, A.; Papoutsis, I.; Salvi, S.; Kontoes, C. On the Segmentation of the Cephalonia–Lefkada Transform Fault Zone (Greece) from an InSAR Multi-Mode Dataset of the Lefkada 2015 Sequence. Remote Sens. 2019, 11, 1848. [Google Scholar] [CrossRef]
- Royden, L.H.; Papanikolaou, D.J. Slab segmentation and late Cenozoic disruption of the Hellenic arc. Geochem. Geophys. Geosystems 2011, 12, Q03010. [Google Scholar] [CrossRef]
- Hermanns, R.L.; Niedermann, S.; Villanueva Garcia, A.; Sosa Gomez, J.; Strecker, M.R. Neotectonics and catastrophic failure of mountain fronts in the southern intra-Andean Puna Plateau, Argentina. Geology 2001, 29, 619–623. [Google Scholar] [CrossRef]
- Hermanns, R.L.; Strecker, M.R. Structural and lithological controls on large Quaternary rock avalanches (sturzstroms) in arid northwestern Argentina. Geol. Soc. Am. Bull. 1999, 111, 934–948. [Google Scholar] [CrossRef]
- Strecker, M.R.; Marrett, R. Kinematic evolution of fault ramps and its role in development of landslides and lakes in the northwestern Argentine Andes. Geology 1999, 27, 307–310. [Google Scholar] [CrossRef]
- Jackson, L.E., Jr. Landslides and landscape evolution in the Rocky Mountains and adjacent foothills area, southwestern Alberta, Canada. In Catastrophic Landslides: Effects, Occurrence, and Mechanisms; Evans, S.G., Degraff, J.V., Eds.; Reviews in Engineering Geology; Geological Society of America: Boulder, CO, USA, 2002; Volume 15, pp. 325–344. [Google Scholar]
- Agliardi, F.; Zanchi, A.; Crosta, G.B. Tectonic vs. gravitational morphostructures in the central Eastern Alps (Italy): Constraints on the recent evolution of the mountain range. Tectonophysics 2009, 474, 250–270. [Google Scholar] [CrossRef]
- Agliardi, F.; Crosta, G.B.; Zanchi, A.; Ravazzi, C. Onset and timing of deep-seated gravitational slope deformations in the eastern Alps, Italy. Geomorphology 2009, 103, 113–129. [Google Scholar] [CrossRef]
- Penna, I.; Hermanns, R.L.; Folguera, A.; Niedermann, S. Multiple slope failures associated with neotectonic activity in the southern central Andes (37°–37°30′ S). Patagonia, Argentina. Geol. Soc. Am. Bull. 2011, 123, 1880–1895. [Google Scholar] [CrossRef]
- Underhill, J.R. Quest for Ithaca. Geoscientist 2006, 16, 4–29. [Google Scholar]
- Underhill, J. Relocating Odysseus’ Homeland. Nat. Geosci. 2009, 2, 455–458. [Google Scholar] [CrossRef]
- Pérouse, E.; Sébrier, M.; Braucher, R.; Chamot-Rooke, N.; Bourles, D.; Briole, P.; Sorel, D.; Dimitrov, D.; Arsenikos, S. Transition from collision to subduction in Western Greece: The Katouna–Stamna active fault system and regional kinematics. Int. J. Earth Sci. 2017, 106, 967–989. [Google Scholar] [CrossRef]
- Mechernich, S.; Schneiderwind, S.; Mason, J.; Papanikolaou, I.D.; Deligiannakis, G.; Pallikarakis, A.; Binnie, A.S.; Dunai, T.J.; Reicherter, K. The Seismic History of the Pisia Fault (Eastern Corinth Rift, Greece) from Fault Plane Weathering Features and Cosmogenic 36Cl Dating. J. Geophys. Res. Solid Earth 2018, 123, 4266–4284. [Google Scholar] [CrossRef]
- Iezzi, F.; Roberts, G.; Faure Walker, J.; Papanikolaou, I.; Ganas, A.; Deligiannakis, G.; Beck, J.; Wolfers, S.; Gheorghiu, D. Temporal and spatial earthquake clustering revealed through comparison of millennial strain-rates from 36Cl cosmogenic exposure dating and decadal GPS strain-rate. Sci. Rep. 2021, 11, 23320. [Google Scholar] [CrossRef]
- Mechernich, S.; Reicherter, K.; Deligiannakis, G.; Papanikolaou, I. Tectonic geomorphology of active faults in Eastern Crete (Greece) with slip rates and earthquake history from cosmogenic 36Cl dating of the Lastros and Orno faults. Quat. Int. 2023, 30, 77–91. [Google Scholar] [CrossRef]
- Zreda, M.G.; Noller, F.M. Ages of prehistoric earthquakes revealed by cosmogenic chlorine-36 in a bedrock fault scarp at Hebgen Lake. Science 1998, 282, 1097–1099. [Google Scholar] [CrossRef]
- Schlagenhauf, A.; Gaudemer, Y.; Benedetti, L.; Manighetti, I.; Palumbo, L.; Schimmelpfennig, I.; Finkel, R.; Pou, K. Using in situ chlorine-36 cosmonuclide to recover past earthquake histories on limestone normal fault scarps: A reappraisal of methodology and interpretations. Geophys. J. Int. 2010, 182, 36–72. [Google Scholar] [CrossRef]
- Mouslopoulou, V.; Moraetis, D.; Benedetti, L.; Guillou, V.; Bellier, O.; Hristopulos, D. Normal faulting in the forearc of the Hellenic subduction margin: Paleoearthquake history and kinematics of the Spili Fault, Crete, Greece. J. Struct. Geol. 2014, 66, 298–308. [Google Scholar] [CrossRef]
- Benedetti, L.; Finkel, R.; King, G.; Armijo, R.; Papanastasiou, D.; Ryerson, F.J.; Flerit, F.; Farber, D.; Stavrakakis, G. Motion on the Kaparelli fault (Greece) prior to the 1981 earthquake sequence determined from 36Cl cosmogenic dating. Terra Nova 2001, 15, 118–124. [Google Scholar] [CrossRef]
- Benedetti, L.; Finkel, R.; Papanastasiou, D.; King, G.; Armijo, R.; Ryerson, F.J.; Farber, D.; Flerit, F. Post-glacial slip history of the Sparta fault (Greece) determined by 36Cl cosmogenic dating: Evidence for non-periodic earthquakes. Geophys. Res. Lett. 2002, 29, 1246. [Google Scholar] [CrossRef]
- Benavente, C.; Zerathe, S.; Audin, L.; Hall, S.R.; Robert, X.; Delgado, F.; Carcaillet, J.; ASTER Team. Active transpressional tectonics in the Andean forearc of southern Peru quantified by 10Be surface exposure dating of an active fault scarp. Tectonics 2017, 36, 1662–1678. [Google Scholar]
- Bierman, P.R.; Gillespie, A.R.; Caffee, M.W. Cosmogenic ages for earthquake recurrence intervals and debris flow fan deposition, Owens Valley. Science 1995, 270, 447–450. [Google Scholar] [CrossRef]
- Ballantyne, C.K.; Stone, J.O.; Fifield, L.K. Cosmogenic Cl-36 dating of post-glacial landsliding at The Storr 1995, Isle of Skye, Scotland. Holocene 1998, 8, 347–351. [Google Scholar] [CrossRef]
- Sewell, R.J.; Barrows, T.T.; Campbell, S.D.; Fifield, L.K. Exposure dating (10Be, 26Al) of natural terrain landslides in Hong Kong, China. Geol. Soc. Am. Spec. Pap. 2006, 415, 131–146. [Google Scholar]
- Le Roux, O.; Schwartz, S.; Gamond, J.F.; Jongmans, D.; Bourles, D.; Braucher, R.; Mahaney, W.; Carcaillet, J.; Leanni, L. CRE dating on the head scarp of a major landslide (Séchilienne, French Alps), age constraints on Holocene kinematics. Earth Planet. Sci. Lett. 2009, 280, 236–245. [Google Scholar] [CrossRef]
- Merchel, S.; Braucher, R.; Alfimov, V.; Bichler, M.; Bourles, D.L.; Reitner, J. The potential of historic rock avalanches and man-made structures as chlorine-36 production rate calibration sites. Quat. Geochronol. 2013, 18, 54–62. [Google Scholar] [CrossRef]
- Zerathe, S.; Braucher, R.; Lebourg, T.; Bourlès, D.; Manetti, M.; Leanni, L. Dating chert (diagenetic silica) using in-situ produced 10Be: Possible complications revealed through the comparison with 36Cl applied on coexisting limestone. Quat. Geochronol. 2013, 17, 81–93. [Google Scholar] [CrossRef]
- Pánek, T. Recent progress in landslide dating: A global overview. Prog. Phys. Geogr. 2015, 39, 168–198. [Google Scholar] [CrossRef]
- Athanassas, C.D.; Bourlès, D.L.; Bracucher, R.; Druitt, T.H.; Nomikou, P.; Leanni, L. Evidence from cosmic ray exposure (CRE) dating for the existence of a pre-Minoan caldera on Santorini, Greece. Bull. Volcanol. 2016, 78, 35. [Google Scholar] [CrossRef]
- Schwartz, S.; Zerathe, S.; Jongmans, D.; Baillet, L.; Carcailet, J.; Audin, L.; Dumont, T.; Bourlès, D.; Braucher, R.; Lebrouc, V. Cosmic ray exposure dating on the large landslide of Séchilienne (Western Alps): A synthesis to constrain slope evolution. Geomorphology 2017, 278, 329–344. [Google Scholar] [CrossRef]
- Zerathe, S.; Lebourg, T.; Braucher, R.; Bourlès, D. Mid-Holocene cluster of large-scale landslides revealed in the Southwestern Alps by 36Cl dating. Insight on an Alpine-scale landslide activity. Quat. Sci. Rev. 2014, 90, 106–127. [Google Scholar] [CrossRef]
- British Petroleum Company. The Geological Results of Petroleum Exploration in Western Greece: Institute of Geology Subsurface Research. Athens 1971, 10, 73. [Google Scholar]
- Gosgrove, J.W. The association of folds and fractures and the link between folding, fracturing and fluid flow during the evolution of a fold–thrust belt: A brief review. In Industrial Structural Geology: Principles, Techniques and Integration; Richards, F.L., Richardson, N.J., Rippington, S.J., Wilson, R.W., Bond, C.E., Eds.; Geological Society of London, Special Publications: Bath, UK, 2015; Volume 421. [Google Scholar]
- Lal, D. Cosmic ray labeling of erosion surfaces: In situ nuclide production rates and erosion models. Earth Planet. Sci. Lett. 1991, 104, 424–439. [Google Scholar] [CrossRef]
- Stone JO, H.; Evans, J.M.; Fifield, L.K.; Allan, G.L.; Cresswell, R.G. Cosmogenic Chlorine-36 Production in Calcite by Muons. Geochim. Cosmochim. Acta 1998, 62, 433–454. [Google Scholar] [CrossRef]
- Stone, J.O. Air pressure and cosmogenic isotope production. J. Geophys. Res. 2000, 105, 23753–23759. [Google Scholar] [CrossRef]
- Cowie, P.A.; Phillips, R.J.; Roberts, G.P.; McCaffrey, K.; Zijerveld, L.J.J.; Gregory, L.C.; Faure Walker, J.; Wedmore, L.N.J.; Dunai, T.J.; Binnie, S.A.; et al. Orogen-scale uplift in the central Italian Apennines drives episodic behaviour of earthquake faults. Sci. Rep. 2017, 7, 44858. [Google Scholar] [CrossRef]
- Beck, J.; Wolfers, S.; Roberts, G.P. Bayesian earthquake dating and seismic hazard assessment using chlorine-36 measurements (BED v1). Geosci. Model. Dev. 2018, 11, 4383–4387. [Google Scholar] [CrossRef]
- Tesson, J.; Benedetti, L. Seismic history from in situ 36Cl cosmogenic nuclide data on limestone fault scarps using Bayesian reversible jump Markov chain Monte Carlo. Quat. Geochronol. 2019, 52, 1–20. [Google Scholar] [CrossRef]
- Tikhomirov, D.; Amiri, N.M.; Ivy-Ochs, S.; Alfimov, V.; Vockenhuber, C.; Akçar, N. Fault Scarp Dating Tool—A MATLAB code for fault scarp dating using in-situ chlorine-36 supplemented with datasets of Yavansu and Kalafat faults. Data Brief 2019, 26, 104476. [Google Scholar] [CrossRef]
- Goodall, H.J.; Gregory, L.C.; Wedmore, L.N.J.; McCaffrey, K.J.W.; Amey, R.M.J.; Roberts, G.P.; Shanks, R.P.; Phillips, R.J.; Hooper, A. Determining Histories of Slip on Normal Faults With Bedrock Scarps Using Cosmogenic Nuclide Exposure Data. Tectonics 2021, 40, e2020TC006457. [Google Scholar] [CrossRef]
- Sanchez, G.; Rolland, Y.; Corsini, M.; Braucher, R.; Bourlès, D.; Arnold, M.; Aumaître, G. Relationships between tectonics, slope instability and climate change: Cosmic ray exposure dating of active faults, landslides and glacial surfaces in the SW Alps. Geomorphology 2010, 117, 1–13. [Google Scholar] [CrossRef]
- Hippolyte, J.C.; Bourlès, D.; Léanni, L.; Braucher, R.; Chauvet, F.; Lebatard, A.E. 10Be ages reveal >12 ka of gravitational movement in a major sackung of the Western Alps (France). Geomorphology 2012, 171–172, 139–153. [Google Scholar] [CrossRef]
- Cardinal, T.; Audin, L.; Rolland, Y.; Schwartz, S.; Petit, C.; Zerathe, S.; Borgniet, L.; Braucher, R.; Nomade, J.; Dumont, T.; et al. Interplay of fluvial incision and rockfalls in shaping periglacial mountain gorges. Geomorphology 2021, 381, 107665. [Google Scholar] [CrossRef]
- Cardinal, T.; Rolland, Y.; Petit, C.; Audin, L.; Zerathe, S.; Schwartz, S.; ASTER Team. Fluvial bedrock gorges as markers for Late-Quaternary tectonic and climatic forcing in the French Southwestern Alps. Geomorphology 2022, 418, 108476. [Google Scholar] [CrossRef]
- Arnold, M.; Merchel, S.; Bourlès, D.L.; Braucher, R.; Benedetti, L.; Finkel, R.C.; Aumaître, G.; Gottdang, A.; Klein, M. The French accelerator mass spectrometry facility ASTER: Improved performance and developments. Nucl. Instrum. Methods Phys. Res. B 2010, 268, 1954–1959. [Google Scholar] [CrossRef]
- Merchel, S.; Bremser, W.; Alfimov, V.; Arnold, M.; Aumaître, G.; Benedetti, L.; Bourlès, D.L.; Caffee, M.; Fifield, L.K.; Finkel, R.C.; et al. Ultra-trace analysis of 36Cl by accelerator mass spectrometry: An interlaboratory study. Anal. Bioanal. Chem. 2011, 400, 3125–3132. [Google Scholar] [CrossRef]
- Schimmelpfennig, I.; Benedetti, L.; Finkel, R.; Pik, R.; Blard, P.H.; Bourlès, D.; Burnard, P.; Williams, A. Sources of in situ 36Cl in basaltic rocks. Implications for calibration of production rates. Quat Geochronol. 2009, 4, 441–461. [Google Scholar] [CrossRef]
- Schimmelpfenning, I.; Benedetti, L.; Garreta, V.; Pik, R.; Blard, H.P.; Burnard, P.; Bourlès, D.; Ammon, K.; Dunai, T. Calibration of cosmogenic 36Cl production rates from Ca and K spallation in lava flows from Mt. Etna (38° N 2011, Italy) and Payun Matru (36° S, Argentina). Geochim. Cosmochim. Acta 2011, 75, 2611–2632. [Google Scholar] [CrossRef]
- Schimmelpfenning, I.; Schaefer, J.M.; Akçar, N.; Koffman, T.; Ivy-Ochs, S.; Schwartz, R.; Finkel, R.C.; Zimmerman, S.; Schlüchter, C. A chronology of Holocene and Little Ice Age glacier culminations of the Steingletscher, Central Alps, Switzerland, based on high-sensitivity beryllium-10 moraine dating. Earth Planet. Sci. Lett. 2014, 393, 220–230. [Google Scholar] [CrossRef]
- Fink, D.; Voght, S.; Hotchkis, M. Cross-sections for 36Cl from Ti at Ep = 35–150 MeV: Applications to in-situ exposure dating. Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. Atoms. 2000, 172, 861–866. [Google Scholar] [CrossRef]
- Stone, J.O.; Fifield, K.; Vasconcelos, P. Terrestrial chlorine-36 production from spallation of iron. In Proceedings of the 10th International Conference on Accelerator Mass Spectrometry, Berkeley, CA, USA, 3–9 September 2005. [Google Scholar]
- Marrero, S.M.; Phillips, F.M.; Brochers, B.; Lifton, N.; Aumer, R.; Balco, G. Cosmogenic nuclide systematics and the CRONUScalc program. Quarter. Geochronol. 2016, 31, 160–187. [Google Scholar] [CrossRef]
- Hashemi, A.; Sarıkaya, M.A.; Wilcken, K.M.; Öztürk, M.Z. Controls on long-term denudation rate of carbonate terrains in the Eastern Mediterranean. Quat. Sci. Rev. 2023, 321, 108351. [Google Scholar] [CrossRef]
- Krklec, K. Long-term denudation rate of karstic North Dalmatian Plain (Croatia) calculated from 36Cl cosmogenic nuclides. Geomorphology 2022, 413, 108358. [Google Scholar] [CrossRef]
- Krklec KDomínguez-Villar, D.; Perica, D. Use of rock tablet method to measure rock weathering and landscape denudation. Earth Sci. Rev. 2021, 212, 103449. [Google Scholar] [CrossRef]
- Desormeaux, C.; Godard, V.; Lague, D.; Duclaux, G.; Fleury, J.; Benedetti, L.; Bellier, O.; ASTER Team. Investigation of stochastic-threshold incision models across a climatic and morphological gradient. Earth Surf. Dynam. 2022, 10, 473–492. [Google Scholar] [CrossRef]
- Spencer, C.J.; Yakymchuk, C.; Ghaznavi, M. Visualising data distributions with kernel density estimation and reduced chi-squared statistic. Geosci. Front. 2017, 8, 1247–1252. [Google Scholar] [CrossRef]
- Hilger, P.; Hermanns, R.L.; Gosse, J.C.; Jacobs, B.; Etzelmüller, B.; Krautblatter, M. Multiple rock-slope failures from Mannen in Romsdal Valley, western Norway, revealed from Quaternary geological mapping and 10Be exposure dating. Holocene 2018, 28, 1841–1854. [Google Scholar] [CrossRef]
- Lenart, J.; Kašing, M.; Pánek, T.; Braucher, R.; Kuda, F. Rare, slow but impressive: >43 ka of rockslide in river canyon incising crystalline rocks of the eastern Bohemian Massif. Landslides 2023, 20, 1705–1718. [Google Scholar] [CrossRef]
- Lekkas, E.; Mavroulis, S. Fault zones ruptured during the early 2014 Cephalonia Island (Ionian Sea, Western Greece) earthquakes (January 26 and February 23, Mw 6.0) based on the associated co-seismic surface ruptures. J. Seismol. 2016, 20, 63–78. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Athanassas, C.D.; Braucher, R.; Vakalas, I.; Apostolopoulos, G. Records of Ground Deformation in Northern Kefalonia Inferred from Cosmogenic 36Cl Geochronology. Geosciences 2025, 15, 94. https://doi.org/10.3390/geosciences15030094
Athanassas CD, Braucher R, Vakalas I, Apostolopoulos G. Records of Ground Deformation in Northern Kefalonia Inferred from Cosmogenic 36Cl Geochronology. Geosciences. 2025; 15(3):94. https://doi.org/10.3390/geosciences15030094
Chicago/Turabian StyleAthanassas, Constantin D., Regis Braucher, Ioannis Vakalas, and George Apostolopoulos. 2025. "Records of Ground Deformation in Northern Kefalonia Inferred from Cosmogenic 36Cl Geochronology" Geosciences 15, no. 3: 94. https://doi.org/10.3390/geosciences15030094
APA StyleAthanassas, C. D., Braucher, R., Vakalas, I., & Apostolopoulos, G. (2025). Records of Ground Deformation in Northern Kefalonia Inferred from Cosmogenic 36Cl Geochronology. Geosciences, 15(3), 94. https://doi.org/10.3390/geosciences15030094








