Optical Soliton Solutions of the Cubic-Quartic Nonlinear Schrödinger and Resonant Nonlinear Schrödinger Equation with the Parabolic Law
Abstract
:Featured Application
Abstract
1. Introduction
2. Instructions for the Methods
3. Application to the Expansion Method
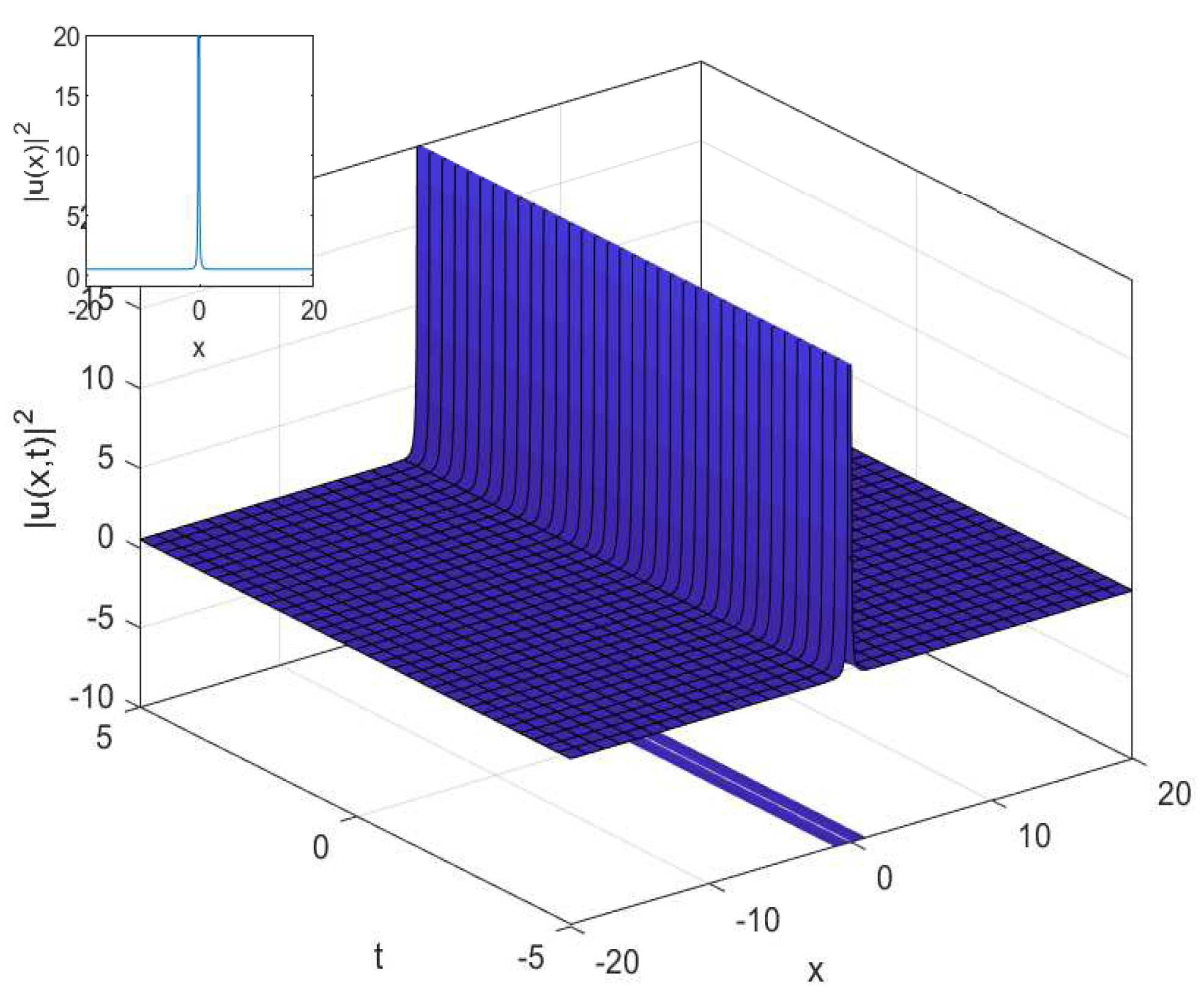
3.1. The Cubic-Quartic Nonlinear Schrödinger Equation
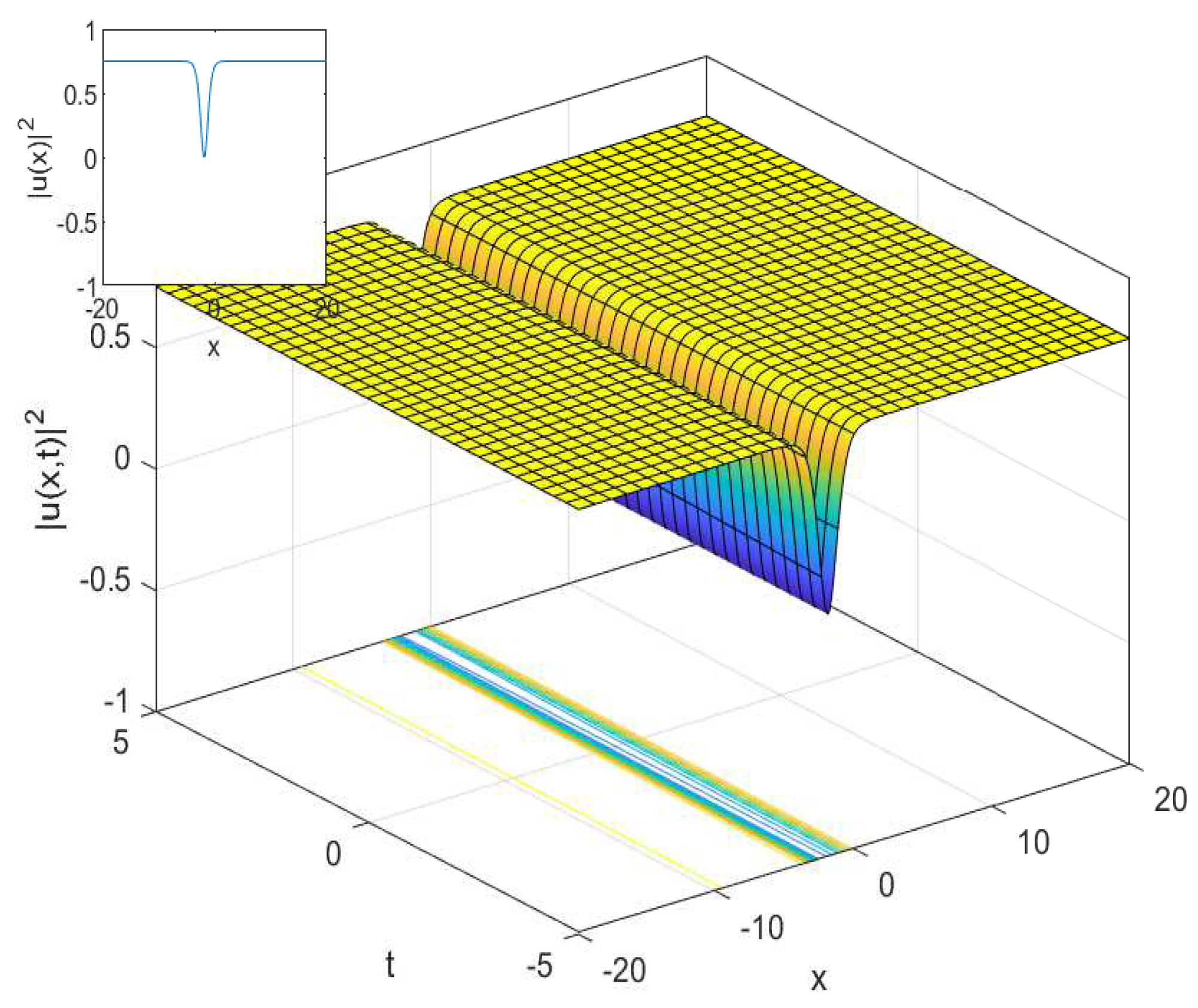
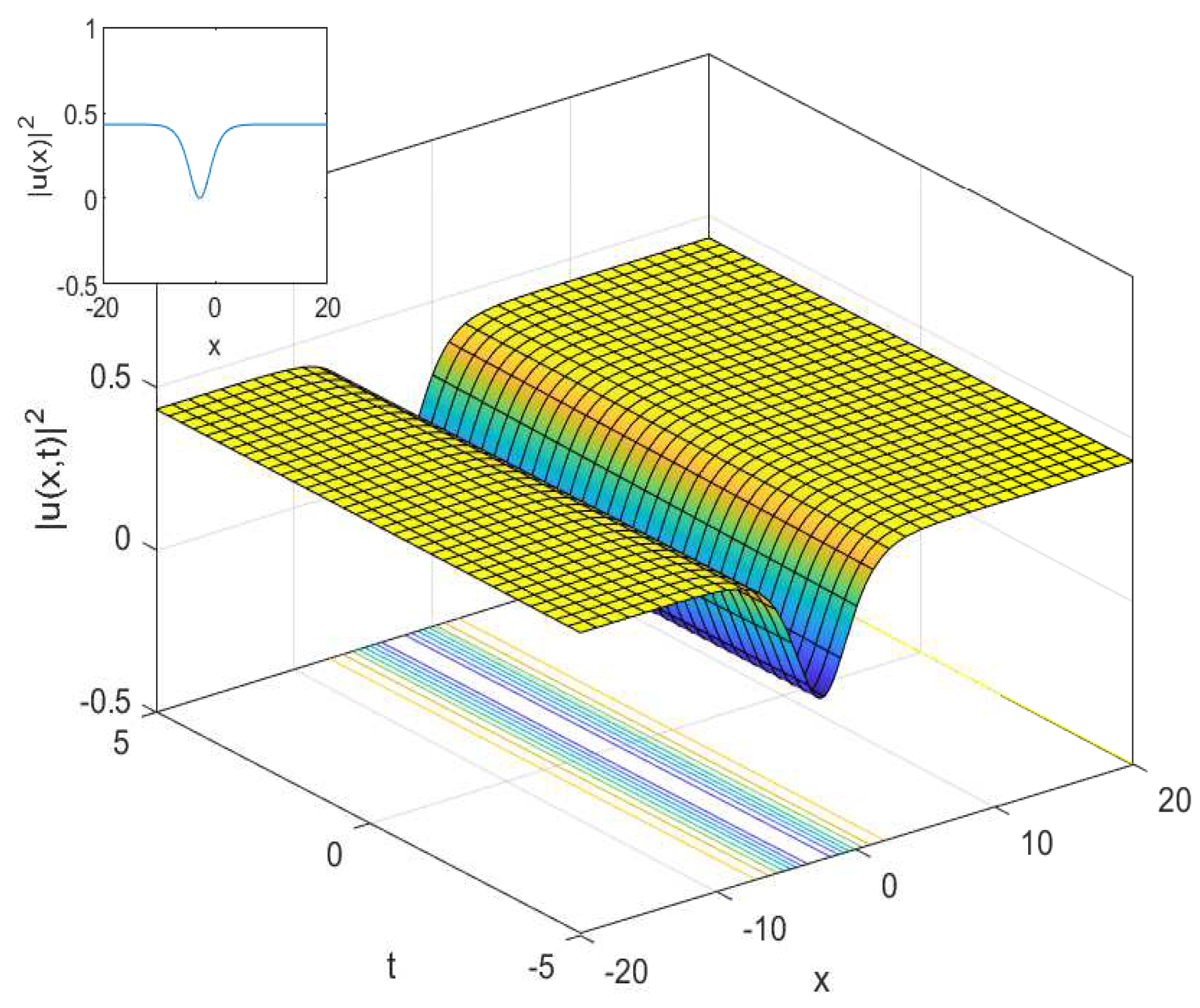
3.2. The Cubic-Quartic Resonant Nonlinear Schrödinger Equation
4. Application to the Expansion Method
4.1. The Cubic-Quartic Nonlinear Schrödinger Equation
4.2. The Cubic-Quartic Resonant Nonlinear Schrödinger Equation
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| NLPDE | Nonlinear partial differential equation |
| ODE | Ordinary differential equation |
References
- Plastino, A. Entropic aspects of nonlinear partial differential equations: Classical and quantum mechanical perspectives. Entropy 2017, 19, 166. [Google Scholar] [CrossRef] [Green Version]
- Ilhan, O.A.; Esen, A.; Bulut, H.; Baskonus, H.M. Singular solitons in the pseudo-parabolic model arising in nonlinear surface waves. Results Phys. 2019, 12, 1712–1715. [Google Scholar] [CrossRef]
- Arshad, M.; Seadawy, A.R.; Lu, D. Exact bright–dark solitary wave solutions of the higher-order cubic–quintic nonlinear Schrödinger equation and its stability. Optik 2017, 138, 40–49. [Google Scholar] [CrossRef]
- Arshad, M.; Seadawy, A.R.; Lu, D. Elliptic function and solitary wave solutions of the higher-order nonlinear Schrödinger dynamical equation with fourth-order dispersion and cubic-quintic nonlinearity and its stability. Eur. Phys. J. Plus 2017, 132, 371. [Google Scholar] [CrossRef]
- Yousif, M.A.; Mahmood, B.A.; Ali, K.K.; Ismael, H.F. Numerical simulation using the homotopy perturbation method for a thin liquid film over an unsteady stretching sheet. Int. J. Pure Appl. Math. 2016, 107, 289–300. [Google Scholar] [CrossRef] [Green Version]
- Baskonus, H.M.; Bulut, H. On the numerical solutions of some fractional ordinary differential equations by fractional Adams-Bashforth-Moulton method. Open Math. 2015, 13, 547–556. [Google Scholar] [CrossRef]
- Ismael, H.F. Carreau-Casson fluids flow and heat transfer over stretching plate with internal heat source/sink and radiation. Int. J. Adv. Appl. Sci. 2017, 4, 11–15. [Google Scholar] [CrossRef]
- Ali, K.K.; Ismael, H.F.; Mahmood, B.A.; Yousif, M.A. MHD Casson fluid with heat transfer in a liquid film over unsteady stretching plate. Int. J. Adv. Appl. Sci. 2017, 4, 55–58. [Google Scholar] [CrossRef]
- Ismael, H.F.; Arifin, N.M. Flow and heat transfer in a maxwell liquid sheet over a stretching surface with thermal radiation and viscous dissipation. JP J. Heat Mass Transf. 2018, 15, 847–866. [Google Scholar] [CrossRef]
- Zeeshan, A.; Ismael, H.F.; Yousif, M.A.; Mahmood, T.; Rahman, S.U. Simultaneous Effects of Slip and Wall Stretching/Shrinking on Radiative Flow of Magneto Nanofluid Through Porous Medium. J. Magn. 2018, 23, 491–498. [Google Scholar] [CrossRef]
- Gao, W.; Partohaghighi, M.; Baskonus, H.M.; Ghavi, S. Regarding the group preserving scheme and method of line to the numerical simulations of Klein–Gordon model. Results Phys. 2019, 15, 102555. [Google Scholar] [CrossRef]
- Yokus, A.; Baskonus, H.M.; Sulaiman, T.A.; Bulut, H. Numerical simulation and solutions of the two-component second order KdV evolutionarysystem. Numer. Methods Partial Differ. Equ. 2018, 34, 211–227. [Google Scholar] [CrossRef]
- Sulaiman, T.A.; Bulut, H.; Yokus, A.; Baskonus, H.M. On the exact and numerical solutions to the coupled Boussinesq equation arising in ocean engineering. Indian J. Phys. 2019, 93, 647–656. [Google Scholar] [CrossRef]
- Bulut, H.; Ergüt, M.; Asil, V.; Bokor, R.H. Numerical solution of a viscous incompressible flow problem through an orifice by Adomian decomposition method. Appl. Math. Comput. 2004, 153, 733–741. [Google Scholar] [CrossRef]
- Ismael, H.F.; Ali, K.K. MHD casson flow over an unsteady stretching sheet. Adv. Appl. Fluid Mech. 2017, 20, 533–541. [Google Scholar] [CrossRef]
- Baskonus, H.M.; Bulut, H.; Sulaiman, T.A. New Complex Hyperbolic Structures to the Lonngren-Wave Equation by Using Sine-Gordon Expansion Method. Appl. Math. Nonlinear Sci. 2019, 4, 141–150. [Google Scholar] [CrossRef] [Green Version]
- Cattani, C.; Sulaiman, T.A.; Baskonus, H.M.; Bulut, H. On the soliton solutions to the Nizhnik-Novikov- Veselov and the Drinfel’d-Sokolov systems. Opt. Quantum Electron. 2018, 50, 138. [Google Scholar] [CrossRef]
- Eskitaşçıoğlu, E.İ.; Aktaş, M.B.; Baskonus, H.M. New Complex and Hyperbolic Forms for Ablowitz–Kaup– Newell–Segur Wave Equation with Fourth Order. Appl. Math. Nonlinear Sci. 2019, 4, 105–112. [Google Scholar] [CrossRef] [Green Version]
- Gao, W.; Ismael, F.H.; Bulut, H.; Baskonus, H.M. Instability modulation for the (2+1)-dimension paraxial wave equation and its new optical soliton solutions in Kerr media. Phys. Scr. 2019. [Google Scholar] [CrossRef]
- Houwe, A.; Hammouch, Z.; Bienvenue, D.; Nestor, S.; Betchewe, G. Nonlinear Schrödingers equations with cubic nonlinearity: M-derivative soliton solutions by exp(-Φ(ξ))-Expansion method. Preprints 2019. [Google Scholar] [CrossRef]
- Cattani, C.; Sulaiman, T.A.; Baskonus, H.M.; Bulut, H. Solitons in an inhomogeneous Murnaghan’s rod. Eur. Phys. J. Plus 2018, 133, 228. [Google Scholar] [CrossRef]
- Baskonus, H.M.; Yel, G.; Bulut, H. Novel wave surfaces to the fractional Zakharov-Kuznetsov-Benjamin- Bona-Mahony equation. Proc. Aip Conf. Proc. 2017, 1863, 560084. [Google Scholar]
- Baskonus, H.M.; Bulut, H. Exponential prototype structures for (2+1)-dimensional Boiti-Leon-Pempinelli systems in mathematical physics. Waves Random Complex Media 2016, 26, 189–196. [Google Scholar] [CrossRef]
- Abdelrahman, M.A.E.; Sohaly, M.A. The Riccati-Bernoulli Sub-ODE Technique for Solving the Deterministic (Stochastic) Generalized-Zakharov System. Int. J. Math. Syst. Sci. 2018, 1. [Google Scholar] [CrossRef] [Green Version]
- Gao, W.; Ismael, H.F.; Mohammed, S.A.; Baskonus, H.M.; Bulut, H. Complex and real optical soliton properties of the paraxial nonlinear Schrödinger equation in Kerr media with M-fractional. Front. Phys. 2019, 7, 197. [Google Scholar] [CrossRef] [Green Version]
- Hammouch, Z.; Mekkaoui, T.; Agarwal, P. Optical solitons for the Calogero-Bogoyavlenskii-Schiff equation in (2 + 1) dimensions with time-fractional conformable derivative. Eur. Phys. J. Plus 2018, 133, 248. [Google Scholar] [CrossRef]
- Manafian, J.; Aghdaei, M.F. Abundant soliton solutions for the coupled Schrödinger-Boussinesq system via an analytical method. Eur. Phys. J. Plus 2016, 131, 97. [Google Scholar] [CrossRef]
- Guo, L.; Zhang, Y.; Xu, S.; Wu, Z.; He, J. The higher order rogue wave solutions of the Gerdjikov-Ivanov equation. Phys. Scr. 2014, 89, 035501. [Google Scholar] [CrossRef]
- Ling, L.; Feng, B.F.; Zhu, Z. General soliton solutions to a coupled Fokas–Lenells equation. Nonlinear Anal. Real World Appl. 2018, 40, 185–214. [Google Scholar] [CrossRef] [Green Version]
- Miah, M.M.; Ali, H.M.S.; Akbar, M.A.; Seadawy, A.R. New applications of the two variable G′/G,1/G)-expansion method for closed form traveling wave solutions of integro-differential equations. J. Ocean Eng. Sci. 2019. [Google Scholar] [CrossRef]
- Miah, M.M.; Ali, H.M.S.; Akbar, M.A.; Wazwaz, A.M. Some applications of the (G′/G,1/G)-expansion method to find new exact solutions of NLEEs. Eur. Phys. J. Plus 2017, 132, 252. [Google Scholar] [CrossRef]
- Yokuş, A. Comparison of Caputo and conformable derivatives for time-fractional Korteweg–de Vries equation via the finite difference method. Int. J. Mod. Phys. B 2018, 32, 1850365. [Google Scholar] [CrossRef]
- Yokuş, A.; Kaya, D. Conservation laws and a new expansion method for sixth order Boussinesq equation. Proc. AIP Conf. Proc. 2015, 1676, 020062. [Google Scholar]
- Yang, X.; Yang, Y.; Cattani, C.; Zhu, C.M. A new technique for solving the 1-D Burgers equation. Therm. Sci. 2017, 21, 129–136. [Google Scholar] [CrossRef] [Green Version]
- Vakhnenko, V.O.; Parkes, E.J.; Morrison, A.J. A Bäcklund transformation and the inverse scattering transform method for the generalised Vakhnenko equation. Chaos Solitons Fractals 2003, 17, 683–692. [Google Scholar] [CrossRef]
- Jawad, A.J.M.; Abu-AlShaeer, M.J.; Biswas, A.; Zhou, Q.; Moshokoa, S.; Belic, M. Optical solitons to Lakshmanan-Porsezian-Daniel model for three nonlinear forms. Optik 2018, 160, 197–202. [Google Scholar] [CrossRef]
- Biswas, A.; Triki, H.; Zhou, Q.; Moshokoa, S.P.; Ullah, M.Z.; Belic, M. Cubic–quartic optical solitons in Kerr and power law media. Optik 2017, 144, 357–362. [Google Scholar] [CrossRef]
- Xie, Y.; Yang, Z.; Li, L. New exact solutions to the high dispersive cubic–quintic nonlinear Schrödinger equation. Phys. Lett. Sect. A Gen. At. Solid State Phys. 2018, 382, 2506–2514. [Google Scholar] [CrossRef]
- Li, H.M.; Xu, Y.S.; Lin, J. New optical solitons in high-order dispersive cubic-quintic nonlinear Schrödinger equation. Commun. Theor. Phys. 2004, 41, 829. [Google Scholar]
- Nawaz, B.; Ali, K.; Abbas, S.O.; Rizvi, S.T.R.; Zhou, Q. Optical solitons for non-Kerr law nonlinear Schrödinger equation with third and fourth order dispersions. Chin. J. Phys. 2019, 60, 133–140. [Google Scholar] [CrossRef]
- Biswas, A.; Arshed, S. Application of semi-inverse variational principle to cubic-quartic optical solitons with kerr and power law nonlinearity. Optik 2018, 172, 847–850. [Google Scholar] [CrossRef]
- Seadawy, A.R.; Kumar, D.; Chakrabarty, A.K. Dispersive optical soliton solutions for the hyperbolic and cubic-quintic nonlinear Schrödinger equations via the extended sinh-Gordon equation expansion method. Eur. Phys. J. Plus 2018, 133, 182. [Google Scholar] [CrossRef]
- El-Dessoky, M.M.; Islam, S. Chirped Solitons in Generalized Resonant Dispersive Nonlinear Schrödinger’s equation. Comput. Sci. 2019, 14, 737–752. [Google Scholar]
- Bansal, A.; Biswas, A.; Zhou, Q.; Babatin, M.M. Lie symmetry analysis for cubic–quartic nonlinear Schrödinger’s equation. Optik 2018, 169, 12–15. [Google Scholar] [CrossRef]
- Biswas, A.; Kara, A.H.; Ullah, M.Z.; Zhou, Q.; Triki, H.; Belic, M. Conservation laws for cubic–quartic optical solitons in Kerr and power law media. Optik 2017, 145, 650–654. [Google Scholar] [CrossRef]
- Younis, M.; Cheemaa, N.; Mahmood, S.A.; Rizvi, S.T.R. On optical solitons: The chiral nonlinear Schrödinger equation with perturbation and Bohm potential. Opt. Quantum Electron. 2016, 48, 542. [Google Scholar] [CrossRef]
- Hosseini, K.; Samadani, F.; Kumar, D.; Faridi, M. New optical solitons of cubic-quartic nonlinear Schrödinger equation. Optik 2018, 157, 1101–1105. [Google Scholar] [CrossRef]
- Conte, R.; Musette, M. Elliptic general analytic solutions. Stud. Appl. Math. 2009, 123, 63–81. [Google Scholar] [CrossRef]














© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, W.; Ismael, H.F.; Husien, A.M.; Bulut, H.; Baskonus, H.M. Optical Soliton Solutions of the Cubic-Quartic Nonlinear Schrödinger and Resonant Nonlinear Schrödinger Equation with the Parabolic Law. Appl. Sci. 2020, 10, 219. https://doi.org/10.3390/app10010219
Gao W, Ismael HF, Husien AM, Bulut H, Baskonus HM. Optical Soliton Solutions of the Cubic-Quartic Nonlinear Schrödinger and Resonant Nonlinear Schrödinger Equation with the Parabolic Law. Applied Sciences. 2020; 10(1):219. https://doi.org/10.3390/app10010219
Chicago/Turabian StyleGao, Wei, Hajar Farhan Ismael, Ahmad M. Husien, Hasan Bulut, and Haci Mehmet Baskonus. 2020. "Optical Soliton Solutions of the Cubic-Quartic Nonlinear Schrödinger and Resonant Nonlinear Schrödinger Equation with the Parabolic Law" Applied Sciences 10, no. 1: 219. https://doi.org/10.3390/app10010219
APA StyleGao, W., Ismael, H. F., Husien, A. M., Bulut, H., & Baskonus, H. M. (2020). Optical Soliton Solutions of the Cubic-Quartic Nonlinear Schrödinger and Resonant Nonlinear Schrödinger Equation with the Parabolic Law. Applied Sciences, 10(1), 219. https://doi.org/10.3390/app10010219





