Acute Effects of Midsole Bending Stiffness on Lower Extremity Biomechanics during Layup Jumps

Abstract

Purpose: This study aims to investigate the acute effects of shoe midsole stiffness on the joint biomechanics of the lower extremities during specific basketball movements. Methods: Thirty participants wearing stiff midsole shoes (SS) and control shoes (CS) performed layup jumps (LJs) while the kinematics and ground reaction forces were simultaneously collected via the Vicon motion capture system and Kistler force plates. Furthermore, the joint angles, range of motion (ROM), joint power, joint energy, and jump height were calculated. Results: No significant differences were observed between SS and CS conditions for both jump height and the metatarsophalangeal (MTP) joint biomechanics except that the minimum angular velocity of the MTP joint was significantly lower in SS the condition. However, the ROM in the ankle joint was significantly greater in the SS condition than in the CS condition (p < 0.05). Additionally, the maximum plantarflexion power, energy absorption (EA), and energy generation (EG) in the ankle joint were significantly greater in the SS condition than in the CS condition (p < 0.05). Compared with the CS condition, jump height in the SS condition did not increase. Conclusion: During a single LJ, the longitudinal midsole stiffness did not influence the jump height and MTP joint biomechanical patterns but significantly increased the maximum power, EA, and EG during the push-off phase of the ankle joint. These preliminary results indicate that wearing SS could change the ankle joint mechanical patterns by modulating the lower extremity kinetic chain, and may enhance muscle strength in the ankle.

1. Introduction

Vertical jumping is a critical individual competitive ability for basketball players [1]. Outstanding vertical jumping performance enables basketball players to obtain an advantage in terms of avoiding defenders and placing the ball through the basket [2,3,4]. When a player drives towards the basket, a common strategy is shooting with a layup for short contact time, which will likely disrupt the defense of the opposing team [2,3,4,5]. Therefore, enhancing the layup jump (LJ) ability is essential for basketball players to improve their competitive ability.

Based on existing literature, approaches to enhancing the LJ ability focus on developing training protocols. Young et al. [6] showed that speed and strength are related to LJ ability. However, existing studies have indicated that specific footwear can affect the biomechanical characteristics of the lower extremities and performance during LJs [7,8]. Yang et al. [8] demonstrated that a high collar height would affect the partial kinetics of the ankle joint in the push-off phase of an LJ. Therefore, optimal footwear may change the lower extremity biomechanical characteristics and improve the player’s performance.

In recent studies, the optimization of shoe construction around the metatarsophalangeal (MTP) joint for improved performance has attracted considerable attention from researchers [9,10]. From a biomechanical perspective, the MTP joint is primarily used to dorsiflex in the stance phase, in which the MTP joint absorbs large amounts of energy, while energy generation (EG) is close to zero [10]. The reduction in MTP joint energy loss can significantly improve the performance in the lower extremities. Several studies have proven that increasing the stiffness in the MTP joint can significantly reduce the energy loss in the MTP joint, modulate the pattern of the lower extremity kinetic chain, and improve running performance. Willwacher et al. [11] proposed that the stiff structure around the MTP joint shifts the point-of-force application to the front edge of the shoe–ground contact plane and significantly reduces the negative work. Their results showed that a stiff midsole modulates the reaction force leverage and angular velocity, and subsequently, changes the work condition and power generation capability.

Moreover, Oh et al. [12] indicated that the increased bending stiffness of the MTP joint improves the angular impulse of the MTP, ankle, knee, and hip joints, and enhances the running energetics. In contrast, the influence of stiff midsole shoes (SS) on the lower extremity biomechanics in jumping is limited and inconsistent. Tinoco et al. [13] investigated the effect of stiff midsoles on jumping performance, and stated that it is likely to enhance the jumping performance under fatigue conditions. However, Lam et al. [14] stated that no significant difference existed between the performance of sprint and vertical jumping when 17 collegiate athletes performed 5 m sprints with medial plate shoes. Similarly, Worobets et al. [15] did not find significant changes in concentric squat jumping from a fixed position when three types of shoes with different stiffness levels were tested. Studies on the effect of shoes with a stiff midsole on the lower extremities while performing LJs are limited. Moreover, the effect of shoe midsole stiffness on the kinematics and kinetic energy of the lower extremity chain or performance of LJs remains unclear.

Thus, the present study aimed to determine the effects of different levels of midsole bending stiffness on the performance and biomechanical characteristics of the lower extremities during specific basketball movements, such as LJs, by using basketball shoes with different stiffness levels. We tested the hypothesis that wearing SS reduces the range of motion (ROM) in the MTP joint, increases the ROM in the ankle, knee, and hip joints, correspondingly affects the joint energy of these joints and jump height, and enhances the muscular strength of lower extremities during LJs.

2. Methods

2.1. Participants

Thirty male collegiate basketball players (age, 21.2 ± 1.3 years; height, 183.3 ± 5.0 cm; body mass, 74.0 ± 6.7 kg; shoe size, 9.67 ± 0.52 US) with 4.8 ± 1.4 years of experience in basketball were recruited for this study. The inclusion criteria were as follows: (1) participants have at least two years of experience in basketball; (2) absence of strenuous exercise within 24 h; (3) free of musculoskeletal injuries in the lower extremities for at least six months before the testing session; and (4) normal physical function and athletic performance [8]. All subjects gave their informed consent for inclusion before they participated in the study. The study was conducted in accordance with the Declaration of Helsinki. The protocol was approved by the Ethics Committee of Shanghai University of Sport.

2.2. Instrumentation

2.2.1. 3D Motion Capture System

The sagittal plane kinematics of the dominant lower extremity (defined as the preferred kicking leg) [16] was acquired using a 16-camera, infrared, 3D motion capture system (Vicon T40, Oxford Metrics, Oxford, UK) at a sampling rate of 240 Hz. The collected kinematic variables included: (1) hip, knee, ankle, and MTP joint flexion/plantarflexion angle and angular velocity during LJ; (2) minimum and maximum flexion angle and angular velocity during LJ; (3) joints flexion ROM.

2.2.2. 3D Force Plates

Two 90 × 60 × 10 cm 3D force plates (Kistler 9287B, Kistler Corporation, Switzerland) were used to collect the ground reaction forces. The sampling rate was 1200 Hz. The force plates and Vicon system were systematically synchronized using a terminal box of an A/D converter. The collected kinetic variables of interest included: (1) hip, knee, ankle, and MTP joint minimum and maximum torque during LJ; (2) minimum and maximum power during LJ; (3) Energy Absorption (EA), EG and net energy during LJ.

2.3. Experimental Protocol

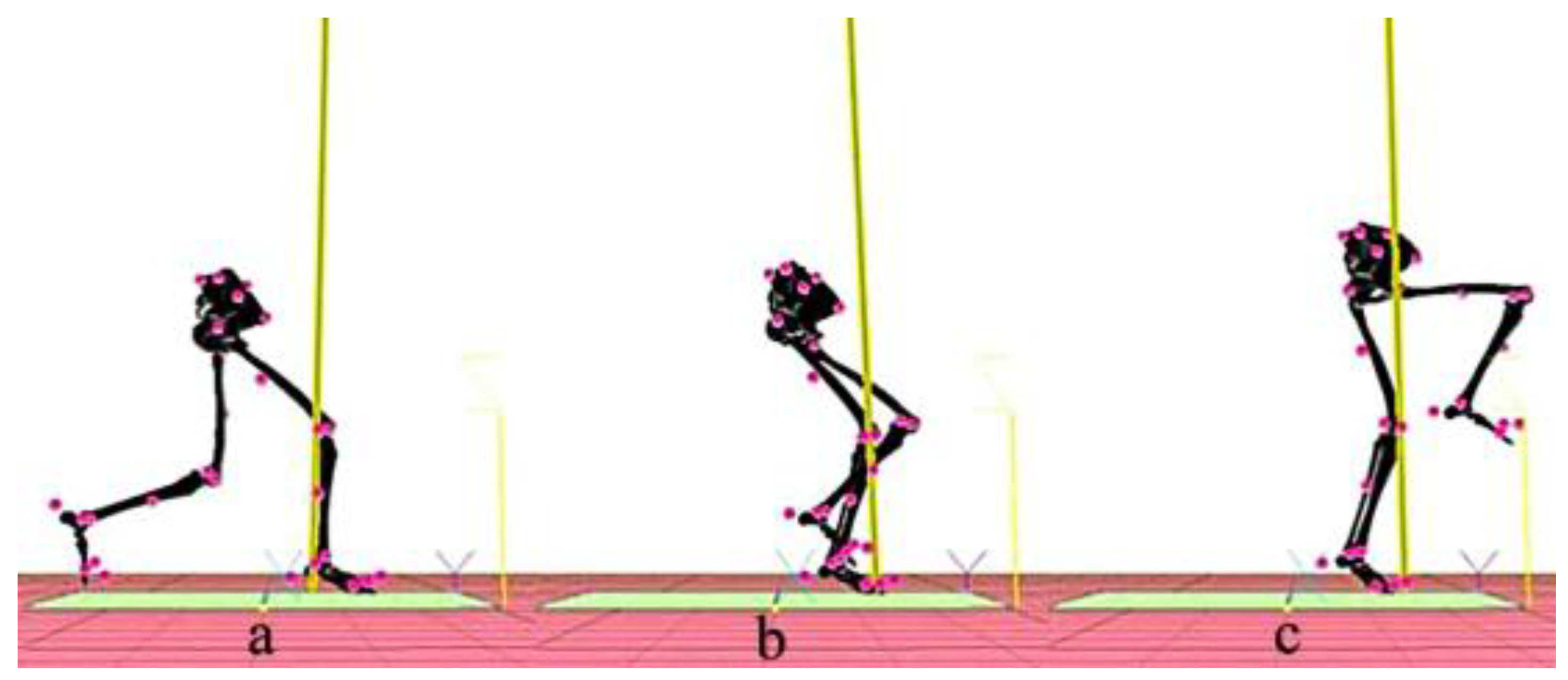
2.3.1. Testing Procedure

Upon arriving at the lab, the project was explained to each participant. Signed informed consent forms were obtained after all questions were answered satisfactorily. Prior to the formal test, the participants warmed up by running on a treadmill for 5–8 min at self-selected pace and familiarized themselves with the jumping tasks. After a short break, the participants performed LJs wearing stiff midsoles and control shoes in a randomized order. For this task, each participant was asked to take one step forward with the second contralateral step placing the foot on one force plate, and subsequently jump up with maximum effort (Figure 1). Three successful trials were required for each shoe condition. Successful trials consisted of satisfactory jump form and completion of the required data collection (Figure 2).

2.3.2. Testing Shoes

Two types of basketball shoes differing only in midsole stiffness were tested in this study; both were of the same make and model, as provided by a commercial shoe company. The shoes had identical designs in terms of their outsole, material, weight, and shape, except for the difference in stiffness in the midsole. Specifically, a carbon-reinforced plate was inserted into the SS.

2.3.3. Marker Setup

Twenty-four retroreflective markers (diameter: 14.0 mm) were used to define the forefoot, rearfoot, leg, and thigh segments [17]. The first and fifth metatarsal laterals and the distal end of the hallux were used to define the forefoot. The medial/lateral malleolus markers, the first/fifth metatarsal lateral markers, and the posterior heel markers were used to define the rearfoot. Figure 3 presents the definition of the sagittal plane angle of the hip, knee, and ankle joints. The MTP joint angle was defined as the angle between the forefoot and rearfoot anatomical coordinate systems [18]. The rotation axis of the MTP joint was defined as the midpoint between the first and fifth metatarsal heads and the conjunction between the markers of the first and fifth MTP joints.

2.4. Data Analysis

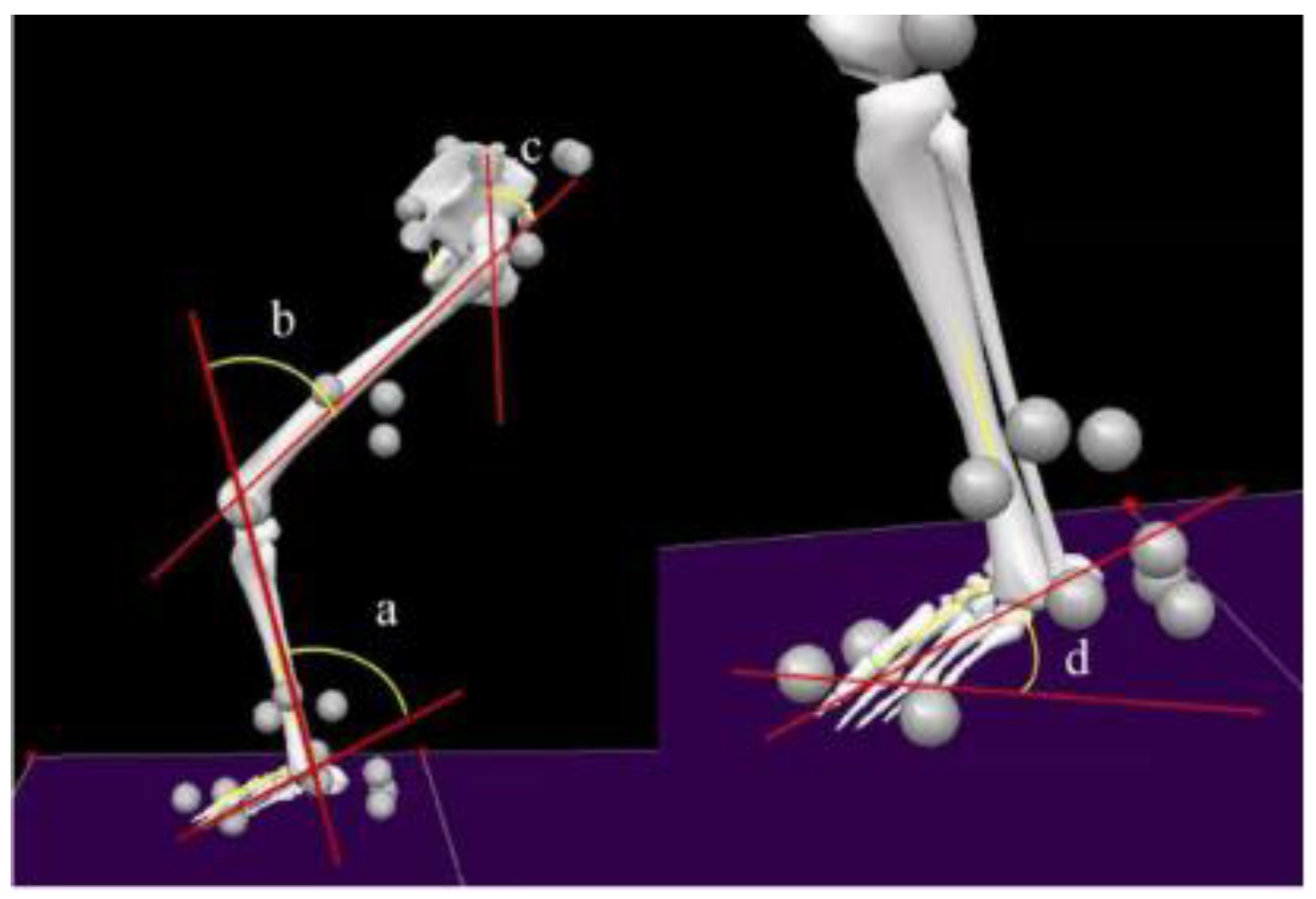
2.4.1. Sagittal Plane Kinematics

The trajectory of reflective markers was filtered using a fourth-order Butterworth low-pass filter at a cut-off frequency of 7 Hz [19]. A visual 3D software (4.00.20, C-Motion Inc., MD, USA) was used to calculate the ankle variables in the sagittal plane [20]. The ankle kinematics included the touchdown ($\theta$), maximum and minimum angles (${\theta }_{\max }$ and ${\theta }_{\min }$), maximum and minimum angular velocities (${\omega }_{\max }$ and ${\omega }_{\min }$), and ROM (${\theta }_{ROM}={\theta }_{\max }-{\theta }_{\min }$). The jump height was calculated using $V_0^2$/2 g (where $V_0$ is the vertical take-off velocity), and was used to determine the jumping performance [21].

2.4.2. Joint Kinetics

Joint torque, including the maximum and minimum torque values of the hip, knee, ankle, and MTP joints ($M_{\max }$ and $M_{\min }$), was determined via inverse dynamics analysis. Joint power, including the maximum and minimum power values ($P_{\max }$ and $P_{\min }$) of four joints, was calculated as the product of instantaneous internal joint torque and instantaneous angular velocity:

$$P_j\left(t\right)=M_j·{\omega }_j\left(t\right)$$

where $M_j$ refers to the joint moment and ${\omega }_j$ denotes the joint angular velocity.

2.4.3. Joint Energy

Joint energy refers to the joint work amplitude within a duration (time-integrated power) [22], and is calculated as follows:

$$EA\left(EG\right)={{\displaystyle \int }}_{t2}^{t1}P\left(t\right)·dt,$$

$$NEC=EG-EA$$

where the negative power occurs during an eccentric contraction (touchdown ground to the knee flexion) and refers to EA, whereas the positive power occurs during a concentric contraction (touchdown ground to take-off) and refers to EG. Net energy consumption (NEC) signifies the net energy consumption during the touchdown phase.

2.5. Statistics

All data were normally distributed on the basis of the Shapiro–Wilk test. The paired student’s t-test was used to examine the differential effects of shoes on jump heights, kinematics, kinetics, and joint energy (SPSS 17.0, SPSS Inc., Chicago, IL, USA). The significance level was set to α = 0.05.

3. Results

3.1. Performance

No significant differences were observed between the SS and control shoes (CS) conditions in terms of jump height (

Table 1. Mean (SD) jump height during LJs (n = 30).

Shoe Condition	Jump Height (cm)	Effect Size	P
SS	66.3 (6.9)	0.043	0.234
CS	66.0 (7.0)

Note: SS, stiff midsole shoes; CS, control shoes; LJ, layup jump; * Significant difference between shoes (p < 0.05).

).

3.2. Kinematics and Kinetics of the MTP Joints

Minimum angular velocities were significantly lower in the SS condition. No significant differences existed in the maximum and minimum angles, ${\theta }_{ROM}$, maximum angular velocities, and kinetic parameters (maximum torque, minimum power, maximum power, EA, EG, and net energy) between the two shoe conditions (

Table 2. MTP joint kinematics and kinetics during LJs (n = 30, Mean (SD)).

Variables	SS	CS	Effect Size	P
Max. angle (°)	31.2 (9.8)	29.7 (5.2)	0.191	0.266
Min. angle (°)	23.3 (8.6)	22.2 (4.9)	0.157	0.82
θROM (°)	7.9 (2.2)	7.5 (2.4)	0.174	0.064
Max. angular velocity (°/s)	78.3 (22.4)	87.1 (25.7)	0.365	0.636
Min. angular velocity (°/s) *	−56.4 (31.2)	−69.1 (25.6)	0.445	0.032
Max. torque (N·M)	75.4 (23.8)	73.0 (21.4)	0.106	0.691
Min. power (W)	−117.8 (57.6)	−86.0 (77.9)	0.464	0.764
Max. power (W)	69.0 (29.0)	67.2 (65.3)	0.035	0.126
EA (J)	−6.7 (4.6)	−4.7 (4.8)	0.425	0.724
EG (J)	1.9 (0.9)	2.0 (2.9)	0.047	0.055
Net energy (J)	−4.8 (3.9)	−2.7 (4.2)	0.518	0.113

Note: MTP, metatarsophalangeal; SS, stiff midsole shoes; CS, control shoes; LJ, layup jump; Max., maximum; Min., minimum; θROM, range of motion; EA, energy absorption; EG, energy generation; * Significant difference between shoes (p < 0.05).

).

3.3. Kinematics and Kinetics of the Hip, Knee and Ankle Joints

Maximum power, absorbed energy, and generated energy of the ankle joint were significantly greater in the SS condition than in the CS condition (

Table 3. Hip, knee, and ankle joint kinematics during LJs (n = 30, Mean (SD)).

Joint	Variables	SS	CS	Effect Size	P
Hip	Max. angle (°)	71.3 (10.2)	71.7 (10.0)	0.039	0.885
	Min. angle (°)	11.9 (7.2)	14.2 (8.1)	0.300	0.262
	θROM (°)	59.4 (8.6)	57.5 (8.6)	0.221	0.406
	Max. angular velocity (°/s)	87.9 (69.8)	87.4 (55.9)	0.008	0.971
Knee	Max. angle (°)	55.0 (6.7)	53.6 (5.7)	0.225	0.394
	Min. angle (°)	10.5 (5.6)	11.0 (5.1)	0.093	0.698
	θROM (°)	44.5 (6.2)	42.65 (5.4)	0.318	0.204
	Max. angular velocity (°/s)	65.6 (50.9)	68.0 (65.4)	0.041	0.823
Ankle	Max. angle (°)	10.8 (6.5)	10.0 (7.9)	0.110	0.226
	Min. angle (°)	−19.7 (8.8)	−19.7 (8.8)	0.000	0.804
	θROM (°) *	30.8 (6.2)	29.5 (5.9)	0.215	0.013
	Max. angular velocity (°/s)	245.0 (108.2)	245.0 (94.3)	0.000	1.000

Note: SS, stiff midsole shoes; CS, control shoes; LJ, layup jump; Max., maximum; Min., minimum; θROM, range of motion. * Significant difference between shoes (p < 0.05).

and

Table 4. Hip, knee and, ankle joint kinetics during LJs (n = 30, Mean (SD)).

Joint	Variables	SS	CS	Effect Size	P
Hip	Max. torque (N·m)	98.4 (32.8)	94.5 (31.7)	0.121	0.729
	Min. torque (N·m)	−612.7 (162.1)	−613.1(151.4)	0.003	0.994
	Min. power (W)
	Max. power (W)	1379.7 (776.3)	1551.4 (765.4)	0.223	0.407
	EA (J)	21.8 (15.2)	18.5 (12.4)	0.238	0.495
	EG (J)	93.6 (25.6)	100.9 (27.5)	0.275	0.484
	Net energy (J)	71.8 (33.9)	82.4 (35.2)	0.307	0.447
Knee	Max. torque (N·m)	273.1 (44.7)	266.3 (38.1)	0.164	0.515
	Min. torque (N·m)	−150.1 (56.5)	−156.0 (61.7)	0.100	0.756
	Min. power (W)	−552.5 (209.5)	−564.1 (169.2)	0.061	0.861
	Max. power (W)	1261.4 (305.1)	1211.1 (265.9)	0.176	0.492
	EA (J)	38.5 (13.5)	35.5 (13.6)	0.221	0.445
	EG (J)	78.9 (27.1)	71.4 (28.1)	0.272	0.260
	Net energy (J)	40.4 (17.6)	35.9 (25.3)	0.206	0.458
Ankle	Max. torque (N·m)	19.7 (12.1)	19.7 (9.8)	0.000	0.977
	Min. torque (N·m)	−301.3 (51.9)	−291.7 (33.5)	0.220	0.365
	Min. power (W)	−557.6 (212.9)	−474.9 (180.5)	0.419	0.122
	Max. power (W) *	1359.6 (249.7)	1191.7 (283.9)	0.628	0.033
	EA (J) *	39.1 (11.5)	32.4 (10.4)	0.611	0.028
	EG (J) *	84.5 (18.6)	70.9 (19.5)	0.714	0.012
	Net energy (J)	45.4 (19.3)	38.5 (17.8)	0.372	0.211

Note: SS, stiff midsole shoes; CS, control shoes; LJ, layup jump; Max., maximum; Min., minimum.; EA, energy absorbed; EG, energy generated. * Significant difference between shoes (p < 0.05).

). No significant differences were observed in the kinetics variables of the hip and knee. The ankle joint ${\theta }_{ROM}$ was significantly greater in SS than in CS. However, no significant differences existed in the kinematic variables of the hip and knee joints (Table 3).

4. Discussion

To explore the effect of the stiff midsole on LJs, our study investigated the influence of a stiff midsole on the biomechanics of the lower extremities while performing an LJ with SS and CS. The results partially supported our original hypothesis that SS increased the ankle maximum power, EA, and EG during an LJ. No significant changes were observed in the kinematics of the MTP sagittal plane, kinetics, and height performance.

4.1. Performance

A contradictory result was observed in our study. In our results, the jump height of LJs did not improve with SS, which was contrary to our hypothesis that a stiff midsole in MTP would enhance performance. This observation is inconsistent with the performance improvement mechanism of decrease absorbed energy by SS. Stefanyshyn et al. [23] claimed that MTP absorbs approximately 24 J during the push-off phase, which could theoretically improve the jump height of a mass body (70 kg) by approximately 3.5 cm. This phenomenon may be caused by the failure of SS to modulate the net energy of the lower extremities during LJs. In our study, only the absorbed energy, generated energy, and ROM of the ankle joint significantly changed, but the net energy remained the same (Table 4); this may be explained that the SS increased the ROM of the ankle joint. The increased ROM simultaneously improved the absorbed energy and the generated energy in the ankle, which resulted in no significant change in net energy in the ankle. Similarly, Roy et al. [18] investigated the effect of stiff midsoles on the mechanical energy of the lower extremities; their results indicated no significant difference. Oh et al. [12] suggested that SS did not change the take-off velocity of the center of mass while running. Therefore, SS did not alter the net energy of the lower extremities and did not increase the jump height of LJs.

4.2. Biomechanics of the MTP Joints

To improve athletes’ performance, special footwear with differing midsole stiffness levels was designed to reduce the EA in the MTP joint. However, in our study, except for the fact that the minimum angular velocity of the MTP joint was significantly lower in SS, no significant difference was observed in the other MTP joint sagittal kinematics or kinetics (Table 2). These results were inconsistent with those of Stefanyshyn [22], whereby increasing MTP joint stiffness decreased MTP joint ROM, dissipating energy by 36.7%, and improving performance. Several factors might have caused this discrepancy. Firstly, different motion types were used, and the abovementioned studies selected running to determine the effect of stiff midsoles on the lower extremities, whereas our study tested LJs. The different movement types led to a large discrepancy in the MTP joint angular velocity during the push-off phase. The current MTP joint angular velocity was 87.1°/s, which was significantly lower than that of the running study at 730.7°/s [11]. The small MTP joint angular velocity might have led to a minimal change in LJs. Moreover, the MTP joint rotation center had differing definitions. In this study, a single axis was used to define the MTP joint rotation center, which was consistent with the link line between the first and fifth reflective markers. Contrary to this methodology, Smith [24] developed an elegant methodology that may be further representative in defining the MTP joint using two axes, which were constructed by connecting the first to the second MTP joint and connecting the second to the fifth MTP joint. In addition, the inconsistent observations across different studies [7,10,11,22,25] concerning the effect of stiff midsoles on the MTP joint might be caused by various factors, such as shoe materials and structures. Wunsch et al. [25] compared the effect of a leaf spring structured midsole shoe (LEAF) and a stand-foam midsole shoe (FOAM) on the joint mechanics of the lower extremities; they indicated that LEAF is more effective in improving running performance. Therefore, additional studies are required to determine the effect of stiff midsoles on the biomechanics of the MTP joint, and an increasingly optimized strategy for modulating midsole stiffness should be explored.

4.3. Biomechanics of the Ankle Joint

The hypothesis regarding the biomechanical characteristics of the ankle joint is partially accepted. Our results showed that stiff midsoles significantly improve the ankle ROM (p < 0.05), maximum power, absorbed energy, and generated energy (p < 0.05). The results were similar to those of Oh et al. [12], who reported that SS modulated the biomechanical characteristics of the ankle joint. This phenomenon might have been caused by the complementary changes in the kinetic chain of the lower extremity joint. The SS shifted the point of force application towards the front edge of the shoe–ground interface, which increased the lever arm and angular impulse of the ankle [26,27]. This case may contribute to the improvement in maximum power and joint ROM of the ankle joint, as presented in our study (Table 3 and Table 4). Then, the increased ROM in the ankle joint caused a significant improvement in the absorbed energy and generated energy, while the net energy of the ankle joint remained unchanged (Table 4). The complementary mechanism of the ankle joint was also included in Willwacher [28]. Three types of shoes with different stiffness levels were compared, and the observations demonstrated that high-stiffness shoes caused the point of force application to lean forward, increased the lever arm, and improved the contact time in the first step of running. Therefore, the stiff midsole modulated the biomechanical pattern of the ankle joint through the complementary changes of the lower extremities without changing the net energy of the ankle joint.

4.4. Limitations

Electromyographic data were not collected in this study. This type of data would have been helpful in better understanding the neuromuscular activity of the lower extremities during LJs. Moreover, the shoe-bending effect in the MTP joint should be considered when explaining the MTP joint mechanics. In addition, more jumping tasks should be measured to determine the effect of SS. Finally, the lack of repeated measures could also be a limitation in the present study.

5. Conclusions

During a single LJ, the longitudinal midsole stiffness did not influence the jump height and MTP joint biomechanical patterns, but significantly increased the maximum power, EA, and EG during the push-off phase of the ankle joint. These preliminary results indicated that wearing SS could change the lower limb kinetic chain, improve the work of dorsiflexion and plantarflexion muscles, and increase the strength in the ankle. Our analyses imply that using the SS has the potential to enhance muscle strength, especially in the explosive strength of the ankle joint.