Design of the Input and Output Filter for a Matrix Converter Using Evolutionary Techniques

Abstract

This work presents a new methodology for designing input and output filters to be applied in a three-phase matrix converter, with an optimization problem solved by a metaheuristic algorithm. The algorithm determines the optimal values of the passive elements of the filters, taking as the fundamental performance criterion the response in magnitude. It is based on the resonant frequency, in order to eliminate the harmonics in the input currents and the output voltages produced by the bidirectional switching and disturbances in the power supply. These filters are implemented in a direct matrix converter using a Venturini-type modulation in open loop configuration. Other developments for this filter design have been carried out, but most of them were based on the conventional procedure of determining a random value for some element and then finding the magnitude of the remaining elements. That approach does not ensure the best dynamic filter response. The proposed design technique requires no initial elements, since all values are calculated by the metaheuristic algorithm.

1. Introduction

An AC-AC Matrix Converter (MC) is an array of bidirectional switches that fit a three-phase signal by controlling its characteristics, as required for the process in the following stage. They are of great interest in industrial applications due mainly to their reduced dimension compared to other types of converters and because they have the ability to control the amplitude, frequency, and phase of the output voltage signal independently, converting both the input and output currents into sinusoidal signals. By their nature, they have the additional advantage of operating in all four quadrants, allowing the regeneration of energy since their switching elements are bidirectional. However, the design of this kind of converter is a difficult issue, due mainly to the technical limitations found in their construction, the number of semiconductor devices required, and the complexity in the control stage. Different MC topologies have been developed despite their complexity, to provide better performance and efficient energy consumption [1].

The study of the three-phase MC was presented for the first time by Alesina and Venturini [2] in 1980. They developed the basic configuration of a power circuit as a matrix of bidirectional switches that connects each of the output phases to the input phases, introducing the term of the matrix converter. Their work included a rigorous mathematical model, in which they demonstrated that it was possible to obtain an ideal electronic converter, transforming an alternating polyphase electric input signal with fixed characteristics (magnitude, frequency, and phase) into an alternating polyphase signal of variable parameters, independently controlling each of them [3]. They proposed a control strategy known as Venturini modulation [2], based on a matrix of low-frequency modulation for the voltage and current, generating a maximum output of $50\%$ with respect to the input $(q=0.5)$, without the ability to control the power factor. Alesina and Venturini [4,5] also presented a method to increase the maximum output voltage of the converter at a ratio of 0.866 with respect to the input. This was achieved by including the third harmonic in the modulation equation for both the voltage and current signals, with a technique known as the Venturini optimo method.

A transfer ratio between the input and output voltages lower than one is the main disadvantage of the matrix converters [1,6]. In 1983, Rodriguez [7] proposed a new control technique based on a virtual direct current bus approach. In that modulation, the voltage inputs were first rectified in virtual form and then transformed to an alternating current, generating a variable-frequency output signal. In this method, each output line switches between positive and negative values of the input lines using pulse width modulation (PWM) techniques, similar to those used in back-to-back inverters. This control strategy was developed by Ziogas with a method known as indirect transfer [8,9], achieving a maximum input/output ratio of 1053 in an MC. However, the maximum voltage obtained presented low-frequency distortions.

In 1987, Roy and April [10] proposed a new control strategy called the scalar method, where the signals for the switch activation were calculated by measuring the input voltage lines. Similar to the Venturini method, the transfer ratio was limited to a value of 0.5 with a maximum of 0.866, due to low-frequency disturbances in the power supply and to variable loads [11,12]. Another limitation was that the method could not control the power factor. A similar approach to the scalar method was proposed by Ishiguro [13], generating the switching signals from the measurement of the line-to-line input voltage, different from the scalar method, where the signals were measured with reference to neutral. The maximum voltage ratio was $0.75$.

Spatial vector modulation (SVM) was first proposed by Huber [14,15] to be applied to matrix converters. This is based on the instantaneous representation of spatial vectors for voltages and currents, for both the input and output. One of the most outstanding works was presented by Casandei [16], called the spatial vector approach of service cycle, applied to matrix converters. It uses the spatial vector notation and introduces the concept of the spatial vector of service cycle. These levels are obtained by a high switching speed, but implying a greater loss of energy. There are additional works in which other types of modulation and control schemes for matrix converters were planned, such as control by sliding modes [17] and PWM control based on carrier signals [18].

In the design of matrix converters, the use of electronic power devices such as bidirectional switches requires input filters. This reduces the high harmonic content in the input current, increasing the system protection against transitory effects coming from the power supply. Furthermore, the ripple in the waveform and excessive currents or voltages in the converter are reduced. The peaks generated in the waveform of the input voltages can cause an erroneous measurement of the instantaneous values and can affect the calculations made by any implemented modulation strategy. A low-pass filter can be employed to avoid these unwanted harmonic signals in the input. In [19,20], different filter arrays suitable to be integrated into the MC topology were proposed, since the only inertial elements in these topologies were the filter components. However, the filter parameters significantly affected the system dynamics.

The input filter design was discussed in [21,22,23], where due to the cost and weight considerations, it was found that the $LC$ single-stage filter was the most suitable topology, although the set of filter elements $(L_s,C_s)$ for a particular resonance frequency was infinite. In [22], a very large $C_f$ was proposed to ensure a minimum input displacement factor for low loads. A comprehensive treatment of the input/output filters focused on reducing electromagnetic interference and common mode voltages was done in [24,25].

The works so far in the design and analysis of the filters were developed independently and were focused on satisfying the stability, power, or cost requirements. They were based on the arbitrary choice of some of the elements, and the values of the remaining parts were determined by means of the characteristic equations of the filter, without establishing if these elements generated the best dynamic response. Most of these works did not consider the integral design of the filters in the context of dynamic performance improvement or the reliability of the switching hardware. The only optimal approach so far was [26], which was proposed for the input filter and considered the objectives of both stable and dynamic states. It used a genetic algorithm to derive the values of the parameters and a test with three different topologies.

A metaheuristic is a non-deterministic approximated method for solving complex optimization problems. Diverse metaheuristics have been developed [27]. The Differential Evolution (DE) algorithm was a metaheuristic presented by Storn and Price [28] that has been applied to the solution of complex engineering problems. It is one of the most popular metaheuristics [29,30] because of its fast convergence and easy implementation.

In this paper, an alternative design technique for the input and output filters of an MC is presented. This approach is based on the modeling of an optimization problem, which to the best of the authors’ knowledge has not been proposed in any previous work. This technique proposes the solution in the design of the filters directly, without the use of analytical substitution techniques, which allows guaranteeing the optimal solution to the optimization problem and obtaining an efficient response in the filters. This problem is solved by applying differential evolution. The proposed design technique requires no initial elements, since all values are calculated by the metaheuristic algorithm. This proposal carries out the design of both the output and input filters, taking as the main criterion a magnitude reduction in the Bode analysis, applied in three frequency values with respect to the resonant frequency. This implies stability in the complete system, guaranteeing that its gain does not increase in frequencies around the resonant frequency. The optimization problem is based on the equations that characterize the behavior of both filters and considers constraints corresponding to necessary design conditions such as the nominal power of the converter, resonant frequency, switching frequency, and damping factor. The main advantage when using the methodology proposed in this novel work is that the solution to the design of the filters is based on the frequency response of the system considered in the optimization problem posed and on a series of design restrictions. As a case study, the proposed methodology is applied to a direct MC [2], and the obtained results surpass the previously reported values [21,22,23].

The document is organized as follows: Section 2 includes a general description of the matrix converter, while in Section 3, the input filter is presented, including its topology, modeling, related constraints, and optimization. A similar analysis is carried out for the output filter in Section 4. Section 5 includes an explanation of the differential evolution algorithm and its application for solving the MC optimization problems. The results of the case study are presented in Section 6, with graphs for the output voltages, the input currents, and the Bode diagrams. Finally, Section 7 corresponds to the conclusions and future works.

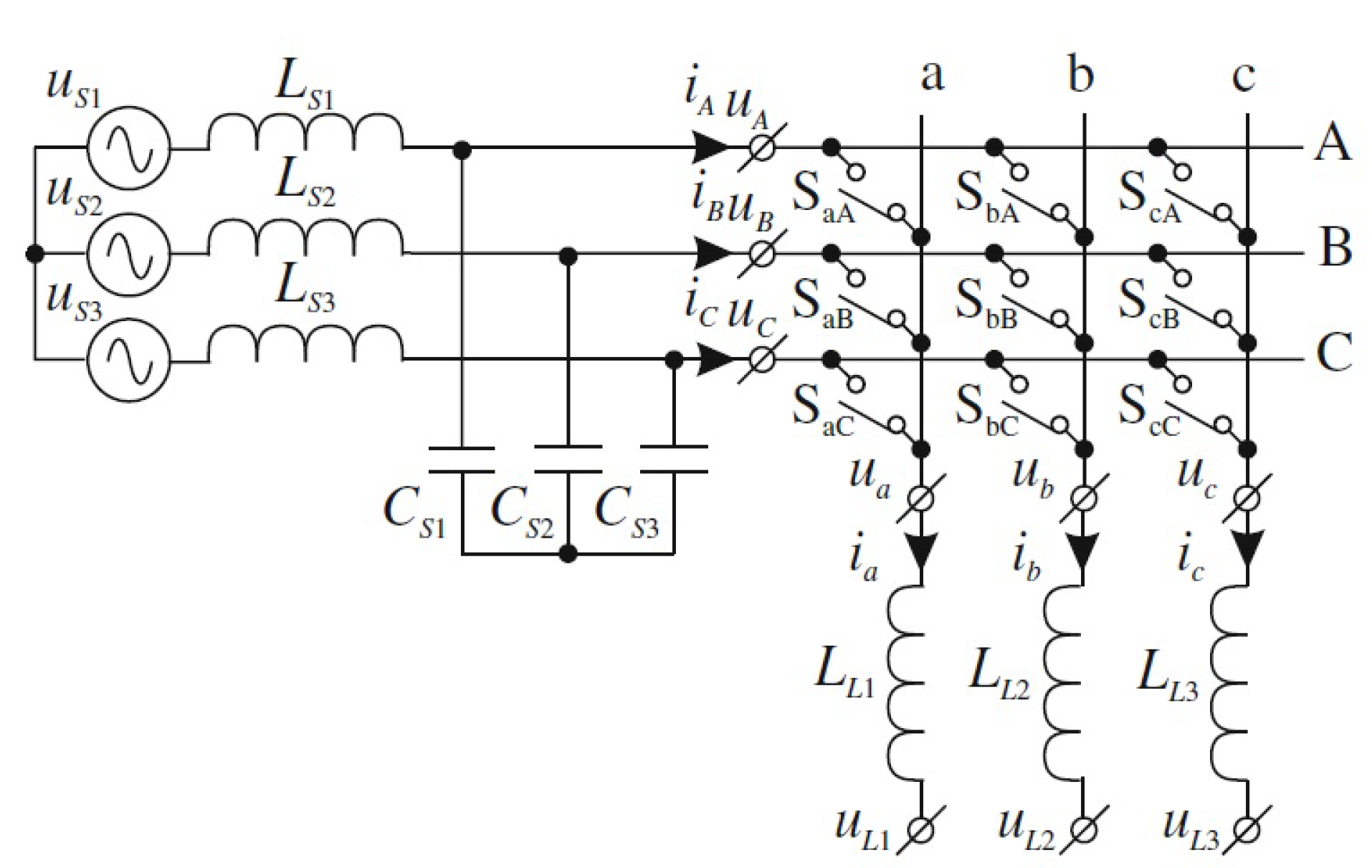
2. Matrix Converter

The matrix converter consists of an array of electronic bidirectional power switches and passive elements that act as filters for the input and output signals, as seen in Figure 1. The power switches carry out the control by means of different modulation techniques. A matrix converter of dimension $(n\times m)$ consists of an array of $(n·m)$ switches. This device is connected directly to the power lines, as input, and the load is connected directly to the converter output. In the case of a three-phase converter (Figure 1), there are three input signals and three output signals, so the matrix dimension is $(3\times 3)$ with nine switches [6].

The advantages of an MC with respect to a conventional AC-AC are:

• The waveform of the input current is sinusoidal, and this allows minimizing the harmonic and subharmonic content injected by the converter into the AC power supply.
• The energy flow can be bidirectional.
• The minimum requirement of passive components, which implies a reduction of their resignations by up to 50% compared to other converter topologies.
• Power-factor control.

Disadvantages:

• Complex implementation.
• It requires bidirectional switches, so the number of electronic devices in the switching matrix increases, depending on the number of stands and outputs.
• It presents a problem of both synchronization and protection.
• In open loop operation, there is distortion of the input and output waveforms due to the high sensitivity of the converter.
• The maximum voltage ratio is $0.866$.

The behavior of the modulation function for an output phase is defined by the activation and deactivation of the switches that are connected to each input phase, as indicated in Expression (1):

$$\mathrm{switch}{s}_{jK}=\left\{\begin{array}{cc}1,\hfill & \mathrm{close}\hfill \\ 0,\hfill & \mathrm{open}\hfill \end{array}\right.$$

(1)

where $K\in \{a,b,c\}$ are the output phases and $j\in \{A,B,C\}$ are the input phases. Two switches should not be activated at the same time to avoid short circuits in the input phases. In the same way, it should be avoided that at any moment of operation, all the switches are deactivated, since it would cause a high voltage differential at the output. This operation constraint is expressed in Equation (2):

$$s_{ja}+s_{jb}+s_{jc}=1$$

(2)

The voltages and the input and output currents referred to the neutral line can be expressed with their vector representation, as seen in (3) and (4):

$$\left[\begin{array}{c}{u}_a\left(t\right)\\ u_b\left(t\right)\\ u_c\left(t\right)\end{array}\right]=\left[\begin{array}{ccc}{s}_{aA}\left(t\right)& s_{aB}\left(t\right)& s_{aC}\left(t\right)\\ s_{bA}\left(t\right)& s_{bB}\left(t\right)& s_{bC}\left(t\right)\\ s_{cA}\left(t\right)& s_{cB}\left(t\right)& s_{cC}\left(t\right)\end{array}\right]\left[\begin{array}{c}{u}_A\left(t\right)\\ u_B\left(t\right)\\ u_C\left(t\right)\end{array}\right]$$

(3)

$$\left[\begin{array}{c}{i}_A\left(t\right)\\ i_B\left(t\right)\\ i_C\left(t\right)\end{array}\right]=\left[\begin{array}{ccc}{s}_{aA}\left(t\right)& s_{aB}\left(t\right)& s_{aC}\left(t\right)\\ s_{bA}\left(t\right)& s_{bB}\left(t\right)& s_{bC}\left(t\right)\\ s_{cA}\left(t\right)& s_{cB}\left(t\right)& s_{cC}\left(t\right)\end{array}\right]\left[\begin{array}{c}{i}_a\left(t\right)\\ i_b\left(t\right)\\ i_c\left(t\right)\end{array}\right]$$

(4)

The power filters $(L_{Li},L_{si},C_{si})$ located at the input and output of each of the lines of the converter mitigate the high-frequency components, generating current signals from input and sinusoidal output voltages, avoiding the generation of over-voltages. The over-voltage is caused by the fast switching in the input currents due to the presence of the short-circuit reactance of any real power supply. An adequate design of these filters is very important in the operation of matrix converters [23,31].

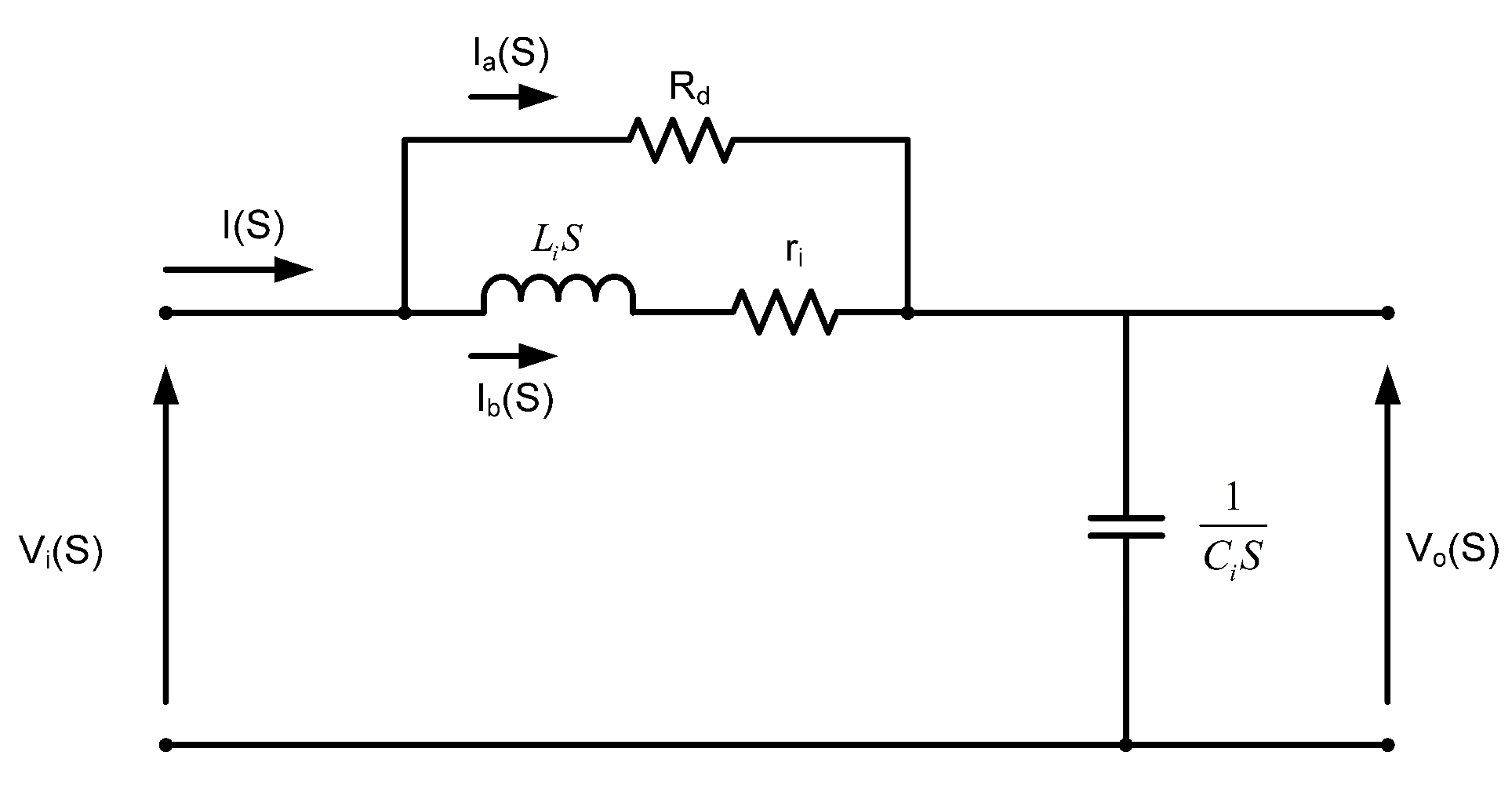
3. Input Filter

As mentioned previously, the bidirectional switches in the design of an MC require the use of input filters. Disturbances in the input voltages can cause an erroneous measurement of the instantaneous values and affect the calculations made by the modulation strategy. To avoid these unwanted harmonic signals in the input, a low pass filter $LC$ is implemented. There are different $LC$ filter arrays that can be integrated into the MC topology [19,20], as shown in Figure 2:

For this work, a single-state $LC$ filter was selected because it uses few components and has a proper response in the frequency domain [19]. The presence of reactive elements improves the behavior of the MC in the following circumstances:

• $C_F$: Limits the over-voltages of the input originated by the distortions or disturbances of the power supply network. The parasitic inductances through the switches of the MC can generate over-voltages on both sides of the converter due to the high values of $\frac{di}{dt}$ in the switching periods. To reduce this effect, capacitor $C_F$ should be placed as close as possible to the MC switches.
• $L_F$: The switching process in the MC causes the appearance of over-currents, which are generated from small discontinuities that occur when switching from a switch on an input phase to another switch on a different input phase. The inductor $L_F$ is responsible for smoothing the slope of that over-current.

Figure 3 shows the $LC$ input filter with a damping resistance in parallel with the filter inductor. The internal resistance of the inductor is represented by $r_i$.

The transfer function of the input filter is expressed in Equation (5):

$$\frac{V_o\left(s\right)}{V_i\left(s\right)}=\frac{\left[\frac{1}{R_d{C}_i}\right]s+\left[\frac{1}{L_i{C}_i}+\frac{r_i}{R_d{L}_i{C}_i}\right]}{s^2+\left[\frac{r_i}{L_i}+\frac{1}{R_d{C}_i}\right]s+\left[\frac{1}{L_i{C}_i}+\frac{r_i}{R_d{L}_i{C}_i}\right]}$$

(5)

The input frequency is the frequency of the electric network, 60 Hz. The input filter must be able to attenuate the multiples of higher order harmonics of the power frequency. The values of its components should be as small as possible to minimize the dimensions of the converter. The harmonic reduction effect of the filter depends on its resonance frequency, which is determined by Equation (6):

$$f_{i0}=\frac{1}{2\pi \sqrt{L_i{C}_i}}$$

(6)

The value of the capacitance must be selected according to the nominal power value of the MC. There is no criterion to establish the appropriate power factor at the input of the inverter in low power conditions, but a power factor higher than $0.8$ when the input power is $10\%$ of its nominal value is acceptable [32]. The capacitive reactive power $Q_C$ is selected according to Equation (7).

$$Q_C=3{\omega }_i{C}_i{V}_i^2⩽\frac{3}{4}10\%P_n$$

(7)

where $C_i$ is the value of the input capacitance, $V_i$ is the input RMS voltage, $P_n$ is the nominal power of the MC at the input expressed in watts, and ${\omega }_i$ the angular frequency in the input. The value for the capacitor should be in the range according to Equation (8):

$$C_i⩽\frac{P_n}{40{\omega }_i{V}_i^2}$$

(8)

The design of the input filter is related to the switching frequency and the voltage drops in the $LC$ filter inductor. The voltage drops must not be higher than $5\%$ of the input voltage. The resonance frequency $\left(f_{i0}\right)$ must be higher than the fundamental frequency of the voltage supply and lower than the switching frequency. Normally, $f_{i0}$ must be higher than 20 for the power frequency $\left(f_i\right)$ and lower than one third of the frequency in the load $\left(f_s\right)$, as in Expression (9).

$$20f_i⩽f_{i0}⩽\frac{1}{3}{f}_s$$

(9)

From the denominator of Equation (5), the natural frequency $\left({\omega }_{in}\right)$ and the buffer $\left({\zeta }_i\right)$ from the input filter can be obtained, expressed in Equations (10) and (11) [32].

$${\omega }_{in}=\frac{1}{\sqrt{L_i{C}_i}}$$

(10)

$${\zeta }_i=\frac{1}{2R_d}\sqrt{\frac{L_i}{C_i}}$$

(11)

The complete notation used for the equations is specified in the Appendix A at the end of this work.

Input Filter Optimization

In this subsection, an optimization problem corresponding to the input filter is proposed, minimizing the response in the filter amplitude in a frequency analysis to obtain the magnitude of the passive elements of the filter. The problem is solved by means of the DE algorithm. A second order filter is proposed, as shown in Figure 3, with the elements $C_i$, $L_i$, and $R_{d_i}$ and considering the buffer ${\zeta }_i$. Therefore, the design variables are as in Expression (12):

$$\mathbf{x}={\{C_i,L_i,{\zeta }_i\}}^T$$

(12)

The design vector can be expressed in terms of the optimization problem, as stated in Equation (13):

$$\mathbf{x}={\{x_1,x_2,x_3\}}^T$$

(13)

Considering that the filter is designed from a fixed resonance frequency, an expression for conditioning the value of $C_i$ and $L_i$ is obtained by means of Equation (6), as observed in Equation (14):

$$L_i\ge \frac{1}{4{\pi }^2}{f}_{i0}^2{C}_i$$

(14)

The goal is to minimize the objective function, which corresponds to the amplitude response of the filter in the frequency behavior of Equation (5), evaluated at three frequencies to obtain the numerical behavior of the function at three points, and the main value is the switching frequency, which in this design is proposed as 1200 Hz and two frequencies plus one with a higher value and the other with a lower value, as expressed in (15):

minimize:

$$\begin{array}{cc}\hfill f(x_1,x_2,x_3)& =-20log|H_1\left(j{\omega }_1\right){|}_{{\omega }_1=900Hz}\hfill \\ \hfill & -20log|H_2\left(j{\omega }_2\right){|}_{{\omega }_2=1200Hz}\hfill \\ \hfill & -20log|H_3\left(j{\omega }_3\right){|}_{{\omega }_3=3000Hz}\hfill \end{array}$$

(15)

subject to Expressions (16) to (19):

$$g_1(x_1,x_2,x_3)=x_2-{\displaystyle \frac{1}{4{\pi }^2{f}_{i0}^2{x}_1}}\ge 0$$

(16)

$$1\times {10}^{-6}\le x_1\le 2.8\times {10}^{-6}$$

(17)

$$1\times {10}^{-4}\le x_2\le 1\times {10}^{-2}$$

(18)

$$0.01\le x_3\le 0.9$$

(19)

The expression $H_i\left(j{\omega }_i\right)$ in Equation (15) is defined by Equations (20)–(22):

$$H_i\left(j{\omega }_i\right)=\sqrt{{\left[G_1\right]}^2+{\left[G_2\right]}^2}i=1,2,3$$

(20)

$$G_{1i}=\frac{B_i(B_i-{\omega }_i^2)+A_i{C}_i{\omega }_i^2}{{(B_i-{\omega }_i)}^2+C_i^2{\omega }_i^2}i=1,2,3$$

(21)

$$G_{2i}=\frac{A_i{\omega }_i(B_i-{\omega }_i^2)-B_i{C}_i{\omega }_i}{{(B_i-{\omega }_i)}^2+C_i^2{\omega }_i^2}i=1,2,3$$

(22)

where:

$$A_i=\frac{1}{R_{d_i}{x}_1}i=1,2,3$$

(23)

$$B_i=\frac{1}{x_2{x}_1}+\frac{r_i}{R_{d_i}{x}_1{x}_2}i=1,2,3$$

(24)

$$C_i=\frac{r_i}{x_2}+\frac{1}{R_{d_i}{x}_1}i=1,2,3$$

(25)

$$R_{d_i}=\frac{1}{2x_3}\sqrt{\frac{x_2}{x_1}}i=1,2,3$$

(26)

The search space for the design variables is limited to a margin in which it is considered feasible to build these elements in a future CM; however, in the use of this methodology for the design of another filter, other search limitations can be established depending on the desired nominal power.

In order to test the proposed methodology, it was applied to the design of a converter with a nominal power of $P_n=746$ W, with a three-phase voltage source $V_i=117$ V and a frequency $f_{i0}=60$ Hz. The switching frequency of the bidirectional power switches was $f_s=1200$ Hz, with a total power factor of the converter $PF=0.8$.

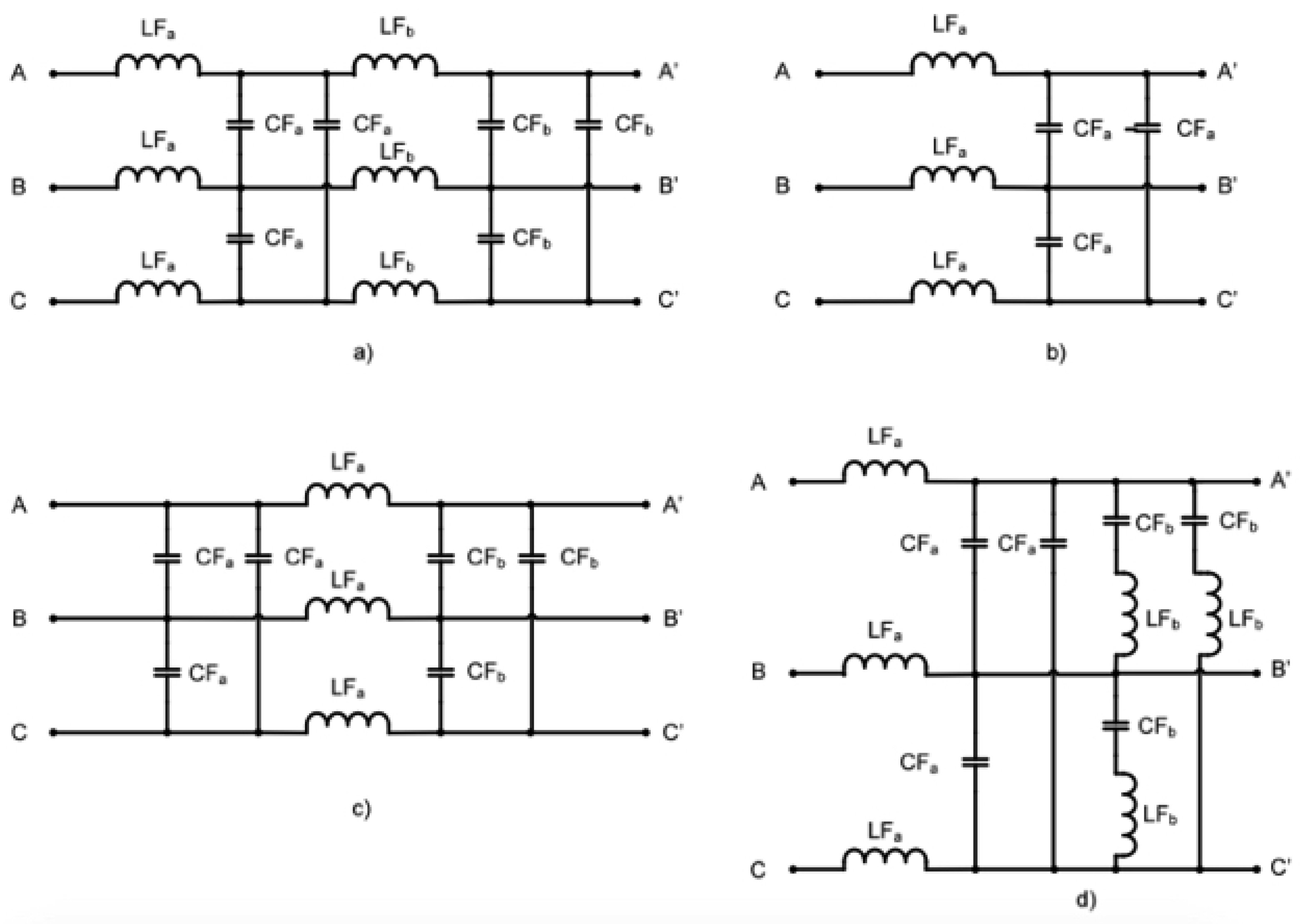
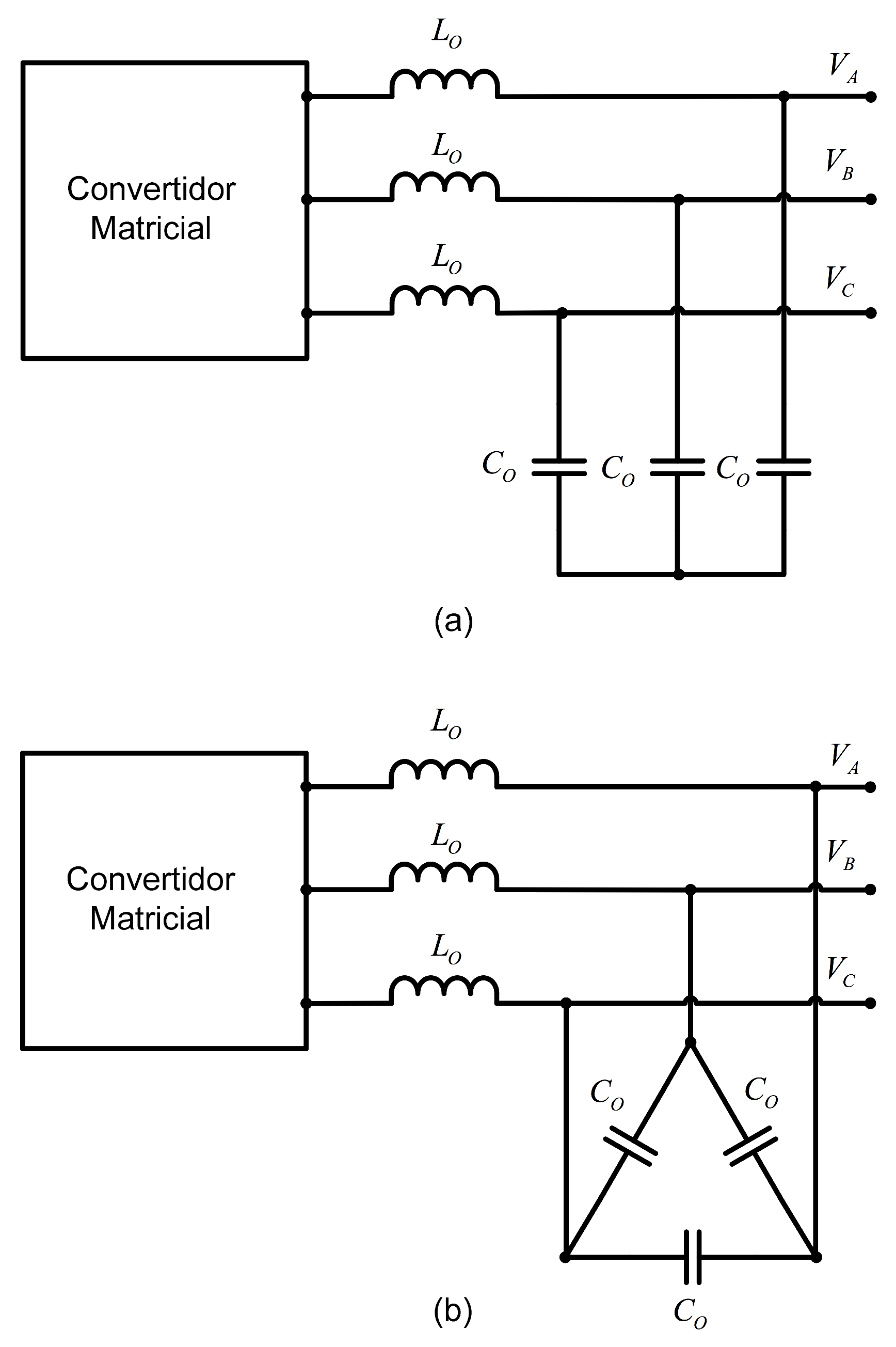
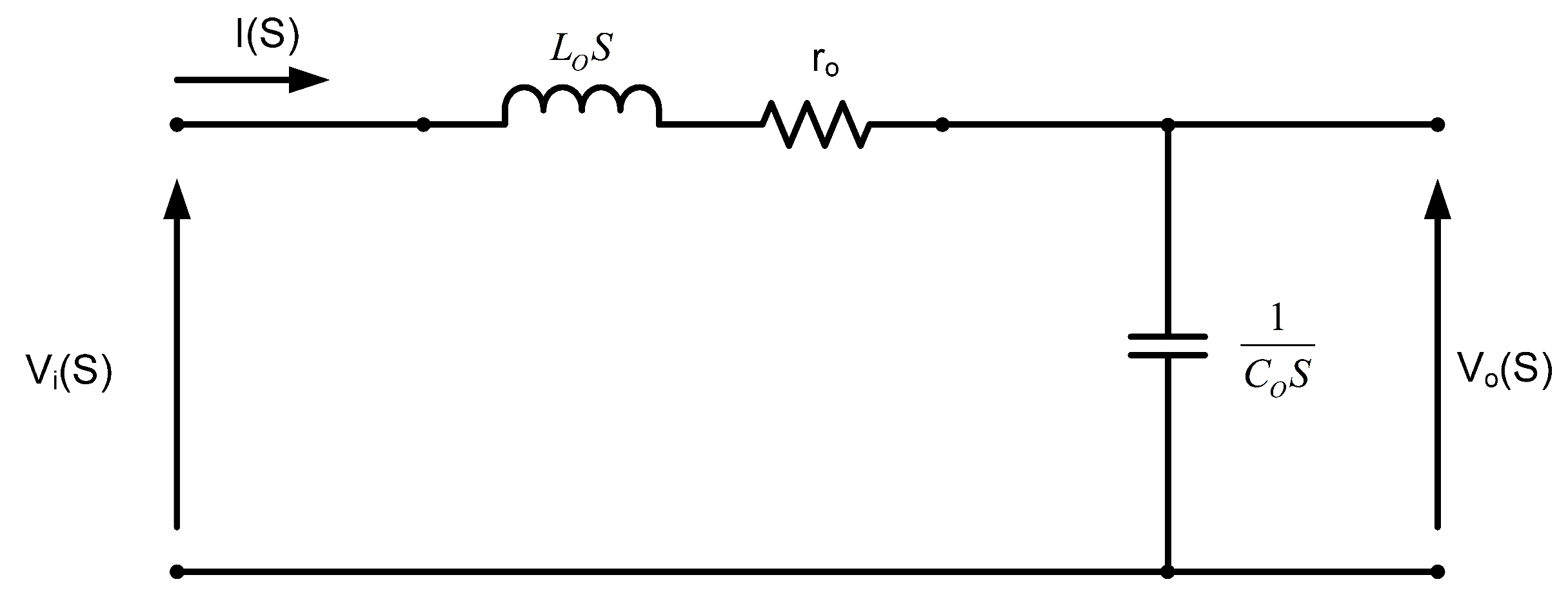
4. Output Filter

Passive $LC$ filters are commonly used in the output terminals of power converters when the output voltages of the system are the main control objective. Am MC requires low pass $LC$ filters in its outputs in order to reduce the harmonics generated in the output voltage waveform, due to the bidirectional switching. A power converter with a high resonance frequency will result in a small filter size [33]. The output impedance of the power converter must be close to zero to operate as an ideal voltage source, since there must be no distortion in the output voltage even under unbalanced conditions in the connected load.

In motor control applications, it is advantageous to use $LC$ filters in the MC output. The filter can compensate the reactive power consumption needed by the motor, which is supplied by the filter capacitor [34]. There are two variants shown in Figure 4. The resonance frequency $f_{out0}$ of the filter for the inverter output attenuates the voltage laps caused by the switching frequency $f_s$. The attenuation effect is increased when the resonance frequency is low compared to the switching frequency of the converter according to the relationship in Expression (27):

$$-40log\left(\frac{f_s}{f_{out0}}\right)dB$$

(27)

There are diverse combinations of inductor and capacitor values for a given resonance frequency. The currents in the transient state of the converter can be reduced by increasing the inductance. However, the output voltages can be affected by small disturbances in the load due to the small values of the capacitance compared to the inductance. On the other hand, large inductors not only increase the cost and size of the output filter, they also increase the stress on the voltage of the inverter switches, because the voltage drops of the inductor result in losses in the output voltage. The resonance frequency determines the damping and stability of the output filter [35]. Its resonance frequency $f_{out0}$ is determined by the product of the inductance and capacitance, as show in Equation (28):

$$f_{out0}=\frac{1}{2\pi \sqrt{L_o{C}_0}}$$

(28)

where $L_o$ and $C_o$ are the inductance and capacitance of the filter, respectively. The optimal design of the $LC$ output filter has been addressed in various publications, and several investigations are based on a filter cost function. This cost function is defined by the typical reactive power in the filter components under steady state conditions [33,36].

From the point of view of cost and weight, the capacitor is the most economical design element. As for the output current of the converter, a large inductance has the advantage of limiting abrupt variations, but it is the most expensive design element. Figure 5 shows the scheme of the output filter in the frequency domain $\left(s\right)$, where $r_o$ represents the internal resistance of the inductor. The transfer function of the output filter is determined with Equation (29):

$$\frac{V_o\left(s\right)}{V_i\left(s\right)}=\left(\frac{\frac{1}{L_o{C}_o}}{s^2+\left(\frac{r_0}{L_o}\right)s+\left(\frac{1}{L_o{C}_o}\right)}\right)$$

(29)

The second order characteristic equation in the denominator of Equation (29) gives the relation between the damping factor $\zeta$ and the values of $L_o$ and $C_o$, in Equation (30):

$$L_o=\frac{r_o^2{C}_o}{4{\zeta }_o^2}$$

(30)

Like in the input filter, the resonance frequency for the output filter must be higher than the fundamental frequency of the output voltage and lower than one third of the switching frequency to avoid resonance problems, as in expression (31):

$$f_o\le f_{out0}\le \frac{1}{3}{f}_s$$

(31)

Output Filter Optimization

In this subsection, the problem of the output filter is solved using DE, optimizing the performance by means of the elements that make it up. The output filter shown in Figure 5 contains the elements $C_o$ and $L_o$. Considering the damping ${\zeta }_o$ and the resonant frequency of the filter $f_{out0}$, therefore the design variables are as in Expression (32):

$$\mathbf{x}={\{C_o,L_o,{\zeta }_o,f_{out0}\}}^T$$

(32)

Expression (33) is obtained by representing the design vector in terms of the optimization problem,

$$\mathbf{x}={\{x_1,x_2,x_3,x_4\}}^T$$

(33)

The objective function to minimize corresponds to the amplitude response of the output filter in the frequency behavior, declared in Equation (34);

minimize:

$$\begin{array}{cc}\hfill f(x_1,x_2,x_3,x_4)& =-20log|H_1\left(j{\omega }_1\right){|}_{{\omega }_1=x_4}\hfill \\ \hfill & -20log|H_2\left(j{\omega }_2\right){|}_{{\omega }_2=x_4+500}\hfill \\ \hfill & -20log|H_3\left(j{\omega }_3\right){|}_{{\omega }_3=x_4-500}\hfill \end{array}$$

(34)

subject to Expressions (35) to (41):

$$h_1(x_1,x_2,x_3,x_4)=x_1-{\displaystyle \frac{1}{{\left(2\pi x_4\right)}^2{x}_2}}=0$$

(35)

$$h_2(x_1,x_2,x_3,x_4)=x_3-\sqrt{\frac{r_i^2{x}_1}{4x_2}}=0$$

(36)

$$h_3(x_1,x_2,x_3,x_4)=x_2-{\displaystyle \frac{1}{2\pi \sqrt{x_2{x}_1}}}=0$$

(37)

$$1\times {10}^{-6}\le x_1\le 6\times {10}^{-6}$$

(38)

$$1\times {10}^{-6}\le x_2\le 1\times {10}^{-2}$$

(39)

$$0.01\le x_3\le 0.9$$

(40)

$$1000\le x_4\le 4000$$

(41)

The expression $H_i\left(j{\omega }_i\right)$ in Equation (34) is defined by Equations (43) to (44):

$$\begin{array}{c}\hfill H_i\left(j{\omega }_i\right)=\sqrt{{\left[G_{1i}\right]}^2+{\left[G_{2i}\right]}^2}i=1,2,3\end{array}$$

(42)

$$\begin{array}{c}\hfill G_{1i}=\frac{\frac{1}{x_2^2{x}_1^2}-\frac{{\left({\omega }_i\right)}^2}{x_2{x}_1}}{{\left(\frac{1}{x_1{x}_2}-{\left({\omega }_i\right)}^2\right)}^2+{\left(\frac{r_o{\omega }_i}{x_2}\right)}^2}i=1,2,3\end{array}$$

(43)

$$\begin{array}{c}\hfill G_{2i}=\frac{\frac{-r_o{\omega }_i}{x_2^2{x}_1}}{{\left(\frac{1}{x_1{x}_2}-{\left({\omega }_i\right)}^2\right)}^2+{\left(\frac{r_o{\omega }_i}{x_2}\right)}^2}i=1,2,3\end{array}$$

(44)

In order to test the proposed methodology, it was applied to the design of a converter with a nominal power of $P_n=746$ W, with a maximum variable output frequency of $f_{out0}=480$ Hz, switching frequency of $f_s=1200$ Hz, and a variable voltage up to 117 V.

5. Differential Evolution

DE is an optimization algorithm that simulates the evolutionary process of a species known as natural selection. It is based on two operators (crossover $Cr$ and mutation F) and a population of individuals $NP$. DE selects three different individuals (the first is the parent) and generates a new one. This new individual is compared to the parent, and the best survives. Algorithm 1 presents the pseudo-code corresponding to DE. For this work, the selection was made by means of the so-called rules of Deb [37], which are as follows:

• Between two feasible individuals, the one with the best aptitude is selected.
• Between a feasible individual and an unfeasible one, the feasible one is selected.
• Between two non-feasible individuals, the one that least violates the sum of constraints is selected.

Algorithm 1: Differential evolution.

6. Results

The results were obtained by solving the proposed optimization problem using a modified differential evolution algorithm that included Deb’s rules for constraint management. For both filters (input and output), the selection of the ED parameters was adjusted with a trial and error approach, considering the number of design variables and the population. We executed the algorithm from a combination of initial parameters and adjusted it manually during ten executions, until we obtained a stable behavior [38]. The selection of these parameters was directly related to the order of the filter to be designed, and it may be necessary to adjust manually for more than ten executions in order to obtain a convergence in the population with feasible quality solutions.

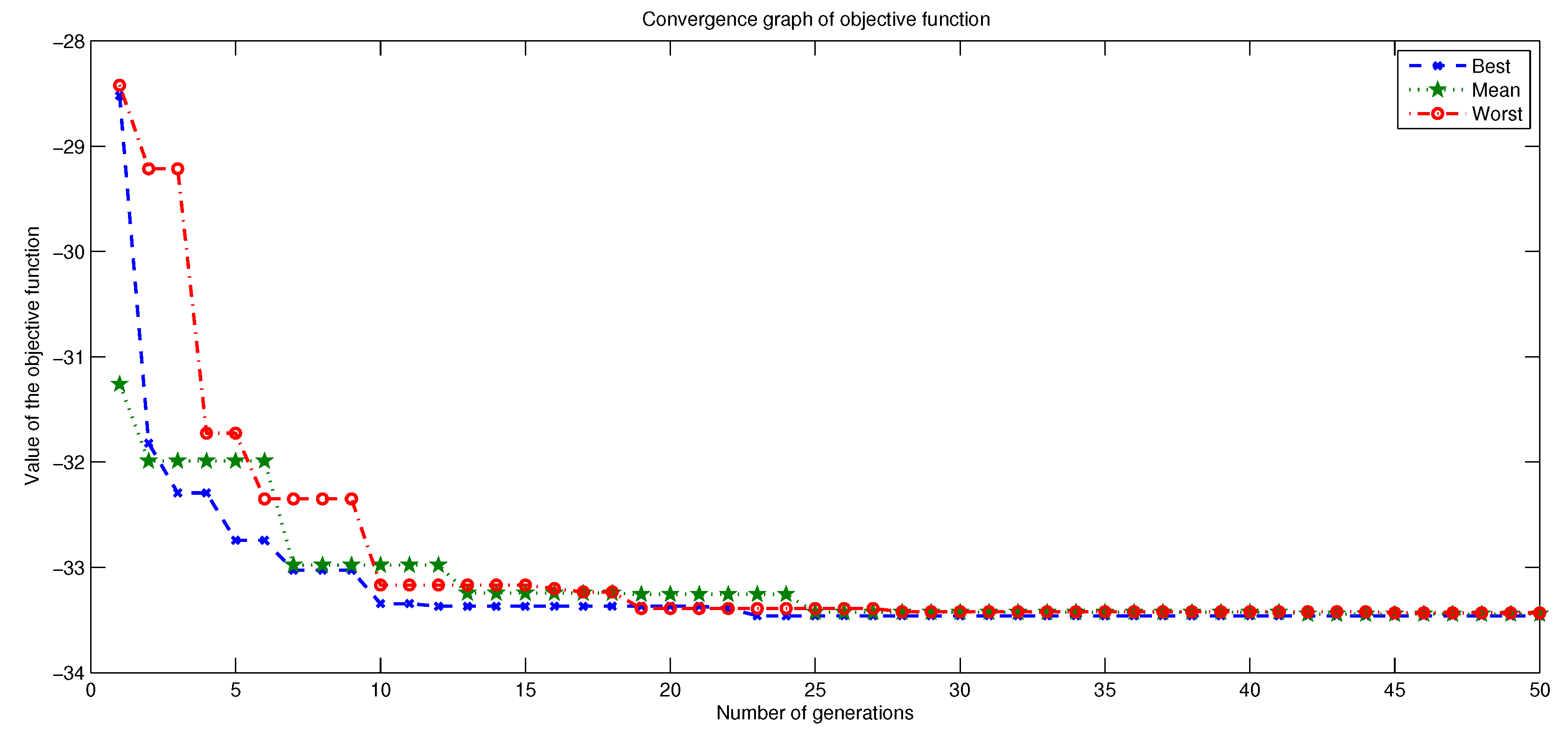
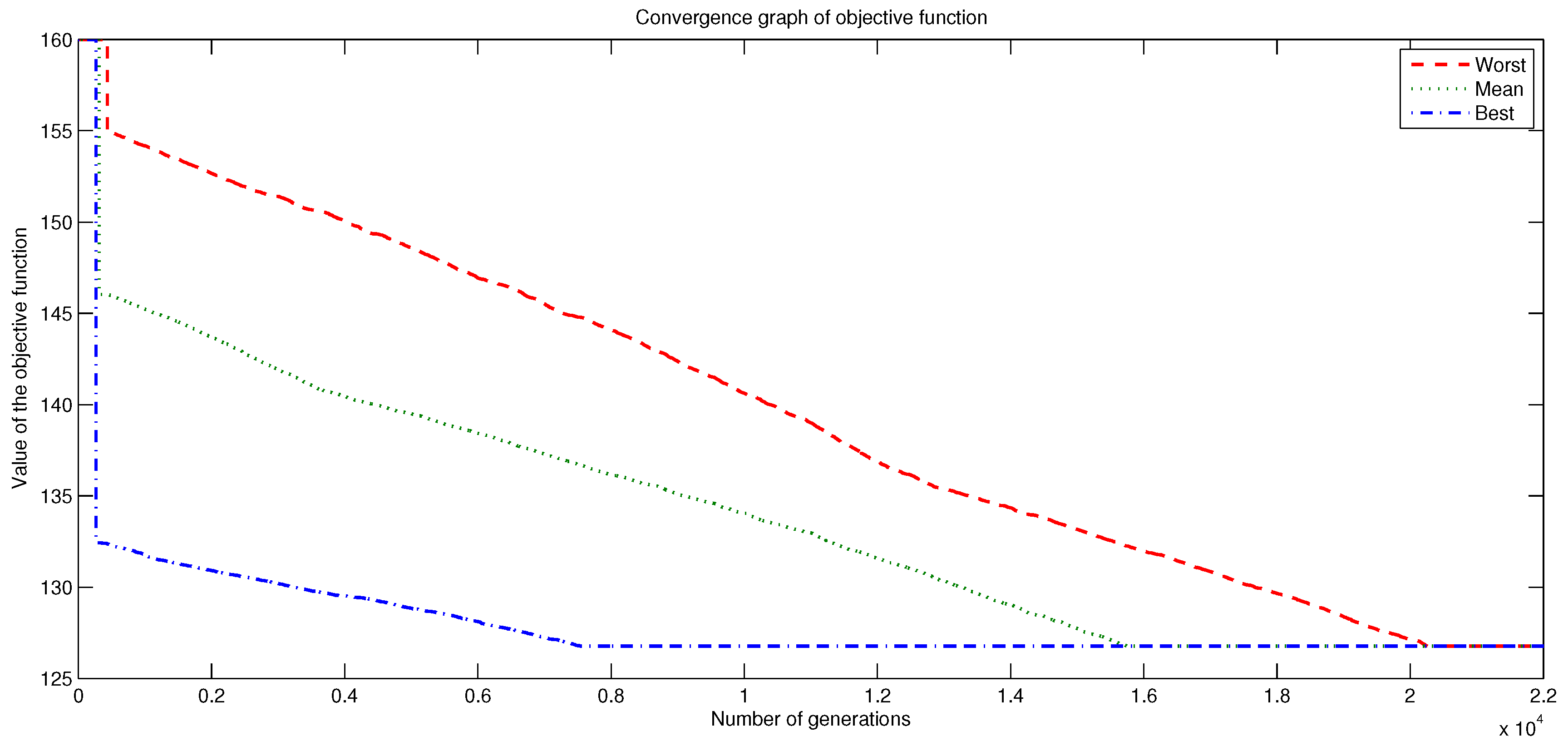
6.1. Optimal Solutions

- Input filter:

The tuning parameters of DE for the input filter were:

• Design variables: 3
• Individuals: 50
• Generations: 500
• Mutation factor: random from $0.4$ to $0.9$
• Cross factor: random from $0.3$ to $0.9$

The mutation factor was randomly obtained by generation, while the cross factor was randomly generated for each run, in order to diversify the exploitation and exploration while solving the optimization problem. The population number was determined in the tuning to have a large initial exploration and thus achieved the convergence of the algorithm in a smaller number of generations. The algorithm was executed 30 times to observe its convergence, and it produced the same results in each run.

Table 1. Best individuals obtained for the input filter problem.

${\mathit{x}}_1\left[\mathit{F}\right]$	${\mathit{x}}_2\left[\mathit{H}\right]$	${\mathit{x}}_3\left[\mathit{\zeta }\right]$
$2.80\times {10}^{-6}$	$0.00628000000000028$	$0.187555316441543$
$2.80\times {10}^{-6}$	$0.00628000000000017$	$0.187555316657591$
$\mathbf{2.80}\times {\mathbf{10}}^{-\mathbf{6}}$	$\mathbf{0.00628000000000007}$	$\mathbf{018755531746872}$${}^a$
$2.80\times {10}^{-6}$	$0.0062800000000000$	$0.187555315666788$
$2.80\times {10}^{-6}$	$0.00628000000000013$	$0.187555317687343$
$2.80\times {10}^{-6}$	$0.00628000000000008$	$0.187555317484832$
$2.80\times {10}^{-6}$	$0.00628000000000026$	$0.187555315207603$
$2.80\times {10}^{-6}$	$0.00628000000000005$	$0.187555313375193$
$2.80\times {10}^{-6}$	$0.00628000000000013$	$0.187555317687343$
$2.80\times {10}^{-6}$	$0.00628000000000026$	$0.187555317149325$
$2.80\times {10}^{-6}$	$0.00628000000000025$	$0.187555316290116$
$2.80\times {10}^{-6}$	$0.00628000000000012$	$0.187555317506824$
$2.80\times {10}^{-6}$	$0.00628000000000013$	$0.187555315746281$
$2.80\times {10}^{-6}$	$0.00628000000000026$	$0.187555315616287$
$2.80\times {10}^{-6}$	$0.00628000000000013$	$0.187555317592858$
$2.80\times {10}^{-6}$	$0.00628000000000017$	$0.187555316425078$
$2.80\times {10}^{-6}$	$0.00628000000000012$	$0.187555317506824$
$2.80\times {10}^{-6}$	$0.00628000000000013$	$0.18755531680009$
$2.80\times {10}^{-6}$	$0.00628000000000022$	$0.187555316555392$
$2.80\times {10}^{-6}$	$0.00628000000000022$	$0.187555316820297$
$2.80\times {10}^{-6}$	$0.00628000000000018$	$0.187555316346879$
$2.80\times {10}^{-6}$	$0.00628000000000013$	$0.187555317471883$
$2.80\times {10}^{-6}$	$0.00628000000000013$	$0.187555315714449$
$2.80\times {10}^{-6}$	$0.00628000000000022$	$0.187555315578319$
$2.80\times {10}^{-6}$	$0.00628000000000026$	$0.187555316660662$
$2.80\times {10}^{-6}$	$0.00628000000000008$	$0.187555314295145$
$2.80\times {10}^{-6}$	$0.00628000000000038$	$0.18755531713709$
$2.80\times {10}^{-6}$	$0.00628000000000026$	$0.187555315947656$
$2.80\times {10}^{-6}$	$0.00628000000000026$	$0.187555316199455$
$2.80\times {10}^{-6}$	$0.00628000000000012$	$0.187555317592252$

${}^a$ Selected solution vector for the simulation stage.

lists the best individual of each execution, and for all of them, the value of the objective function was $-33.4705013527862$ dB.

The statistical analysis of the results is presented in

Table 2. Table of the results from the statistical analysis.

Parameter	Objective Function (dB)
Best	$-33.4705013527862$
Worst	$-33.4705013527862$
Average	$-33.4705013527862$
Standard deviation	$1.519204271928768\times {10}^{-14}$
Variance	$5.2357\times {10}^{-29}$

. As can be seen, the variance and the standard deviation were almost zero, which indicated the stability of the algorithm. Additionally, the convergence graph is presented in Figure 6 including the best, the average, and the worst convergence behavior of the thirty runs. It can be seen that differential evolution converged to the same minimum value of the objective function. The values obtained in the design vector in the 30 runs were the same; therefore, it could be concluded that these were the best optimal values found for the optimization problem.

- Output filter:

The results obtained for the output filter are presented. The tuning parameters of DE were as follows:

• Design variables: 4
• Individuals: 20
• Generations: 30,000
• Mutation factor: random from $0.6$ to $0.9$
• Cross factor: random from $0.5$ to $0.9$

As for the input filter case, the mutation factor was randomly obtained by generation, while the cross factor was randomly generated by run. The algorithm was evaluated thirty times.

Table 3. The 30 best individuals for the output filter problem.

${\mathit{x}}_1\left(\mathit{F}\right)$	${\mathit{x}}_2\left(\mathit{H}\right)$	${\mathit{x}}_3\left(\mathit{\zeta }\right)$	${\mathit{x}}_4$ (Hz)
$6.00\times {10}^{-6}$	$0.00422172331848171$	$0.0100202556790679$	1000
$6.00\times {10}^{-6}$	$0.00422172331847941$	$0.010052461482379$	1000
$6.00\times {10}^{-6}$	$0.00422172331855535$	$0.0100084096245472$	1000
$6.00\times {10}^{-6}$	$0.00422172331855535$	$0.0100248266410373$	1000
$6.00\times {10}^{-6}$	$0.00422172331855619$	$0.0100240795211493$	1000
$6.00\times {10}^{-6}$	$0.00422172331855619$	$0.0100240795211493$	1000
$6.00\times {10}^{-6}$	$0.00422172331855619$	$0.0100240795211493$	1000
$6.00\times {10}^{-6}$	$0.00422172331855619$	$0.0100240795211493$	1000
$6.00\times {10}^{-6}$	$0.00422172331855619$	$0.0100240795211493$	1000
$6.00\times {10}^{-6}$	$0.00422172331855535$	$0.0100367173404247$	$1000.078$
$6.00\times {10}^{-6}$	$0.00422172331855619$	$0.0100240795211493$	1000
$6.00\times {10}^{-6}$	$0.00422172331855619$	$0.0100240795211493$	1000
$6.00\times {10}^{-6}$	$0.00422172331855535$	$0.0100139735214739$	1000
$6.00\times {10}^{-6}$	$0.00422172331848171$	$0.0100242889101986$	1000
$6.00\times {10}^{-6}$	$0.00426652020811279$	$0.0100348335707767$	1000
$6.00\times {10}^{-6}$	$0.00422172331855619$	$0.0100240795211493$	1000
$\mathbf{6.00}\times {\mathbf{10}}^{-\mathbf{6}}$	$\mathbf{0.00422172331855619}$	$\mathbf{0.0100240795211493}$	$\mathbf{1000}$${}^a$
$6.00\times {10}^{-6}$	$0.00422172331855619$	$0.0101197674322796$	1000
$6.00\times {10}^{-6}$	$0.00422172331855619$	$0.0100240795211493$	1000
$6.00\times {10}^{-6}$	$0.00422172331855619$	$0.0100240795211493$	1000
$6.00\times {10}^{-6}$	$0.00422172331855619$	$0.0100240795211493$	1000
$6.00\times {10}^{-6}$	$0.00422172331855619$	$0.0100614407958508$	1000
$6.00\times {10}^{-6}$	$0.00422172331855619$	$0.0100240795211493$	1000
$6.00\times {10}^{-6}$	$0.00422172331855619$	$0.0100240795211493$	1000
$6.00\times {10}^{-6}$	$0.00422172331855619$	$0.0100240795211493$	1000
$6.00\times {10}^{-6}$	$0.00422172331855619$	$0.0100240795211493$	1000
$6.00\times {10}^{-6}$	$0.00422172331855535$	$0.0100348335707767$	1000
$6.00\times {10}^{-6}$	$0.00422172331855619$	$0.0100240795211493$	1000
$6.00\times {10}^{-6}$	$0.00422172331848171$	$0.0100601087728762$	1000
$6.00\times {10}^{-6}$	$0.00422172331855619$	$0.0100240795211493$	1000

${}^a$ Selected solution vector for the simulation stage.

shows the best result of each run. For each individual, the value of the objective function was 126.767966149519 dB.

The statistical analysis of these results is presented in

Table 4. Table of the results from the statistical analysis.

Parameters	Objective Function (dB)
Best	$126.767966149519$
Worst	$126.767966149519$
Average	$126.767966149519$
Standard deviation	$2.6203521364587013\times {10}^{-14}$
Variance	$6.8662245317\times {10}^{-28}$

. As can be seen, the variance and the standard deviation were approximated to zero, which indicated the stability of the algorithm. The convergence graph is presented in Figure 7, including the best, the average, and the worst convergence behavior of the 30 runs. It could be seen that DE converged uniformly to a minimum of the objective function. The values obtained in the design vector in the 30 runs were the same, and based on the convergence of the algorithm, it was concluded that the values found were optimal for the problem posed.

The tuning parameters for the DE algorithm reported in each of the problems for both the input filter and the output filter were different because the chosen topology for each and the constraints were different, which implied two distinct optimization problems.

The methodology proposed in this work was different from that found so far in the literature because it approached the design of filters from an optimal approach. Based on the filter response and a series of constraints with a well-defined, the work carried out so far dealt with the design of the filter, taking into account only the design restrictions and part of a fixed value of some of the parameters to obtain the others.

6.2. Stability

The structure of the bidirectional switching cell shown in Figure 1 was formed by an arrangement of switches. To obtain the model of the cell, the switches were considered as ideal. This was possible because the switching frequency was much greater than the modulation frequency [39,40], and with the switching constraint in Equation (2), the system dynamics were only related to the input and output filters. Figure 8 shows the diagram of the electrical equivalent.

The stability of the system was determined by the model of the input and output filters, for which Equation (45) represents the transfer function of one of the phases of the system, thus replacing the values found by DE. The characteristic polynomial was a Hurwits type, which guaranteed that the system was stable.

$$\frac{V_o\left(s\right)}{V_i\left(s\right)}=\frac{\left[\frac{1}{R_d{C}_i{L}_o{C}_o}\right]s+\left[\frac{1}{L_i{C}_i}+\frac{r_i}{R_d{L}_i{C}_i}\right]\frac{1}{L_o{C}_o}}{s^4+A{s}^3+B{s}^2+Cs+D}$$

(45)

where:

$$A=\frac{r_0}{L_o}+\frac{r_i}{L_i}+\frac{1}{R_d{C}_i}$$

(46)

$$B=\frac{1}{L_o{C}_o}+\left[\frac{r_i}{L_i}+\frac{1}{R_d{C}_i}\right]\left[\frac{r_0}{L_o}\right]+\left[\frac{1}{L_i{C}_i}+\frac{r_i}{R_d{L}_i{C}_i}\right]$$

(47)

$$C=\left[\frac{r_i}{L_i}+\frac{1}{R_d{C}_i}\right]\frac{1}{L_o{C}_o}+\left[\frac{1}{L_i{C}_i}+\frac{r_i}{R_d{L}_i{C}_i}\right]\frac{r_0}{L_o}$$

(48)

$$D=\left[\frac{1}{L_i{C}_i}+\frac{r_i}{R_d{L}_i{C}_i}\right]\frac{1}{L_o{C}_o}$$

(49)

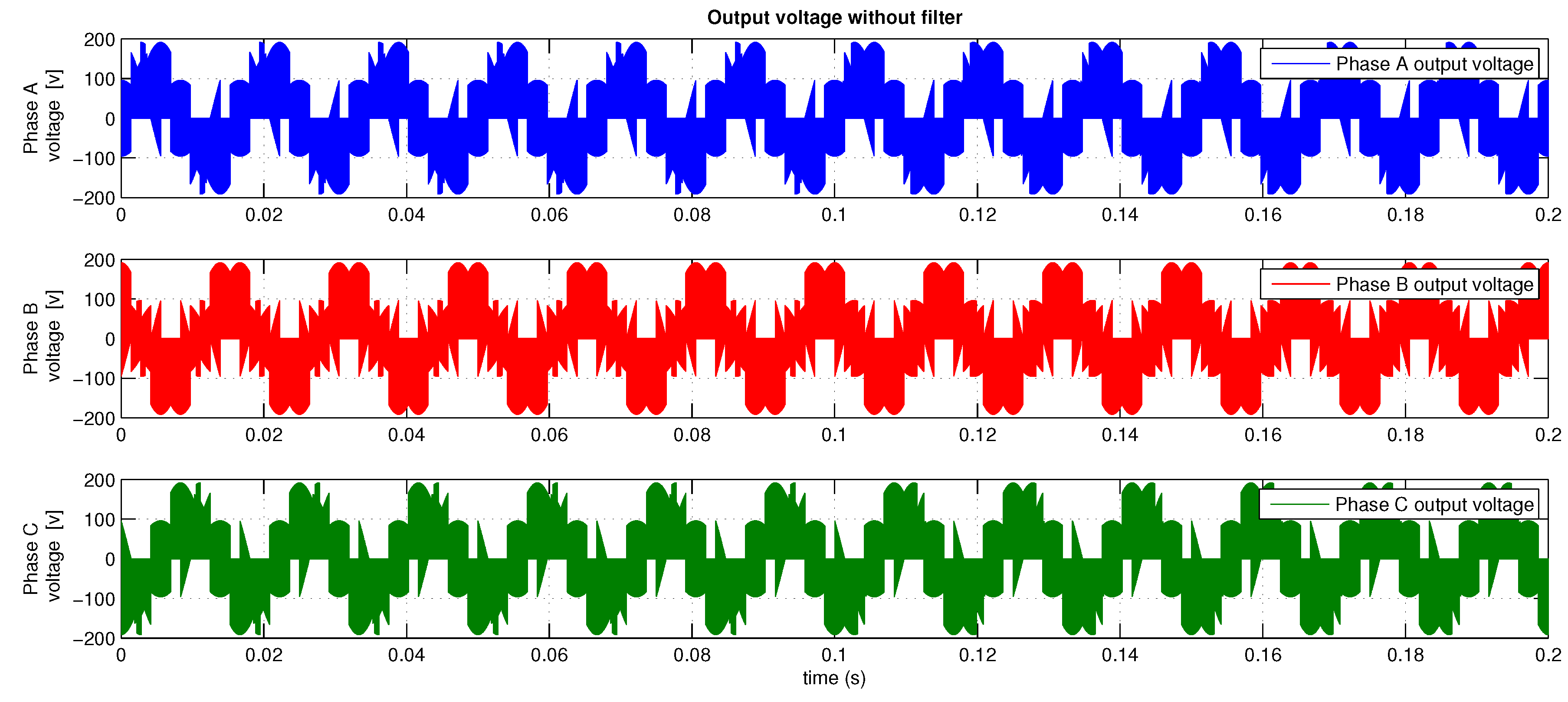
6.3. Simulations

The input and output filters obtained from the solution of the optimization problems were simulated in an implementation of a three-phase converter with each of the input and output lines, applying a Venturini modulation technique. The response of the converter without input filter can be seen in Figure 9 and Figure 10. Both graphs show the three-phase signals of the output voltage and input current switched, produced by the bidirectional switches, which generated harmonics in both signals.

Figure 11 shows the output voltage when the output filter was implemented. As could be seen, this signal did not present considerable harmonic disturbances, only at the beginning and at $0.1$ s, when a transition stage was presented in two steps of the desired signals of 50 peak voltage and 80peak voltage. In Figure 12, the input current signal for the same desired signal is presented. It could be seen that there were no harmonic disturbances in the currents of each phase, only in the transient points, at the beginning and at the two desired voltage levels. It is important to mention that there was an inductive output load of 1 mH and a resistive load of $100\Omega$.

As a final result, the magnitude and phase graphs for each of the filters are presented. The graph of the input filter is shown in Figure 13, including two signals. One is the filter response with the elements obtained by means of the optimization problem $(C_i=2.80\mathsf{\mu }$f, $L_i=6.28$ mH, ${\zeta }_i=0.187)$, and the other is the response of the filter with elements obtained by the analytic method $(C_i=2\mathsf{\mu }$f, $L_i=7$ mH, ${\zeta }_i=0.1)$. It could be determined that the proposed optimization problem obtained a smaller response in magnitude, in a ratio $39.5\%$ lower than obtained through the analytic method. In Figure 14, the response of the output filter is presented, and in the same way, two signals obtained by means of the analytic method $(C_o=6.33\mathsf{\mu }$f, $L_o=1$ mH, ${\zeta }_o=0.006,f_{out0}=2000)$ are shown and the other by means of the optimization problem $(C_o=6\mathsf{\mu }$f, $L_o=4.22$ mH, ${\zeta }_o=0.01,f_{out0}=1000)$. The magnitude graph presents a relationship of $14.3\%$ lower than that obtained by the analytic method.

In Figure 13 and Figure 14, it can be seen that small variations in the parameters of each of the filter elements showed a significant advantage in their response. The design parameters initially set were for a 746 W nominal power converter, an input frequency of 60 Hz, with a voltage of 117 V, a maximum variable output frequency of 400 Hz, and a variable amplitude voltage. The design methodology proposed in this work offered a better input and output filter response compared to an analogousdesign methodology, but it could be applied to the design of a converter with other design parameters.

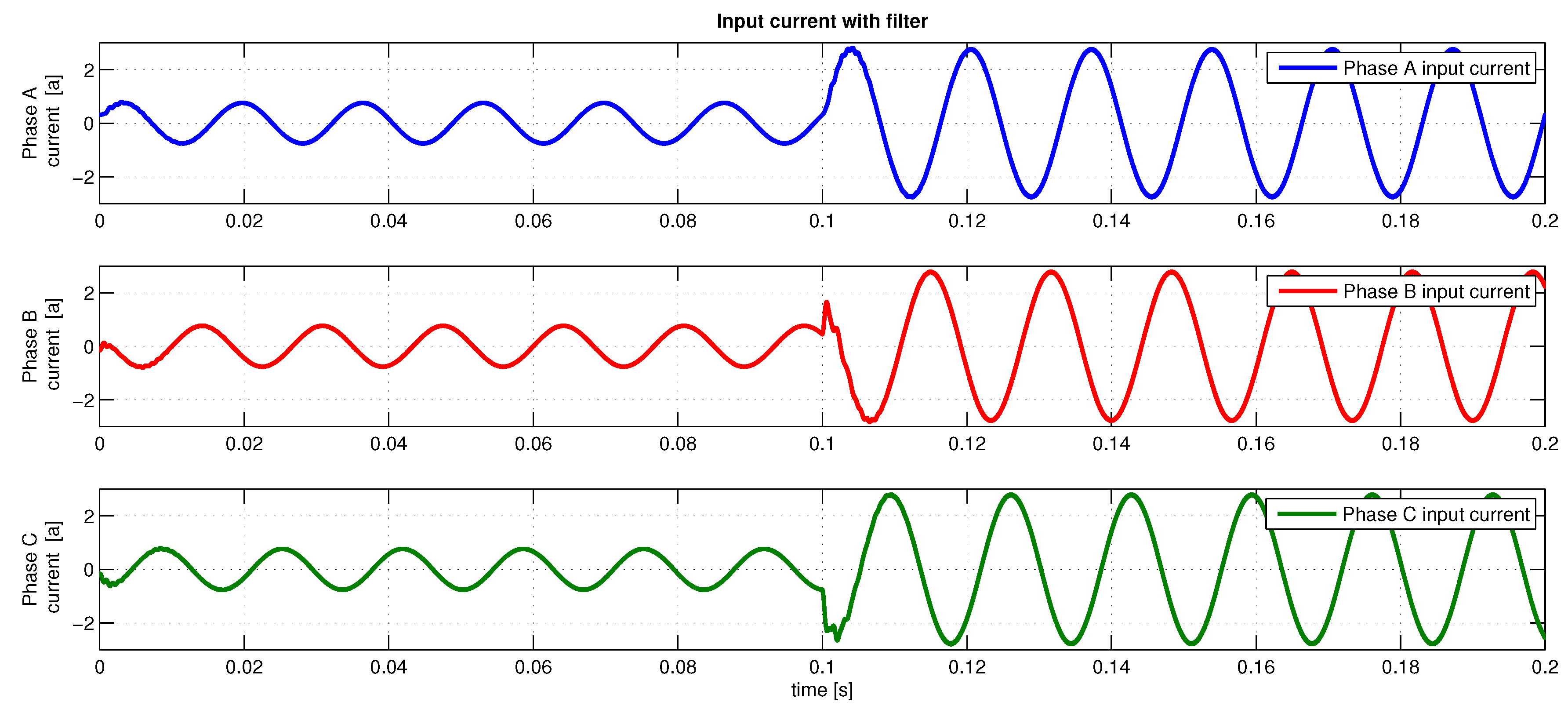
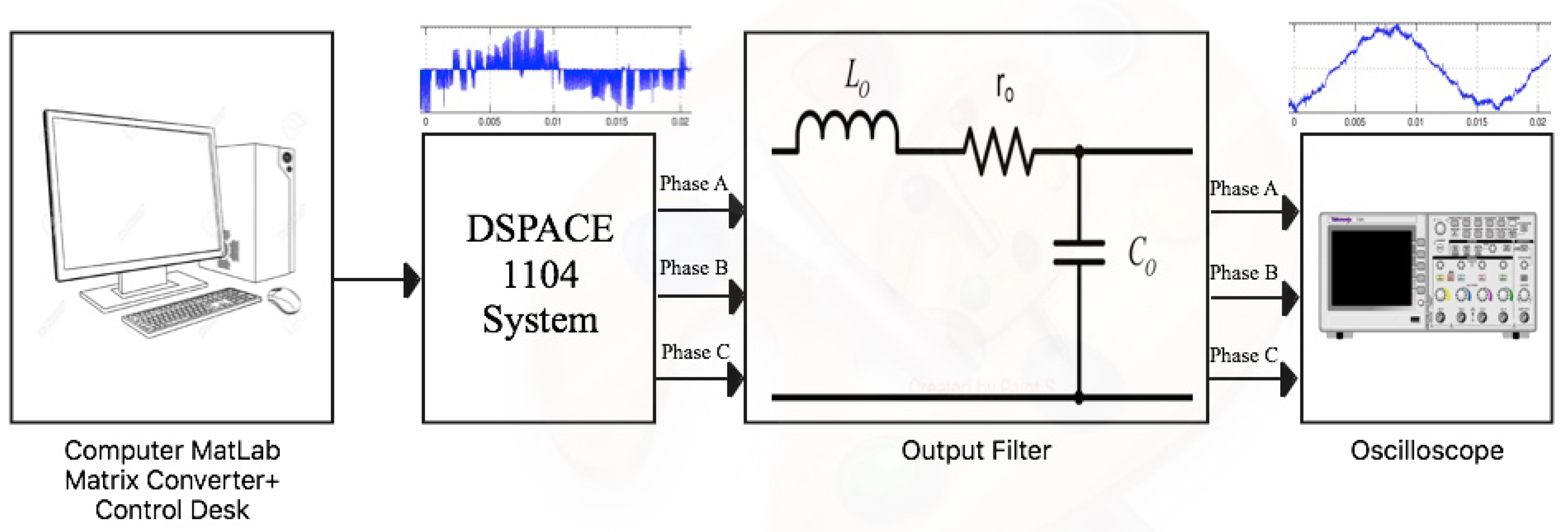
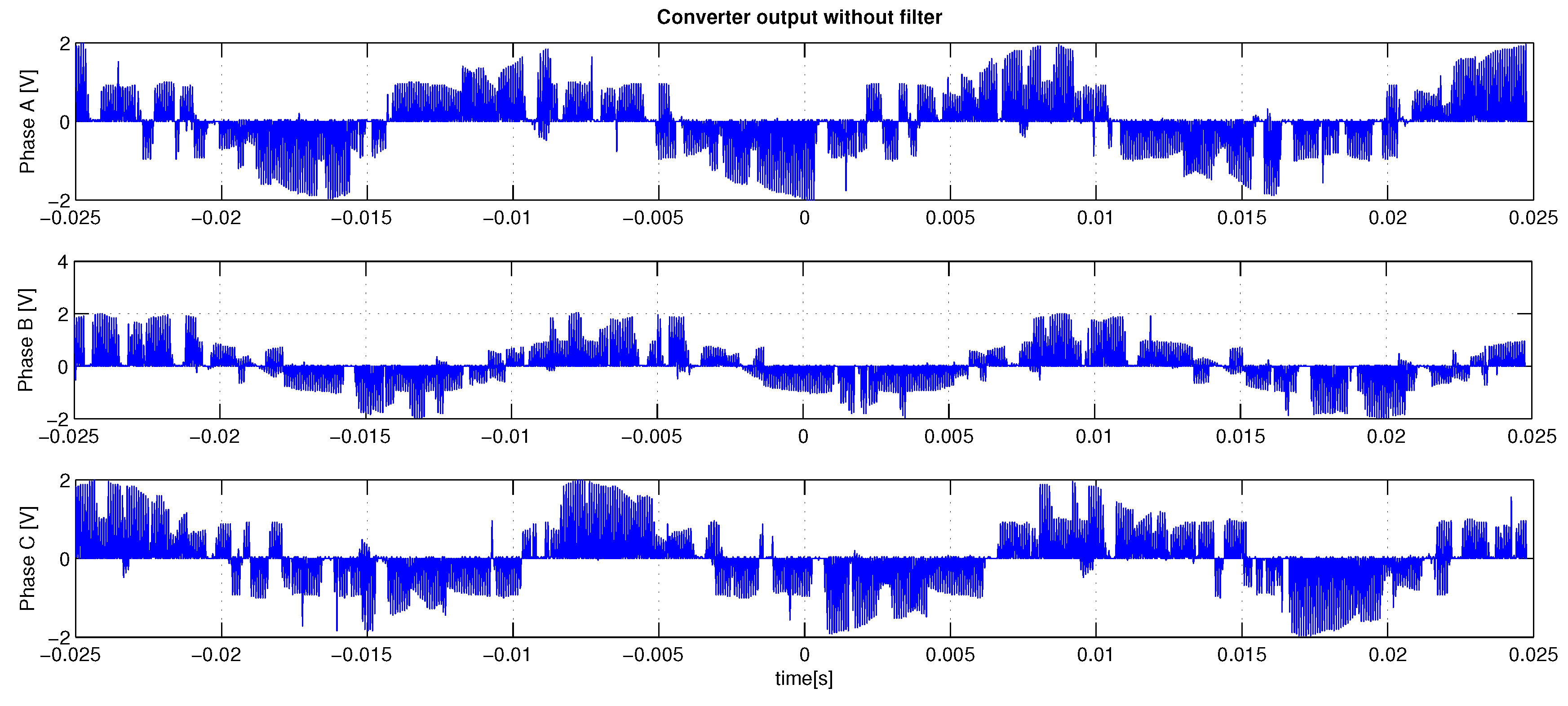
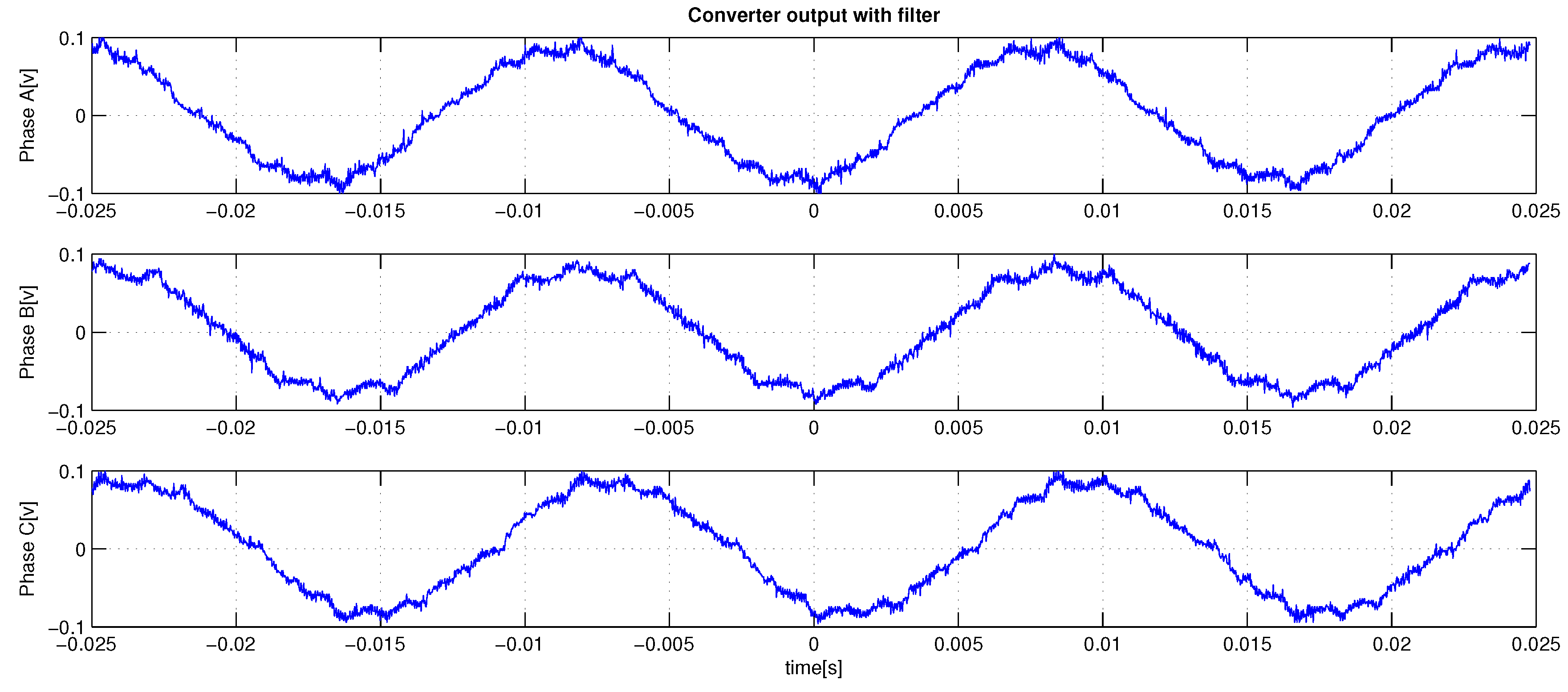
6.4. Experimental Result

The experimental implementation of the optimization scheme proposed in this work was carried out by means of a dSPACE DS1104 control board, supported by the software MATLAB 2009™(MathWorks™, Natick, MA, USA). Through this device, a direct MC was designed to emulate the behavior of a real converter powered by a three-phase source of alternating voltage at 60 Hz. The converts implemented in the control board generated the three output phase modules through the Venturi technique, to reconstruct a 10 V output signal, as shown in Figure 15. The modulated signals were generated by means of three channels of the control board (Figure 16). Each of these signals was the input source of three low-pass filters, as shown in Figure 5. The output filters were constructed with the values obtained by DE. The results can be seen in Figure 17.

It should be pointed out that the maximum frequency supported by the DS1104 control board was lower than the calculated frequency for the system, so the emulation of the implemented MC in the DS1104 did not allow solving the problem with a higher resolution because the obtained signals presented some harmonic distortions. In spite of this, the output filter produced the desired 10 V signal.

7. Conclusions

In this paper, a novel methodology for the design of the input and output filters for a matrix converter using evolutionary technical was presented. Both filters were designed as constrained numerical optimization problems, which in turn were solved by means of the differential evolution algorithm. The proposed objective function corresponded to the minimization of the frequency response. The first advantage of this method was that the nominal values of all filter elements were obtained simultaneously, without requiring a base element value. The second advantage was that the topology of both filters was defined from the problem statement as a second order basic configuration. The numerical results in both optimization problems had a stable behavior with a standard deviation of 30 corrections for each problem with a value of 1.519204271928768 $\times {10}^{-14}$ and 2.6203521364587013 $\times {10}^{-14}$. In a comparative analysis of the nominal values requested by the method proposed in this work and an analytical method, a reduction in the response of the input filter $39.5\%$ and for the output filter $14.3\%$ was obtained, which implied an improvement in the performance of both filters.

The quality of the results generated by the method proposed for the case study exceeded the reports for the conventional approach, presenting a significant reduction in the amplitude of the frequency response. He demonstrated the usefulness of metaheuristic methods such as the differential evolution algorithm to solve complex engineering problems, presenting an important alternative to classical methods.

In order to facilitate the test of the system stability, a simplified model was proposed that considered the bidirectional switches as ideal elements, reducing the order of the characteristic polynomial associated with the system. As a result of the adequate calculation of the input and output filters in the matrix converter, there was a complete elimination of harmonic signals that affected both the output voltages and the input currents produced by the power switches. This was verified by solving a well-known case study from the related literature applying the proposed method and comparing the obtained results with those corresponding to the solution of the same problem by the analytic method.

As future work, it is proposed to combine the design of both filters, input and output, in the same optimization problem with discrete search spaces. Another future work is to propose a multi-objective optimization model that can involve other aspects of development such as cost, topology, stability, and energy. Further work needs to be done on the implementation of a control technique, which can be self-tuned by means of another metaheuristic algorithm. Finally, the implementation of the design methodology proposed in this paper in matrix converters worked with unbalanced loads and four legs. Finally, since the implementation of the converter was carried out in a control board that emulated its behavior, the analysis of power losses is considered for future work.