Adaptive Model-Free Coupling Controller Design for Multi-Axis Motion Systems
Abstract
1. Introduction
2. Multi-Axis Systems and Recurrent Fuzzy Neural Networks
2.1. System Description
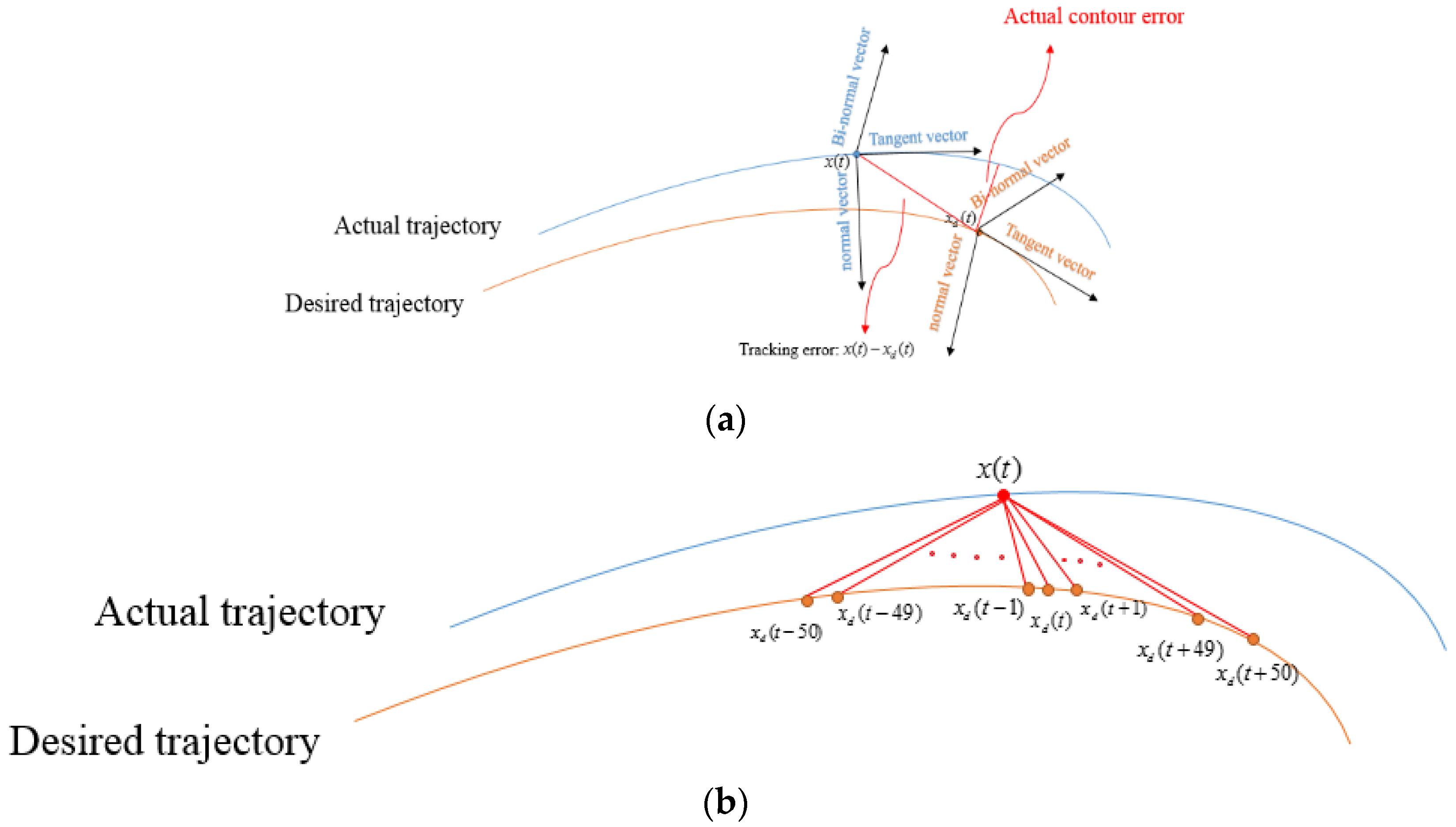
- (a)
- (b)
- Skew-symmetric: matrices and skew-symmetric and satisfy , .
- (c)
- Linear parameterize: system (4) can be linearized parameterized , where contains the unknown constant system parameters and denotes a regression matrix [11].
2.2. Recurrent Fuzzy Neural Network
3. Adaptive Model-Free Coupling Controller Design Using RFNN
4. Simulation Results
4.1. Calculation Contour Error and Actual Contour Error
4.2. Discussion on Adaptation of Controller
4.3. Comparison Controller Using FNN, RFNN
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Altintas, Y. Manufacturing Automation: Metal Cutting Mechanics, Machine Tool Vibrations, and CNC Design; Cambridge University Press: New York, USA, Cambridge, UK, 2012. [Google Scholar]
- Suh, S.H.; Chung, S.K.; Stround, D.H. Theory and Design of CNC Systems; Springer: Cham, Switzerland, 2008. [Google Scholar]
- Bretschneider, J.; Menzel, T. Virtual optimization of machine tools and production process. Int. J. Autom. Technol. 2007, 1, 136–140. [Google Scholar] [CrossRef]
- Koren, Y. Cross-coupled biaxial computer control for manufacturing systems. ASME Trans. J. Dyn. Syst. Meas. Control 1980, 102, 265–272. [Google Scholar] [CrossRef]
- Koren, Y.; Lo, C.C. Variable-gain cross-coupling controller for contouring. Ann. CIRP 1991, 40, 371–374. [Google Scholar] [CrossRef]
- Huang, C.H.; Lin, C.L. Evolutionary neural networks and DNA computing algorithms for dual-axis motion control. Eng. Appl. Artif. Intell. 2011, 24, 1263–1273. [Google Scholar] [CrossRef]
- Yeh, S.S.; Hsu, P.L. Estimation of the contouring error vector for the cross-coupled control design. IEEE/ASME Trans. Mechatron. 2002, 7, 44–51. [Google Scholar]
- Chen, S.L.; Wu, K.C. Contouring control of smooth paths for multi-axis motion systems based on equivalent errors. IEEE Trans. Control Syst. Technol. 2007, 15, 1151–1158. [Google Scholar] [CrossRef]
- Sencer, B.; Altintas, Y.; Croft, E. Modeling and Control of Contouring Errors for Five-Axis Machine Tools—Part I: Modeling. J. Manuf. Sci. Eng. 2009, 131, 031006. [Google Scholar] [CrossRef]
- Sencer, B.; Altintas, Y. Modeling and control of contouring errors for five-axis machine tools—Part II: Precision contour controller design. J. Manuf. Sci. Eng. 2009, 131, 031007. [Google Scholar] [CrossRef]
- Lee, J.; Dixon, W.E.; Ziegert, J.C. Adaptive nonlinear contour coupling control for a machine tool system. Int. J. Adv. Manuf. Technol. 2012, 61, 1057–1065. [Google Scholar] [CrossRef]
- Shi, R.; Lou, Y. A novel contouring error estimation for three-dimensional contouring control. IEEE Robot. Autom. Lett. 2016, 2, 128–134. [Google Scholar] [CrossRef]
- Ghaffari, A.; Galip Ulsoy, A. Dynamic contour error estimation and feedback modification for high-precision contouring. IEEE/ASME Trans. Mechatron. 2016, 21, 1732–1741. [Google Scholar] [CrossRef]
- Wang, Z.; Hu, C.; Zhu, Y.; He, S.; Zhang, M.; Haihua, M. Newton-ILC contouring error estimation and coordinated motion control for precision multiaxis systems with comparative experiments. IEEE Trans. Ind. Electron. 2018, 65, 1470–1480. [Google Scholar] [CrossRef]
- Jia, Z.J.; Ma, J.W.; Song, D.N.; Wang, F.J.; Liu, W. A review of contouring-error reduction method in multi-axis CNC machining. Int. J. Mach. Tools Manuf. 2018, 125, 34–35. [Google Scholar] [CrossRef]
- Chuang, H.Y.; Liu, C.H. Cross-coupled adaptive feedrate control for multiaxis machine tools. ASME Trans. J. Dyn. Syst. Meas. Control 1991, 113, 451–457. [Google Scholar] [CrossRef]
- Bascetta, L.; Rocco, P. Force ripple compensation in linear motors based on closed-loop position-dependent identification. IEEE/ASME Trans. Mechatron. 2010, 15, 349–359. [Google Scholar] [CrossRef]
- Shi, W.J.; Zhang, D.C. Adaptive robust control of linear motor with ripple force compensation. In Proceedings of the 2011 Third Pacific-Asia Conference on Circuits, Communications and System (PACCS), Wuhan, China, 17–18 July 2011; pp. 1–4. [Google Scholar]
- Song, F.; Liu, Y.; Xu, J.X. Iterative learning identification and compensation of space-periodic disturbance in PMLSM systems with time delay. IEEE Trans. Ind. Electron. 2018, 65, 7579–7589. [Google Scholar] [CrossRef]
- Rohrig, C.; Jochheim, A. Identification and compensation of force ripple in linear permanent magnet motors. In Proceedings of the American Control Conference, Arlington, VA, USA, 25–27 June 2001. [Google Scholar]
- Lee, C.H.; Teng, C.C. Identification and control of dynamic systems using recurrent fuzzy neural networks. IEEE Trans. Fuzzy Syst. 2000, 8, 349–366. [Google Scholar]
- Yabuta, T.; Yamada, T. Learning control using neural networks. In Proceedings of the IEEE International Conference on Robotics and Automation, Sacramento, CA, USA, 9–11 April 1991; pp. 740–745. [Google Scholar]
- Lee, C.H.; Chang, F.Y.; Lin, C.M. An efficient interval type-2 fuzzy CMAC for chaos time-series prediction and synchronization. IEEE Trans. Cybern. 2013, 44, 329–341. [Google Scholar] [CrossRef]
- Chen, H.R.; Su, K.H.; Cheng, M.Y.; Lu, J.S. Parameter-based contour error estimation for contour following accuracy improvements. In Proceedings of the 2014 International Conference on Information Science, Sapporo, Japan, 26–28 April 2014; Volume 3, pp. 1638–1642. [Google Scholar]
- Chen, C.S.; Chen, L.Y. Robost cross-coupling synchronous control by shaping position commands in multiaxes systems. IEEE Trans. Ind. Electron. 2012, 59, 4761–4773. [Google Scholar] [CrossRef]
- Sun, D. Position synchronization of multiple motion axes with adaptive coupling control. Automatica 2003, 39, 997–1005. [Google Scholar] [CrossRef]
- Sun, D. Synchronization and Control of Multiagent Systems; CRC Press, Inc.: Boca Raton, FL, USA, 2010. [Google Scholar]
- Chen, B.S. Adaptive Coupling Controller Design for Multi-Axis Systems. Master Thesis, Department of Mechanical Engineering, National Chung Hsing University, Taichung, Taiwan, 2017. [Google Scholar]
- Tan, K.K.; Huang, S.N.; Lee, T.H. Robust adaptive numerical compensation for friction and force ripple in prmanent-magnet linear motors. IEEE Trans. Magn. 2002, 38, 221–228. [Google Scholar] [CrossRef]







| Case 1 | Case 2 | Case 3 | |
|---|---|---|---|
| Max. contour error (mm) | 0.0118 | 15.0848 | 13.8432 |
| Total contour error (mm) | 26.3217 | 106000 | 60349.14 |
| RMSE (mm) | 0.1660 | 9.0539 | 4.8477 |
| FNN | RFNN | |
|---|---|---|
| Max contour error (mm) | 16.1006 | 15.3143 |
| Total contour error (mm) | 113410.19 | 61780.23 |
| RMSE (mm) | 9.4631 | 4.6759 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, B.-S.; Lee, C.-H. Adaptive Model-Free Coupling Controller Design for Multi-Axis Motion Systems. Appl. Sci. 2020, 10, 3592. https://doi.org/10.3390/app10103592
Chen B-S, Lee C-H. Adaptive Model-Free Coupling Controller Design for Multi-Axis Motion Systems. Applied Sciences. 2020; 10(10):3592. https://doi.org/10.3390/app10103592
Chicago/Turabian StyleChen, Bo-Sheng, and Ching-Hung Lee. 2020. "Adaptive Model-Free Coupling Controller Design for Multi-Axis Motion Systems" Applied Sciences 10, no. 10: 3592. https://doi.org/10.3390/app10103592
APA StyleChen, B.-S., & Lee, C.-H. (2020). Adaptive Model-Free Coupling Controller Design for Multi-Axis Motion Systems. Applied Sciences, 10(10), 3592. https://doi.org/10.3390/app10103592






