Carbonation Process of Reinforced Concrete Beams under the Combined Effects of Fatigue Damage and Environmental Factors
Abstract
:1. Introduction
- Analyzing RC beams subjected to low fatigue damage, medium fatigue damage, and high fatigue damage;
- Determining the deterioration in the pore structure from the accumulation of fatigue damage;
- Experimentally investigating the carbonation process of RC beams under the combined effects of carbonation and fatigue damage in an environmental simulation chamber.
- Creating a numerical model to simulate the carbonation process in RC beams considering the combined effects of carbonation and cyclic loading.
2. Experimental Procedure
2.1. Materials and Specimens
2.2. Fatigue Damage Test
2.3. Mercury Intrusion Test
2.4. Carbonation Test
2.4.1. Artificial Accelerated Simulation Environment Test
2.4.2. Concrete Carbonation Depth Test
3. Test Results and Analysis
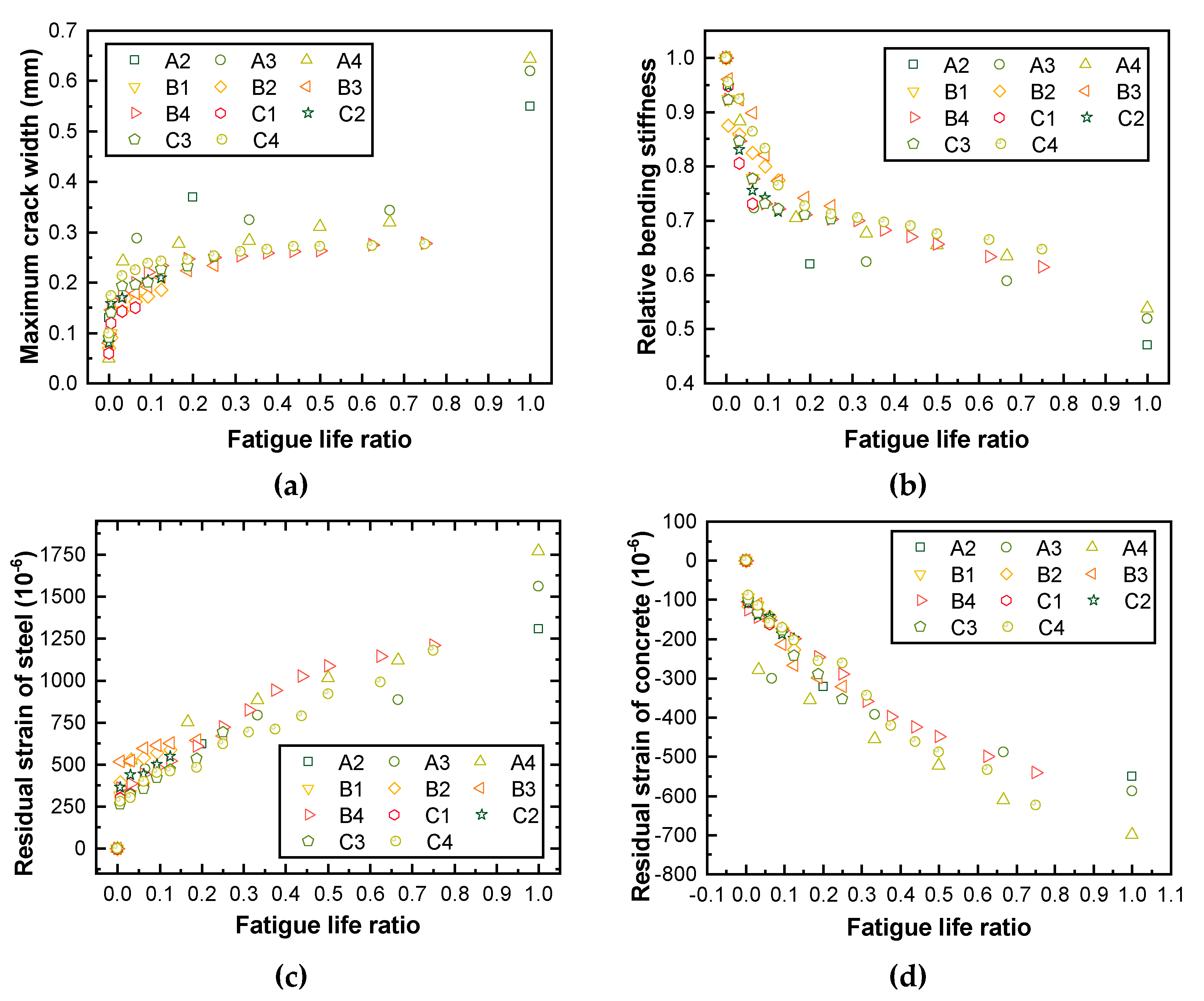
3.1. Fatigue Behavior and Index of RC Beams
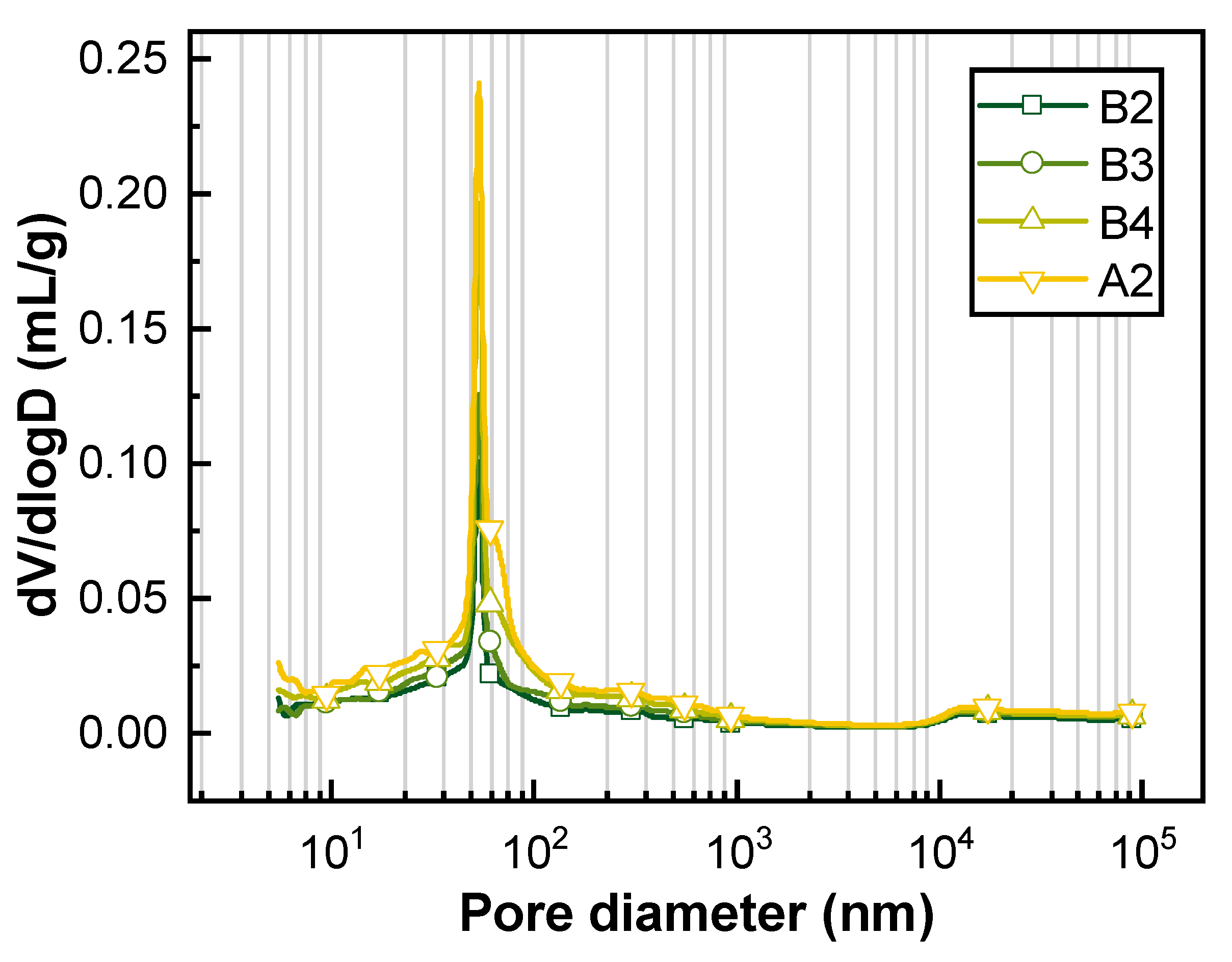
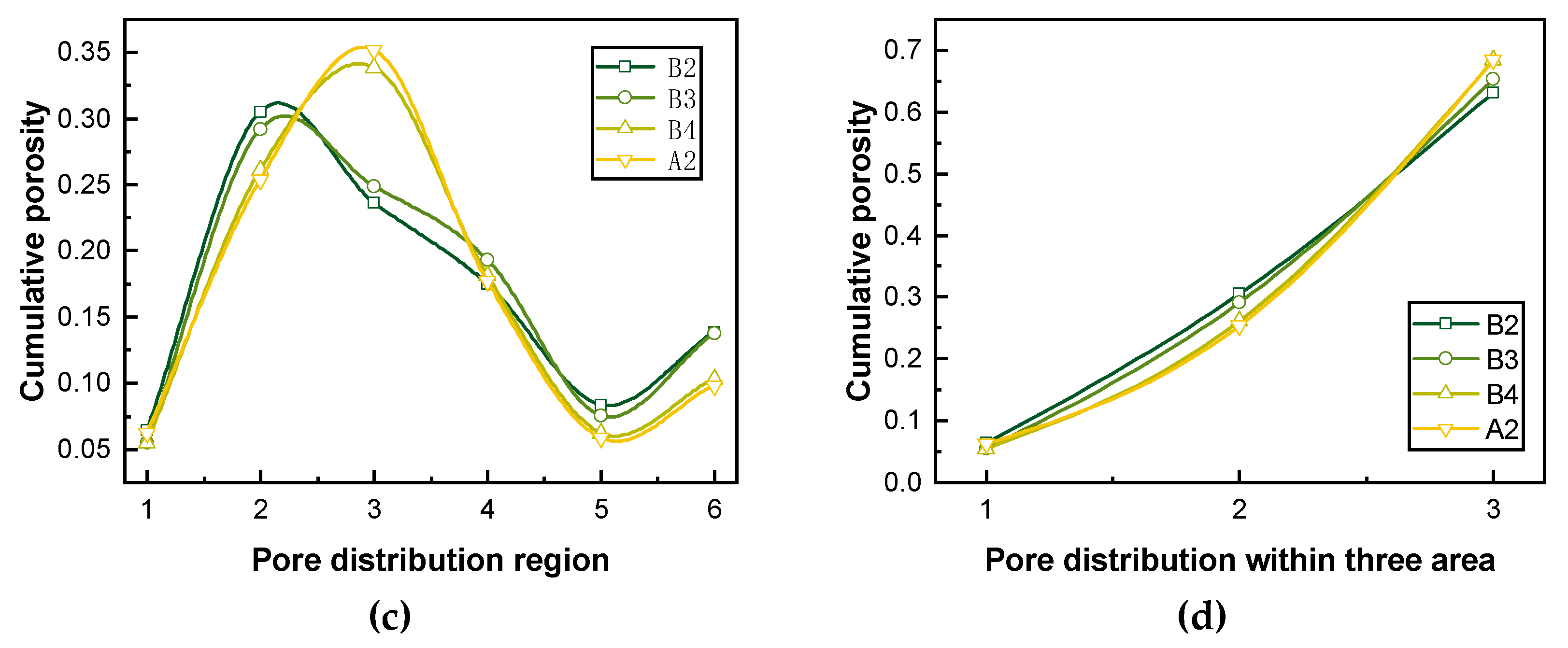
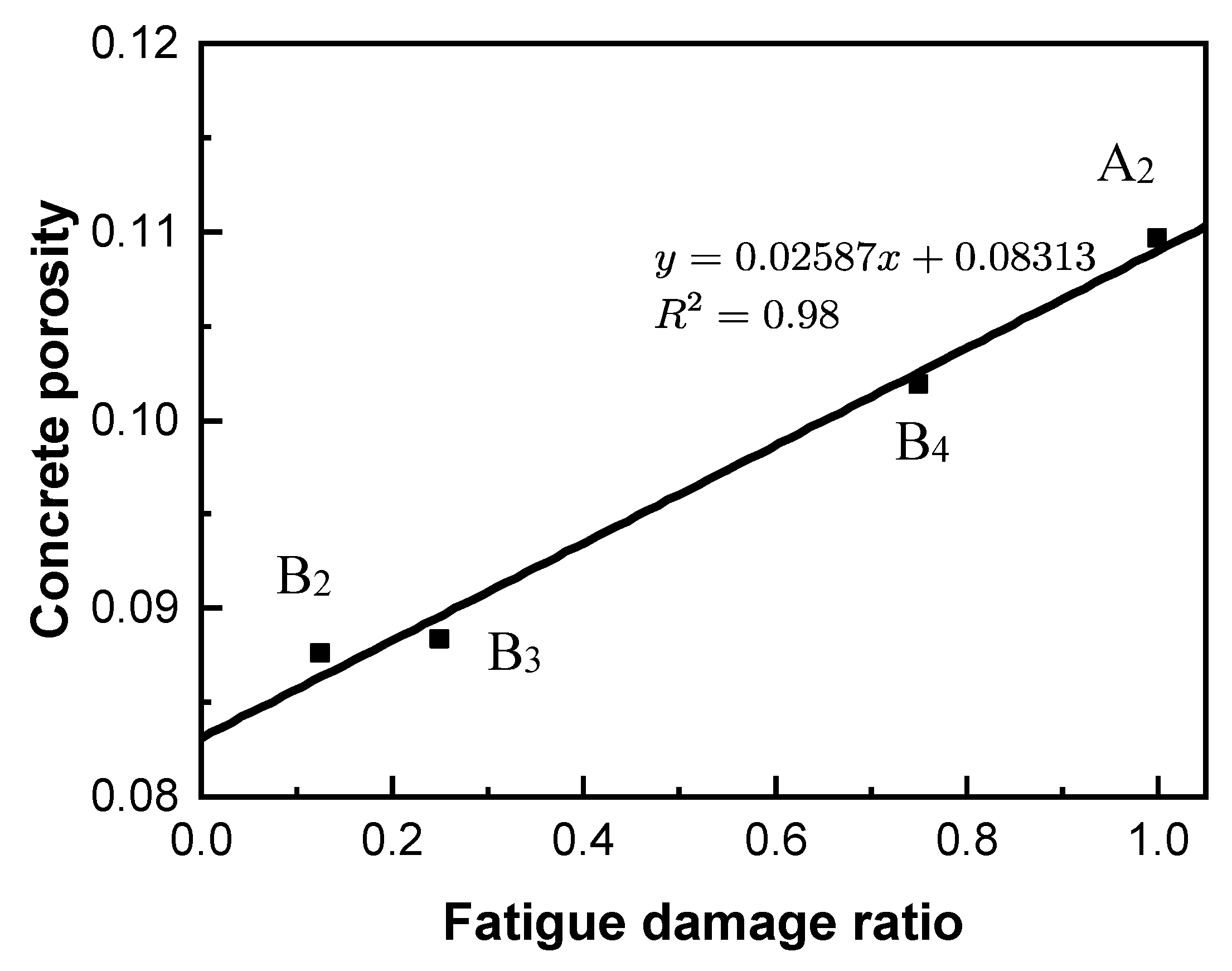
3.2. Pore Structure and Damage Index of Concrete
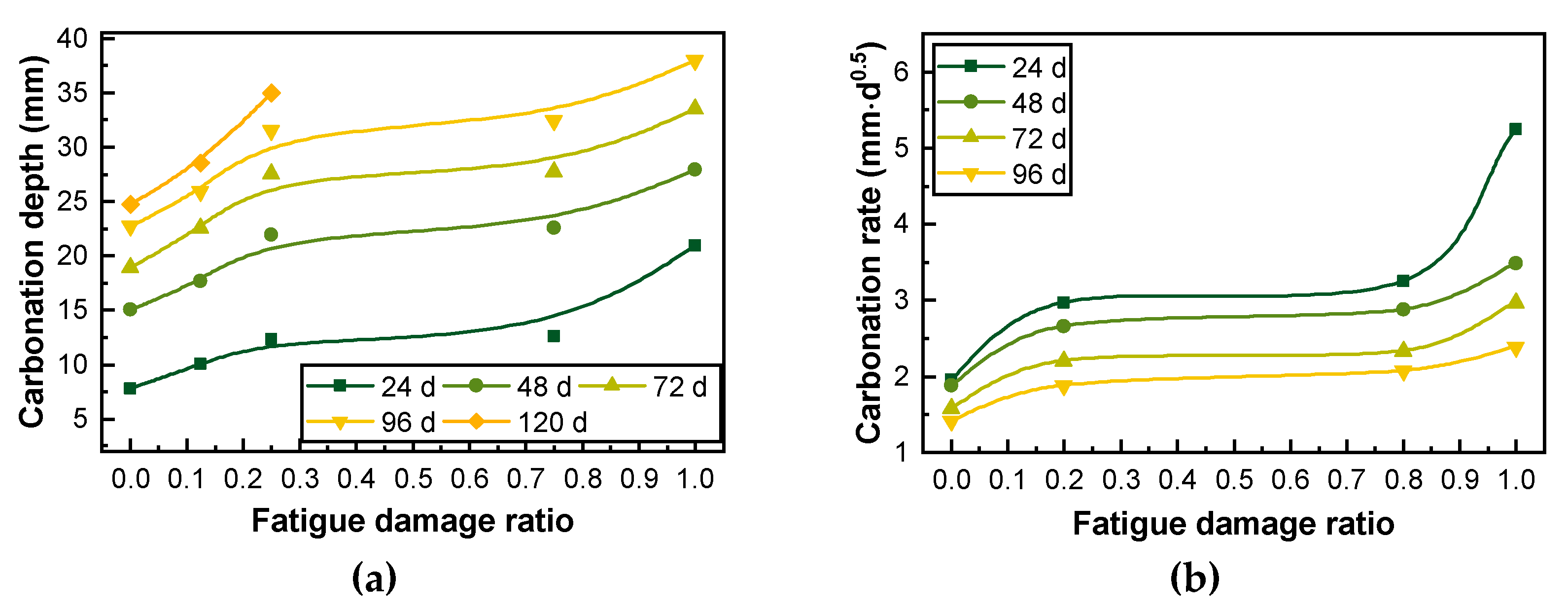
3.3. Carbonation Test of RC Beams
4. Numerical Modeling of the Carbonation Process in Fatigue-Damaged RC Beams
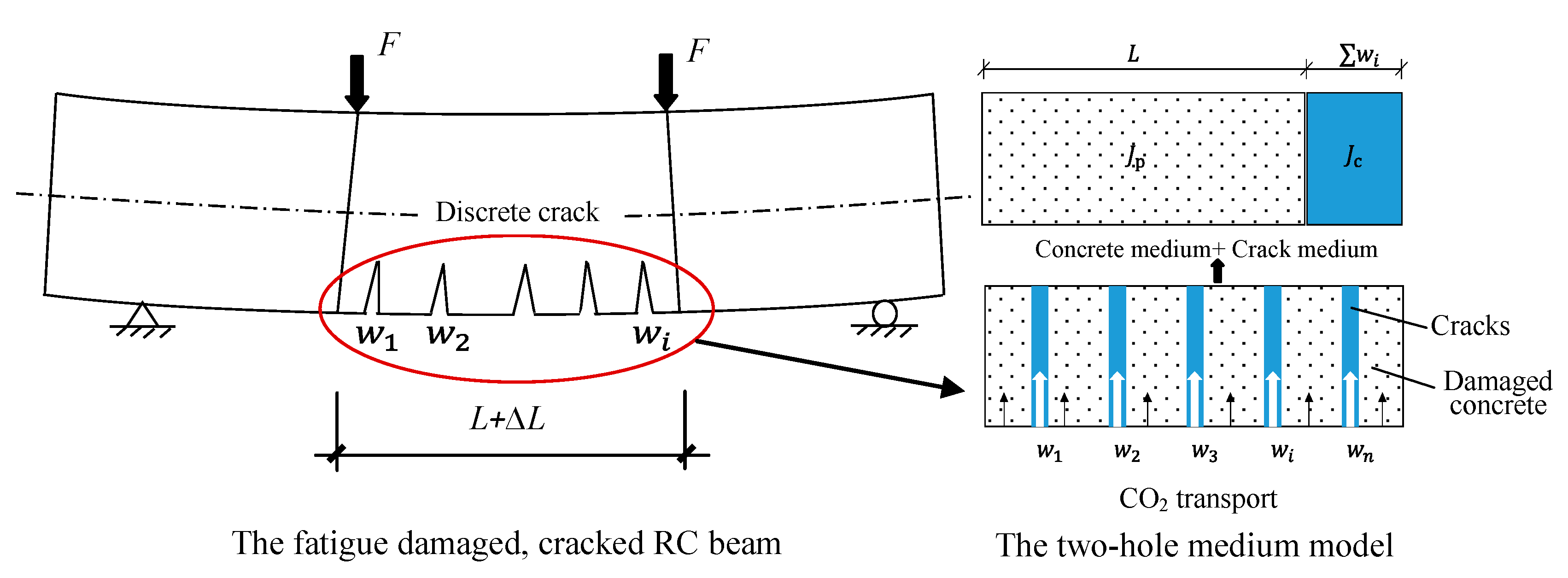
4.1. Modeling of Carbonation in Fatigue-Damaged RC Beams
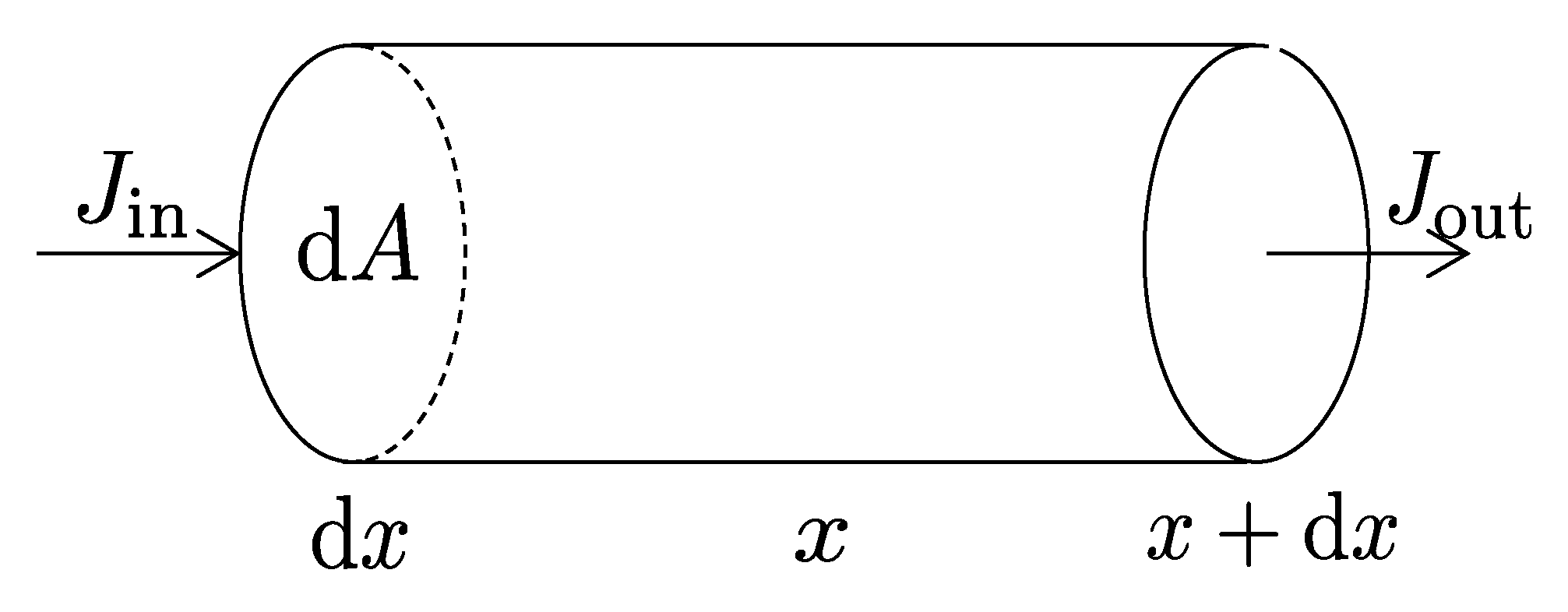
4.1.1. Effective Diffusion Coefficient of Carbon Dioxide in Fatigue-Damaged Concrete
4.1.2. Carbonation Reaction Rate of Fatigue-Damaged Concrete
4.1.3. Saturation of Concrete
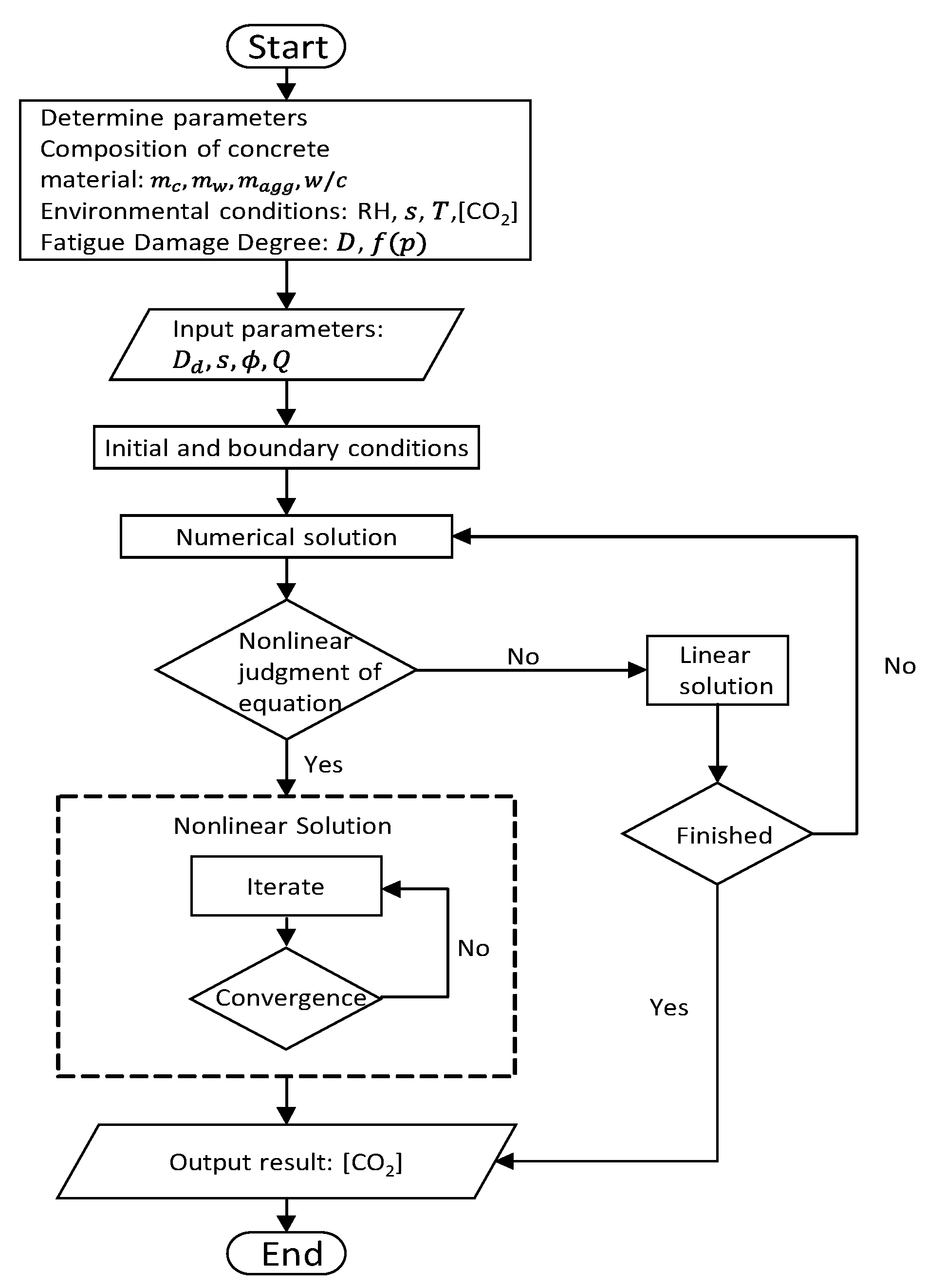
4.2. Carbonation Simulation of Fatigue-Damaged RC Beams
4.2.1. Initial and Boundary Conditions
4.2.2. Determination of the Carbonation Depth
4.2.3. Numerical Simulation of the Carbonation Process in Fatigue-Damaged RC Beams
4.3. Experimental Verification
5. Model Application
5.1. Effect of Environmental Temperature on the Carbonation of Fatigue-Damaged RC Beams
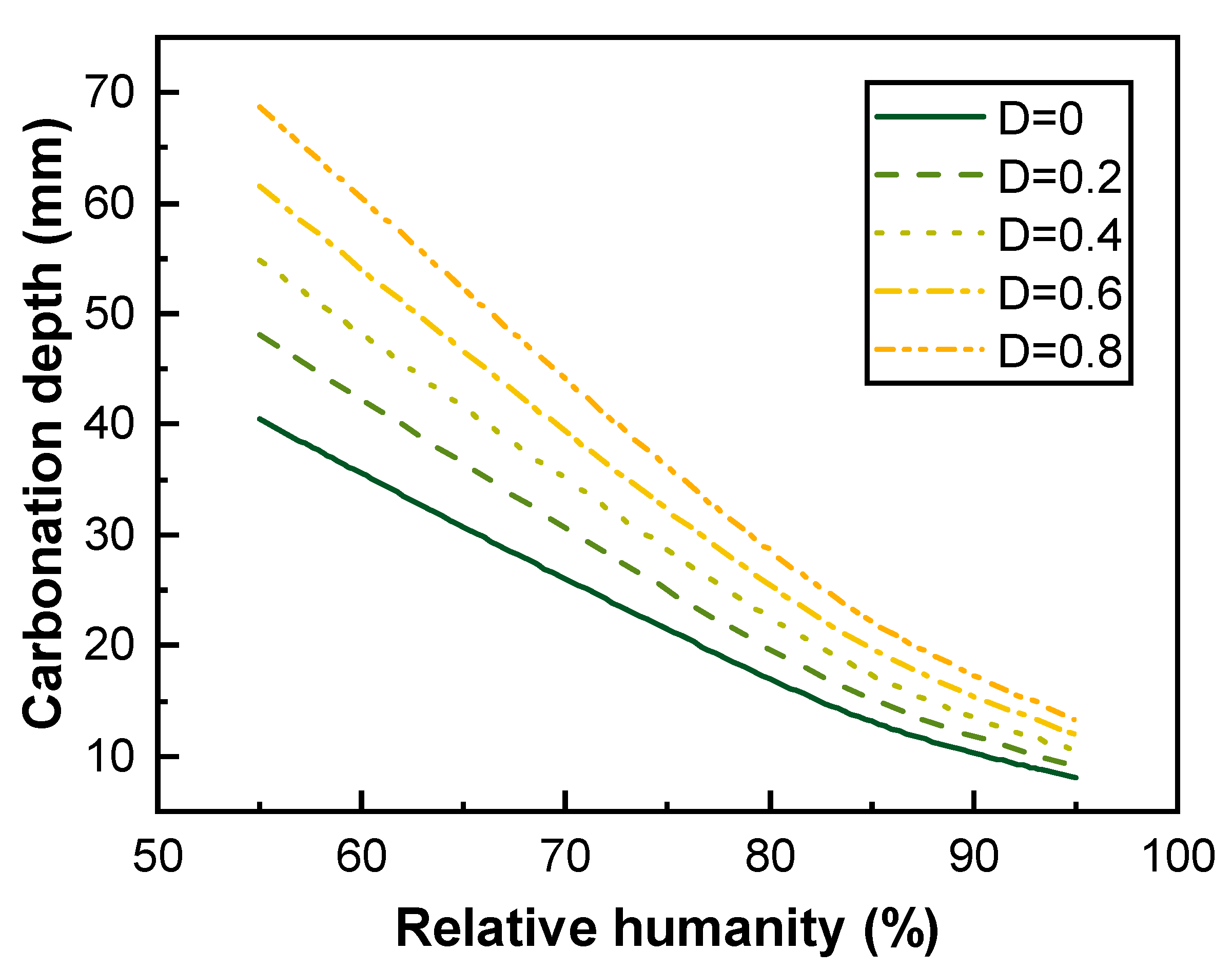
5.2. Effect of Relative Humidity on the Carbonation of Fatigue-Damaged RC Beams
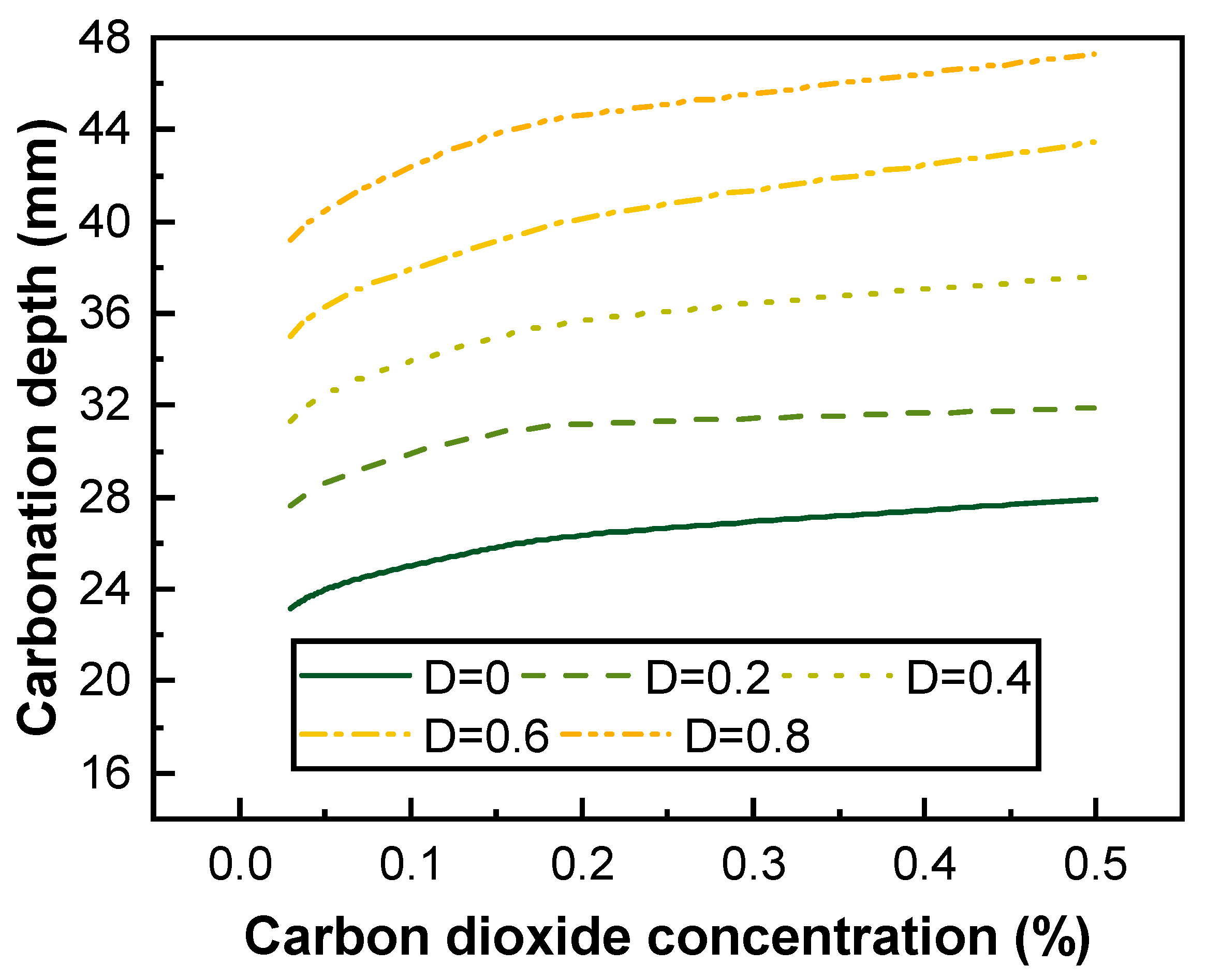
5.3. Effect of Carbon Dioxide Concentration on the Carbonation of Fatigue-Damaged RC Beams
6. Conclusions
- Compared with the macroscopic index, such as beam stiffness and material residual strain, the change in concrete pore structure can more directly and accurately characterize the effect of fatigue damage on the transport characteristics of concrete materials. The porosity of concrete notably increases with increasing fatigue damage. The distribution of pore size will change with the development of fatigue damage, among which the harmful mesopores and macropores exhibit the most notable changes. The fatigue damage has no obvious effect on the peak pore diameter of concrete; however, the fatigue damage will substantially increase the peak strength.
- Fatigue damage not only significantly increases the depth of complete carbonation but also significantly increases the depth and rate of carbonation. Both the carbonation depth and fatigue damage exhibit a three-stage development law. The depth and rate of carbonation are related to concrete pores and macroscopic cracks. In the carbonation analysis of fatigue-damaged RC beams, the changes in both the pore structure and fatigue cracks caused by repeated fatigue loading on carbonation should be considered.
- The increase in environmental temperature notably increases the carbonation depth and rate of RC beams. When the environmental relative humidity is 70%, the carbon dioxide concentration is 15%, the environmental temperature increases from 5 to 15 °C, and the samples are carbonized for 120 days, as the fatigue damage develops from 0 to 0.2, 0.4, and 0.8, the carbonation depth and rate increase by 62.25%, 85.93%, and 133.29%, respectively. When the environmental relative humidity is 70%, the carbon dioxide concentration is 15%, the environmental temperature increases from 15 to 35 °C, and the samples are carbonized for 120 days, as the fatigue damage develops from 0 to 0.2, 0.4, and 0.8, the carbonation depth and rate increase by 89.53%, 117.55%, and 172.22%, respectively.
- The increase in the environmental relative humidity significantly decreases the carbonation depth and rate of RC beams. When the environmental temperature is 25 °C, the carbon dioxide concentration is 15%, the environmental relative humidity increases from 55.0% to 65.0%, and the samples are carbonized for 120 days, as the fatigue damage develops from 0 to 0.2, 0.4, and 0.8, the carbonation depth and carbonation rate decrease by 9.97%, 2.44%, and 28.67%, respectively. When the environmental temperature is 25 °C, the carbon dioxide concentration is 15%, the environmental relative humidity increases from 65.0% to 95.0%, and the samples are carbonized for 120 days, as the fatigue damage develops from 0 to 0.2, 0.4, and 0.8, the carbonation depth and carbonation rate decrease by 70.01%, 65.37%, and 56.37%, respectively.
- The increase in carbon dioxide concentration substantially increases the carbonation depth and rate of RC beams. When the environmental temperature is 25 °C, the environmental relative humidity is 70%, the carbon dioxide concentration increases from 3% to 5%, and the samples are carbonized for 120 days, as the fatigue damage develops from 0 to 0.2, 0.4, and 0.8, the carbonation depth and rate increase by 24.76%, 41.59%, and 76.01%, respectively. When the environmental temperature is 25 °C, the environmental relative humidity is 70%, the carbon dioxide concentration increases from 5% to 20%, and the samples are carbonized for 120 days, as the fatigue damage develops from 0 to 0.2, 0.4, and 0.8, the carbonation depth and rate increase by 28.89%, 47.98%, and 84.56%, respectively.
Author Contributions
Funding
Conflicts of Interest
References
- Shamsad, A. Reinforcement corrosion in concrete structures, its monitoring and service life prediction—A review. Cem. Concr. Compos. 2003, 25, 459–471. [Google Scholar]
- Saetta, A.; Vitaliani, R.V. Experimental investigation and numerical modeling of carbonation process in reinforced concrete structures. Cem. Concr. Res. 2004, 34, 571–579. [Google Scholar] [CrossRef]
- Raupach, M. Models for the propagation phase of reinforcement corrosion—An overview. Mater. Corros. 2006, 57, 605–613. [Google Scholar] [CrossRef]
- Vu, K.A.T.; Stewart, M.G. Structural reliability of concrete bridges including improved chloride-induced corrosion models. Struct. Saf. 2000, 22, 313–333. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Hubler, M.H. Theory of cyclic creep of concrete based on Paris law for fatigue growth of subcritical microcracks. J. Mech. Phys. Solids 2014, 63, 187–200. [Google Scholar] [CrossRef]
- Chen, N.; Mahadevan, S. Chloride-induced reinforcement corrosion and concrete cracking simulation. Cem. Concr. Compos. 2008, 30, 227–238. [Google Scholar] [CrossRef]
- Vidal, T.; Castel, A.; François, R. Analyzing crack width to predict corrosion in reinforced concrete. Cem. Concr. Res. 2004, 34, 165–174. [Google Scholar] [CrossRef]
- Alonso, C.; Andrade, C.; Rodriguez, J.; Diez, J.M. Factors controlling cracking of concrete affected by reinforcement corrosion. Mater. Struct. 1998, 31, 435–441. [Google Scholar] [CrossRef]
- Ngala, V.; Page, C. Effects of carbonation on pore structure and diffusional properties of hydrated cement pastes. Cem. Concr. Res. 1997, 27, 995–1007. [Google Scholar] [CrossRef]
- Johannesson, B.; Utgenannt, P. Microstructural changes caused by carbonation of cement mortar. Cem. Concr. Res. 2001, 31, 925–931. [Google Scholar] [CrossRef]
- Younsi, A.; Turcry, P.; Aït-Mokhtar, A.; Staquet, S.; Turcry, P. Accelerated carbonation of concrete with high content of mineral additions: Effect of interactions between hydration and drying. Cem. Concr. Res. 2013, 43, 25–33. [Google Scholar] [CrossRef]
- Wang, X.-Y.; Park, K.-B. Analysis of Compressive Strength Development and Carbonation Depth of High-Volume Fly Ash Cement Pastes. ACI Mater. J. 2016, 113. [Google Scholar] [CrossRef]
- Lye, C.-Q.; Dhir, R.K.; Ghataora, G.S. Carbonation resistance of fly ash concrete. Mag. Concr. Res. 2015, 67, 1150–1178. [Google Scholar] [CrossRef]
- Isgor, O.B.; Razaqpur, A.G. Finite element modeling of coupled heat transfer, moisture transport and carbonation processes in concrete structures. Cem. Concr. Compos. 2004, 26, 57–73. [Google Scholar] [CrossRef]
- Cui, H.; Tang, W.; Liu, W.; Dong, Z.; Xing, F. Experimental study on effects of CO2 concentrations on concrete carbonation and diffusion mechanisms. Constr. Build. Mater. 2015, 93, 522–527. [Google Scholar] [CrossRef]
- Visser, J. Influence of the carbon dioxide concentration on the resistance to carbonation of concrete. Constr. Build. Mater. 2014, 67, 8–13. [Google Scholar] [CrossRef]
- Jiang, C.; Gu, X.; Zhang, W.; Zou, W. Modeling of carbonation in tensile zone of plain concrete beams damaged by cyclic loading. Constr. Build. Mater. 2015, 77, 479–488. [Google Scholar] [CrossRef]
- Jiang, C.; Huang, Q.; Gu, X.; Zhang, W. Experimental investigation on carbonation in fatigue-damaged concrete. Cem. Concr. Res. 2017, 99, 38–52. [Google Scholar] [CrossRef]
- JCI Committee 984. Long-Term Durability of Concrete; Japan Concrete Institute: Tokyo, Japan, 2000. [Google Scholar]
- Perez, N. Electrochemistry and Corrosion Science; Kluwer Academic Publishers: Boston, MA, USA, 2004. [Google Scholar]
- Papadakis, V.G.; Fardis, M.N.; Vayenas, C.G. Effect of composition, environmental factors and cement-lime mortar coating on concrete carbonation. Mater. Struct. 1992, 25, 293–304. [Google Scholar] [CrossRef]
- Song, H.-W.; Kwon, S.-J.; Byun, K.-J.; Park, C.-K. Predicting carbonation in early-aged cracked concrete. Cem. Concr. Res. 2006, 36, 979–989. [Google Scholar] [CrossRef]
- China National Standard. Code for Design of Concrete Structures (GB50010-2010); China Architecture & Building Press: Beijing, China, 2009. [Google Scholar]
- Papadakis, V.G.; Vayenas, C.G.; Fardis, M.N. A reaction engineering approach to the problem of concrete carbonation. AIChE J. 1989, 35, 1639–1650. [Google Scholar] [CrossRef]
- Papadakis, V.G.; Vayenas, C.G.; Fardis, M.N. Experimental investigation and mathematical modeling of the concrete carbonation problem. Chem. Eng. Sci. 1991, 46, 1333–1338. [Google Scholar] [CrossRef]
- Khunthongkeaw, J.; Tangtermsirikul, S. Model for Simulating Carbonation of Fly Ash Concrete. J. Mater. Civ. Eng. 2005, 17, 570–578. [Google Scholar] [CrossRef]
- Han, D.J. Damage Mechanism and Service Life Prediction of Cementitious Materials under Loading and Carbonation Coupling Effects. Ph.D. Thesis, University of Dongnan, Shanghai, China, 2008. [Google Scholar]
- Song, X.B. Corrosion of Reinforcement Bars in Reinforced Concrete Structures. Ph.D. Thesis, Tsinghua University, Beijing, China, 1999. [Google Scholar]
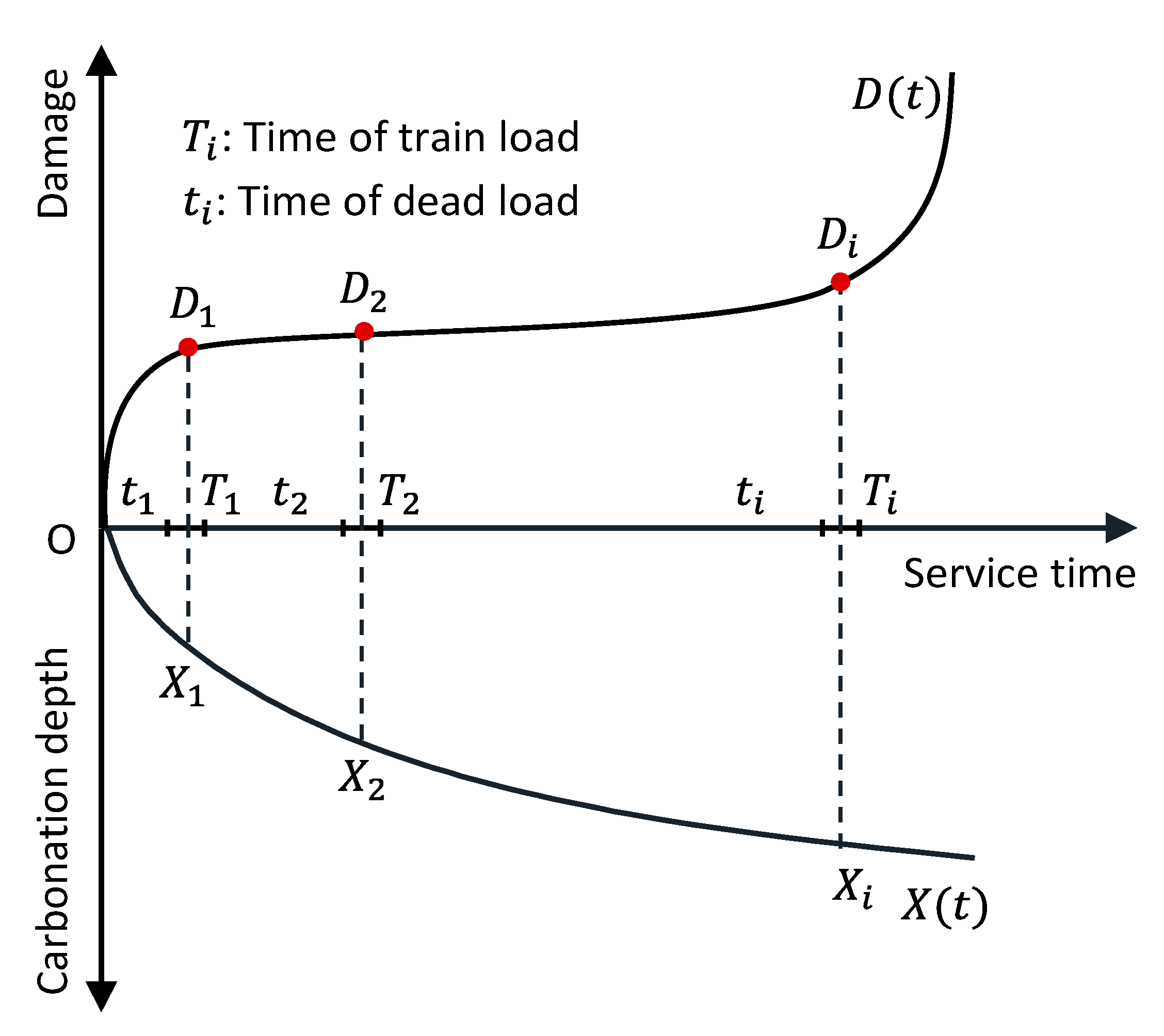
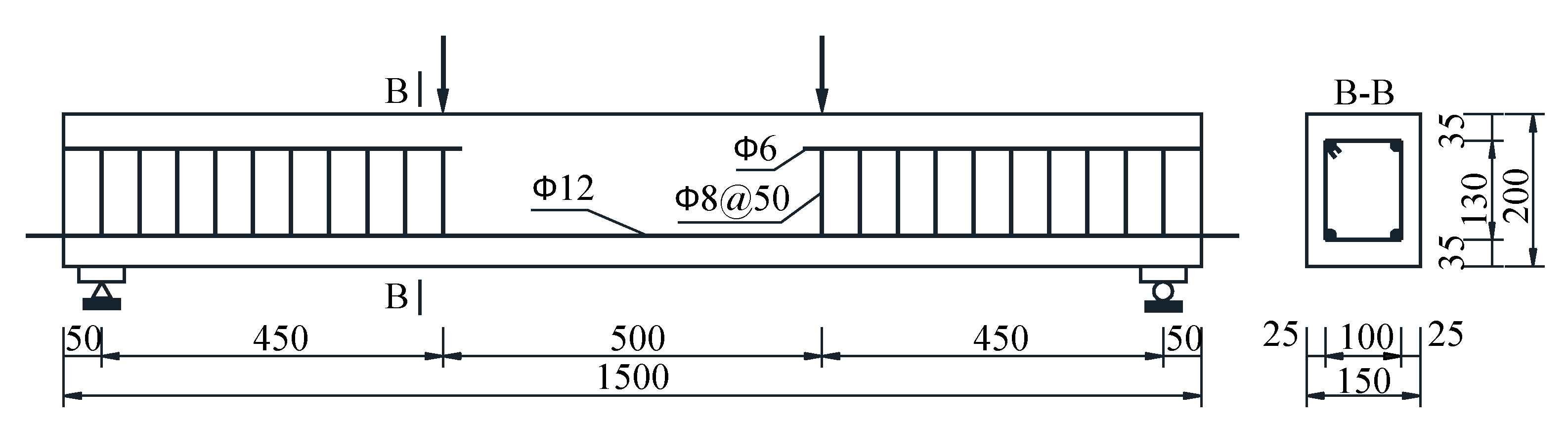

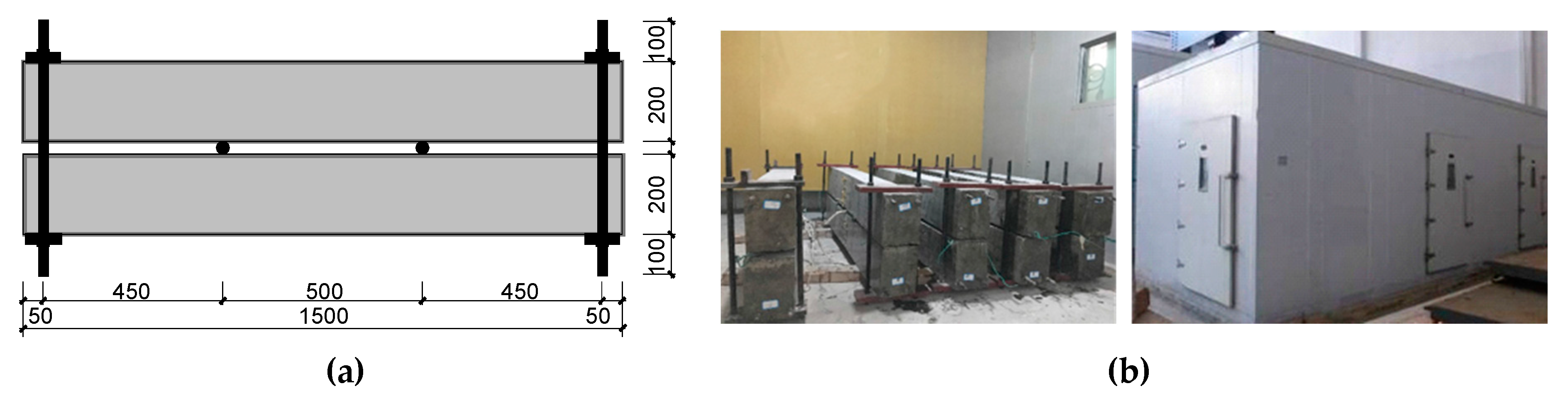
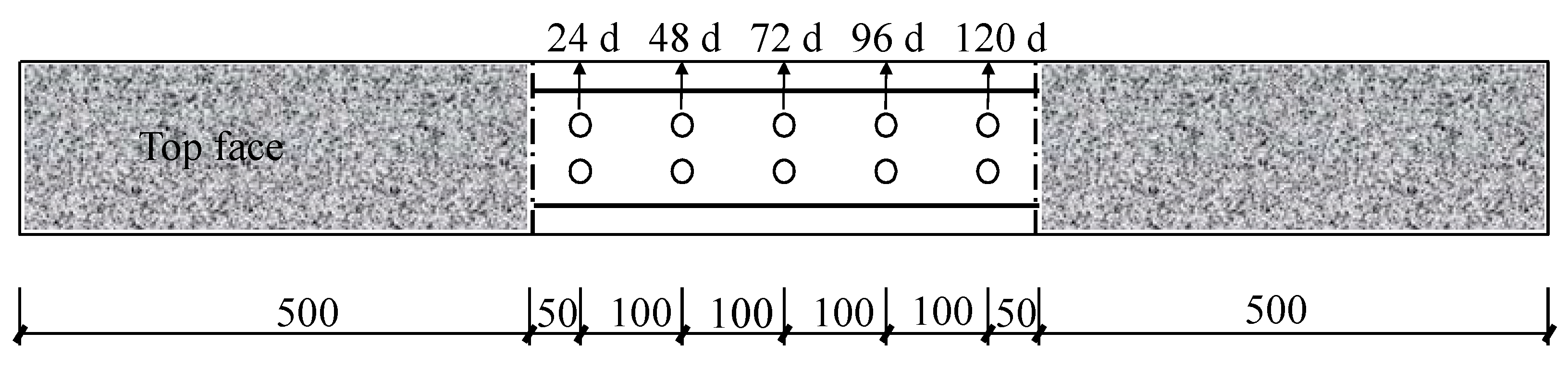





| Spec. | Procedure | Fatigue Load | Load a | Cycles (104) | Damage |
|---|---|---|---|---|---|
| Pmin/Pmax (kN) | |||||
| A1 | Static failure | - | - | - | - |
| A2 | Fatigue failure | 10/40 | 45% | 150.6 | - |
| A3 | Fatigue failure | 10/50 | 55% | 75.2 | - |
| A4 | Fatigue failure | 10/70 | 75% | 25.3 | - |
| B0 | Carbonation | 10/35 | 40% | 0 | Undamaged |
| C0 | Corrosion | ||||
| B1 | Carbonation | 10/35 | 40% | 50 | Low |
| C1 | Corrosion | ||||
| B2 | Carbonation | 10/35 | 40% | 100 | Medium |
| C2 | Corrosion | ||||
| B3 | Carbonation | 10/35 | 40% | 200 | Medium |
| C3 | Corrosion | ||||
| B4 | Carbonation | 10/35 | 40% | 600 | High |
| C4 | Corrosion |
| Mix Proportion of Concrete (kg) | ||||||
| Water | Cement | Fine Aggregate | Coarse Aggregate | w/c | - | - |
| 176 | 495 | 648 | 1152 | 0.36 | - | - |
| Mineral composition of cement (wt%) | ||||||
| Others | - | |||||
| 55.5 | 19.1 | 6.5 | 10.1 | 5.0 | 3.8 | - |
| Chemical composition of cement (wt %) | ||||||
| Others | ||||||
| 62.60 | 21.35 | 4.67 | 3.31 | 3.08 | 2.25 | 2.84 |
| Temperature Phase | Low | Middle | High | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Month | 11 | 12 | 1 | 2 | 3 | 4 | 10 | 5 | 6 | 7 | 8 | 9 |
| Temperature (°C) | 13.2 | 7.4 | 5.2 | 7.9 | 12.9 | 18.2 | 19.1 | 23 | 26.7 | 29.8 | 28.3 | 24.6 |
| Relative humidity (%) | 72.3 | 72.5 | 74.9 | 75.5 | 72.6 | 73.1 | 73.4 | 73.1 | 75.7 | 72.1 | 74.5 | 72.8 |
| CO2 concentration (ppm) | 430 | 440 | 419 | 420 | 425 | 428 | 424 | 405 | 398 | 350 | 370 | 373 |
| Average temperature (°C) | 9.3 | 18.7 | 26.5 | |||||||||
| Average RH (%) | 73.6 | 73.3 | 73.6 | |||||||||
| Cycle (Day) | Temperature (°C) | Relative Humidity (%) | CO2 Concentration (%) | Corresponding Month |
|---|---|---|---|---|
| 2.5 | 14 | 73.6 ± 1 | 15 ± 2 | 11–3 (low) |
| 1.0 | 28 | 73.3 ± 1 | 15 ± 2 | 4, 10 (medium) |
| 2.5 | 40 | 73.6 ± 1 | 15 ± 2 | 5–9 (high) |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, L.; Liu, J.-l.; Cui, C.-x.; Yu, Z.-w.; Fan, Z.-w.; Hou, J. Carbonation Process of Reinforced Concrete Beams under the Combined Effects of Fatigue Damage and Environmental Factors. Appl. Sci. 2020, 10, 3981. https://doi.org/10.3390/app10113981
Song L, Liu J-l, Cui C-x, Yu Z-w, Fan Z-w, Hou J. Carbonation Process of Reinforced Concrete Beams under the Combined Effects of Fatigue Damage and Environmental Factors. Applied Sciences. 2020; 10(11):3981. https://doi.org/10.3390/app10113981
Chicago/Turabian StyleSong, Li, Jin-liang Liu, Chen-xing Cui, Zhi-wu Yu, Zhi-wei Fan, and Jian Hou. 2020. "Carbonation Process of Reinforced Concrete Beams under the Combined Effects of Fatigue Damage and Environmental Factors" Applied Sciences 10, no. 11: 3981. https://doi.org/10.3390/app10113981
APA StyleSong, L., Liu, J.-l., Cui, C.-x., Yu, Z.-w., Fan, Z.-w., & Hou, J. (2020). Carbonation Process of Reinforced Concrete Beams under the Combined Effects of Fatigue Damage and Environmental Factors. Applied Sciences, 10(11), 3981. https://doi.org/10.3390/app10113981




