1. Introduction
Recently, aerial manipulation platforms have been demonstrated to be a very efficient solution for applications, including contact inspection in industrial environments, cooperative free-flying for assembly and contact inspection in sites that are inaccessible by conventional means (e.g., European projects ARCAS FP7 [
1] and the AEROARMS H2020 [
2]). All of these achievements have been possible thanks to the additional manipulation capabilities added to standard UAVs providing them with the ability to interact with the environment. In the current GRIFFIN European project under funding scheme ERC Advanced Grant [
3], we move a step forward in the development of aerial vehicles with manipulation capabilities, moving from multirotor platforms to bio-inspired aerial locomotion ones with flapping wings [
4]. Thus, although electric-powered multirotor platforms have been demonstrated to be very efficient for manipulation, unfortunately they still suffer some technology deficiencies e.g., the well-known relatively short flight missions of about few minutes and other inherent limitations when interacting within the proximity of people like noise and hazard situations. Moreover, multirotor platforms have important limitations for application in potentially explosive environments such as oil and gas plants.
A more energy efficient alternative to rotor propellers is the use of wings [
5], as such, animal flapping flight has attracted an enormous interest in the last few years [
6,
7]. The animal-like aerial locomotion exhibits two clear different modes: (1) powered while flapping and (2) unpowered while gliding or soaring. In unpowered modes, birds use the aerodynamic lift force of their wings in order to remain in the air and save energy, allowing them to travel long distances with a very small energy demand. In powered mode, birds use wings to generate lift and thrust forces while flapping, allowing them to accelerate or even decelerate to perch in difficult to reach places, offering them important advantages in terms of safety and accessibility. While airplanes require runways and most aerial robots need flat landing areas, arboreal birds consistently land on perches with a wide range of different geometries and surfaces, from electric lines to branches of trees.
Perching helps small aerial robots to stay at rest, extending their autonomy by saving battery power. Recent advances in perching capabilities of aerial robots have improved their landing ability on engineered surfaces. In [
8], a new framework is proposed to perform UAV perching and resting on a set of common structures and in [
9], a new control technique for a perching maneuver of an aerial robot inspired by birds is proposed. Yet, few studies have focused on how they adapt to different surfaces once the feet had made contact with the surface, or how their toes and claws adapt to different places and even use them to balance while performing some tasks.
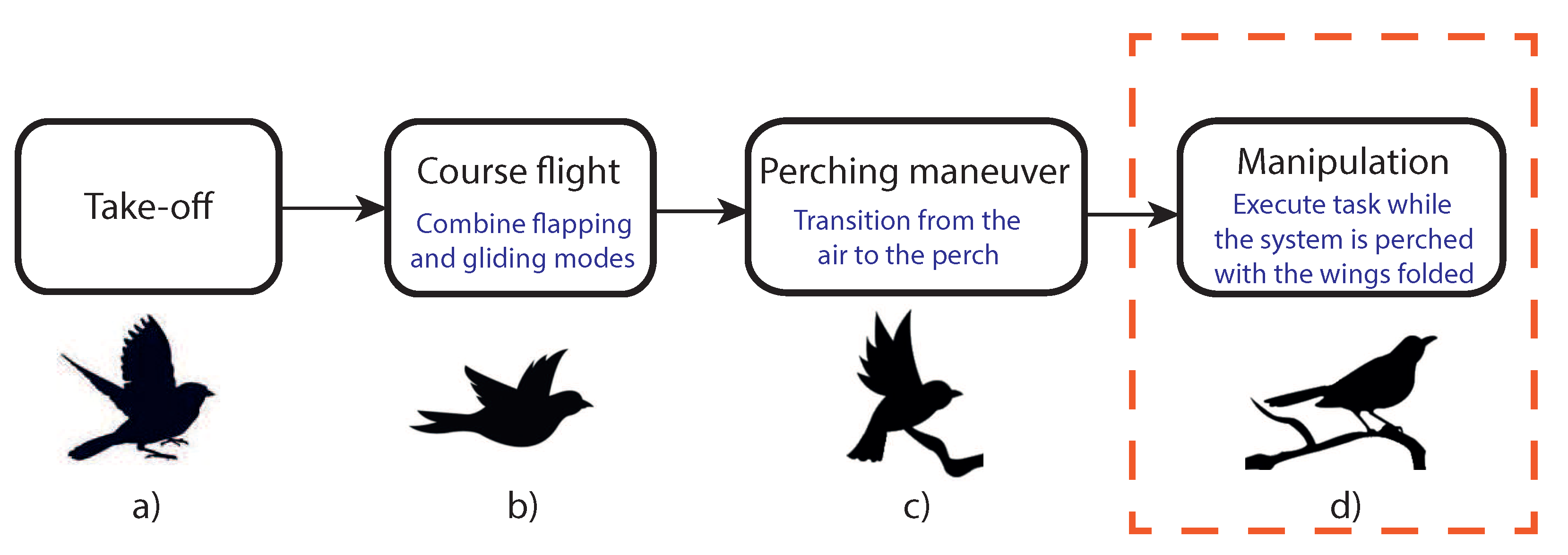
Figure 1 shows the main phases of flapping wing aerial robots carrying out a mission. Our current GRIFFIN European project covers all these phases, for example, previous results have been recently published about the control of perching in [
10] and the development of a bio-inspired claw for grasping and perching in [
11]. The limited payload capacity of the flapping-wing platforms motivated the design of a small-scale compliant dual arm [
12] whose weight is one order of magnitude lower compared to the arms typically integrated in multirotor platforms. Later, we introduced the concept of winged aerial manipulation robot [
4] with the aim to reduce the total weight, using the robotic arms with a double functionality, for manipulating and as flapping wings. Each phase is being investigated separately due to the novelty of aerial robots with flapping wings. This work focuses on the development of a first prototype of a lightweight manipulator to be used as ornithopter limbs and explore its control possibilities (Phase (d) of
Figure 1). The objective is to maintain the equilibrium while the system performs some tasks such as contact inspection in sites inaccessible by conventional means such as oil and gas refineries (see
Figure 2a). We consider the case of the robot with the body perched and the wings folded, which implies that the aerodynamic effects of the body vibration induced by the wing flapping do not need to be considered. To do that, it is very important to understand how birds maintain the equilibrium. Unlike standard manipulators, the most important requirement is being lightweight, which limits the frame size and torques and hence the use of micro motors. Under these hard constraints, their control becomes very challenging with two main objectives: to balance the body while the system is perched; to follow trajectories with the end effector of the manipulator in order to perform some kind of task.
Moreover, in this work we focus on an underactuated manipulator prototype, i.e., fewer control inputs than degrees of freedom, because it is not actuated at the point of perching (passive joint). Underactuated mechanical systems are well known and their inherent difficulty of a lack of actuation have made them a challenge for the control community. In particular, the current system has a similar behavior to the acrobot [
13]. This system is a two-link underactuated robot that mimics the human acrobat who hangs from a bar and tries to balance his/her body. The control of n-link underactuated robot with passive first joint has been studied recently as in e.g., [
14,
15,
16,
17]. Moreover, very few researchers have addressed the control of n-link underactuated robot with the influence of a static friction on the unactuated passive joint [
18,
19] due to the great difficulty of controlling these systems with a static friction in the passive joint. Two main control objectives have been studied with these kind of systems: (1) the stabilization of the system around an equilibrium point and (2) the swing-up problem.
Unlike these standard underactuated manipulators, in this work we demonstrate that the use of a grabbing mechanism at its base imitating a bird’s claw allows us to simplify the controller design by combining the friction exerted by the mechanism and an adequate control of the system posture, and, more importantly, it allows us to follow trajectories with the end effector of the manipulator while balancing the system, which will be necessary for performing manipulation tasks such as the contact inspection following trajectories with an ultrasonic sensor mounted in the end-effector. In this work, a simple mechanism was assembled to exert an equivalent friction torque at the base, which was used to verify the effectiveness of the proposed control methodology. In parallel, a bio-inspired claw was developed in our lab for the final prototype.
The main contributions of this work are: the mechanical design of a manipulator imitating the skeleton of a bird with lightweight micro motors and, a novel bio-inspired control methodology that accounts for the static friction in the first passive joint (claw) for a precise control of the posture of the system. Moreover, this work addresses, for the first time, the control of an n-link manipulator with underactuation and static friction at its first passive joint in order to follow a path with the end-effector (beak).
Both the design of the manipulator and the control approach are bio-inspired and based on the behaviors of bird species. Our results show that this framework opens the possibility to perform manipulation, such as performing contact inspection when the system is perched, and the early prototype developed successfully mimics the anatomy of birds and allows us to verify the efficiency of the proposed control strategy and, therefore, it is a good starting point for future developments. Finally,
Table 1 summarizes the main differences of the previously mentioned works and ours, where we show that our work is the only work that has proposed a methodology to perform trajectory tracking with the end-effector of an n-link underactuated robot with the first passive joint.
The article is organized as follows.
Section 2 presents the description of the system and the actual manipulator prototype is described.
Section 3 describes the mathematical modeling of the system.
Section 4 describes the proposed control strategy. The experimental results are reported in
Section 5. Finally, the conclusions are given in
Section 6.
Notation: For any vector , and stands for its Jacobian.
3. Mathematical Modeling of the System
To simplify, let us start with the derivation of the dynamics of an
n-link under actuated manipulator. Thus, let
∈
denote the vector of generalized coordinates for the joint angles, and
∈
the vector of inputs and generalized torques. Hence the Lagrange dynamic equations read
where
∈
is a symmetric positive definite inertia matrix;
∈
is the gyroscopic and Coriolis matrix;
∈
is the vector of gravitational terms. To complete the dynamics we add the nonlinear external torque exerted by the claw at the base, i.e.,
. To this end, let us split the dynamics (
1) into passive (claw) and active (control) subsystems as follows
where we have defined
,
in a similar way,
and
stands for the external friction torque modeling the claw of a bird (see
Figure 2c). Now, from the first equation of (
2), let us denote the resulting dynamic torque at the base
whenever
as
. Thus, as described in
Section 2.3, the grasping torque provided by the claw, namely
, can be modeled as a static friction—so called stiction—as follows
where
is its maximum value of the grasping torque. Notice that, if
does not surpass
, the angular coordinate of the first joint remains unchanged, i.e.,
,
. More information about friction models see [
23].
Remark 1. Notice that the grasping torque of the claw in (3) strongly modifies the dynamics when compared with a frictionless underactuated manipulator with a passive joint at its base, as it can be seen e.g., [15,16,24]. The static friction, so-called stiction
, induces a highly nonlinear behavior around , constant. Finally, the Cartesian position (
,
) of the end effector and joint coordinates are related through the geometric constraint, with respect to the reference frame
defined in
Figure 2c, and it reads
4. Nonlinear Control Design
In this section we propose a new control methodology for the manipulation of ornithopters while they are perched. Roughly speaking, it aims to track a desired trajectory for the end effector (beak) of the underactuated manipulator while the passive joint (claw) maintains the equilibrium. The proposed control methodology is stated for an
n-link manipulator with the first joint passive by means of a grabbing mechanism. For experimental validation the prototype had
as described in
Section 2.2.
The proposed control strategy relies on the fact that the grasping torque the claws can exert is limited and unknown a priori because it depends on both contact surfaces. Furthermore, since the pose of the whole system depends on the balance of torques at the passive joint, the control strategy accounts for both the limited grasping torque and the system posture. Thus, the condition to maintain the passive joint at equilibrium can be obtained directly from (
2) and (
3) as
and
. To simplify the control problem, we know that at slow speed the inertial and centripetal terms in (
2) are very small in comparison with gravity ones, and the previous equilibrium condition is well approximated by
where the function
accounts for the resultant torque at the passive joint due to gravity and
constant parameters. Even with that simplification, the control of this robot is challenging because the grasping torque is unknown and its accurate estimation is not available for feedback.
The controller must, therefore, adequately perform for different values of grasping torque, which means to control the position of the system while the base is at equilibrium. To this end, we propose to minimize the resultant torque at the passive joint, for any initial angle , through the first active joint and achieve a nearly perfect tracking in the position of the remaining active joints . This can be mathematically formulated as follows.
Control problem statement: Consider the input (
) to output (
) nonlinear control system given by
with
,
. The control objective is to guarantee the asymptotic output tracking of a
smooth bounded desired output
with bounded
and
for
.
Remark 2. Notice that, in (7) even though is measured, it is not defined as a direct output. However, its desired behavior is indirectly defined through the output . Setting minimizes the gravitational forces at the passive link and eventually maintains this joint at equilibrium by enforcing of (5). To control the system (
6) and (
7) we used feedback linearization. The gist of this control technique is to cancel out all the nonlinearities so that the output dynamics become linear. In essence it transforms the original system into an equivalent linear form by change of coordinates and feedback. Thus, since the whole state
is measurable, the system can be linearized by means of a fictitious control input
.
To facilitate the understanding we split the controller design in an inner and outer loop. The inner loop is in charge of linearizing the actuated dynamics. Thus, from (
6) we have
with
the fictitious input, resulting in the following
On the other hand, the outer loop is in charge of calculating the fictitious input
as a function of the outputs
. For, the output dynamics of
become
where
and whose components read
Therefore, together with (
8) the output dynamics become
where
is the identity matrix. These dynamics represent an explicit relationship between
and
. By defining
,
, the linear output dynamics become
Finally, we enforce the closed-loop Hurwitz,
, with
where
. To sump up, the controller designed reads
The controller (
12) cancels out all the nonlinearities of the system and assures that the tracking error of the outputs of the closed-loop system converges to zero exponentially while keeping the whole state bounded. In
Figure 7, we depict the block diagram of the control architecture.
The stability result is summarized in the proposition below.
Proposition 1. Consider the input–output nonlinear system (6) and (7) and a desired output trajectory , , such that defined through (9) is away from zero. Then, the smooth
static-state feedback (12), with , ensures that the origin of the output error is locally exponentially stable in , for any initial condition inside the set Proof. First, any initial condition in
implies that the passive link is at equilibrium at
. Secondly, the condition
guarantees the existence of the static-state feedback (
12) that forces an equivalent dynamics between
and
, from (
8) to (
10). Thus, defining
, the closed-loop output linear dynamics become
with
which is Hurwitz for any
. Thus, the latter guarantees exponential convergence and hence the closed-loop output error can be confined in a ball
such that the condition
holds for
, concluding the proof. □
5. Experimental Results
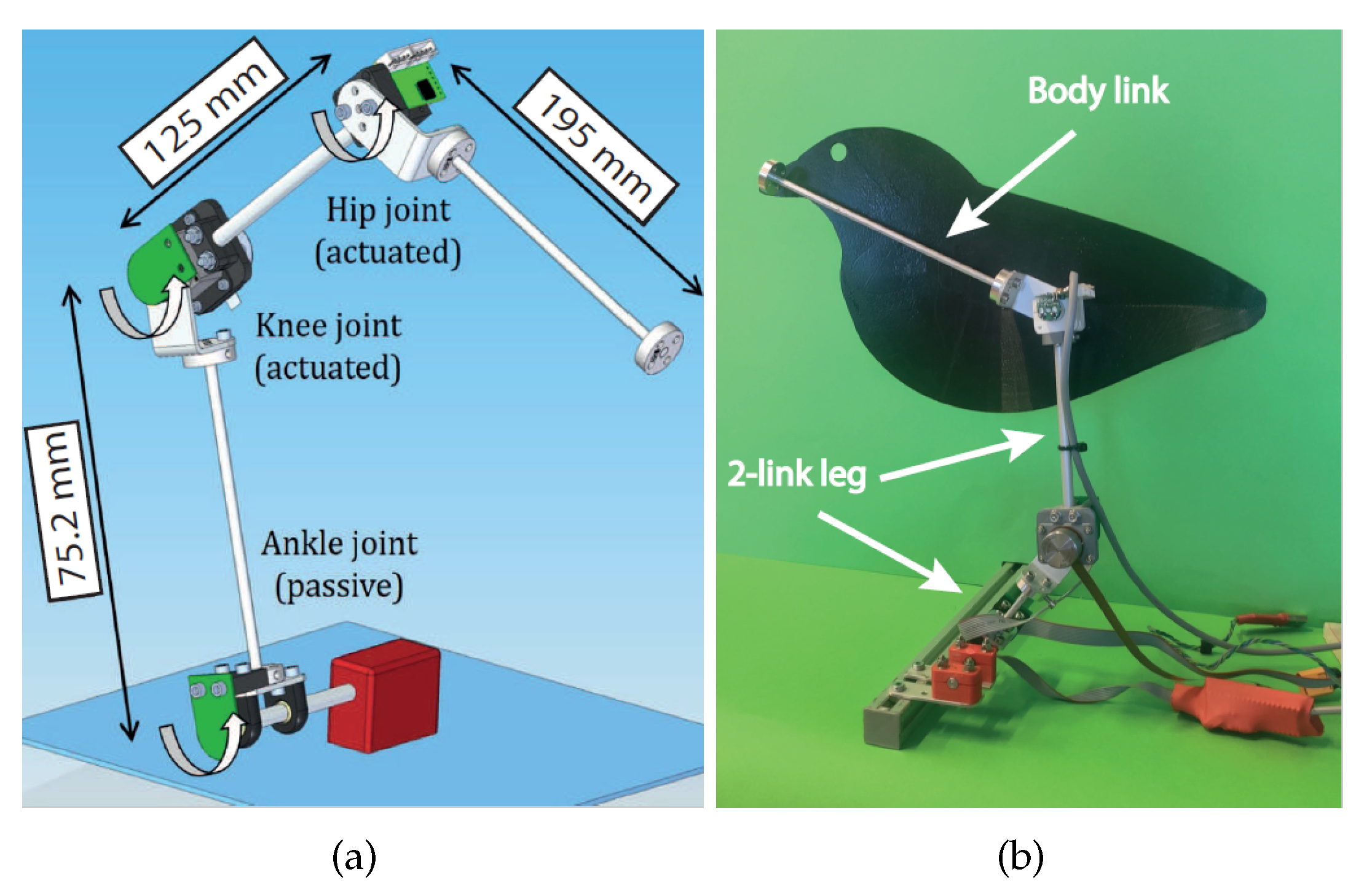
Experiments were carried out with the prototype described in the
Section 2.2 in order to demonstrate the efficiency and robustness of the proposed technique. It is important to highlight that the proposed control strategy is valid for a system with
n links,
. Our prototype has three links and two actuators, with one passive joint, which is the ankle joint, and two active joints actuated by micro motors, which are the knee and hip joints (see
Figure 3). The parameters of the mathematical model for our prototype are in
Table 3, where the components of the matrices can be found in [
24]. Notice that the masses
are much higher than the
. The controller design parameters of (
12) were specified to place the poles of the system in the real axis and sufficiently far from the imaginary axis with
and
(
). For the experiment, the passive joint angle was fixed to
. The third joint of the prototype acts like the beak (see
Figure 3), which has to follow a desired path with measurements taken at different target points. A grasping torque with the value
= 0.015 Nm (position of the screw Δ
x = 0.5 mm from
Table 4) was exerted at the base. A large trajectory was designed to cover a wide range of the workspace, assuring that
, which means that the matrix
is full rank and the control law (
12) can be implemented. The desired trajectory of the end effector of the manipulator is defined by
and, the desired trajectory
imposed by the control objective
, with
. The desired trajectory,
is defined by Bezier curves, which are smooth curves that can be differentiated indefinitely where the position and time of the trajectory is defined in
Table 5.
Figure 8 shows the angular coordinates of the passive joint, of the second joint (active) to maintain the equilibrium at the passive joint and of the third joint (active) together with its reference. Notice that the controller achieves an almost perfect tracking positioning in the third joint while maintaining the equilibrium at the first one. In essence, the controller balances the resultant torque at the second joint.
In
Figure 9 we show the torque of the motors during the movement. The saturation torque of the motors is
Nm, and therefore, they are far from saturating along the experiment, which is essential in this bio-inspired application.
Recall that the resultant torque at the passive joint was defined as
. Let us define the inertial and centripetal terms in this joint as
, so that
. Thus, in
Figure 10a we show a new experiment following the trajectory
defined in
Table 5 but with the second joint locked instead of using the proposed controller. The points where the passive joint would have collapsed and the system would have fallen down if the grasping torque was not increased are depicted with circles. This experiment demonstrates that: (1) inertial and centripetal terms are very small in comparison with the gravity ones, and hence corroborating our approximation (
5) of
implying that
; (2) without control we have to increase the grasping torque to be able of maintaining the equilibrium at the passive joint (at least
Nm which correspond to
= 1 mm). On the other hand, in
Figure 10b, we show the performance of the proposed controller along the same trajectory of the initial experiment. It is clear that the controller is able to minimize the gravity term
up to the same size of
, and therefore, guaranteeing
while the posture is maintained.
Finally, in
Figure 11 we show the complete movement of the system in Cartesian coordinates along the time (blue line) using the proposed controller.
The sketch of the manipulator at
is included to have a better idea of the posture of the system during the movement. The desired trajectory that the end effector (beak) has to follow is also depicted (red line). In
Figure 12 the different desired positions of
Table 5 was enumerated in order to show the positions of the entire structures along the experiment. We can see that a good trajectory tracking with the end effector is achieved while the system maintains the equilibrium. The maximum error is around 8 mm during the transitions, however, when the system finished these transitions, the error is less than 2 mm. These errors are negligible in comparison with the size of the manipulator (length of the manipulator is around 400 mm). In summary, these experiments validate the proposed controller for this bio-inspired prototype with small-torque motors opening the possibility to perform trajectory tracking with the end effector of underactuated manipulators which has promising applications, such as performing contact inspection.
6. Conclusions
In this study, we investigated the control possibilities of adding manipulation capabilities to ornithopters. Manipulation with ornithopters is of the utmost interest in several applications due to their promising advantages respect to multirotor platforms. The methodology proposed in this work provides a simple alternative design framework to control these systems. Although our prototype has three links, the proposed methodology is generalizable for manipulators with more links. The control law is designed to minimize the position deviation of the end effector of the manipulator from the nominal path. The control problem is split into two: the leg subsystem (underactuated), composed of the two first links to maintain the equilibrium and the remaining links of the system, which are fully actuated to follow a desired trajectory with the end effector of the manipulator.
The control scheme is based on input–output feedback linearization proposing a novel bio-inspired output. Another contribution is the first prototype of lightweight manipulator to be mounted in ornithopters. The manipulator imitates the birds skeleton, which has two-link legs and the body link. This prototype has a lot to move forward, however, it allows us to verify the efficiency of the developed control methodology. Successful experimental results demonstrate the validity of the approach.
Regarding the actuation system, more complex control systems could have been devised with different actuators. However, the weight of the motors would have increased, violating the aforementioned critical requirement for aerial robots, namely its minimum weight and energy consumption.
Currently, work is underway to design an ultra-lightweight version of the manipulator (including a bio-inspired claw) and the necessary mechanisms to mount it in a real ornithopter.