Experimental Study on Aerodynamic Characteristics of a Gurney Flap on a Wind Turbine Airfoil under High Turbulent Flow Condition
Abstract
:1. Introduction
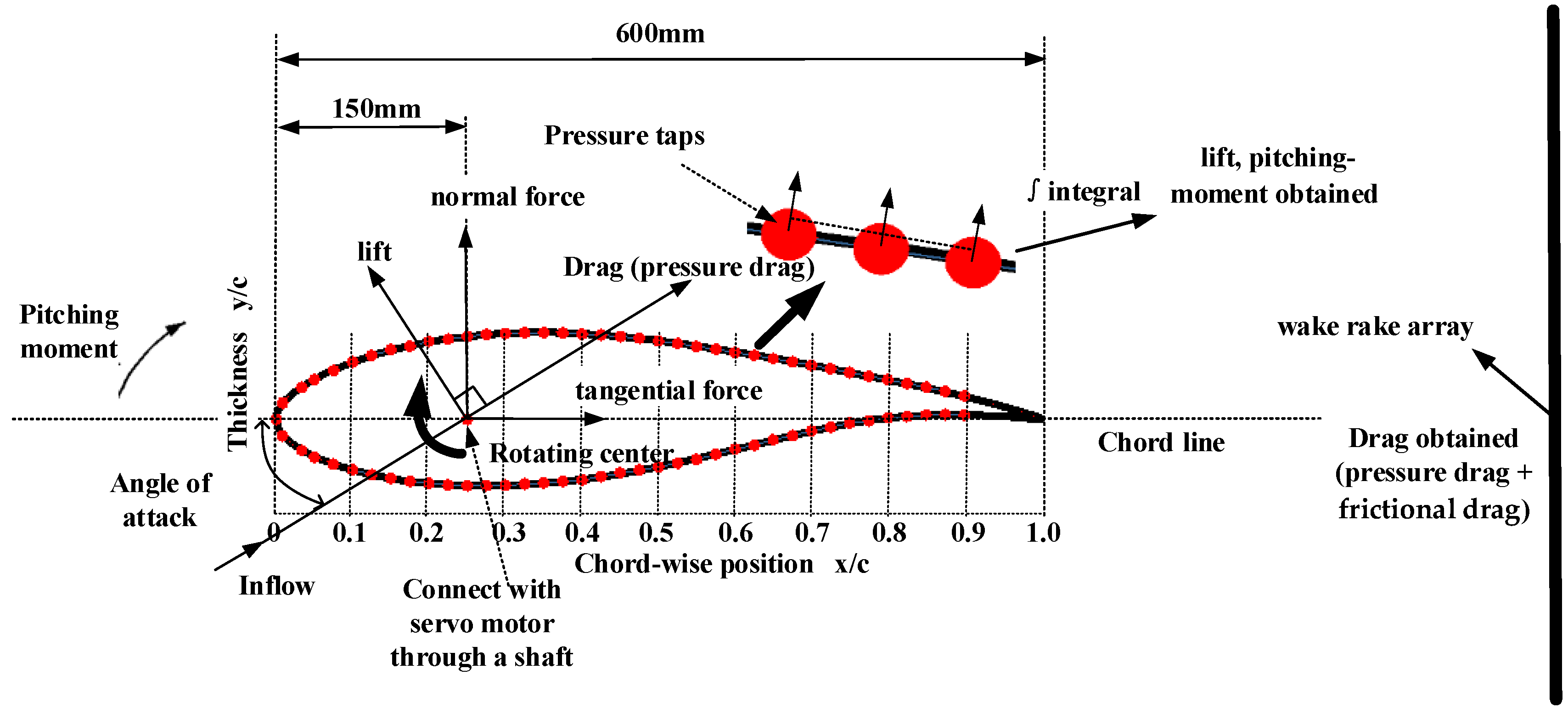
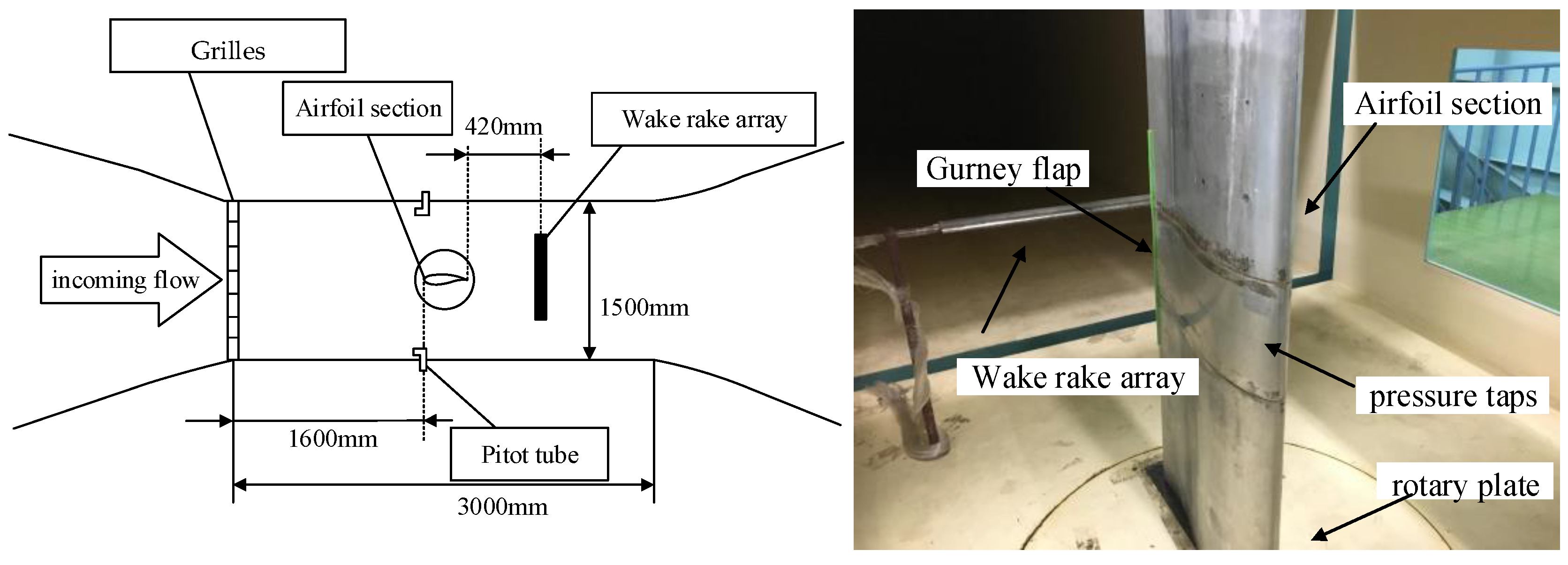
2. Experimental Setup
3. Experimental Results and Analysis
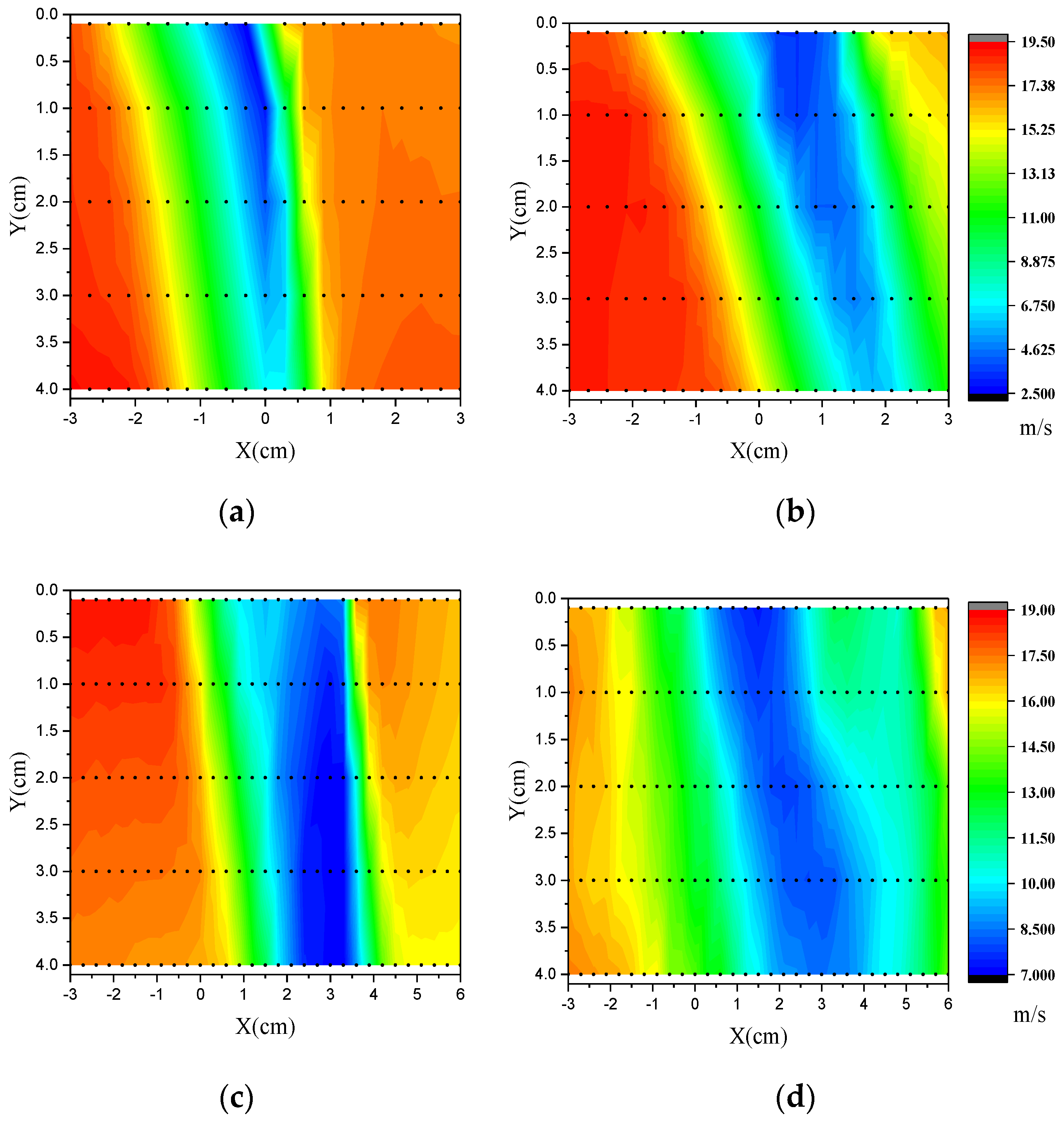
3.1. Experimental Results of Grille Turbulence Generator
3.2. Experimental Results of the Baseline Airfoil
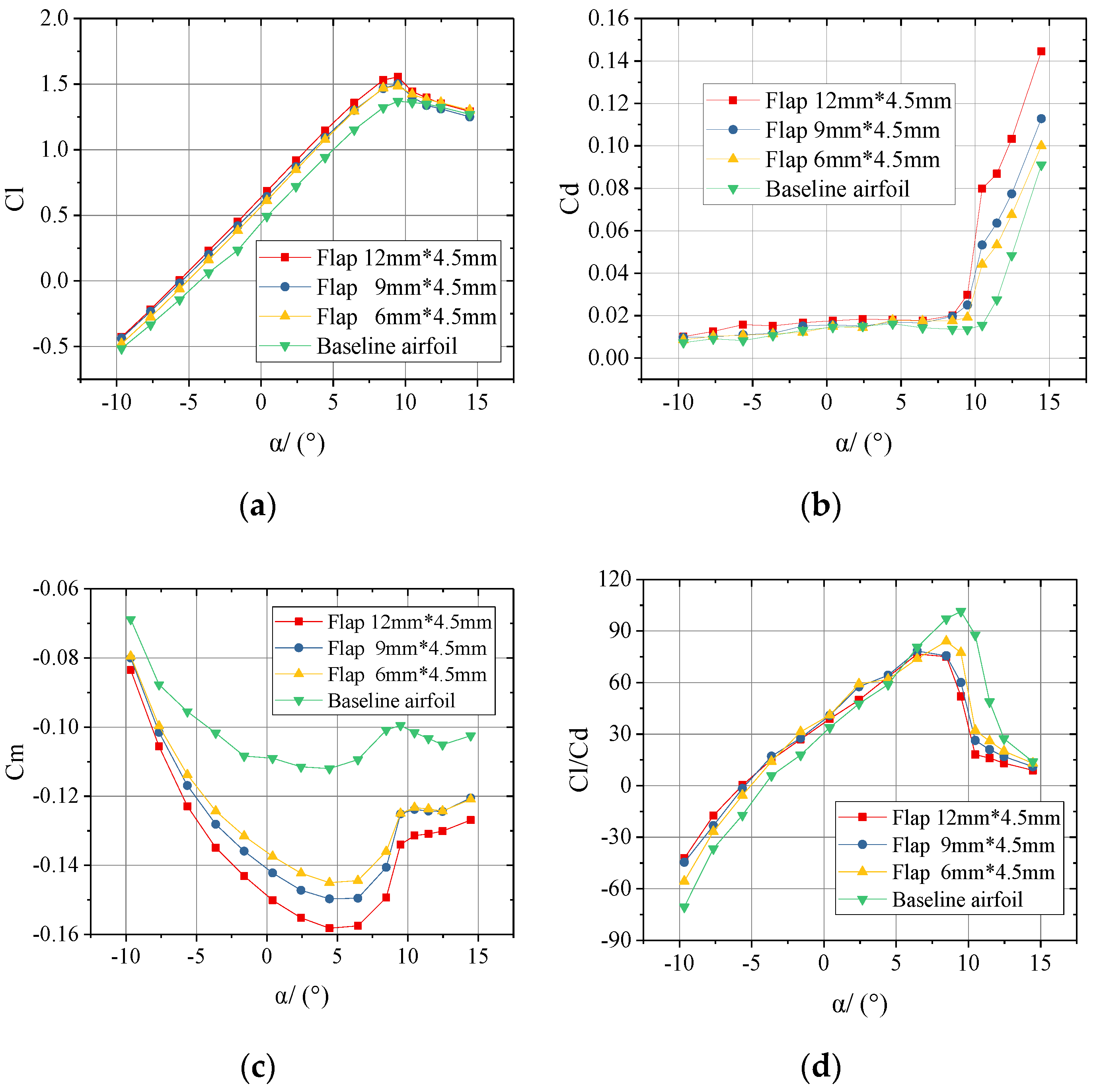
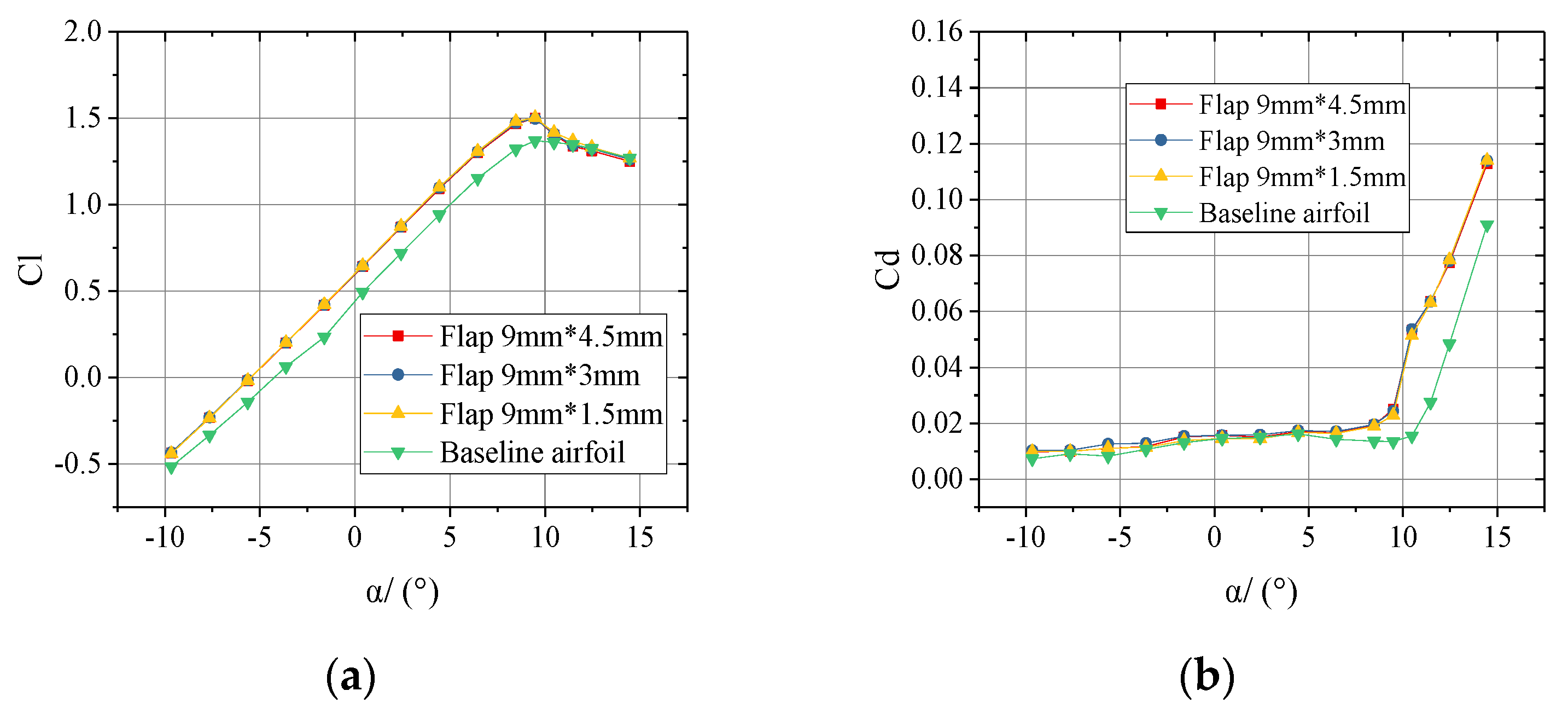
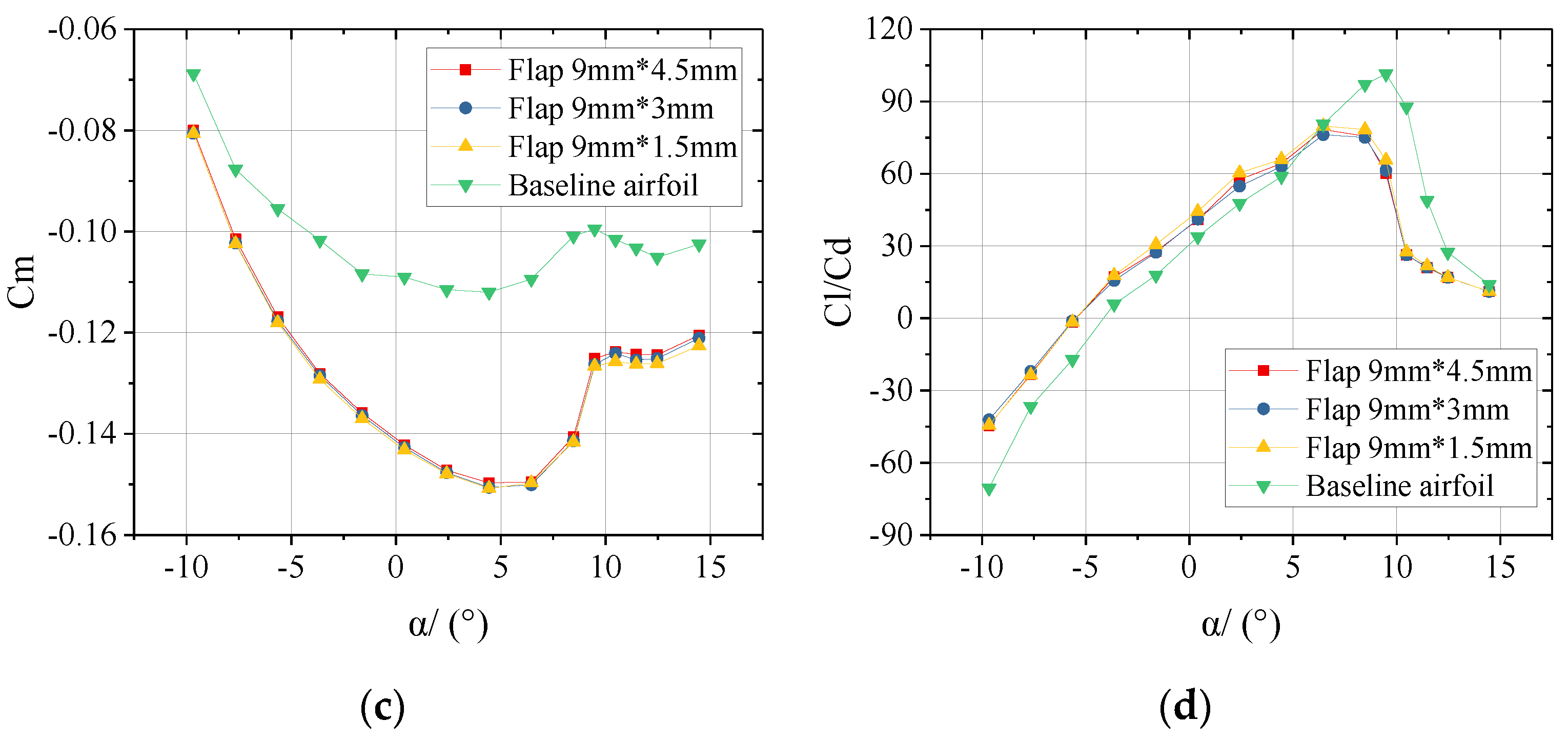
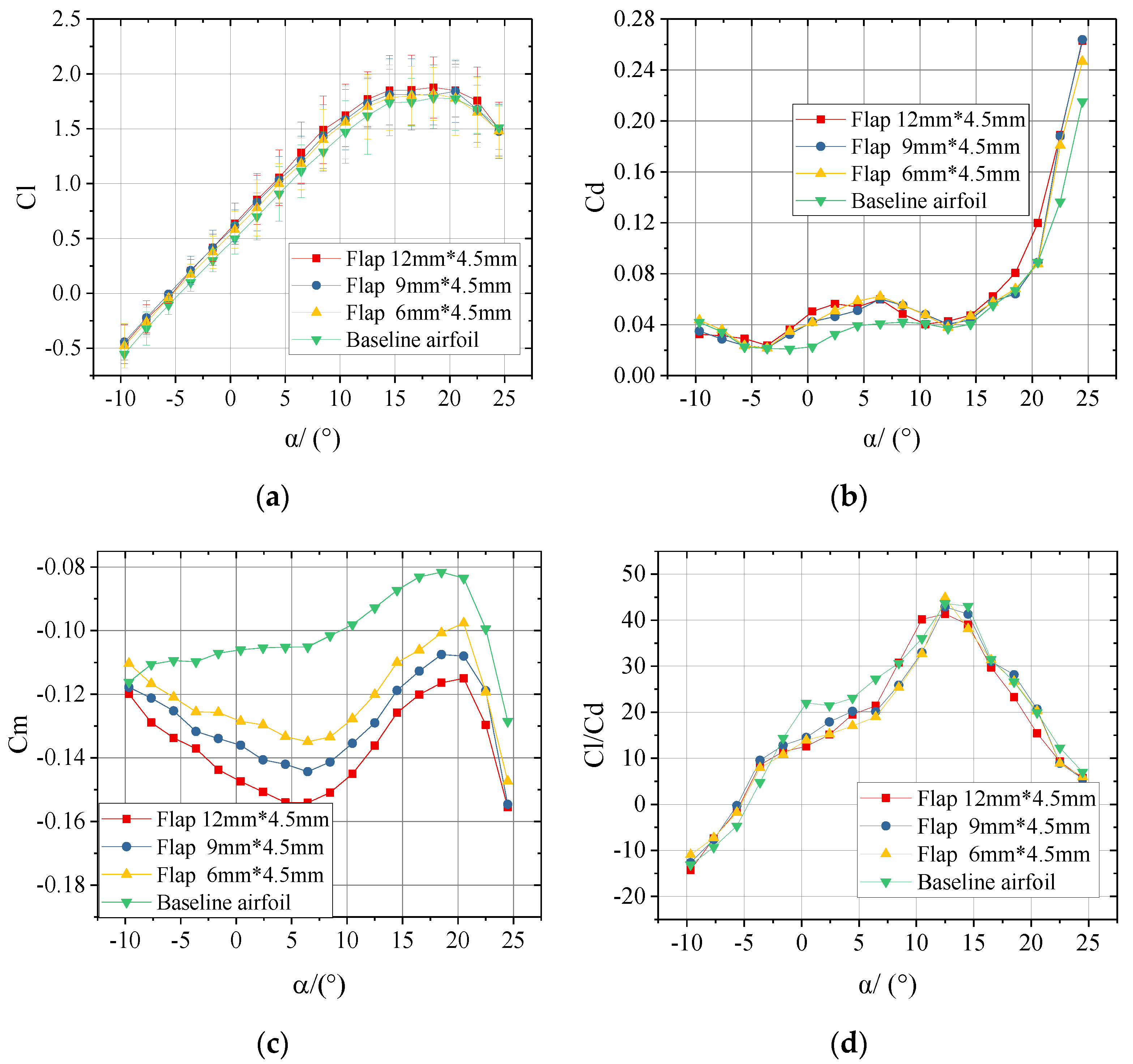
3.3. Experimental Results of the Gurney Flap under Uniform Inflow
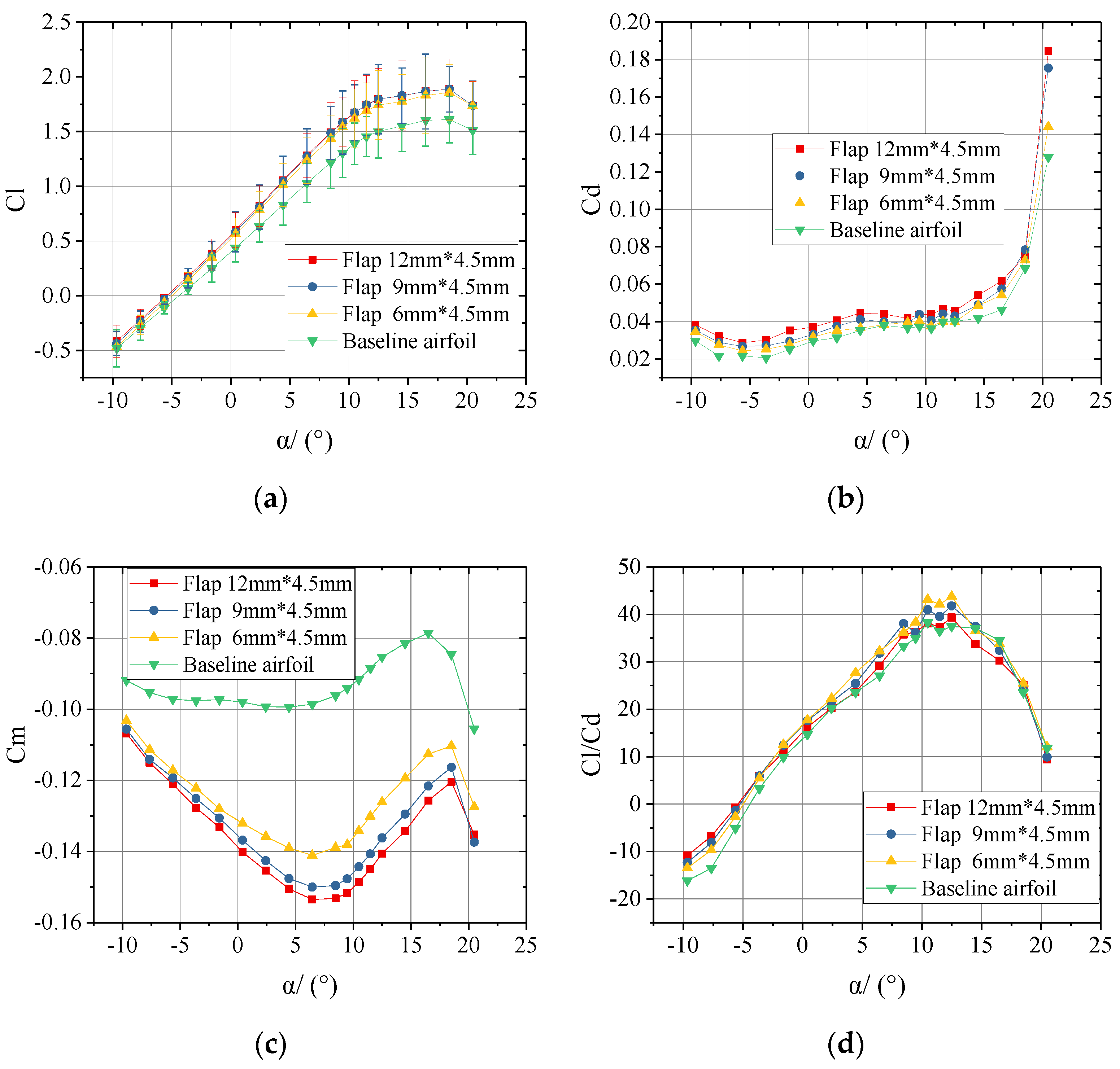
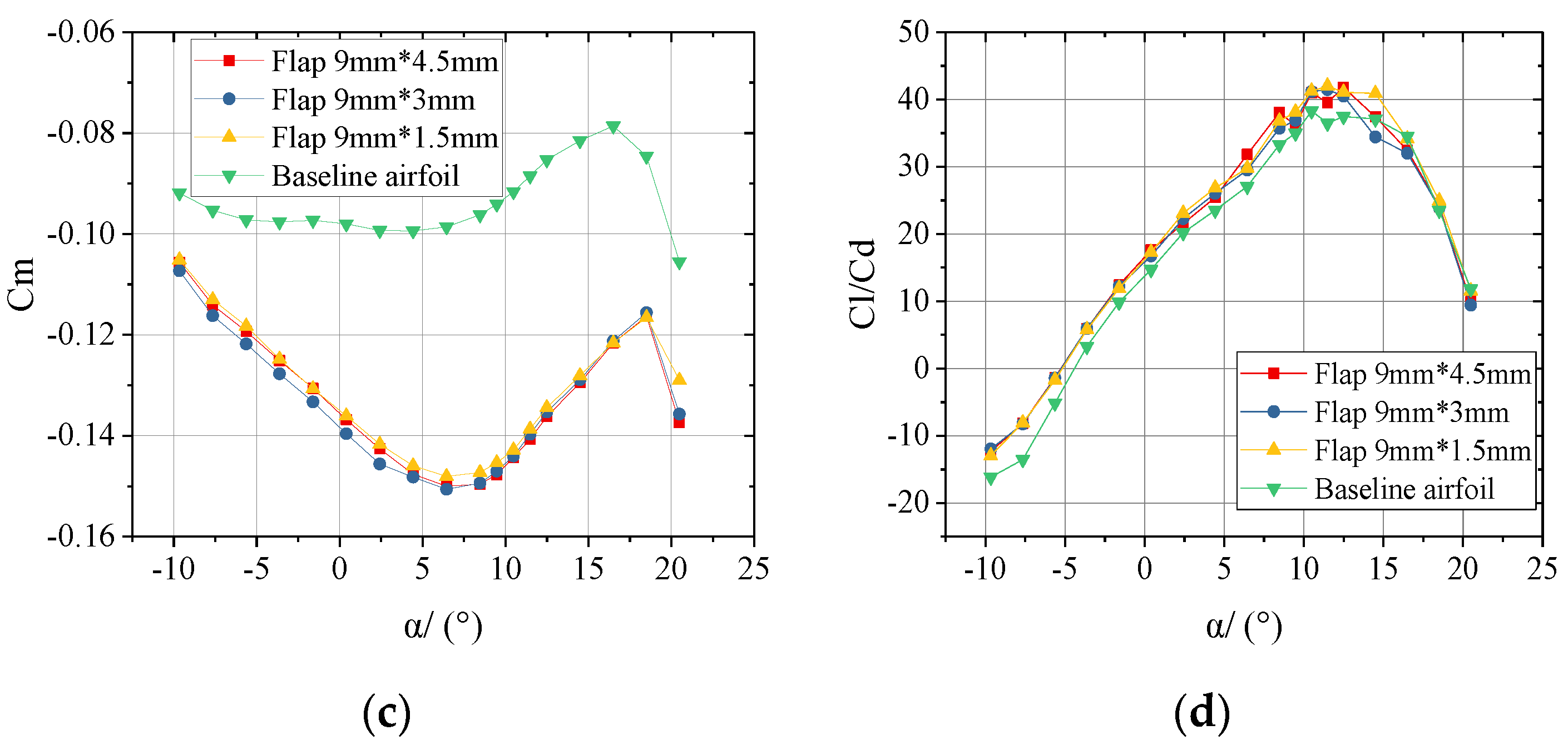
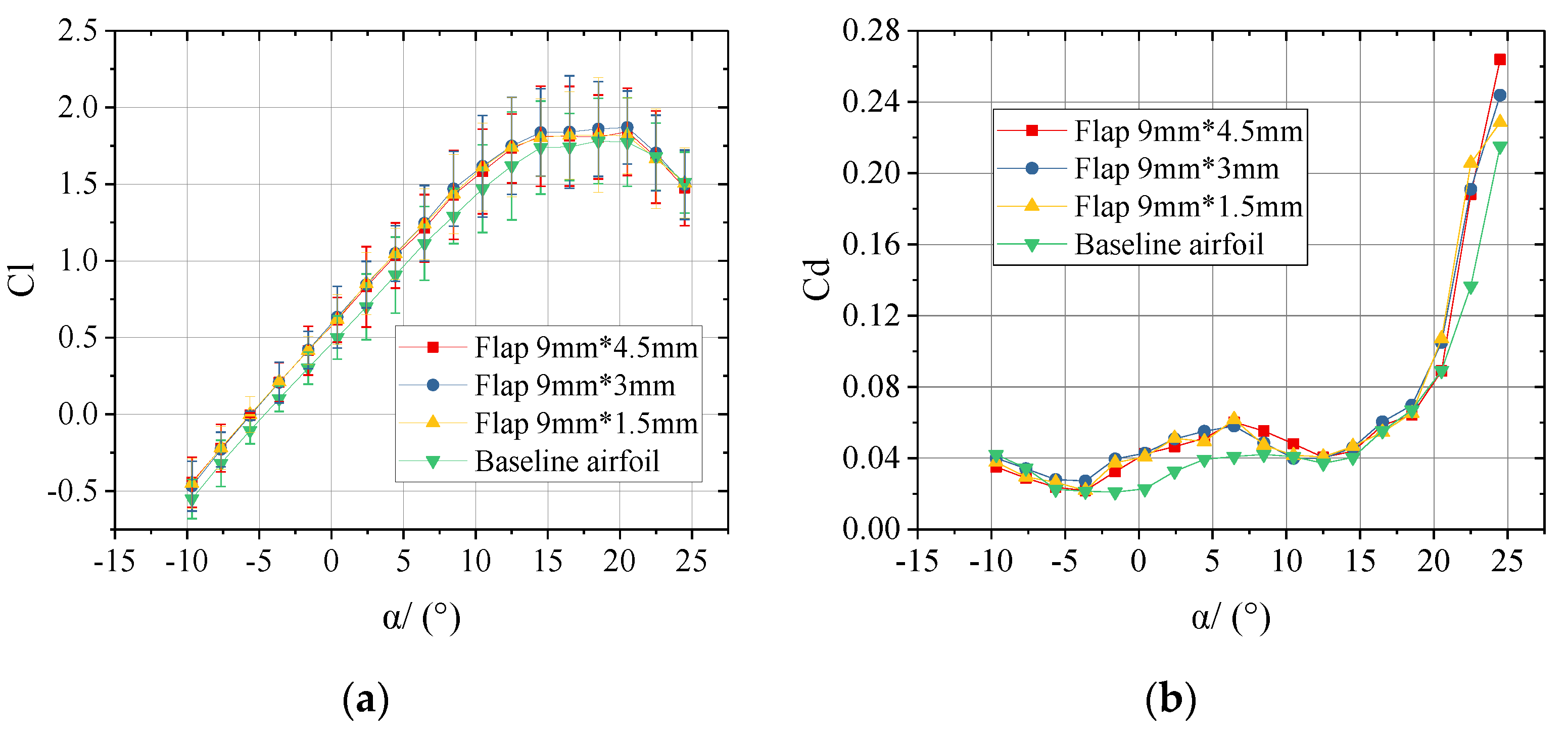
3.4. Experimental Results of Gurney Flap under 10.5% Turbulence Intensity
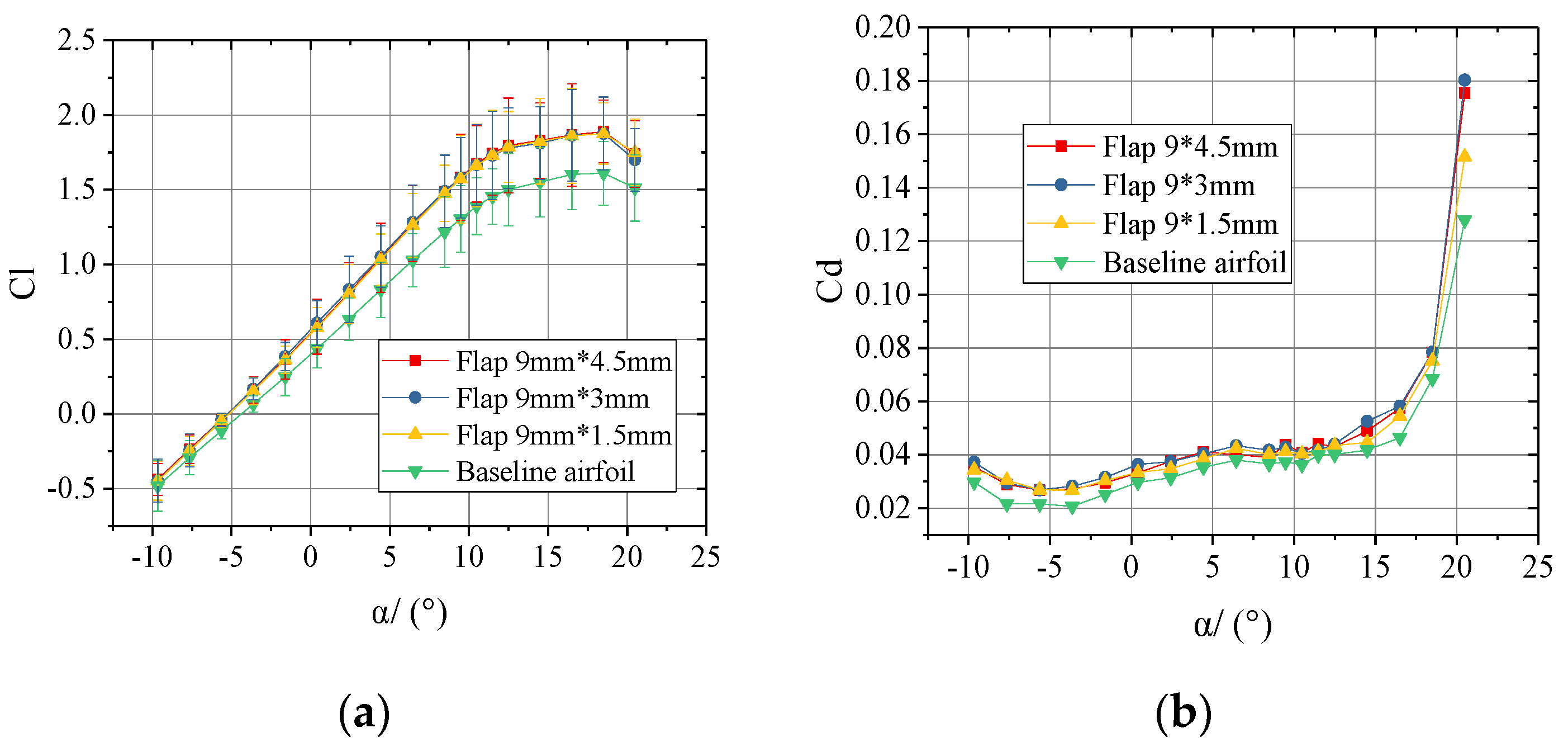
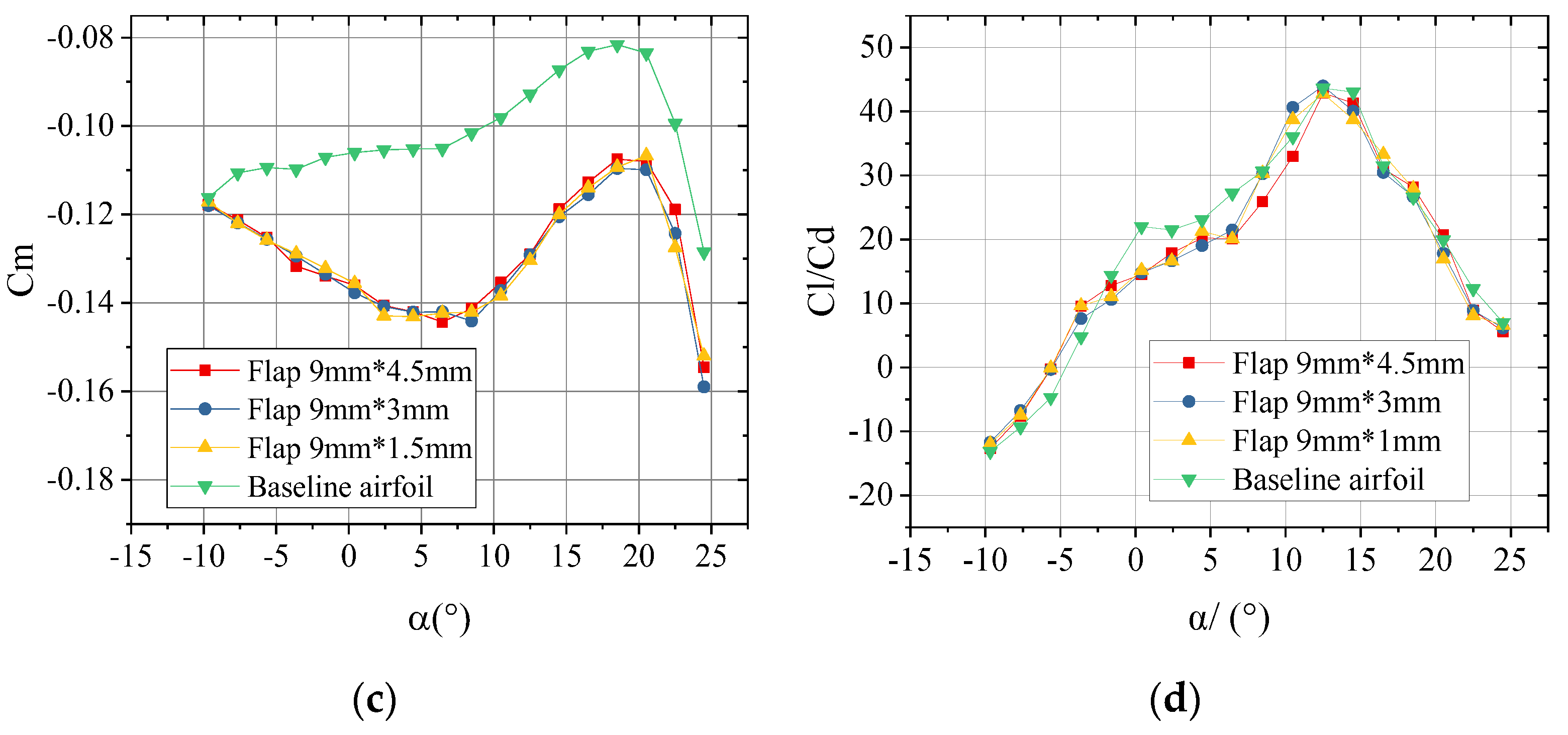
3.5. Experimental Results of Gurney Flap under the 19.0% Turbulence Intensity
3.6. Surface Pressure Characteristics
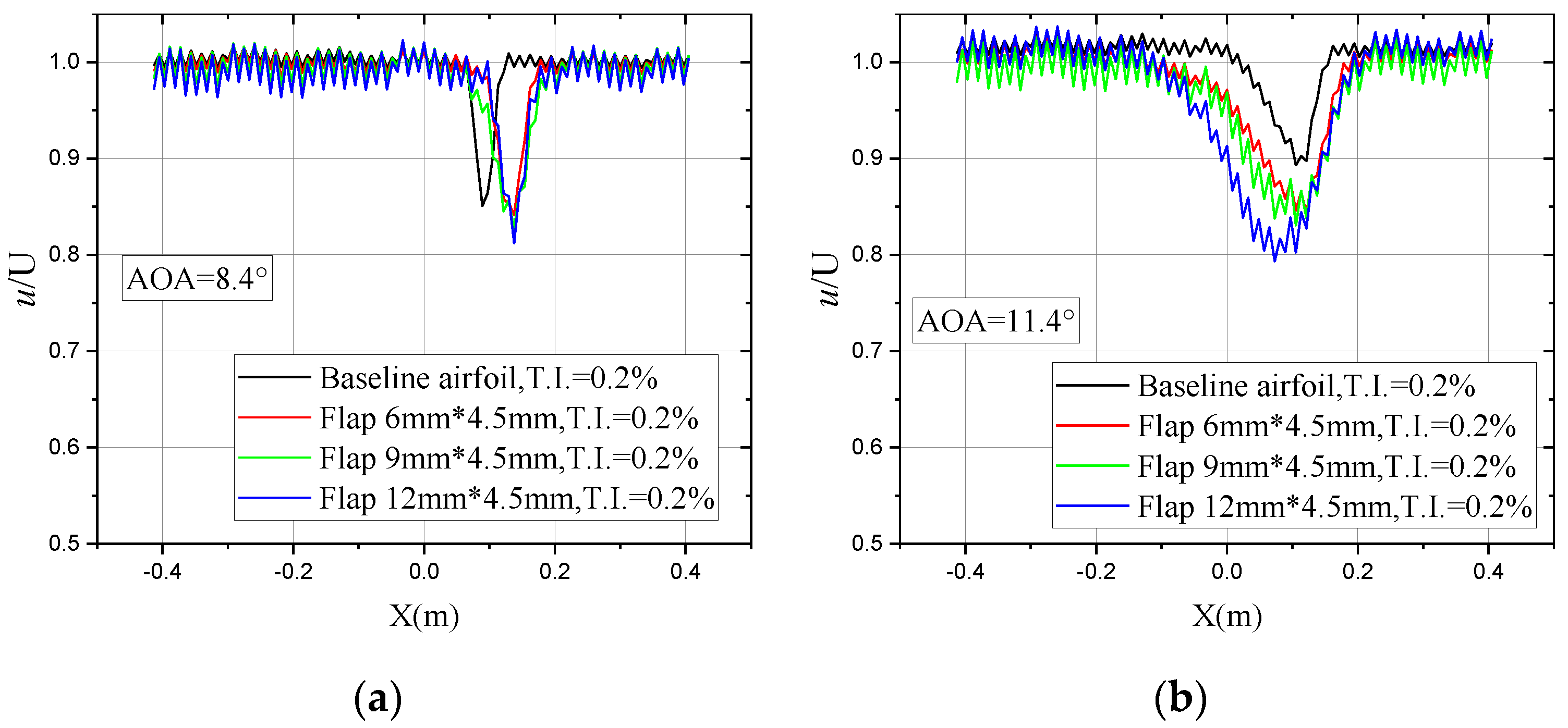
3.7. Wake Profile Characteristics
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Liebeck, R.H. Design of subsonic airfoils for high lift. J. Aircr. 1978, 15, 547–561. [Google Scholar] [CrossRef]
- Amini, Y.; Emdad, H.; Farid, M. Adjoint shape optimization of airfoils with attached Gurney flap. Aerosp. Sci. Technol. 2015, 41, 216–228. [Google Scholar] [CrossRef]
- Xu, H.R.; Yang, H.; Liu, C. Numerical value analysis on aerodynamic performance of DU series airfoil with thickened trailing edge. Trans. Chin. Soc. Agric. Eng. 2014, 30, 101–108. [Google Scholar]
- Yashodhar, V.; Humrutha, G.; Kaushik, M.; Khan, S.A. CFD Studies on Triangular Micro-Vortex Generators in Flow Control. In IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2017; Volume 184, p. 012007. [Google Scholar]
- Lee, T.; Su, Y.Y. Unsteady airfoil with a harmonically deflected trailing-edge flap. J. Fluids Struct. 2011, 27, 1411–1424. [Google Scholar] [CrossRef]
- Seyednia, M.; Masdari, M.; Vakilipour, S. The influence of oscillating trailing-edge flap on the dynamic stall control of a pitching wind turbine airfoil. J. Braz. Soc. Mech. Sci. Eng. 2019, 41, 192. [Google Scholar] [CrossRef]
- Lu, W.S.; Tian, Y.; Liu, P.P. Aerodynamic optimization and mechanism design of flexible variable camber trailing-edge flap. Chin. J. Aeronaut. 2017, 30, 988–1003. [Google Scholar] [CrossRef]
- Zhang, W.G.; Bai, X.J.; Wang, Y.F.; Han, Y.; Hu, Y. Optimization of sizing parameters and multi-objective control of trailing edge flaps on a smart rotor. Renew. Energy 2018, 129, 75–91. [Google Scholar] [CrossRef]
- Zhang, H.; Zhao, Z.D.; Zhou, G.X.; Kang, S. Experimental investigation of the effect of Gurney flap on DU93-W-210 airfoil aerodynamics performance. Acta Energ. Solaris Sinica 2017, 38, 601–606. [Google Scholar]
- Amini, Y.; Liravi, M.; Izadpanah, E. The effects of Gurney flap on the aerodynamic performance of NACA 0012 airfoil in the rarefied gas flow. Comput. Fluids 2018, 170, 93–105. [Google Scholar] [CrossRef]
- Lee, T.; Su, Y.Y. Lift enhancement and flow structure of airfoil with joint trailing-edge flap and Gurney flap. Exp. Fluids 2011, 50, 1671–1684. [Google Scholar] [CrossRef]
- Chen, Z.J.; Stol, K.A.; Mace, B.R. Wind turbine blade optimization with individual pitch and trailing edge flap control. Renew. Energy 2016, 103, 750–765. [Google Scholar] [CrossRef]
- Medina, A.; Ol, M.V.; Mancini, P.; Jones, A. Revisiting Conventional Flaps at High Deflection Rate. AIAA J. 2017, 55, 1–10. [Google Scholar] [CrossRef]
- Elsayed, O.A.; Asrar, W.; Omar, A.A.; Kwon, K.; Jung, H.J. Experimental Investigation of Plain and Flapped-Wing Tip Vortex. J. Aircr. 2009, 46, 254–262. [Google Scholar] [CrossRef]
- Little, J.; Nishihara, M.; Adamovich, I.; Saminy, M. High-lift airfoil trailing edge separation control using a single dielectric barrier discharge plasma actuator. Exp. Fluids 2010, 48, 521–537. [Google Scholar] [CrossRef]
- Bergami, L.; Poulsen, N.K. A smart rotor configuration with linear quadratic control of adaptive trailing edge flaps for active load alleviation. Wind Energy 2015, 18, 625–641. [Google Scholar] [CrossRef] [Green Version]
- Edward, T.; Christoph, B. Upstream shear-layer stabilization via self-oscillating trailing edge flaplets. Exp. Fluids 2018, 59, 145. [Google Scholar]
- Straub, F.K.; Anand, V.R.; Lau, B.H.; Birchette, T.S. Wind Tunnel Test of the SMART Active Flap Rotor. J. Am. Helicopter Soc. 2018, 63, 012002. [Google Scholar] [CrossRef]
- Traub, L.W. Prediction of Gurney-Flap Lift Enhancement for Airfoils and Wings. AIAA J. 2014, 52, 2087–2090. [Google Scholar] [CrossRef]
- Lario, A.; Arina, R. Discontinuous Galerkin Method for the Study of Active Gurney Flaps. J. Aircr. 2017, 54, 1–11. [Google Scholar] [CrossRef]
- Li, C.F.; Xu, Y.; Zhao, X.L.; Xu, J.Z. Analysis on Dynamic Performance Trailing Edge Flap on Wind Turbine Airfoil. J. Eng. Thermophys. 2014, 35, 883–887. [Google Scholar]
- Zhu, W.J.; Behrens, T.; Shen, W.Z.; Sørensen, J.N. Hybrid Immersed Boundary Method for Airfoils with a Trailing-Edge Flap. AIAA J. 2013, 51, 30–41. [Google Scholar] [CrossRef]
- Ng, B.F.; Palacios, R.; Kerrigan, E.C.; Graham, J.M.R.; Hesse, H. Aerodynamic load control in horizontal axis wind turbines with combined aeroelastic tailoring and trailing edge flaps. Wind Energy 2016, 19, 243–263. [Google Scholar] [CrossRef]
- Colman, J.; Marañón Di Leo, J.; Delnero, J.S.; Martinez, M.; Boldes, U.; Bacchi, F. Lift and drag coefficients behaviour at low Reynolds number in an airfoil with Gurney flap submitted to a turbulent flow: Part 1. Lat. Am. Appl. Res. 2008, 38, 195–200. [Google Scholar]
- Di Leo, J.M.; Martínez, M.A.; Delnero, J.S.; Saínz, M.G. Experimental study of the effect of the wake generated by oscillating Gurney flap. Lat. Am. Appl. Res. 2019, 49, 289–296. [Google Scholar]
- Li, W.J.; Zhang, P.; Yang, S.F.; Fu, X.H.; Xiao, Y. An Experimental Method for Generating Shear-Free Turbulence Using Horizontal Oscillating Grids. Water 2020, 12, 591. [Google Scholar] [CrossRef] [Green Version]
- Cheng, J.T.; Zhu, W.J.; Fischer, A.; García, N.R.; Madsen, J.; Chen, J.; Shen, W.Z. Design and validation of the high performance and low noise CQU-DTU-LN1 airfoils. Wind Energy 2013, 17, 1817–1833. [Google Scholar] [CrossRef]
- ROHA. LSWT Campaign Report on DTU-C21; LM Internal Report: Jupitervej, Denmark, 2012. [Google Scholar]
- Allen, H.J.; Vlncenti, W.G. Wall Interference in a Two-Dimensional Flow Wind Tunnel, with Consideration of the effect of Compressibility. NACA Rep. 1944, 782, 155–184. [Google Scholar]
- Timmer, W.A.; van Rooij, R.P.J.O.M. Summary of the Delft University Wind Turbine Dedicated Airfoils. J. Sol. Energy Eng. 2003, 125, 11–21. [Google Scholar] [CrossRef]
- Llorente, E.; Gorostidi, A.; Jacobs, M.; Timmer, W.A.; Munduate, A.; Pires, A. Wind Tunnel Tests of Wind Turbine Airfoils at High Reynolds Numbers. J. Phys. Conf. 2014, 524, 012012. [Google Scholar] [CrossRef]
- Pires, O.; Munduate, X.; Ceyhan, O.; Jacobs, M.; Madsen, J.; Schepers, J.G. Analysis of the high Reynolds number 2D tests on a wind turbine airfoil performed at two different wind tunnels. J. Phys. Conf. Ser. 2016, 749, 012014. [Google Scholar] [CrossRef] [Green Version]
- Troolin, D.R. A Quantitative Study of the Lift-Enhancing Flow Field Generated by an Airfoil with a Gurney Flap. Ph.D. Dissertation, University of Minnesota, Minnesota, MN, USA, 2009. [Google Scholar]
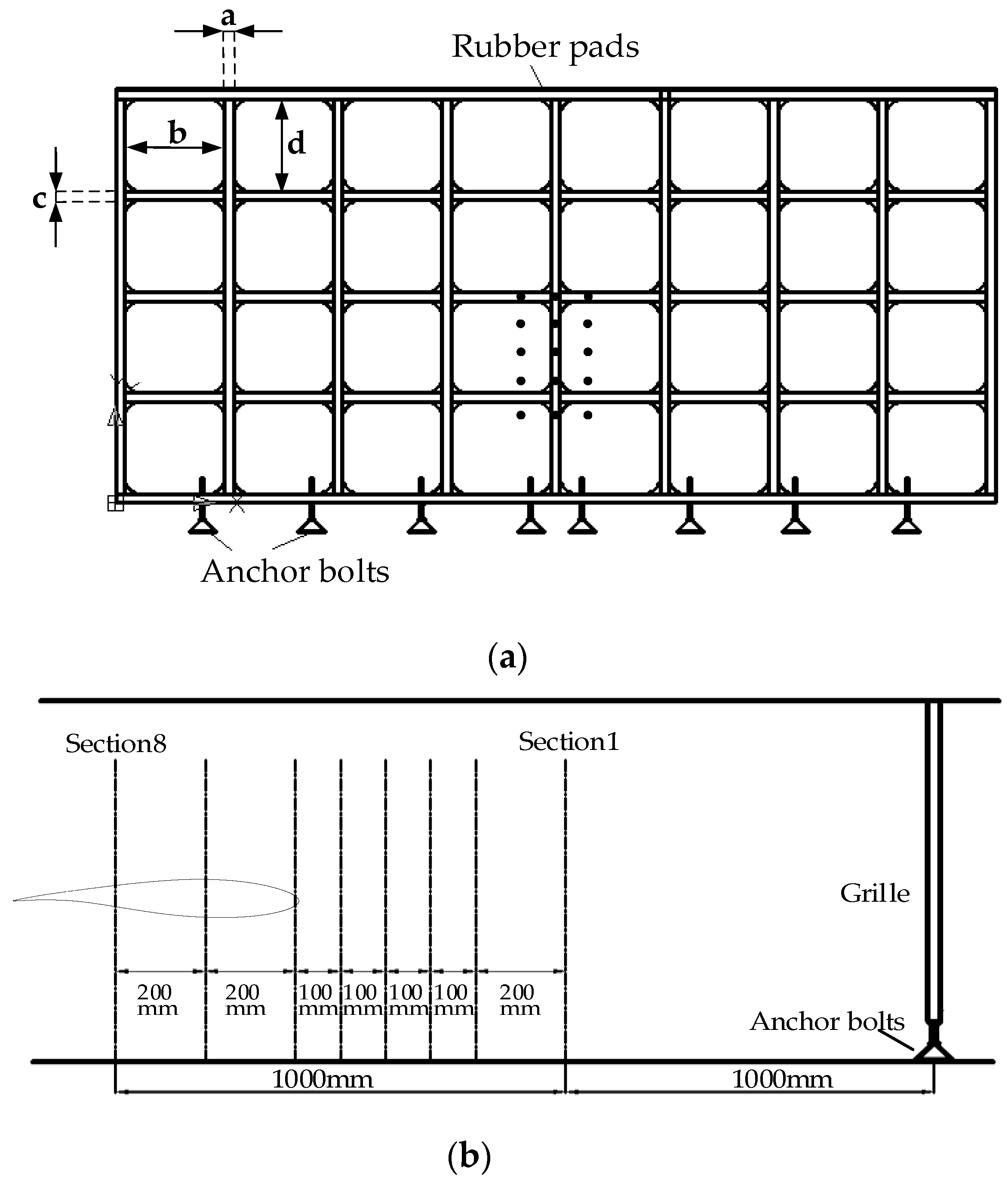








| a (cm) | b (cm) | c (cm) | d (cm) | e (cm) | |
|---|---|---|---|---|---|
| Scheme 1 | 3 | 34 | 3 | 32 | 3 |
| Scheme 2 | 6 | 31.4 | 6 | 29.8 | 3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, J.; Yang, H.; Zhu, W.; Li, N.; Yuan, Y. Experimental Study on Aerodynamic Characteristics of a Gurney Flap on a Wind Turbine Airfoil under High Turbulent Flow Condition. Appl. Sci. 2020, 10, 7258. https://doi.org/10.3390/app10207258
Yang J, Yang H, Zhu W, Li N, Yuan Y. Experimental Study on Aerodynamic Characteristics of a Gurney Flap on a Wind Turbine Airfoil under High Turbulent Flow Condition. Applied Sciences. 2020; 10(20):7258. https://doi.org/10.3390/app10207258
Chicago/Turabian StyleYang, Junwei, Hua Yang, Weijun Zhu, Nailu Li, and Yiping Yuan. 2020. "Experimental Study on Aerodynamic Characteristics of a Gurney Flap on a Wind Turbine Airfoil under High Turbulent Flow Condition" Applied Sciences 10, no. 20: 7258. https://doi.org/10.3390/app10207258
APA StyleYang, J., Yang, H., Zhu, W., Li, N., & Yuan, Y. (2020). Experimental Study on Aerodynamic Characteristics of a Gurney Flap on a Wind Turbine Airfoil under High Turbulent Flow Condition. Applied Sciences, 10(20), 7258. https://doi.org/10.3390/app10207258






