Multilayered Artificial Dura-Mater Models for a Minimally Invasive Brain Surgery Simulator
Abstract
:Featured Application
Abstract
1. Introduction
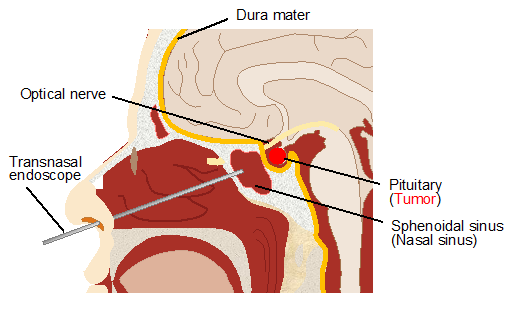
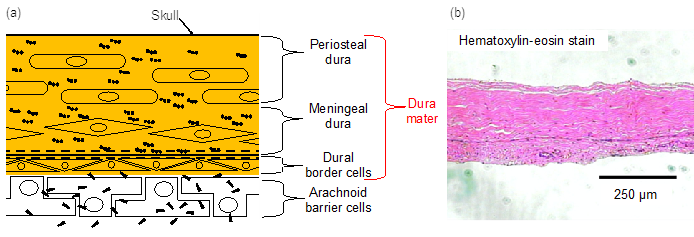
2. Dura-Mater
3. Fabrication Procedure of Dura-Mater Models
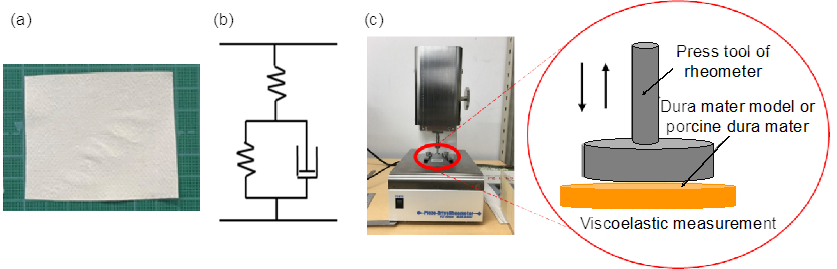
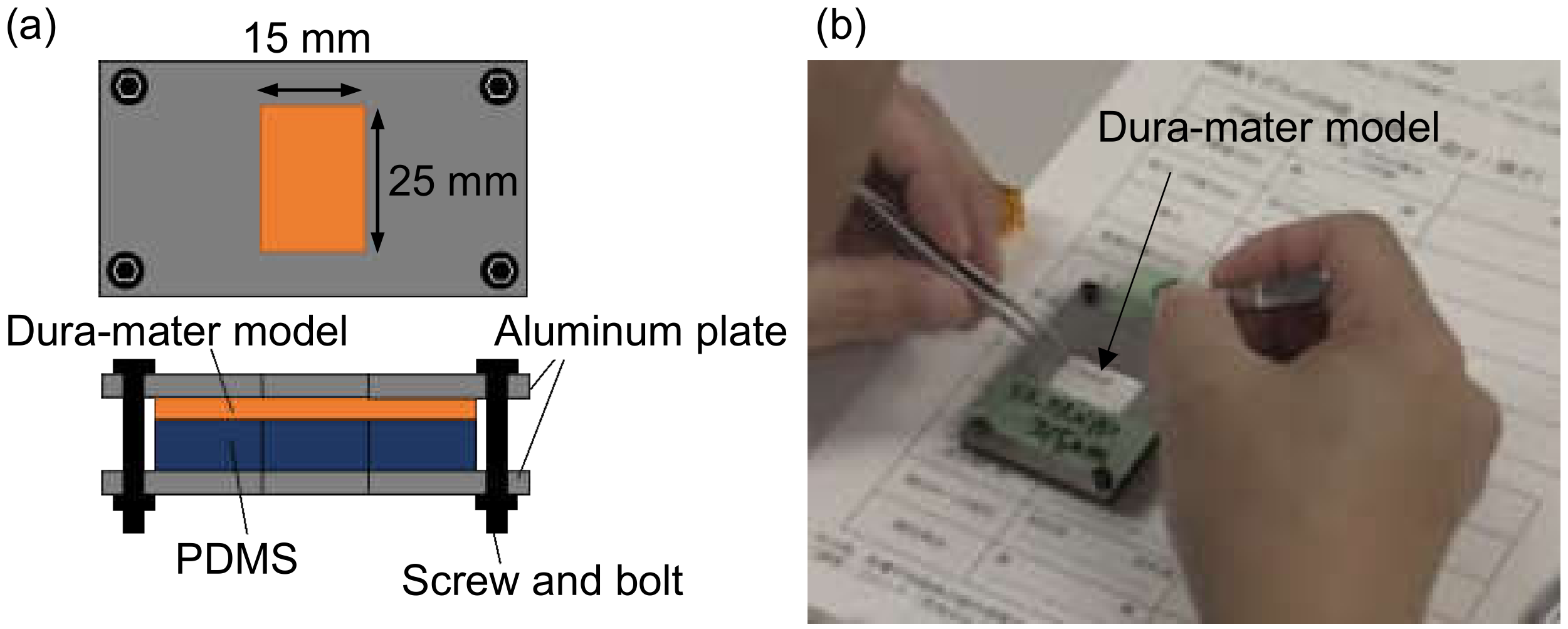
4. Evaluation of Dura-Mater Models
4.1. Viscoelastic Measurements
4.2. Tensile Test
4.3. Microscissors Test
5. Sensory Test by Neurosurgeons
6. Integration in a Surgical Simulator
7. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Fried, M.P.; Sadoughi, B.; Weghorst, S.J.; Zeltsan, M.; Cuellar, H.; Uribe, J.I.; Sasaki, C.T.; Ross, D.A.; Jacobs, J.B.; Lebowitz, R.A.; et al. Construct validity of the endoscopic sinus surgery simulator. Arch. Otolaryngol. Head Neck Surg. 2007, 133, 350–357. [Google Scholar] [CrossRef] [Green Version]
- Gallagher, A.G.; Ritter, E.M.; Satava, R.M. Fundamental principles of validation, and reliability: Rigorous science for the assessment of surgical education and training. Surg. Endosc. 2003, 17, 1525–1529. [Google Scholar] [CrossRef] [PubMed]
- Woodrum, D.T.; Andreatta, P.B.; Yellamanchilli, R.K.; Feryus, L.; Gauger, P.G.; Minter, R.M. Construct validity of the LapSim laparoscopic surgical simulator. Am. J. Surg. 2006, 191, 28–32. [Google Scholar] [CrossRef] [PubMed]
- Maithel, S.; Sierra, R.; Korndorffer, J.; Neumann, P.; Dawson, S.; Callery, M.; Jones, D.; Scott, D. Construct and face validity of MIST-VR, Endotower, and CELTS: Are we ready for skills assessment using simulators? Surg. Endosc. 2006, 20, 104–112. [Google Scholar] [CrossRef] [PubMed]
- Yagmurlu, K.; Safavi-Abbasi, S.; Belykh, E.; Kalani, M.Y.S.; Najahu, P.; Rhoton, A.L., Jr.; Spetzler, R.F.; Preul, M.C. Quantitative anatomical analysis and clinical experience with mini-pterional and mini-orbitozygomatic approaches for intracranial aneurysm surgery. J. Neurosurg. 2017, 127, 646–659. [Google Scholar] [CrossRef]
- Okuda, T.; Kataoka, K.; Kato, A. Training in endoscopic endonasal transsphenoidal surgery using a skull model and eggs. Acta Neurochir. 2010, 152, 1801–1804. [Google Scholar] [CrossRef] [PubMed]
- Wen, G.; Cong, Z.; Liu, K.; Tang, C.; Zhong, C.; Li, L.; Dai, X.; Ma, C. A practical 3D printed simulator for endoscopic endonasal transsphenoidal surgery to improve basic operational skills. Child’s Nerv. Syst. 2016, 32, 1109–1116. [Google Scholar] [CrossRef]
- Tai, B.L.; Wang, A.C.; Joseph, J.R.; Wang, P.I.; Sullivan, S.E.; McKean, E.L.; Shih, A.J.; Rooney, D.M. A physical simulator for endoscopic endonasal drilling techniques: Technical note. J. Neurosurg. 2016, 124, 811–816. [Google Scholar] [CrossRef] [Green Version]
- Joseph, F.J.; Weber, S.; Raabe, A.; Bervini, D. Neurosurgical simulator for training aneurysm microsurgery—A user suitability study involving neurosurgeons and residents. Acta Neurochir. 2020, 162, 2313–2321. [Google Scholar] [CrossRef]
- McGarvey, K.A.; Lee, J.M.; Boughner, D.R. Mechanical suitability of glycerol-preserved human dura mater for construction of prosthetic cardiac valves. Biomaterials 1984, 5, 109–117. [Google Scholar] [CrossRef]
- Shetye, S.S.; Deault, M.M.; Puttlitz, C.M. Biaxial reaponse of ovine spinal cord dura mater. J. Mech. Behav. Biomed. Mater. 2014, 34, 146–153. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kizmazoglu, C.; Aydin, H.E.; Kaya, I.; Atar, M.; Husemoglu, B.; Kalemci, O.; Sozer, G.; Havitcioglu, H. Comparison of biomechanical properties of dura mater substitutes and cranial human dura mater: An in vitro study. J. Korean Neurosurg. Soc. 2019, 62, 635–642. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Deopujari, C.E.; Karmarkar, V.S.; Shaikh, S.T.; Gadgil, U.S. Developing a dynamic simulator for endoscopic intraventricular surgeries. Child’s Nerv. Syst. 2019, 35, 621–627. [Google Scholar] [CrossRef] [PubMed]
- Ryan, J.R.; Almefty, K.K.; Nakaji, P.; Frakes, D.H. Cerebral aneurysm clipping surgery simulation using patient-specific 3D printing and silicone casting. World Neurosurg. 2016, 88, 175–181. [Google Scholar] [CrossRef] [PubMed]
- Garling, R.J.; Jin, X.; Yang, J.; Khasawneh, A.H.; Harris, C.A. Low-cost endoscopic third ventriculostomy simulator with mimetic endoscope. J. Neurosurg. Pediatr. 2018, 22, 137–146. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Craven, C.L.; Baxter, D.; Cooke, M.; Carline, L.; Alberti, S.J.M.M.; Beard, J.; Murphy, M. Development of a modelled anatomical replica for training young neurosurgeons. Br. J. Neurosurg. 2014, 28, 707–712. [Google Scholar] [CrossRef] [PubMed]
- Eastwood, K.W.; Bodani, V.P.; Haji, F.A.; Looi, T.; Naguib, H.; Drake, J.M. Development of synthetic simulators for endoscope-assisted repair of metopic and sagittal craniosynostosis. J. Neurosurg. Pediatr. 2018, 22, 128–136. [Google Scholar] [CrossRef]
- Carrau, R.L.; Jho, H.D.; Ko, Y. Transnasal-transsphenoidal endoscopic surgery of the pituitary gland. Laryngoscope 1996, 106, 914–918. [Google Scholar] [CrossRef]
- Schaberg, M.R.; Anand, V.K.; Schwartz, T.H.; Cobb, W. Microscopic versus endoscopic transnasal pituitary surgery. Curr. Opin. Otolaryngol. Head Neck Surg. 2010, 18, 8–14. [Google Scholar] [CrossRef]
- Miller, K.; Wittek, A.; Joldes, G. Biomechanics of the brain for computer-integrated surgery. Acta Bioeng. Biomech. 2010, 12, 25–37. [Google Scholar]
- Patin, D.J.; Eckstein, E.C.; Harum, K.; Pallares, V.S. Anatomic and biomechanical properties of human lumbar dura mater. Anesth. Analg. 1993, 76, 535–540. [Google Scholar] [CrossRef] [PubMed]
- Reina, M.A.; Dittmann, M.; López Garcia, A.; van Zundert, A. New perspectives in the microscopic structure of human dura mater in the dorsolumbar region. Reg. Anesth. Pain Med. 1997, 22, 161–166. [Google Scholar] [PubMed]
- Hasegawa, A.; Ichikawa, A.; Uchida, K.; Kotani, N.; Hayakawa, S.; Takeuchi, M.; Fukuda, T. Fabrication of dry dura mater models for medical surgical simulator. In Proceedings of the 2017 IEEE International Conference on Cyborg and Bionic Systems (CBS), Beijing, China, 17–19 October 2017; pp. 293–297. [Google Scholar]
- Zwirner, J.; Scholze, M.; Waddell, J.N.; Ondruschka, B.; Hammer, N. Mechanical properties of human dura mater in tension—An analysis at an age range of 2 to 94 years. Sci. Rep. 2019, 9, 16655. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hayakawa, S.; Takeuchi, M.; Ichikawa, A.; Hasegawa, A.; Uchida, K.; Fukuda, T.; Huang, Q. Multi-layered Artificial Dura Mater Models for Medical Surgical Simulators. In Proceedings of the 2019 IEEE International Conference on Cyborg and Bionic Systems (CBS), Munich, Germany, 18–20 September 2019; pp. 310–315. [Google Scholar]
- Yue, X.; Wang, L.; Sun, S.; Tong, L. Viscoelastic finite-element analysis of human skull-dura mater system as intracranial pressure changing. Afr. J. Biotechnol. 2008, 7, 689–695. [Google Scholar]
- Mahvash, M.; Voo, L.M.; Kim, D.; Jeung, K.; Wainer, J.; Okamura, A.M. Modeling the forces of cutting with scissors. IEEE Trans. Biomed. Eng. 2008, 55, 848–856. [Google Scholar] [CrossRef] [Green Version]
- Masuda, T.; Kanako, H.; Adachi, S.; Arai, F.; Omata, S.; Morita, A.; Kin, T.; Saito, N.; Yamashita, J.; Chinzei, K.; et al. Patients simulator for transsphenoidal surgery. In Proceedings of the 2018 International Symposium on Micro-Nano Mechatronics and Human Science (MHS), Nagoya, Japan, 9–12 December 2018; Volume 28, pp. 1–2. [Google Scholar]
- Someya, Y.; Omata, S.; Hayakawa, T.; Mitsuishi, M.; Sugita, N.; Harada, K.; Noda, Y.; Ueta, T.; Totsuka, K.; Araki, F.; et al. Training system using Bionic-eye for internal limiting membrane peeling. In Proceedings of the 2016 International Symposium on Micro-NanoMechatronics and Human Science (MHS), Nagoya, Japan, 28–30 November 2016; pp. 67–68. [Google Scholar]
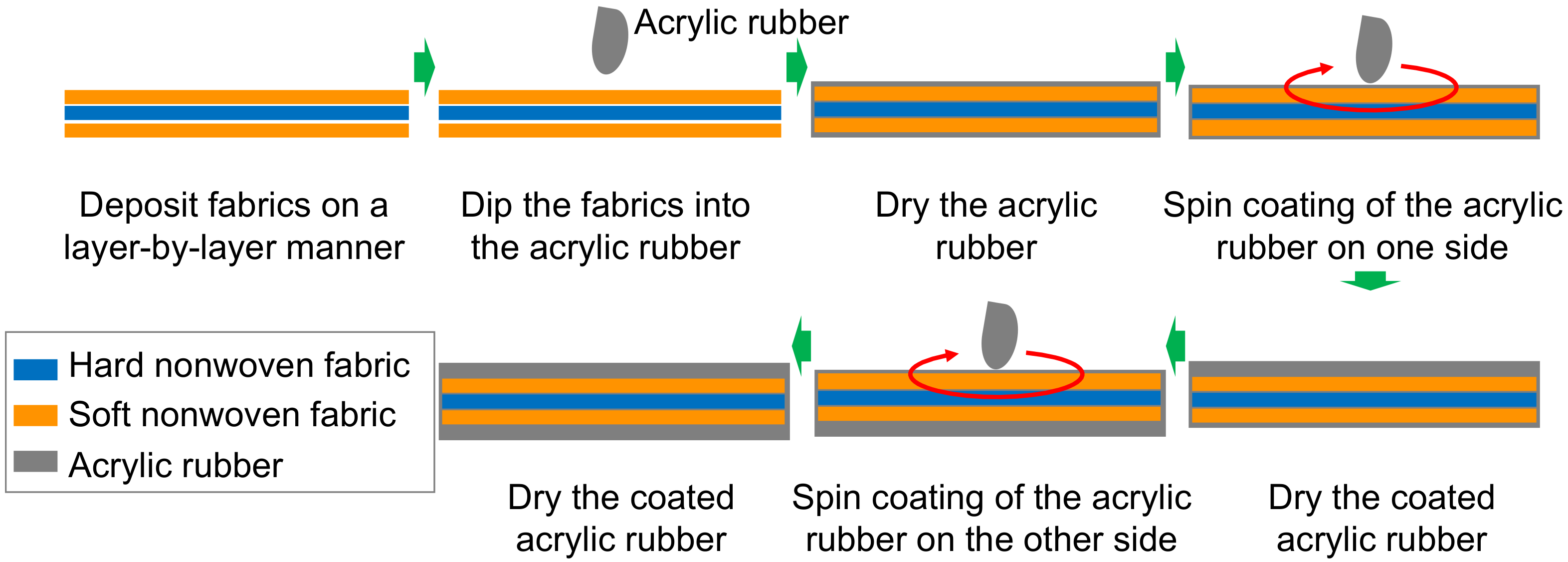

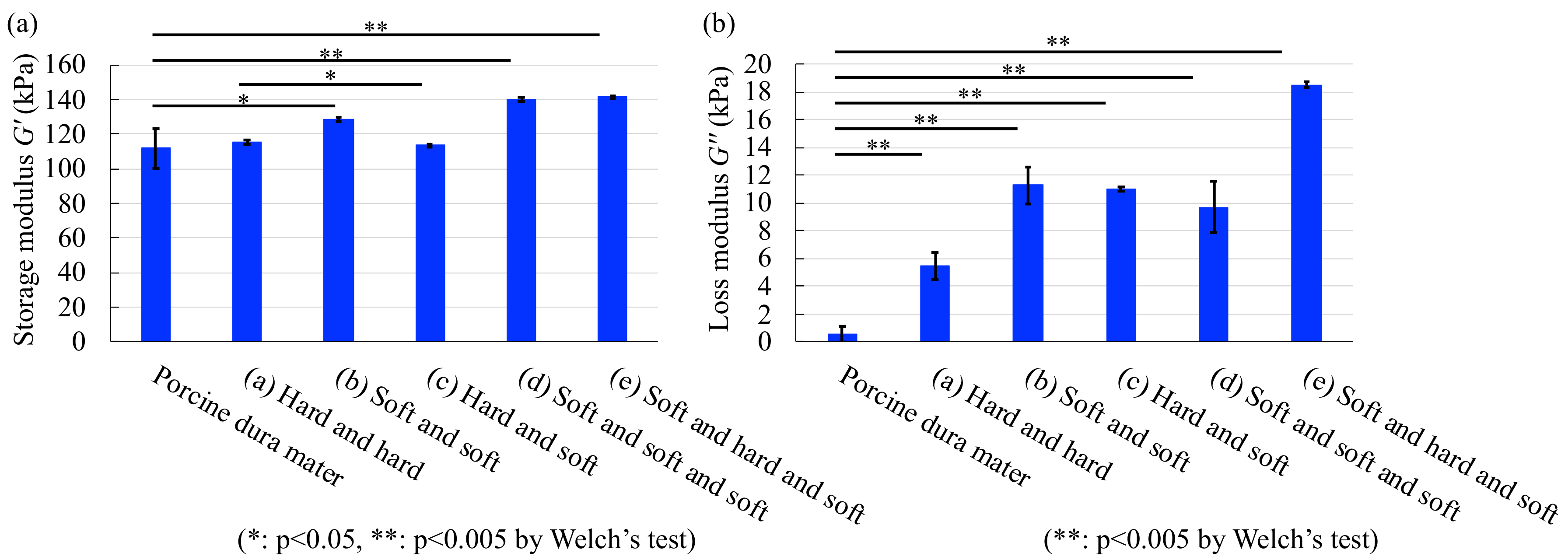

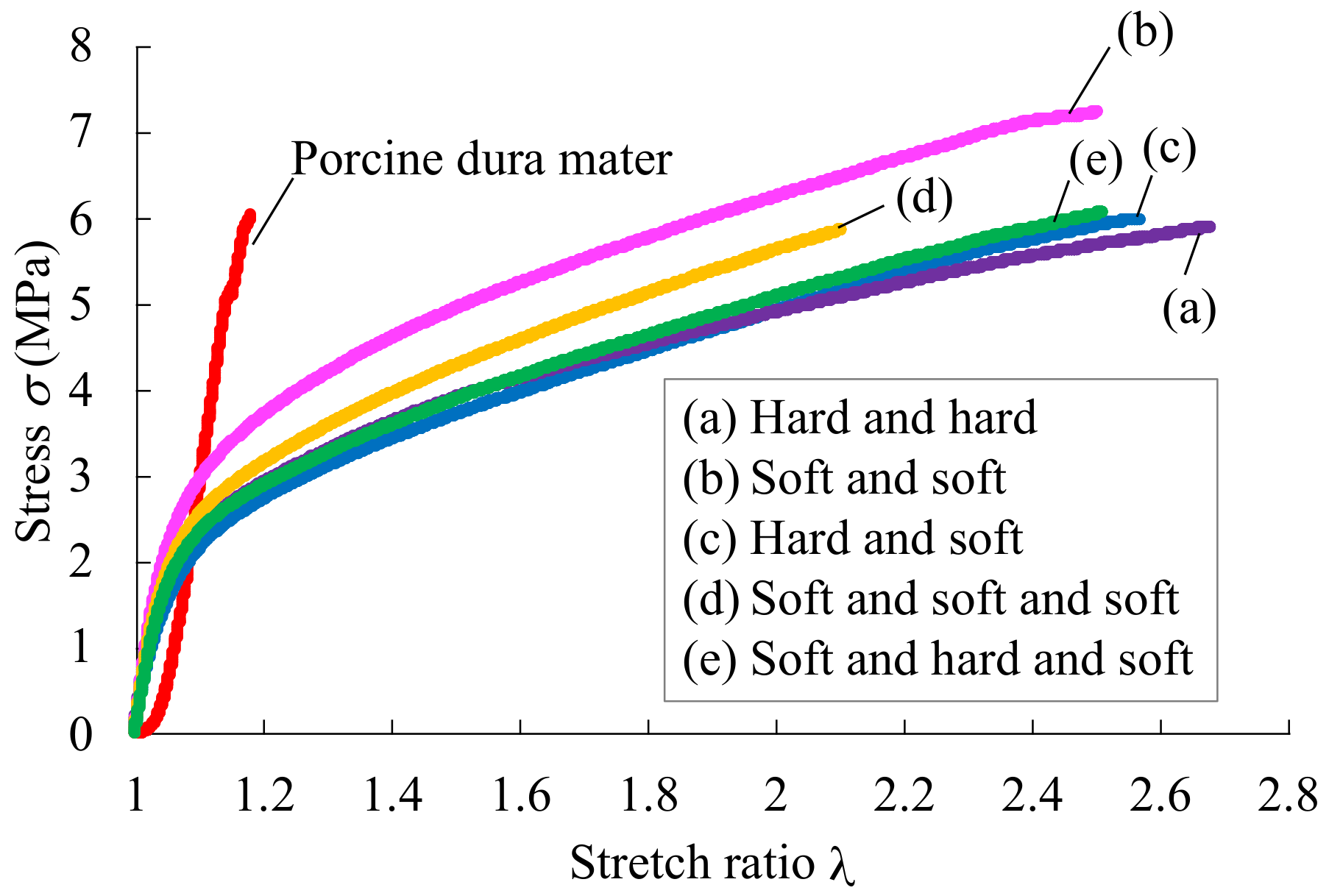
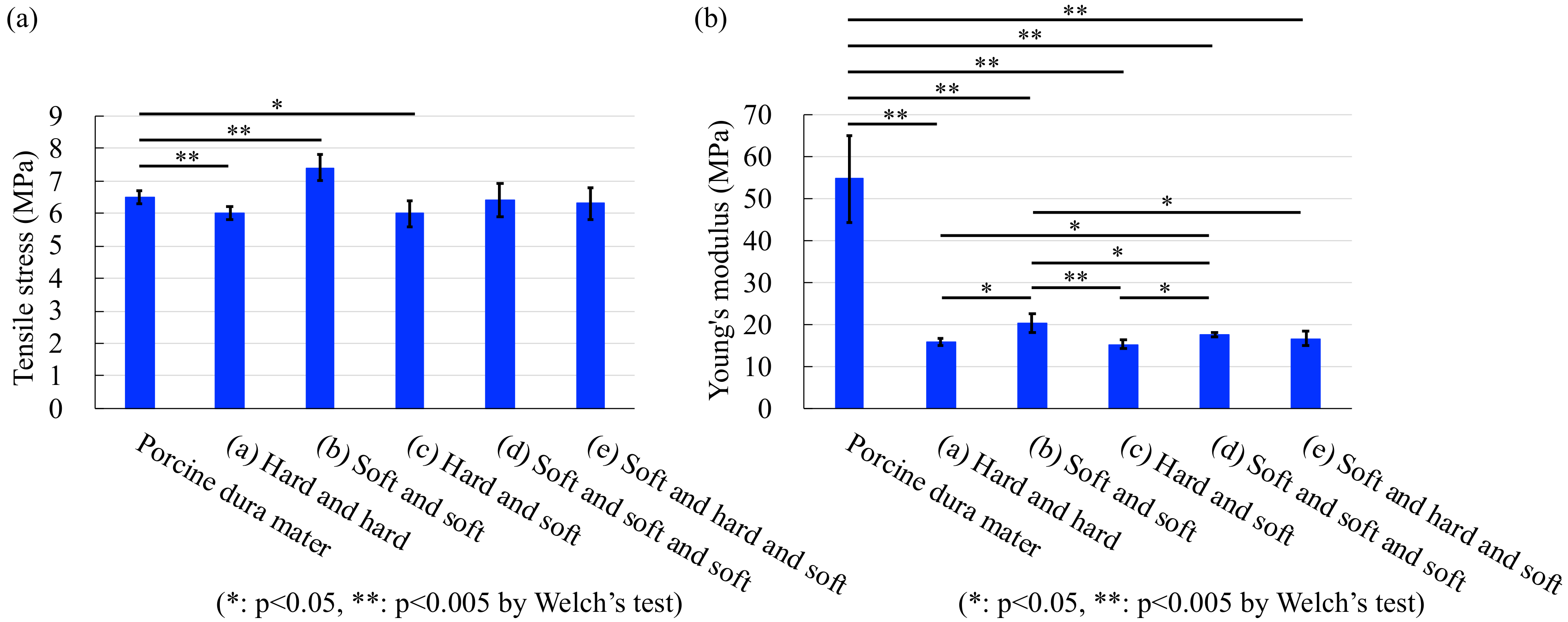


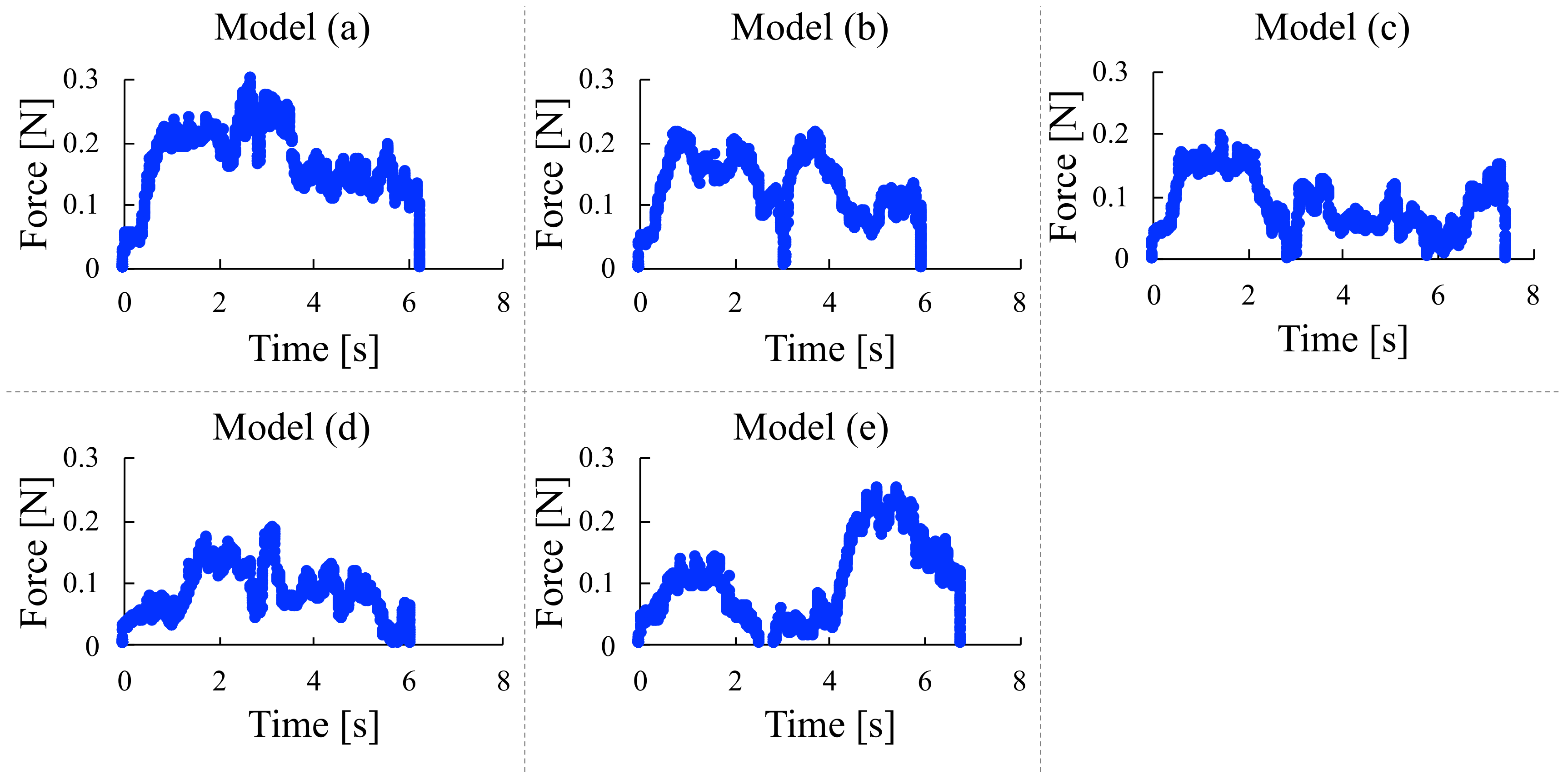



| Hard non-woven fabric Thickness: 120 ± 10 μ mFiber diameter: 40 ± 10 μm |  |
| Soft non-woven fabric Thickness: 80 ±10 μ mFiber diameter: 20 ± 10 μm |  |
| - | Dura-Mater Models | ||||
|---|---|---|---|---|---|
| (a) | (b) | (c) | (d) | (e) | |
| Storage modulus | 3.0 | 14.8 | 24.8 | 25.3 | 26.4 |
| Loss modulus | 256.2 | 635.3 | 620.4 | 531.6 | 1110 |
| Tensile stress | 9.1 | 12.4 | 8.3 | 2.4 | 4.4 |
| Young’s modulus | 71.1 | 62.7 | 72.3 | 68.0 | 69.7 |
| Microscissors test | 30.1 | 58.9 | 50.8 | 38.9 | 31.7 |
| - | Model | ||||
|---|---|---|---|---|---|
| (a) | (b) | (c) | (d) | (e) | |
| Dr. M | 5 | 2 | 4 | 3 | 1 |
| Dr. K | 3 | 1 | 6 | 3 | 2 |
| Total | 4 | 1 | 5 | 3 | 1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Takeuchi, M.; Hayakawa, S.; Ichikawa, A.; Hasegawa, A.; Hasegawa, Y.; Fukuda, T. Multilayered Artificial Dura-Mater Models for a Minimally Invasive Brain Surgery Simulator. Appl. Sci. 2020, 10, 9000. https://doi.org/10.3390/app10249000
Takeuchi M, Hayakawa S, Ichikawa A, Hasegawa A, Hasegawa Y, Fukuda T. Multilayered Artificial Dura-Mater Models for a Minimally Invasive Brain Surgery Simulator. Applied Sciences. 2020; 10(24):9000. https://doi.org/10.3390/app10249000
Chicago/Turabian StyleTakeuchi, Masaru, Shusaku Hayakawa, Akihiko Ichikawa, Akiyuki Hasegawa, Yasuhisa Hasegawa, and Toshio Fukuda. 2020. "Multilayered Artificial Dura-Mater Models for a Minimally Invasive Brain Surgery Simulator" Applied Sciences 10, no. 24: 9000. https://doi.org/10.3390/app10249000
APA StyleTakeuchi, M., Hayakawa, S., Ichikawa, A., Hasegawa, A., Hasegawa, Y., & Fukuda, T. (2020). Multilayered Artificial Dura-Mater Models for a Minimally Invasive Brain Surgery Simulator. Applied Sciences, 10(24), 9000. https://doi.org/10.3390/app10249000






