Light Emitting Devices Based on Quantum Well-Dots
Abstract
:1. Introduction
2. Growth and Structural Properties
3. Optical Properties
3.1. The Impact of Indium Composition and Average Layer Thickness on Photoluminescence Spectra
3.2. Lateral Carrier Diffusion in QWDs
3.3. Dynamic Characteristics of QWDs
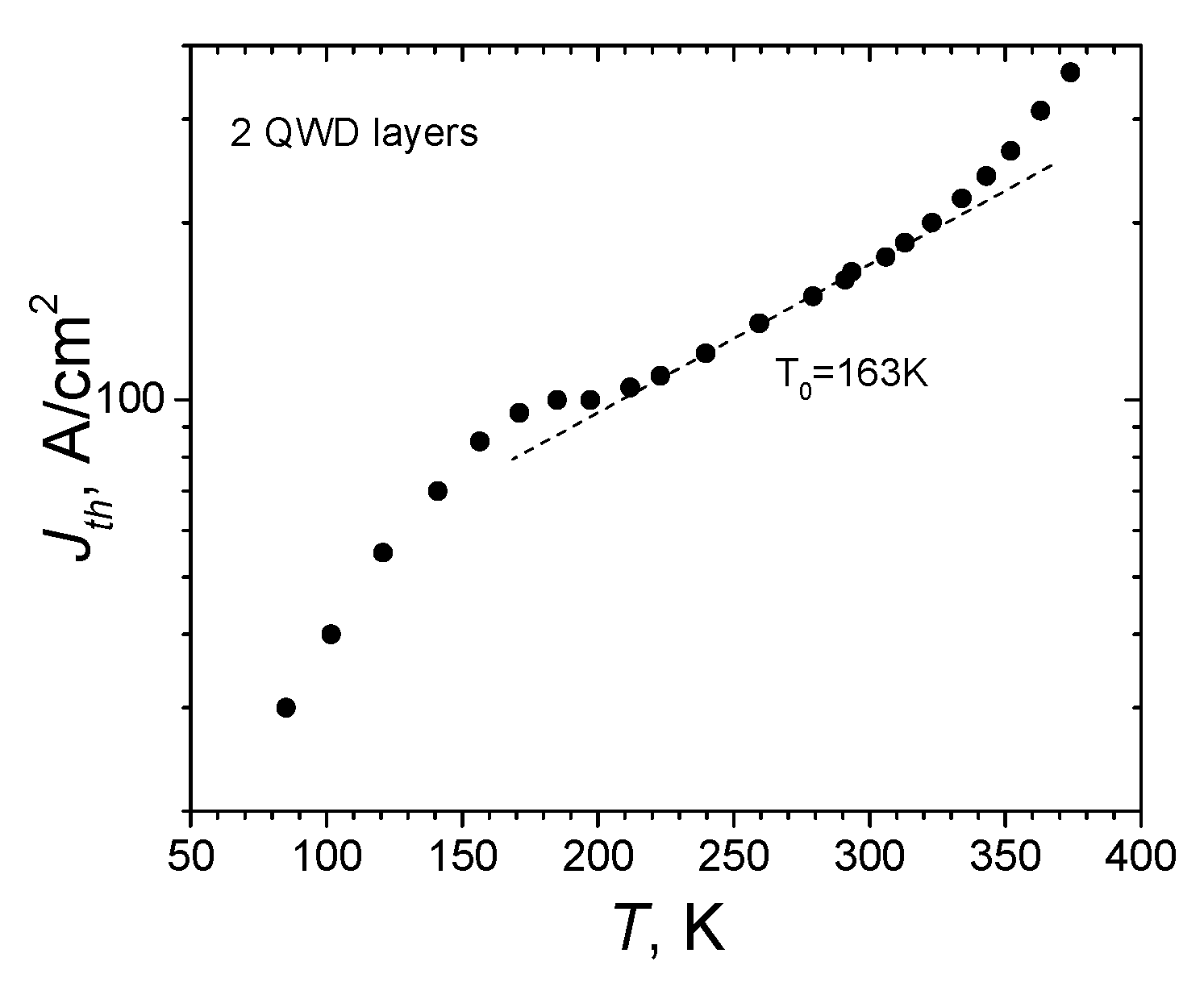
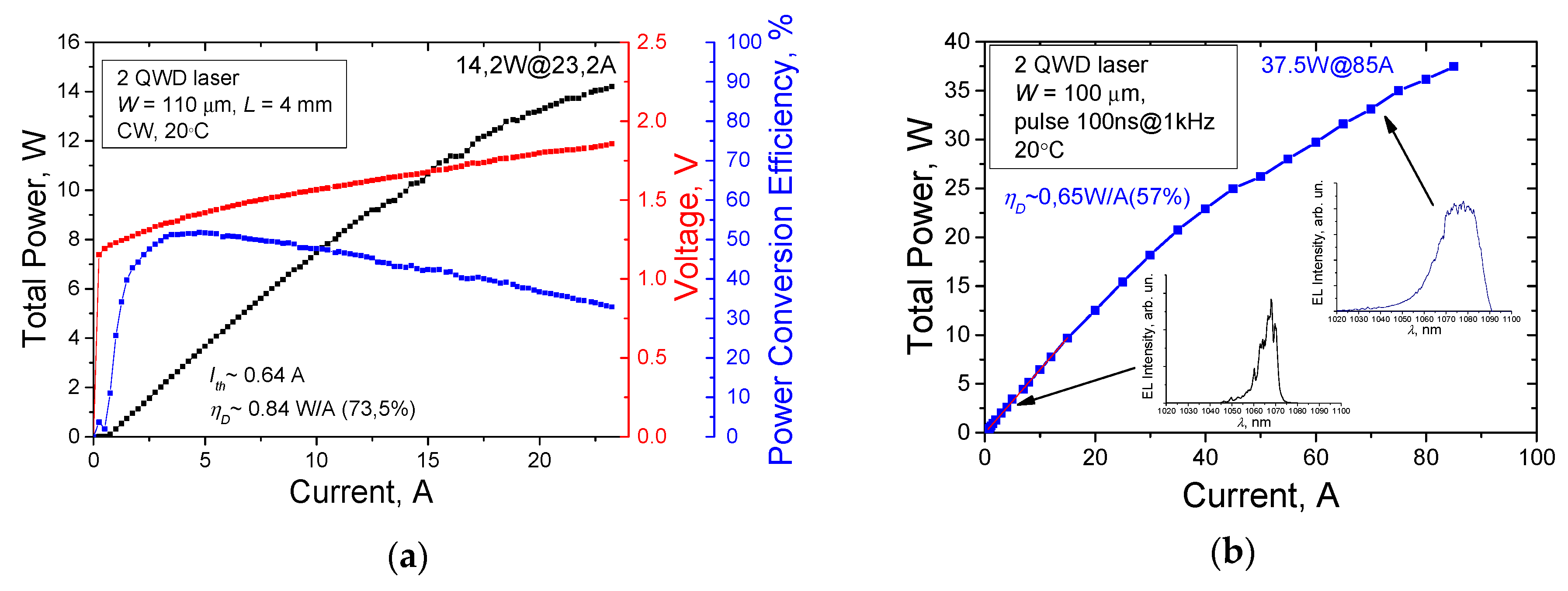
3.4. Edge Emitting Lasers
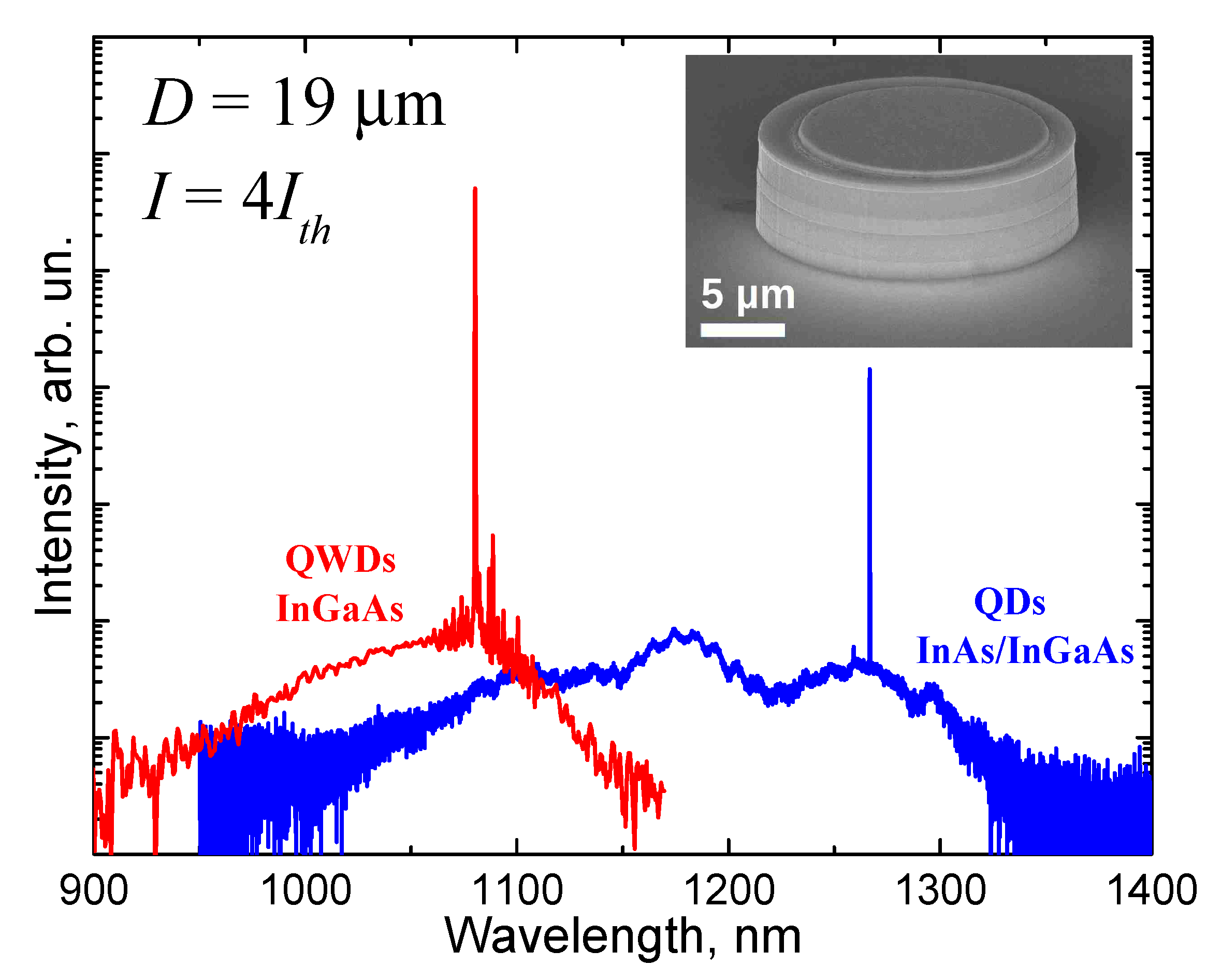
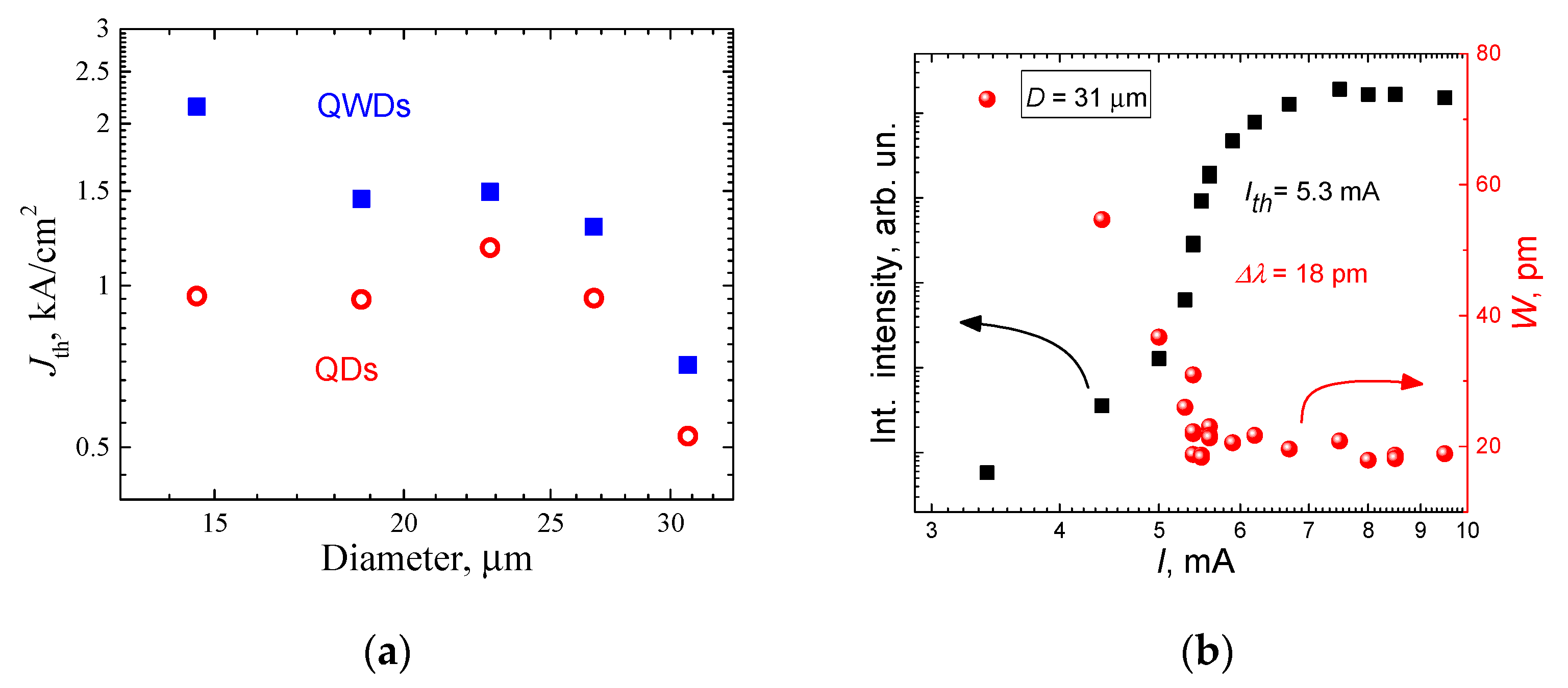
3.5. Microdisk Lasers Based on QWDs
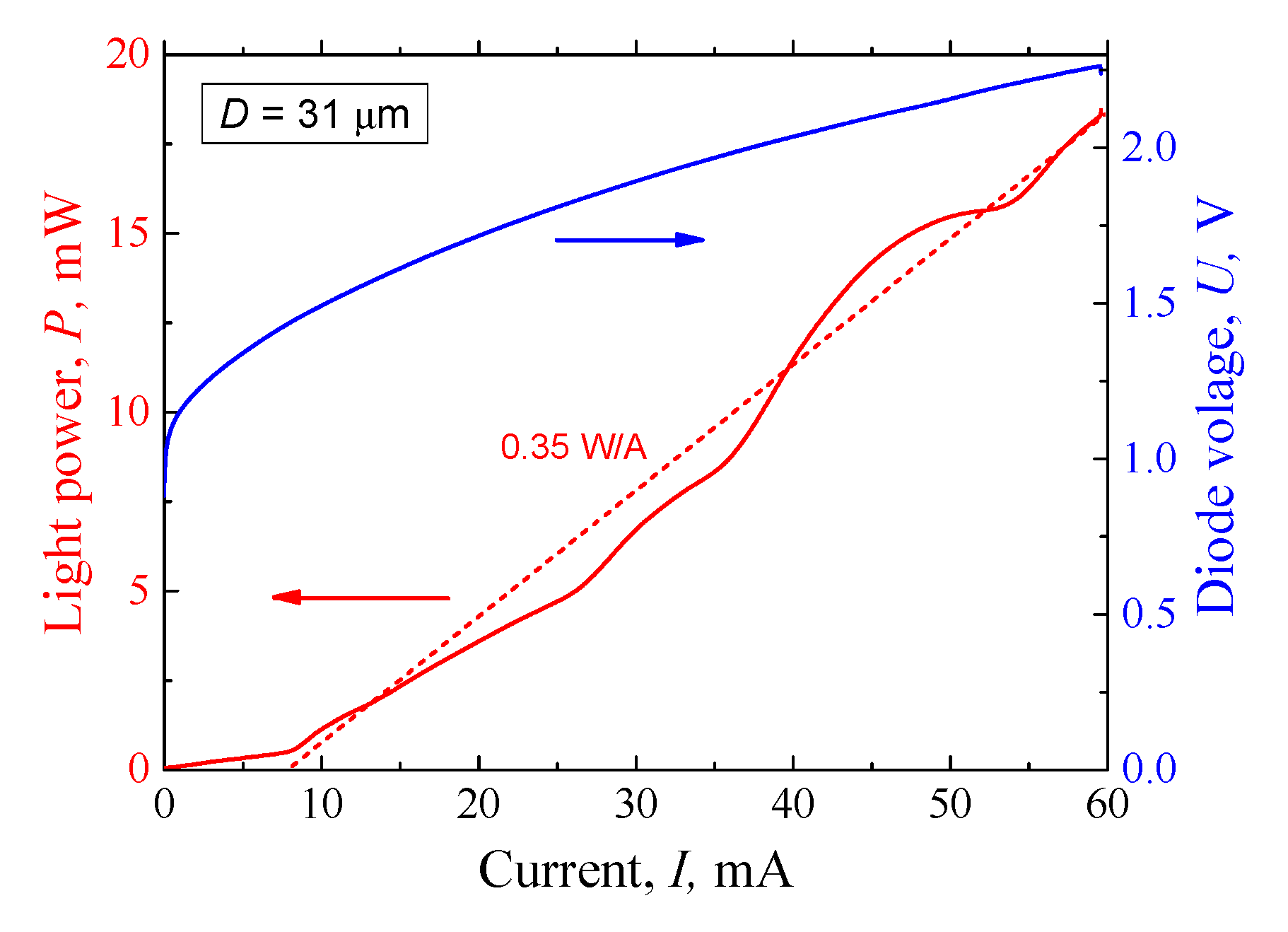
3.5.1. CW Performance
3.5.2. Dynamic Characteristics and Data Transmission
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Bimberg, D.; Grundmann, M.; Ledentsov, N.N. Quantum Dot Heterostructures; Wiley: Hoboken, NJ, USA, 1999; ISBN 978-0-471-97388-1. [Google Scholar]
- Michler, P.; Kiraz, A.; Zhang, L.; Becher, C.; Hu, E.; Imamoglu, A. Laser emission from quantum dots in microdisk structures. Appl. Phys. Lett. 2000, 77, 184–186. [Google Scholar] [CrossRef] [Green Version]
- Reitzenstein, S.; Bazhenov, A.; Gorbunov, A.; Hofmann, C.; Münch, S.; Löffler, A.; Kamp, M.; Reithmaier, J.P.; Kulakovskii, V.D.; Forchel, A. Lasing in high-Q quantum-dot micropillar cavities. Appl. Phys. Lett. 2006, 89, 051107. [Google Scholar] [CrossRef]
- Chu, D.Y.; Chin, M.K.; Sauer, N.J.; Xu, Z.; Chang, T.Y.; Ho, S.T. 1.5-mu m InGaAs/InAlGaAs quantum-well microdisk lasers. IEEE Photonics Technol. Lett. 1993, 5, 1353–1355. [Google Scholar] [CrossRef]
- Luque, A.; Martí, A. Increasing the Efficiency of Ideal Solar Cells by Photon Induced Transitions at Intermediate Levels. Phys. Rev. Lett. 1997, 78, 5014–5017. [Google Scholar] [CrossRef]
- Kailuweit, P.; Kellenbenz, R.; Philipps, S.P.; Guter, W.; Bett, A.W.; Dimroth, F. Numerical simulation and modeling of GaAs quantum-well solar cells. J. Appl. Phys. 2010, 107, 064317. [Google Scholar] [CrossRef]
- Toprasertpong, K.; Fujii, H.; Thomas, T.; Führer, M.; Alonso-Álvarez, D.; Farrell, D.J.; Watanabe, K.; Okada, Y.; Ekins-Daukes, N.J.; Sugiyama, M.; et al. Absorption threshold extended to 1.15 eV using InGaAs/GaAsP quantum wells for over-50%-efficient lattice-matched quad-junction solar cells. Prog. Photovolt. Res. Appl. 2016, 24, 533–542. [Google Scholar] [CrossRef] [Green Version]
- Ho, W.-J.; Lee, Y.-Y.; Yang, G.-C.; Chang, C.-M. Optical and electrical characteristics of high-efficiency InGaP/InGaAs/Ge triple-junction solar cell incorporated with InGaAs/GaAs QD layers in the middle cell. Prog. Photovolt. Res. Appl. 2016, 24, 551–559. [Google Scholar] [CrossRef]
- Herz, L.M.; Phillips, R.T. Fine lines from dots. Nat. Mater. 2002, 1, 212–213. [Google Scholar] [CrossRef]
- Shchukin, V.A.; Ledentsov, N.N.; Bimberg, D. Epitaxy of Nanostructures; NanoScience and Technology; Springer: Berlin/Heidelberg, Germany, 2004; ISBN 978-3-642-08735-6. [Google Scholar]
- Griffin, P.R.; Barnes, J.; Barnham, K.W.J.; Haarpaintner, G.; Mazzer, M.; Zanotti-Fregonara, C.; Grünbaum, E.; Olson, C.; Rohr, C.; David, J.P.R.; et al. Effect of strain relaxation on forward bias dark currents in GaAs/InGaAs multiquantum well p–i–n diodes. J. Appl. Phys. 1996, 80, 5815–5820. [Google Scholar] [CrossRef]
- Wu, Q.; Grober, R.D.; Gammon, D.; Katzer, D.S. Excitons, biexcitons, and electron-hole plasma in a narrow 2.8-nm GaAs/AlxGa1−xAs quantum well. Phys. Rev. B 2000, 62, 13022–13027. [Google Scholar] [CrossRef]
- Gammon, D.; Snow, E.S.; Katzer, D.S. Excited state spectroscopy of excitons in single quantum dots. Appl. Phys. Lett. 1995, 67, 2391–2393. [Google Scholar] [CrossRef]
- Micallef, J.; Li, E.H.; Weiss, B.L. The effects of strain on the confinement profile of disordered InGaAs/GaAs single quantum wells. Superlattices Microstruct. 1993, 13, 125–132. [Google Scholar] [CrossRef]
- Unsleber, S.; Deppisch, M.; Krammel, C.M.; Vo, M.; Yerino, C.D.; Simmonds, P.J.; Lee, M.L.; Koenraad, P.M.; Schneider, C.; Höfling, S. Bulk AlInAs on InP(111) as a novel material system for pure single photon emission. Opt. Express 2016, 24, 23198–23206. [Google Scholar] [CrossRef] [Green Version]
- Martini, S.; Quivy, A.; Ugarte, D.; Lange, C.; Richter, W.; Tokranov, V. Step bunching in InGaAs/GaAs quantum wells grown by molecular beam epitaxy on GaAs(001) vicinal surfaces. J. Cryst. Growth 2001, 227–228, 46–50. [Google Scholar] [CrossRef]
- De Sales, F.V.; Soler, M.A.G.; Ugarte, D.; Quivy, A.A.; Da Silva, S.W.; Martini, S.; Morais, P.C. Step-Bunching Evidence in Strained InxGa1−xAs/GaAs Quantum Wells Grown on Vicinal (001) Substrates. Phys. Status Solidi Appl. Res. 2001, 187, 253–256. [Google Scholar] [CrossRef]
- Dong, H.; Sun, J.; Ma, S.; Liang, J.; Lu, T.; Liu, X.; Xu, B. Influence of substrate misorientation on the photoluminescence and structural properties of InGaAs/GaAsP multiple quantum wells. Nanoscale 2016, 8, 6043–6056. [Google Scholar] [CrossRef]
- Fujii, H.; Watanabe, K.; Nakano, Y.; Sodabanlu, H.; Sugiyama, M.; Toprasertpong, K.; Katoh, T. Thickness-modulated InGaAs/GaAsP superlattice solar cells on vicinal substrates. J. Appl. Phys. 2015, 117, 154501. [Google Scholar] [CrossRef]
- Alonso-Álvarez, D.; Thomas, T.; Führer, M.; Hylton, N.P.; Ekins-Daukes, N.J.; Lackner, D.; Philipps, S.P.; Bett, A.W.; Sodabanlu, H.; Fujii, H.; et al. InGaAs/GaAsP strain balanced multi-quantum wires grown on misoriented GaAs substrates for high efficiency solar cells. Appl. Phys. Lett. 2014, 105, 083124. [Google Scholar] [CrossRef] [Green Version]
- Poser, F.; Bhattacharya, A.; Weeke, S.; Richter, W. Growth of spatially ordered InAs quantum dots on step-bunched vicinal GaAs (1 0 0) substrates. J. Cryst. Growth 2003, 248, 317–321. [Google Scholar] [CrossRef]
- Lee, S.; Akabori, M.; Shirahata, T.; Takada, K.; Motohisa, J.; Fukui, T. The initial stage of InGaAs growth by MOVPE on multiatomic-stepped GaAs structures. J. Cryst. Growth 2001, 231, 75–81. [Google Scholar] [CrossRef]
- alhuda Al Saqri, N.; Felix, J.F.; Aziz, M.; Kunets, V.P.; Jameel, D.; Taylor, D.; Henini, M.; Abd El-sadek, M.S.; Furrow, C.; Ware, M.E.; et al. Investigation of electrically active defects in InGaAs quantum wire intermediate-band solar cells using deep-level transient spectroscopy technique. Nanotechnology 2017, 28, 045707. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kunets, V.P.; Furrow, C.S.; Morgan, T.A.; Hirono, Y.; Ware, M.E.; Dorogan, V.G.; Mazur, Y.I.; Kunets, V.P.; Salamo, G.J. InGaAs quantum wire intermediate band solar cell. Appl. Phys. Lett. 2012, 101, 041106. [Google Scholar] [CrossRef]
- Sugiyama, M.; Fujii, H.; Katoh, T.; Toprasertpong, K.; Sodabanlu, H.; Watanabe, K.; Alonso-Álvarez, D.; Ekins-Daukes, N.J.; Nakano, Y. Quantum wire-on-well (WoW) cell with long carrier lifetime for efficient carrier transport. Prog. Photovolt. Res. Appl. 2016, 24, 1606–1614. [Google Scholar] [CrossRef] [Green Version]
- Cho, H.; Toprasertpong, K.; Sodabanlu, H.; Watanabe, K.; Sugiyama, M.; Nakano, Y. Stability and controllability of InGaAs/GaAsP wire-on-well (WoW) structure for multi-junction solar cells. J. Cryst. Growth 2017, 464, 86–93. [Google Scholar] [CrossRef]
- Yu, Q.; Li, X.; Jia, Y.; Lu, W.; Zheng, M.; Zhang, X.; Ning, Y.; Wu, J. InGaAs-Based Well-Island Composite Quantum-Confined Structure with Superwide and Uniform Gain Distribution for Great Enhancement of Semiconductor Laser Performance. ACS Photonics 2018, 5, 4896–4902. [Google Scholar] [CrossRef]
- Talalaev, V.G.; Novikov, B.V.; Verbin, S.Y.; Novikov, A.B.; Thath, D.S.; Shchur, I.V.; Gobsch, G.; Goldhahn, R.; Stein, N.; Golombek, A.; et al. Recombination emission from InAs quantum dots grown on vicinal GaAs surfaces. Semiconductors 2000, 34, 453–461. [Google Scholar] [CrossRef]
- Evtikhiev, V.P.; Tokranov, V.E.; Kryzhanovskii, A.K.; Boiko, A.M.; Suris, R.A.; Titkov, A.N.; Nakamura, A.; Ichida, M. Growth of InAs quantum dots on vicinal GaAs(001) surfaces misoriented in the [010] direction. Semiconductors 1998, 32, 765–769. [Google Scholar] [CrossRef]
- Yazdanpanah, V.; Wang, Z.M.; Lee, J.H.; Salamo, G.J. Structural evolution in strained In0.18Ga0.82As stacking multilayers on vicinal GaAs surfaces. New J. Phys. 2006, 8, 233. [Google Scholar] [CrossRef] [Green Version]
- Ledentsov, N.N.; Bimberg, D.; Shernyakov, Y.M.; Kochnev, V.; Maximov, M.V.; Sakharov, A.V.; Krestnikov, I.L.; Egorov, A.Y.; Zhukov, A.E.; Tsatsul’nikov, A.F.; et al. Properties of strained (In, Ga, Al)As lasers with laterally modulated active region. Appl. Phys. Lett. 1997, 70, 2888–2890. [Google Scholar] [CrossRef]
- Mintairov, S.A.; Kalyuzhnyy, N.A.; Maximov, M.V.; Nadtochiy, A.M.; Rouvimov, S.; Zhukov, A.E. GaAs quantum well-dots solar cells with spectral response extended to 1100 nm. Electron. Lett. 2015, 51, 1602–1604. [Google Scholar] [CrossRef]
- Löffler, A.; Reithmaier, J.P.; Forchel, A.; Sauerwald, A.; Peskes, D.; Kümmell, T.; Bacher, G. Influence of the strain on the formation of GaInAs/GaAs quantum structures. J. Cryst. Growth 2006, 286, 6–10. [Google Scholar] [CrossRef]
- Hubbard, S.M.; Podell, A.; Mackos, C.; Polly, S.; Bailey, C.G.; Forbes, D.V. Effect of vicinal substrates on the growth and device performance of quantum dot solar cells. Sol. Energy Mater. Sol. Cells 2013, 108, 256–262. [Google Scholar] [CrossRef]
- Bhattacharya, A.; Mawst, L.J.; Nayak, S.; Li, J.; Kuech, T.F. Interface structures of InGaAs/InGaAsP/InGaP quantum well laser diodes grown by metalorganic chemical vapor deposition on GaAs substrates. Appl. Phys. Lett. 1996, 68, 2240–2242. [Google Scholar] [CrossRef]
- Nadtochiy, A.M.; Maximov, M.V.; Mintairov, S.A.; Kalyuzhnyy, N.A.; Nevedomskiy, V.N.; Rouvimov, S.S.; Zhukov, A.E. Gradual Evolution From Quantum-Well-Like to Quantum-Dot-Like Characteristics in InGaAs/GaAs Nanostructures. Phys. Status Solidi 2018, 255, 1800123. [Google Scholar] [CrossRef]
- De Sales, F.V.; Soler, M.A.G.; Ugarte, D.; Abramof, E.; Quivy, A.A.; Da Silva, S.W.; Martini, S.; Morais, P.C.; Leite, J.R. Investigation of optical and structural properties of InxGa1−xAs/GaAs quantum wells grown on vicinal GaAs(0 0 1) substrates. Phys. B Condens. Matter 2002, 311, 285–291. [Google Scholar] [CrossRef]
- Strand, T.A.; Thibeault, B.J.; Coldren, L.A. Reduced lateral carrier diffusion for improved miniature semiconductor lasers. J. Appl. Phys. 1997, 81, 3377–3381. [Google Scholar] [CrossRef]
- Kim, J.K.; Naone, R.L.; Coldren, L.A. Lateral carrier confinement in miniature lasers using quantum dots. IEEE J. Sel. Top. Quantum Electron. 2000, 6, 504–510. [Google Scholar] [CrossRef]
- Naone, R.L.; Floyd, P.D.; Young, D.B.; Hegblom, E.R.; Strand, T.A.; Coldren, L.A. Interdiffused quantum wells for lateral carrier confinement in VCSELs. IEEE J. Sel. Top. Quantum Electron. 1998, 4, 706–714. [Google Scholar] [CrossRef]
- Gérard, J.M.; Cabrol, O.; Sermage, B. InAs quantum boxes: Highly efficient radiative traps for light emitting devices on Si. Appl. Phys. Lett. 1996, 68, 3123–3125. [Google Scholar] [CrossRef]
- Piva, P.G.; Goldberg, R.D.; Mitchell, I.V.; Labrie, D.; Leon, R.; Charbonneau, S.; Wasilewski, Z.R.; Fafard, S. Enhanced degradation resistance of quantum dot lasers to radiation damage. Appl. Phys. Lett. 2000, 77, 624–626. [Google Scholar] [CrossRef] [Green Version]
- Ribbat, C.; Sellin, R.; Grundmann, M.; Bimberg, D.; Sobolev, N.A.; Carmo, M.C. Enhanced radiation hardness of quantum dot lasers to high energy proton irradiation. Electron. Lett. 2001, 37, 174–175. [Google Scholar] [CrossRef]
- Arzberger, M.; Böhm, G.; Amann, M.-C.; Abstreiter, G. Continuous room-temperature operation of electrically pumped quantum-dot microcylinder lasers. Appl. Phys. Lett. 2001, 79, 1766–1768. [Google Scholar] [CrossRef]
- Luo, K.J.; Xu, J.Y.; Cao, H.; Ma, Y.; Chang, S.H.; Ho, S.T.; Solomon, G.S. Ultrafast dynamics of InAs/GaAs quantum-dot microdisk lasers. Appl. Phys. Lett. 2001, 78, 3397–3399. [Google Scholar] [CrossRef]
- Fiore, A.; Chen, J.X.; Ilegems, M. Scaling quantum-dot light-emitting diodes to submicrometer sizes. Appl. Phys. Lett. 2002, 81, 1756–1758. [Google Scholar] [CrossRef] [Green Version]
- Kryzhanovskaya, N.V.; Zhukov, A.E.; Maximov, M.V.; Moiseev, E.I.; Shostak, I.I.; Nadtochiy, A.M.; Kudashova, Y.V.; Lipovskii, A.A.; Kulagina, M.M.; Troshkov, S.I. Room Temperature Lasing in 1-μm Microdisk Quantum Dot Lasers. IEEE J. Sel. Top. Quantum Electron. 2015, 21, 709–713. [Google Scholar] [CrossRef]
- Zeng, K.C.; Lin, J.Y.; Jiang, H.X. Effects of alloy disorder on the transport properties of AlxGa1−xN epilayers probed by persistent photoconductivity. Appl. Phys. Lett. 2000, 76, 1728–1730. [Google Scholar] [CrossRef] [Green Version]
- Kurtz, S.R.; Klem, J.F.; Allerman, A.A.; Sieg, R.M.; Seager, C.H.; Jones, E.D. Minority carrier diffusion and defects in InGaAsN grown by molecular beam epitaxy. Appl. Phys. Lett. 2002, 80, 1379–1381. [Google Scholar] [CrossRef]
- Maximov, M.V.; Volovik, B.V.; Sotomayor Torres, C.M.; Ramushina, E.M.; Skopina, V.I.; Tanklevskaya, E.M.; Gurevich, S.A.; Ustinov, V.M.; Alferov, Z.I.; Ledentsov, N.N.; et al. Impact of Carrier Lateral Transport and Surface Recombination on the PL Efficiency of Mesas with Self-Organized Quantum Dots. Phys. Status Solidi 2001, 188, 955–959. [Google Scholar] [CrossRef]
- Maximov, M.V.; Tsatsul’nikov, A.F.; Volovik, B.V.; Sizov, D.S.; Shernyakov, Y.M.; Kaiander, I.N.; Zhukov, A.E.; Kovsh, A.R.; Mikhrin, S.S.; Ustinov, V.M.; et al. Tuning quantum dot properties by activated phase separation of an InGa(Al)As alloy grown on InAs stressors. Phys. Rev. B 2000, 62, 16671–16680. [Google Scholar] [CrossRef]
- Fiore, A.; Rossetti, M.; Alloing, B.; Paranthoen, C.; Chen, J.X.; Geelhaar, L.; Riechert, H. Carrier diffusion in low-dimensional semiconductors: A comparison of quantum wells, disordered quantum wells, and quantum dots. Phys. Rev. B 2004, 70, 205311. [Google Scholar] [CrossRef] [Green Version]
- Nadtochiy, A.M.; Mintairov, S.A.; Kalyuzhnyy, N.A.; Maximov, M.V.; Sannikov, D.A.; Yagafarov, T.F.; Zhukov, A.E. Time-Resolved Photoluminescence of InGaAs Nanostructures Different in Quantum Dimensionality. Semiconductors 2019, 53, 1489–1495. [Google Scholar] [CrossRef]
- Heitz, R.; Born, H.; Lüttgert, T.; Hoffmann, A.; Bimberg, D. Resonantly Excited Time-Resolved Photoluminescence Study of Self-Organized InGaAs/GaAs Quantum Dots. Phys. Status Solidi 2000, 221, 65–70. [Google Scholar] [CrossRef]
- Markus, A.; Fiore, A.; Ganière, J.D.; Oesterle, U.; Chen, J.X.; Deveaud, B.; Ilegems, M.; Riechert, H. Comparison of radiative properties of InAs quantum dots and GaInNAs quantum wells emitting around 1.3 μm. Appl. Phys. Lett. 2002, 80, 911–913. [Google Scholar] [CrossRef] [Green Version]
- Nasr, O.; Chauvin, N.; Alouane, M.H.H.; Maaref, H.; Bru-Chevallier, C.; Sfaxi, L.; Ilahi, B. Carrier dynamics of strain-engineered InAs quantum dots with (In)GaAs surrounding material. J. Opt. 2017, 19, 025401. [Google Scholar] [CrossRef]
- Karachinsky, L.Y.; Pellegrini, S.; Buller, G.S.; Shkolnik, A.S.; Gordeev, N.Y.; Evtikhiev, V.P.; Novikov, V.B. Time-resolved photoluminescence measurements of InAs self-assembled quantum dots grown on misorientated substrates. Appl. Phys. Lett. 2004, 84, 7–9. [Google Scholar] [CrossRef] [Green Version]
- Pellegrini, S.; Buller, G.S.; Karachinsky, L.Y.; Shkolnik, A.S.; Gordeev, N.Y.; Zegrya, G.G.; Evtikhiev, V.P.; Sellers, I.R.; Skolnick, M.S.; Liu, H.Y.; et al. Time-resolved photoluminescence measurements of InAs self-assembled quantum dots (Invited Paper). In Proceedings of the Ultrafast Phenomena in Semiconductors and Nanostructure Materials IX, San Francisco, CA, USA, 20 April 2011. [Google Scholar]
- Gurioli, M.; Vinattieri, A.; Colocci, M.; Deparis, C.; Massies, J.; Neu, G.; Bosacchi, A.; Franchi, S. Temperature dependence of the radiative and nonradiative recombination time in GaAs/AlxGa1−xAs quantum-well structures. Phys. Rev. B 1991, 44, 3115–3124. [Google Scholar] [CrossRef]
- Maximov, M.V.; Zhukov, A.E. Quantum Dot Lasers. In Dekker Encyclopedia of Nanoscience and Nanotechnology; CRC: Boca Raton, FL, USA, 2014. [Google Scholar]
- Browne, B.; Lacey, J.; Tibbits, T.; Bacchin, G.; Wu, T.-C.; Liu, J.Q.; Chen, X.; Rees, V.; Tsai, J.; Werthen, J.-G. Triple-junction quantum-well solar cells in commercial production. In Proceedings of the AIP Conference Proceedings, Tallahassee, FL, USA, 1–2 August 2012; pp. 3–5. [Google Scholar] [CrossRef] [Green Version]
- Fujii, H.; Toprasertpong, K.; Wang, Y.; Watanabe, K.; Sugiyama, M.; Nakano, Y. 100-period, 1.23-eV bandgap InGaAs/GaAsP quantum wells for high-efficiency GaAs solar cells: Toward current-matched Ge-based tandem cells. Prog. Photovolt. Res. Appl. 2014, 22, 784–795. [Google Scholar] [CrossRef]
- Bailey, C.G.; Forbes, D.V.; Polly, S.J.; Bittner, Z.S.; Dai, Y.; Mackos, C.; Raffaelle, R.P.; Hubbard, S.M. Open-Circuit Voltage Improvement of InAs/GaAs Quantum-Dot Solar Cells Using Reduced InAs Coverage. IEEE J. Photovolt. 2012, 2, 269–275. [Google Scholar] [CrossRef]
- Forbes, D.V.; Hubbard, S.M.; Bailey, C.; Polly, S.; Andersen, J.; Raffaelle, R. III-V Quantum Dot Enhanced Photovoltaic Devices. In Proceedings of the Next Generation (Nano) Photonic and Cell Technologies for Solar Energy Conversion, San Diego, CA, USA, 1–5 August 2010; Tsakalakos, L., Ed.; p. 77720C. [Google Scholar] [CrossRef]
- Kalyuzhnyy, N.A.; Mintairov, S.A.; Salii, R.A.; Nadtochiy, A.M.; Payusov, A.S.; Brunkov, P.N.; Nevedomsky, V.N.; Shvarts, M.Z.; Martí, A.; Andreev, V.M.; et al. Increasing the quantum efficiency of InAs/GaAs QD arrays for solar cells grown by MOVPE without using strain-balance technology. Prog. Photovolt. Res. Appl. 2016, 24. [Google Scholar] [CrossRef] [Green Version]
- Weber, H.; Loosen, P.; Poprawe, R. (Eds.) Landolt-Börnstein—Group VIII Advanced Materials and Tec hnologies. In Laser Systems; Springer: Berlin/Heidelberg, Germany, 2011; Volume 3, ISBN 978-3-642-14176-8. [Google Scholar]
- Zhukov, A.E.; Maksimov, M.V.; Kovsh, A.R. Device characteristics of long-wavelength lasers based on self-organized quantum dots. Semiconductors 2012, 46, 1225–1250. [Google Scholar] [CrossRef]
- Adams, A.R. Strained-Layer Quantum-Well Lasers. IEEE J. Sel. Top. Quantum Electron. 2011, 17, 1364–1373. [Google Scholar] [CrossRef]
- Serin, A.A.; Payusov, A.S.; Shernyakov, Y.M.; Kalyuzhnyy, N.A.; Mintairov, S.A.; Maximov, M.V.; Gordeev, N.Y.; Zhukov, A.E. Reducing of thermal resistance of edge-emitting lasers based on coupled waveguides. J. Phys. Conf. Ser. 2018, 1124, 041016. [Google Scholar] [CrossRef]
- Rauch, S.; Wenzel, H.; Radziunas, M.; Haas, M.; Tränkle, G.; Zimer, H. Impact of longitudinal refractive index change on the near-field width of high-power broad-area diode lasers. Appl. Phys. Lett. 2017, 110, 263504. [Google Scholar] [CrossRef]
- Nadtochiy, A.M.; Maximov, M.V.; Rouvimov, S.S.; Zhukov, A.E.; Shernyakov, Y.M.; Payusov, A.S.; Kalyuzhnyy, N.A.; Mintairov, S.A. Effect of the bimodality of a QD array on the optical properties and threshold characteristics of QD lasers. Semiconductors 2015, 49, 1090–1094. [Google Scholar] [CrossRef]
- Gordeev, N.Y.; Payusov, A.S.; Shernyakov, Y.M.; Mintairov, S.A.; Kalyuzhnyy, N.A.; Kulagina, M.M.; Maximov, M.V. Transverse single-mode edge-emitting lasers based on coupled waveguides. Opt. Lett. 2015, 40, 2150–2152. [Google Scholar] [CrossRef]
- Pietrzak, A.; Zorn, M.; Huelsewede, R.; Meusel, J.; Sebastian, J. Development of highly-efficient laser diodes emitting around 1060nm for medical and industrial applications. In Proceedings of the High-Power Diode Laser Technology XVII, San Francisco, CA, USA, 2–7 February 2019; Zediker, M.S., Ed.; p. 17. [Google Scholar] [CrossRef]
- McCall, S.L.; Levi, A.F.J.; Slusher, R.E.; Pearton, S.J.; Logan, R.A. Whispering-gallery mode microdisk lasers. Appl. Phys. Lett. 1992, 60, 289–291. [Google Scholar] [CrossRef]
- Borselli, M.; Johnson, T.J.; Painter, O. Beyond the Rayleigh scattering limit in high-Q silicon microdisks: Theory and experiment. Opt. Express 2005, 13, 1515–1530. [Google Scholar] [CrossRef] [Green Version]
- Stock, E.; Albert, F.; Hopfmann, C.; Lermer, M.; Schneider, C.; Höfling, S.; Forchel, A.; Kamp, M.; Reitzenstein, S. On-Chip Quantum Optics with Quantum Dot Microcavities. Adv. Mater. 2013, 25, 707–710. [Google Scholar] [CrossRef]
- Srinivasan, K.; Borselli, M.; Painter, O.; Stintz, A.; Krishna, S. Cavity Q, mode volume, and lasing threshold in small diameter AlGaAs microdisks with embedded quantum dots. Opt. Express 2006, 14, 1094–1105. [Google Scholar] [CrossRef] [Green Version]
- Zubov, F.I.; Kryzhanovskaya, N.V.; Moiseev, E.I.; Polubavkina, Y.S.; Simchuk, O.I.; Kulagina, M.M.; Zadiranov, Y.M.; Troshkov, S.I.; Lipovskii, A.A.; Maximov, M.V.; et al. Laser characteristics of an injection microdisk with quantum dots and its free-space outcoupling efficiency. Semiconductors 2016, 50, 1408–1411. [Google Scholar] [CrossRef]
- Moiseev, E.; Kryzhanovskaya, N.; Maximov, M.; Zubov, F.; Nadtochiy, A.; Kulagina, M.; Zadiranov, Y.; Kalyuzhnyy, N.; Mintairov, S.; Zhukov, A. Highly efficient injection microdisk lasers based on quantum well-dots. Opt. Lett. 2018, 43, 4554–4557. [Google Scholar] [CrossRef] [PubMed]
- Kryzhanovskaya, N.V.; Lebedev, M.V.; L’vova, T.V.; Kudashova, Y.V.; Shostak, I.I.; Moiseev, E.I.; Zhukov, A.E.; Maximov, M.V.; Kulagina, M.M.; Nadtochiy, A.M.; et al. The effect of sulfide passivation on luminescence from microdisks with quantum wells and quantum dots. Tech. Phys. Lett. 2015, 41, 654–657. [Google Scholar] [CrossRef]
- Liu, L.; Roelkens, G.; Van Campenhout, J.; Brouckaert, J.; Van Thourhout, D.; Baets, R. III–V/Silicon-on-Insulator Nanophotonic Cavities for Optical Network-on-Chip. J. Nanosci. Nanotechnol. 2010, 10, 1461–1472. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hofrichter, J.; Raz, O.; Keyvaninia, S.; de Vries, T.; Dorren, H.J.S.; Morf, T.; Offrein, B.J. High-Speed Direct-Modulation of InP Microdisk Lasers. In Proceedings of the 39th European Conference and Exhibition on Optical Communication (ECOC 2013), London, UK, 22–26 September 2013; pp. 351–353. [Google Scholar] [CrossRef]
- Lv, X.-M.; Huang, Y.-Z.; Zou, L.-X.; Long, H.; Du, Y. Optimization of direct modulation rate for circular microlasers by adjusting mode Q factor. Laser Photon. Rev. 2013, 7, 818–829. [Google Scholar] [CrossRef]
- Yang, Y.-D.; Zhang, Y.; Huang, Y.-Z.; Poon, A.W. Direct-modulated waveguide-coupled microspiral disk lasers with spatially selective injection for on-chip optical interconnects. Opt. Express 2014, 22, 824–838. [Google Scholar] [CrossRef]
- Zou, L.-X.; Huang, Y.-Z.; Liu, B.-W.; Lv, X.-M.; Ma, X.-W.; Yang, Y.-D.; Xiao, J.-L.; Du, Y. Thermal and high speed modulation characteristics for AlGaInAs/InP microdisk lasers. Opt. Express 2015, 23, 2879–2888. [Google Scholar] [CrossRef]
- Kapsalis, A.; Syvridis, D.; Troppenz, U.; Hamacher, M.; Heidrich, H. 7Gb/s Direct Modulation of Vertically Coupled Microring Lasers. In Proceedings of the Optical Fiber Communication Conference/National Fiber Optic Engineers Conference, San Diego, CA, USA, 24–28 February 2008. [Google Scholar]
- Sokół, A.K.; Sarzała, R.P. Comparative analysis of thermal problems in GaAs- and InP-based 1.3-μm VECSELs. Opt. Appl. 2013, 43, 325–341. [Google Scholar] [CrossRef]
- Mao, M.-H.; Chien, H.-C. Transient behaviors of current-injection quantum-dot microdisk lasers. Opt. Express 2012, 20, 3302–3310. [Google Scholar] [CrossRef]
- Zubov, F.; Maximov, M.; Kryzhanovskaya, N.; Moiseev, E.; Muretova, M.; Mozharov, A.; Kaluzhnyy, N.; Mintairov, S.; Kulagina, M.; Ledentsov, N.; et al. High speed data transmission using directly modulated microdisk lasers based on InGaAs/GaAs quantum well-dots. Opt. Lett. 2019, 44, 5442–5445. [Google Scholar] [CrossRef]
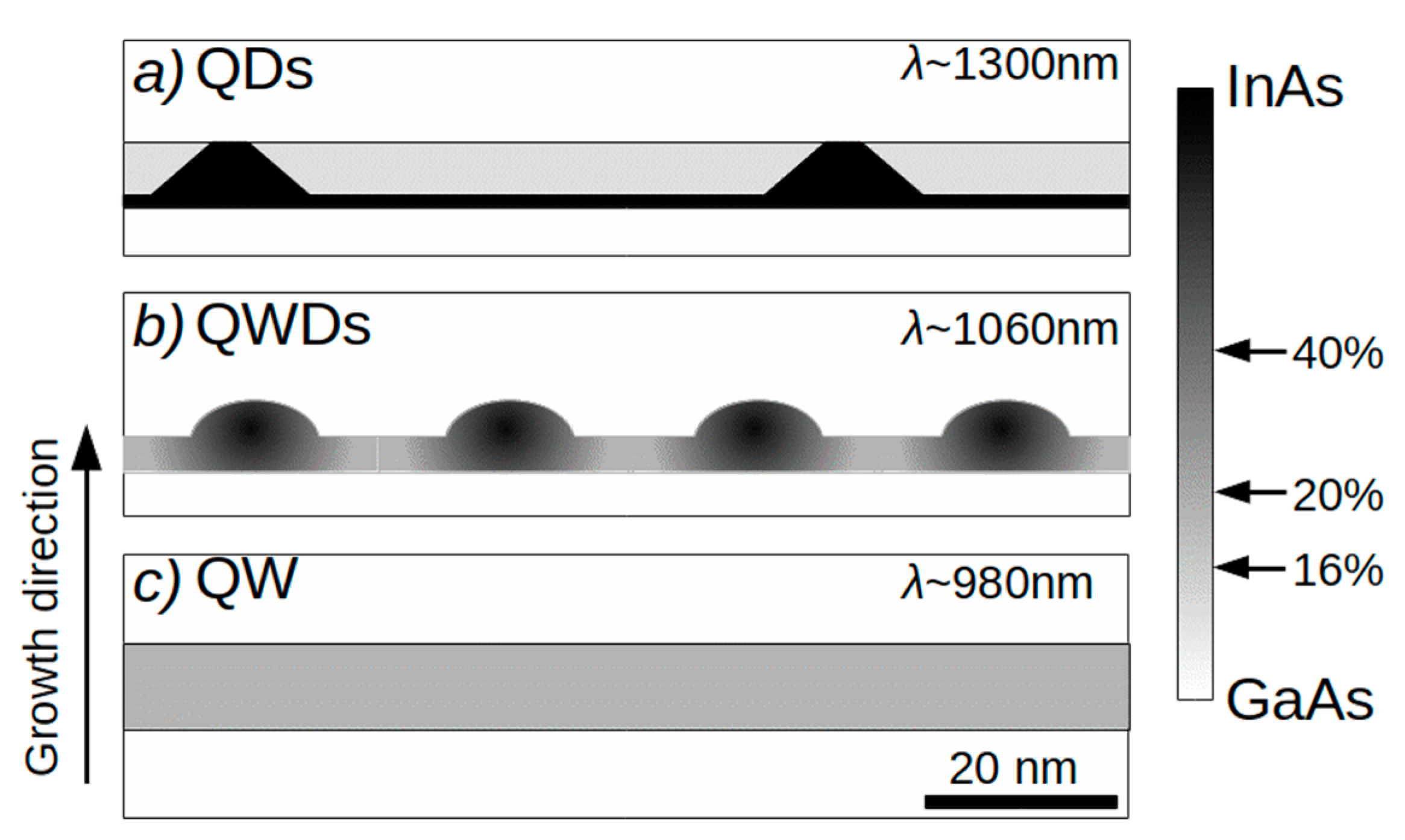
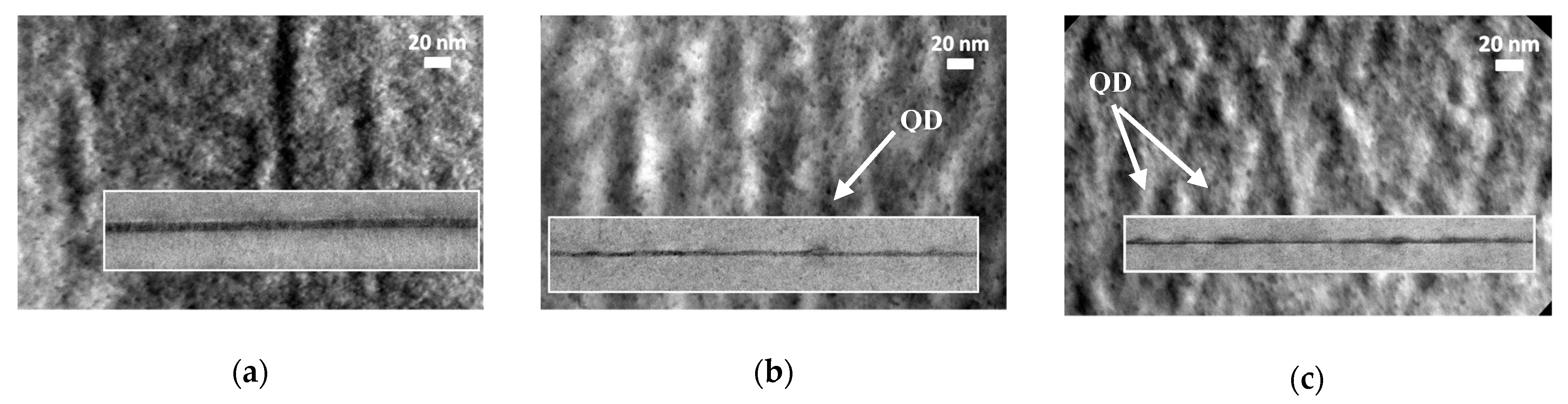






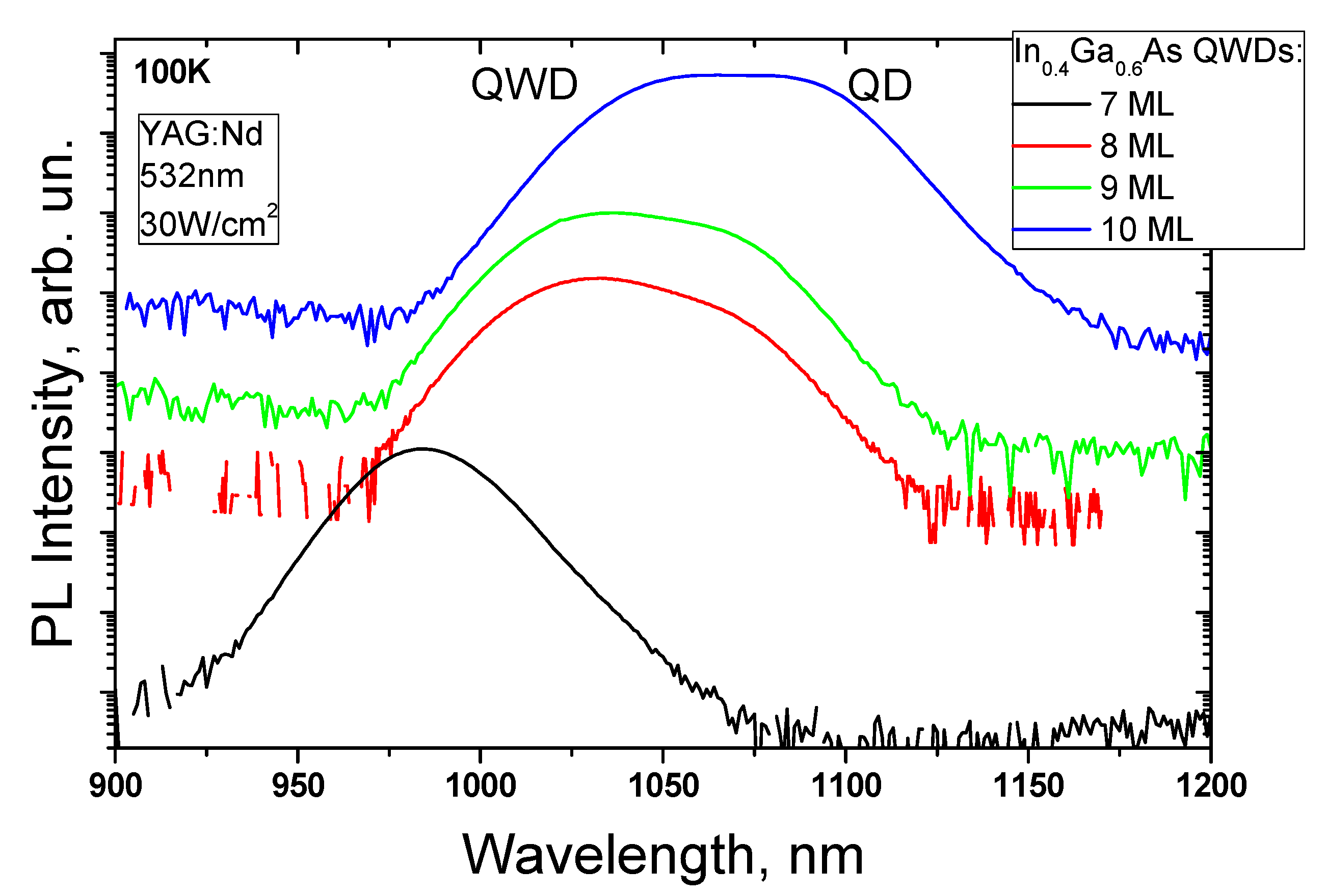




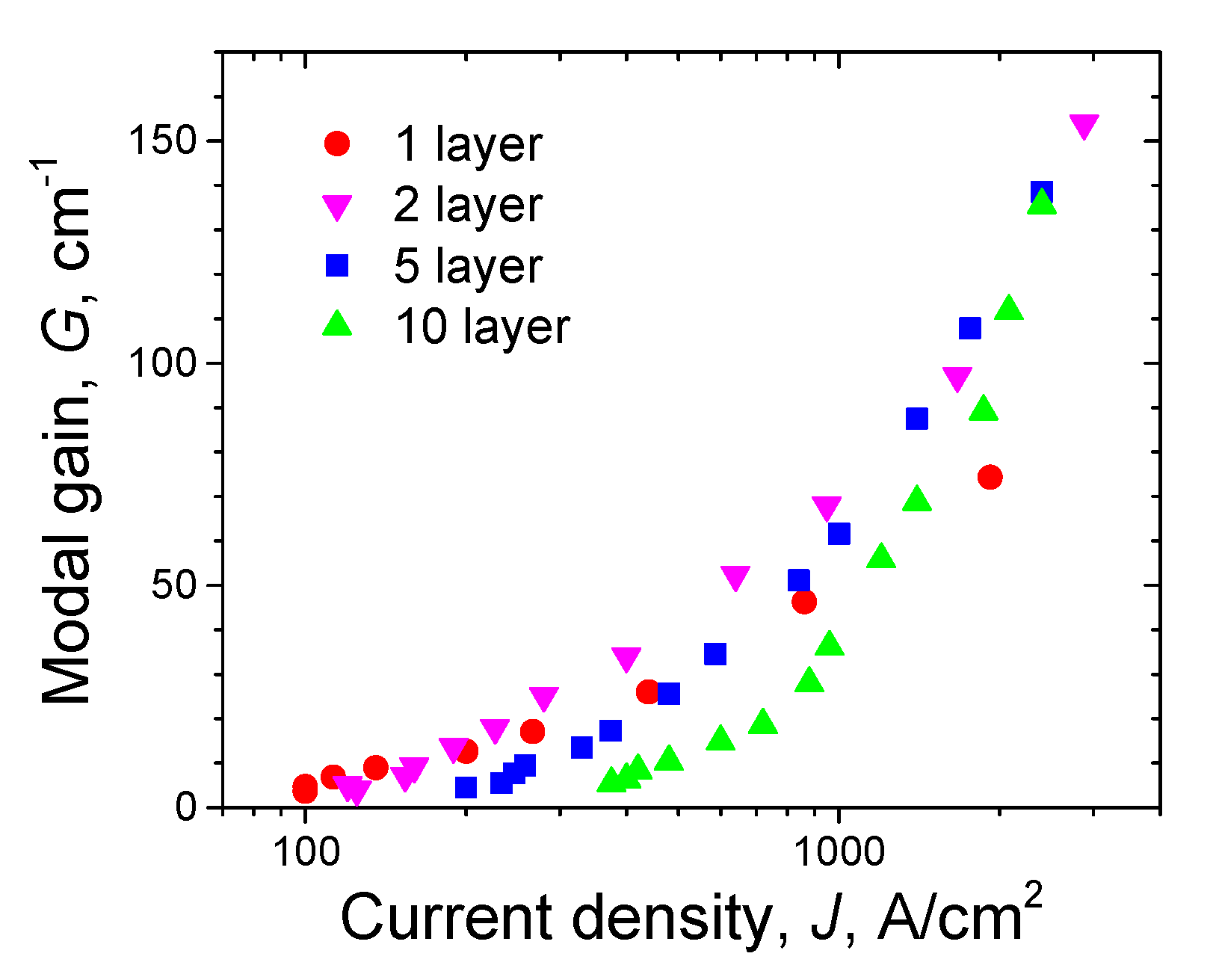



| Properties | InGaAs/GaAs QWs | In(Ga)As/GaAs QDs |
|---|---|---|
| Carrier localization | 2D | 0D |
| Maximal emission wavelength | 1100 nm | 1350 nm |
| Optical gain/absorption per layer | >20 cm−1 | 3–8 cm−1 |
| Carrier lateral diffusion length | Several microns | ~100 nm |
| Number of stacked layers (without strain compensation) | 3 (for λ ~ 1 μm) | 15–20 (for λ 1.1–1.3 μm) |
| Carrier capture time | ~ps or less | few ps |
| Number of QWD Layers | Threshold Current Density, A/cm2 | Lasing Wavelength (λ), nm | Internal Quantum Efficiency (ηi) | Internal Loss (αi), cm−1 | |
|---|---|---|---|---|---|
| Total (jth) | Per QWD Layer (jQWD) | ||||
| 1 | 100 | 100 | 1088 | 0.79 | 0.7 |
| 2 | 120 | 60 | 1092 | 0.85 | 1.0 |
| 5 | 200 | 40 | 1108 | 0.82 | 1.5 |
| 10 | 375 | 38 | 1117 | 0.78 | 2.4 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Maximov, M.V.; Nadtochiy, A.M.; Mintairov, S.A.; Kalyuzhnyy, N.A.; Kryzhanovskaya, N.V.; Moiseev, E.I.; Gordeev, N.Y.; Shernyakov, Y.M.; Payusov, A.S.; Zubov, F.I.; et al. Light Emitting Devices Based on Quantum Well-Dots. Appl. Sci. 2020, 10, 1038. https://doi.org/10.3390/app10031038
Maximov MV, Nadtochiy AM, Mintairov SA, Kalyuzhnyy NA, Kryzhanovskaya NV, Moiseev EI, Gordeev NY, Shernyakov YM, Payusov AS, Zubov FI, et al. Light Emitting Devices Based on Quantum Well-Dots. Applied Sciences. 2020; 10(3):1038. https://doi.org/10.3390/app10031038
Chicago/Turabian StyleMaximov, Mikhail V., Alexey M. Nadtochiy, Sergey A. Mintairov, Nikolay A. Kalyuzhnyy, Natalia V. Kryzhanovskaya, Eduard I. Moiseev, Nikita Yu. Gordeev, Yuriy M. Shernyakov, Alexey S. Payusov, Fedor I. Zubov, and et al. 2020. "Light Emitting Devices Based on Quantum Well-Dots" Applied Sciences 10, no. 3: 1038. https://doi.org/10.3390/app10031038
APA StyleMaximov, M. V., Nadtochiy, A. M., Mintairov, S. A., Kalyuzhnyy, N. A., Kryzhanovskaya, N. V., Moiseev, E. I., Gordeev, N. Y., Shernyakov, Y. M., Payusov, A. S., Zubov, F. I., Nevedomskiy, V. N., Rouvimov, S. S., & Zhukov, A. E. (2020). Light Emitting Devices Based on Quantum Well-Dots. Applied Sciences, 10(3), 1038. https://doi.org/10.3390/app10031038




