The simulation test of the impact overload environment requires the axial acceleration of the unit being tested to reach the set value within 0.2 s, which is the typical characteristics of instantaneous and high impacts. When the unit being tested is driven by the centrifuge, its axial direction will point toward the axis of the arm. The normal and tangential directions of the arm are the axial and radial directions of the unit being tested. When the centrifuge accelerates, the normal acceleration of the arm increases, and the tangential acceleration fluctuates. When the centrifuge is running at a constant speed, the normal acceleration of the arm is constant and the tangential acceleration is zero g, which meet the requirements of the acceleration amplitude of the impact loading environment. However, the acceleration time lasts nearly 1 s, failing to meet the impact loading requirements. To tackle this problem, we propose a simulation method for the impact loading environment using an air cannon booster and a motor relay.
3.1. Impact Process of Air Cannon
The structure of the adopted air cannon is shown in
Figure 2. The air cannon consists of a back-pressure chamber, a high-pressure chamber, a quick-opening chamber, a control valve, a piston, and a piston rod. Initially, the pressure in the quick-opening chamber is higher than the pressure in the high-pressure chamber. Thus, the high-pressure chamber is sealed by the piston, and the piston rod remains stationary. When the control valve is opened, the pressure in the quick-opening chamber drops sharply, and the piston moves to the right quickly. Under this circumstance, the high-pressure chamber is opened, the high pressure gas drives the piston rod to move to the left, thus the air cannon is fired.
The resultant force F
P on the piston rod and arm and the thrust
FArm on the arm are expressed as follows:
where
s1 = 0.02 m
2, which is the area of the piston;
s2 = 0.0188 m
2, which is the area of the piston minus the area of the piston rod;
P1 denotes the air pressure in the high-pressure chamber during the launch;
P2 denotes the air pressure in the back pressure chamber during the launch; A
1 is the acceleration of the piston rod in the propulsion process;
θ is the rotation angle of the arm in the propulsion process; m
P = 44.7 kg, which is the equivalent load of the piston rod;
L is the distance between the propulsion point and the axis of the arm; x is the displacement of the piston rod; and
A2 is the tangential acceleration of the arm during the propulsion process.
The motion of the piston pushed by the air is assumed to follow an adiabatic expansion process of an ideal gas. The equation for the state of the gas is described below.
where
V1 denotes the volume of the high-pressure chamber during the launch,
M indicates the mass of the air,
μg stands for the molar mass of the air,
R is the gas constant, and
T is the air temperature.
Based on the polytropic relationship of the gas in the high-pressure chamber during the compression and expansion process [
15], the polytropic index of the air during the charging and discharging processes of the pneumatic system are set to 1.2. Thus, the pressures of the high-pressure chamber and the back-pressure chamber are expressed as follows:
where
P10 is the initial pressure of the high-pressure chamber at the time of launch,
V10 = 0.0972 m
3, which is the initial volume of the high-pressure chamber,
P20 is the initial pressure of the back-pressure chamber at the time of launch, and
V20 = 0.02827 m
3, which is the initial volume of the back-pressure chamber.
Substituting Equation (3) into Equation (1) yields
where
mArm = 45.7 kg, which is the effective load of the arm at the impact point, including the equivalent mass of the arm, the unit being tested, and the vector distributer.
As shown in Equation (4), the acceleration of the arm during the impact is determined by the initial air pressures and volumes of the back-pressure and high-pressure chambers, the areas of the piston and piston rod, the displacement of the piston rod while it is propelled, the propulsion location, and the effective loads of the arm and piston. In the tests, only the initial pressures of the back-pressure and high-pressure chamber are variables, so the initial air pressure of the high-pressure and back-pressure chambers can be adjusted to obtain different launch accelerations. As the displacement of the piston rod increases, the thrust becomes increasingly small, and the piston rod can reverse directions. To avoid reverse collision between the arm and the recoiling air cannon, the piston rod and the arm are not placed at the same level. The collision end of the piston rod is machined into a pulley. The pulley rises before and falls after the collision. When simulating an impact overload environment of 10
g, the simulated air pressures of the high-pressure and back-pressure chambers versus the displacement of the piston rod can be obtained.
Figure 3 plots the relationship between the pressure and the displacement of the piston rod.
At the displacement of 0.5 m, the air cannon is fired, which is controlled by the air pressures of the high-pressure and back-pressure chambers. The acceleration of the pulley and the tangential acceleration of the arm are plotted in
Figure 4. As can be observed, with an impact overload of 10
g, 2 ms after the air cannon launching, the accelerations peak 10.74
g and then drop rapidly. The accelerations remain between 6 and 7
g for about 30 ms, after which they decrease. The entire propulsion time lasts 119.5 ms. At the end of the launching, the tangential acceleration of the arm is zero
g.
3.2. Clutch Engagement Process
During the simulation analysis, the air cannon pushes the arm. The starting time is very short, the acceleration amplitude and loading time meet the simulation test requirements of the impact overload environment. The air cannon propulsion duration is about 120 ms, but the acceleration rise time is only 2 ms. The duration of the peak is too short. To maintain the impact state, the clutch needs to be engaged and the motor needs to be relayed to power the arm rotation. If the relay is completed at the peak of the acceleration, the acceleration of the arm can be achieved.
The driving plate of the clutch is driven by the main control motor. The load on the driven plate includes the effective loads of the reducer, the rotating shaft, the arm, the vector distributor, and the unit being tested. The power transmission system of the centrifuge is shown in
Figure 5.
The dynamic equation of the clutch transmission can be written as
where
Jc,
ωc, and
Tc are the moment of inertia, revolution speed, and output torque of the main control motor, respectively,
Tf is the friction torque transmitted by the clutch, and
Jd,
ωd and
Td are the effective moment of inertia, revolution speed, equivalent resistance torque of the driven plate, respectively.
When the clutch is completely disengaged,
Tf = 0 Nm, and when the clutch is fully engaged,
Tf =
Tc =
Td. The main operation process of the clutch in the test system varies from a fully engaged sliding friction state to a fully disengaged sliding friction state. The initial speed of the driven plate is zero rad/s. When the clutch is engaged, the speeds of the driven plate are shown in
Figure 6.
At the beginning, the driven plate keeps stationary, the clutch is disengaged, and the motor is idling. Therefore, Td, Tf, and Tc are all zero Nm, and the motor’s speed ωc is a constant. When the clutch is engaged, the difference in the speeds of revolution becomes large. Tf is gradually increased, the revolution speed (ωd) of the driven plate increases, and the output torque (Tc) of the motor also increases. Because Tf is less than Tc, the motor accelerates. The difference in the speeds of revolution increases. Tf increases faster than Tc, and the driven plate accelerates. When Tf > Tc, the motor decelerates, the difference in the revolution speeds becomes increasingly small, and Tf gradually decreases. When Tf = Tc, the clutch is fully engaged, the motor drives the load to accelerate and stabilize after attaining the set revolution speed.
In the simulation tests, the arm is started by the air cannon. Ideally, the engagement process will be completed when the propelled acceleration reaches the maximum, and the tangential acceleration of the arm after launching will be equal to the set normal acceleration of the arm. Since the propulsion time is very short, ωd should be less than ωc, and the arm always accelerates during the engagement process. If the timing is missed, the engagement occurs too late, the arm decelerates, the impact acceleration is less than zero g, and the impact overload cannot be simulated. Therefore, it is necessary to select a suitable clutch and proper engagement timing. By controlling the pressures of the air cannon chambers and the revolution speed of the motor, the rapid transition between the impact overload and the continuous acceleration can be achieved.
3.3. Speed Control System Based on Active Disturbance Rejection Control
When the clutch is engaged, the output torque of the motor is increased rapidly, and the serious load disturbance is produced. In order to improve the precision of the speed control system, it becomes necessary to compensate for the disturbance. Active disturbance rejection control (ADRC) considers perturbations to the model and parameters and unmeasurable external disturbances as the total disturbance of the system. Based on the feedback control of the system error, the total disturbance is observed and corrected, which alleviates the conflict between the response speed and overshoot in the PID controls. ADRC does not rely on any precise mathematical model of a system, and it significantly improves the dynamic response characteristics of the controller through a proper nonlinear combination. The load disturbance can be estimated and compensated by a speed controller based on active disturbance rejection control (ADRC). Many researchers have studied the application of the speed controller based on ADRC. In order to improve pointing accuracy and rotation speed, Li et al. [
16] designed the inner and outer loops of an ADRC for the antenna pointing control of a large flexible satellite system. Kang et al. [
17] designed an idle speed controller based on ADRC to compensate for varying engine load and friction torque in passenger car diesel engines.
ADRC consists of three main components: a tracking differentiator (TD), an extended state observer (ESO), and a nonlinear state error feedback law (NSEFL). The TD arranges the transition process for the system and extracts the differential signal. ESO is the core of ADRC and can estimate the total disturbance of the system in real time and compensate for it. The outputs of the TD and ESO are compared to obtain the systematic error. Based on the error signal, the NSEFL produces a control strategy for the controlled object.
Based on the torque and motion equations of the three-phase asynchronous motor, the differential equation of the speed loop can be obtained as follows:
where
ω is the rotor speed,
ρ is the number of the pole pairs of the motor,
Lm is the stator-rotor mutual inductance,
J is the system moment of inertia converted to the motor shaft,
Lr is the rotor inductance,
ψr is the rotor magnetic flux,
iTs is the torque component of the stator current, and
TL is the load torque.
There is a coupling term in Equation (6). When the load disturbance is considered,
g3(
t), the equation of state of the revolution speed becomes
The corresponding second-order ESO is as follows:
where α
3, β
21, β
22, δ
3 are four adjustable parameters,
fal( ) is a nonlinear function, and it can be defined as follows:
The nonlinear state error feedback control law is
The control quantity
iTs obtained by the nonlinear state error feedback control can be written as
Substituting Equation (11) into Equation (7) yields the following formula
After the ADRC is adjusted, the coupling term is no longer included in the speed state equation and the total disturbance of the system is canceled. The structure of the speed outer loop ADRC system is illustrated in
Figure 7.
Similarly, the first-order ADRC regulations for the excitation current loop and the torque current loop can also be obtained. The differential equation for the rotor flux is shown as follows:
where
Rr is the rotor resistance and
iMs is the excitation component of the stator current. Equation (13) does not contain a coupling term, and the rotor flux outer loop can be regulated by a PI controller. The first-order ADRC regulators are applied to the speed outer loop, the excitation current loop, and the torque current loop. Thus, the asynchronous motor speed control system based on the ADRC is designed.
Simulation and analysis of the speed control system based on ADRC are conducted. The parameters of the asynchronous motor are shown in
Table 1. The appropriate parameters of the speed control system should be set before the simulation. The discretization time values are all set to 100 ms in this system. The regulator coefficients of the speed outer loop are:
β21 = 1000,
β22 = 16,000, a
3 = 0.5,
δ3 = 0.01,
β3 = 10. The regulator coefficients of the excitation current loop are: β
01 = 10,000, β
02 = 120,000, a
1 = 0.5, δ
1 = 0.01, β
1 = 100. The regulator coefficients of the torque current loop are: β
11 = 10,000, β
12 = 120,000, a
2 = 0.5, δ
2 = 0.01, β
2 = 100. The regulator coefficients of the rotor flux outer loop are: K
p = 2, T
I = 10.
In the simulation test of the system operation, the motor is started without a load. When the clutch is engaged, the load disturbance is produced, and the torque transmitted depends on the speed difference between the driving plate and the driven plate.
The load torque is shown as follows:
where
Jd = 10 Kgm
2, which is the load moment of inertia, α = 3 rad/s
2, which is the load angular acceleration.
The set motor speed is 200 rpm, and the motor relay is set as 0.4 s with a load of 30 Nm added abruptly. The simulation results of the motor speed controlled by ADRC are shown in
Figure 8 and
Figure 9. As can be observed, the system response does not exhibit overshoot and the rise time is 0.037 s. When the load is suddenly applied at 0.4 s, the motor speed drops to 196.9 rpm. After 0.01 s, the speed quickly returns to a stable state. The load is driven by the air cannon before the clutch is engaged, and it is driven by the motor after the clutch is engaged. The load is rotating in the same direction as the motor and the speed difference between the load and the motor is small, so the change of the speed will become small and the recovery time will get short. Accompanied with the improved disturbance-rejection performance of the system, an orderly transition between the impact overload and steady-state overload can be achieved.
3.4. Loading Test of Impact Overload Environment
Both the air cannon firing and the electromagnetic clutch engagement commands are sent by the digital output module to the driving system controlled by the relay. The electromagnetic clutch is controlled by the carbon brush, and the air cannon is controlled by the electric solenoid valve. The response speeds of the two mechanisms are different.
The revolution speed of the arm is set as 105 rpm. After the air cannon is fired, the electromagnetic clutch is immediately relayed. The measured impact acceleration of the arm is shown in
Figure 10. The steady-state normal acceleration of the arm is 11
g. The acceleration rise time is 74.56 ms. The loading time of the launch acceleration is 819 ms, which does not meet the simulation test requirements for the impact overload environment. The electromagnetic clutch has a longer response time than the solenoid valve, causing too long loading time. As can be measured, the electromagnetic clutch has a response time that is 250 ms slower than that of the electric solenoid valve. Therefore, the loading time of the impact acceleration can be regulated by adjusting the operating time sequence of the air cannon launch and the clutch engagement. After the clutch has been engaged for 250 ms, the air cannon is fired.
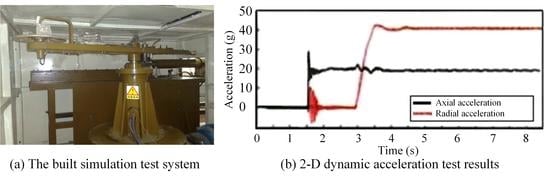
The unit being tested is fixed on the arm. The axial direction of the unit being tested points to the normal direction of the arm, and the radial direction of the unit being tested points to the tangential direction of the arm. So, the tangential acceleration of the arm is the radial acceleration of the unit, and the normal acceleration of the arm is the axial acceleration of the unit. The impact overload acceleration is set as 20
g. After 2.5 s of the air cannon being fired, the motor accelerates, and the axial acceleration of the unit being tested is set as 40
g. The 2-D acceleration test results of the unit being tested are shown in
Figure 11. The air cannon propels the arm and produces a powerful impact. The radial acceleration of the unit being tested rises instantaneously. After the clutch is engaged, the motor drives the arm to rotate at a constant revolution speed, while the radial acceleration of the unit being tested is zero
g. After 2.5 s, the motor is accelerated, and the axial acceleration of the unit being tested reaches 40
g.
As shown in
Figure 11, the loading time of the impact overload acceleration is 120 ms, and the loading time of the flight overload acceleration is 335 ms, which satisfy the speed requirements of the axial acceleration of the unit being tested. However, during the acceleration of the arm, the tangential acceleration occurs, which does not meet the requirements of the simulation test for the radial acceleration of the unit being tested. Therefore, the vector shaft angle needs to be adjusted to track or decompose the resultant acceleration of the arm to achieve dynamically controllable loading of the radial acceleration of the unit being tested.