Directional DC Charge-Transfer Resistance on an Electrode–Electrolyte Interface in an AC Nyquist Curve on Lead-Acid Battery
Abstract
:Featured Application
Abstract
1. Introduction
2. AC Equivalent First Principle Linear Circuit and Experiments
2.1. Requirements of FPLCACequ
2.2. Analysis of AC Equivalent Impedances on Electrode–Electrolyte Interfaces by Average Switch Modeling
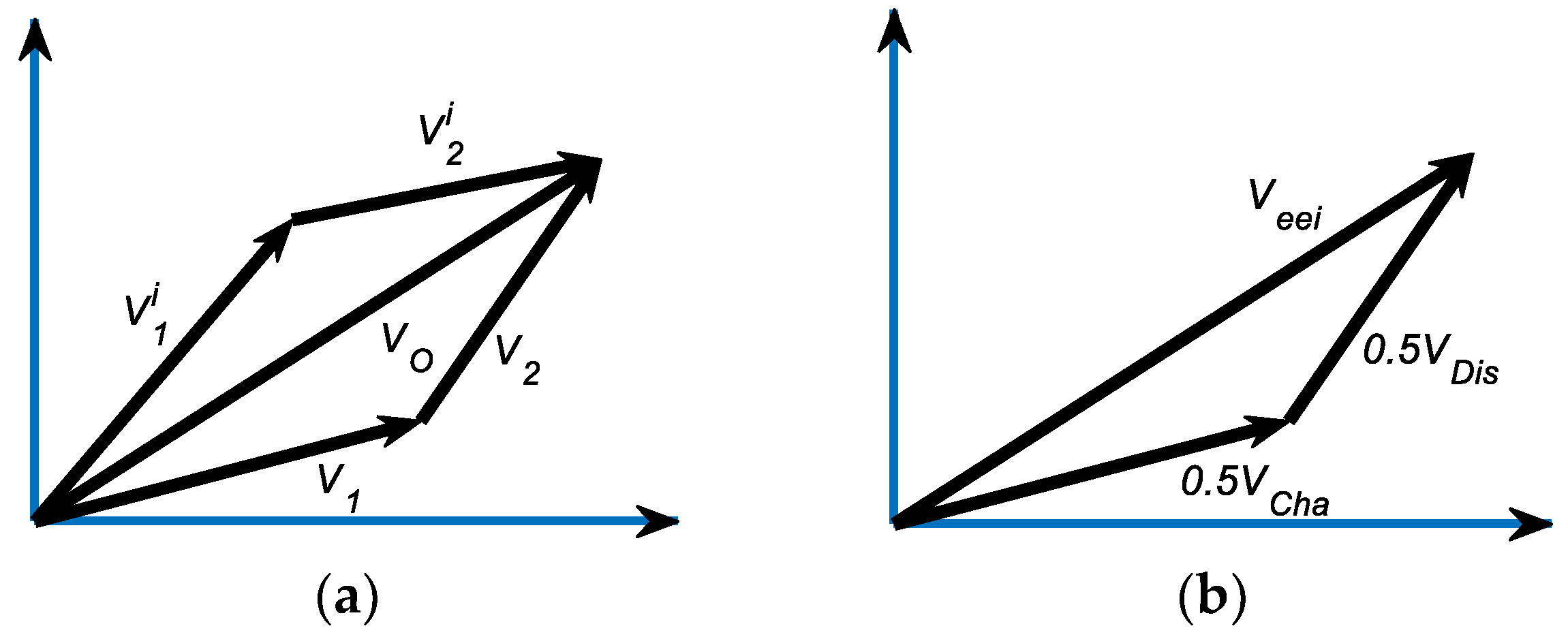
2.2.1. Vector Analysis of Responses of Electrode–Electrolyte Interfaces by Average Switch Modeling
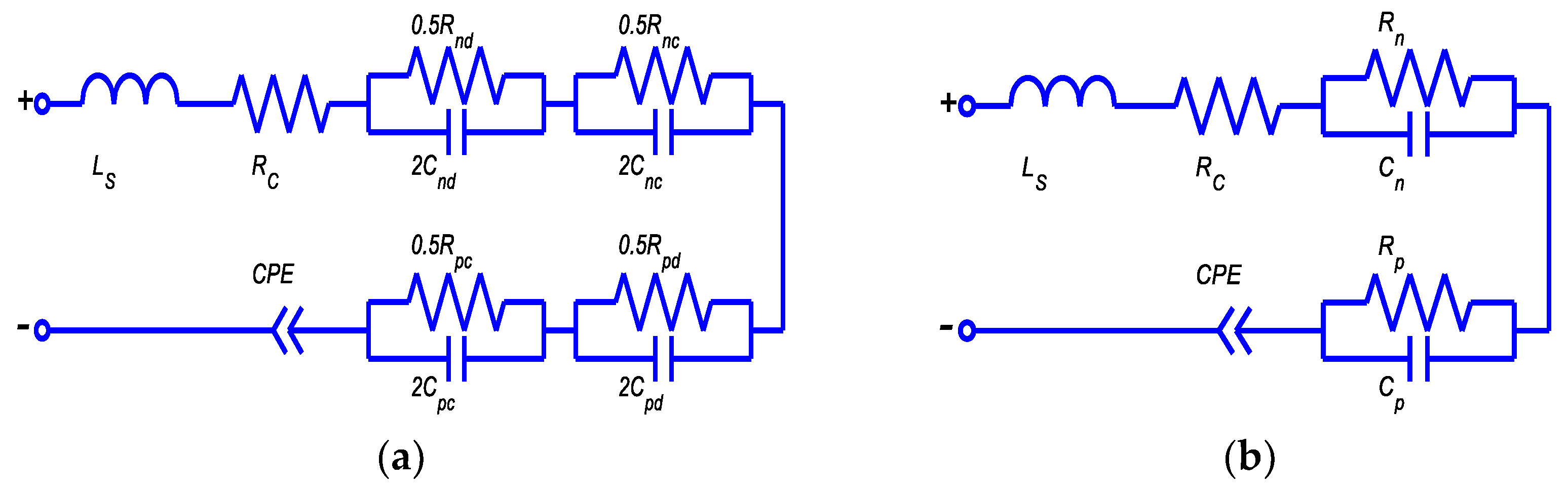
2.2.2. AC Equivalent Frist Principle Impedances on Electrode–Electrolyte Interfaces
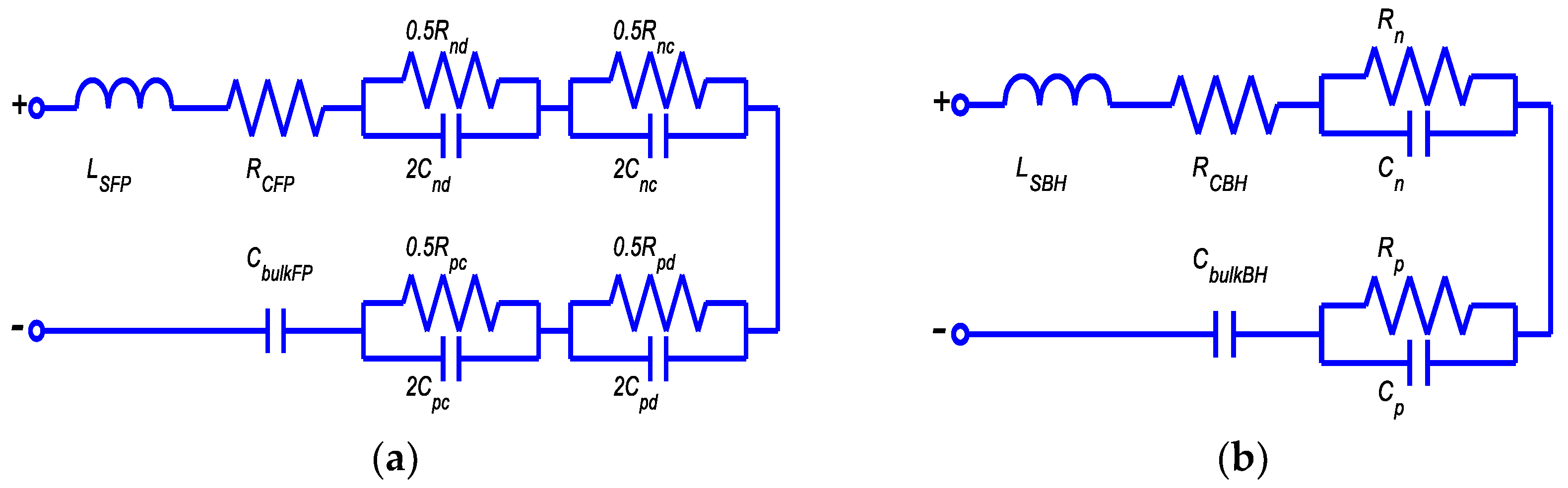
2.3. AC Equivalent First Principle Linear Elements on Batteries
2.4. Experiment of Steady-state DC static Voltage Responses on Battery Terminals
2.5. Experiment of Steady-State AC fundamental Voltage Responses on Battery Terminals
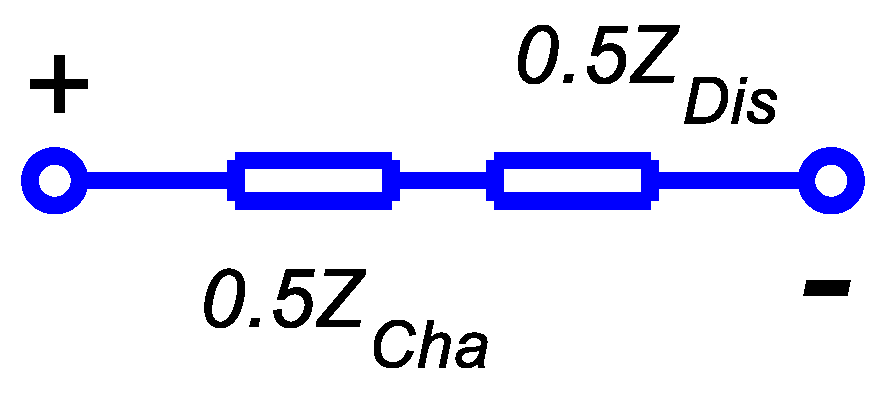
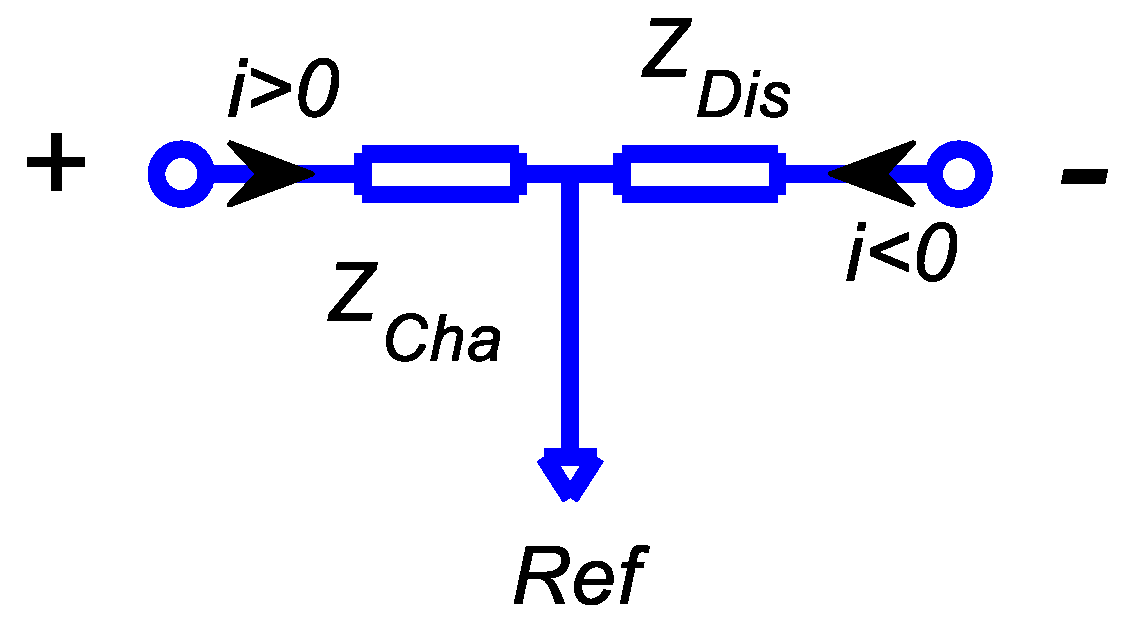
2.6. Experiment of Extracting Directional Charge-Transfer Resistance in AC Nyquist Curve
3. Results and Discussion
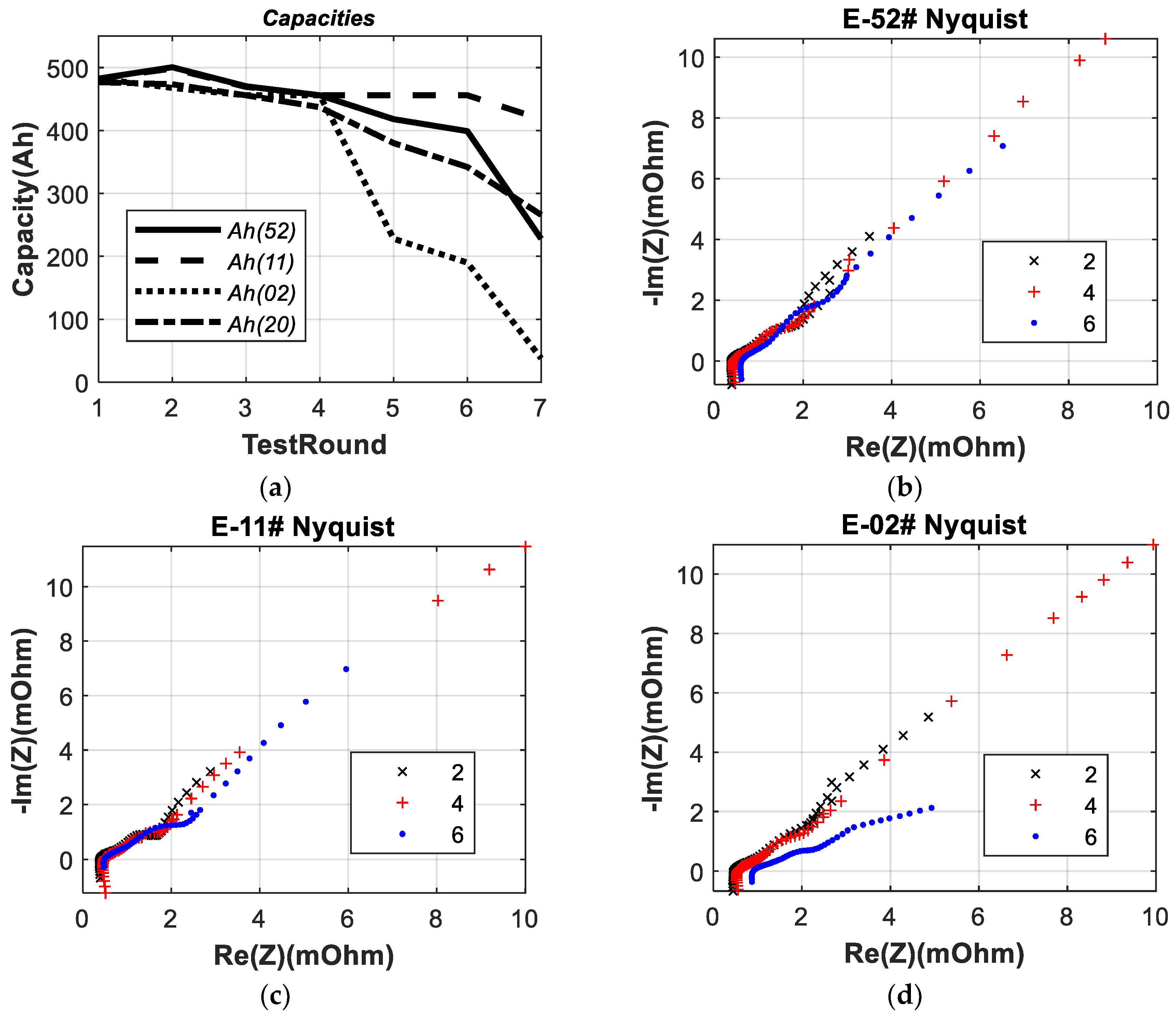
3.1. Results of Steady-State DC Static Voltage Responses on Battery Terminals
3.2. Results of Steady-State AC Fundamental Voltage Responses on Battery Terminals
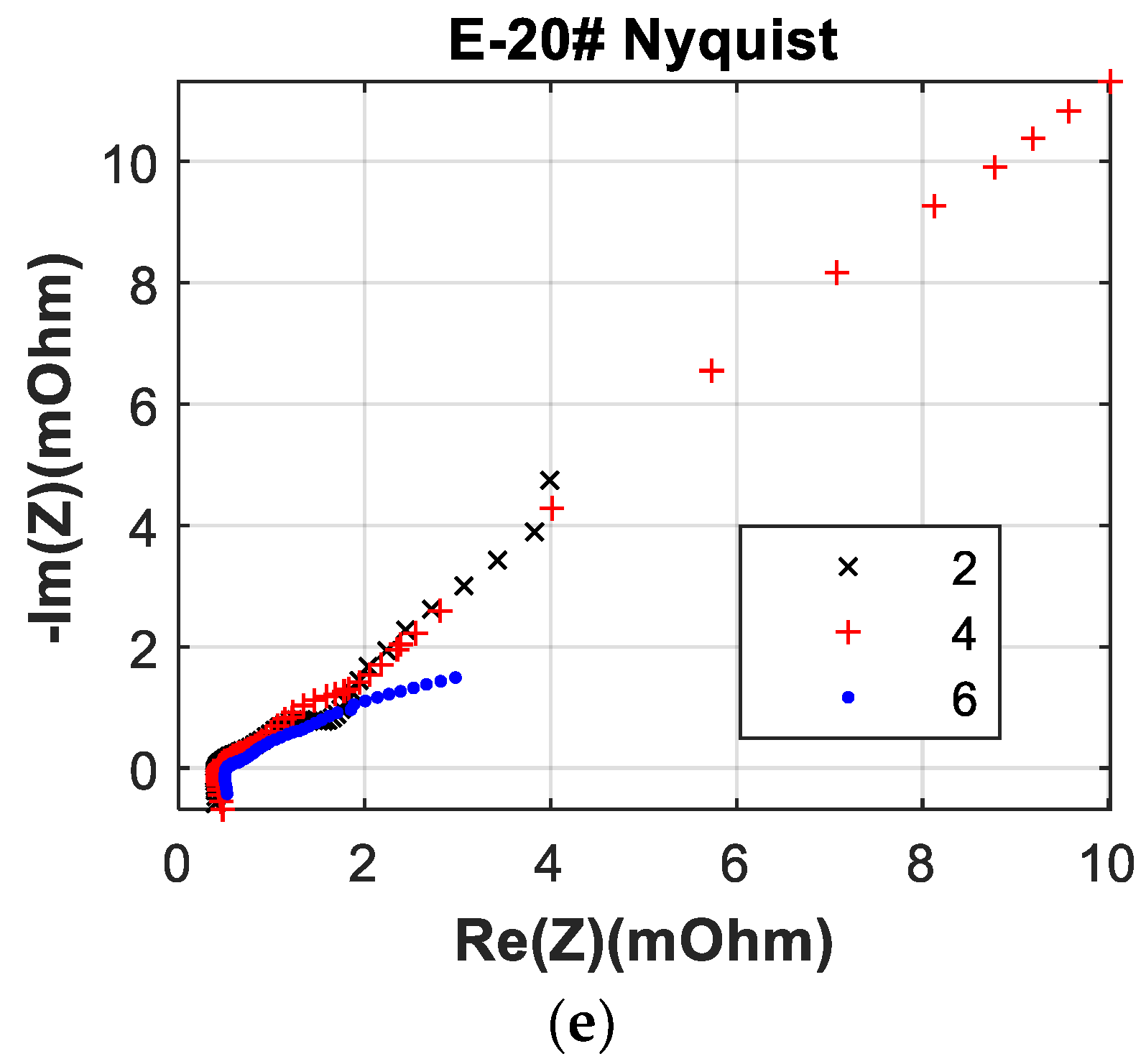
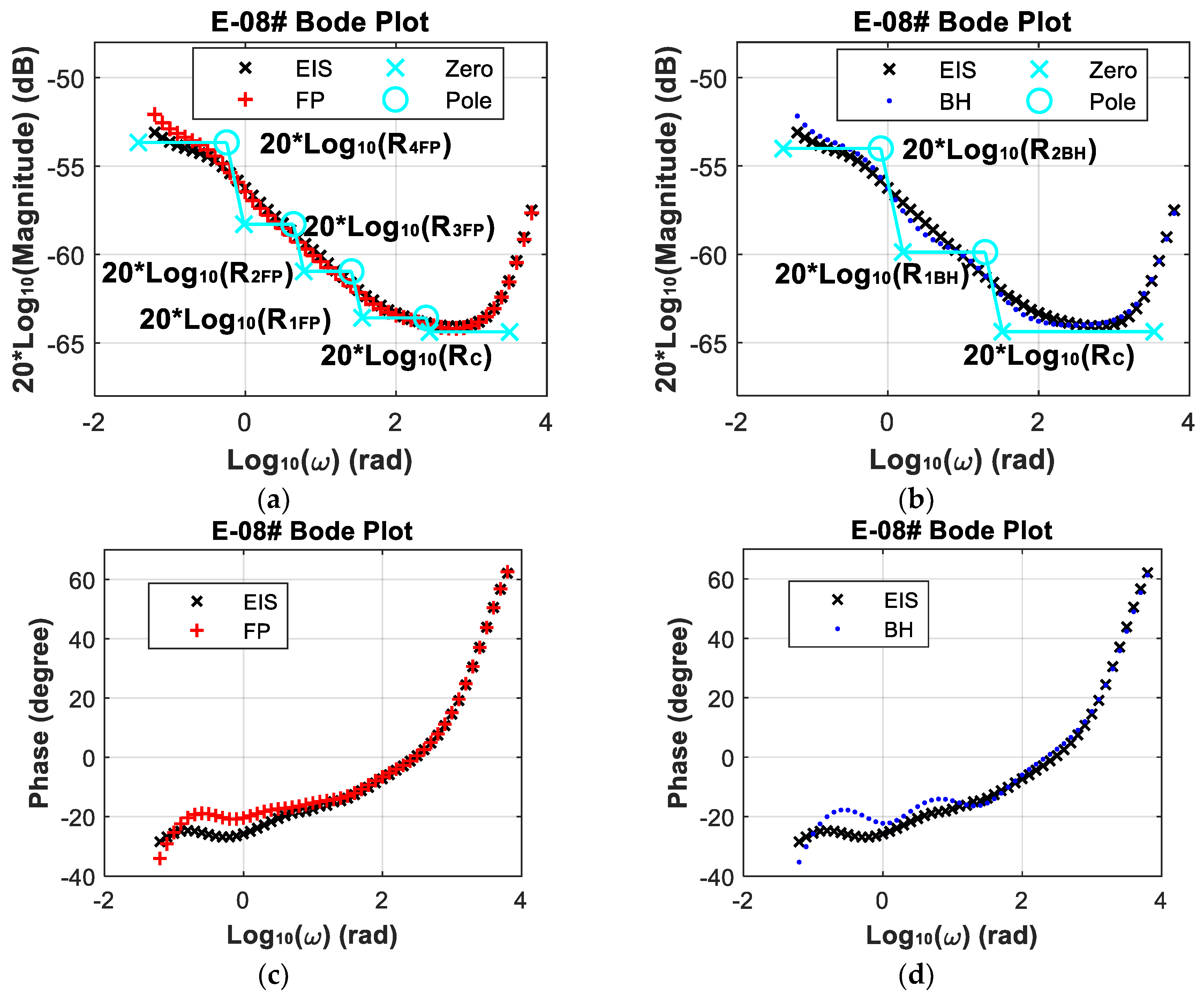
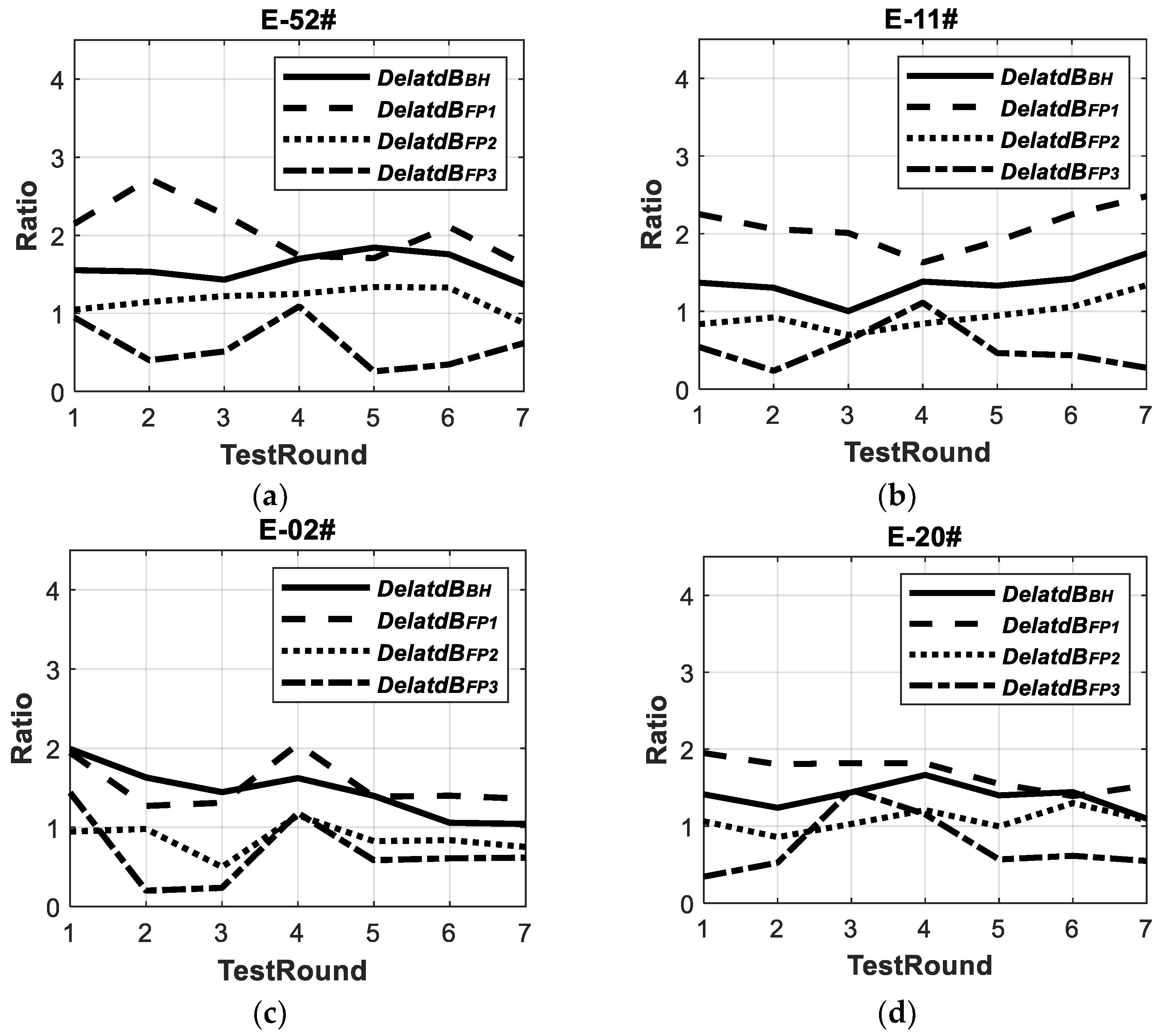
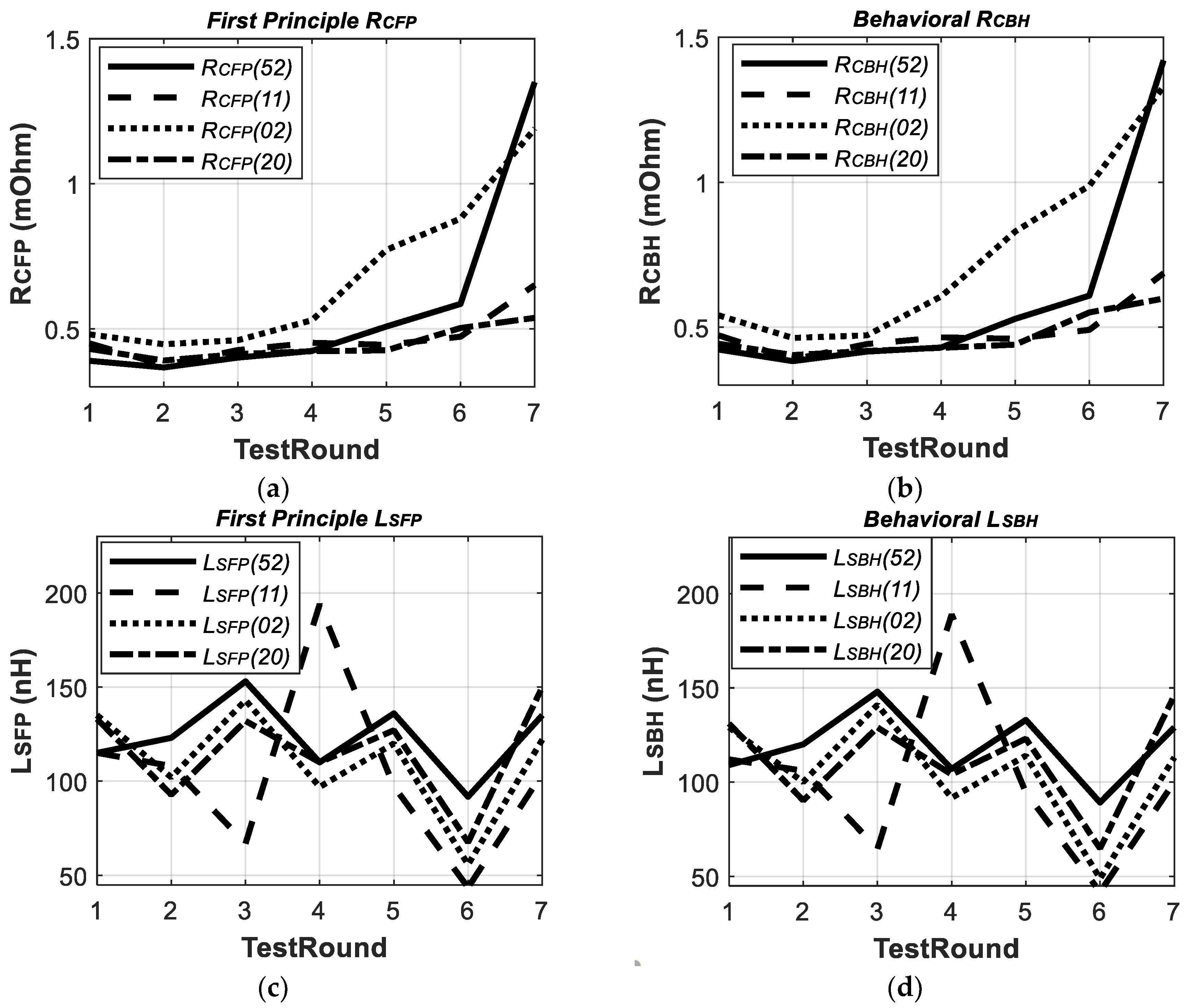
3.3. Results of Extraction Directional Charge-Transfer Resistance in AC Nyquist Curve
3.4. Boundary between First Principle Circuits on Batteries in Field Applications
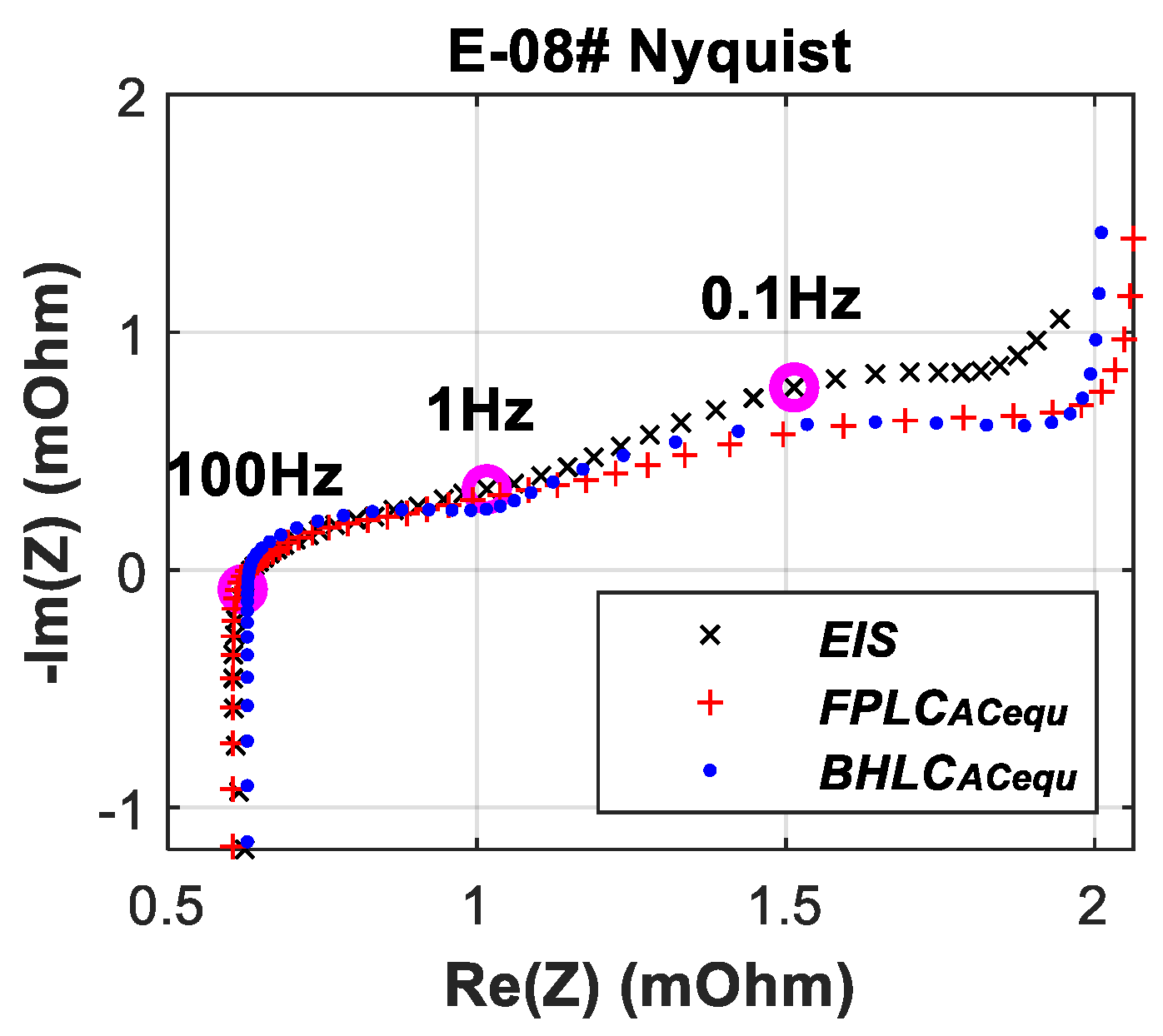
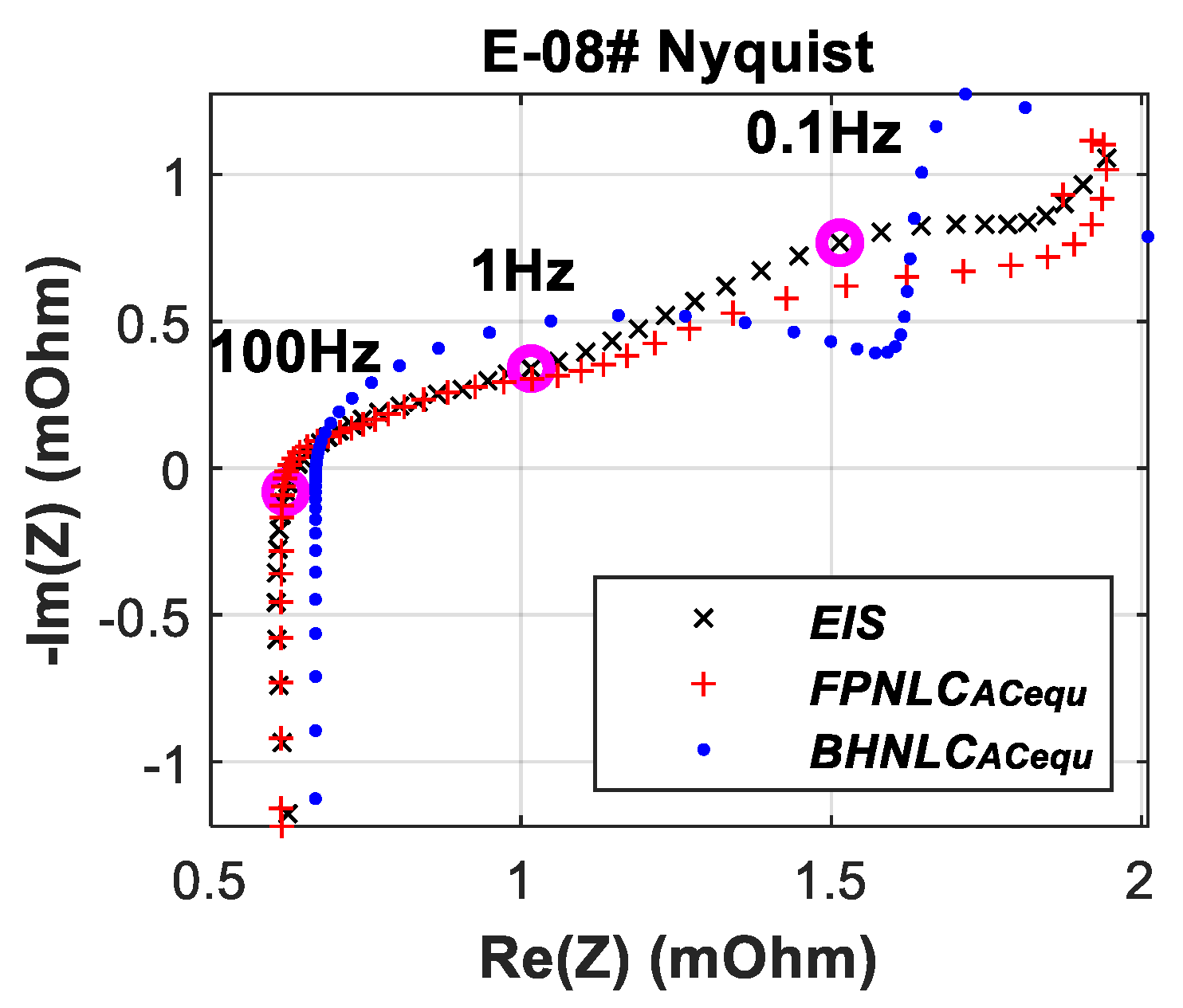
3.5. Comparing with Nonlinear Models
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
| Item | Elements | Physical Meanings |
|---|---|---|
| 1 | EIS | electrochemical impedance spectroscopy |
| 2 | FPLCDCequ | DC equivalent first principle linear circuit |
| 3 | SoH | state of health |
| 4 | FPLCACequ | AC equivalent first principle linear circuit |
| 5 | BMS | battery management system |
| 6 | SoC | state of charge |
| 7 | BHLCACequ | AC equivalent behavioral linear circuit |
| 8 | contacting resistor | |
| 9 | stray inductor | |
| 10 | electrolyte bulk capacitor | |
| 11 | charge-transfer resistor | |
| 12 | double-layer capacitor | |
| 13 | impedance on electrode–electrolyte interface | |
| 14 | charge-transfer resistor for charging on electrode–electrolyte interface of positive electrode | |
| 15 | charge-transfer resistor for charging on electrode–electrolyte interface of negative electrode | |
| 16 | charge-transfer resistor for discharging on electrode–electrolyte interface of positive electrode | |
| 17 | charge-transfer resistor for discharging on electrode–electrolyte interface of negative electrode | |
| 18 | double-layer capacitor for both charging and discharging on electrode–electrolyte interface of positive electrode | |
| 19 | double-layer capacitor for both charging and discharging on electrode–electrolyte interface of negative electrode | |
| 20 | double-layer capacitor for charging on electrode–electrolyte interface of positive electrode | |
| 21 | double-layer capacitor for charging on electrode–electrolyte interface of negative electrode | |
| 22 | double-layer capacitor for discharging on electrode–electrolyte interface of positive electrode | |
| 23 | double-layer capacitor for discharging on electrode–electrolyte interface of negative electrode | |
| 24 | fixed frequency sinusoidal current, excited from electrochemical workstation, is the amplitude and is the static angle (rad) in rotating space vector plane | |
| 25 | linear impedances for charging on electrode–electrolyte interfaces of both positive and negative electrodes, is the amplitude and is the static angle (rad) in rotating space vector plane | |
| 26 | linear impedances for discharging on electrode–electrolyte interfaces of both positive and negative electrodes, is the amplitude and is the static angle (rad) in rotating space vector plane | |
| 27 | equivalent linear impedances on electrode–electrolyte interfaces of both positive and negative electrodes, is the amplitude and is the static angle (rad) in rotating space vector plane | |
| 28 | charging voltage vector, steady-state AC fundamental response of linear impedances , in rotating space vector plane | |
| 29 | discharging voltage vector, steady-state AC fundamental voltage response of linear impedances , in rotating space vector plane | |
| 30 | new charging voltage vector, half amplitude of , in rotating space vector plane | |
| 31 | new discharging voltage vector, half amplitude of , in rotating space vector plane | |
| 32 | target voltage vector, steady-state AC fundamental response of impedance , in rotating space vector plane | |
| 33 | fixed period of sinusoidal current, excited from electrochemical workstation | |
| 34 | transient voltage responses of impedances for charging on electrode–electrolyte interfaces of both positive and negative electrodes | |
| 35 | transient voltage responses of impedances for discharging on electrode–electrolyte interfaces of both positive and negative electrodes | |
| 36 | average voltage response of impedances for charging on electrode–electrolyte interfaces of both positive and negative electrodes during the period | |
| 37 | average voltage response of impedances for discharging on electrode–electrolyte interfaces of both positive and negative electrodes during the period | |
| 38 | complex variable in transfer function | |
| 39 | transfer function of band pass filter | |
| 40 | quality factor of band pass filter | |
| 41 | electrical angular velocity of sinusoidal current, excited from electrochemical workstation | |
| 42 | linear contacting resistance, reflecting conductivity of path which currents flowing along terminals, cell connectors, plate connectors, electrodes, and electrolyte; is the amplitude and 0 is the static angle (rad) in rotating space vector plane | |
| 43 | linear stray inductance, in series with linear contacting resistance; is the amplitude and is the static angle (rad) in rotating space vector plane | |
| 44 | linear electrolyte bulk capacitance, reflecting capacity characteristics of bulk electrolyte; is the amplitude and is the static angle (rad) in rotating space vector plane | |
| 45 | contacting resistance voltage vector, steady-state AC fundamental responses on linear contacting resistance | |
| 46 | transient voltage responses of linear contacting resistance | |
| 47 | average voltage response of linear contacting resistance during the period | |
| 48 | inductance voltage vector, steady-state AC fundamental responses on linear stray inductance | |
| 49 | transient voltage responses of linear stray inductance | |
| 50 | average voltage response of linear stray inductance during the period | |
| 51 | electrolyte bulk capacitance voltage vector, steady-state AC fundamental responses on linear electrolyte bulk capacitance | |
| 52 | transient voltage responses of linear electrolyte bulk capacitance | |
| 53 | average voltage response of linear electrolyte bulk capacitance during the period | |
| 54 | Warburg impedance | |
| 55 | diffusion constant of Warburg impedance | |
| 56 | ohmic constant of Warburg impedance | |
| 57 | Constant Phase Element | |
| 58 | diffusion constant of Constant Phase Element | |
| 59 | P | constant of power function |
| 60 | charge-transfer resistor for both charging and discharging on electrode–electrolyte interface of positive electrode | |
| 61 | charge-transfer resistor for both charging and discharging on electrode–electrolyte interface of negative electrode | |
| 62 | linear contacting resistance of FPLCACequ | |
| 63 | linear contacting resistance of BHLCACequ | |
| 64 | linear stray inductance of FPLCACequ | |
| 65 | linear stray inductance of BHLCACequ | |
| 66 | FPNLCACequ | AC equivalent first principle nonlinear circuit |
| 67 | BHNLCACequ | AC equivalent behavioral nonlinear circuit |
References
- Kwiecien, M.; Badeda, J.; Huck, M.; Komut, K.; Duman, D.; Sauer, D.U. Determination of SoH of Lead-Acid Batteries by Electrochemical Impedance Spectroscopy. Appl. Sci. 2018, 8, 873. [Google Scholar] [CrossRef] [Green Version]
- Srinivasan, V.; Wang, G.Q.; Wang, C.Y. Mathematical Modeling of Current-Interrupt and Pulse Operation of Valve-Regulated Lead Acid Cells. J. Electrochem. Soc. 2003, 150, A316–A325. [Google Scholar] [CrossRef] [Green Version]
- Rahn, C.D.; Wang, C.Y. Battery Systems Engineering; John Wiley& Sons: New York, NY, USA, 2013; pp. 4–6, 27–28, 102–103, 122. [Google Scholar] [CrossRef] [Green Version]
- Brik, K.; Ammar, F.B. Causal Tree Analysis of Depth Degradation of the Lead Acid Battery. J. Power Sources 2013, 228, 39–46. [Google Scholar] [CrossRef]
- Boškoski, P.; Debenjak, A.; Boshkoska, B.M. Fast Electrochemical Impedance Spectroscopy; Springer: Cham, Switzerland, 2017; p. 1. [Google Scholar] [CrossRef]
- Kwiecien, M.; Huck, M.; Badeda, J.; Zorer, C.; Komut, K.; Yu, Q.; Sauer, D.U. Variation of Impedance in Lead-Acid Batteries in the Presence of Acid Stratification. Appl. Sci. 2018, 8, 1018. [Google Scholar] [CrossRef] [Green Version]
- Shen, Z.; Rahn, C.D. Physics-Based Model of a Valve-Regulated Lead-Acid Battery and an Equivalent Circuit. J. Dyn. Syst. Meas. Control 2013, 135, 041011. [Google Scholar] [CrossRef]
- Sadabadi, K.K.; Ramesh, P.; Tulpule, P.; Rizzoni, G. Design and Calibration of a Semi-empirical Model for Capturing Dominant Aging Mechanisms of a PbA Battery. J. Energy Storage 2019, 24, 100789. [Google Scholar] [CrossRef]
- Yahmadi, R.; Brik, K.; Ammar, F.B. Causal Tree Analysis for Quality Control of the Lead Acid Battery manufacturing process. Int. J. Energy Res. 2018, 42, 1738–1759. [Google Scholar] [CrossRef]
- Kwiecien, M.; Huck, M.; Badeda, J.; Sauer, D.U. Correct Processing of Impedance Spectra for Lead-acid Batteries to Parameterize the Charge-Transfer Process. J. Appl. Electrochem. 2018, 48, 885–900. [Google Scholar] [CrossRef]
- Badeda, J.; Kwiecien, M.; Schulte, D.; Sauer, D.U. Battery State Estimation for Lead-Acid Batteries under Float Charge Conditions by Impedance: Benchmark of Common Detection Methods. Appl. Sci. 2018, 8, 1308. [Google Scholar] [CrossRef] [Green Version]
- Meiwes, H.B.; Kowal, J.; Sauer, D.U.; Karden, E. Influence of Measurement Procedure on Quality of Impedance Spectra on Lead–acid Batteries. J. Power Sources 2011, 196, 10415–10423. [Google Scholar] [CrossRef]
- Ansari, A.B.; Vahid, E.; Torabi, F. Discharge, Rest and Charge Simulation of Lead-acid Batteries Using an Efficient Reduced Order Model Based on Proper Orthogonal Decomposition. Appl. Energy 2016, 173, 152–167. [Google Scholar] [CrossRef]
- Cao, Y.; Kroeze, R.C.; Krein, P.T. Multi-timescale Parametric Electrical Battery Model for Use in Dynamic Electric Vehicle Simulations. IEEE Trans. Transp. Electrif. 2016, 2, 432–442. [Google Scholar] [CrossRef]
- Aksakal, C.; Sisman, A. On the Compatibility of Electric Equivalent Circuit Models for Enhanced Flooded Lead Acid Batteries Based on Electrochemical Impedance Spectroscopy. Energies 2018, 11, 118. [Google Scholar] [CrossRef] [Green Version]
- Monfared, N.A.; Gharib, N.; Moqtaderi, H.; Hejabi, M.; Amiri, M.; Torabi, F.; Mosahebi, A. Prediction of State-of-Charge Effects on Lead-Acid Battery Characteristics Using Neural Network Parameter Modifier. J. Power Sources 2006, 158, 932–935. [Google Scholar] [CrossRef]
- Piłatowicz, G.; Marongiu, A.; Drillkens, J.; Sinhuber, P.; Sauer, D.U. A Critical Overview of Definitions and Determination Techniques of the Internal Resistance Using Lithium-Ion, Lead-Acid, Nickel Metal-Hydride Batteries and Electrochemical Double-Layer Capacitors as Examples. J. Power Sources 2015, 296, 365–376. [Google Scholar] [CrossRef]
- Cacciato, M.; Nobile, G.; Scarcella, G.; Scelba, G. Real-Time Model-Based Estimation of SOC and SOH for Energy Storage Systems. IEEE Trans. Power Electron. 2016, 32, 794–803. [Google Scholar] [CrossRef]
- Karden, E. Using Low-Frequency Impedance Spectroscopy for Characterization, Monitoring, and Modeling of Industrial Batteries. Ph.D. Thesis, RWTH Aachen University, Aachen, Germany, December 2001; pp. 13–24. [Google Scholar]
- Shen, Z. Control-Oriented Modeling, State-of-Charge, State-of-Health, and Parameter Estimation of Batteries. Ph.D. Thesis, The Pennsylvania State University, University Park, PA, USA, August 2013; pp. 23–27, 28–30. [Google Scholar]
- Nejad, S. Adaptive Techniques for Estimation and Online Monitoring of Battery Energy Storage Devices. Ph.D. Thesis, University of Sheffield, Sheffield, UK, May 2017; pp. 161–169. [Google Scholar]
- Gu, W.B.; Wang, G.Q.; Wang, C.Y. Modeling the Overcharge Process of VRLA Batteries. J. Power Sources 2002, 108, 174–184. [Google Scholar] [CrossRef]
- Erickson, R.W.; Maksimovic, D. Fundamentals of Power Electronics, 2nd ed.; Springer: New York, NY, USA, 2001; pp. 13–15, 45–50. [Google Scholar]
- Barsoukov, E.; Macdonald, J.R. Impedance Spectroscopy Theory, Experiment, and Applications, 2nd ed.; John Wiley& Sons: New York, NY, USA, 2005; pp. 4–7. [Google Scholar]
- Berndt, D.; Teutsch, U. Float Charging of Valve-Regulated Lead-Acid Batteries: A Balancing Act between Secondary Reactions. J. Electrochem. Soc. 1996, 143, 790. [Google Scholar] [CrossRef]
- Wu, B.; Narimani, M. High-Power Converters and AC Drives, 2nd ed.; John Wiley& Sons: New York, NY, USA, 2017; pp. 101–105. [Google Scholar]
- Wang, C.Y.; Xia, J.K.; Sun, Y.B. Modern Control Technique of Electric Machines; China Machine Press: Beijing, China, 2008; p. 41. [Google Scholar]
- Mauracher, P.; Karden, E. Dynamic Modelling of Lead/Acid Batteries Using Impedance Spectroscopy for Parameter Identification. J. Power Sources 1997, 67, 69–84. [Google Scholar] [CrossRef]
- Wang, W.B.; Yao, W.X.; Chen, W.; Chen, D.; Lu, Z.Y. Characteristic Charge Transfer Resistance of Electrodes on Lead-Acid Batteries. J. Electrochem. Soc. 2020, 167, 040515. [Google Scholar] [CrossRef]





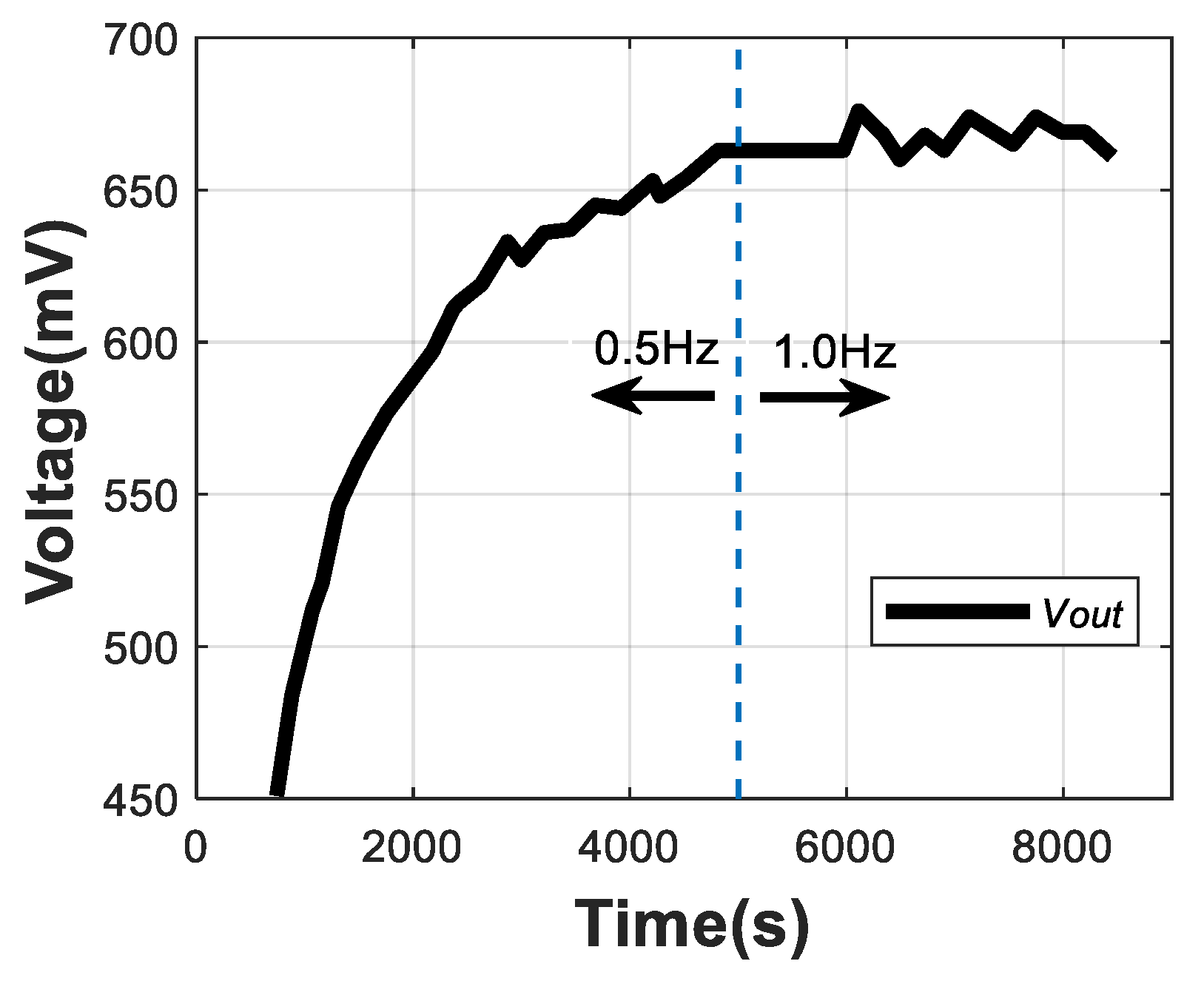


| No. | Pulse Procedures | Timing (s) | Output (mV) |
|---|---|---|---|
| 1 | Before 0.5 Hz/5 A injection | 740 | 451 |
| 2 | 150 s after 0.5 Hz/5 A injection close | 880 | 484 |
| 3 | 150 s after 0.5 Hz/5 A injection open | 1070 | 512 |
| 4 | Before 0.5 Hz/5 A injection | 1160 | 521 |
| 5 | 150 s after 0.5 Hz/5 A injection close | 1310 | 546 |
| 6 | 150 s after 0.5 Hz/5 A injection open | 1490 | 560 |
| 7 | Before 0.5 Hz/5 A injection | 1580 | 566 |
| 8 | 180 s after 0.5 Hz/5 A injection close | 1760 | 577 |
| 9 | 180 s after 0.5 Hz/5 A injection open | 1950 | 586 |
| 10 | Before 0.5 Hz/5 A injection | 2000 | Missing |
| 11 | 180 s after 0.5 Hz/5 A injection close | 2180 | 597 |
| 12 | 180 s after 0.5 Hz/5 A injection open | 2370 | 611 |
| 13 | Before 0.5 Hz/5 A injection | 2420 | 613 |
| 14 | 210 s after 0.5 Hz/5 A injection close | 2630 | 619 |
| 15 | 210 s after 0.5 Hz/5 A injection open | 2870 | 633 |
| 16 | Before 0.5 Hz/5 A injection | 3000 | 627 |
| 17 | 210 s after 0.5 Hz/5 A injection close | 3210 | 636 |
| 18 | 210 s after 0.5 Hz/5 A injection open | 3450 | 637 |
| 19 | Before 0.5 Hz/5 A injection | 3680 | 645 |
| 20 | 240 s after 0.5 Hz/5 A injection close | 3920 | 644 |
| 21 | 240 s after 0.5 Hz/5 A injection open | 4210 | 653 |
| 22 | Before 0.5 Hz/5 A injection | 4280 | 648 |
| 23 | 240 s after 0.5 Hz/5 A injection close | 4520 | 654 |
| 24 | 240 s after 0.5 Hz/5 A injection open | 4815 | 663 |
| 25 | Before 1.0 Hz/5 A injection | 5970 | 663 |
| 26 | 150 s after 1.0 Hz/5 A injection close | 6110 | 676 |
| 27 | 150 s after 1.0 Hz/5 A injection open | 6340 | 668 |
| 28 | Before 1.0 Hz/5 A injection | ||
| 29 | 150 s after 1.0 Hz/5 A injection close | 6490 | 660 |
| 30 | 150 s after 1.0 Hz/5 A injection open | 6720 | 668 |
| 31 | Before 1.0 Hz/5 A injection | ||
| 32 | 180 s after 1.0 Hz/5 A injection close | 6900 | 663 |
| 33 | 180 s after 1.0 Hz/5 A injection open | 7130 | 674 |
| 34 | Before 1.0 Hz/5 A injection | ||
| 35 | 180 s after 1.0 Hz/5 A injection close | 7310 | 670 |
| 36 | 180 s after 1.0 Hz/5 A injection open | 7535 | 665 |
| 37 | Before 1.0 Hz/5 A injection | ||
| 38 | 210 s after 1.0 Hz/5 A injection close | 7745 | 674 |
| 39 | 210 s after 1.0 Hz/5 A injection open | 7990 | 669 |
| 40 | Before 1.0 Hz/5 A injection | ||
| 41 | 210 s after 1.0 Hz/5 A injection close | 8200 | 669 |
| 42 | 210 s after 1.0 Hz/5 A injection open | 8450 | 661 |
| Elements in First Principle Circuit | Values | Error | Error% | Elements in Behavioral Circuit | Values | Error | Error% |
|---|---|---|---|---|---|---|---|
| 604.4 μΩ | 10.9 μΩ | 1.8 | 628.2 μΩ | 8.1 μΩ | 1.3 | ||
| 185.9 nH | 4.9 nH | 2.6 | 182.5 nH | 5.2 nH | 2.9 | ||
| 12,300.0 F | 754.9 F | 6.1 | 11,855.0 F | 737.5 F | 6.2 | ||
| 116.2 μΩ | 59.452 μΩ | 51.1 | 410.0 μΩ | 24.6 μΩ | 6.0 | ||
| 467.6 μΩ | 147.8 μΩ | 31.6 | 978.5 μΩ | 42.1 μΩ | 4.3 | ||
| 643.2 μΩ | 153.6 μΩ | 23.9 | 125.2 F | 10.8 F | 8.7 | ||
| 1711.6 μΩ | 153.9 μΩ | 9.0 | 1272.0 F | 111.8 F | 8.8 | ||
| 34.5 F | 19.2 F | 55.7 | |||||
| 83.9 F | 25.7 F | 30.7 | |||||
| 358.0 F | 196.9 F | 55.0 | |||||
| 1036.0 F | 227.0 F | 31.6 | |||||
| Chi-squared | 0.011 | Chi-squared | 0.013 | ||||
| Weighted sum of squares | 0.98 | Weighted sum of squares | 1.27 |
| Elements in First Principle Circuit | Values | Error | Error% | Elements in Behavioral Circuit | Values | Error | Error% |
|---|---|---|---|---|---|---|---|
| 613.2 μΩ | 6.7 μΩ | 1.1 | 668.5 μΩ | 13.5 μΩ | 2.0 | ||
| 184.9 nH | 3.7 nH | 2.0 | 179.3 nH | 9.5 nH | 5.3 | ||
| 2.9 | 4.4 | 15.3 | 2.9 | 7.9 | 27.3 | ||
| 2.95 | 4.8 | 1.6 | 3.1 | 6.6 | 2.1 | ||
| 283.2 μΩ | 54.6 μΩ | 19.3 | 957.0 μΩ | 44.8 μΩ | 4.7 | ||
| 748.9 μΩ | 65.4 μΩ | 8.7 | 8.5 × 1019 Ω | 1 × 1020 Ω | 117.6 | ||
| 1702.2 μΩ | 83.0 μΩ | 4.9 | 258.3 F | 21.8 F | 8.4 | ||
| 2.0 Ω | 2.0 Ω | 100 | 5874.0 F | 585.2 F | 10.0 | ||
| 44.5 F | 7.3 F | 16.4 | |||||
| 148.3 F | 27.5 F | 18.6 | |||||
| 794.0 F | 86.5 F | 10.9 | |||||
| 3884.0 F | 235.3 F | 6.1 | |||||
| Chi-squared | 0.006 | Chi-squared | 0.047 | ||||
| Weighted sum of squares | 0.57 | Weighted sum of squares | 4.42 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, W.; Yao, W.; Chen, W.; Chen, D.; Ma, Z.; Lu, Z. Directional DC Charge-Transfer Resistance on an Electrode–Electrolyte Interface in an AC Nyquist Curve on Lead-Acid Battery. Appl. Sci. 2020, 10, 1907. https://doi.org/10.3390/app10061907
Wang W, Yao W, Chen W, Chen D, Ma Z, Lu Z. Directional DC Charge-Transfer Resistance on an Electrode–Electrolyte Interface in an AC Nyquist Curve on Lead-Acid Battery. Applied Sciences. 2020; 10(6):1907. https://doi.org/10.3390/app10061907
Chicago/Turabian StyleWang, Wubin, Wenxi Yao, Wei Chen, Dong Chen, Zhen Ma, and Zhengyu Lu. 2020. "Directional DC Charge-Transfer Resistance on an Electrode–Electrolyte Interface in an AC Nyquist Curve on Lead-Acid Battery" Applied Sciences 10, no. 6: 1907. https://doi.org/10.3390/app10061907
APA StyleWang, W., Yao, W., Chen, W., Chen, D., Ma, Z., & Lu, Z. (2020). Directional DC Charge-Transfer Resistance on an Electrode–Electrolyte Interface in an AC Nyquist Curve on Lead-Acid Battery. Applied Sciences, 10(6), 1907. https://doi.org/10.3390/app10061907





