Opportunities for Two-Color Experiments in the Soft X-ray Regime at the European XFEL
Abstract
:1. Introduction
2. Project Scope
3. Scientific Relevance
4. Numerical Simulations for Two-Color Generation and Transport
4.1. Generation and Transport of fs-Long Two-Color Pulses in the Low-Charge Mode of Operation
4.2. Performance of the Fresh-Slice Upgrade Option
4.3. Comparison of Low-Charge and Fresh-Slice Options
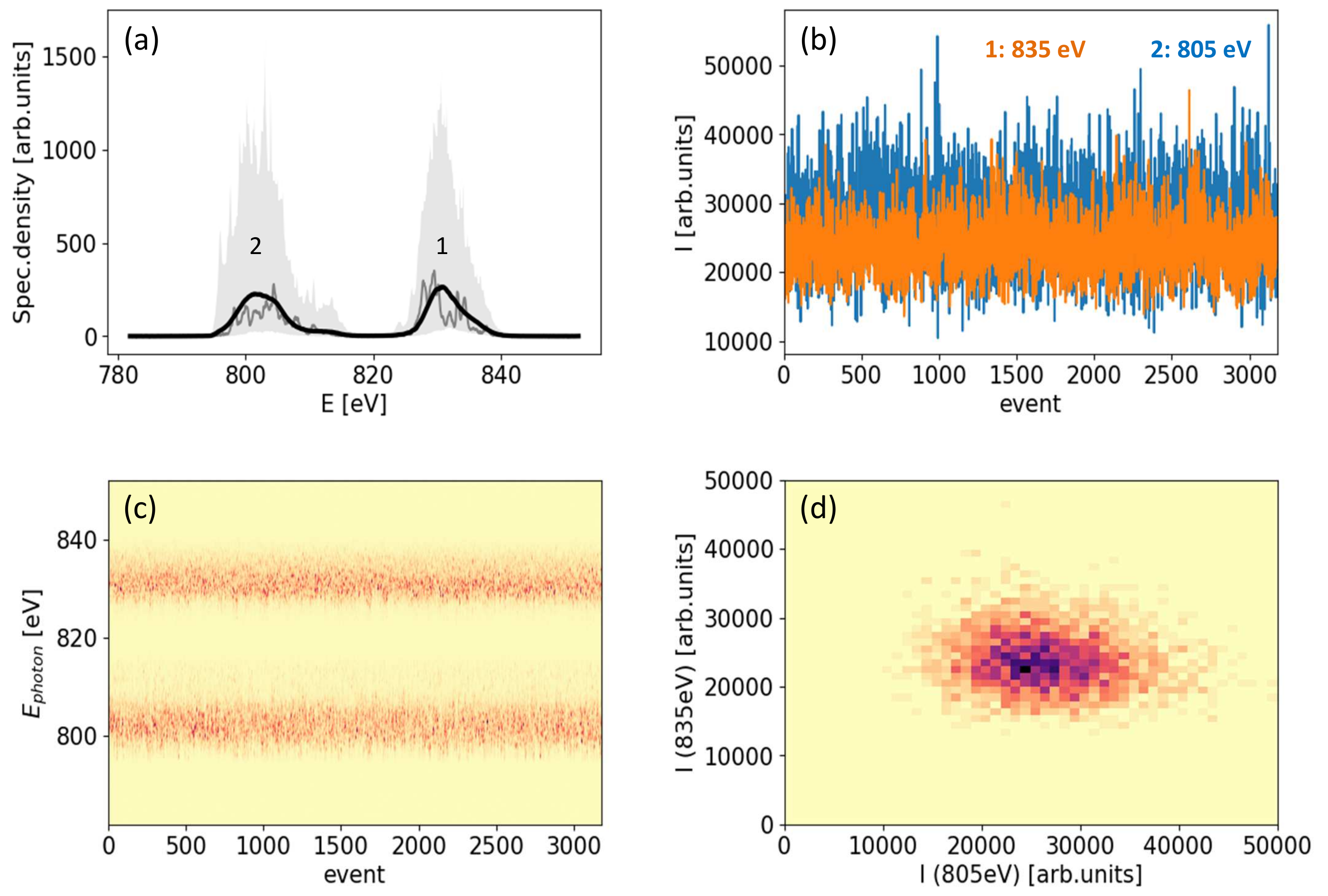
5. Results of First Measurements
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
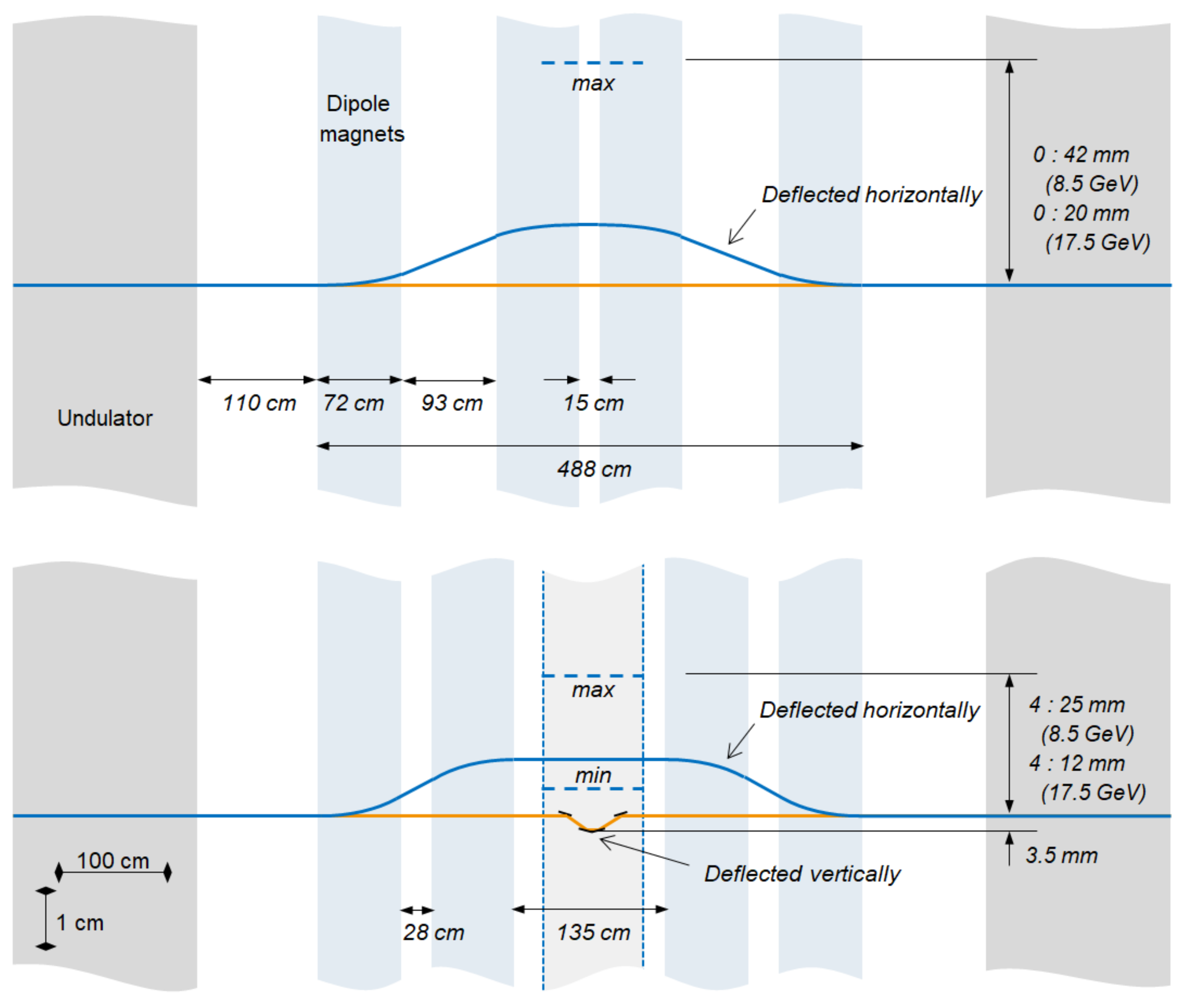
Appendix A. Design Concept


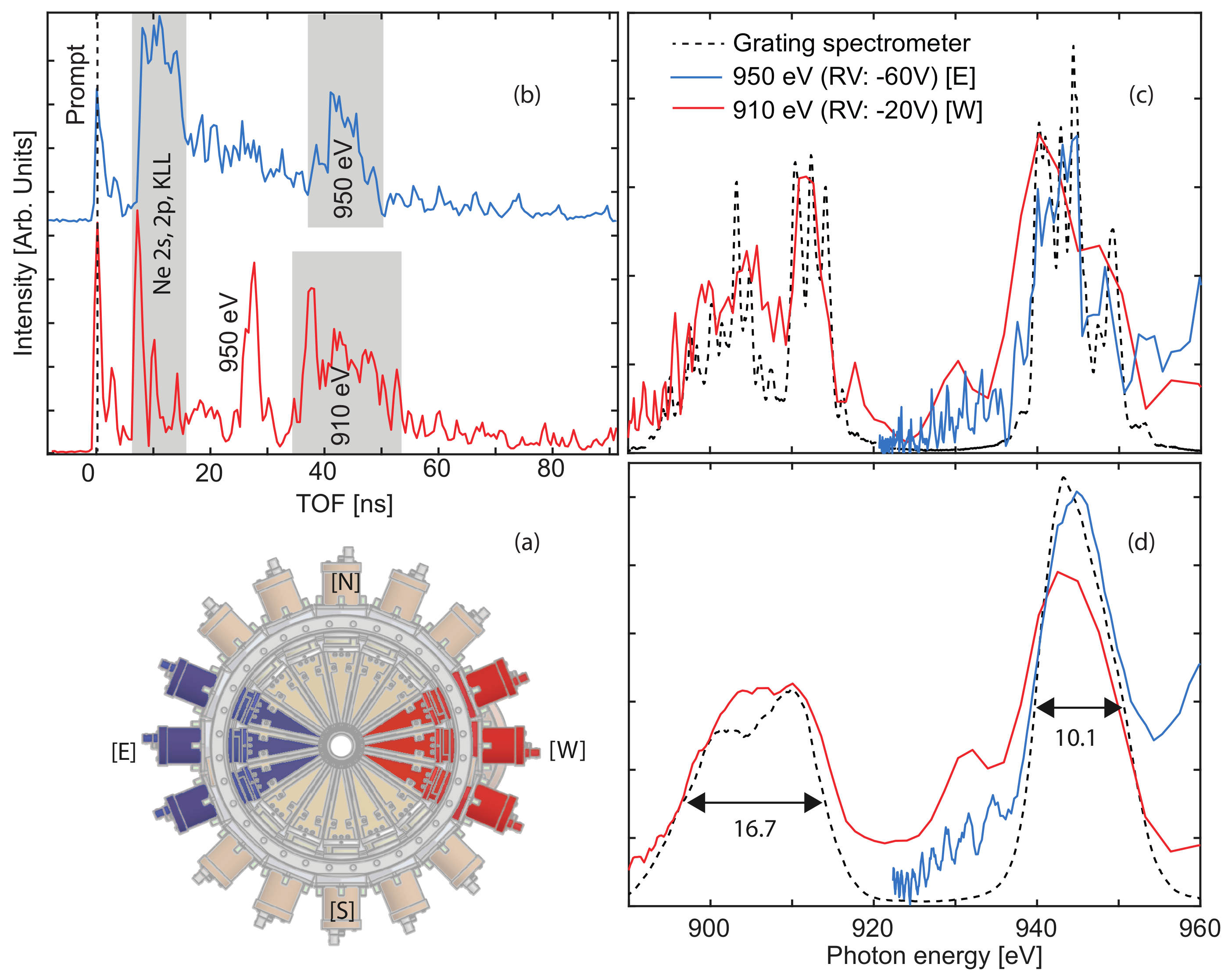
Appendix B. Time-Of-Flight Photo-Electron Spectrometer

References
- Kraus, P.M.; Zürch, M.; Cushing, S.K.; Neumark, D.M.; Leone, S.R. The ultrafast X-ray spectroscopic revolution in chemical dynamics. Nat. Rev. Chem. 2018, 2, 82. [Google Scholar] [CrossRef]
- Pfeifer, T.; Spielmann, C.; Gerber, G. “Femtosecond X-ray science” reports. Prog. Phys. 2006, 69, 443. [Google Scholar] [CrossRef]
- Wuilleumier, F.J.; Meyer, M. Pump–probe experiments in atoms involving laser and synchrotron radiation: An overview. J. Phys. B At. Mol. Opt. Phys. 2006, 39, R425–R477. [Google Scholar] [CrossRef]
- Reduzzi, M.; Carpeggiani, P.; Kühn, S.; Calegari, F.; Nisoli, M.; Stagira, S.; Vozzi, C.; Dombi, P.; Kahaly, S.; Tzallas, P.; et al. Advances in high-order harmonic generation sources for time-resolved investigations. J. Electron Spectrosc. Rel. Phenom. 2015, 204, 257. [Google Scholar] [CrossRef] [Green Version]
- Ullrich, J.; Rudenko, A.; Moshammer, R. Free-Electron Lasers: New Avenues in Molecular Physics and Photochemistry. Annu. Rev. Phys. Chem. 2012, 63, 635. [Google Scholar] [CrossRef]
- Young, L.; Ueda, K.; Gühr, M.; Bucksbaum, P.H.; Simon, M.; Mukamel, S.; Rohringer, N.; Prince, K.C.; Masciovecchio, C.; Meyer, M.; et al. Roadmap of ultrafast X-ray atomic and molecular physics. J. Phys. B At. Mol. Opt. Phys. 2018, 51, 032003. [Google Scholar] [CrossRef]
- Ueda, K.; Sokell, E.; Schippers, S.; Aumayr, F.; Sadeghpour, H.; Burgdörfer, J.; Lemell, C.; Tong, X.; Pfeifer, T.; Calegari, F.; et al. Roadmap on photonic, electronic and atomic collision physics I. Light-matter interaction. J. Phys. B At. Mol. Opt. Phys. 2019, 52, 171001. [Google Scholar] [CrossRef]
- Prince, K.C.; Allaria, E.; Callegari, C.; Cucini, R.; De Ninno, G.; Di Mitri, S.; Diviacco, B.; Ferrari, E.; Finetti, P.; Gauthier, D.; et al. Coherent control with a short-wavelength Free Electron Laser. Nat. Photonics 2016, 10, 176. [Google Scholar] [CrossRef]
- Ferrari, E.; Spezzani, C.; Fortuna, F.; Delaunay, R.; Vidal, F.; Nikolov, I.; Cinquegrana, P.; Diviacco, B.; Gauthier, D.; Penco, G.; et al. Widely tunable two-colour seeded free-electron laser source for resonant-pump resonant-probe magnetic scattering. Nat. Commun. 2016, 7, 10343. [Google Scholar] [CrossRef] [Green Version]
- Marinelli, A.; Ratner, D.; Lutman, A.; Turner, J.; Welch, J.; Decker, F.; Loos, H.; Behrens, C.; Gilevich, S.; Miahnahri, A.; et al. High-intensity double-pulse X-ray free-electron laser. Nat. Commun. 2015, 6, 6369. [Google Scholar] [CrossRef] [Green Version]
- Hara, T.; Inubushi, Y.; Katayama, T.; Sato, T.; Tanaka, H.; Tanaka, T.; Togashi, T.; Togawa, K.; Tono, K.; Yabashi, M.; et al. Two-colour hard X-ray free-electron laser with wide tunability. Nat. Commun. 2013, 4, 3919. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Tschentscher, T.; Bressler, C.; Grünert, J.; Madsen, A.; Mancuso, A.; Meyer, M.; Scherz, A.; Sinn, H.; Zastrau, U. Photon beam transport and scientific instruments at the European XFEL. Appl. Sci. 2017, 7, 592. [Google Scholar] [CrossRef] [Green Version]
- Mazza, T.; Zhang, H.; Meyer, M. Scientific Instrument SQS. Tech. Des. Rep. 2012. [Google Scholar] [CrossRef]
- Scherz, A.; Krupin, O.; Buck, J.; Gerasimova, N.; Palmer, G.; Poolton, N.; Samoylova, L. Scientific Instrument Spectroscopy and Coherent Scattering(SCS). Concept. Des. Rep. 2013. [Google Scholar] [CrossRef]
- Geloni, G.; Kocharyan, V.; Saldin, E. Scheme for femtosecond-resolution pump–probe experiments at XFELs with two-color ten GW-level X-ray pulses. arXiv 2010, arXiv:1001.3510. [Google Scholar]
- Lutman, A.A.; Coffee, R.; Ding, Y.; Huang, Z.; Krzywinski, J.; Maxwell, T.; Messerschmidt, M.; Nuhn, H. Experimental Demonstration of Femtosecond Two-ColorX-ray Free-Electron Lasers. Phys. Rev. Lett. 2013, 110, 134801. [Google Scholar] [CrossRef]
- Lutman, A.A.; Maxwell, T.J.; MacArthur, J.P.; Guetg, M.W.; Berrah, N.; Coffee, R.N.; Ding, Y.; Huang, Z.; Marinelli, A.; Moeller, S.; et al. Fresh-slice multicolour X-ray free-electron lasers. Nat. Phot. 2016, 10, 745–750. [Google Scholar] [CrossRef]
- Liu, S.; Decking, W.; Kocharyan, V.; Saldin, E.; Serkez, S.; Shayduk, R.; Sinn, H.; Geloni, G. Preparing for high-repetition rate hard X-ray self-seeding at the European X-ray Free Electron Laser: Challenges and opportunities. Phys. Rev. AB 2019, 22, 060704. [Google Scholar] [CrossRef] [Green Version]
- Jonauskas, V.; Partanen, L.; Kučas, S.; Karazija, R.; Huttula, M.; Aksela, S.; Aksela, H. Auger cascade satellites following 3d ionization in xenon. J. Phys. B At. Mol. Opt. Phys. 2003, 36, 4403–4416. [Google Scholar] [CrossRef]
- Forbes, R.; De Fanis, A.; Bomme, C.; Rolles, D.; Pratt, S.T.; Powis, I.; Besley, N.A.; Simon, M.; Nandi, S.; Milosavljević, A.R.; et al. Photoionization of the iodine 3d, 4s, and 4p orbitals in methyl iodide. J. Chem. Phys. 2018, 149, 144302. [Google Scholar] [CrossRef]
- Kivilompolo, M.; Kivimäki, A.; Aksela, H.; Huttula, M.; Aksela, S.; Fink, R.F. The gas phase L2,3VV Auger electron spectra of chlorinein XCl (X = H, D, Li, Na, K) molecules. J. Chem. Phys. 2000, 113, 662–675. [Google Scholar] [CrossRef]
- Golubev, N.V.; Kuleff, A.I. Control of charge migration in molecules by ultrashort laser pulses. Phys. Rev. 2015, A91, 051401. [Google Scholar] [CrossRef] [Green Version]
- Erk, B.; Boll, R.; Trippel, S.; Anielski, D.; Foucar, L.; Rudek, B.; Epp, S.W.; Coffee, R.; Carron, S.; Schorb, S.; et al. Imaging charge transfer in iodomethane upon X-ray photoabsorption. Science 2014, 345, 288–291. [Google Scholar] [CrossRef] [PubMed]
- Li, Z.; El-Amine Madjet, M.; Vendrell, O.; Santra, R. Core-level transient absorption spectroscopy as a probe of electron hole relaxation in photoionized H+(H2O)n. Faraday Discuss. 2014, 171, 457–470. [Google Scholar] [CrossRef] [PubMed]
- Abela, R.; Aghababyan, A.; Altarelli, M.; Altucci, C.; Amatuni, G.; Anfinrud, P.; Audebert, P.; Ayvazyan, V.; Baboi, N.; Baehr, J.; et al. XFEL: The European X-ray Free-Electron Laser - Technical Design Report; DESY XFEL Project Group European XFEL Project Team Deutsches Elektronen-Synchrotron Member of the Helmholtz Association: Hamburg, Germany, 2006; ISBN 978-3-935702-17-1. [Google Scholar] [CrossRef]
- Brinkmann, R.; Schneidmiller, E.; Yurkov, M. Possible operation of the Euro-pean XFEL with ultra-low emittance beams. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2010, 616, 81–87. [Google Scholar] [CrossRef] [Green Version]
- Rossbach, J.; Saldin, E.L.; Schneidmiller, E.A.; Yurkov, M.V. Interdependence of parameters of an X-ray FEL. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 1996, 374, 401–407. [Google Scholar] [CrossRef] [Green Version]
- Rossbach, J.; Saldin, E.L.; Schneidmiller, E.A.; Yurkov, M.V. Fundamental limitations of an X-ray FEL operation due to quantum fluctuations of undulator radiation. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 1997, 393, 152–156. [Google Scholar] [CrossRef]
- Zagorodnov, I. DESY MPY Start-to-End Simulations Page. 2014. Available online: http://www.desy.de/fel-beam/s2e/xfel.html (accessed on 15 December 2019).
- Reiche, S. GENESIS 1.3: A fully 3-D time dependent FEL simulation code. Nucl. Instrum. Meth. 1999, A429, 243–248. [Google Scholar] [CrossRef]
- Ocelot Collaboration Project. Available online: https://github.com/ocelot-collab/ocelot (accessed on 15 December 2019).
- Sinn, H.; Dommach, M.; Dong, X.; La Civita, D.; Samoylova, L.; Villanueva, R.; Yang, F. Technical Design Report: X-ray Optics and Beam Transport; European X-ray Free-Electron Laser Facility GmbH: Hamburg, Germany, 2012. [Google Scholar] [CrossRef]
- Zagorodnov, I. Beam Manipulation and Diagnostics with Wakefield Structure at the European XFEL. 2017. Available online: http://www.desy.de/xfel-beam/data/talks/files/2018.05.15_08_15_15_02_1_TEMF2018_Zagorodnov.pdf (accessed on 15 December 2019).
- Gerasimova, N. Performance of the SASE3 Monochromator equipped with Provisional Short Grating; European X-ray Free-Electron Laser Facility GmbH: Schenefeld, Germany, 2018. [Google Scholar] [CrossRef]
- Laksman, J.; Buck, J.; Glaser, L.; Planas, M.; Dietrich, F.; Liu, J.; Maltezopoulos, T.; Scholz, F.; Seltmann, J.; Hartmann, G.; et al. Commissioning of a photoelectron spectrometer for soft X-ray photon diagnostics at European XFEL. J. Synchrotron Rad. 2019, 26, 1010. [Google Scholar] [CrossRef]
- Maltezopoulos, T.; Dietrich, F.; Freund, W.; Jastrow, U.F.; Koch, A.; Laksman, J.; Liu, J.; Planas, M.; Sorokin, A.A.; Tiedtke, K.; et al. Operation of X-ray gas monitors at the European XFEL. J. Synchrotron Rad. 2019, 26, 1045. [Google Scholar] [CrossRef] [Green Version]
- Sokorin, A.A.; Bican, Y.; Bonfigt, S.; Brachmanski, M.; Braune, M.; Jastrow, U.F.; Gottwald, A.; Kaser, H.; Richter, M.; Tiedtke, K. An X-ray gas monitor detector for free-electron lasers. J. Synchrotron Rad. 2019, 26, 1092. [Google Scholar]












| Electron Energy [GeV] | Minimum Photon Energy [eV] | Maximum Photon Energy [eV] | Maximum Delay [fs] |
|---|---|---|---|
| 8.5 | 240 | 1080 | 3000 |
| 12 | 480 | 2160 | 1500 |
| 14 | 650 | 2930 | 1200 |
| 17.5 | 1020 | 3000 | 750 |
| Generation Method, Photon Energies [eV] | 420 eV + 280 eV | 1500 eV + 420 eV | 2000 eV + 1500 eV |
|---|---|---|---|
| Low charge (20 pC) | 100 J, 1 fs + 10 J, 1.5fs | 5 J, 1 fs + 130 J, 1.3 fs | 20 J, 1 fs + 6 J, 1 fs |
| Fresh-slice (2 m structure) | 400 J, 15 fs + 250 J, 15fs | 100 J, 12 fs + 500 J, 12 fs | 80 J, 6 fs + 50 J, 10 fs |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Serkez, S.; Decking, W.; Froehlich, L.; Gerasimova, N.; Grünert, J.; Guetg, M.; Huttula, M.; Karabekyan, S.; Koch, A.; Kocharyan, V.; et al. Opportunities for Two-Color Experiments in the Soft X-ray Regime at the European XFEL. Appl. Sci. 2020, 10, 2728. https://doi.org/10.3390/app10082728
Serkez S, Decking W, Froehlich L, Gerasimova N, Grünert J, Guetg M, Huttula M, Karabekyan S, Koch A, Kocharyan V, et al. Opportunities for Two-Color Experiments in the Soft X-ray Regime at the European XFEL. Applied Sciences. 2020; 10(8):2728. https://doi.org/10.3390/app10082728
Chicago/Turabian StyleSerkez, Svitozar, Winfried Decking, Lars Froehlich, Natalia Gerasimova, Jan Grünert, Marc Guetg, Marko Huttula, Suren Karabekyan, Andreas Koch, Vitali Kocharyan, and et al. 2020. "Opportunities for Two-Color Experiments in the Soft X-ray Regime at the European XFEL" Applied Sciences 10, no. 8: 2728. https://doi.org/10.3390/app10082728
APA StyleSerkez, S., Decking, W., Froehlich, L., Gerasimova, N., Grünert, J., Guetg, M., Huttula, M., Karabekyan, S., Koch, A., Kocharyan, V., Kot, Y., Kukk, E., Laksman, J., Lytaev, P., Maltezopoulos, T., Mazza, T., Meyer, M., Saldin, E., Schneidmiller, E., ... Geloni, G. (2020). Opportunities for Two-Color Experiments in the Soft X-ray Regime at the European XFEL. Applied Sciences, 10(8), 2728. https://doi.org/10.3390/app10082728






