A Numerical and Experimental Study on an Interconnected Metamaterial for Flexural Vibration Control Based on Modal Strain Energy
Abstract
:1. Introduction
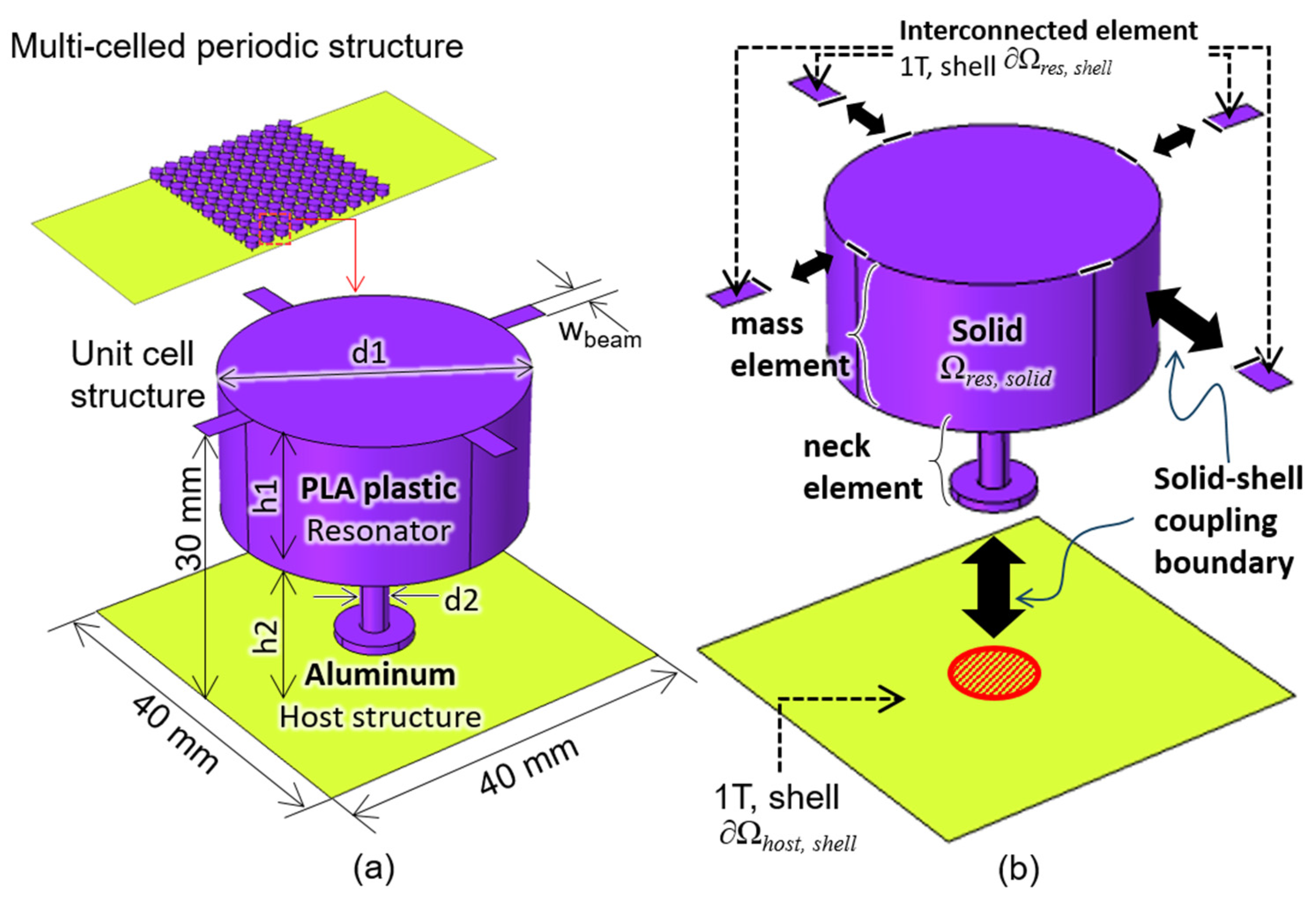
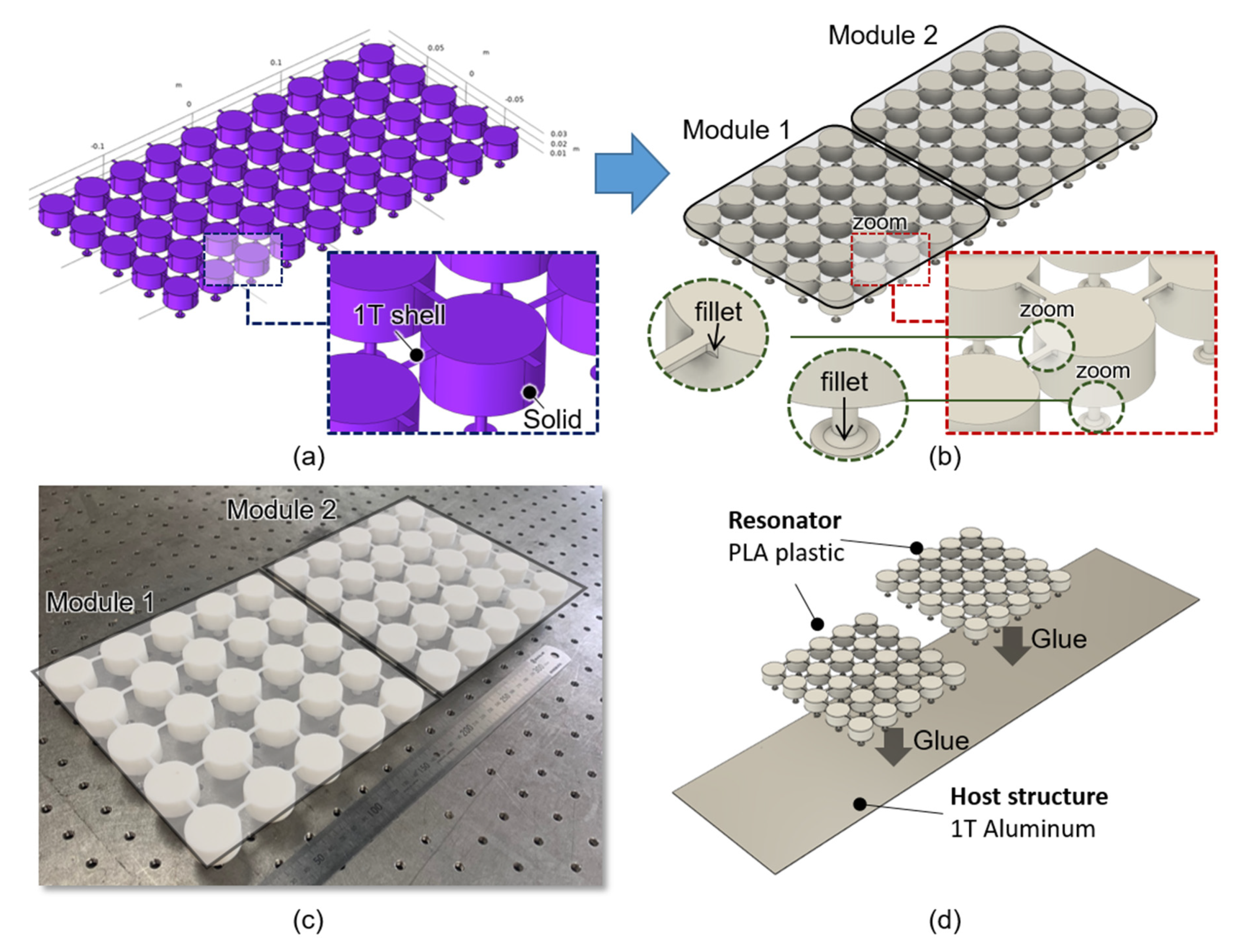
2. Finite Element Modeling of Interconnection Metamaterial
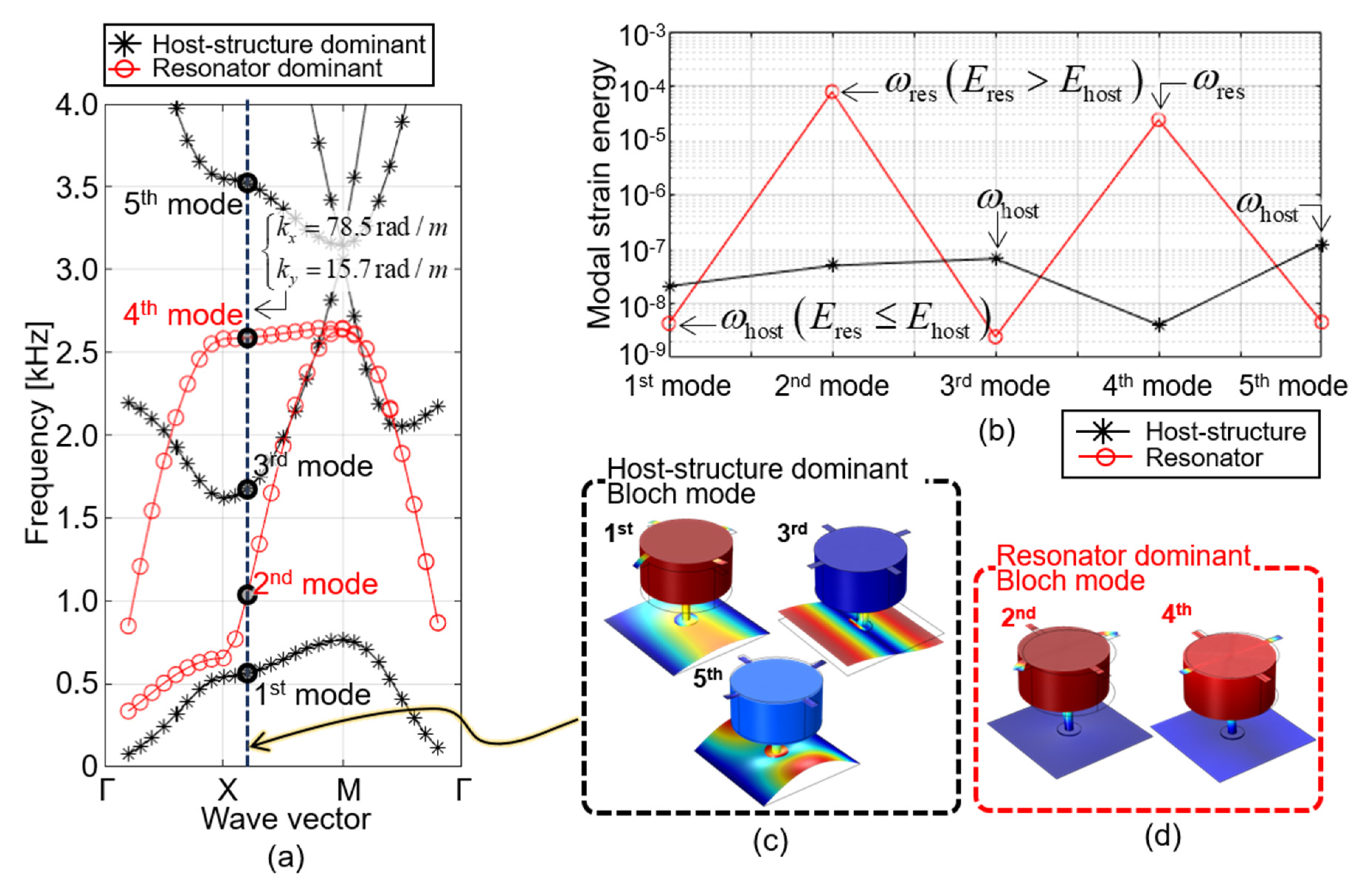
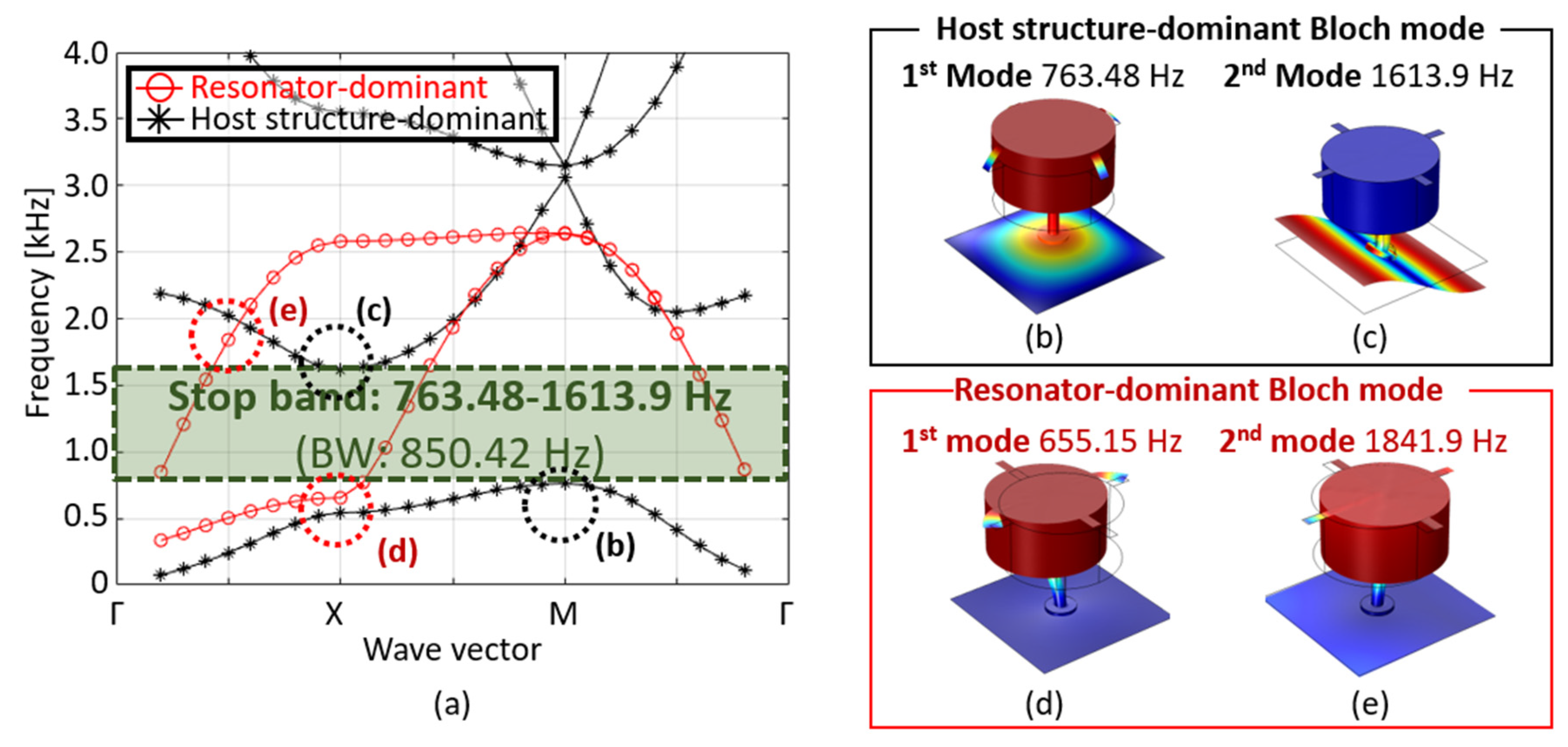
2.1. Stopband Analysis of Interconnected Metamaterials
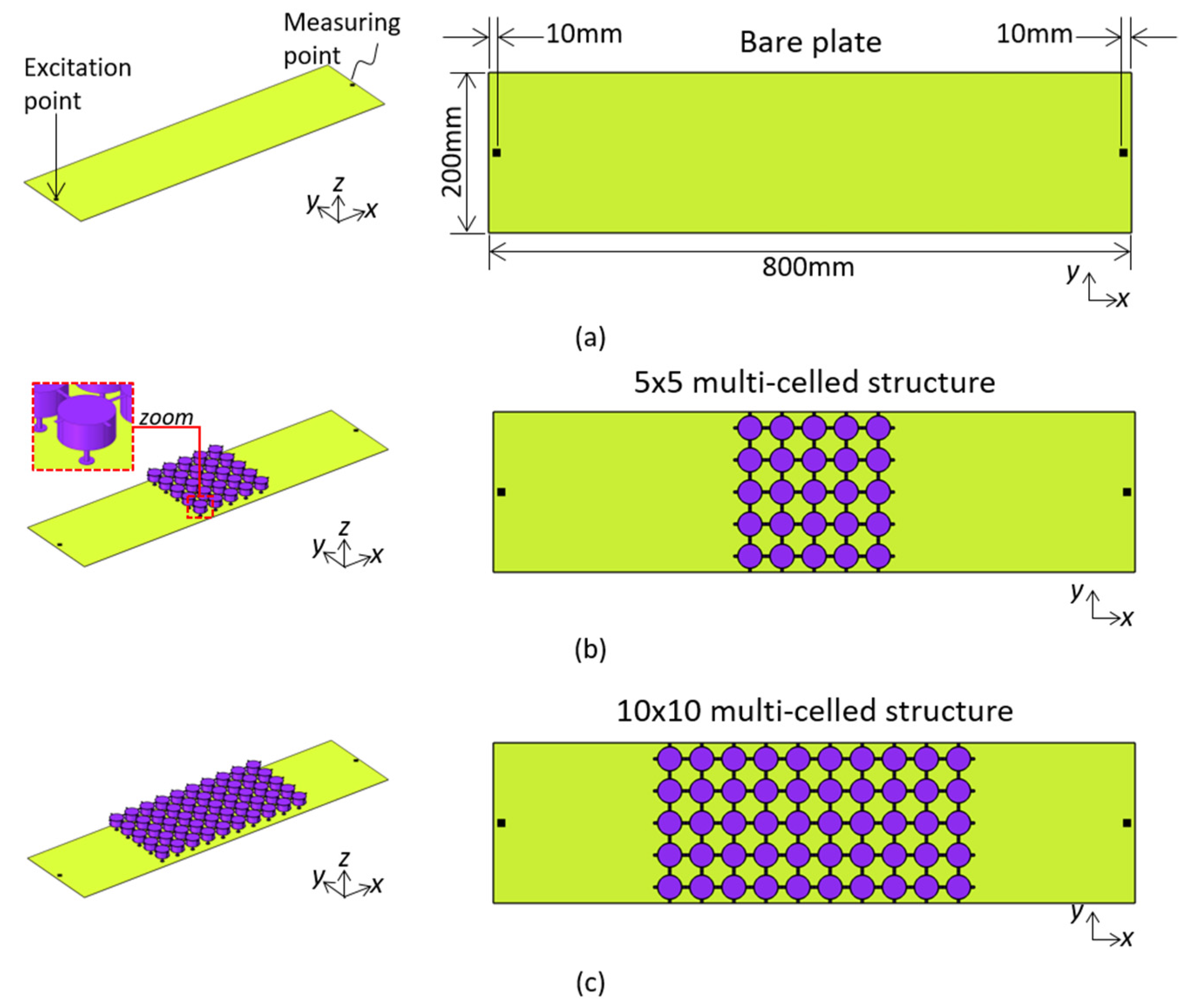
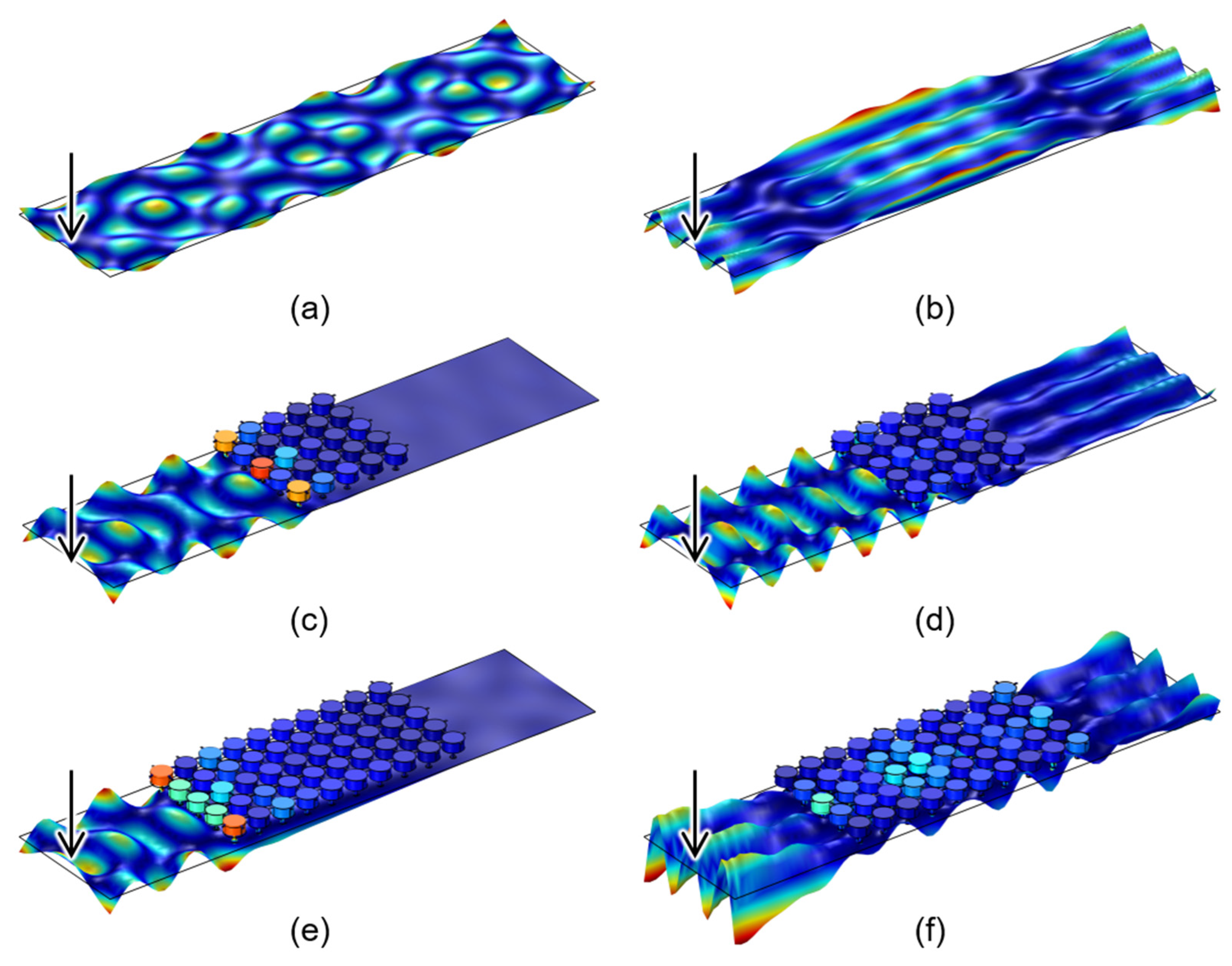
2.2. Frequency Response Analysis for Multi-Celled Interconnected Metamaterial
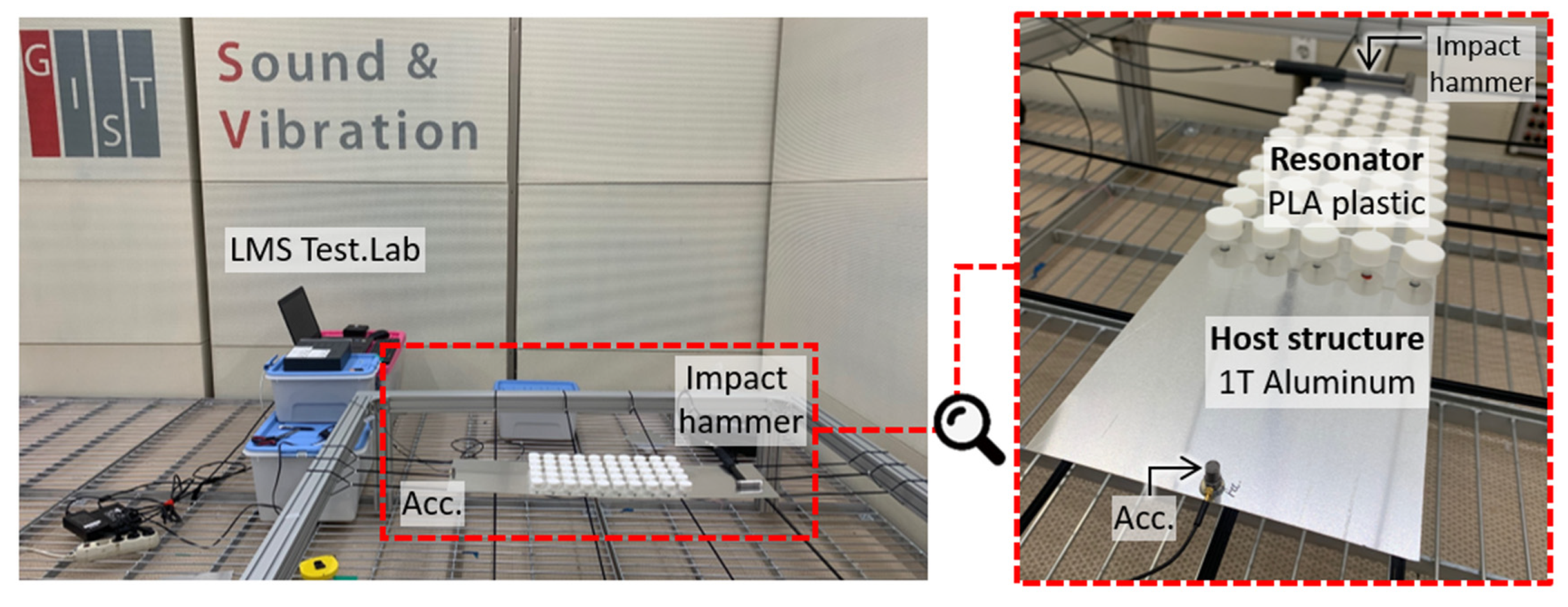
3. Fabrication and Experimental Verification of Interconnected Metamaterials
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Askari, M.; Hutchins, D.A.; Thomas, P.J.; Astolfi, L.; Watson, R.L.; Abdi, M.; Ricci, M.; Laureti, S.; Nie, L.; Freear, S. Ad-ditive manufacturing of metamaterials: A review. Addit. Manuf. 2020, 36, 101562. [Google Scholar]
- Craster, R.V.; Guenneau, S. Acoustic Metamaterials: Negative Refraction, Imaging, Lensing and Cloaking; Springer: Amsterdam, The Netherlands, 2012. [Google Scholar]
- Sharma, G.S.; Skvortsov, A.; MacGillivray, I.; Kessissoglou, N. Sound scattering by a bubble metasurface. Phys. Rev. B 2020, 102, 214308. [Google Scholar] [CrossRef]
- Sharma, G.S.; Skvortsov, A.; MacGillivray, I.; Kessissoglou, N. On superscattering of sound waves by a lattice of disk-shaped cavities in a soft material. Appl. Phys. Lett. 2020, 116, 041602. [Google Scholar] [CrossRef]
- Liu, Z.; Zhang, X.; Mao, Y.; Zhu, Y.Y.; Yang, Z.; Chan, C.T.; Sheng, P. Locally resonant sonic materials. Science 2000, 289, 1734–1736. [Google Scholar] [CrossRef]
- Varanasi, S.; Bolton, J.S.; Siegmund, T.H.; Cipra, R.J. The low frequency performance of metamaterial barriers based on cellular structures. Appl. Acoust. 2013, 74, 485–495. [Google Scholar] [CrossRef] [Green Version]
- Varanasi, S.; Bolton, J.S.; Siegmund, T. Experiments on the low frequency barrier characteristics of cellular metamaterial panels in a diffuse sound field. J. Acoust. Soc. Am. 2017, 141, 602–610. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kim, H.-G.; Goo, S.; Jung, J.; Wang, S. Design optimization of a cellular-type noise insulation panel to improve transmis-sion loss at low frequency. J. Sound Vib. 2019, 447, 105–119. [Google Scholar] [CrossRef]
- Jung, J.; Goo, S.; Wang, S. Investigation of flexural wave band gaps in a locally resonant metamaterial with plate-like resonators. Wave Motion 2020, 93, 102492. [Google Scholar] [CrossRef]
- Jung, J.; Goo, S.; Kook, J. Design of a local resonator using topology optimization to tailor bandgaps in plate structures. Mater. Des. 2020, 191, 108627. [Google Scholar] [CrossRef]
- Jung, J.; Kim, H.-G.; Goo, S.; Chang, K.-J.; Wang, S. Realisation of a locally resonant metamaterial on the automobile pan-el structure to reduce noise radiation. Mech. Syst. Signal Process. 2019, 122, 206–231. [Google Scholar] [CrossRef]
- Sanchez-Perez, J.V.; Rubio, C.; Martinez-Sala, R.; Sanchez-Grandia, R.; Gomez, V. Acoustic barriers based on periodic arrays of scatterers. Appl. Phys. Lett. 2002, 81, 5240–5242. [Google Scholar] [CrossRef]
- Sharma, G.S. Acoustic Performance of Periodic Composite Materials. In Proceedings of the Acoustics 2015 Hunter Valley, Harrogate, UK, 15–18 November 2015; 2015. [Google Scholar]
- Hussein, M.I. Reduced Bloch mode expansion for periodic media band structure calculations. Proc. R. Soc. A Math. Phys. Eng. Sci. 2009, 465, 2825–2848. [Google Scholar] [CrossRef] [Green Version]
- Liu, L.; Hussein, M.I. Wave motion in periodic flexural beams and characterization of the transition between Bragg scattering and local resonance. J. Appl. Mech. 2012, 79, 011003. [Google Scholar] [CrossRef]
- Li, H.; Yang, H.; Hu, S.-L.J. Modal strain energy decomposition method for damage localization in 3D frame structures. J. Eng. Mech. 2006, 132, 941–951. [Google Scholar] [CrossRef]
- Brehm, M.; Zabel, V.; Bucher, C. An automatic mode pairing strategy using an enhanced modal assurance criterion based on modal strain energies. J. Sound Vib. 2010, 329, 5375–5392. [Google Scholar] [CrossRef]
- Hu, W.; Ren, Z.; Wan, Z.; Qi, D.; Cao, X.; Li, Z.; Wu, W.; Tao, R.; Li, Y. Deformation behavior and band gap switching function of 4D printed multi-stable metamaterials. Mater. Des. 2021, 200, 109481. [Google Scholar] [CrossRef]
- Gorshkov, V.; Sareh, P.; Navadeh, N.; Tereshchuk, V.; Fallah, A.S. Multi-resonator metamaterials as multi-band meta-structures. Mater. Des. 2021, 202, 109522. [Google Scholar] [CrossRef]
- An, X.; Lai, C.; Fan, H.; Zhang, C. 3D acoustic metamaterial-based mechanical metalattice structures for low-frequency and broadband vibration attenuation. Int. J. Solids Struct. 2020, 191–192, 293–306. [Google Scholar] [CrossRef]
- Huang, T.-Y.; Shen, C.; Jing, Y. Membrane- and plate-type acoustic metamaterials. J. Acoust. Soc. Am. 2016, 139, 3240–3250. [Google Scholar] [CrossRef] [Green Version]
- Elford, D.P.; Chalmers, L.; Kusmartsev, F.V.; Swallowe, G.M. Matryoshka locally resonant sonic crystal. J. Acoust. Soc. Am. 2011, 130, 2746–2755. [Google Scholar] [CrossRef] [Green Version]
- Comsol Multiphysics Users Guide. 2011. Available online: https://blogs.ethz.ch/ps_comsol/files/2020/05/COMSOLMultiphysicsUsersGuide.pdf (accessed on 15 May 2021).
- Tofangchi, A.; Han, P.; Izquierdo, J.; Iyengar, A.; Hsu, K. Effect of ultrasonic vibration on interlayer adhesion in fused fila-ment fabrication 3D printed ABS. Polymers 2019, 11, 315. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sharma, G.S.; Faverjon, B.; Dureisseix, D.; Skvortsov, A.; MacGillivray, I.; Audoly, C.; Kessissoglou, N. Acoustic performance of a periodically voided viscoelastic medium with uncertainty in design parameters. J. Vib. Acoust. 2020, 142, 061002. [Google Scholar] [CrossRef]




| Parameter | Value (mm) |
|---|---|
| 25 | |
| 3 | |
| 15 | |
| 15 | |
| 2.5 |
| Resonator | Host Structure | |
|---|---|---|
| Type | PLA plastic | Aluminum |
| Elastic modulus (GPA) | 4.16 | 71.89 |
| Poisson’s ratio (1) | 0.403 | 0.284 |
| Density (kg/m3) | 1400 | 2669 |
| Properties | Values |
|---|---|
| Infill density | 100% |
| Layer height | 0.2 mm |
| Printing velocity | 40 mm/s |
| Fixing bed | Raft |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, H.-G.; Jeon, O.; Wang, S. A Numerical and Experimental Study on an Interconnected Metamaterial for Flexural Vibration Control Based on Modal Strain Energy. Appl. Sci. 2021, 11, 4530. https://doi.org/10.3390/app11104530
Kim H-G, Jeon O, Wang S. A Numerical and Experimental Study on an Interconnected Metamaterial for Flexural Vibration Control Based on Modal Strain Energy. Applied Sciences. 2021; 11(10):4530. https://doi.org/10.3390/app11104530
Chicago/Turabian StyleKim, Hyun-Guk, Onyu Jeon, and Semyung Wang. 2021. "A Numerical and Experimental Study on an Interconnected Metamaterial for Flexural Vibration Control Based on Modal Strain Energy" Applied Sciences 11, no. 10: 4530. https://doi.org/10.3390/app11104530
APA StyleKim, H.-G., Jeon, O., & Wang, S. (2021). A Numerical and Experimental Study on an Interconnected Metamaterial for Flexural Vibration Control Based on Modal Strain Energy. Applied Sciences, 11(10), 4530. https://doi.org/10.3390/app11104530






