1. Introduction
In the last years, open cell metal foams have been extensively investigated [
1]. Metal foams are cellular structured materials consisting of stochastically oriented open cells. Their struts interconnect at nodes assuming the conventional shape of an open cell. Stochastic metal foams have specific features which make them suitable to be implemented and integrated in different applications related to heat transfer processes [
2]. Open foams are characterized by high heat transfer area to volume ratio, attractive stiffness and strength, and they possess the ability to enhance the flow mixing. As an enhancement heat transfer technique, metal foams can be adopted in different fields, for instance: biomedical, geothermal operations, multifunctional heat exchangers, and cladding on buildings, as an air cooling means for condensers or for acting as compact heat sinks for power electronics. They have been proposed to be an interesting solution to solve the limited air cooling capacity in electronic equipment [
3]. The thermal-hydraulic behavior of stochastic metal foams depends upon their geometrical characteristics such as the pore diameter, the pore density (number of pores per linear inch), struts diameter and length, the porosity, and the relative density as well as on the operating conditions. The porosity and the relative density are defined as the ratio between the volume of void and the total volume, and the ratio between density of the foam and of the metal one, respectively [
2].
Extensive experimental studies involving open cell foams can be retrieved in the open literature. The influence of geometrical features on the pressure drop and heat transfer performance were investigated by Lu et al. [
4]. Adopting the Brinkman-extended Darcy momentum model and a heat transfer one developed for porous structures based on two equations, they obtained that, compared with the reference tubes, the metal foams performed up to 40 times better if considering the heat transfer. Aluminum metal foams with pore densities ranging from 10 to 45 PPI and porosities from 75% to 85% were used by Incera Garrido et al. [
5]. The authors aimed at analysing mass transfer and pressure drop properties. Results revealed that the higher the pore density, the higher the pressure drops. On the contrary, the lower the porosity, the higher the pressure drops. Yu et al. [
6] investigated the air forced convection using five graphite foams. By means of an electrical device, the samples were heated and thermal properties were analyzed. The authors stated that the pressure drop increases as the pore dimension decreases whilst the thermal performance improves as the inlet velocity increases. Kim et al. [
7] focused on forced convection across aluminum foams to study the effect of the porosity on the heat transfer performance. Three samples had constant porosity (0.92) and pore densities between 10 and 40 PPI, while the other three presented different porosities but constant pore density (20 PPI). Zhao et al. [
8] experimentally evaluated the thermal-hydraulic behavior of six copper alloy foams. They measured heat transfer coefficient and pressure drops, finding that the overall heat transfer was affected by cell size more than by porosity. Aiming at obtaining a trade-off between pressure drops and heat transfer, Zhao et al. [
8] proposed an optimal porosity which rose as Reynolds number reduced. Abadi et al. [
9] experimentally investigated 20 to 60 PPI metal foams by measuring heat transfer coefficient and pressure drop. If compared to the compact-plate heat exchanger with empty channels, the foamed one presented heat transfer coefficients which increased from 3.5 to 5.1 times and pressure drops from 3.2 to 5.7 times.
From this brief overview, stochastic open cell foams can potentially improve the thermal performance in air forced convection as compared to other extended surfaces. At the same time, it was demonstrated how their geometrical parameters highly influenced their performance. Besides, it must be considered that, due to their intrinsic stochastic nature, they cannot be reproduced with always the same geometrical characteristics. It is evident that this can limit their heat transfer enhancement capacity, and, consequently, their adoption in practical applications.
Therefore, periodic metal foams (i.e., known as periodic cellular structures) have recently received great attention by researchers. The potentiality of periodic foams is, undoubtedly, their tunability [
10], which can lead to a high level of final product customization [
11]. Nowadays, different technologies can support the ordered foam development and diffusion [
12]. One of them is the additive manufacturing (AM) technology. The latter has been continuously developing and it is considered suitable to generate complex periodic structures [
13] with, in some cases, affordable costs [
14].
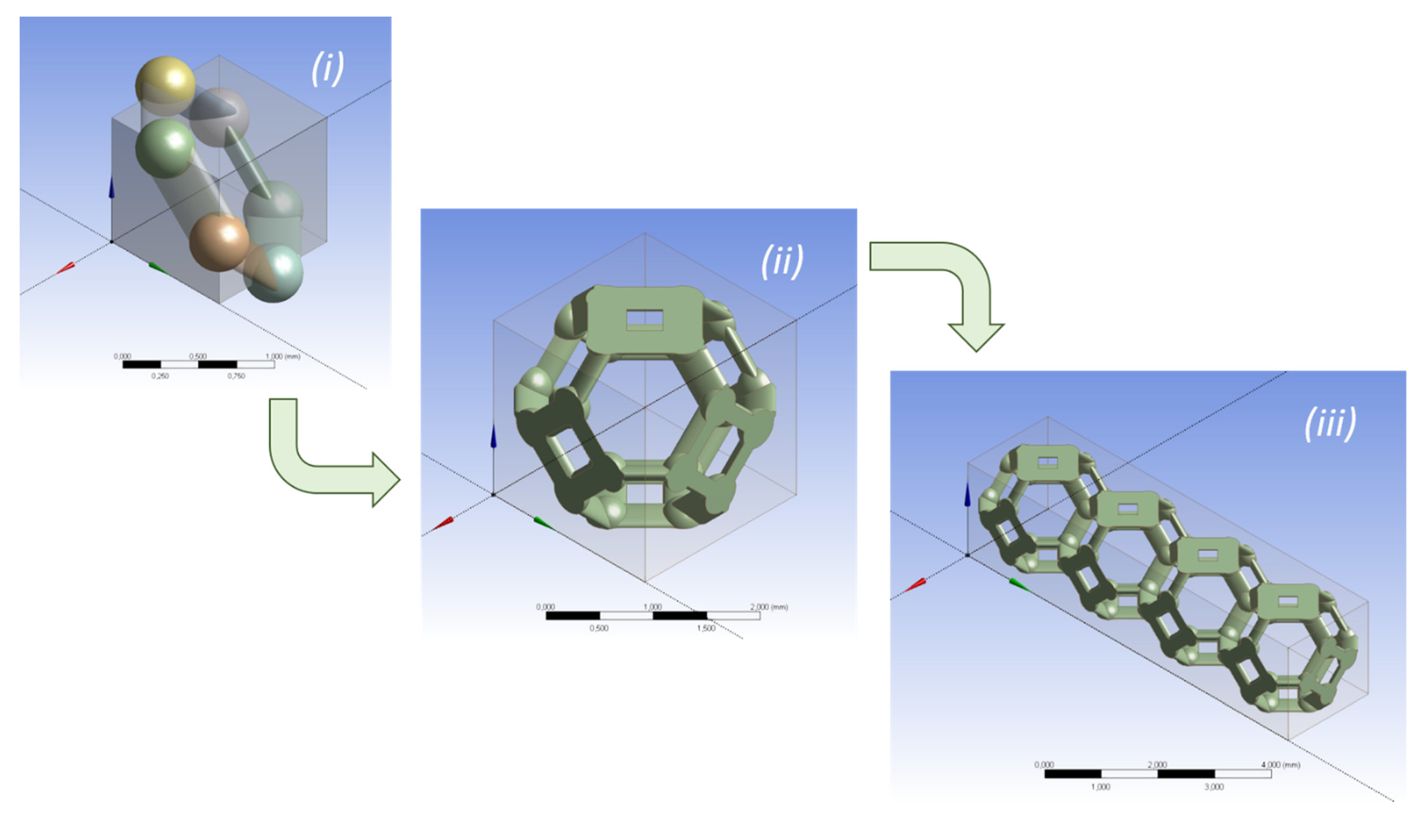
Pioneering work on ordered foams can be attributed to Lord Kelvin [
15]. The author revealed that the best approximation of an open cell foam can be obtained by the replication of a single cell shaped as a tetrakaidecahedron with slightly curved faces. Lord Kelvin [
15] considered the so-built tetrakaidecahedron to be the cell shape which caused the surface area per unit volume to be minimized. Starting from Lord Kelvin’s [
15] work, Weaire and Phelan [
16] developed a new structure consisting of 8 isovolumetric polyhedra. Six of them have 14-faced cells (12 pentagonal faces and 2 hexagonal ones) whilst the others are two pentagonal dodecahedra. Compared with a same-volume porous structure made of Lord Kelvin cells, the Weaire and Phelan [
16] one leads to a surface area which is some of 0.3% smaller. Even though other models can be retrieved in literature [
17,
18,
19,
20,
21], Lord Kelvin [
15] and Weaire and Phelan [
16] ones are considered the best representation of real open cell foams [
22].
From a numerical point of view, one approach to investigate thermal-hydraulic behavior of stochastic foams is to firstly reproduce them via tomography-based techniques [
23,
24] and, successively, importing the obtained foams in a CFD software. It is known that numerical analyses can usually be cheaper than experimental ones. Nevertheless, the generation of the geometry by means of computational techniques is the cheapest way to describe transport phenomena [
22]. Therefore, generating periodic cell foams seems suitable to address this purpose. Iasiello et al. [
25] made a comparison between numerically estimated heat transfer coefficients and pressure drops in a structure obtained by means of tomography-based procedure with the same variables calculated in Lord Kelvin’s structure. Cunsolo et al. [
22] adopted the Lord Kelvin and Weaire–Phelan models to find a simple geometric approximation of an open cell to describe convective heat transfer and pressure drop. The authors compared the two models. Different porosities (0.85, 0.9, 0.95) were tested. In terms of friction factor, Lord Kelvin structures led to 10% always lower values than Weaire–Phelan ones. Therefore, it was stated that the simplest Lord Kelvin cells did not affect the solution accuracy significantly.
As it was affirmed for stochastic open cell foams, geometrical parameters influence the thermo fluid dynamic performance even of the structured porous media. Additionally, in periodic foams, the strut shapes were found to have a not-negligible impact on the thermal-hydraulic behavior. Ambrosio et al. [
26] investigated metal foams with concave or convex triangular struts at constant porosity. The authors revealed that the concave one showed better convective heat transfer performance with contemporary lower pressure drop than the convex one. Moon et al. [
27] studied pressure drop and heat transfer performance of Kelvin cell-based foams with circular and elliptical struts. In particular, the authors transformed the circular struts into elliptical ones by adopting two different criteria: the first was to maintain the same section strut perimeter while the second was to maintain constant the section area. Different air velocities were tested. The elliptical struts obtained through the first criterion led to 32% lower pumping power being needed if compared with circular struts.
Nevertheless, to the best of the authors’ knowledge, the effect of fiber orientation has not been investigated yet. Therefore, in this work, starting from Kelvin cell-based foams developed by Moon et al. [
27], four different fiber orientation angles were tested (0, 15, 30, 45°) with respect to the main air flow. Air forced convection was solved by means of numerical simulations run through Ansys Fluent 18.2. Five different air inlet velocities, corresponding to five different Reynolds numbers, were set. Results revealed that by increasing the elliptical struts orientation (up to 30°), a significant reduction in pressure drops as velocity increased, if compared with the circular struts. On the contrary, only the 45° foams presented a worse hydraulic behavior. Focusing on the thermal performance, uniform results were not obtained, and these are accurately discussed in the following sections.
3. Results
As shown in
Figure 6a,b, an additional plane (denoted as “Plane+”) was used to capture the velocity, pressure and temperature contours inside the foams. The plane was inclined of 45° with respect to the horizontal axis in order to comprehensively investigate the hydraulic and thermal performances of the air crossing the periodic aluminum foams.
To analyze the effect of the elliptical fiber orientation on the heat transfer and pressure drops with respect to the reference circular strut (subscript “0”), the ratios of Colbourn numbers
and friction factors
as function of the air mass velocity (
G) were used, respectively. The friction factor
f was calculated by Equation (4), while the Colbourn number
j was obtained by means of the following equation:
HTC (W m
−2 K
−1) is the interstitial convective heat transfer coefficient defined as the ratio between the thermal power
Q (see Equation (5)) and the total heat transfer area
A multiplied by the logarithmic mean temperature difference
, as shown in Equation (7):
G (kg m
−2 s
−1), mass velocity, is defined as the product between the air density and the air velocity:
As shown in
Figure 7, results for E2-1, E1-2, and E2-2 were reported. To avoid redundancy, graphs for E1-1 foams were not considered due to their strong geometrical similarity and thermal-hydraulic behavior with E2-1 ones (see
Table 1).
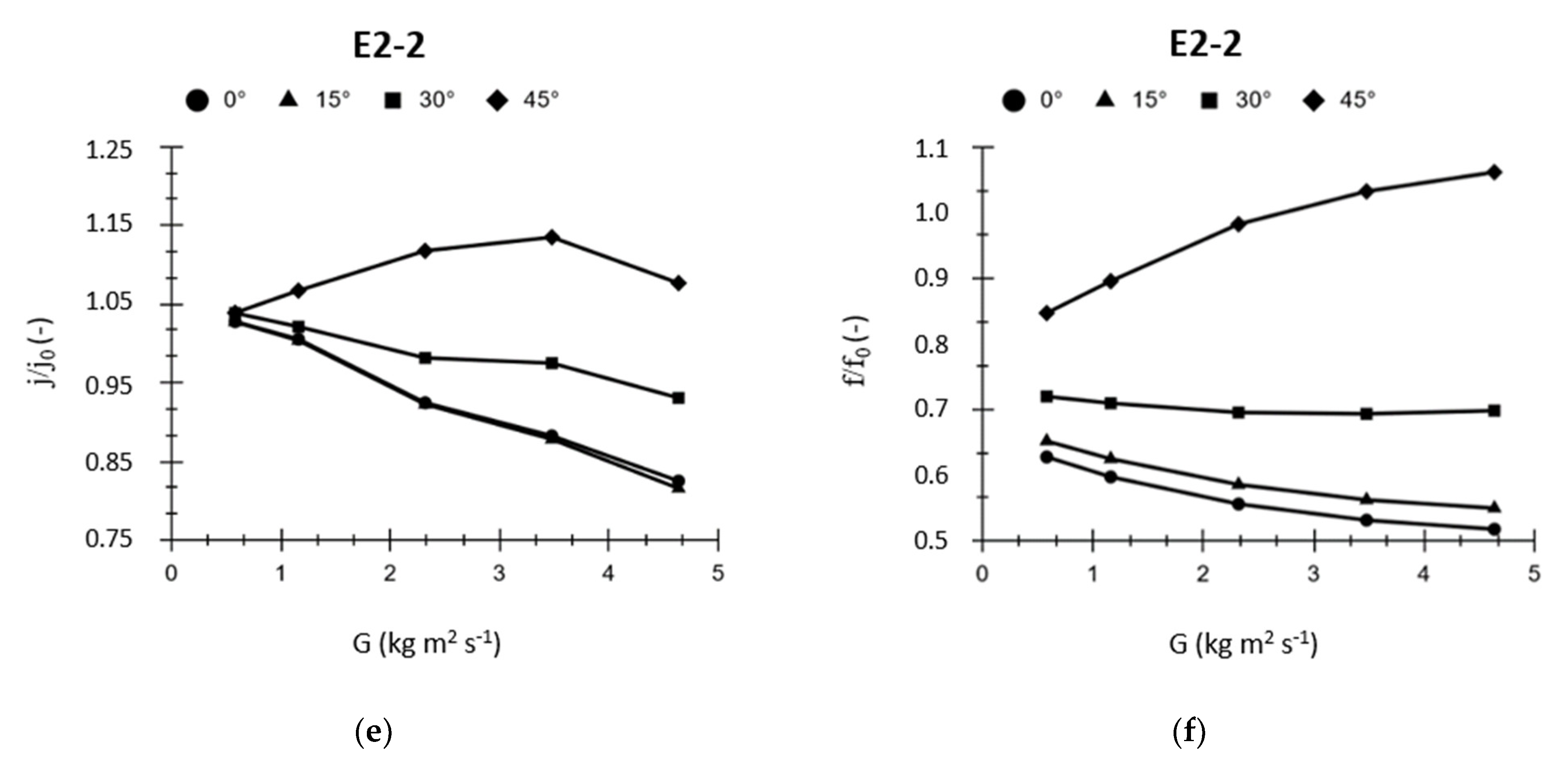
Focusing on the heat transfer performance, it is interesting to notice that the elliptical E2-1 foams (
Figure 7a) outperformed the reference circular ones for all the investigated angles of attack at all the air mass velocities. In fact, the ratio
j/j0 is always greater than 1. However, it must be underlined that while 0–15–30° show a substantial decrease of the rate of heat transfer enhancement as the velocity increases, the 45° cases show a growing enhancement rate only up to 3 m s
−1 and then they start decreasing with a slope comparable with other fibers’ inclination. Hence, inlet velocity equals to 3 m s
−1 can be considered as the point of maximum. The same trends were obtained for E1-2 (
Figure 7c) and E2-2 (
Figure 7e).
This phenomenon can be explained by investigating the contours of air velocity inside the foams before the 3 m s
−1 (i.e., 2 m s
−1 inlet velocity) (
Figure 8a,c,e,g) and this value (i.e., 4 m s
−1 inlet velocity) (
Figure 8b,d,f,h). At 2 m s
−1, the highest value of the velocity through the fibers grows as the orientation increases. In fact, it can be noticed that from 0° (
Figure 8a) to 45° (
Figure 8g), the maximum velocity increases from 5.15 m s
−1 to 6.17 m s
−1. Therefore, the increasing tortuosity could justify the enhancement of the heat transfer due to the continuous mixing of the fluid. Nevertheless, considering 4 m s
−1 inlet velocity something different can be inferred. All the foams show longer stagnation zones behind the fibers, i.e., dead zone. The stagnation zones can be detrimental for the heat transfer, because extremely low velocities cause the convective heat transfer coefficient to decrease. Moreover, for 4 m s
−1, the local maximum velocity is not completely developed as it was for 2 m s
−1. The worst case is reached with 45° fibers’ inclination (
Figure 8h) because the velocity achieves its maximum value only in the central zones of the periodic foam.
In
Figure 9, the negative effect of the stagnation zones can be appreciated. In fact, as shown in
Figure 9a, the air temperature behind the fibers is higher if compared with air temperature shown in
Figure 9b. Despite that, the latter presents a
j/j0 ratio which is over the unity (1.064). This means that E1-2, 45° performs better than the reference circular struts even at the highest investigated velocity.
Nevertheless, when considering a possible application of heat exchangers adopting periodic open cell metal foams, it is needed to deeply investigate even the hydraulic performance of periodic foams.
From
Figure 7b,d,f, it can be noticed that the elliptical foams, up to 30° fiber inclination, present lower (or, at most, equal) friction factors if compared with the reference structures. Moreover, the friction factor ratios tend to decrease as the velocity increases. Only the 45° oriented struts show
f/f0 always greater than 1 for all the 5 investigated inlet velocities. When comparing the velocity contours at fixed inlet velocity (for example, 4 m s
−1,
Figure 8b,d,f,h, it is clear that, as the angle attack increases, a greater frontal fiber surface is seen by the airflow. Therefore, the greater frontal surface acts as an additional obstacle to the main flow. If the inclination of 45° (
Figure 8h) is likened to 0° (
Figure 8b), it is evident how the stagnant zones are remarkably more extended in the first case as compared to the second one, justifying the increase in pressure drops (in terms of friction factors). From the hydraulic performance point of view, the elliptical E2-2 Kelvin cell-based metal foam (
Figure 7f) can be considered the worst case: in fact, 30° presents a
f/f0 curve which flattens along the unity, meaning that no hydraulic performance improvement can be obtained with respect to the circular struts’ reference foam. Moreover, even worse, 45° (
Figure 7f) shows a noticeable increase in
f/f0 curve which, at 4 m s
−1, is almost two times higher than the value for reference case (
f/f0 = 1.904).
For the same orientation and inlet velocity values, E1-2 has only some of 7% greater friction factor value as compared to C0 (f/f0 = 1.071).
The relevant different hydraulic behavior can be attributed to their intrinsic geometrical properties. From
Table 1, it can be noticed that both foams present the same β (0.556) but different α (1.359 for E1-2, 1.800 for E2-2). A greater α results in a higher frontal surface seen by the air, causing additional flow disturbances and flow restrictions (
Figure 10) as well as an increase in abrupt pressure drops (
Figure 11), consequently. Besides, more inhomogeneous pressure gradients can be observed for E2-2 if compared with E1-2, thus affecting the hydraulic behavior negatively.
As shown in
Figure 11b, E2-2 can have local pressure gradients which can be some of 65% higher than E2-1. In fact, a maximum local pressure difference of 246.7 Pa was calculated for E2-2, being 150.6 Pa for E1-2.
By employing the Darcy–Forcheimer equation (Equation (10)), which approximates the pressure gradient’s curve with a second-order function of the parameter χ defined as the product of the foam porosity and the inlet velocity, the permeability
K (Equation (11)) and the Forcheimer coefficient
φ (Equation (12)) for all the simulated geometries can be calculated, and the results are reported in
Figure 12.
For the sake of brevity, only the values of pressure gradients for E1-2 and E2-2 were reported in
Figure 13.
It can be appreciated that for all the fiber orientations, the pressure drops have a parabolic trend, which is in line with Equation (10). As the angle of attack increases, for a given inlet velocity, the pressure gradient increases. In fact, as shown in
Figure 12, 45° fiber inclination foams present greater values of K and
φ if compared against the same geometry at lower angles of attack. It can be concluded that, from a hydraulic point of view, an increase of the angle of attack results in a pressure drop increase.
Nevertheless, aiming at establishing the best thermal performance among the different investigated configurations, it is interesting to analyze the interstitial HTC (Equation (7)) as a function of the pumping power per area density (PPD) (W m
−2). This parameter is defined as the ratio between the product of pressure drops Δ
P (Pa) and volumetric flow rate
(m
3 s
−1), and heat transfer area
A (m
2) as given by:
From
Figure 14, the results show that E1-1 and E1-2 (which are very similar, see
Table 1) present a better thermal performance if compared with other configurations. Assuming a constant value for the PPD, E1-1 and E1-2 give a value for HTC which is always higher, for all the investigated fiber orientations.
Nevertheless, considering a possible implementation of periodic cell metal foams in real applications, it is fundamental to analyze the global performance of the foams. In particular, it must be taken into account the contemporary needs of high thermal transfer characteristics and low pressure drops. Usually, a trade-off between these two conflicting needs must be found.
Therefore, among the possible Performance Evaluation Criteria (PEC) [
28], the following one, as reported in Equation (14), was used as a function of Reynolds number (Equation (15)), to compare the different configurations:
Focusing on
Figure 15, the PEC as function of Re can be appreciated. It is evident that the elliptical struts overcome the circular ones for almost all the investigated configurations. Only E2-2 30° and 45° present PEC values lower than C0. For all the Kelvin cell- based metal foams, the global performance becomes worse as the fiber inclination increases for all the Re numbers. As already explained, higher angles of attack led to a better thermal performance at the expanse of pressure drop. Therefore, if interested in evaluating the global enhancement of the thermal-hydraulic behavior of the elliptical struts’ foams with respect to circular ones, E1-2 with 0° angle of attack can be proposed as the best compromise, reaching a PEC value which is 22% higher than that for reference case, at Re = 200.