Stability Analysis of Soil Flow Protector and Design Method for Estimating Optimal Length
Abstract
:1. Introduction
2. Scaled Model Experiment
2.1. Test Programs
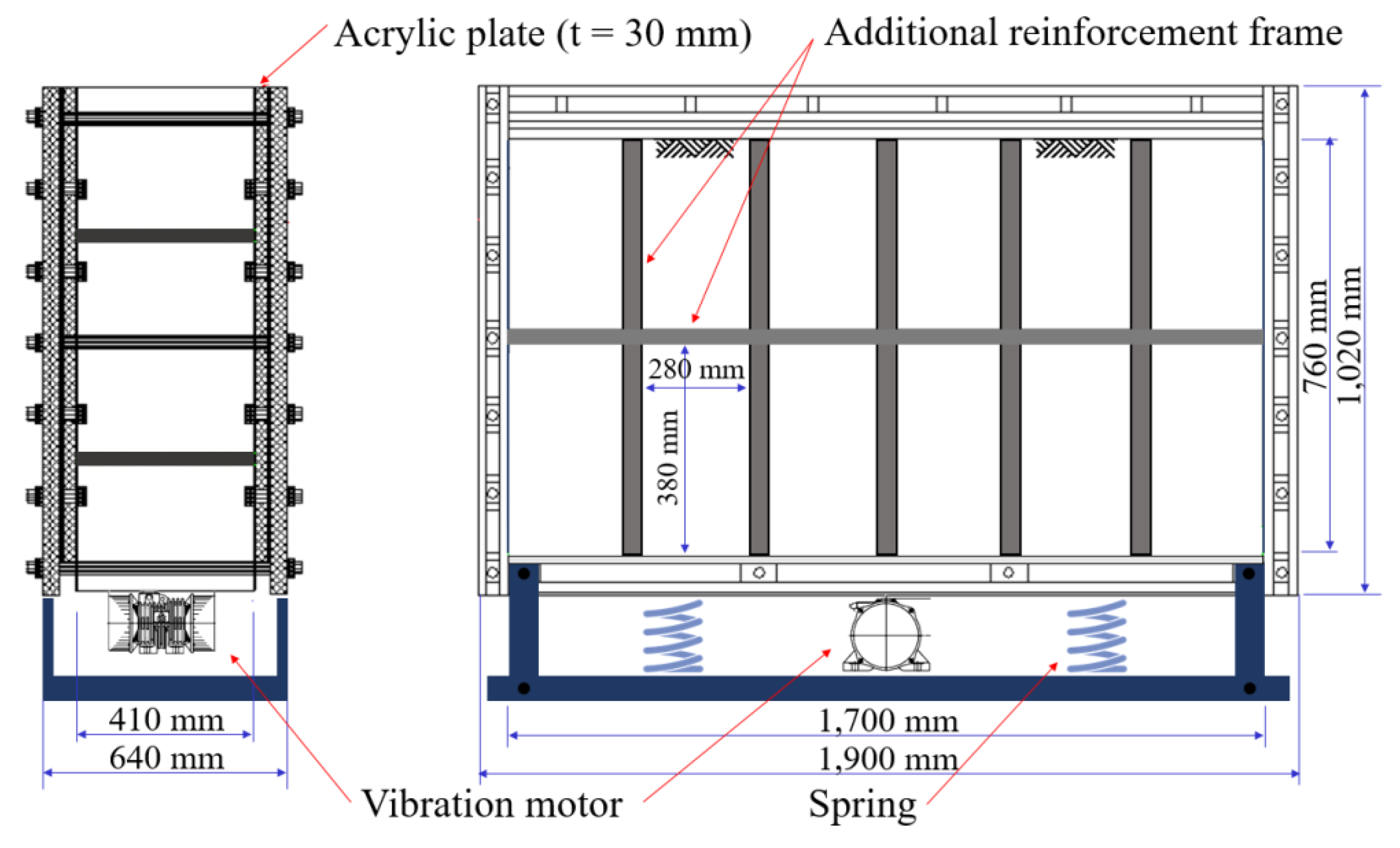
2.2. Experimental Setup
2.2.1. Similitude Law
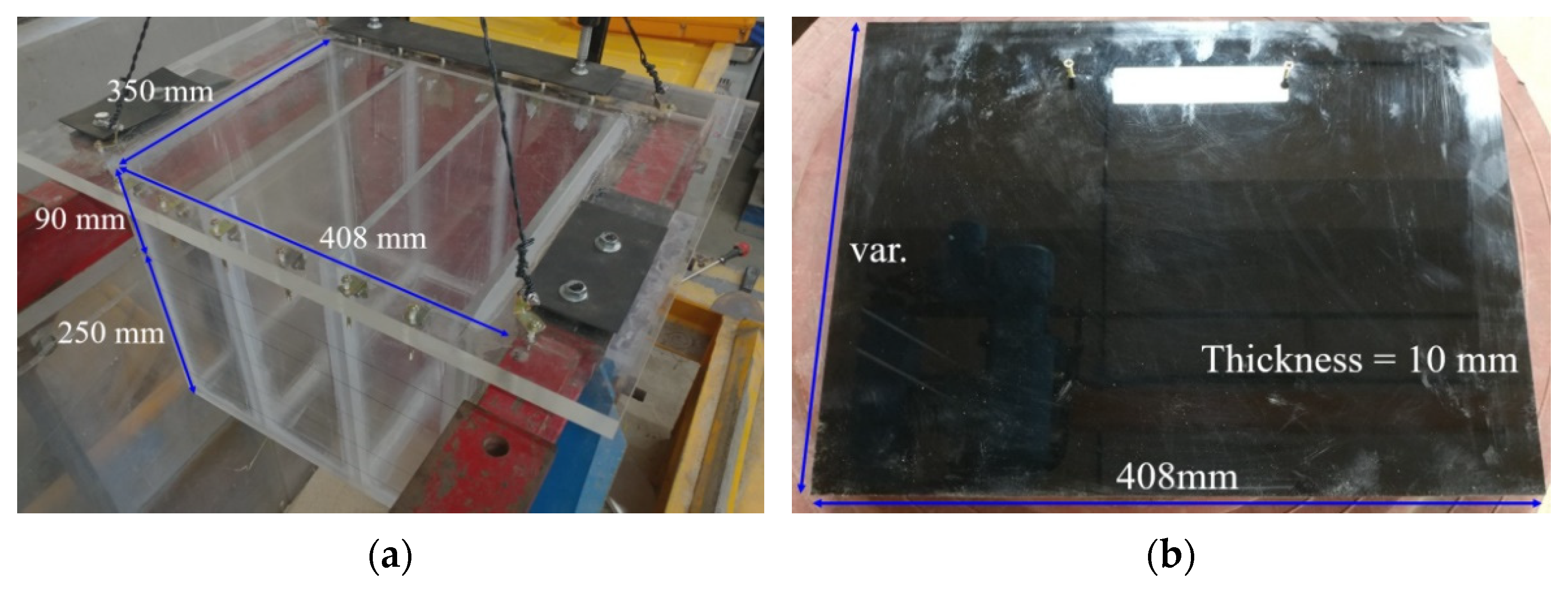
2.2.2. Plane Strain Soil Tank
2.2.3. Experimental Model
2.2.4. Ground Material
2.3. Experimental Method
3. Results and Analysis
3.1. Settlement
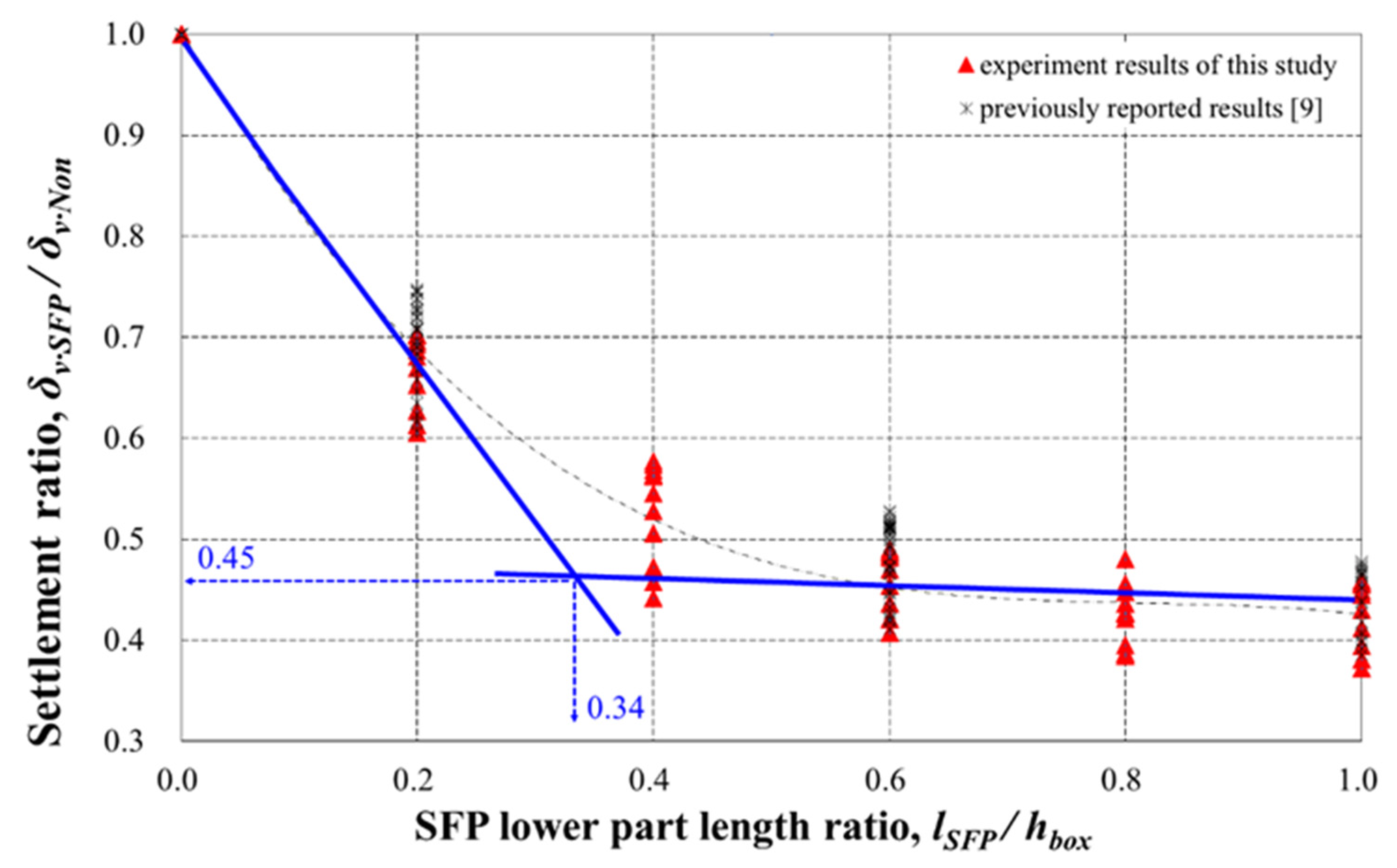
3.2. Optimal Length Ratio
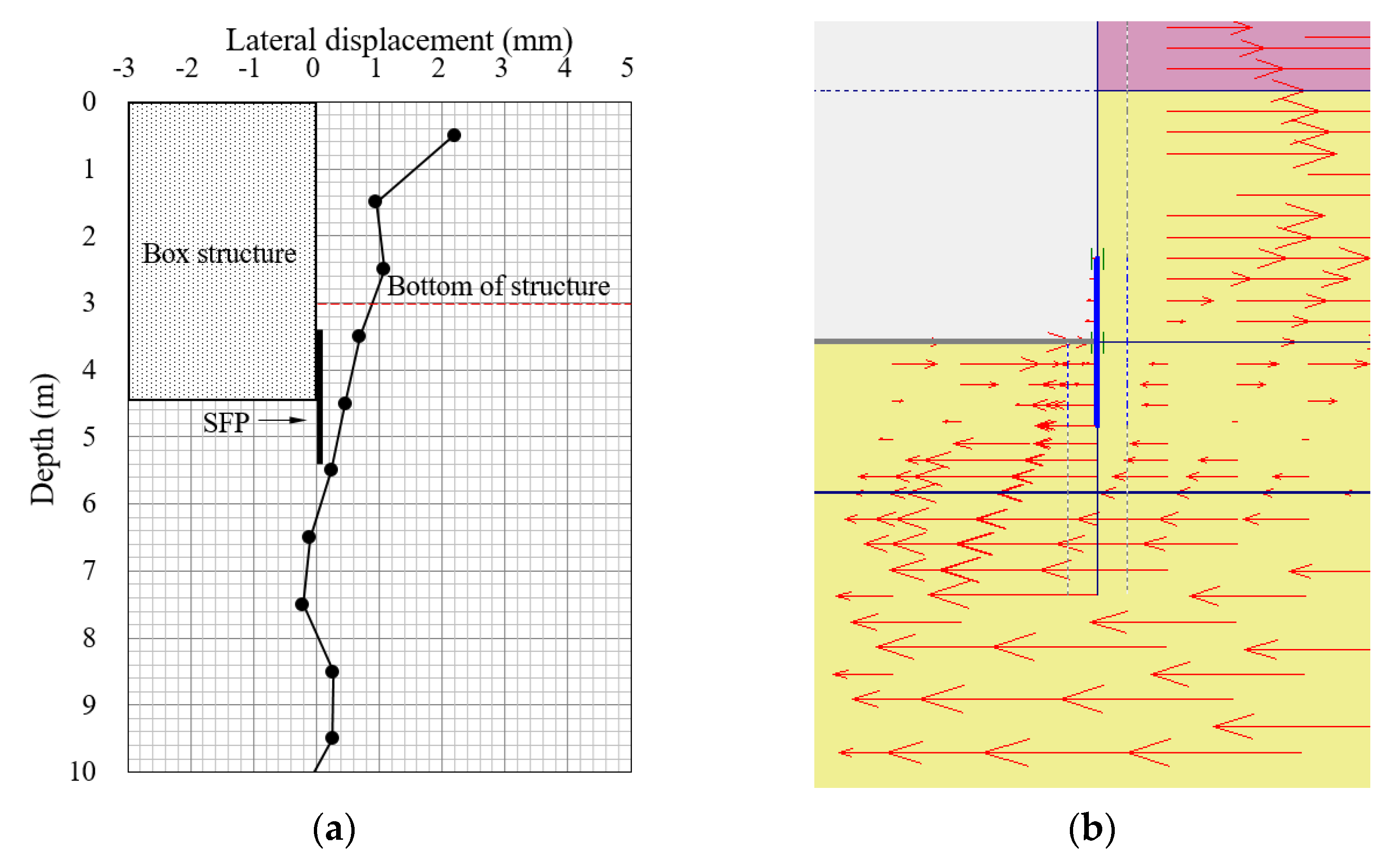
3.3. Stability
3.4. Design Method
4. Conclusions
- We summarized the surface settlement due to the development of a cavity below the box structure as the average settlement ratio when the SFP is installed. We compared the situations with and without SFP installation, and found that the installation of the SFP could effectively reduce the surface settlement ratio in the range of 0.44–0.72. A visual analysis of the structure without the SFP showed that the soil flowed into the cavity, which caused a rapid settlement of the adjoining ground of the box structure. In comparison, by installing the SFP, the surface settlement was reduced because the inflow of soil into the cavity underneath the box structure could be blocked.
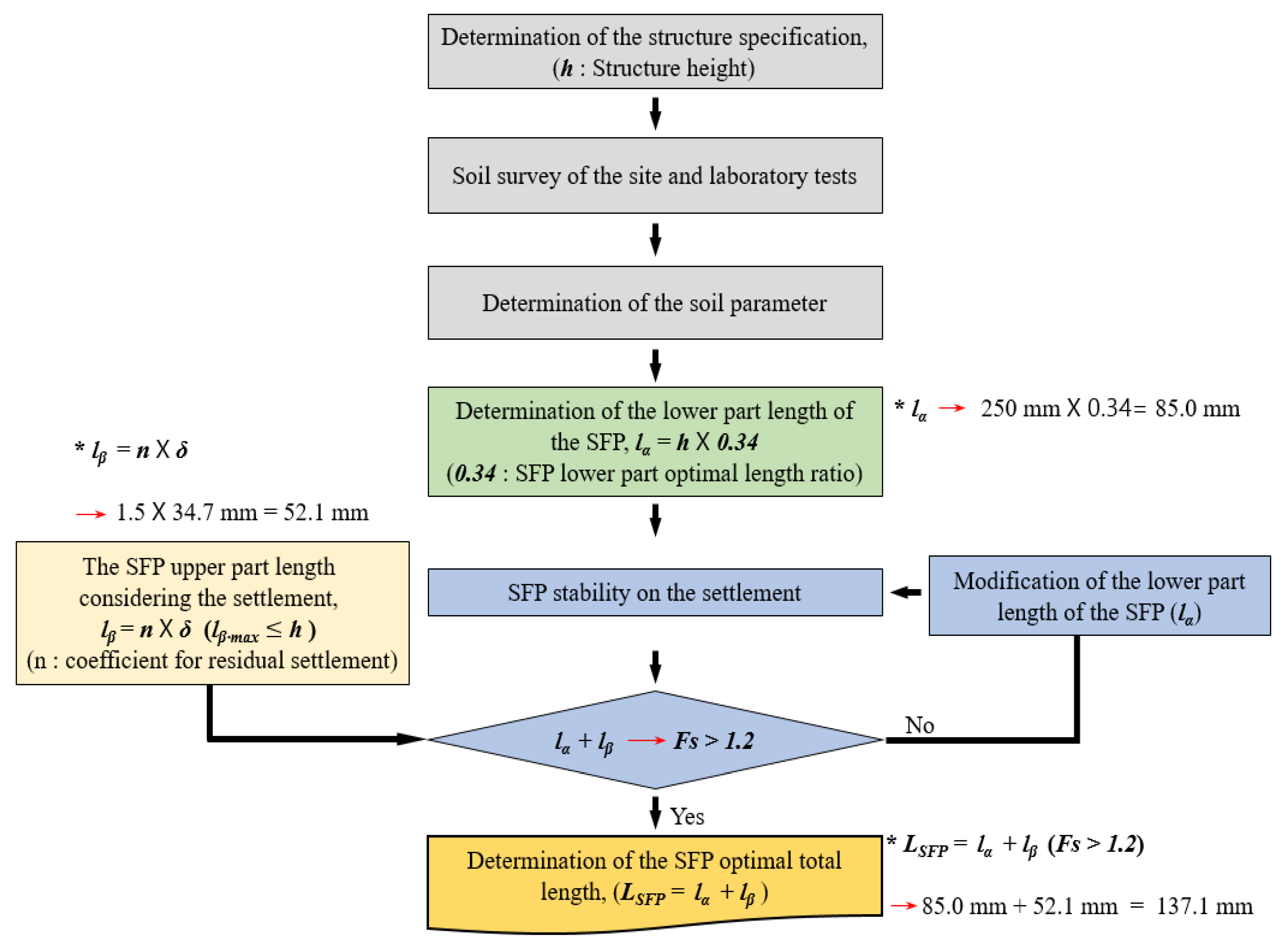
- The settlement decreased with an increase in the lower length of the SFP, and the trend gradually converged above a certain length. The optimum length ratio of the SFP was 0.34, and the surface settlement ratio was 0.45.
- The overall average settlement ratios were 0.71, 0.47, and 0.44, corresponding to SFP lower lengths of 50, 100, and 150 mm, respectively; the settlement decreased with an increase in the lower length. However, the effect of the settlement reduction due to the increase in the upper length was minimal when compared with that due to an increase in the lower length. Thus, we confirmed that surface settlement was dominantly affected by the change in the SFP lower length.
- We calculated the safety factor (Fs) by considering the active earth pressure at the lower front surface of the SFP, the passive earth pressure on the rear surface, and the earth pressure generated at the upper front surface of the SFP, which we considered to be zero. When the optimum length ratio was 0.34 and the ground settlement ratio was 0.45, the safety factor was 1.69; even after ground settlement of 82.3 mm, the SFP remained stable with a safety factor of 1.2 or higher.
- Our comprehensive analysis of the results showed that the lower length of the SFP is the key factor causing the reduction in the surface settlement, whereas the upper length influences the SFP stability with regard to the ground settlement. Based on these results, we proposed an equation for calculating an efficient and economical optimal length (LSFP), while considering the function and role of each SFP. This calculated length has a significant settlement reduction effect, and it satisfies the safety factor (Fs) of 1.2. Based on this design method, we found the sum of the 85.0 mm lower length (lα) (OLR of 0.34 and a ground settlement ratio of 0.45) and the 52.1 mm upper length (lβ), while using a margin factor of 1.5, to be 137.1 mm.
- In this study, we proved the settlement reduction effect of the SFP based on the test results; we calculated the OLR and proposed a design method. Apart from the settlement analyses, further studies are required to establish a more reasonable and comprehensive design method; these studies must focus on a variety of conditions, such as the earth pressure and underground conditions, which are used to identify different properties, such as the soil viscosity, for various construction site applications. We expect the findings of this study to be beneficial for urban construction. The proposed methodology can be applied to analyze the settlement under various SFP lengths and to determine the optimal length to ensure the robustness and safety of structures. However, the scaled model experiment performed during this study only analyzed the results obtained via settlements arising from vibrations in loose sandy soil. Therefore, further studies will be required on the effects of ground surface settlement reduction using SFP installations under conditions where actual ground surface settlement occurs, such as settlement due to the consolidation of clayey ground or settlement due to overburdening.
Author Contributions
Funding
Conflicts of Interest
References
- Hearn, G. Synthesis on Faulted Pavements at Bridge Abutments; Report No. CDOT-DTD-97-11; Colorado Department of Transportation: Denver, CO, USA, 1997. [Google Scholar]
- White, D.J.; Mekkawy, M.M.; Sritharan, S.; Suleiman, M.T. “Underlying” causes for settlement of bridge approach pavement systems. J. Perform. Constr. Fac. 2007, 21, 273–282. [Google Scholar] [CrossRef]
- Briaud, J.; James, R.W.; Hoffman, S.B. Settlement of Bridge Approaches (The Bump at the End of the Bridge). In NCHRP Synthesis of Highway Practice 234; Transportation Research Board; National Academy Press: Washington, DC, USA, 1997. [Google Scholar]
- Sato, M.; Kuwano, R. Effects of buried structures on the formation of underground cavity. In Proceedings of the 18th International Conference on Soil Mechanics and Geotechnical Engineering, Paris, France, 2–6 September 2013; pp. 1769–1772. [Google Scholar]
- Schaefer, V.R.; Koch, J.C. Void Development under Bridge Approaches; Final Report; The National Academies of Sciences, Engineering, and Medicine: Washington, DC, USA, 1992. [Google Scholar]
- Wahls, H.E. Design and Construction of Bridge Approaches; Transportation Research Board; National Research Council: Washington, DC, USA, 1990; Volume 159. [Google Scholar]
- Choi, B.-H.; Lee, K.; Lee, J.; Kim, D. Restoration efficiency analysis of expansive material implemented trenchless underground cavity restoration method varying number and location of bore holes. J. Korean Geosynth. Soc. 2019, 18, 25–37. [Google Scholar] [CrossRef]
- Suchowerska, A.M.; Merifield, R.S.; Carter, J.P.; Clausen, J. Prediction of underground cavity roof collapse using the Hoek–Brown failure criterion. Comput. Geotech. 2012, 44, 93–103. [Google Scholar] [CrossRef]
- Yoo, J.-W.; Seo, M.-S.; Son, S.-W.; Im, J.-C. An experimental study on the estimation of optimum length of soil flow protector with wall stiffness. J. Korean Soc. Civ. Eng. 2019, 39, 789–799. [Google Scholar] [CrossRef]
- Son, S.W. Evaluation of Seismic Performance of Geotechnical Seismic Foundation Isolation System. Ph.D. Thesis, Pusan National University, Busan, Korea, 2018. [Google Scholar]
- Kagawa, T. On the similitude in model vibration tests of earth-structures. Proc. Jpn. Soc. Civ. Eng. 1978, 1978, 69–77. [Google Scholar] [CrossRef]
- Kokusho, T.; Iwatate, T. Scaled model tests and numerical analysis on nonlinear dynamic response of soft grounds. Proc. Jpn. Soc. Civ. Eng. 1979, 1979, 57–67. [Google Scholar] [CrossRef] [Green Version]
- Iai, S. Similitude for shaking table tests on soil-structure-fluid model in 1G gravitational field. Soils Found. 1989, 29, 105–118. [Google Scholar] [CrossRef] [Green Version]
- Iai, S.; Sugano, T. Soil-structure interaction studies through shaking table tests. In Proceedings of the 2nd International Conference on Earthquake Geotechnical Engineering, Lisbon, Portugal, 21–25 June 1999. [Google Scholar]
- Choi, C.Y.; Kim, H.K.; Park, J.H. Comparison of performance with backfill inclination slope and shape in railway abutment and transitional zone using centrifuge model tester. J. Korean Geosynth. Soc. 2018, 17, 85–93. [Google Scholar]
- Tatsuoka, F.; Tateyama, M.; Koseki, J.; Yonezawa, T. Geosynthetic-reinforced soil structures for railways in Japan. Transp. Infrastruct. Geotechnol. 2014, 1, 3–53. [Google Scholar] [CrossRef] [Green Version]
- Kim, Y.-S.; Ko, H.-W.; Kim, J.-H.; Lee, J.-G. Dynamic deformation characteristics of Joomunjin standard sand using cyclic triaxial test. J. Korean Geotech. Soc. 2012, 28, 53–64. [Google Scholar] [CrossRef]
- Song, Y.-S. Estimation on unsaturated hydraulic conductivity function of Jumoonjin sand for various relative densities. J. Korean Soc. Civ. Eng. 2013, 33, 2369–2379. [Google Scholar] [CrossRef] [Green Version]
- Suh, H.; Yun, T.; Kim, K. Prediction of soil-water characteristic curve and relative permeability of Jumunjin sand using pore network model. J. Korean Geotech. Soc. 2016, 32, 55–62. [Google Scholar] [CrossRef]
- Bautista, F.E.; Park, L.K.; Im, J.C.; Lee, Y.N. Variation of earth pressure acting on cut-and-cover tunnel lining with settlement of backfill. J. Korean Geotech. Soc. 2006, 22, 27–40. [Google Scholar]
- Kim, C.; Kwon, J.; Im, J.C.; Hwang, S. A method for analyzing the self-supported earth-retaining structure using stabilizing piles. Mar. Georesour. Geotec. 2012, 30, 313–332. [Google Scholar] [CrossRef]
- Ko, H.S.; Im, J.C.; Park, L.K.; Oh, M.L. Model tests on the behaviour of sandy ground during tunneling. J. Korean Soc. Civ. Eng. 1996, 1996, 371–374. [Google Scholar]
- Son, J.H.; Im, J.C.; Park, L.K.; Lee, T.H. A study on the group effect of micropiles installed beneath shallow foundations. J. Korean Soc. Civ. Eng. 2003, 2003, 3354–3357. [Google Scholar]
- Vaid, Y.P.; Negussey, D. Preparation of reconstituted sand specimens. ASTM Int. 1988, 977, 405–417. [Google Scholar] [CrossRef]
- Miura, S.; Toki, S. A sample preparation method and its effect on static and cyclic deformation-strength properties of sand. Soils Found. 1982, 22, 61–77. [Google Scholar] [CrossRef] [Green Version]
- Yoo, J.; Son, S.; Kim, S. Development and performance analysis of soil flow protector to reduce soft soil settlement caused by cavity formation. Sustainability 2020, 12, 3641. [Google Scholar] [CrossRef]
- Ministry of Land, Infrastructure and Transport. Temporary Earth Retaining Wall Design Standard (KDS 21 30 00:2020). 2020. Available online: https://www.kcsc.re.kr/Search/List (accessed on 9 August 2021). (In Korean).









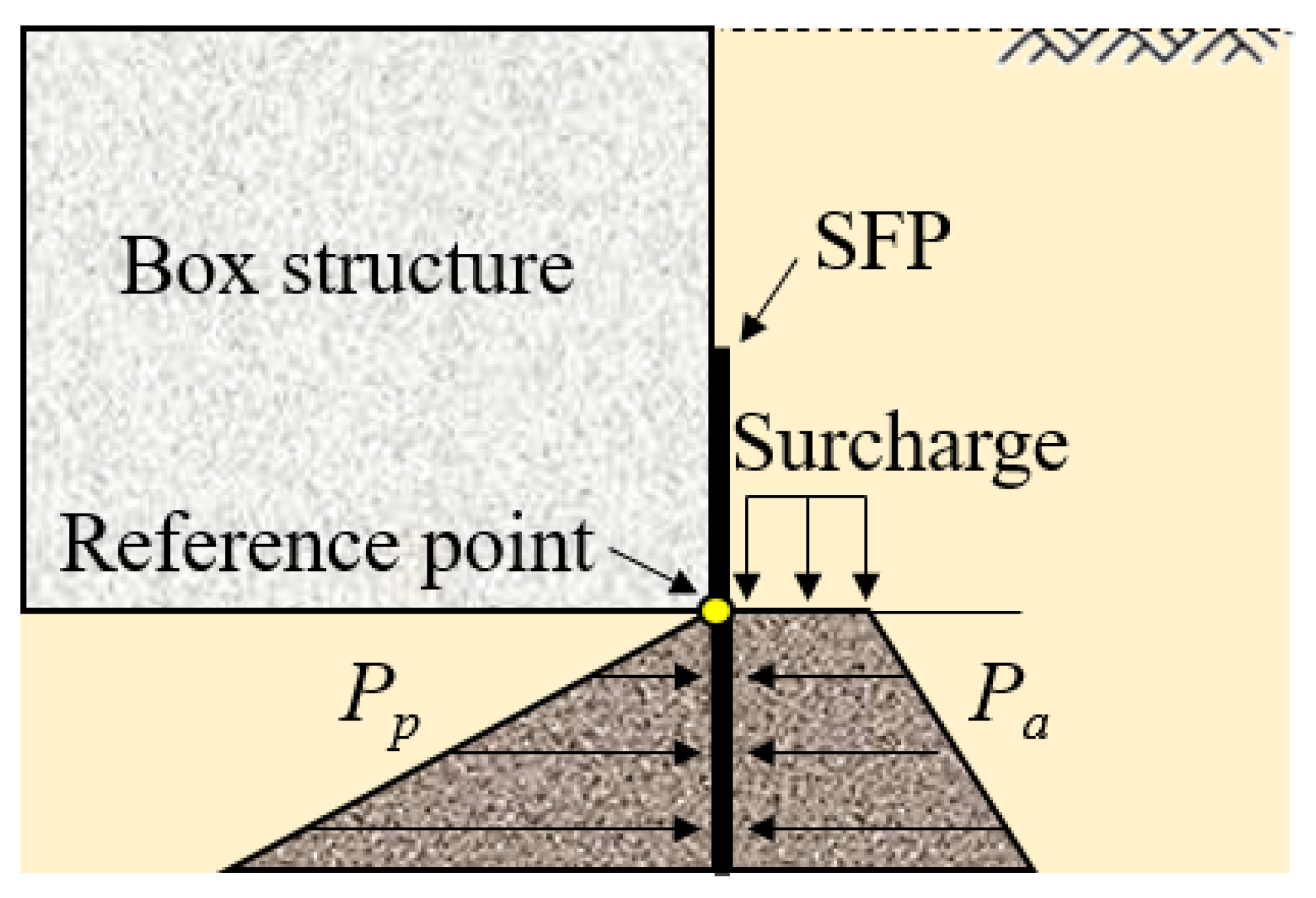
| Case | Length of Upper Part of SFP (mm) | Length of Lower Part of SFP (mm) | Total Length of SFP (mm) |
|---|---|---|---|
| Non_SFP | - | - | - |
| U50_L50 | 50 | 50 | 100 |
| U100_L50 | 100 | 50 | 150 |
| U150_L50 | 150 | 50 | 200 |
| U50_L150 | 50 | 150 | 200 |
| U100_L150 | 100 | 150 | 250 |
| U150_L150 | 150 | 150 | 300 |
| U200_L150 | 200 | 150 | 350 |
| U50_L250 | 50 | 250 | 300 |
| U100_L250 | 100 | 250 | 350 |
| Contents | Iai’s Similitude Ratio Type 3 |
|---|---|
| Length | λ |
| Time | λ 0.5 |
| Acceleration | 1 |
| Displacement | λ |
| Stress | λ |
| Strain | 1 |
| Stiffness | λ |
| Specific gravity, Gs | 2.650 |
| Water content, w (%) | 0.90 |
| Average particle size, D50 (mm) | 0.580 |
| Effective particle size, D10 (mm) | 0.448 |
| Uniformity coefficient, Cu | 1.370 |
| Coefficient of the curvature, Cg | 0.960 |
| Case | Minimum Settlement (mm) | Average Settlement (mm) | Maximum Settlement (mm) | Standard Deviation | Average Settlement Ratio | |
|---|---|---|---|---|---|---|
| Non_SFP | 63.3 | 77.1 | 89.9 | 6.81 | Non_SFP/Non_SFP | 1.00 |
| U50_L50 | 46.1 | 55.6 | 65.6 | 6.47 | U50_L50/Non_SFP | 0.72 |
| U100_L50 | 42.2 | 54.2 | 63.7 | 7.42 | U100_L50/Non_SFP | 0.70 |
| U150_L50 | 42.6 | 53.4 | 64.2 | 7.35 | U150_L50/Non_SFP | 0.69 |
| U50_L150 | 29.6 | 36.7 | 43.8 | 4.80 | U50_L150/Non_SFP | 0.48 |
| U100_L150 | 27.9 | 36.8 | 43.7 | 5.59 | U100_L150/Non_SFP | 0.48 |
| U150_L150 | 27.2 | 35.7 | 43.9 | 5.76 | U150_L150/Non_SFP | 0.46 |
| U200_L150 | 27.8 | 36.2 | 43.7 | 5.46 | U200_L150/Non_SFP | 0.47 |
| U50_L250 | 26.6 | 33.7 | 41.2 | 4.97 | U50_L250/Non_SFP | 0.44 |
| U100_L250 | 26.3 | 33.9 | 39.8 | 4.95 | U100_L250/Non_SFP | 0.44 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Son, S.; Choi, M.; Yoo, J. Stability Analysis of Soil Flow Protector and Design Method for Estimating Optimal Length. Appl. Sci. 2021, 11, 7314. https://doi.org/10.3390/app11167314
Son S, Choi M, Yoo J. Stability Analysis of Soil Flow Protector and Design Method for Estimating Optimal Length. Applied Sciences. 2021; 11(16):7314. https://doi.org/10.3390/app11167314
Chicago/Turabian StyleSon, Suwon, Moonbong Choi, and Jaewon Yoo. 2021. "Stability Analysis of Soil Flow Protector and Design Method for Estimating Optimal Length" Applied Sciences 11, no. 16: 7314. https://doi.org/10.3390/app11167314
APA StyleSon, S., Choi, M., & Yoo, J. (2021). Stability Analysis of Soil Flow Protector and Design Method for Estimating Optimal Length. Applied Sciences, 11(16), 7314. https://doi.org/10.3390/app11167314






