Tuning of Graphene-Based Optical Devices Operating in the Near-Infrared
Abstract
:1. Introduction
2. Graphene Optical Properties
2.1. Graphene Conductivity
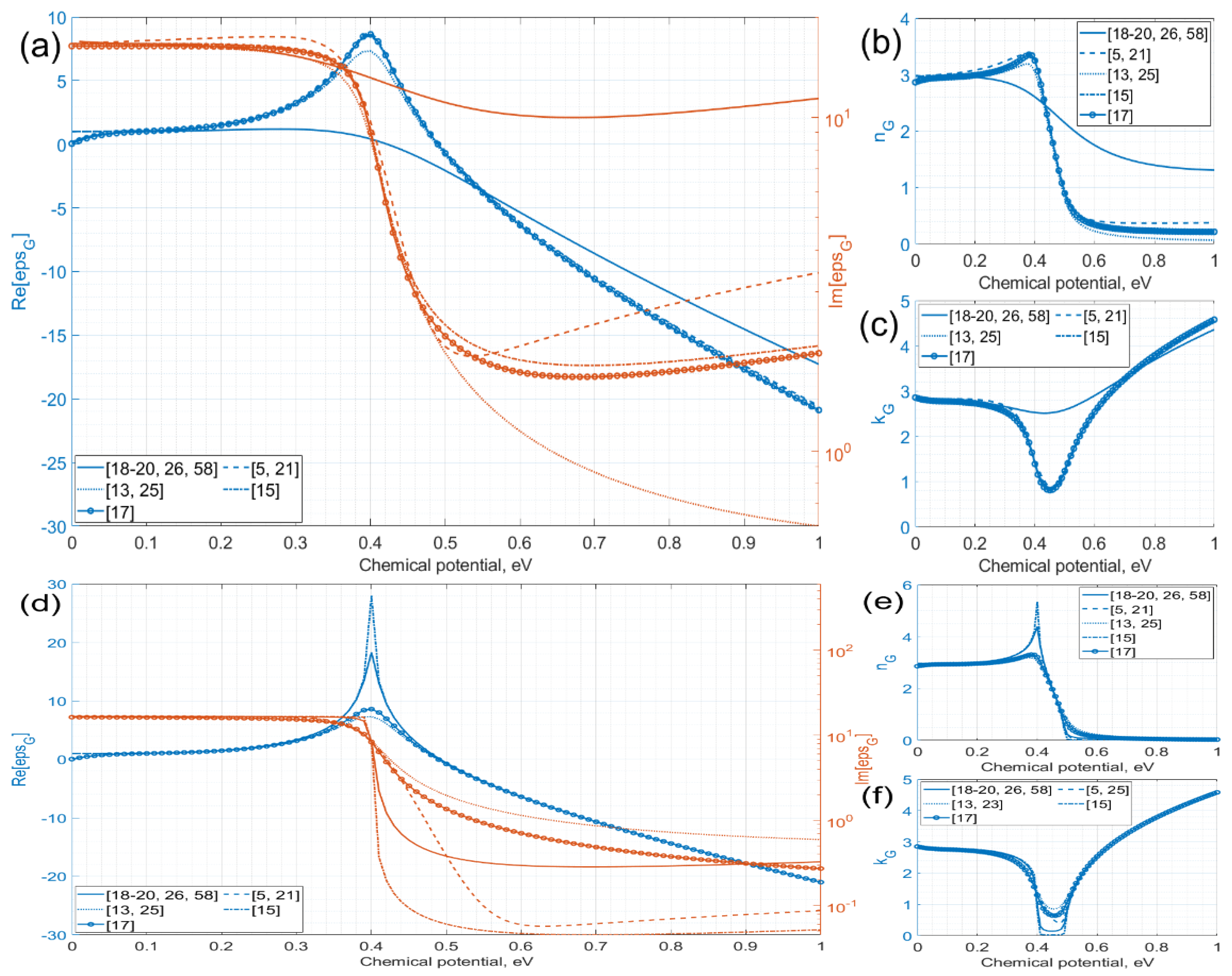
2.1.1. Dependence of Graphene Complex Permittivity
2.1.2. Graphene Conductivity Formula
2.2. Graphene Electrode Configurations
3. Graphene-Based Optical Devices and Tuning
Wavelength Tuning
4. Conclusions and Future Work
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Castro Neto, A.H.; Guinea, F.; Peres, N.M.R.; Novoselov, K.S.; Geim, A.K. The electronic properties of graphene. Rev. Mod. Phys. 2009, 81, 109–162. [Google Scholar] [CrossRef] [Green Version]
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Katsnelson, M.I.; Grigorieva, I.V.; Dubonos, S.V.; Firsov, A.A. Two-dimensional gas of massless Dirac fermions in graphene. Nature 2005, 438, 197–200. [Google Scholar] [CrossRef] [PubMed]
- Rinaldi, G. Nanoscience and Technology: A Collection of Reviews from Nature Journals. Assem. Autom. 2010, 30, 2. [Google Scholar] [CrossRef]
- Wolf, E.L. Applications of Graphene; SpringerBriefs in Materials; Springer International Publishing: Cham, Switzerland, 2014; ISBN 978-3-319-03945-9. [Google Scholar]
- de Ceglia, D.; Vincenti, M.A.; Grande, M.; Bianco, G.V.; Bruno, G.; D’Orazio, A.; Scalora, M. Tuning infrared guided-mode resonances with graphene. J. Opt. Soc. Am. B 2016, 33, 426. [Google Scholar] [CrossRef]
- Wang, F.; Zhang, Y.; Tian, C.; Girit, C.; Zettl, A.; Crommie, M.; Shen, Y.R. Gate-Variable Optical Transitions in Graphene. Science 2008, 320, 206. [Google Scholar] [CrossRef]
- Abdollahi Shiramin, L.; Xie, W.; Snyder, B.; De Heyn, P.; Verheyen, P.; Roelkens, G.; Van Thourhout, D. High Extinction Ratio Hybrid Graphene-Silicon Photonic Crystal Switch. IEEE Photonics Technol. Lett. 2018, 30, 157–160. [Google Scholar] [CrossRef]
- Mohsin, M.; Neumaier, D.; Schall, D.; Otto, M.; Matheisen, C.; Lena Giesecke, A.; Sagade, A.A.; Kurz, H. Experimental verification of electro-refractive phase modulation in graphene. Sci. Rep. 2015, 5, 10967. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Shu, H.; Su, Z.; Huang, L.; Wu, Z.; Wang, X.; Zhang, Z.; Zhou, Z. Significantly High Modulation Efficiency of Compact Graphene Modulator Based on Silicon Waveguide. Sci. Rep. 2018, 8, 991. [Google Scholar] [CrossRef]
- Cheng, Z.; Zhu, X.; Galili, M.; Frandsen, L.H.; Hu, H.; Xiao, S.; Dong, J.; Ding, Y.; Oxenløwe, L.K.; Zhang, X. Double-layer graphene on photonic crystal waveguide electro-absorption modulator with 12 GHz bandwidth. Nanophotonics 2019, 9, 2377–2385. [Google Scholar] [CrossRef]
- Liu, M.; Yin, X.; Ulin-Avila, E.; Geng, B.; Zentgraf, T.; Ju, L.; Wang, F.; Zhang, X. A graphene-based broadband optical modulator. Nature 2011, 474, 64–67. [Google Scholar] [CrossRef]
- Liu, M.; Yin, X.; Zhang, X. Double-Layer Graphene Optical Modulator. Nano Lett. 2012, 12, 1482–1485. [Google Scholar] [CrossRef] [PubMed]
- Phatak, A.; Cheng, Z.; Qin, C.; Goda, K. Design of electro-optic modulators based on graphene-on-silicon slot waveguides. Opt. Lett. 2016, 41, 2501. [Google Scholar] [CrossRef]
- Hu, Y.; Pantouvaki, M.; Van Campenhout, J.; Brems, S.; Asselberghs, I.; Huyghebaert, C.; Absil, P.; Van Thourhout, D. Broadband 10 Gb/s operation of graphene electro-absorption modulator on silicon: Broadband 10 Gb/s operation of graphene electro-absorption modulator on silicon. Laser Photonics Rev. 2016, 10, 307–316. [Google Scholar] [CrossRef] [Green Version]
- Bahadori-Haghighi, S.; Ghayour, R.; Sheikhi, M.H. Double-layer graphene optical modulators based on Fano resonance in all-dielectric metasurfaces. J. Appl. Phys. 2019, 125, 073104. [Google Scholar] [CrossRef]
- Koester, S.J.; Li, M. High-speed waveguide-coupled graphene-on-graphene optical modulators. Appl. Phys. Lett. 2012, 100, 171107. [Google Scholar] [CrossRef]
- Luo, X.; Zhai, X.; Li, H.; Liu, J.; Wang, L. Tunable Nonreciprocal Graphene Waveguide With Kerr Nonlinear Material. IEEE Photonics Technol. Lett. 2017, 29, 1903–1906. [Google Scholar] [CrossRef]
- Xu, C.; Jin, Y.; Yang, L.; Yang, J.; Jiang, X. Characteristics of electro-refractive modulating based on Graphene-Oxide-Silicon waveguide. Opt. Express 2012, 20, 22398. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Majumdar, A.; Kim, J.; Vuckovic, J.; Wang, F. Electrical Control of Silicon Photonic Crystal Cavity by Graphene. Nano Lett. 2013, 13, 515–518. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gan, X.; Shiue, R.-J.; Gao, Y.; Mak, K.F.; Yao, X.; Li, L.; Szep, A.; Walker, D.; Hone, J.; Heinz, T.F.; et al. High-Contrast Electrooptic Modulation of a Photonic Crystal Nanocavity by Electrical Gating of Graphene. Nano Lett. 2013, 13, 691–696. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sorianello, V.; Midrio, M.; Romagnoli, M. Design optimization of single and double layer Graphene phase modulators in SOI. Opt. Express 2015, 23, 6478. [Google Scholar] [CrossRef]
- Phare, C.T.; Lee, Y.-H.D.; Cardenas, J.; Lipson, M. 30 GHz Zeno-based Graphene Electro-optic Modulator. In Proceedings of the CLEO: 2015, San Jose, CA, USA, 5–10 May 2015; OSA: San Jose, CA, USA, 2015; p. SW4I.4. [Google Scholar]
- Cai, M.; Wang, S.; Liu, Z.; Wang, Y.; Han, T.; Liu, H. Graphene Electro-Optical Switch Modulator by Adjusting Propagation Length Based on Hybrid Plasmonic Waveguide in Infrared Band. Sensors 2020, 20, 2864. [Google Scholar] [CrossRef]
- Ding, Y.; Zhu, X.; Xiao, S.; Hu, H.; Frandsen, L.H.; Mortensen, N.A.; Yvind, K. Effective Electro-Optical Modulation with High Extinction Ratio by a Graphene–Silicon Microring Resonator. Nano Lett. 2015, 15, 4393–4400. [Google Scholar] [CrossRef] [Green Version]
- Qiu, C.; Gao, W.; Vajtai, R.; Ajayan, P.M.; Kono, J.; Xu, Q. Efficient Modulation of 1.55 μm Radiation with Gated Graphene on a Silicon Microring Resonator. Nano Lett. 2014, 14, 6811–6815. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yang, L.; Hu, T.; Shen, A.; Pei, C.; Li, Y.; Dai, T.; Yu, H.; Li, Y.; Jiang, X.; Yang, J. Proposal for a 2X2 Optical Switch Based on Graphene-Silicon-Waveguide Microring. IEEE Photonics Technol. Lett. 2014, 26, 235–238. [Google Scholar] [CrossRef]
- Grande, M.; Bianco, G.V.; Laneve, D.; Capezzuto, P.; Petruzzelli, V.; Scalora, M.; Prudenzano, F.; Bruno, G.; D’Orazio, A. Gain and phase control in a graphene-loaded reconfigurable antenna. Appl. Phys. Lett. 2019, 115, 133103. [Google Scholar] [CrossRef]
- Grande, M.; Bianco, G.V.; Perna, F.M.; Capriati, V.; Capezzuto, P.; Scalora, M.; Bruno, G.; D’Orazio, A. Reconfigurable and optically transparent microwave absorbers based on deep eutectic solvent-gated graphene. Sci. Rep. 2019, 9, 5463. [Google Scholar] [CrossRef]
- Grande, M.; Bianco, G.V.; Capezzuto, P.; Petruzzelli, V.; Prudenzano, F.; Scalora, M.; Bruno, G.; D’Orazio, A. Amplitude and phase modulation in microwave ring resonators by doped CVD graphene. Nanotechnology 2018, 29, 325201. [Google Scholar] [CrossRef] [PubMed]
- Sensale-Rodriguez, B.; Yan, R.; Kelly, M.M.; Fang, T.; Tahy, K.; Hwang, W.S.; Jena, D.; Liu, L.; Xing, H.G. Broadband graphene terahertz modulators enabled by intraband transitions. Nat. Commun. 2012, 3, 780. [Google Scholar] [CrossRef]
- Sensale-Rodriguez, B.; Yan, R.; Rafique, S.; Zhu, M.; Li, W.; Liang, X.; Gundlach, D.; Protasenko, V.; Kelly, M.M.; Jena, D.; et al. Extraordinary Control of Terahertz Beam Reflectance in Graphene Electro-absorption Modulators. Nano Lett. 2012, 12, 4518–4522. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lee, C.-C.; Suzuki, S.; Xie, W.; Schibli, T.R. Broadband graphene electro-optic modulators with sub-wavelength thickness. Opt. Express 2012, 20, 5264. [Google Scholar] [CrossRef] [PubMed]
- Ono, M.; Hata, M.; Tsunekawa, M.; Nozaki, K.; Sumikura, H.; Chiba, H.; Notomi, M. Ultrafast and energy-efficient all-optical switching with graphene-loaded deep-subwavelength plasmonic waveguides. Nat. Photonics 2020, 14, 37–43. [Google Scholar] [CrossRef] [Green Version]
- Garmire, E. Nonlinear optics in daily life. Opt. Express 2013, 21, 30532. [Google Scholar] [CrossRef] [PubMed]
- Hafez, H.A.; Kovalev, S.; Tielrooij, K.; Bonn, M.; Gensch, M.; Turchinovich, D. Terahertz Nonlinear Optics of Graphene: From Saturable Absorption to High-Harmonics Generation. Adv. Opt. Mater. 2020, 8, 1900771. [Google Scholar] [CrossRef] [Green Version]
- Bowlan, P.; Martinez-Moreno, E.; Reimann, K.; Elsaesser, T.; Woerner, M. Ultrafast terahertz response of multilayer graphene in the nonperturbative regime. Phys. Rev. B 2014, 89, 041408. [Google Scholar] [CrossRef]
- Hafez, H.A.; Kovalev, S.; Deinert, J.-C.; Mics, Z.; Green, B.; Awari, N.; Chen, M.; Germanskiy, S.; Lehnert, U.; Teichert, J.; et al. Extremely efficient terahertz high-harmonic generation in graphene by hot Dirac fermions. Nature 2018, 561, 507–511. [Google Scholar] [CrossRef]
- Deinert, J.-C.; Alcaraz Iranzo, D.; Pérez, R.; Jia, X.; Hafez, H.A.; Ilyakov, I.; Awari, N.; Chen, M.; Bawatna, M.; Ponomaryov, A.N.; et al. Grating-Graphene Metamaterial as a Platform for Terahertz Nonlinear Photonics. ACS Nano 2021, 15, 1145–1154. [Google Scholar] [CrossRef]
- Hasan, T.; Sun, Z.; Wang, F.; Bonaccorso, F.; Tan, P.H.; Rozhin, A.G.; Ferrari, A.C. Nanotube–Polymer Composites for Ultrafast Photonics. Adv. Mater. 2009, 21, 3874–3899. [Google Scholar] [CrossRef]
- Sobon, G.; Sotor, J.; Pasternak, I.; Krzempek, K.; Strupinski, W.; Abramski, K.M. A tunable, linearly polarized Er-fiber laser mode-locked by graphene/PMMA composite. Laser Phys. 2013, 23, 125101. [Google Scholar] [CrossRef]
- Jung, M.; Koo, J.; Park, J.; Song, Y.-W.; Jhon, Y.M.; Lee, K.; Lee, S.; Lee, J.H. Mode-locked pulse generation from an all-fiberized, Tm-Ho-codoped fiber laser incorporating a graphene oxide-deposited side-polished fiber. Opt. Express 2013, 21, 20062. [Google Scholar] [CrossRef] [PubMed]
- Lin, Y.-H.; Yang, C.-Y.; Liou, J.-H.; Yu, C.-P.; Lin, G.-R. Using graphene nano-particle embedded in photonic crystal fiber for evanescent wave mode-locking of fiber laser. Opt. Express 2013, 21, 16763. [Google Scholar] [CrossRef]
- Choi, S.Y.; Jeong, H.; Hong, B.H.; Rotermund, F.; Yeom, D.-I. All-fiber dissipative soliton laser with 10.2 nJ pulse energy using an evanescent field interaction with graphene saturable absorber. Laser Phys. Lett. 2014, 11, 015101. [Google Scholar] [CrossRef]
- Morell, N.; Reserbat-Plantey, A.; Tsioutsios, I.; Schädler, K.G.; Dubin, F.; Koppens, F.H.L.; Bachtold, A. High Quality Factor Mechanical Resonators Based on WSe2 Monolayers. Nano Lett. 2016, 16, 5102–5108. [Google Scholar] [CrossRef] [Green Version]
- Chhowalla, M.; Shin, H.S.; Eda, G.; Li, L.-J.; Loh, K.P.; Zhang, H. The chemistry of two-dimensional layered transition metal dichalcogenide nanosheets. Nat. Chem. 2013, 5, 263–275. [Google Scholar] [CrossRef]
- Low, T.; Chaves, A.; Caldwell, J.D.; Kumar, A.; Fang, N.X.; Avouris, P.; Heinz, T.F.; Guinea, F.; Martin-Moreno, L.; Koppens, F. Polaritons in layered two-dimensional materials. Nat. Mater. 2017, 16, 182–194. [Google Scholar] [CrossRef] [Green Version]
- Correas-Serrano, D.; Gomez-Diaz, J.S.; Melcon, A.A.; Alù, A. Black phosphorus plasmonics: Anisotropic elliptical propagation and nonlocality-induced canalization. J. Opt. 2016, 18, 104006. [Google Scholar] [CrossRef] [Green Version]
- Xia, F.; Wang, H.; Jia, Y. Rediscovering black phosphorus as an anisotropic layered material for optoelectronics and electronics. Nat. Commun. 2014, 5, 4458. [Google Scholar] [CrossRef] [Green Version]
- Wang, H.; Yu, X. Few-Layered Black Phosphorus: From Fabrication and Customization to Biomedical Applications. Small 2018, 14, 1702830. [Google Scholar] [CrossRef] [PubMed]
- Huang, Y.; Qiao, J.; He, K.; Bliznakov, S.; Sutter, E.; Chen, X.; Luo, D.; Meng, F.; Su, D.; Decker, J.; et al. Interaction of Black Phosphorus with Oxygen and Water. Chem. Mater. 2016, 28, 8330–8339. [Google Scholar] [CrossRef] [Green Version]
- Li, L.; Yu, Y.; Ye, G.J.; Ge, Q.; Ou, X.; Wu, H.; Feng, D.; Chen, X.H.; Zhang, Y. Black phosphorus field-effect transistors. Nat. Nanotechnol. 2014, 9, 372–377. [Google Scholar] [CrossRef] [Green Version]
- Das, S.; Zhang, W.; Demarteau, M.; Hoffmann, A.; Dubey, M.; Roelofs, A. Tunable Transport Gap in Phosphorene. Nano Lett. 2014, 14, 5733–5739, Correction in 2016, 16, 2122–2122. [Google Scholar] [CrossRef] [PubMed]
- Rudenko, A.N.; Yuan, S.; Katsnelson, M.I. Toward a realistic description of multilayer black phosphorus: From G W approximation to large-scale tight-binding simulations. Phys. Rev. B 2015, 92, 085419. [Google Scholar] [CrossRef] [Green Version]
- Kozawa, D.; Kumar, R.; Carvalho, A.; Kumar Amara, K.; Zhao, W.; Wang, S.; Toh, M.; Ribeiro, R.M.; Castro Neto, A.H.; Matsuda, K.; et al. Photocarrier relaxation pathway in two-dimensional semiconducting transition metal dichalcogenides. Nat. Commun. 2014, 5, 4543. [Google Scholar] [CrossRef] [PubMed]
- Low, T.; Rodin, A.S.; Carvalho, A.; Jiang, Y.; Wang, H.; Xia, F.; Castro Neto, A.H. Tunable optical properties of multilayer black phosphorus thin films. Phys. Rev. B 2014, 90, 075434. [Google Scholar] [CrossRef] [Green Version]
- Depine, R.A. Electromagnetics of graphene. In Graphene Optics: Electromagnetic Solution of Canonical Problems; Morgan & Claypool Publishers: San Rafael, CA, USA, 2016; pp. 1–136. ISBN 978-1-68174-309-7. [Google Scholar]
- Hanson, G.W. Dyadic Green’s functions and guided surface waves for a surface conductivity model of graphene. J. Appl. Phys. 2008, 103, 064302. [Google Scholar] [CrossRef] [Green Version]
- Polycarpou, A.C. Introduction to the Finite Element Method in Electromagnetics; Morgan & Claypool: San Rafael, CA, USA, 2006; pp. 1–126. ISBN 978-1-59829-047-9. [Google Scholar]
- Introduction—MEEP Documentation. Available online: https://meep.readthedocs.io/en/latest/Introduction/ (accessed on 19 April 2021).
- Wartak, M.S. Computational Photonics: An Introduction with MATLAB; Cambridge University Press: Cambridge, UK, 2013; ISBN 978-1-107-00552-5. [Google Scholar]
- Tan, Y.-W.; Zhang, Y.; Bolotin, K.; Zhao, Y.; Adam, S.; Hwang, E.H.; Das Sarma, S.; Stormer, H.L.; Kim, P. Measurement of Scattering Rate and Minimum Conductivity in Graphene. Phys. Rev. Lett. 2007, 99, 246803. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bao, Q.; Loh, K.P. Graphene Photonics, Plasmonics, and Broadband Optoelectronic Devices. ACS Nano 2012, 6, 3677–3694. [Google Scholar] [CrossRef] [PubMed]
- Timurdogan, E.; Sorace-Agaskar, C.M.; Sun, J.; Shah Hosseini, E.; Biberman, A.; Watts, M.R. An ultralow power athermal silicon modulator. Nat. Commun. 2014, 5, 4008. [Google Scholar] [CrossRef] [Green Version]
- Dalir, H.; Xia, Y.; Wang, Y.; Zhang, X. Athermal Broadband Graphene Optical Modulator with 35 GHz Speed. ACS Photonics 2016, 3, 1564–1568. [Google Scholar] [CrossRef]
- Chen, X.; Wang, F.; Gu, Q.; Yang, J.; Yu, M.; Kwong, D.; Wong, C.W.; Yang, H.; Zhou, H.; Zhou, S. Multifunctional optoelectronic device based on graphene-coupled silicon photonic crystal cavities. Opt. Express 2021, 29, 11094. [Google Scholar] [CrossRef]
- Abdollahi Shiramin, L.; Van Thourhout, D. Graphene Modulators and Switches Integrated on Silicon and Silicon Nitride Waveguide. IEEE J. Sel. Top. Quantum Electron. 2017, 23, 94–100. [Google Scholar] [CrossRef]
- Sorianello, V.; Midrio, M.; Contestabile, G.; Asselberghs, I.; Van Campenhout, J.; Huyghebaert, C.; Goykhman, I.; Ott, A.K.; Ferrari, A.C.; Romagnoli, M. Graphene–silicon phase modulators with gigahertz bandwidth. Nat. Photonics 2018, 12, 40–44. [Google Scholar] [CrossRef]
- Phare, C.T.; Daniel Lee, Y.-H.; Cardenas, J.; Lipson, M. Graphene electro-optic modulator with 30 GHz bandwidth. Nat. Photonics 2015, 9, 511–514. [Google Scholar] [CrossRef]
- Ferrari, A.C.; Bonaccorso, F.; Fal’ko, V.; Novoselov, K.S.; Roche, S.; Bøggild, P.; Borini, S.; Koppens, F.H.L.; Palermo, V.; Pugno, N.; et al. Science and technology roadmap for graphene, related two-dimensional crystals, and hybrid systems. Nanoscale 2015, 7, 4598–4810. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Youngblood, N.; Li, M. Integration of 2D materials on a silicon photonics platform for optoelectronics applications. Nanophotonics 2016, 6, 1205–1218. [Google Scholar] [CrossRef]
- Kim, K.S.; Zhao, Y.; Jang, H.; Lee, S.Y.; Kim, J.M.; Kim, K.S.; Ahn, J.-H.; Kim, P.; Choi, J.-Y.; Hong, B.H. Large-scale pattern growth of graphene films for stretchable transparent electrodes. Nature 2009, 457, 706–710. [Google Scholar] [CrossRef] [PubMed]
- Li, H.-M.; Xu, K.; Bourdon, B.; Lu, H.; Lin, Y.-C.; Robinson, J.A.; Seabaugh, A.C.; Fullerton-Shirey, S.K. Electric Double Layer Dynamics in Poly(ethylene oxide) LiClO4 on Graphene Transistors. J. Phys. Chem. C 2017, 121, 16996–17004. [Google Scholar] [CrossRef]






| Symbol | Description |
|---|---|
| λ and Δλ | wavelength and its shift |
| σ (), and | surface conductivity (graphene surface conductivity), intraband and interband conductivities |
| ε (), and | complex permittivity (graphene complex permittivity), its real and imaginary parts |
| n () and Δn | refractive index (graphene refractive index) and its change |
| ns | graphene surface carrier density |
| k () | extinction coefficient (graphene extinction coefficient) |
| e | electron charge |
| radian frequency | |
| , reduced Planck constant | |
| and | phenomenological scattering rate and , relaxation time |
| , Fermi-Dirac distribution (–energy) | |
| Boltzmann’s constant | |
| T | temperature |
| chemical potential | |
| μ | charge carrier mobilities |
| Fermi velocity | |
| and | graphene and insulator thicknesses |
| L | graphene length |
| d | spacing between minima or free spectral range |
| distance between graphene electrodes | |
| φ | phase shift |
| and | applied voltage and flat-band voltage corresponding to the charge-neutral Dirac point |
| oxide (insulator) capacitance per unit area | |
| GE | Graphene Electrode |
| GIW | Graphene-Insulator-Waveguide |
| GIG | Graphene-Insulator-Graphene |
| EGWC | Electrolyte/ion-gel-Graphene-Waveguide+PhC Cavity |
| GEin | Graphene-Insulator-Graphene inside waveguide |
| M-ZM | Mach–Zehnder Graphene Modulator |
| CNM | Crystal Nanocavity-based Modulator |
| MRR | Micro-ring Resonator |
| Ref. | tG, nm | TMM | tI, nm | Γ, s−1 | Insulator Material | VG | λ, µm | Device Type | |
|---|---|---|---|---|---|---|---|---|---|
| [7] * | - | EGWC | - | 3.8462e13 | PEO + LiClO4 | −1.2 ÷ 1.2 V | 1.56907 | CNM | 800 |
| [8] * | 0.33 | GIG | 90 | 5e11 ÷ 1e14 | Al2O3 | −40 ÷ 40 V | 1.53–1.57 | M-ZM | 140 |
| [9] * | 0.7 | GIG | 10 | 5e12 ÷ 1e14, 8e13 (Γexp) | Al2O3 | 0 ÷ 3 V 0 ÷ 1 V/1 ÷ 3 V | 1.552 | M-ZM | 173/385 |
| [10] * | - | GIG | 5 | - | Al2O3 | 0 ÷ 15 V | 1.549 | CNM | 66/165 |
| [15] | 0.34 | GIG | 70 | 1e14 | SiO2 | −4.95 ÷ 4.95 V | 1.539–1.559 | WOM | 10 |
| [19] * | 1 | EGWC | 5 | 2.2789e14 | Al2O3 & ion-gel | −2 ÷ 0 V | 1.55 | CNM | 1000 |
| [20] * | 0.34 | EGWC | 10 | 7.5963e13 | HfO2 & PEO + LiClO4 | −7 ÷ 6 V | 1.55 | CNM | 1000 |
| [24] * | 1 | GIW | - | - | Al2O3 | −12.5 ÷ 0 V | 1.548–1.557 | MRR | 2000 |
| [25] * | 0.5 | GIW | 25 | 1e14 | Al2O3 | −6 ÷ 6 V | 1.55 | MRR | 125 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vorobev, A.S.; Bianco, G.V.; Bruno, G.; D’Orazio, A.; O’Faolain, L.; Grande, M. Tuning of Graphene-Based Optical Devices Operating in the Near-Infrared. Appl. Sci. 2021, 11, 8367. https://doi.org/10.3390/app11188367
Vorobev AS, Bianco GV, Bruno G, D’Orazio A, O’Faolain L, Grande M. Tuning of Graphene-Based Optical Devices Operating in the Near-Infrared. Applied Sciences. 2021; 11(18):8367. https://doi.org/10.3390/app11188367
Chicago/Turabian StyleVorobev, Artem S., Giuseppe Valerio Bianco, Giovanni Bruno, Antonella D’Orazio, Liam O’Faolain, and Marco Grande. 2021. "Tuning of Graphene-Based Optical Devices Operating in the Near-Infrared" Applied Sciences 11, no. 18: 8367. https://doi.org/10.3390/app11188367
APA StyleVorobev, A. S., Bianco, G. V., Bruno, G., D’Orazio, A., O’Faolain, L., & Grande, M. (2021). Tuning of Graphene-Based Optical Devices Operating in the Near-Infrared. Applied Sciences, 11(18), 8367. https://doi.org/10.3390/app11188367








