Bearing Capacity of Single Pile-Friction Wheel Composite Foundation on Sand-over-Clay Deposit under V-H-M Combined Loadings
Abstract
:1. Introduction
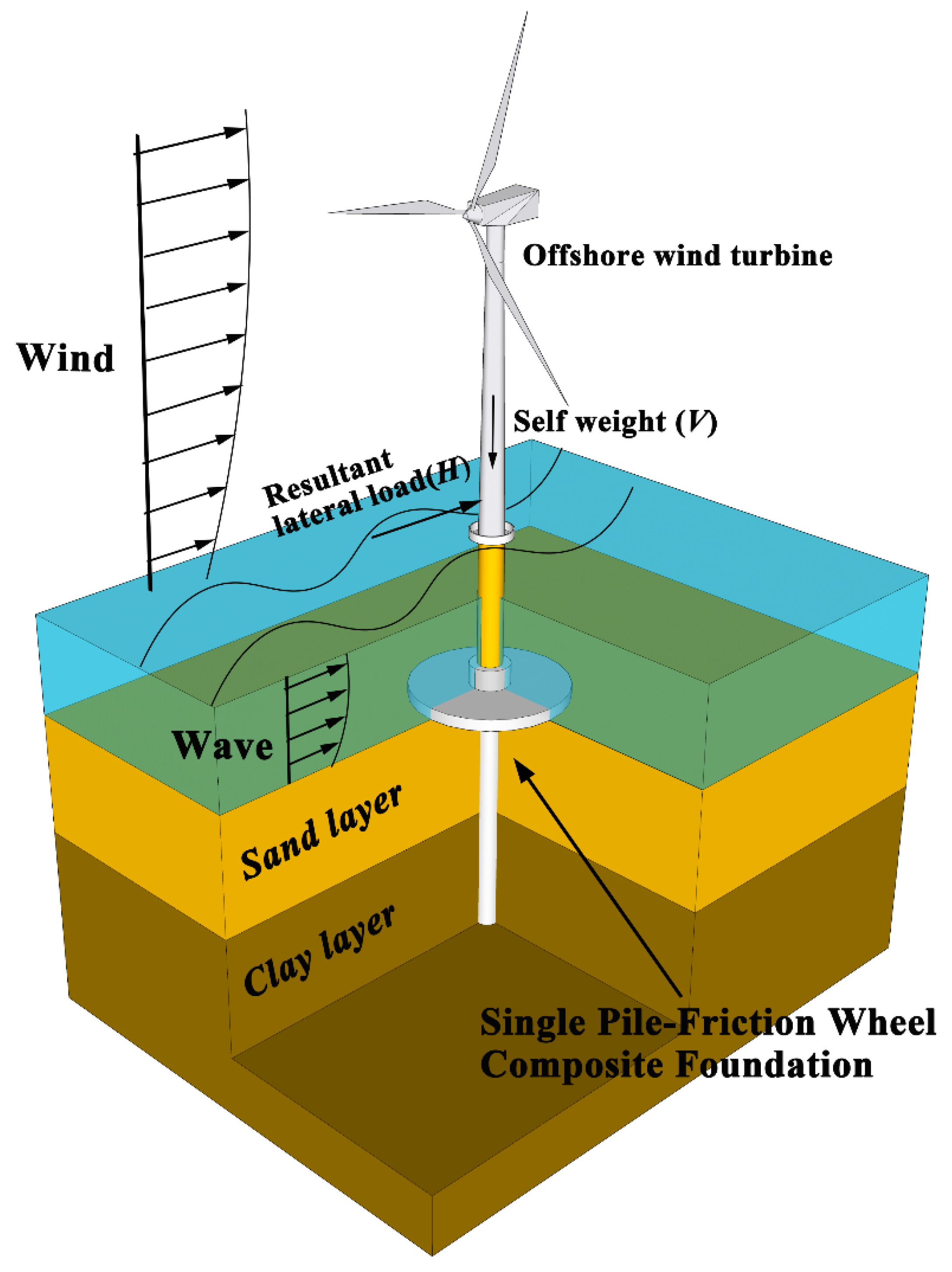
1.1. Concept
1.2. Previous Work
2. Materials and Methods
2.1. Finite Element Model
2.2. Constitutive Model and Soil Properties
2.3. Numerical Model Validation
3. Effect of Different Influencing Factors
3.1. Effects of Wheel Diameter and Pile Embedment Depth (Dw/L and L/Dp)
3.2. Effects of Pre-Vertical Load and Lateral Loading Height (V/Vult and e/Dp)
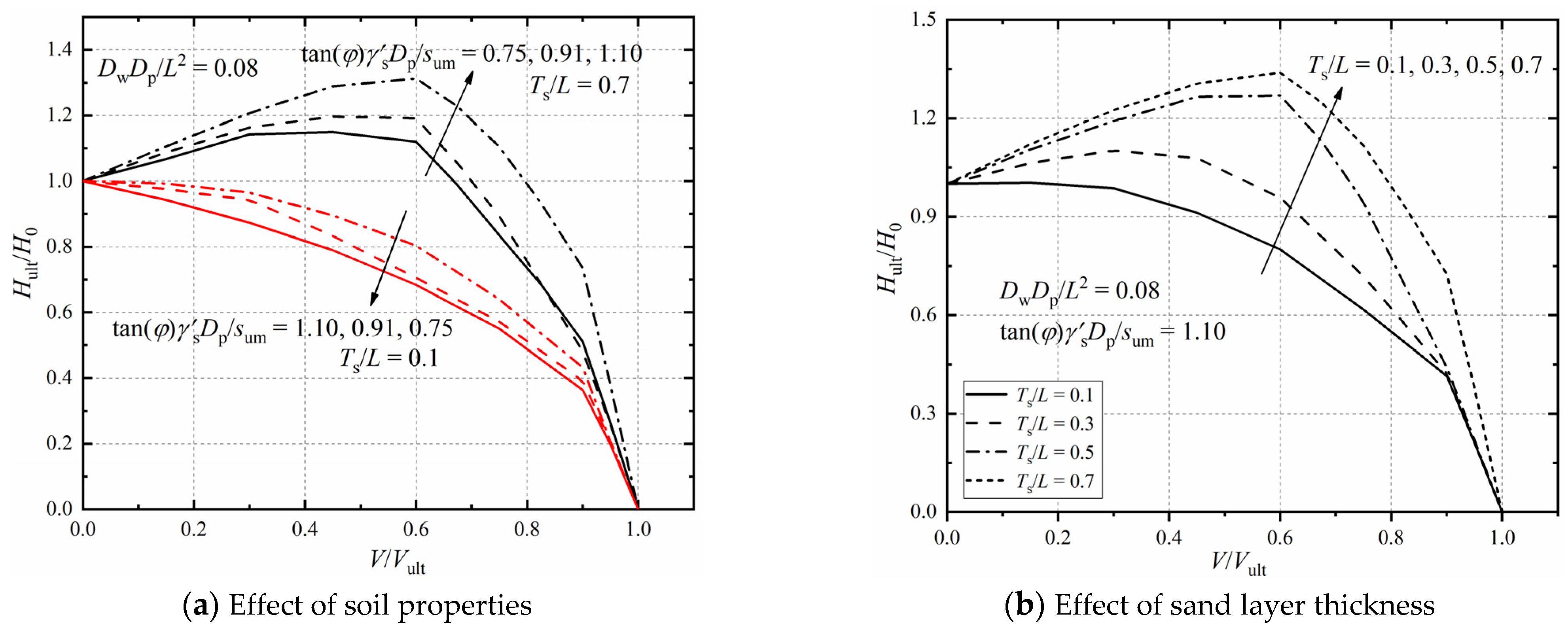
3.3. Effects of Sand Layer Thickness and Soil Properties (sum, k and φ)
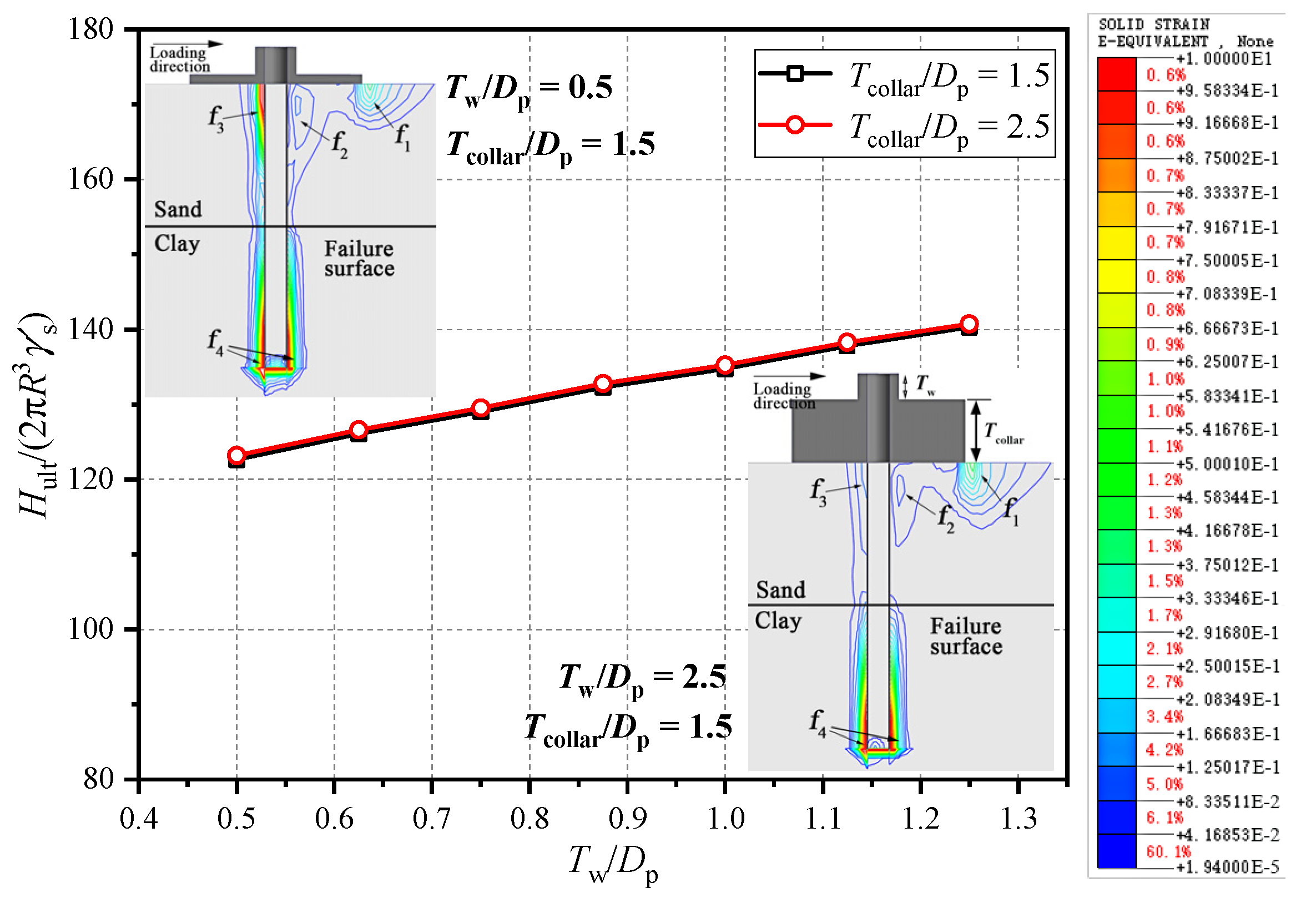
3.4. Effects of Wheel Thickness and Height of Collar (Tw/Dp and Tcollar/Dp)
4. Failure Envelopes in the V-H-M Domain
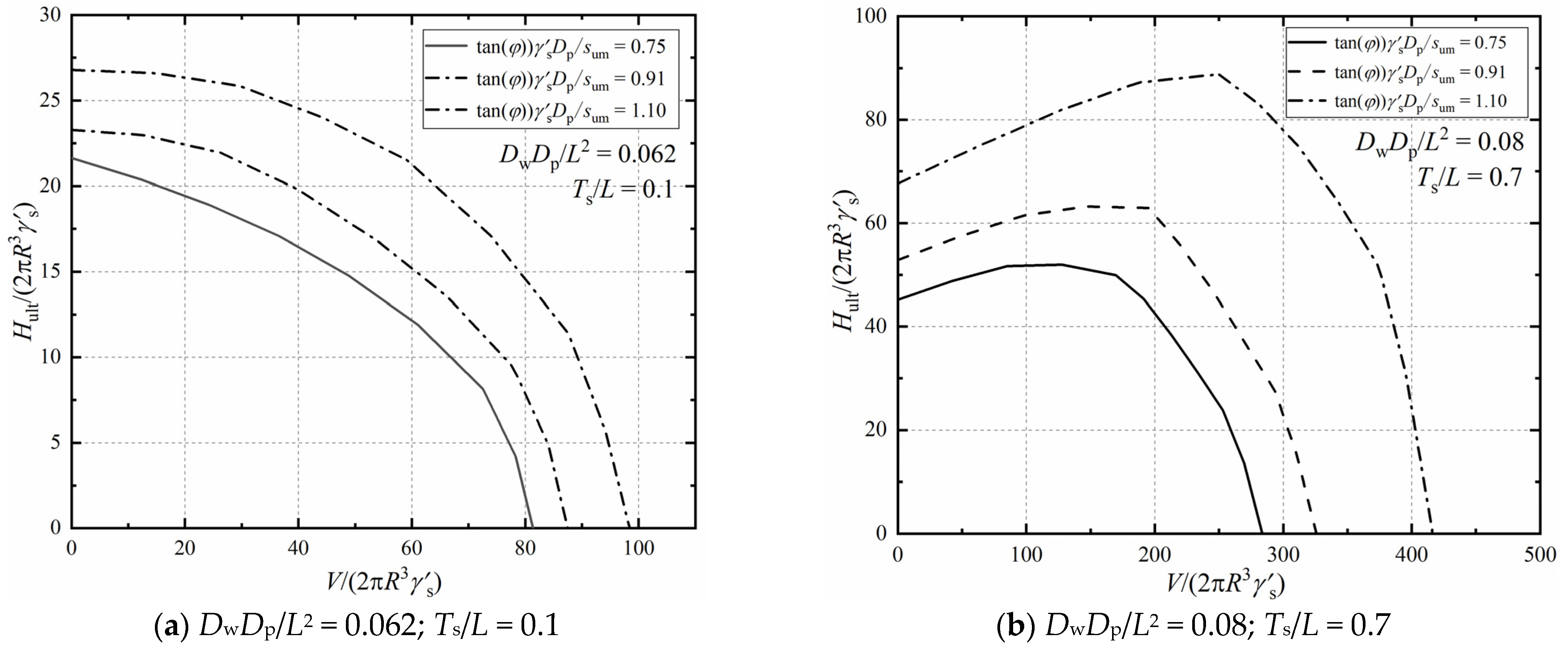
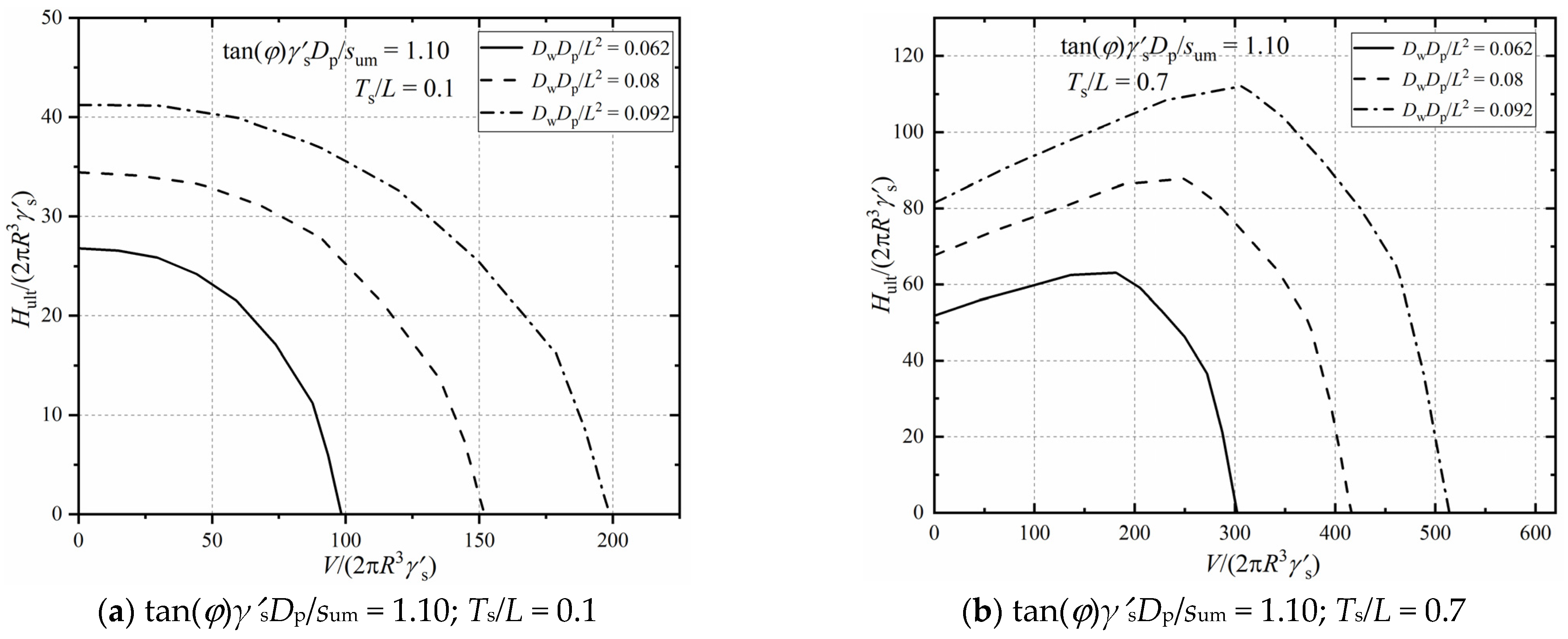
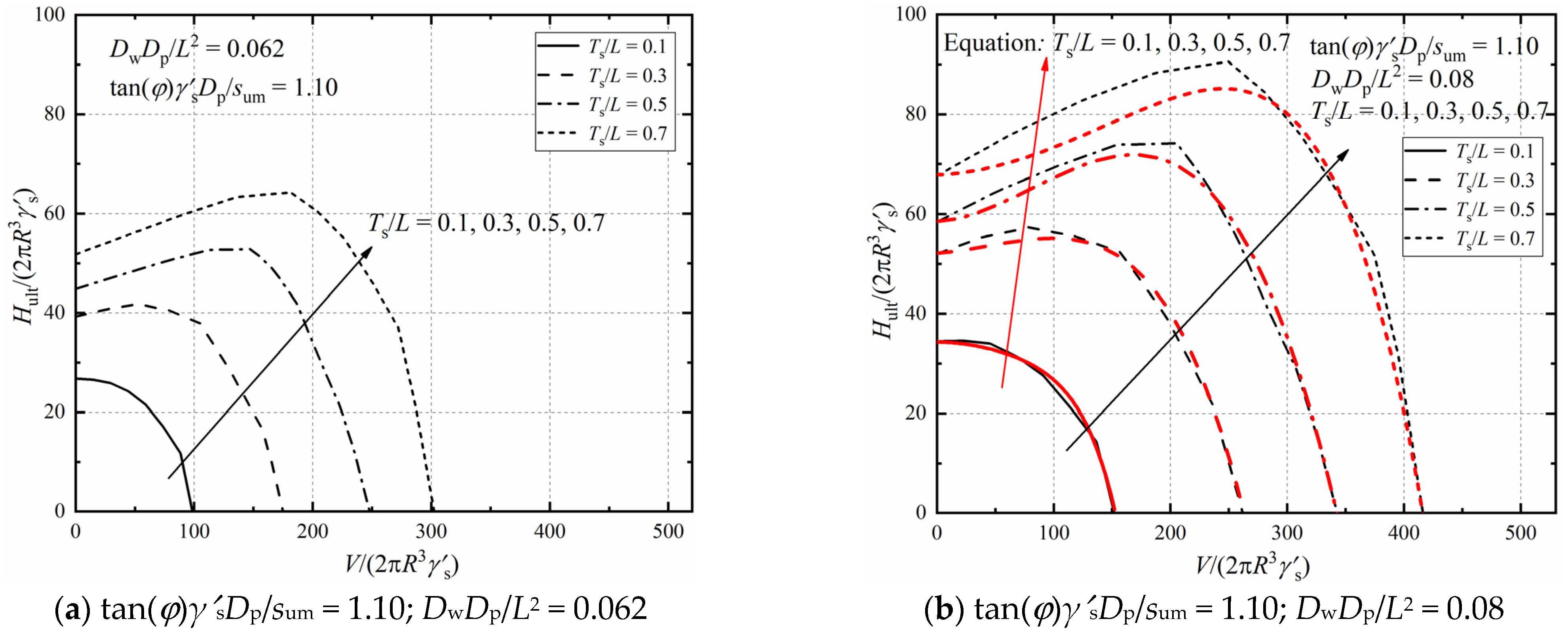
4.1. Parametric Study
4.2. Approximate Expression of the Bearing Capacity in the V-H-M Domain
4.3. Empirical Design Procedure and Validation
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Notations
| α | soil/pile friction coefficient |
| Dw | diameter of friction wheel |
| Dw0 | dimensionless foundation geometry |
| Dp | diameter of pile |
| dw | distance to the foundation center |
| e | lateral loading height |
| e0 | normalized lateral loading height |
| Ec | Young’s modulus of clay |
| Es | Young’s modulus of sand |
| H | lateral load |
| Hult | lateral capacity |
| H0 | uniaxial horizontal capacity |
| h0 | dimensionless uniaxial horizontal capacity |
| HBD | vertical boundary width |
| K0 | horizontal earth pressure coefficient |
| k | gradient of strength with depth |
| L | embedment length of pile |
| M | moment |
| Pw | soil passive resistance under the wheel |
| Pv | vertical pressure under the wheel |
| Pp | soil passive resistance along the pile |
| R | radius of the pile |
| su | undrained shear strength of clay |
| sum | undrained shear strength of clay at the mudline |
| Tw | thickness of friction wheel |
| Ts | thickness of sand layer |
| Tcollar | thickness of wheel collar |
| v0 | dimensionless vertical load |
| VBD | vertical boundary distance |
| V | vertical load |
| Vult | vertical capacity |
| γ′c | effective unit weight of clay |
| γ′s | effective unit weight of sand |
| z | depth below the mudline alongside the pile |
| zmax | depth of maximum earth pressure |
| φ | internal friction angle of sand fill |
| φ0 | normalized internal friction angle of sand |
| ψ | dilation angle |
| υ | Poisson’s ratio |
| A, B, C, D, E | coefficients of Equation (2) |
| a1, b1, c1, d1, e1, f1, g1, h1 | coefficients of Equation (3) |
| a2, b2, c2, d2 | coefficients of Equation (4) |
References
- Lee, M.; Bae, K.; Lee, I.; Yoo, M. Cyclic p-y Curves of Monopiles in Dense Dry Sand Using Centrifuge Model Tests. Appl. Sci. 2019, 9, 1641. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Z.; Guan, P.; Xu, J.; Wang, B.; Li, H.; Dong, Y. Horizontal Loading Performance of Offshore Wind Turbine Pile Foundation Based on DPP-BOTDA. Appl. Sci. 2020, 10, 492. [Google Scholar] [CrossRef] [Green Version]
- Plodpradit, P.; Dinh, V.; Kim, K. Coupled Analysis of Offshore Wind Turbine Jacket Structures with Pile-Soil-Structure Interaction Using FAST v8 and X-SEA. Appl. Sci. 2019, 9, 1633. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Y.; Andersen, K.; Tedesco, G. Ultimate bearing capacity of laterally loaded piles in clay—Some practical considerations. Mar. Struct. 2016, 50, 260–275. [Google Scholar] [CrossRef]
- Jeong, Y.; Park, M.; Kim, J.; Song, S. Wave Force Characteristics of Large-Sized Offshore Wind Support Structures to Sea Levels and Wave Conditions. Appl. Sci. 2019, 9, 1855. [Google Scholar] [CrossRef] [Green Version]
- Pandit, R.; Kolios, A. SCADA Data-Based Support Vector Machine Wind Turbine Power Curve Uncertainty Estimation and Its Comparative Studies. Appl. Sci. 2020, 10, 8685. [Google Scholar] [CrossRef]
- Schafer, M.; Madabhushi, S.P.G. Uplift Resistance of Enlarged Base Pile Foundations. Indian Geotech. J. 2020, 50, 426–441. [Google Scholar] [CrossRef]
- Yang, Y.; Qiu, L. MPM simulation of uplift resistance of enlarged base piles in sand. Soils Found. 2020, 60, 1322–1330. [Google Scholar] [CrossRef]
- Xu, H.; Yue, Z.; Qian, Q. Predicting uplift resistance of deep piles with enlarged bases. Proc. Inst. Civil Eng. Geotech. Eng. 2009, 162, 225–238. [Google Scholar] [CrossRef]
- Wang, X.; Zeng, X.; Li, J.; Yang, X. Lateral bearing capacity of hybrid monopile-footing foundation for offshore wind turbines by centrifuge modelling. Ocean Eng. 2018, 148, 182–192. [Google Scholar] [CrossRef]
- Yang, X.; Zeng, X.; Wang, X.; Berrila, J.; Li, X. Performance and bearing behavior of monopile-footing foundations under lateral-moment loading for offshore wind turbines. Ocean Eng. 2019, 184, 159–172. [Google Scholar] [CrossRef]
- Wang, X.; Zeng, X.; Yang, X.; Li, J. Seismic response of offshore wind turbine with hybrid monopile foundation based on centrifuge modelling. Appl. Energy 2019, 235, 1335–1350. [Google Scholar] [CrossRef]
- Carder, D.; Watson, G.; Chandler, R.; Powrie, W. Long-term performance of an embedded retaining wall with a stabilizing base slab. Proc. Inst. Civil. Eng. Geotech. Eng. 1999, 137, 63–74. [Google Scholar] [CrossRef]
- Mokwa, R.; Duncan, J. Experimental evaluation of lateral-load resistance of pile caps. J. Geotech. Geoenviron. Eng. 2001, 127, 185–192. [Google Scholar] [CrossRef]
- Rollins, K.; Cole, R. Cyclic lateral load behavior of a pile cap and backfill. J. Geotech. Geoenviron. Eng. 2006, 132, 1143–1153. [Google Scholar] [CrossRef]
- Wang, Z.; Hu, R.; Leng, H.; Liu, H.; Bai, Y.; Lu, W. Deformation Analysis of Large Diameter Monopiles of Offshore Wind Turbines under Scour. Appl. Sci. 2020, 10, 7579. [Google Scholar] [CrossRef]
- Yang, B.; Wei, K.; Yang, W.; Li, Y.; Qin, B.; Ning, L. A Feasibility Study for Using Fishnet to Protect Offshore Wind Turbine Monopile Foundations from Damage by Scouring. Appl. Sci. 2019, 9, 5023. [Google Scholar] [CrossRef] [Green Version]
- Zou, X.; Hu, Y.; Hossain, M.; Zhou, M. Capacity of skirted foundations in sand-over-clay under combined V-H-M loading. Ocean Eng. 2018, 159, 201–218. [Google Scholar] [CrossRef]
- Yang, C.; Zhu, Z.; Xiao, Y. Bearing Capacity of Ring Foundations on Sand Overlying Clay. Appl. Sci. 2020, 10, 4675. [Google Scholar] [CrossRef]
- Jan, M.; Adeel, M.; Pervaiz, U.; Park, D. Estimation of Distribution Factor for Peak Penetration Resistance Prediction of Spudcan Foundations in Loose to Medium-Dense Sand Overlying Clay. Appl. Sci. 2020, 10, 8795. [Google Scholar] [CrossRef]
- Hu, P.; Cassidy, M.; Randolph, M. Bearing capacity on sand overlying clay: An analytical model for predicting post peak behaviour. Mar. Struct. 2018, 59, 94–104. [Google Scholar] [CrossRef]
- Hossain, M.; Hu, P.; Cassidy, M.; Menzies, D.; Wingate, A. Measured and calculated spudcan penetration profiles for case histories in sand-over-clay. Appl. Ocean Res. 2019, 82, 447–457. [Google Scholar] [CrossRef]
- Zheng, G.; Zhao, J.; Zhou, H.; Zhang, T. Ultimate Bearing Capacity of Strip Footings on Sand Overlying Clay under Inclined Loading. Comput. Geotech. 2018, 106, 266–273. [Google Scholar] [CrossRef]
- Stone, K.; Newson, T.; Sandon, J. An Investigation of the Performance of a ‘Hybrid’ Monopile-footing Foundation for Offshore Structures. In Proceedings of the 6th International Offshore Site Investigation and Geotechnics Conference: Confronting New Challenges and Sharing Knowledge, London, UK, 11–13 September 2007; pp. 11–13. [Google Scholar]
- El-Marassi, M. Investigation of Hybrid Monopile-Footing Foundation Systems Subjected to Combined Loading. Ph.D. Thesis, The University of Western Ontario, London, ON, Canada, 2011. [Google Scholar]
- Lehane, B.; Powrie, W.; Doherty, J. Centrifuge model tests on piled footings in clay for offshore wind turbines. In Proceedings of the International Conference in Physical Modelling in Geotechnics, Zurich, Switzerland, 28 June–1 July 2010; Balkema: Rotterdam, The Netherlands, 2010. [Google Scholar]
- Lehane, B.; Pedram, B.; Doherty, J.; Powrie, W. Improved performance of monopiles when combined with footings for tower foundations in sand. J. Geotech. Geoenviron. Eng. 2014, 140, 04014027. [Google Scholar] [CrossRef]
- Anastasopoulos, I.; Theofilou, M. Piled footing for offshore wind turbines: Environmental and seismic loading. Soil Dyn. Earthq. Eng. 2016, 80, 192–209. [Google Scholar] [CrossRef]
- Abdelkader, A.; Naggar, E.; Hesham, M. Piled footing System for Offshore Wind Turbine. Geotech. Geol. Eng. 2018, 36, 2921–2937. [Google Scholar] [CrossRef]
- Pedram, B. Behaviour of Hybrid Piled Footing Structures in Sands. Geotech. Geol. Eng. 2018, 36, 2273–2292. [Google Scholar] [CrossRef]
- Wang, X.; Li, J. Parametric study of hybrid monopile foundation for offshore wind turbines in cohesionless soil. Ocean Eng. 2020, 218, 108172. [Google Scholar] [CrossRef]
- Yang, X.; Zeng, X.; Wang, X.; Yu, H. Performance of monopile-footing foundations under lateral loading for offshore wind turbines. Appl. Ocean Res. 2018, 78, 14–24. [Google Scholar] [CrossRef]
- Trojnar, K. Multi scale studies of the new piled footings for offshore wind turbines. Ocean Eng. 2019, 192, 106506. [Google Scholar] [CrossRef]
- Trojnar, K. Simplified design of new hybrid monopile foundations for offshore wind turbines. Ocean Eng. 2021, 219, 108046. [Google Scholar] [CrossRef]
- Li, X.; Zeng, X.; Wang, X. Feasibility study of monopile-footing-bucket piled footing for offshore wind turbine. Ocean Eng. 2020, 204, 107276. [Google Scholar] [CrossRef]
- Stone, K.; Arshi, H.; Zdravkovic, L. Use of a bearing plate to enhance the lateral capacity of monopiles in sand. J. Geotech. Geoenviron. Eng. 2018, 144, 04018051. [Google Scholar] [CrossRef]
- Dassault Systèmes. Abaqus Analysis User’s Manual; Simulia Corp.: Santa Clara, CA, USA, 2016. [Google Scholar]
- Hao, E.; Liu, C. Evaluation and comparison of anti–impact performance to offshore wind turbine foundations: Monopile, tripod, and jacket. Ocean Eng. 2017, 130, 218–227. [Google Scholar] [CrossRef]
- Moulas, D.; Shafiee, M.; Mehmanparast, A. Damage analysis of ship collisions with offshore wind turbine foundations. Ocean Eng. 2017, 143, 149–162. [Google Scholar] [CrossRef]
- Malhotra, S. Selection, design and construction of offshore wind turbine foundations. In Wind Turbines; Al-Bahadly, I., Ed.; InTechOpen: London, UK, 2011. [Google Scholar]
- Al-Mhaidib, A.I. Experimental investigation of the behavior of pile groups in sand under different loading rates. Geotech. Geol. Eng. 2006, 24, 889–902. [Google Scholar] [CrossRef]
- Hung, L.; Kim, S. Evaluation of undrained bearing capacities of bucket foundations under combined loads. Mar. Georesour. Geotechnol. 2014, 32, 76–92. [Google Scholar] [CrossRef]
- Zhou, M.; Hossain, M.; Hu, Y.; Liu, H. Behaviour of ball penetrometer in uniform single-and double-layer clays. Géotechnique 2013, 63, 682–694. [Google Scholar] [CrossRef]
- Vulpe, C.; Gourvenec, S.; Leman, B.; Fung, K. Failure envelope approach for consolidated undrained capacity of shallow foundations. J. Geotech. Geoenviron. Eng. 2016, 142, 04016036. [Google Scholar] [CrossRef] [Green Version]
- Gourvenec, S.; Barnett, S. Undrained failure envelope for skirted foundations under general loading. Géotechnique 2011, 61, 263–270. [Google Scholar] [CrossRef]
- Gourvenec, S.; Mana, D. Undrained vertical bearing capacity factors for shallow foundations. Géotech. Lett. 2011, 1, 101–108. [Google Scholar] [CrossRef]
- Randolph, M.; Gourvenec, S.; Acosta-Martinez, H. Experimental Study of Uplift Resistance of Shallow Skirted Foundations in Clay under Transient and Sustained Concentric Loading. Géotechnique 2009, 59, 525–537. [Google Scholar]
- Mahmoud, G.; Javad, N. Bearing capacity of geosynthetic encased stone columns. Geotext. Geomembr. 2013, 38, 26–36. [Google Scholar]
- Jung, S.; Kim, S.; Patil, A.; Hung, L. Effect of monopile foundation modeling on the structural response of a 5-MW offshore wind turbine tower. Ocean Eng. 2015, 109, 479–488. [Google Scholar] [CrossRef] [Green Version]
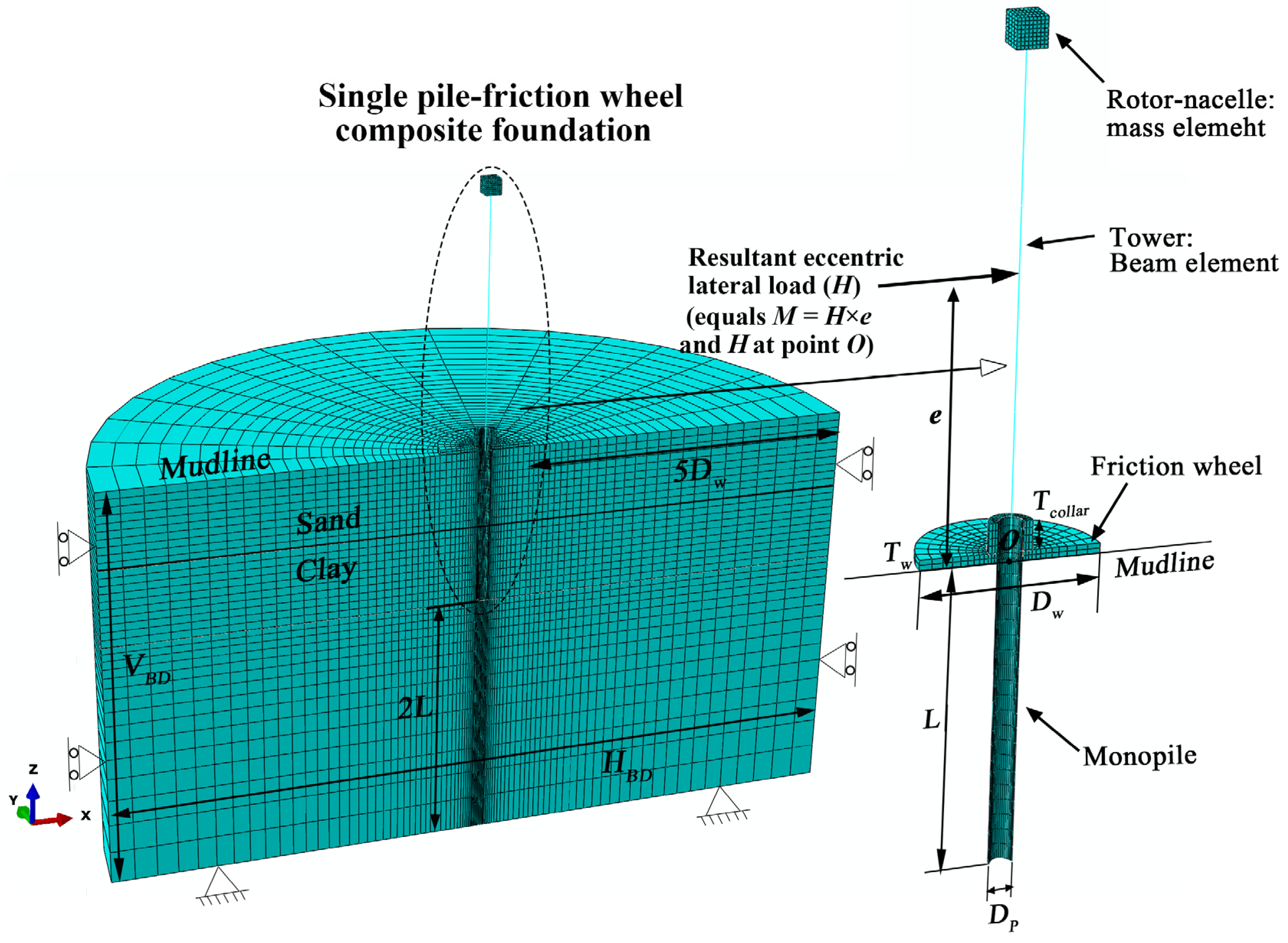
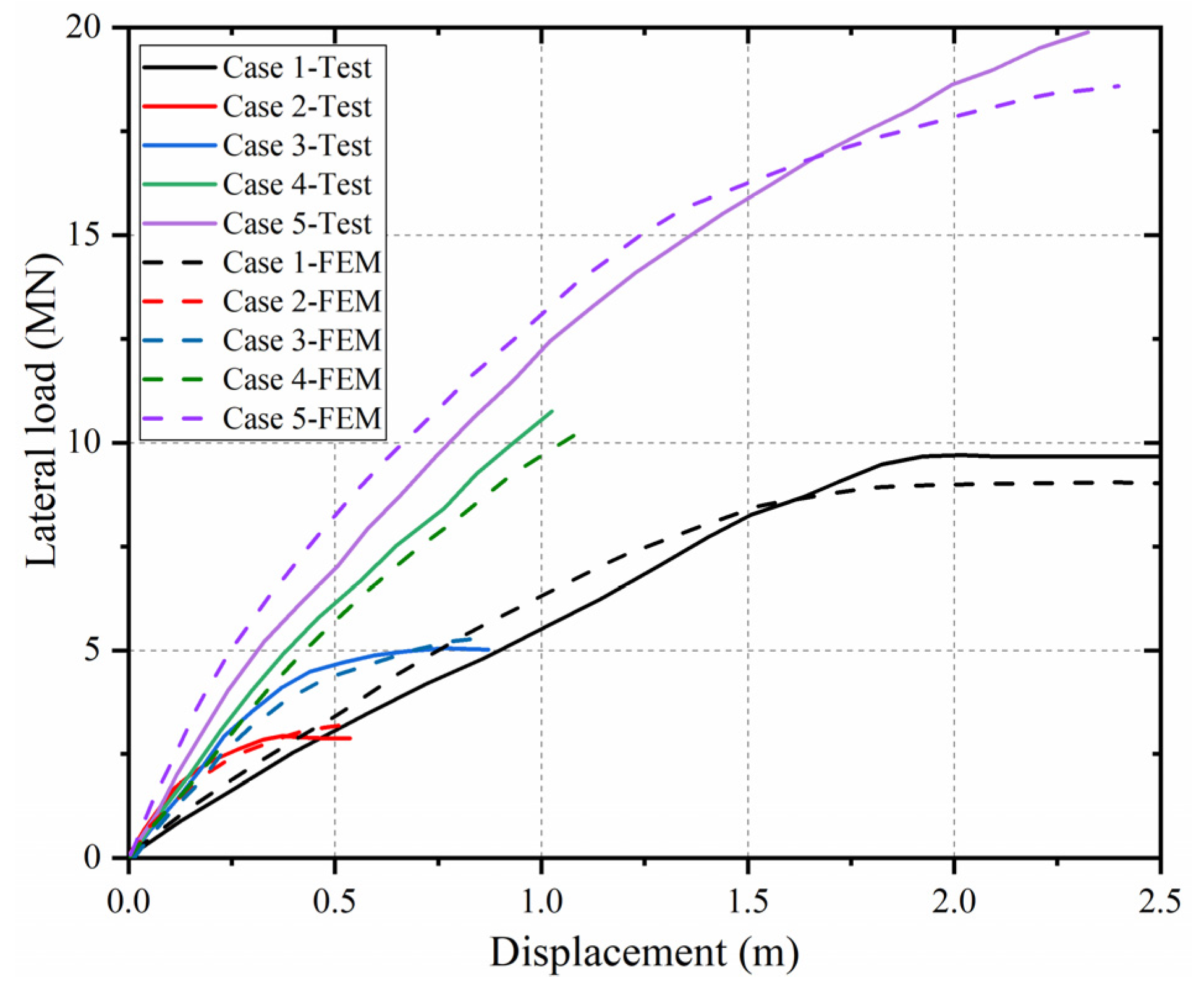



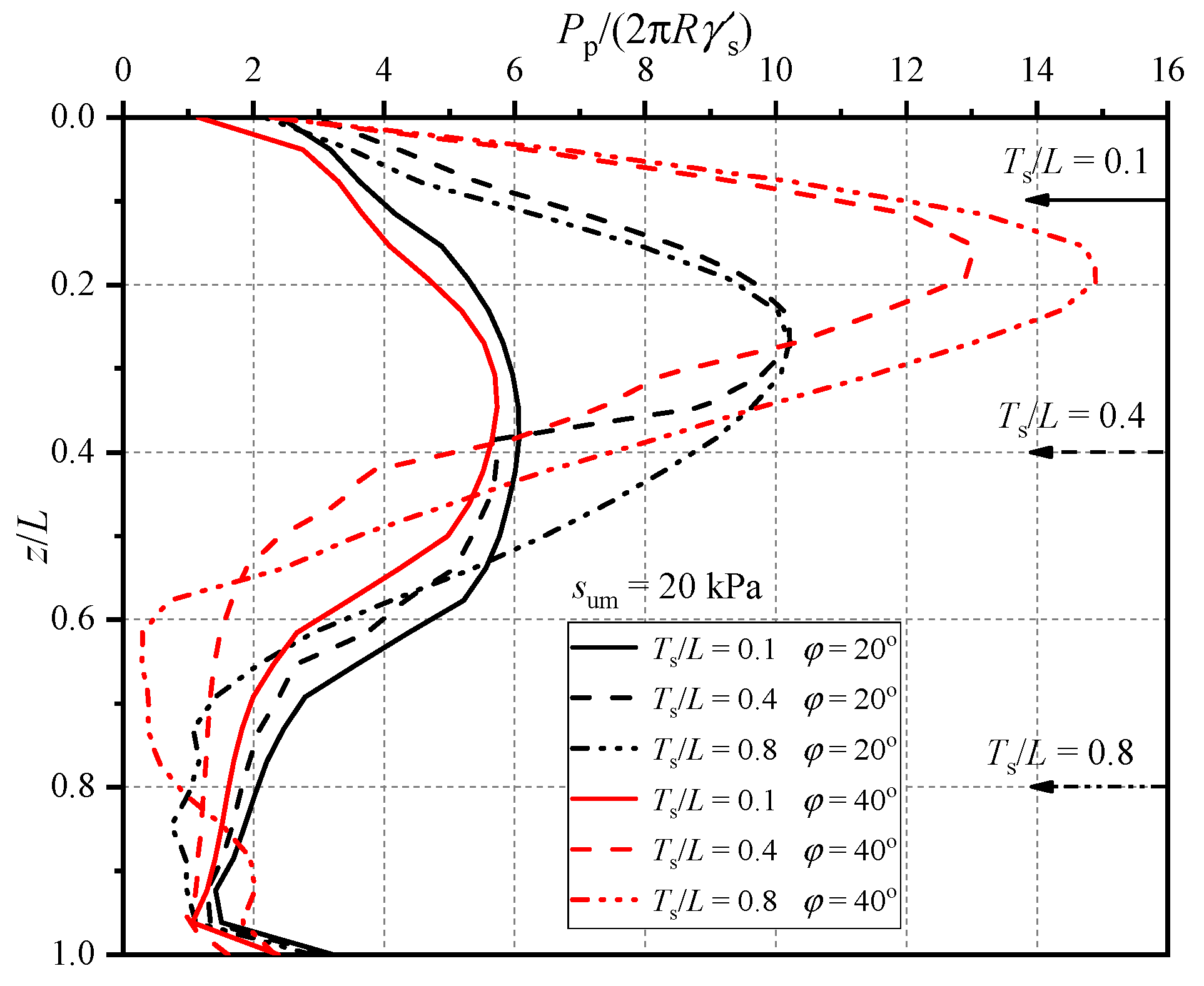
| Analysis | Dw/L | V/Vult | e/Dp | Ts/L | sum | φ (sand) | Tw/Dp | Notes |
|---|---|---|---|---|---|---|---|---|
| Group I | 0, 0.5, wheel | 5.9, 12.7, 6.4, 13.2 (MN) | 9 | 1 | 0 | 33° | 0.8 | Numerical model validation [27] |
| Group II | 0~0.8 | 0 | 10 | 0.5 | 30 kPa | 33° | 0.5 | Investigation for the effect of the wheel diameter |
| Group III | 0.6 | 0~95% | 6~16 | 0.5 | 30 kPa | 33° | 0.5 | Investigation for the effect of the combined pre-vertical load and moment |
| Group IV | 0.6 | 0 | 10 | 0.1~0.8 | 20 kPa, 40 kPa (k = 1.75 and 2 kPa) | 20~40° | 0.5 | Investigation for the effect of thickness of sand layer |
| Group V | 0.6 | 0 | 10 | 0.5 | 30 kPa | 33° | 0.5~1.25 | Investigation for the effect of wheel thickness |
| Ts/L | tan(φ)γ′sDp/sum | A | B | C | D | E |
|---|---|---|---|---|---|---|
| 0.1 | 0.754 | 1 | 1.177 | −0.505 | 0.439 | 3.784 |
| 0.1 | 0.848 | 1 | 1.847 | −0.790 | 0.209 | 27.125 |
| 0.1 | 0.949 | 1 | 2.200 | −0.790 | 0.210 | 45 |
| 0.1 | 1.096 | 0.739 | 1.913 | −0.551 | 0.774 | 4.49 |
| 0.3 | 0.754 | 1 | 2.172 | 0.508 | 1.411 | 0.194 |
| 0.3 | 0.848 | 1 | 2.8 | 2.725 | 3.659 | 0.201 |
| 0.3 | 0.949 | 1 | 1.85 | 0.351 | 1.307 | 1.55 |
| 0.3 | 1.096 | 1 | 1.355 | 0.322 | 1.314 | 2.552 |
| 0.5 | 0.754 | 1 | 1.256 | 0.914 | 1.653 | 0.891 |
| 0.5 | 0.848 | 1 | 1.831 | 14.907 | 15.665 | 0.084 |
| 0.5 | 0.949 | 1 | 0.961 | 0.305 | 1.300 | 3.593 |
| 0.5 | 1.096 | 1 | 1.718 | 1.598 | 2.623 | 1.621 |
| 0.7 | 0.754 | 0.302 | 0.015 | 0.203 | 3.422 | 4.655 |
| 0.7 | 0.848 | 0.602 | 0.321 | 0.161 | 1.794 | 4.572 |
| 0.7 | 0.949 | 0.874 | 0.869 | 0.314 | 1.448 | 3.357 |
| 0.7 | 1.096 | 1.063 | 1.709 | 0.889 | 1.862 | 3.471 |
| Ts/L | a1 | b1 | c1 | d1 | e1 | f1 | g1 | h1 |
|---|---|---|---|---|---|---|---|---|
| 0.1 | 1.61 | 230 | −0.21 | −2.78 | −1.22 | 399.44 | 0.19 | −12.22 |
| 0.3 | −15.99 | 457.22 | 0.53 | −11.67 | −16.99 | 957.22 | 1.33 | −37.78 |
| 0.5 | −2.25 | 164.44 | 0.07 | −2.22 | 0.09 | 948.33 | −0.035 | −25.56 |
| 0.7 | 7.52 | −260.56 | −0.6 | 15 | −9.02 | 1506.67 | 0.54 | −45 |
| Ts/L | a2 | b2 | c2 | d2 |
|---|---|---|---|---|
| 0.1 | −72.57 | 1876.67 | −11.97 | 997.22 |
| 0.3 | −101.01 | 2206.11 | −21.93 | 2400 |
| 0.5 | −74.47 | 1263.89 | −3.82 | 3653.89 |
| 0.7 | −136.69 | 1598.33 | 42.76 | 4313.89 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Zou, X.; Hu, J. Bearing Capacity of Single Pile-Friction Wheel Composite Foundation on Sand-over-Clay Deposit under V-H-M Combined Loadings. Appl. Sci. 2021, 11, 9446. https://doi.org/10.3390/app11209446
Wang Y, Zou X, Hu J. Bearing Capacity of Single Pile-Friction Wheel Composite Foundation on Sand-over-Clay Deposit under V-H-M Combined Loadings. Applied Sciences. 2021; 11(20):9446. https://doi.org/10.3390/app11209446
Chicago/Turabian StyleWang, Yikang, Xinjun Zou, and Jianfeng Hu. 2021. "Bearing Capacity of Single Pile-Friction Wheel Composite Foundation on Sand-over-Clay Deposit under V-H-M Combined Loadings" Applied Sciences 11, no. 20: 9446. https://doi.org/10.3390/app11209446
APA StyleWang, Y., Zou, X., & Hu, J. (2021). Bearing Capacity of Single Pile-Friction Wheel Composite Foundation on Sand-over-Clay Deposit under V-H-M Combined Loadings. Applied Sciences, 11(20), 9446. https://doi.org/10.3390/app11209446






