Dual-Gratings Imaging Spectrometer
Abstract
:1. Introduction
2. Dual-Gratings Imaging Spectrometer
2.1. Traditional Imaging Spectrometer
2.2. The Role of Double Grating
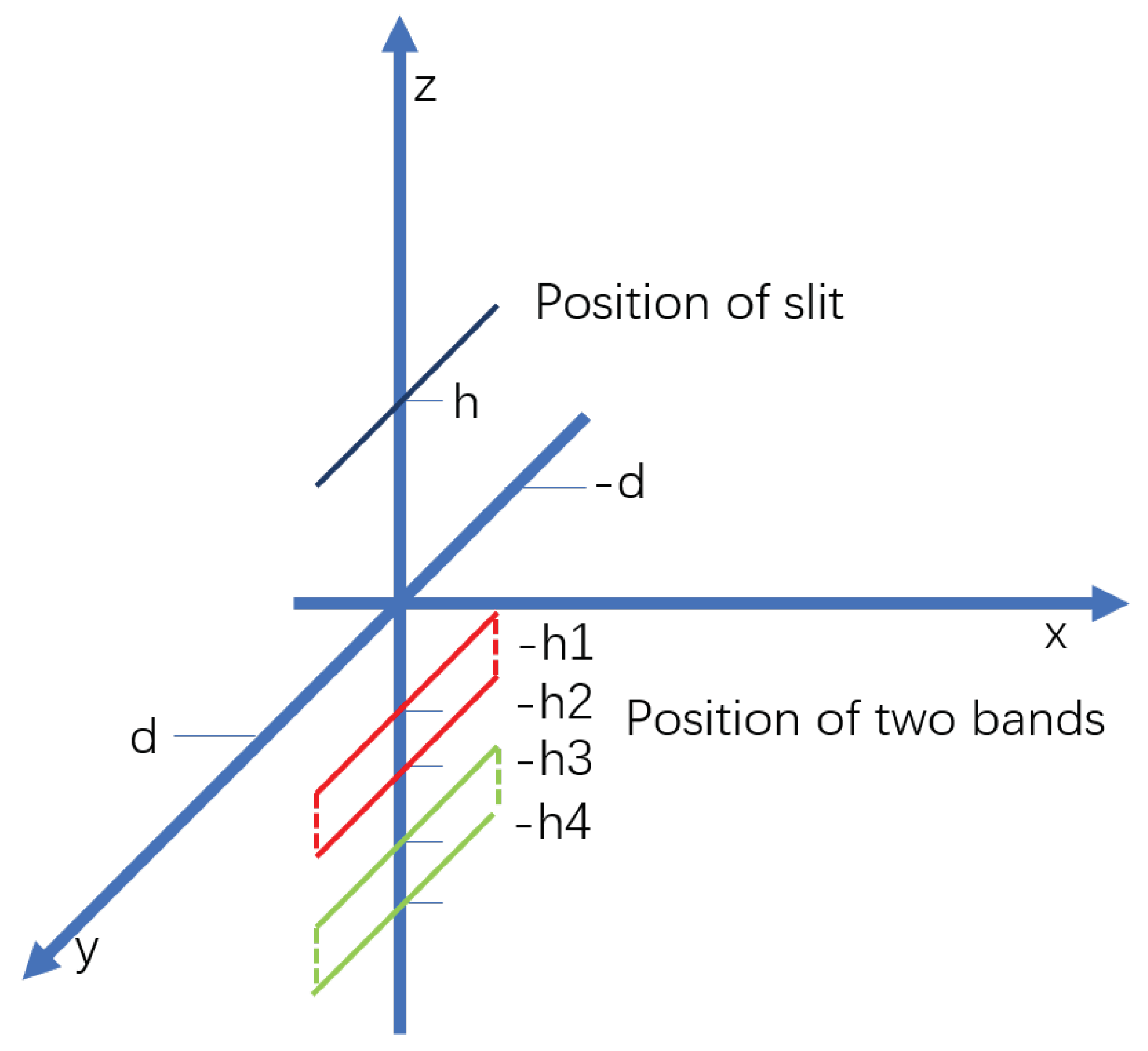
2.3. Relative Position of Double Gratings
2.4. Overlap between Two Spectra
- (1)
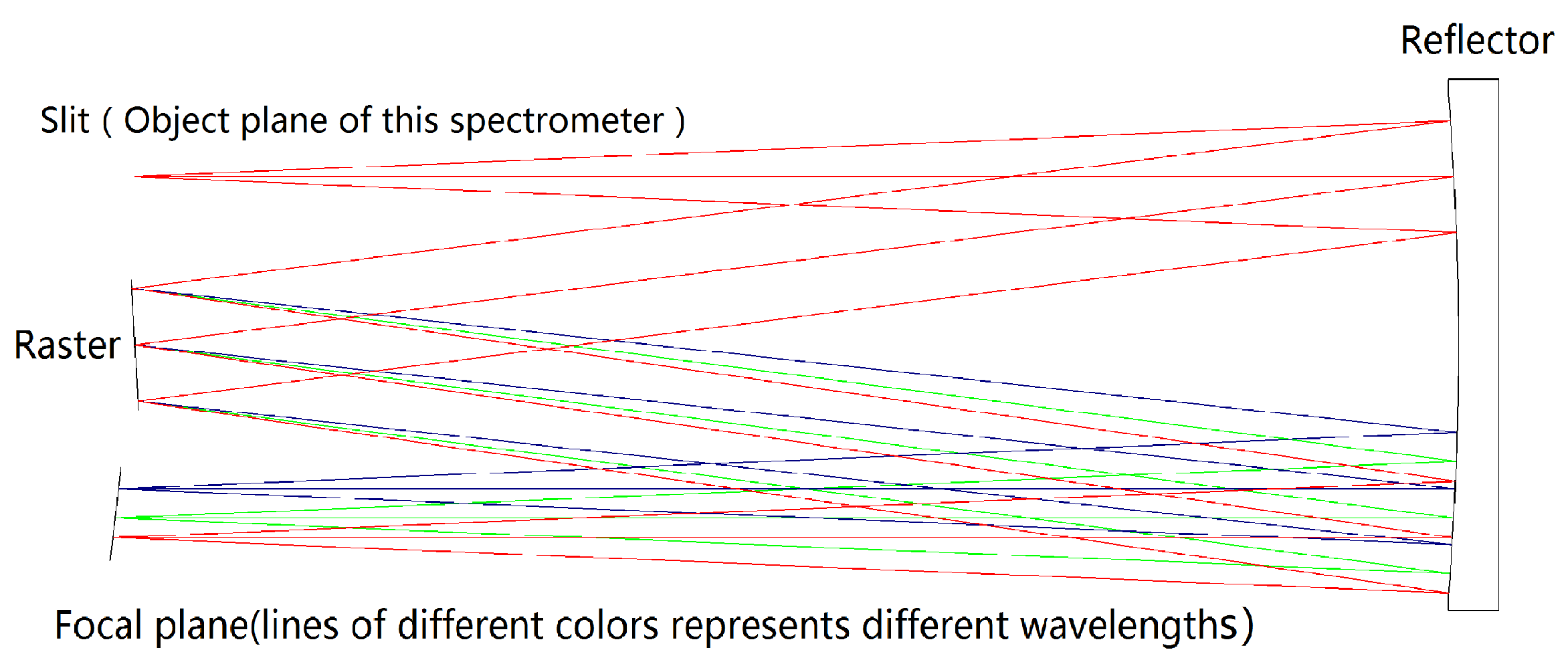
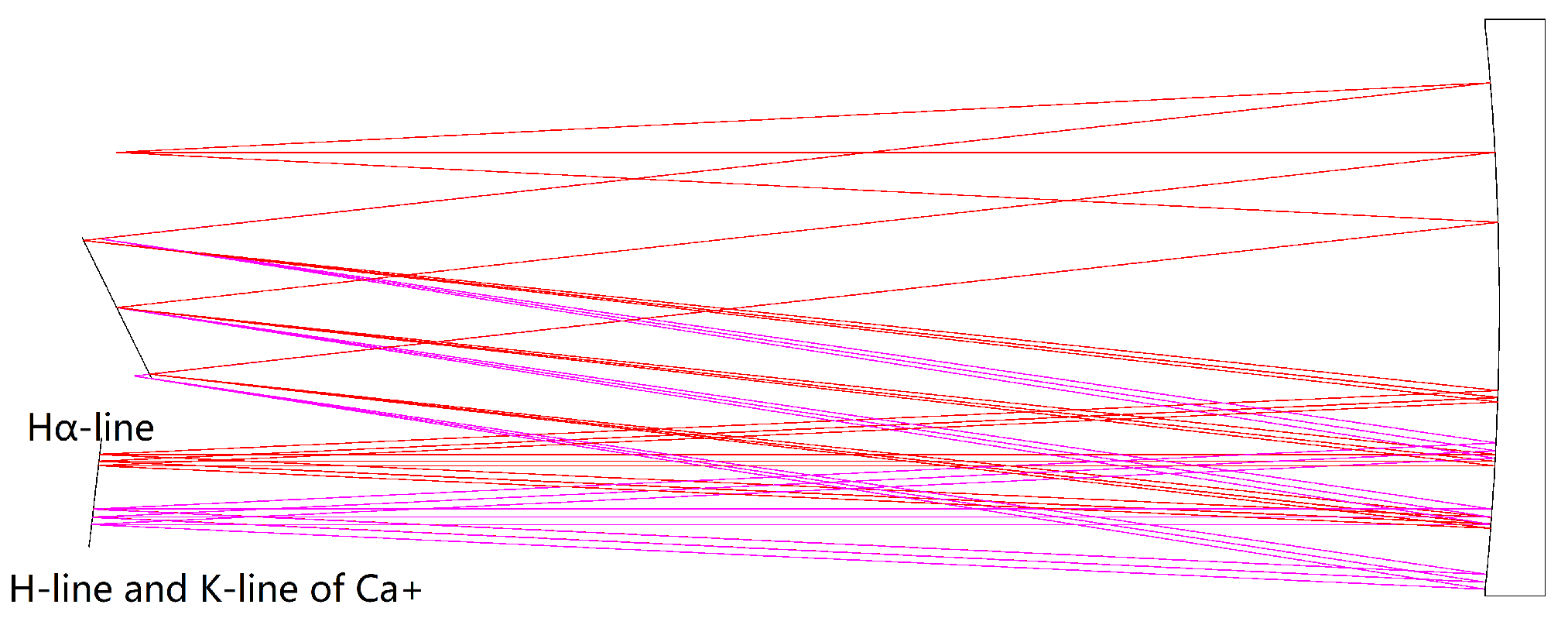
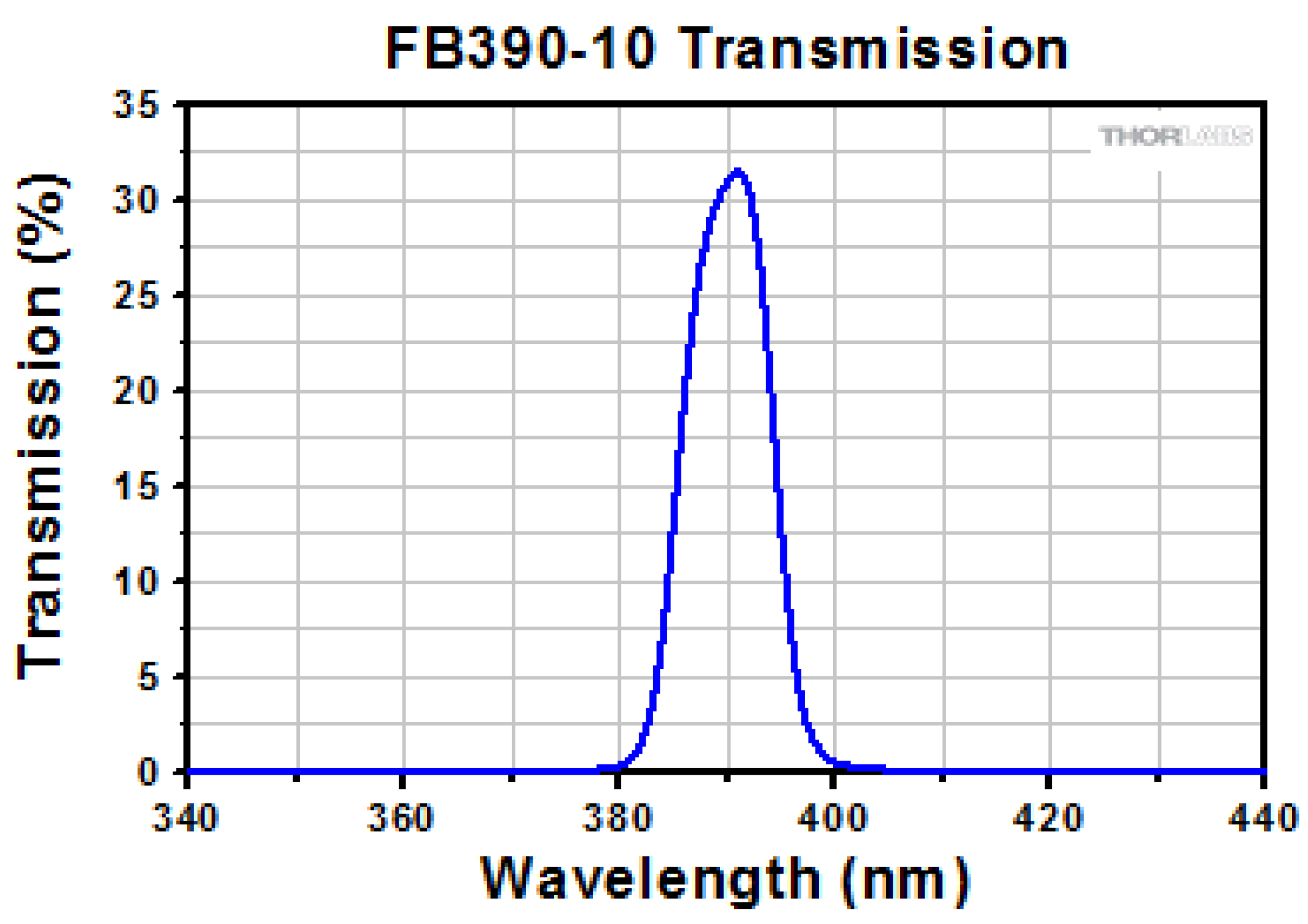
- For overlap between double spectra, this article has two ideas; the first one is the traditional method, adding filters in front of the gratings to prevent aliasing by constraining the imaging bands. The second is to use the response range of the detector. Imaging the second waveband in the unresponsive area of the first waveband can reduce one filter as shown in Figure 6. The second scheme is suitable for situations where the imaging band is close to the edge of the detector’s response band.
- (2)
- For the overlap between high-order spectrum and imaging bands, there is another way to be considered, that is, one grating works at +1 order, and the other grating works at −1 order. In this way, the high-order spectral imaging area can be staggered from the imaging area as shown in Figure 7.
2.5. Rotation Angle of Double Gratings
2.6. Optical Structure of the Dual-Gratings Type Imaging Spectrometer
2.7. The Relationship between the Slit and Image
2.8. Double Images Offset Problem
2.9. The Effect of Double-Gratings on MTF
2.10. Limitations of Dual-Grating Imaging Spectrometers
- (1)
- Cost increase. In order to prevent overlapping of spectra, the dual grating imaging spectrometer needs to add two filters and one grating, which increases the cost.
- (2)
- The low energy utilization rate. This shortcoming is composed of two reasons. The first is because the double gratings bisects the aperture into an image, resulting in only half of the total energy in each band. The second reason is because in the imaging process, it needs to pass through the filter twice, which reduces the energy utilization rate.
- (3)
- Stray light is more complicated. Due to the lower energy utilization rate of dual gratings, the stray light in the system is more than that of traditional single gratings.
3. Simulation of a Dual-Gratings Spectrometer
4. Results
5. Comparison with Traditional Scheme
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Wolfe, W.L. Introduction to Imaging Spectrometers. Opt. Photonics News 1997, 9, 142–149. [Google Scholar]
- Chabrillat, S.; Goetz, A.F.H.; Krosley, L.; Olsen, H.W. Use of hyperspectral images in the identification and mapping of expansive clay soils and the role of spatial resolution. Remote Sens. Environ. 2002, 82, 431–445. [Google Scholar] [CrossRef]
- Chang, C.I. Hyperspectral Imaging: Techniques for Spectral Detection and Classification. In Hyperspectral Imaging: Techniques for Spectral Detection and Classification; Kluwer Academic; Plenum Publishers: New York, NY, USA, 2003. [Google Scholar]
- Goetz, A.F.H. Three decades of hyperspectral remote sensing of the Earth: A personal view. Remote Sens. Environ. 2009, 113, S5–S16. [Google Scholar] [CrossRef]
- Wessman, C.A.; Aber, J.D.; Peterson, D.L.; Melillo, J.M. Remote sensing of canopy chemistry and nitrogen cycling in temperate forest ecosystems. Nature 1988, 335, 154–156. [Google Scholar] [CrossRef]
- Castro-Esau, K.L.; Sánchez-Azofeifa, G.A.; Benoit, R.; Wright, J.S.; Mauricio, Q. Variability in leaf optical properties of Mesoamerican trees and the potential for species classification. J. Abbr. 2006, 93, 517–530. [Google Scholar] [CrossRef] [PubMed]
- Cheng, Y.B.; Ustin, S.L.; Ria, D.; Vanderbilt, V.C. Water content estimation from hyperspectral images and MODIS indexes in Southeastern Arizona. Remote Sens. Environ. 2008, 112, 363–374. [Google Scholar] [CrossRef]
- Cheng, Y.B.; Zarco-Tejada, P.J.; Ria, D.; Rueda, C.A.; Ustin, S.L. Estimating vegetation water content with hyperspectral data for different canopy scenarios: Relationships between AVIRIS and MODIS indexes. J. Abbr. 2006, 105, 354–366. [Google Scholar] [CrossRef]
- Bruniquel-Pinel, V.; Gastellu-Etchegorry, J.P. Sensitivity of Texture of High Resolution Images of Forest to Biophysical and Acquisition Parameters. Remote Sens. Environ. 1998, 65, 61–85. [Google Scholar] [CrossRef]
- Zheng, Y.Q.; Wang, H.; Wang, Y.F.T. Selection and design of optical systems for spaceborne hyperspectral imagers. Opt. Precis. Eng. 2009, 11, 2629–2637. [Google Scholar]
- Xie, Y.; Liu, C.; Liu, S.; Fan, X. Optical design of imaging spectrometer based on linear variable filter for nighttime light remote sensing. Sensors 2021, 21, 4313. [Google Scholar] [CrossRef] [PubMed]
- Reimers, J.; Bauer, A.; Thompson, K.; Rolland, J.P. Freeform spectrometer enabling increased compactness. Light Sci. Appl. 2017, 6, e17026. [Google Scholar] [CrossRef] [PubMed]
- Kong, P.; Tang, Y.; Bayanheshig, X.Q.; Li, W.; Cui, J. Double-Grating Minitype Flat-Field Holographic Concave Grating Spectrograph. Acta Opt. Sin. 2013, 33, 0105001. [Google Scholar] [CrossRef]
- Liu, J.; Qi, X.; Zhang, S.; Sun, C.; Zhu, J.; Cui, J.; Li, X. Backscattering Raman spectroscopy using multi-grating spatial heterodyne Raman spectrometer. Appl. Opt. 2018, 57, 9735–9745. [Google Scholar] [CrossRef] [PubMed]
- Xue, Q.; Lu, F.; Duan, M.; Zheng, Y.; Wang, X.; Cao, D.; Lin, G.; Tian, J. Optical design of double-grating and double wave band spectrometers using a common CCD. Appl. Opt. 2018, 57, 6823. [Google Scholar] [CrossRef] [PubMed]
- Feng, Z.; Xia, G.; Lu, R.; Cai, X.; Hu, M. High-performance ultra-thin spectrometer optical design based on coddington’s equations. Sensors 2021, 21, 323. [Google Scholar] [CrossRef] [PubMed]
- Mouroulis, P.; Green, R.O.T. Review of high fidelity imaging spectrometer design for remote sensing. Opt. Eng. 2018, 57, 040901. [Google Scholar] [CrossRef] [Green Version]
- Shafer, A.B.; Megill, L.R.; Droppleman, L. Optimization of the czerny-turner spectrometer. J. Opt. Soc. Am. 1964, 54, 879–886. [Google Scholar] [CrossRef]
- Schmidt, W.; Beck, C.; Kentischer, T.; Elmore, D.; Lites, B. POLIS: A spectropolarimeter for the VTT and for GREGOR. Astron. Nachrichten 2010, 324, 300–301. [Google Scholar] [CrossRef]
- Kuzin, V.S.; Bogachev, A.S.; Zhitnik, A.I.; Pertsov, A.A.; Bugaenko, O.I. Tesis experiment on euv imaging spectroscopy of the sun. Adv. Space Res. 2009, 43, 1001–1006. [Google Scholar] [CrossRef]






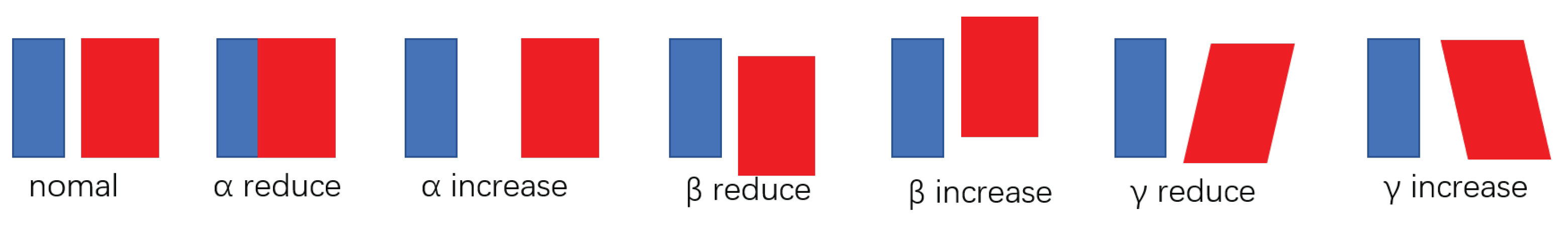

| 3D Angle | Change | Influence |
|---|---|---|
| Increase | Distance between the two images expand | |
| Decrease | Distance between the two images shrink | |
| Increase | Dislocation in the spatial dimension. | |
| Decrease | Opposite dislocation in the spatial dimension. | |
| Increase | Tilt between the two images | |
| Decrease | Opposite tilt between the two images |
| Parameter | Value |
|---|---|
| System focal length | 600 mm |
| Waveband | 391.4 nm–398.8 nm, and 653.281 nm–658.281 nm |
| Grating line pairs | 1350 L/mm and 1350 L/mm |
| Slit | 16 mm × 5 μm |
| Detector | 5 μm (To calculate the number of spectrum data) |
| NA(object side) | 0.05 |
| Data | Value |
|---|---|
| Spectral resolution | H each band 0.005 nm & H and K each band 0.006 nm |
| MTF in 32 L/mm | higher than 0.6 |
| Smile | H is 3.5 μm & H and K is 3.7 μm |
| The volume of the spectrometer | 0.015 m |
| Number of output spectrum data | H 1000 & H and K 1234 |
| Grating widths | 33 mm × 66 mm × 2 |
| Mirror size | 60 mm × 60 mm and 60 mm × 88 mm |
| Angle between two gratings | 42.85° |
| Dual-Gratings Spectrometer | Traditional Spectrometer | |
|---|---|---|
| Output image | 2-D images (spectral and spatial) | 2-D images (spectral and spatial) |
| Spectral resolution | high | high |
| volume | small | big |
| suitable for satellite | Yes | Yes |
| Currently adopted | rare | rare |
| Dual-Gratings Spectrometer | Filter | |
|---|---|---|
| Output image | 2-D images (spectral and spatial) | Monochrome image |
| Spectral resolution | high | low |
| the acquisition speed | low (need time to scan) | real time |
| Features | Continuous spectrum data | High-precision spatial information |
| Number of spectrum data | Multiple | 1 (Adjustable ) |
| Currently adopted | Rare | Broadly used |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ouyang, R.; Wang, D.; Jin, L.; Zhang, X. Dual-Gratings Imaging Spectrometer. Appl. Sci. 2021, 11, 11048. https://doi.org/10.3390/app112211048
Ouyang R, Wang D, Jin L, Zhang X. Dual-Gratings Imaging Spectrometer. Applied Sciences. 2021; 11(22):11048. https://doi.org/10.3390/app112211048
Chicago/Turabian StyleOuyang, Rui, Duo Wang, Longxu Jin, and Xingxiang Zhang. 2021. "Dual-Gratings Imaging Spectrometer" Applied Sciences 11, no. 22: 11048. https://doi.org/10.3390/app112211048
APA StyleOuyang, R., Wang, D., Jin, L., & Zhang, X. (2021). Dual-Gratings Imaging Spectrometer. Applied Sciences, 11(22), 11048. https://doi.org/10.3390/app112211048






