Enhancement of Subcooled Flow Boiling Heat Transfer with High Porosity Sintered Fiber Metal
Abstract
:1. Introduction
2. Experimental Apparatus and Procedure
2.1. Experimental Apparatus and Procedure
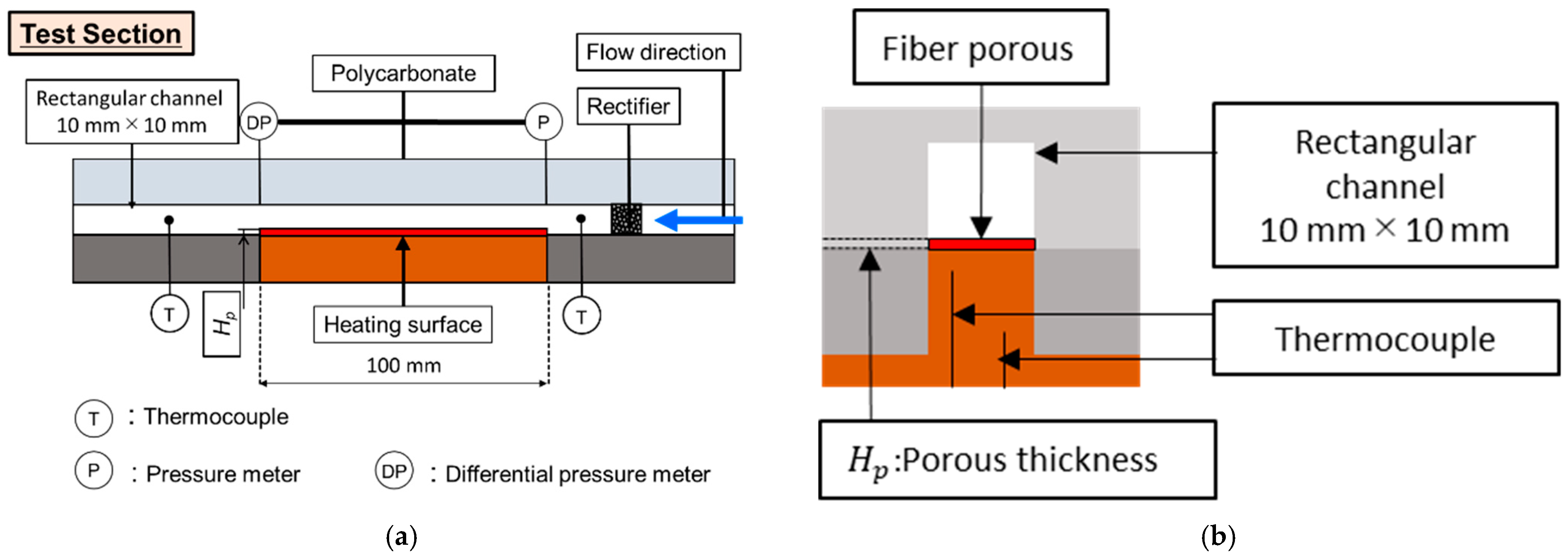
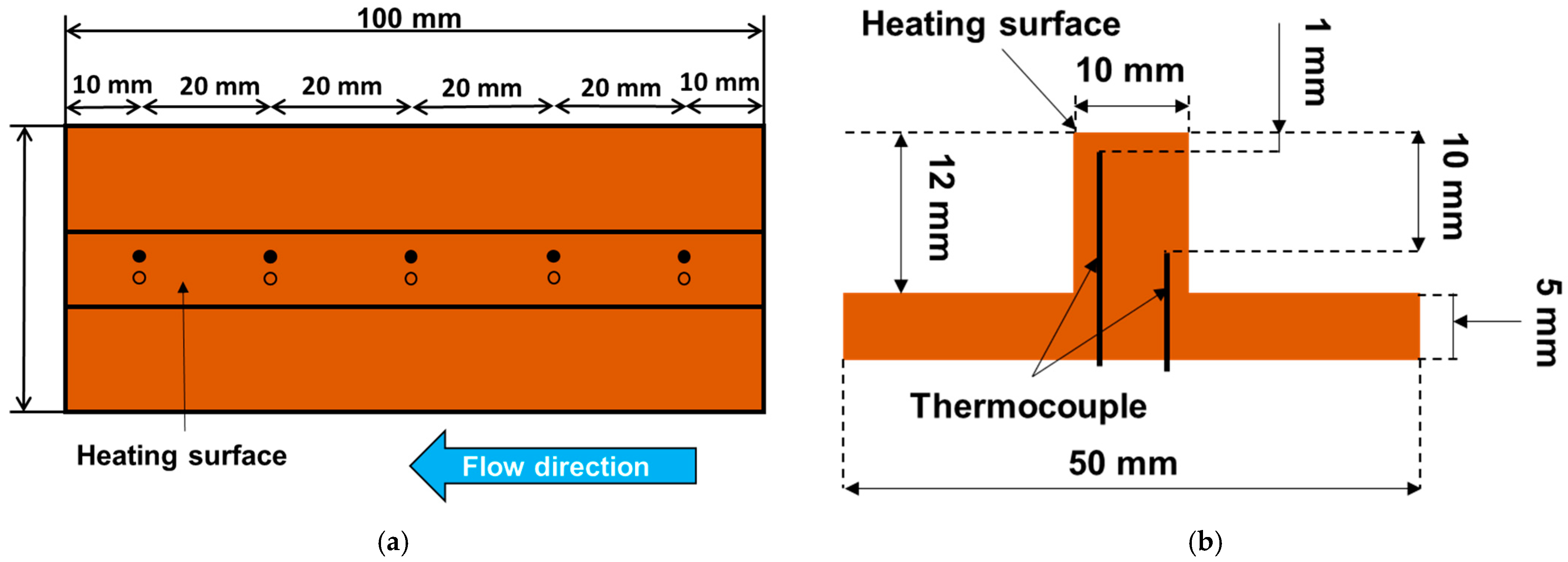
2.2. Test Section and Measurement of Thermal Physical Properties
2.3. High-Porosity Sintered Fiber
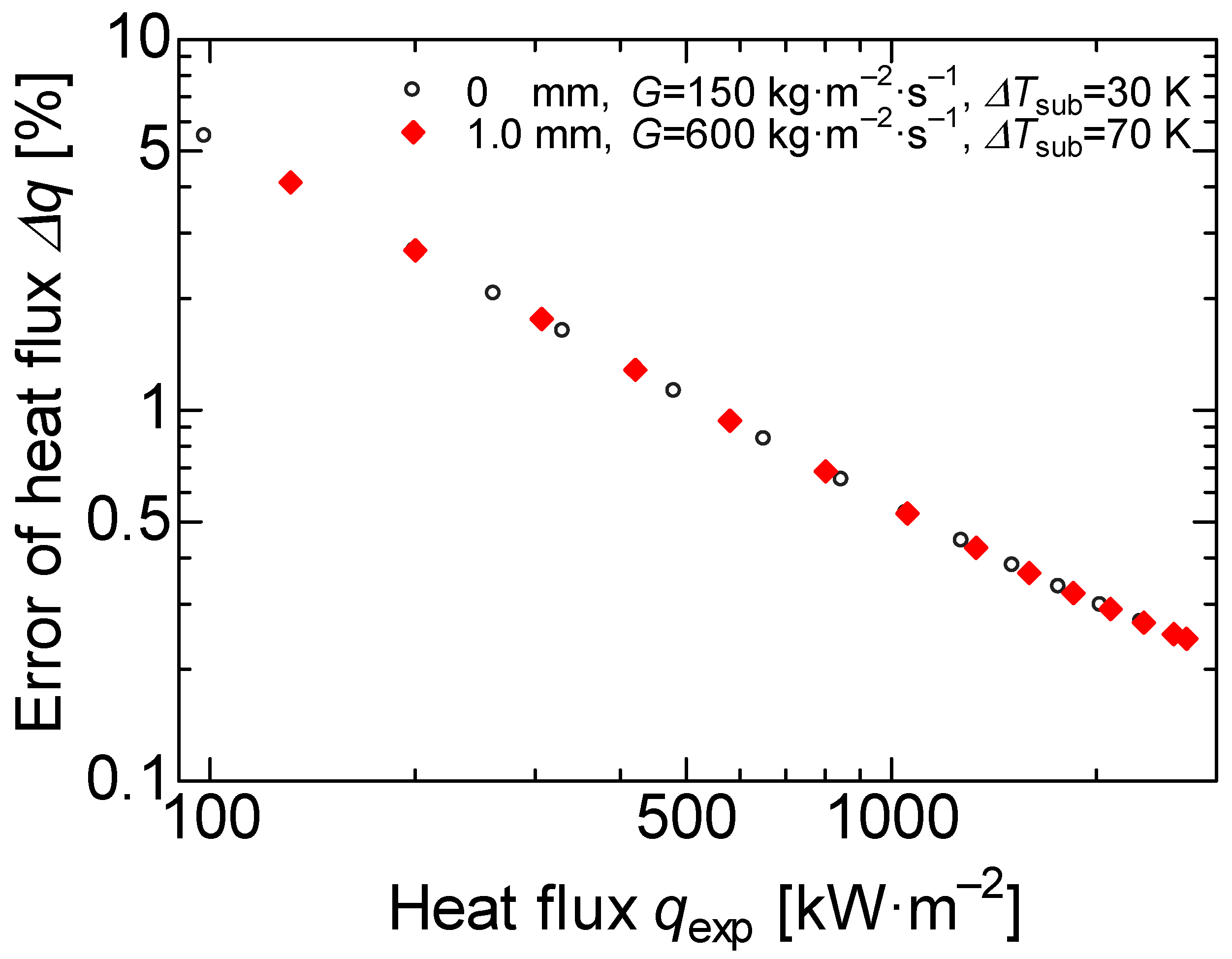
2.4. Uncertainly Analysis
3. Results and Discussion
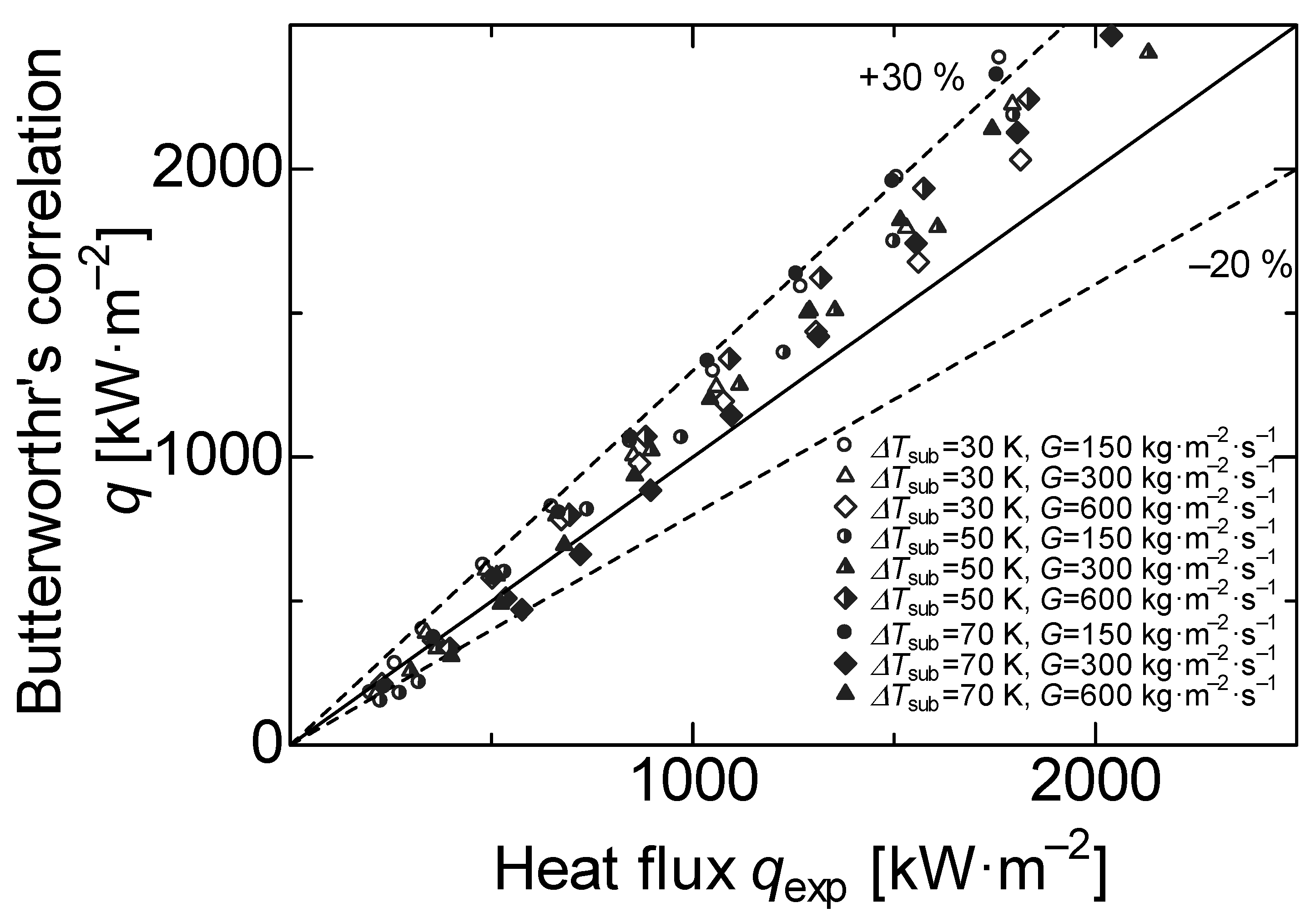
3.1. Comparison of Butterworth’s Correlation for the Bare Surface
3.2. Effect of High-Porosity Sintered Fibrous Metal
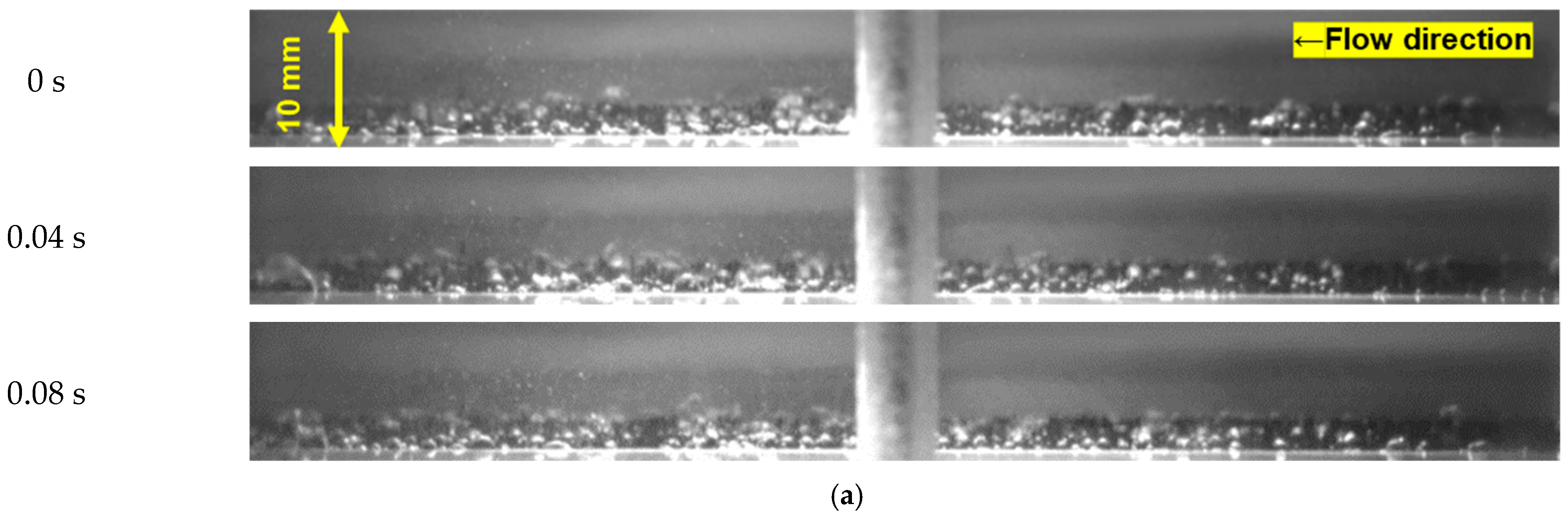
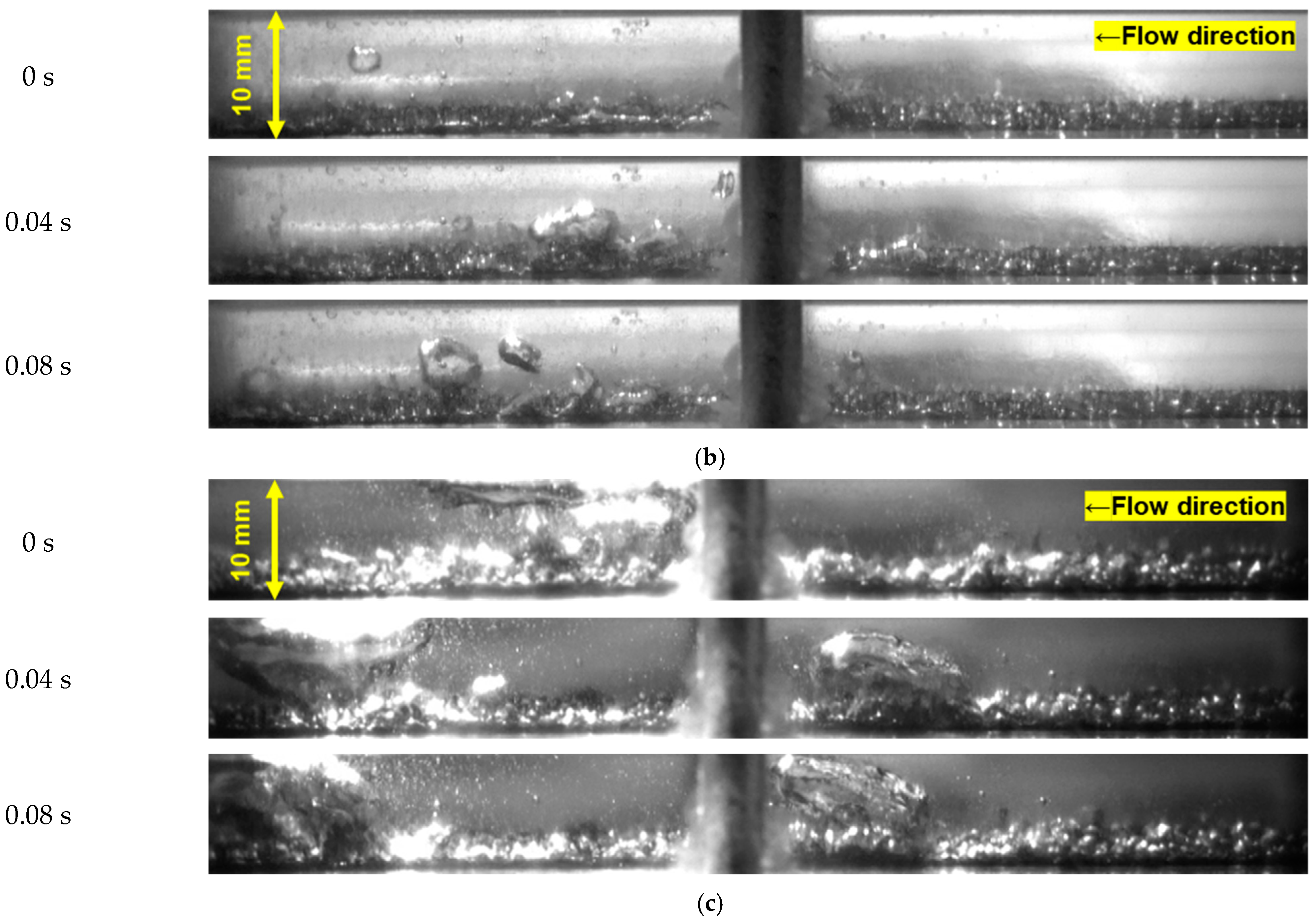
3.2.1. Effect of Porous Thickness and Boiling Curve
3.2.2. Effect of Mass Flux
3.3. Critical Heat Flux
4. Conclusions
- The effect of the porous surface on the heat flux was almost marginal on the single-phase region; however, after the onset nucleate boiling (ONB), the influence of the porous surface is important. At the same wall superheat (40 K), the heat flux on the bare surface was around 1600 kW·m−2; on the other hand, using the porous surface, the heat flux was around 2500 kW·m−2, which represents an enhancement of around 56%. At the same wall superheat (2000 kW·m−2), the wall superheat was around 48 K and around 36 K for the bare surface and the porous surface, respectively; in other words, a reduction of around 12 K on the wall superheat.
- At a higher mass flux and higher porous thickness, the heat flux was higher, and the wall superheat degree was lower. Thus, the ONB shifted to a higher wall heat flux. At the higher porous thickness, the bubble formation rate and departure rate increased.
- Using high sintered fiber as a passive method to promote bubble formation and departure increases the heat flux in comparison with the bare surface. The wall temperature degree variation was more stable using the porous surface. In addition, the wall superheat degree was reduced, avoiding burnout on the surface.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kandlikar, S.G. Heat Transfer Characteristics in Partial Boiling, Fully Developed Boiling, and Significant Void Flow Regions of Subcooled Flow Boiling. J. Heat Transf. 1998, 120, 395–401. [Google Scholar] [CrossRef]
- Chen, X.; Xia, X.; Sun, C.; Yan, X. Transient thermal analysis of the coupled radiative and convective heat transfer in a porous filled tube exchanger at high temperatures. J. Heat Transf. 2017, 108, 2472–2480. [Google Scholar] [CrossRef]
- Wang, L.; Khan, A.R.; Erkan, N.; Gong, H.; Okamoto, K. Critical heat flux enhancement on a downward face using porous honeycomb plate in saturated flow boiling. Int. J. Heat Mass Transf. 2017, 109, 454–461. [Google Scholar] [CrossRef]
- Deng, D.; Wan, W.; Shao, H.; Tang, Y.; Feng, J.; Zeng, J. Effects of operation parameters on flow boiling characteristics of heat sink cooling systems with reentrant porous microchannels. Energy Convers. Manag. 2015, 96, 340–351. [Google Scholar] [CrossRef]
- Dewangan, A.K.; Kumar, A.; Kumar, R. Nucleate boiling of pure and quasi-azeotropic refrigerants from copper coated surfaces. Appl. Eng. 2016, 94, 395–403. [Google Scholar] [CrossRef]
- Li, C.; Peterson, G.P. Evaporation/Boiling in Thin Capillary Wicks (II)—Effects of Volumetric Porosity and Mesh Size. J. Heat Transf. 2006, 128, 1320–1328. [Google Scholar] [CrossRef]
- Sarangi, S.; Weibel, J.A.; Garimella, S.V. Effect of particle size on surface-coating enhancement of pool boiling heat transfer. Int. J. Heat Mass Transf. 2015, 81, 103–113. [Google Scholar] [CrossRef] [Green Version]
- Sahu, R.P.; Sinha-Ray, S.; Sinha-Ray, S.; Yarin, A.L. Pool boiling of Novec 7300 and self-rewetting fluids on electrically-assisted supersonically solution-blown, copper-plated nanofibers. Int. J. Heat Mass Transf. 2016, 95, 83–93. [Google Scholar]
- Pialago, E.J.T.; Kwon, O.K.; Jin, J.S.; Park, C.W. Nucleate pool boiling of R134a on cold sprayed Cu-CNT SiC and Cu-CNT-AIN composite coatings. Appl. Therm. Eng. 2016, 103, 648–694. [Google Scholar]
- El-Genk, M.S. Nucleate Boiling Enhancements on Porous Graphite and Microporous and Macro–Finned Copper Surfaces. Heat Transf. Eng. 2012, 33, 175–204. [Google Scholar]
- Furberg, R.; Palm, B. Boiling heat transfer on a dendritic and micro-porous surface in R134a and FC-72. Appl. Eng. 2011, 31, 3595–3603. [Google Scholar] [CrossRef] [Green Version]
- Ali, A.F.; El-Genk, M.S. Effect of inclination on saturation boiling of PF-5060 dielectric liquid on 80- and 137-l thick copper micro-porous surfaces. Int. J. Therm. Sci. 2012, 53, 42–48. [Google Scholar] [CrossRef]
- Ujereh, S.; Fisher, T.; Mudawar, I. Effects of carbon nanotube arrays on nucleate pool boiling. Int. J. Heat Mass Transf. 2007, 50, 4023–4038. [Google Scholar] [CrossRef]
- Bian, H.; Kurwitz, C.; Sun, Z.; Cheng, K.; Chen, K. Enhanced nucleate boiling on 3D-printed micro-porous struc-tured surface. Appl. Therm. Eng. 2018, 141, 422–434. [Google Scholar] [CrossRef]
- Lin, L.; Kedzierski, M.A. Review of low-GWP refrigerant pool boiling heat transfer on enhanced surfaces. Int. J. Heat Mass Transf. 2019, 131, 1279–1303. [Google Scholar] [CrossRef]
- Qin, J.; Chen, Q.; Yang, C.; Huang, Y. Research process on property and application of metal porous materials. J. Alloy. Compd. 2016, 654, 39–44. [Google Scholar] [CrossRef]
- Manetti, L.L.; Moita, A.S.O.H.; De Souza, R.R.; Cardoso, E.M. Effect of copper foam thickness on pool boiling heat transfer of HFE-7100. Int. J. Heat Mass Transf. 2020, 152, 119547. [Google Scholar] [CrossRef]
- Calmidi, V.V.; Mahajan, R.L. Forced Convection in High Porosity Metal Foams. J. Heat Transf. 2000, 122, 557–565. [Google Scholar] [CrossRef]
- Mori, S.; Okuyama, K. Enhancement of the critical heat flux in saturated pool boiling using honeycomb porous media. Int. J. Multiph. Flow 2009, 35, 946–951. [Google Scholar] [CrossRef]
- Cooke, D.; Kandlikar, S.G. Effect of open microchannel geometry on pool boiling enhancement. Int. J. Heat Mass Transf. 2012, 55, 1004–1013. [Google Scholar] [CrossRef]
- Deng, D.; Wan, W.; Feng, J.; Huang, Q.; Qin, Y.; Xie, Y. Comparative experimental study on pool boiling performance of porous coating and solid structures with reentrant channels. Appl. Eng. 2016, 107, 420–430. [Google Scholar] [CrossRef]
- Liter, S.G.; Kaviany, M. Pool-boiling CHF enhancement by modulated porous-layer coating: Theory and experiment. Int. J. Heat Mass Transf. 2001, 44, 4287–4311. [Google Scholar] [CrossRef]
- Hao, W.; Wang, T.; Jiang, Y.; Guo, C.; Guo, C. Pool boiling heat transfer on deformable structures made of shape-memory-alloys. Int. J. Heat Mass Transf. 2017, 112, 236–247. [Google Scholar] [CrossRef]
- Jayaramu, P.; Gedupudi, S.; Das, S.K. Influence of heating surface characteristics on flow boiling in a copper microchannel: Experimental investigation and assessment of correlations. Int. J. Heat Mass Transf. 2019, 128, 290–318. [Google Scholar] [CrossRef]
- Steiner, H.; Kobor, A.; Gebhard, L. A wall heat transfer model for subcooled boiling flow. Int. J. Heat Mass Transf. 2005, 48, 4161–4173. [Google Scholar]
- Hua, S.; Huang, R.; Li, Z.; Zhou, P. Experimental study on the heat transfer characteristics of subcooled flow boiling with cast iron heating surface. Appl. Eng. 2015, 77, 180–191. [Google Scholar]
- Leong, K.C.; Ho, J.Y.; Wong, K.K. A critical review of pool and flow boiling heat transfer of dielectric fluids on enhancement surfaces. Appl. Therm. Eng. 2017, 112, 999–1019. [Google Scholar] [CrossRef]
- Lemmon, E.W.; Huber, M.L.; McLinden, M.O. NIST Standard Reference Database 23: Reference Fluid Thermodynamic and Transport Properties-REFPROP; Version 9.1; NIST Publications: Gaithersburg, MD, USA, 2013.
- Fang, X.; Yuan, Y.; Xu, A.; Tian, L.; Wu, Q. Review of correlations for subcooled flow boiling heat transfer and assessment of their applicability to water. Fusion Eng. Des. 2017, 122, 52–63. [Google Scholar] [CrossRef]
- Collier, J.G.; Thome, J.R. Convective Boiling and Condensation, 3rd ed.; Oxford University Press: Oxford, UK, 1994. [Google Scholar]
- Chen, J.C. Correlation for Boiling Heat Transfer to Saturated Fluids in Convective Flow. Ind. Eng. Chem. Process. Des. Dev. 1966, 5, 322–329. [Google Scholar]
- Butterworth, D. The Correlation of Cross Flow Pressure Data by Means of a Permeability Concept; Report AERE-R9435; UKAEA Atomic Energy Research Establishment: Didcot, UK, 1979.
- Forster, H.K.; Zuber, N. Dynamics of vapor bubbles and boiling heat transfer. AIChE J. 1955, 1, 531–535. [Google Scholar] [CrossRef]
- Vlachou, M.C.; Lioumbas, J.S.; David, K.; Chasapis, D.; Karapantsios, T. Effect of channel height and mass flux on highly subcooled horizontal flow boiling. Exp. Fluid Sci. 2017, 83, 157–168. [Google Scholar] [CrossRef]








| Mass Flux | [kg·m−2·s−1] | 150, 300, 600 |
| Inlet subcooled temperature | [K] | 30, 50, 70 |
| Porous thickness | [mm] | 0, 0.5, 1.0 |
| Parameter | Uncertainly |
|---|---|
| Mass flux | 0.2% |
| Thermocouples at copper block A | 0.05 K |
| Thermocouple at copper block B | 0.2 K |
| Depth of the measurement point at copper block | 0.02 mm |
| Pressure gage | 0.26 kPa |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Otomo, Y.; Santiago Galicia, E.; Enoki, K. Enhancement of Subcooled Flow Boiling Heat Transfer with High Porosity Sintered Fiber Metal. Appl. Sci. 2021, 11, 1237. https://doi.org/10.3390/app11031237
Otomo Y, Santiago Galicia E, Enoki K. Enhancement of Subcooled Flow Boiling Heat Transfer with High Porosity Sintered Fiber Metal. Applied Sciences. 2021; 11(3):1237. https://doi.org/10.3390/app11031237
Chicago/Turabian StyleOtomo, Yusuke, Edgar Santiago Galicia, and Koji Enoki. 2021. "Enhancement of Subcooled Flow Boiling Heat Transfer with High Porosity Sintered Fiber Metal" Applied Sciences 11, no. 3: 1237. https://doi.org/10.3390/app11031237
APA StyleOtomo, Y., Santiago Galicia, E., & Enoki, K. (2021). Enhancement of Subcooled Flow Boiling Heat Transfer with High Porosity Sintered Fiber Metal. Applied Sciences, 11(3), 1237. https://doi.org/10.3390/app11031237








