Optimal Design and Experimental Verification of Ultrasonic Cutting Horn for Ceramic Composite Material
Abstract
1. Introduction
2. Initial Design and Finite Element Analysis of an Ultrasonic Cutting Horn
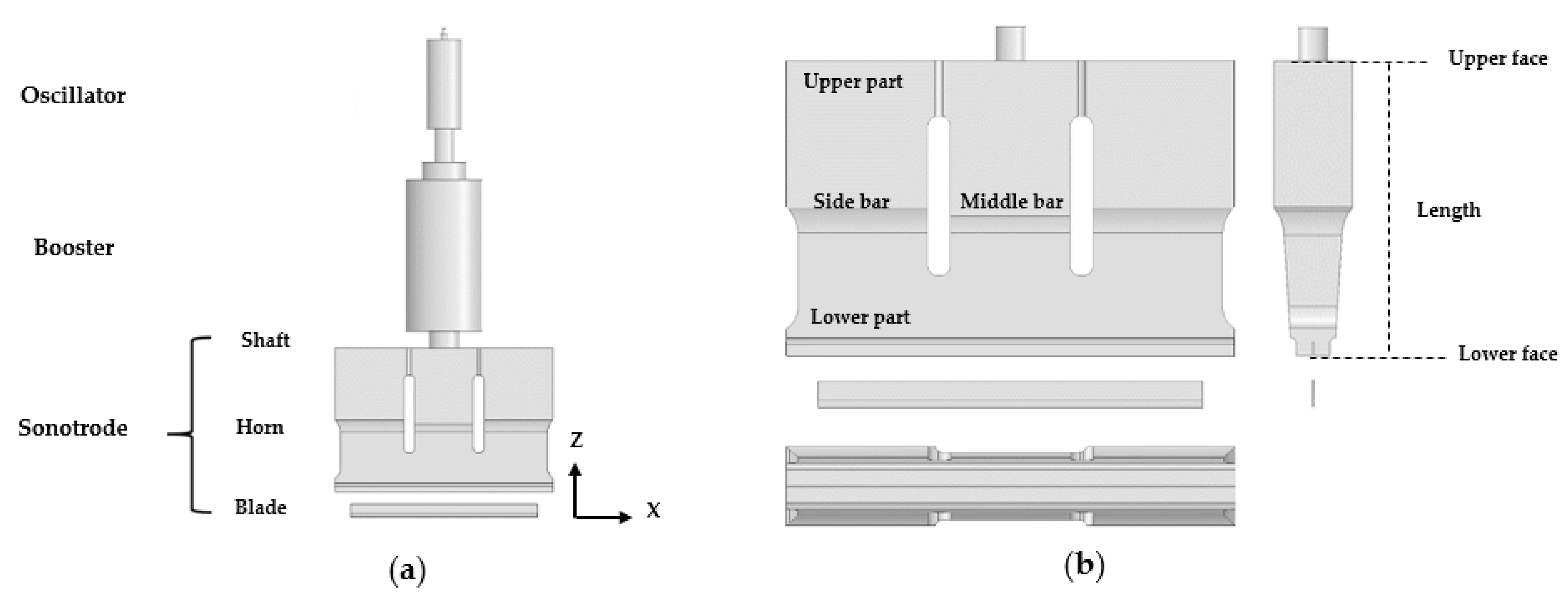
2.1. Conceptual Design of a Sonotrode
2.2. Finite Element Analysis
3. Optimization of the Developed Ultrasonic Cutting Horn
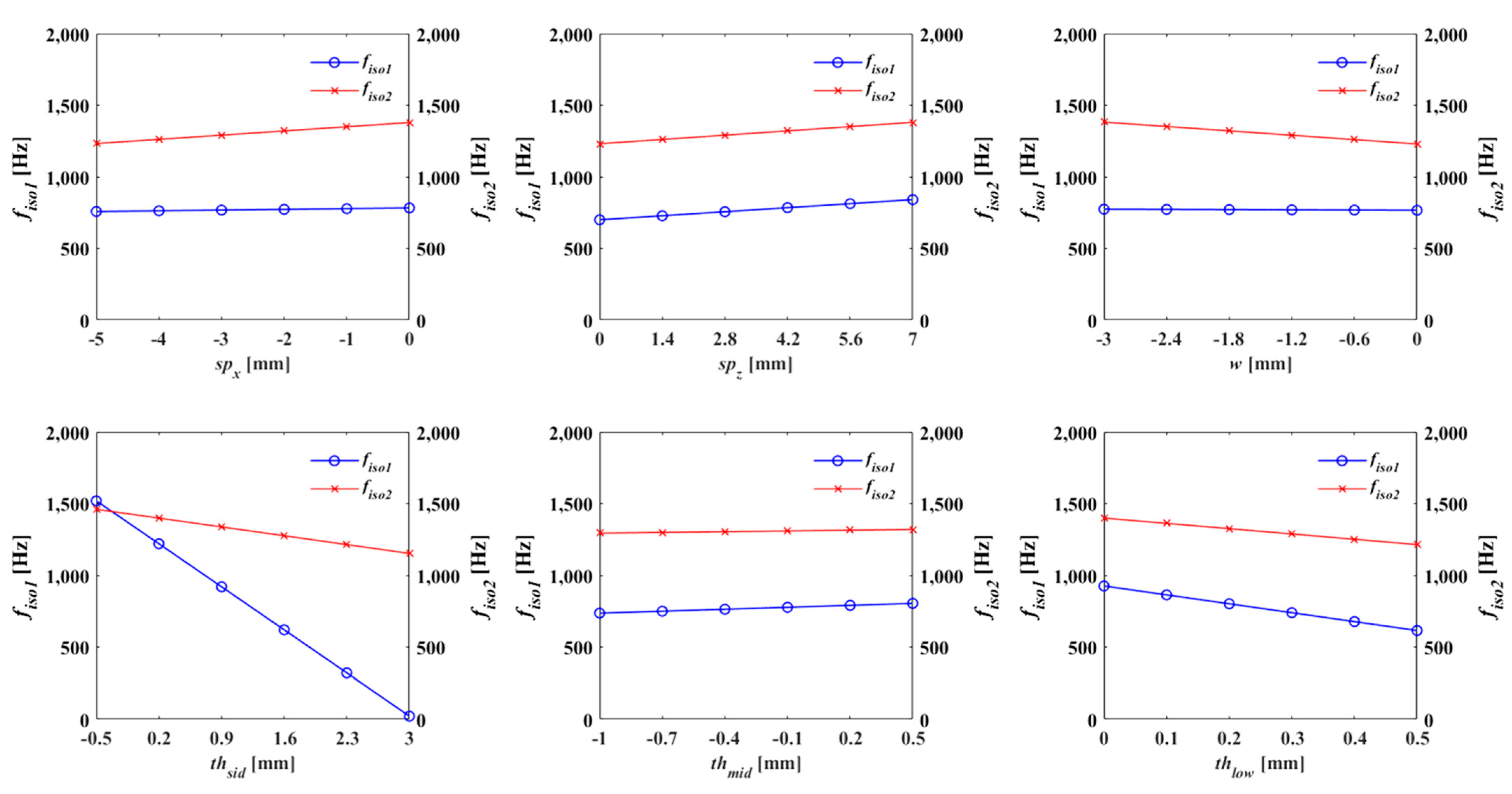
3.1. Sensitivity Analysis
3.2. Optimization
4. Fabrication and Experimental Verification
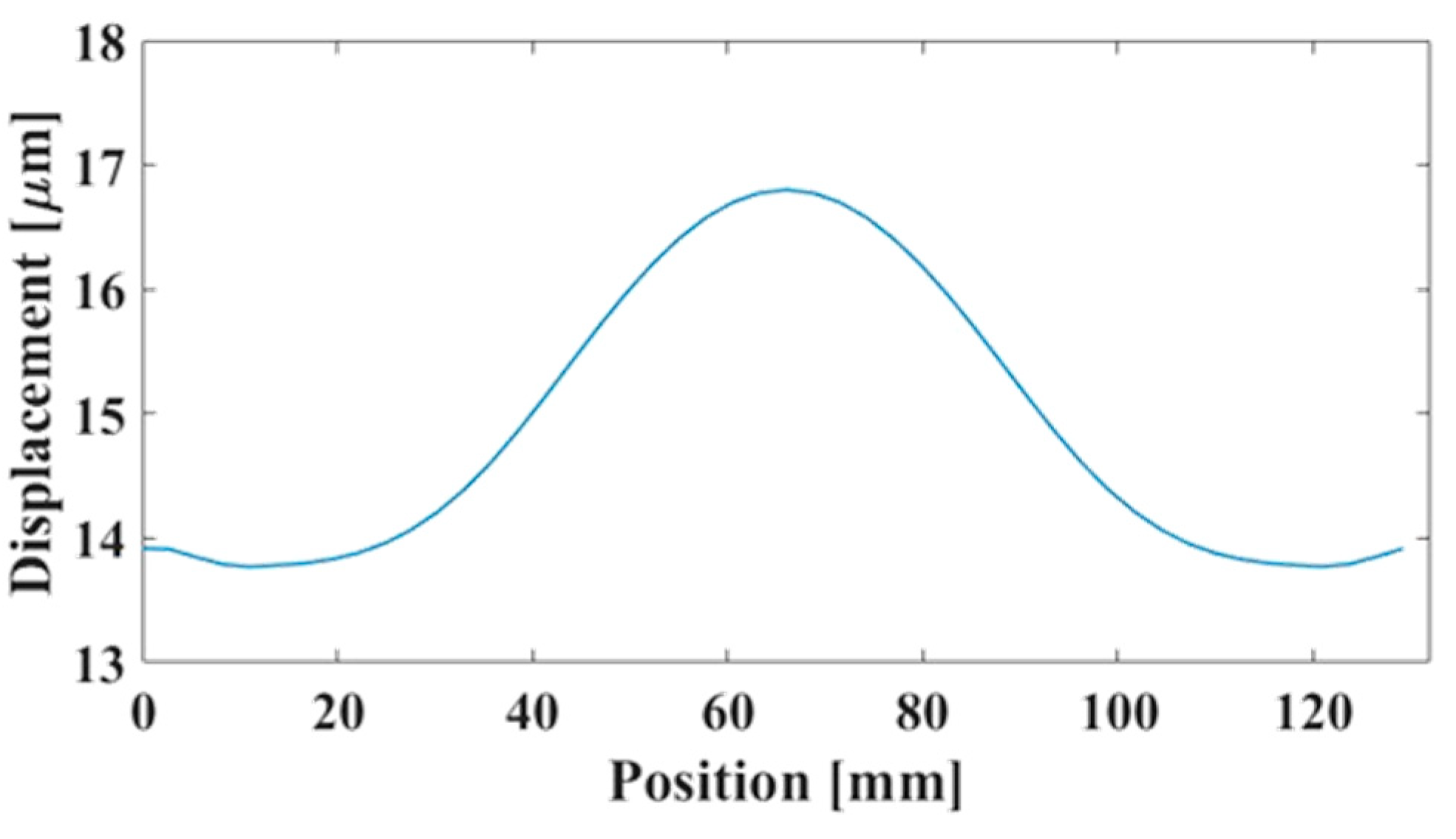
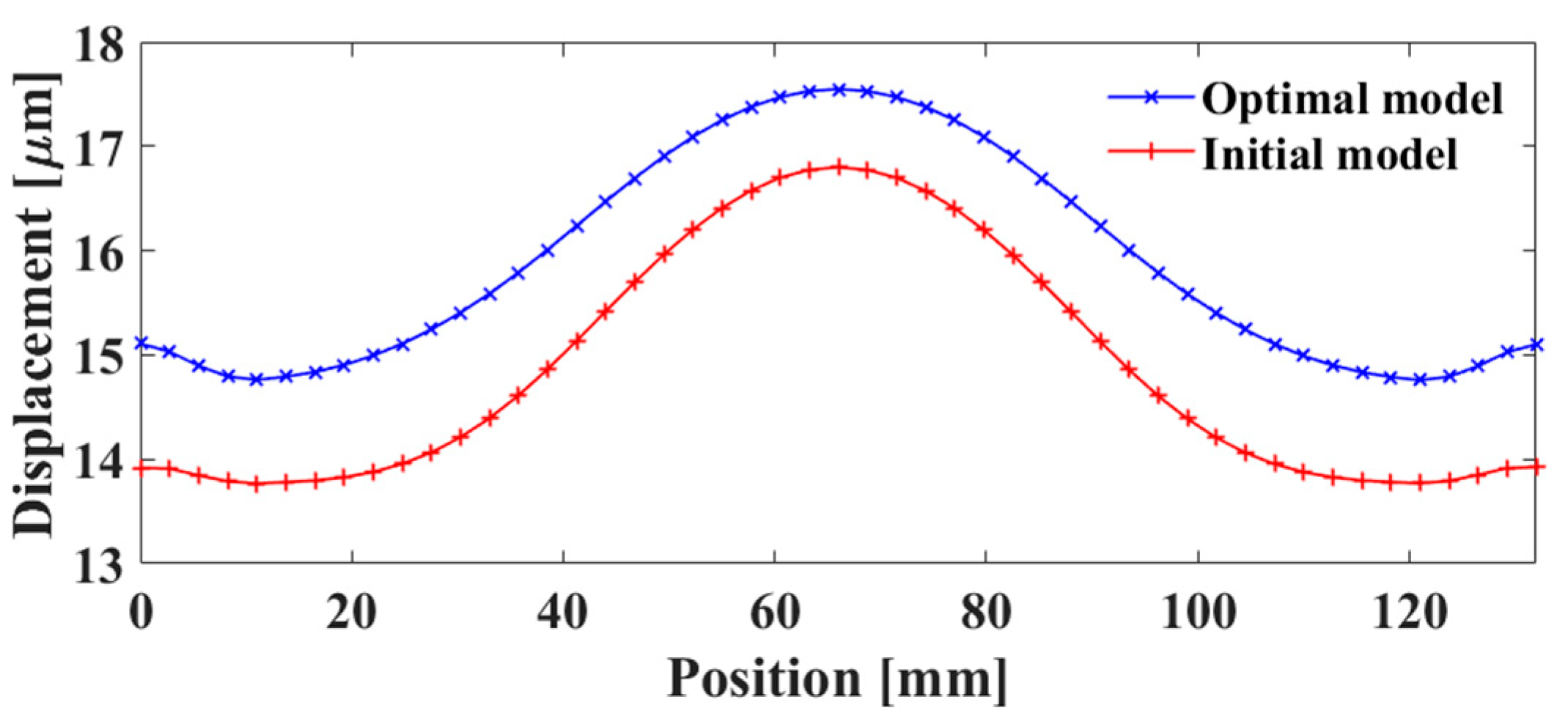
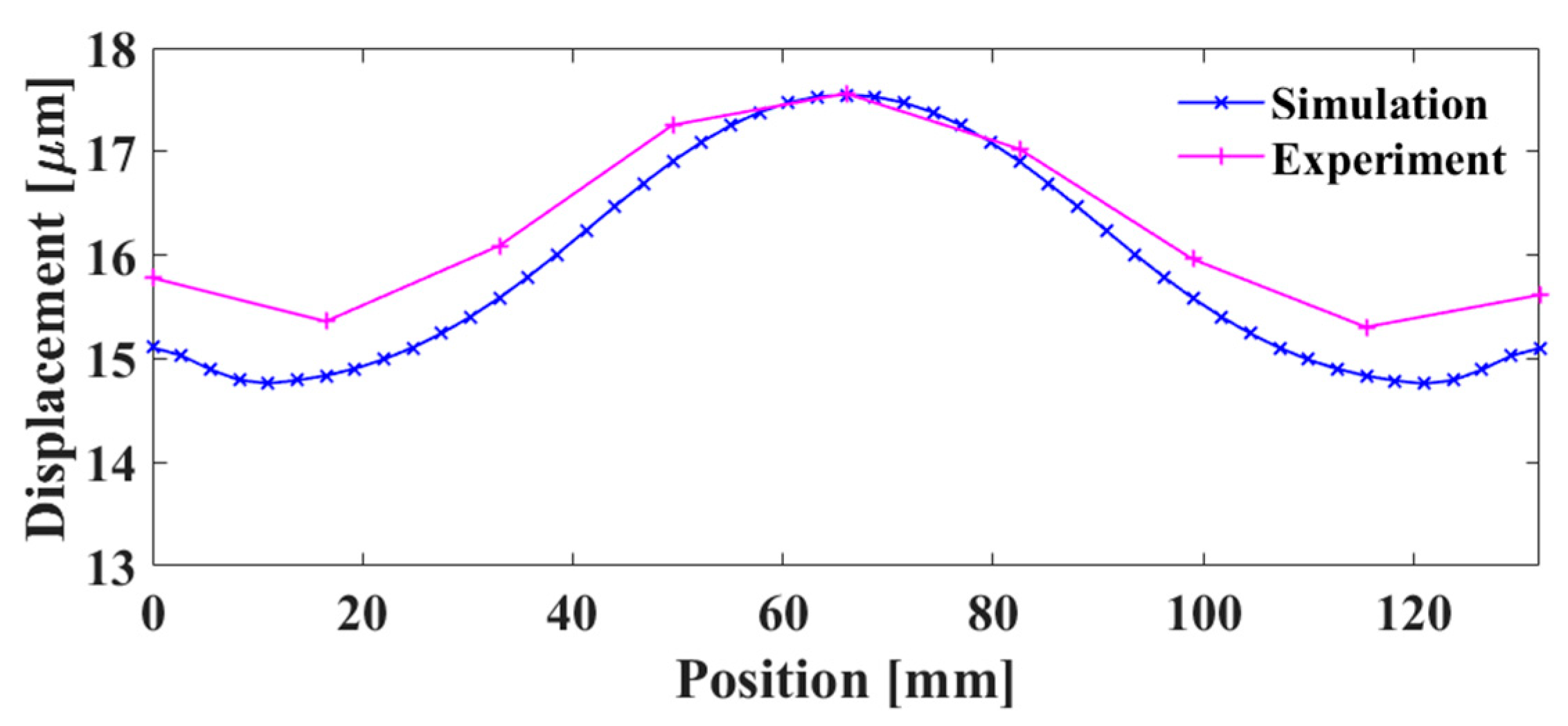
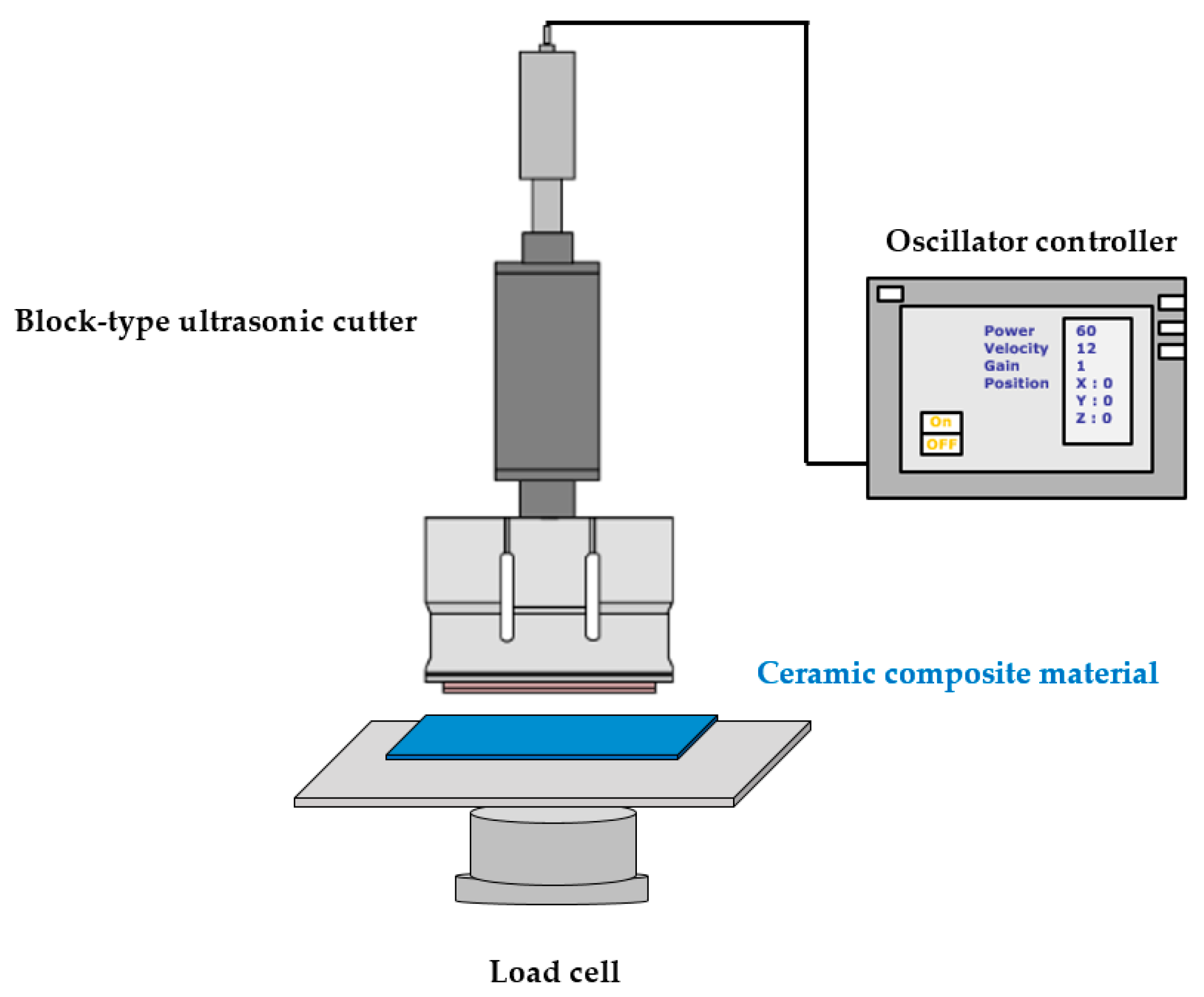
4.1. Displacement Measurement Experiment
4.2. Cutting Experiment
5. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Abdo, B.M.A.; El-Tamimi, A.; Nasr, E.A. Rotary ultrasonic machining of alumina ceramic: An experimental investigation of tool path and tool overlapping. Appl. Sci. 2020, 10, 1667. [Google Scholar] [CrossRef]
- Cao, W.; Zha, J.; Chen, Y. Cutting force prediction and experiment verification of paper honeycomb materials by ultrasonic vibration-assisted machining. Appl. Sci. 2020, 10, 4676. [Google Scholar] [CrossRef]
- Chu, N.-H.; Nguyen, V.-D.; Do, T.-V. Ultrasonic-assisted cutting: A beneficial application for temperature, torque reduction, and cutting ability improvement in deep drilling of Al-6061. Appl. Sci. 2018, 8, 1708. [Google Scholar] [CrossRef]
- Kim, T.-G.; Ahmad, S.F.; Yun, B.-J.; Kim, H.D. Cutting blade measurement of an ultrasonic cutting machine using multi-step detection. Appl. Sci. 2019, 9, 3338. [Google Scholar] [CrossRef]
- Mathieson, A.; Wallace, R.; Cleary, R.; Li, L.; Simpson, H.; Lucas, M. Ultrasonic needles for bone biopsy. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2017, 64, 433–440. [Google Scholar] [CrossRef]
- Harkness, P.; Lucas, M.; Cardoni, A. Maximization of the effective impulse delivered by a high-frequency/low- frequency planetary drill tool. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2011, 58, 2387–2396. [Google Scholar] [CrossRef] [PubMed]
- Kim, S.R.; Lee, J.H.; Yoo, C.D.; Song, J.-Y.; Lee, S.S. Design of highly uniform spool and bar horns for ultrasonic bonding. Ieee Trans. Ultrason. Ferroelectr. Freq. Control 2011, 58, 2194–2201. [Google Scholar] [PubMed]
- Hayashi, M.; Jin, M.; Thipprakmas, S.; Murakawa, M.; Hung, J.-C.; Tsai, Y.-C.; Hung, C.-H. Simulation of ultrasonic-vibration drawing using the finite element method (FEM). J. Mater. Process. Technol. 2003, 140, 30–35. [Google Scholar] [CrossRef]
- Sui, H.; Zhang, X.; Zhang, D.; Jiang, X.; Wu, R. Feasibility study of high-speed ultrasonic vibration cutting titanium alloy. J. Mater. Process. Technol. 2017, 247, 111–120. [Google Scholar] [CrossRef]
- Lucas, M.; Smith, A.C. Redesign of ultrasonic block horns for improved vibration performance. J. Vib. Acoust. 1997, 119, 410–414. [Google Scholar] [CrossRef]
- Zahn, S.; Schneider, Y.; Rohm, H. Ultrasonic cutting of foods: Effects of excitation magnitude and cutting velocity on the reduction of cutting work. Innov. Food Sci. Emerg. Technol. 2006, 7, 288–293. [Google Scholar] [CrossRef]
- Lucas, M.; MacBeath, A.; McCulloch, E.; Cardoni, A. A finite element model for ultrasonic cutting. Ultrasonics 2006, 44 (Suppl. 1), e503–e509. [Google Scholar] [CrossRef]
- Deibel, K.R.; Kaiser, F.; Zimmermann, R.; Meier, L.; Bolt, P.; Wegener, K. Longitudinal ultrasonic vibration assisted guillotining of stacked paper. Ultrasonics 2014, 54, 1585–1593. [Google Scholar] [CrossRef] [PubMed]
- Lucas, M.; Petzing, J.; Cardoni, A.; Smith, L.; McGeough, J. Design and characterisation of ultrasonic cutting tools. Cirp Ann. 2001, 50, 149–152. [Google Scholar] [CrossRef]
- Cardoni, A.; Lucas, M. Enhanced vibration performance of ultrasonic block horns. Ultrasonics 2002, 40, 365–369. [Google Scholar] [CrossRef]
- Nguyen, H.T.; Nguyen, H.D.; Uan, J.Y.; Wang, D.A. A nonrational B-spline profiled horn with high displacement amplification for ultrasonic welding. Ultrasonics 2014, 54, 2063–2071. [Google Scholar] [CrossRef]
- Roopa Rani, M.; Rudramoorthy, R. Computational modeling and experimental studies of the dynamic performance of ultrasonic horn profiles used in plastic welding. Ultrasonics 2013, 53, 763–772. [Google Scholar] [CrossRef] [PubMed]
- Amin, S.G.; Ahmed, M.H.M.; Youssef, H.A. Computer-aided design of acoustic horns for ultrasonic machining using finite-element analysis. J. Mater. Process. Technol. 1995, 55, 254–260. [Google Scholar] [CrossRef]
- Nad, M. Ultrasonic horn design for ultrasonic machining technologies. Appl. Comput. Mech. 2010, 4, 68–78. [Google Scholar]
- Jung, W.; Ra, J.; Park, K. Design optimization of ultrasonic horn for micro-pattern replication. Int. J. Precis. Eng. Manuf. 2012, 13, 2195–2201. [Google Scholar] [CrossRef]
- Choi, Y.-J.; Park, K.-H.; Hong, Y.-H.; Kim, K.-T.; Lee, S.-W.; Choi, H.-Z. Effect of ultrasonic vibration in grinding; horn design and experiment. Int. J. Precis. Eng. Manuf. 2013, 14, 1873–1879. [Google Scholar] [CrossRef]
- Wang, D.A.; Nguyen, H.D. A planar Bezier profiled horn for reducing penetration force in ultrasonic cutting. Ultrasonics 2014, 54, 375–384. [Google Scholar] [CrossRef]
- Wang, T.T.; Wu, J.; Ying, K.M.; Li, W.G.; Guo, H. An ultrasonic micro knife based on triangular silicon horn chip. Key Eng. Mater. 2015, 1363–1367. [Google Scholar] [CrossRef]
- Satpathy, M.P.; Sahoo, S.K. Experimental and numerical studies on ultrasonic welding of dissimilar metals. Int. J. Adv. Manuf. Technol. 2017, 93, 2531–2545. [Google Scholar] [CrossRef]
- Jagadish, R.A. Design and performance analysis of ultrasonic horn with a longitudinally changing rectangular cross section for USM using finite element analysis. J. Braz. Soc. Mech. Sci. Eng. 2018, 40, 359. [Google Scholar] [CrossRef]
- Wang, J.; Zhang, J.; Feng, P.; Guo, P. Experimental and theoretical investigation on critical cutting force in rotary ultrasonic drilling of brittle materials and composites. Int. J. Mech. Sci. 2018, 135, 555–564. [Google Scholar] [CrossRef]






| Component | Material | Young’s Modulus [GPa] | Density [kg/m3] | Poisson’s Ratio |
|---|---|---|---|---|
| Horn | Al7075 | 71.9 | 2802 | 0.34 |
| Blade | WC | 500 | 13,834 | 0.2 |
| Shaft | SCM435 | 205 | 6287 | 0.29 |
| Parameter Name | Symbol | Lower Boundary | Upper Boundary |
|---|---|---|---|
| x-axis position of slot [mm] | spx | 18.4 | 23.4 |
| z-axis position of slot [mm] | spz | 42.0 | 49.0 |
| Width [mm] | w | 61.5 | 64.5 |
| Thickness of side part [mm] | thsid | 21.5 | 25.0 |
| Thickness of middle part [mm] | thmid | 18.0 | 19.5 |
| Thickness of lower part [mm] | thlow | 16.0 | 16.5 |
| Parameter Name | Symbol | Initial Model | Optimal Model |
|---|---|---|---|
| x-axis position of slot [mm] | spx | 23.4 | 21.3 |
| z-axis position of slot [mm] | spz | 42.0 | 43.4 |
| Width [mm] | w | 64.5 | 63.5 |
| Thickness of side part [mm] | thsid | 22.0 | 22.7 |
| Thickness of middle part [mm] | thmid | 19.0 | 19.5 |
| Thickness of lower part [mm] | thlow | 16.0 | 16 |
| Parameter Name | Initial Model | Optimal Model | |
|---|---|---|---|
| Displacement [μm] | Maximum | 16.80 | 17.55 |
| Minimum | 13.76 | 14.76 | |
| Axial mode frequency [Hz] | 28,520 | 28,478 | |
| One lower mode frequency [Hz] | 26,932 | 27,289 | |
| One higher mode frequency [Hz] | 29,366 | 29,659 | |
| 1st frequency isolation [Hz] | 1588 | 1189 | |
| 2nd frequency isolation [Hz] | 846 | 1181 | |
| Displacement [μm] | Axial Mode Frequency [Hz] | ||
|---|---|---|---|
| Maximum | Minimum | ||
| Simulation | 17.55 | 14.76 | 28,478 |
| Experiment | 17.56 | 15.30 | 28,480 |
| Difference [%] | 0.06 | 3.52 | 0.01 |
| Test # | Cutting Force [N] | |
|---|---|---|
| Ultrasonic Cutting | Conventional Cutting | |
| 1 | 76.3 | 235.6 |
| 2 | 77.9 | 240.1 |
| 3 | 82.4 | 228.9 |
| 4 | 75.5 | 232.5 |
| 5 | 76.9 | 234.8 |
| 6 | 78.4 | 239.4 |
| 7 | 80.4 | 229.8 |
| 8 | 81.2 | 234.5 |
| 9 | 76.8 | 231.2 |
| 10 | 78.8 | 233.0 |
| average | 78.5 | 234.0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hahn, M.; Cho, Y.; Jang, G.; Kim, B. Optimal Design and Experimental Verification of Ultrasonic Cutting Horn for Ceramic Composite Material. Appl. Sci. 2021, 11, 1954. https://doi.org/10.3390/app11041954
Hahn M, Cho Y, Jang G, Kim B. Optimal Design and Experimental Verification of Ultrasonic Cutting Horn for Ceramic Composite Material. Applied Sciences. 2021; 11(4):1954. https://doi.org/10.3390/app11041954
Chicago/Turabian StyleHahn, Mibbeum, Yeungjung Cho, Gunhee Jang, and Bumcho Kim. 2021. "Optimal Design and Experimental Verification of Ultrasonic Cutting Horn for Ceramic Composite Material" Applied Sciences 11, no. 4: 1954. https://doi.org/10.3390/app11041954
APA StyleHahn, M., Cho, Y., Jang, G., & Kim, B. (2021). Optimal Design and Experimental Verification of Ultrasonic Cutting Horn for Ceramic Composite Material. Applied Sciences, 11(4), 1954. https://doi.org/10.3390/app11041954







