ILRS Reference Point Determination Using Close Range Photogrammetry
Abstract
:1. Introduction
2. Mathematical Background
2.1. Bundle Adjustment
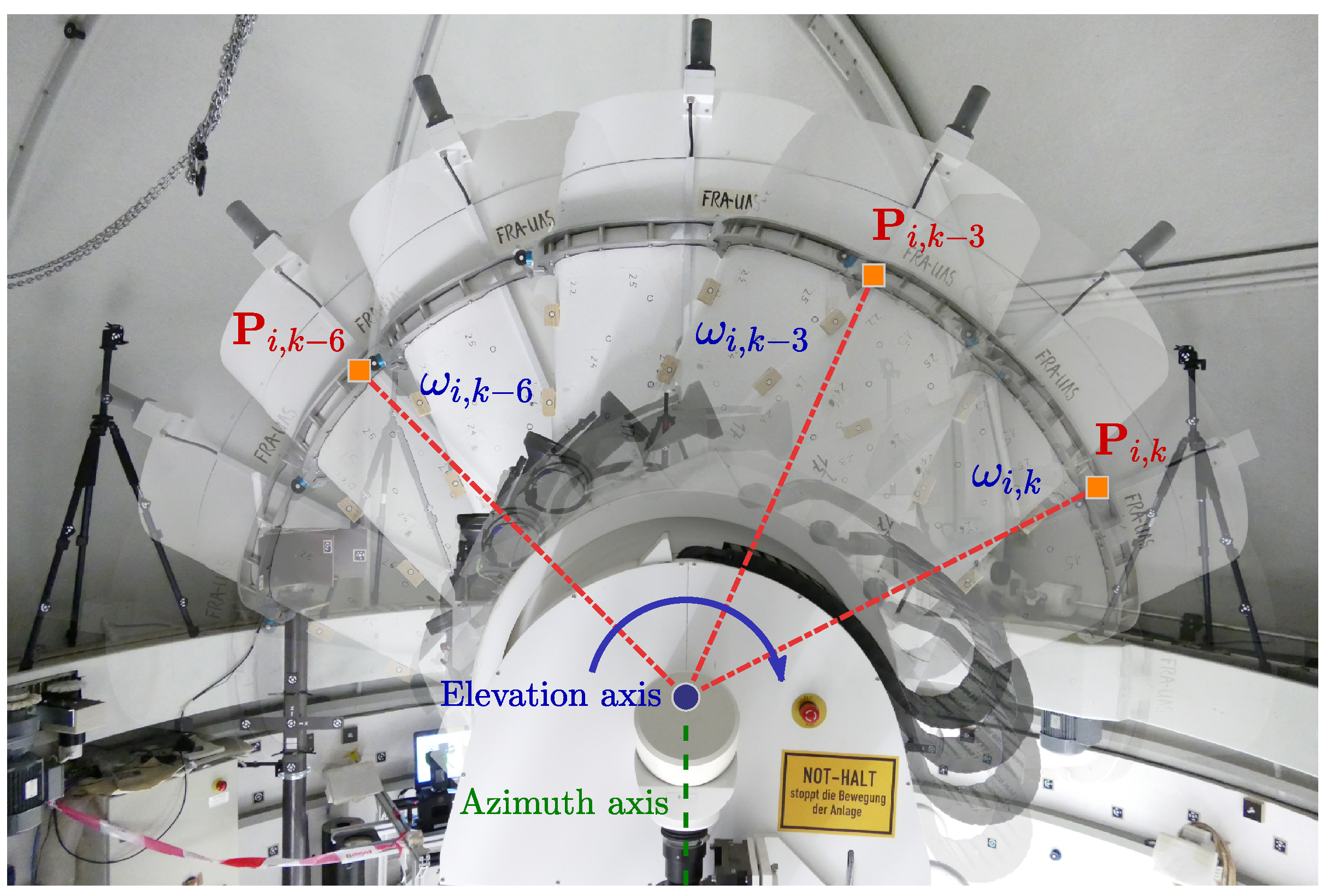
2.2. Reference Point Determination
2.3. Spherical Simplex Unscented Transformation
- 1.
- Choose the weights as:
- 2.
- Set the vector sequence up according to:
- 3.
- Expand the vector sequence recursively for by:
- 4.
- Estimate the -points for as:
3. Satellite Observing System Wettzell
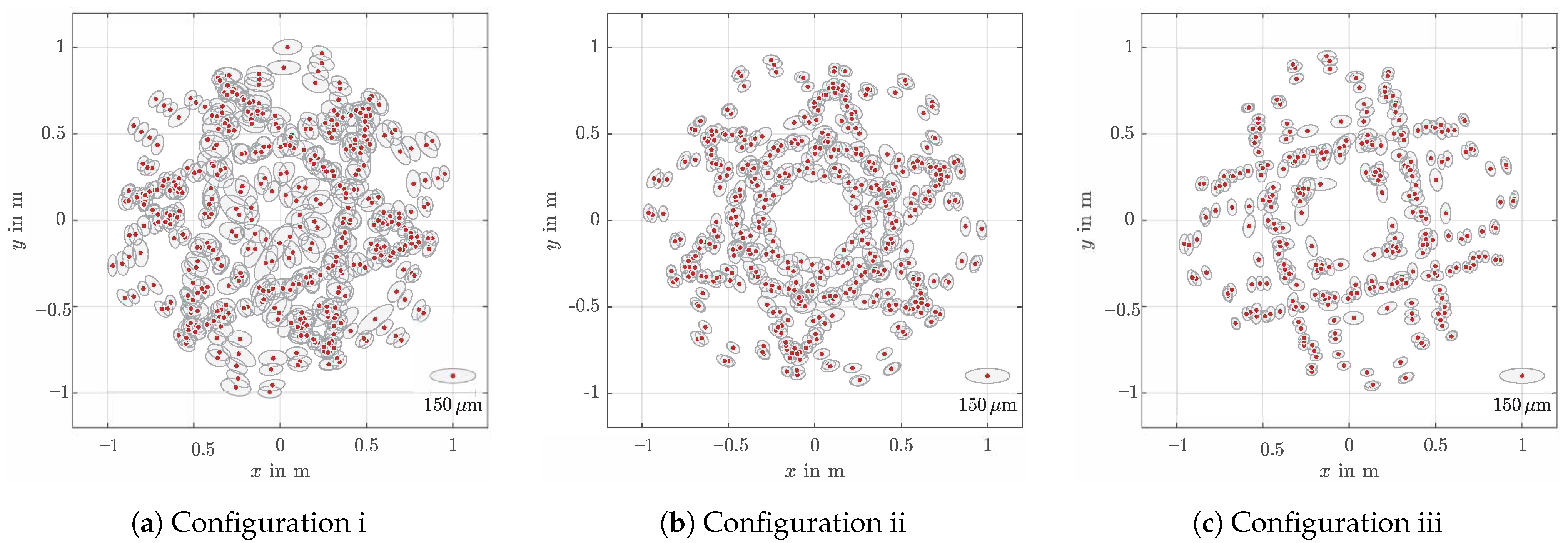
3.1. Measurements and Configurations
3.2. Analysis and Results
3.2.1. Bundle Adjustment
3.2.2. Reference Point Determination
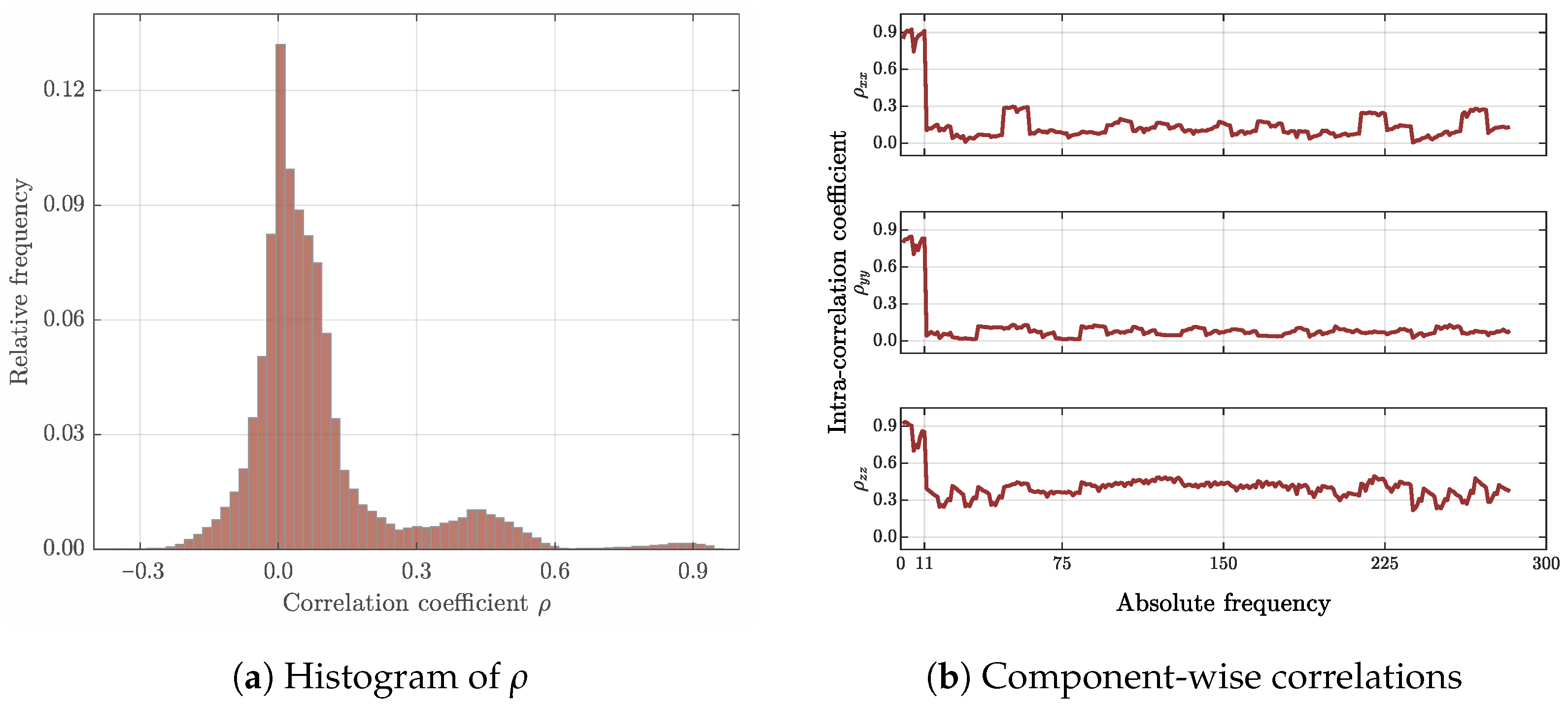
3.2.3. Bias of the Estimates
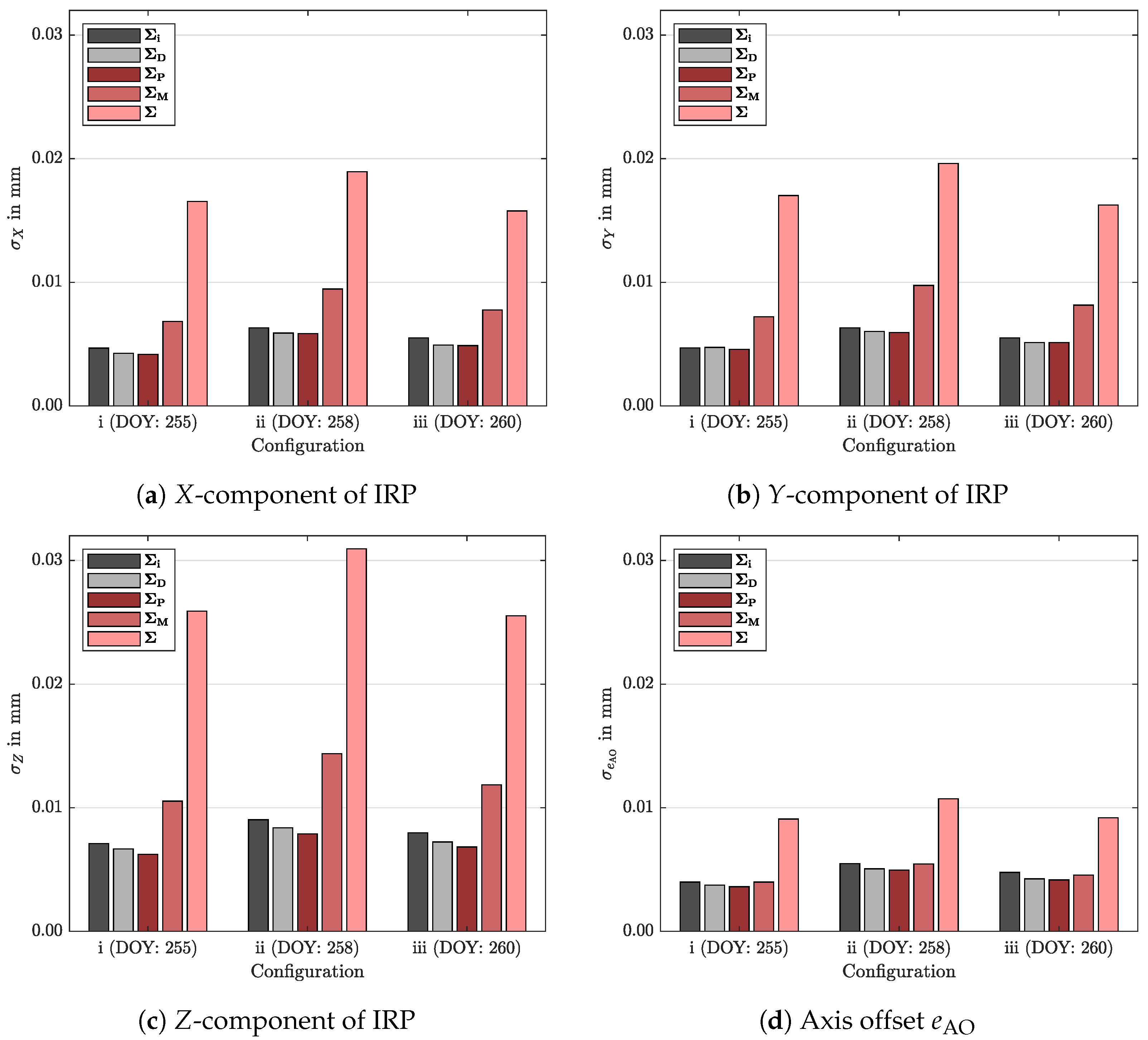
3.2.4. Impact of the Stochastic Model
- 1.
- The most simplified stochastic model readswhich disregards all prior results derived by the bundle adjustment, i.e., the variances of the points and the dependencies between the points are neglected. Such a model is usually applied in curve and surface analyses [59,60]. Here, matrix is the identity matrix, and is the variance of the unit weight.
- 2.
- Using the variances given in yields the most common approach [61], because commercial software packages often provide the standard deviations , , and of the estimated object points. Having n points, this stochastic model readsLike before, all correlations are neglected.
- 3.
- Especially in laser scanning applications [61,62], a block-diagonal matrixis frequently used to define the stochastic model. The number of block matrices is equal to the number of observed points n, and the matrix is the ith symmetric block matrix that corresponds to the sub-matrix of the ith observed position in , i.e.,Here, , , and are the covariances between the coordinate components. Thus, correlations between the coordinate components of the point are taken into account, but correlations between the observed positions are still neglected.
- 4.
- The stochastic model, which takes the correlations between the observed positions realized by a single marker into account, is given bywhereis the symmetric block matrix of the lth mounted marker . The number of block matrices is equal to the number of mounted markers m. The resulting block-diagonal matrix neglects correlations between the positions obtained by different markers.
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| DN | Drift nest |
| DORIS | Doppler orbitography and radiopositioning integrated by satellite |
| GGOS | Global geodetic observing system |
| GGRF | Global geodetic reference frame |
| GGRS | Global geodetic reference system |
| GMM | Gauß-Markov model |
| GNSS | Global navigation satellite system |
| GOW | Geodetic Observatory Wettzell |
| ILRS | International Laser Ranging Service |
| IRP | Invariant reference point |
| ITRF | International Terrestrial Reference Frame |
| MCS | Monte Carlo simulation |
| MPE | Maximum permissible error |
| MSSUT | Minimal skew simplex unscented transformation |
| NNR | No-net-rotation |
| NNS | No-net-scale |
| NNT | No-net-translation |
| UT | Unscented transformation |
| SLR | Satellite laser ranging |
| SOS-W | Satellite Observing System Wettzell |
| SSUT | Spherical simplex unscented transformation |
| VLBI | Very long baseline interferometry |
References
- Gross, R.; Beutler, G.; Plag, H.P. Integrated scientific and societal user requirements and functional specifications for the GGOS. In Global Geodetic Observing System—Meeting the Requirements of a Global Society on a Changing Planet in 2020; Plag, H.P., Pearlman, M., Eds.; Springer: Berlin/Heidelberg, Germany, 2009; pp. 209–224. [Google Scholar] [CrossRef]
- Blewitt, G.; Altamimi, Z.; Davis, J.; Gross, R.; Kuo, C.Y.; Lemoine, F.G.; Moore, A.W.; Neilan, R.E.; Plag, H.P.; Rothacher, M.; et al. Geodetic Observations and Global Reference Frame Contributions to Understanding Sea-Level Rise and Variability. In Understanding Sea-Level Rise and Variability; Church, J.A., Woodworth, P.L., Aarup, T., Wilson, W.S., Eds.; Wiley-Blackwell: Hoboken, NJ, USA, 2010; pp. 256–284. [Google Scholar] [CrossRef] [Green Version]
- Altamimi, Z.; Collilieux, X.; Boucher, C. Accuracy Assessment of the ITRF Datum Definition. In VI Hotine-Marussi Symposium on Theoretical and Computational Geodesy; Xu, P., Liu, J., Dermanis, A., Eds.; Springer: Berlin/Heidelberg, Germany, 2008; Volume 132, pp. 101–110. [Google Scholar] [CrossRef]
- Plag, H.P.; Altamimi, Z.; Bettadpur, S.; Beutler, G.; Beyerle, G.; Cazenave, A.; Crossley, D.; Donnellan, A.; Forsberg, R.; Gross, R.; et al. The goals, achievements, and tools of modern geodesy. In Global Geodetic Observing System—Meeting the Requirements of a Global Society on a Changing Planet in 2020; Plag, H.P., Pearlman, M., Eds.; Springer: Berlin/Heidelberg, Germany, 2009; pp. 15–88. [Google Scholar] [CrossRef]
- Altamimi, Z.; Rebischung, P.; Métivier, L.; Collilieux, X. ITRF2014: A new release of the International Terrestrial Reference Frame modeling nonlinear station motions. J. Geophys. Res. Solid Earth 2016, 121, 6109–6131. [Google Scholar] [CrossRef] [Green Version]
- Seitz, M.; Angermann, D.; Bloßfeld, M.; Drewes, H.; Gerstl, M. The 2008 DGFI realization of the ITRS: DTRF2008. J. Geod. 2012, 86, 1097–1123. [Google Scholar] [CrossRef]
- Schuh, H.; Wickert, J.; Sips, M.; Schöne, T.; Rogaß, C.; Roessner, S.; König, R.; Klemann, V.; Heinkelmann, R.; Dobslaw, H.; et al. Zukunft der globalen Geodäsie und Fernerkundung aus Sicht des Deutschen GeoForschungsZentrum (GFZ), Potsdam. In Handbuch der Geodäsie—Erdmessung und Satellitengeodäsie; Rummel, R., Ed.; Springer Reference Naturwissenschaften; Springer: Berlin/Heidelberg, Germany, 2017; pp. 443–497. [Google Scholar] [CrossRef]
- Glaser, S.; Fritsche, M.; Sośnica, K.; Rodríguez-Solano, C.J.; Wang, K.; Dach, R.; Hugentobler, U.; Rothacher, M.; Dietrich, R. Validation of Components of Local Ties. In REFAG 2014; van Dam, T., Ed.; Springer International Publishing: Cham, Switzerland, 2015; Volume 146, pp. 21–28. [Google Scholar] [CrossRef] [Green Version]
- Altamimi, Z.; Rebischung, P.; Métivier, L.; Collilieux, X. Analysis and Results of ITRF2014; IERS Technical Note 38, International Earth Rotation and Reference Systems Service (IERS); Verlag des Bundesamts für Kartographie und Geodäsie: Frankfurt am Main, Germany, 2017. [Google Scholar]
- GeoMetre. Large-Scale Dimensional Measurements for Geodesy—A Joint Research Project within the European Metrology Research Programme EMPIR; European Commission (EC), Grant Number: 18SIB01; EURAMET e.V.: Brunswick, Germany, 2020. [Google Scholar]
- García-Asenjo, L.; Baselga, S.; Atkins, C.; Garrigues, P. Development of a Submillimetric GNSS-Based Distance Meter for Length Metrology. Sensors 2021, 21, 1145. [Google Scholar] [CrossRef] [PubMed]
- Dawson, J.; Sarti, P.; Johnston, G.M.; Vittuari, L. Indirect approach to invariant point determination for SLR and VLBI systems: An assessment. J. Geod. 2006, 81, 433–441. [Google Scholar] [CrossRef]
- Kenefic, J.F. Ultra-Precise Analytics. Photogramm. Eng. 1971, 37, 1167–1187. [Google Scholar]
- Kim, H.; Yun, H.; Hwang, J.; Hong, S. A Static Displacement Monitoring System for VLBI Antenna Using Close-Range Photogrammetry. Appl. Sci. 2017, 7, 1125. [Google Scholar] [CrossRef] [Green Version]
- Lösler, M.; Haas, R.; Eschelbach, C.; Greiwe, A. Gravitational Deformation of Ring-Focus Antennas for VGOS–First Investigations at the Onsala Twin Telescopes Project. J. Geod. 2019, 93, 2069–2087. [Google Scholar] [CrossRef] [Green Version]
- Luhmann, T.; Robson, S.; Kyle, S.; Boehm, J. Close-Range Photogrammetry and 3D Imaging, 3rd ed.; Walter de Gruyter GmbH: Berlin, Germany, 2019. [Google Scholar] [CrossRef]
- Förstner, W.; Wrobel, B.P. Photogrammetric Computer Vision—Statistics, Geometry, Orientation and Reconstruction, 1st ed.; Geometry and Computing 11; Springer: Cham, Switzerland, 2016. [Google Scholar] [CrossRef] [Green Version]
- Brown, D.C. Decentering Distortion of Lenses. Photogramm. Eng. 1966, 32, 444–462. [Google Scholar]
- Brown, D.C. Close-Range Camera Calibration. Photogramm. Eng. 1971, 37, 855–866. [Google Scholar]
- Dai, F.; Feng, Y.; Hough, R. Photogrammetric error sources and impacts on modeling and surveying in construction engineering applications. Vis. Eng. 2014, 2, 2:1–2:14. [Google Scholar] [CrossRef] [Green Version]
- Brown, D.C. The Bundle Adjustment–Progress and Prospects. Int. Arch. Photogramm. 1976, 21, 1–33. [Google Scholar]
- Papo, H.B. Free Net Analysis in Close-Range Photogrammetry. Photogramm. Eng. Remote Sens. 1982, 48, 571–576. [Google Scholar]
- Pearlman, M.R.; Degnan, J.J.; Bosworth, J.M. The International Laser Ranging Service. Adv. Space Res. 2002, 30, 135–143. [Google Scholar] [CrossRef]
- Seitz, M. Kombination geodätischer Raumbeobachtungsverfahren zur Realisierung eines terrestrischen Referenzsystems. Ph.D. Thesis, Deutsche Geodätische Kommission, München, Germany, 2009. [Google Scholar]
- Lösler, M. New Mathematical Model for Reference Point Determination of an Azimuth-Elevation Type Radio Telescope. J. Surv. Eng. 2009, 135, 131–135. [Google Scholar] [CrossRef]
- Lösler, M.; Eschelbach, C.; Riepl, S. A Modified Approach for Automated Reference Point Determination of SLR and VLBI Telescopes. Tech. Mess. 2018, 85, 616–626. [Google Scholar] [CrossRef]
- Lösler, M.; Eschelbach, C.; Riepl, S.; Schüler, T. Zur Bestimmung des ILRS-Referenzpunktes am Satellite Observing System Wettzell. In Photogrammetrie-Laserscanning-Optische 3D-Messtechnik: Beiträge der 18. Oldenburger 3D-Tage 2019; Luhmann, T., Schumacher, C., Eds.; Wichmann Verlag: Oldenburg, Germany, 2019; pp. 162–175. [Google Scholar] [CrossRef]
- Box, M.J. Bias in Nonlinear Estimation. J. R. Stat. Soc. Ser. B 1971, 33, 171–201. [Google Scholar] [CrossRef]
- Teunissen, P.J.G. First and second moments of non-linear least-squares estimators. Bull. Géodésique 1989, 63, 253–262. [Google Scholar] [CrossRef] [Green Version]
- Lösler, M.; Lehmann, R.; Neitzel, F.; Eschelbach, C. Bias in Least-Squares Adjustment of Implicit Functional Models. Surv. Rev. 2020, 1–12. [Google Scholar] [CrossRef]
- Julier, S.J.; Uhlmann, J.; Durrant-Whyte, H.F. A new method for the nonlinear transformation of means and covariances in filters and estimators. IEEE Trans. Autom. Control 2000, 45, 477–482. [Google Scholar] [CrossRef] [Green Version]
- Carlton, M.A.; Devore, J.L. Probability with Applications in Engineering, Science, and Technology, 2nd ed.; Springer Texts in Statistics; Springer International Publishing: Cham, Switzerland, 2017. [Google Scholar] [CrossRef]
- Julier, S.J.; Uhlmann, J.K. A Consistent, Debiased Method for Converting Between Polar and Cartesian Coordinate Systems. In Proceedings of the AeroSense: The 11th International Symposium on Aerospace/Defense Sensing, Simulation and Controls, Orlando, FL, USA, 21–25 April 1997; pp. 110–121. [Google Scholar] [CrossRef] [Green Version]
- Julier, S.J. The scaled unscented transformation. In Proceedings of the 2002 American Control Conference (IEEE Cat. No.CH37301), Anchorage, AK, USA, 8–10 May 2002; Volume 6, pp. 4555–4559. [Google Scholar] [CrossRef] [Green Version]
- Julier, S.J.; Uhlmann, J.K.; Durrant-Whyte, H.F. A new approach for filtering nonlinear systems. Proceedings of 1995 American Control Conference—ACC’95, Seattle, WA, USA, 21–23 June 1995; Volume 3, pp. 1628–1632. [Google Scholar] [CrossRef]
- Menegaz, H.M.T.; Ishihara, J.Y.; Borges, G.A.; Vargas, A.N. A Systematization of the Unscented Kalman Filter Theory. IEEE Trans. Autom. Control 2015, 60, 2583–2598. [Google Scholar] [CrossRef] [Green Version]
- Julier, S.J.; Uhlmann, J.K. Reduced sigma point filters for the propagation of means and covariances through nonlinear transformations. In Proceedings of the 2002 American Control Conference (IEEE Cat. No.CH37301), Anchorage, AK, USA, 8–10 May 2002; pp. 887–892. [Google Scholar] [CrossRef] [Green Version]
- Julier, S.J. The spherical simplex unscented transformation. In Proceedings of the 2003 American Control Conference, Denver, CO, USA, 4–6 June 2003; pp. 2430–2434. [Google Scholar] [CrossRef]
- Simon, D. Optimal State Estimation–Kalman, H∞, and Nonlinear Approaches; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2006. [Google Scholar] [CrossRef]
- Lösler, M. Modellbildungen zur Signalweg- und in-situ Referenzpunktbestimmung von VLBI-Radioteleskopen. Ph.D. Thesis, Technische Universität Berlin, Institute of Geodesy and Geoinformation Science, Geodesy and Adjustment Theory, Berlin, Germany, 2021. [Google Scholar] [CrossRef]
- Mähler, S.; Klügel, T.; Lösler, M.; Schüler, T. Permanentes Referenzpunkt-Monitoring der TWIN Radioteleskope am Geodätischen Observatorium Wettzell. Allg. Vermess. Nachrichten 2018, 125, 210–219. [Google Scholar]
- Rothacher, M.; Beutler, G.; Behrend, D.; Donnellan, A.; Hinderer, J.; Ma, C.; Noll, C.; Oberst, J.; Pearlman, M.; Plag, H.P.; et al. The Future Global Geodetic Observing System. In Global Geodetic Observing System—Meeting the Requirements of a Global Society on a Changing Planet in 2020; Plag, H.P., Pearlman, M., Eds.; Springer: Berlin/Heidelberg, Germany, 2009; pp. 237–272. [Google Scholar] [CrossRef]
- Riepl, S.; Müller, H.; Mähler, S.; Eckl, J.; Klügel, T.; Schreiber, U.; Schüler, T. Operating Two SLR Systems at the Geodetic Observatory Wettzell–From Local Survey to Space Ties. J. Geod. 2019, 8, 2379–2387. [Google Scholar] [CrossRef]
- Riepl, S.; Schlüter, W.; Dassing, R.; Haufe, K.H.; Brandl, N.; Lauber, P.; Neidhardt, A. SOS-W—A two colour kilohertz SLR system. In Proceedings of the 14th International Laser Ranging Workshop, Boletin ROA; Garate, J., Davila, J., Noll, C., Pearlman, M., Eds.; Ministerio De Defensa, Real Instituto y Observatorio de la Armada (ROA): San Fernando, Spain, 2005. Number 5. [Google Scholar]
- Hexagon. AICON DPA Series—Unrivalled High-End Photogrammetry Systems, Datasheet; Hexagon Manufacturing Intelligence: Brunswick, Germany, 2019. Available online: https://hexagonmi.com (accessed on 14 March 2021).
- Rieke-Zapp, D.H.; Tecklenburg, W.; Peipe, J.; Hastedt, H.; Haig, C. Evaluation of the geometric stability and the accuracy potential of digital cameras — Comparing mechanical stabilisation versus parameterisation. ISPRS J. Photogramm. Remote Sens. 2009, 64, 248–258. [Google Scholar] [CrossRef]
- Guillory, J.; Truong, D.; Wallerand, J.P. Uncertainty assessment of a prototype of multilateration coordinate measurement system. Precis. Eng. 2020. [Google Scholar] [CrossRef]
- Kallio, U.; Poutanen, M. Simulation of Local Tie Accuracy on VLBI Antennas. In Proceedings of the 6th IVS General Meeting 2010—VLBI2010: From Vision to Reality; NASA/CP-2010-215864. Behrend, D., Baver, K.D., Eds.; NASA: Greenbelt, MD, USA, 2010; pp. 360–364. [Google Scholar]
- Eschelbach, C.; Haas, R. The IVS-Reference Point at Onsala—High End Solution for a Real 3D-Determination. In Proceedings of the 16th European VLBI for Geodesy and Astrometry (EVGA) Working Meeting; Schwegmann, W., Thorandt, V., Eds.; Verlag des Bundesamtes für Kartographie und Geodäsie: Frankfurt am Main, Germany, 2003; pp. 109–118. [Google Scholar]
- Lösler, M. Reference point determination with a new mathematical model at the 20 m VLBI radio telescope in Wettzell. J. Appl. Geod. 2008, 2, 233–238. [Google Scholar] [CrossRef] [Green Version]
- Börlin, N.; Murtiyoso, A.; Grussenmeyer, P. Efficient Computation of Posterior Covariance in Bundle Adjustment in DBAT for Projects with Large Number of Object Points. In Proceedings of the XXIV ISPRS Congress, International Society for Photogrammetry and Remote Sensing, Virtual Event, Nice, France, 31 August–2 September 2020; pp. 737–744. [Google Scholar] [CrossRef]
- JAiCov. Java Aicon Covariance matrix—Bundle Adjustment for Close-Range Photogrammetry. 2021. Available online: https://github.com/applied-geodesy/bundle-adjustment (accessed on 14 March 2021).
- Schwarz, H.R.; Köckler, N. Numerische Mathematik, 8th ed.; Vieweg + Teubner Verlag, Springer Fachmedien: Wiesbaden, Germany, 2011. [Google Scholar] [CrossRef]
- Klügel, T.; Mähler, S.; Schade, C. Ground Survey and Local Ties at the Geodetic Observatory Wettzell. In Proceedings of the 17th International Workshop on Laser Ranging—Extending the Range; Schreiber, U., Pearlman, M., Appleby, G., Eds.; Verlag des Bundesamtes für Kartographie und Geodäsie: Frankfurt am Main, Germany, 2012; Volume 48, pp. 127–131. [Google Scholar]
- Lösler, M.; Haas, R.; Eschelbach, C. Automated and continual determination of radio telescope reference points with sub-mm accuracy: Results from a campaign at the Onsala Space Observatory. J. Geod. 2013, 87, 791–804. [Google Scholar] [CrossRef] [Green Version]
- Lehmann, R.; Lösler, M. Hypothesis Testing in Non-Linear Models Exemplified by the Planar Coordinate Transformations. J. Geod. Sci. 2018, 8, 98–114. [Google Scholar] [CrossRef]
- Koch, K.R. Parameter Estimation and Hypothesis Testing in Linear Models; Springer: Berlin/Heidelberg, Germany, 1999. [Google Scholar] [CrossRef]
- Kutterer, H. On the sensitivity of the results of least-squares adjustments concerning the stochastic model. J. Geod. 1999, 73, 350–361. [Google Scholar] [CrossRef]
- Neitzel, F.; Ezhov, N.; Petrovic, S. Total Least Squares Spline Approximation. Mathematics 2019, 7, 462. [Google Scholar] [CrossRef] [Green Version]
- Lösler, M.; Eschelbach, C. Orthogonale Regression—Realität oder Isotropie? Tech. Mess. 2020, 87, 637–646. [Google Scholar] [CrossRef]
- Zhao, X.; Kermarrec, G.; Kargoll, B.; Alkhatib, H.; Neumann, I. Influence of the simplified stochastic model of TLS measurements on geometry-based deformation analysis. J. Appl. Geod. 2019, 13, 199–214. [Google Scholar] [CrossRef]
- Kermarrec, G.; Alkhatib, H.; Neumann, I. On the Sensitivity of the Parameters of the Intensity-Based Stochastic Model for Terrestrial Laser Scanner. Case Study: B-Spline Approximation. Sensors 2018, 18, 2964. [Google Scholar] [CrossRef] [PubMed] [Green Version]





| DOY | Img | Az | El | n | Conf |
|---|---|---|---|---|---|
| 255 | 1336 | 6 | 7 | 478 | i |
| 256 | 1793 | 6 | 7 | 485 | i |
| 257 | 1659 | 6 | 6 | 431 | ii |
| 258 | 1685 | 6 | 6 | 432 | ii |
| 259 | 1129 | 4 | 6 | 286 | iii |
| 260 | 1284 | 4 | 6 | 284 | iii |
| 264 | 1799 | 6 | 7 | 588 | i |
| DOY | ||||||||
|---|---|---|---|---|---|---|---|---|
| 255 | 64,367 | 10,015 | 0.886 | 0.03 | 0.03 | 0.02 | 0.05 | 0.04 |
| 256 | 82,905 | 13,003 | 0.917 | 0.03 | 0.03 | 0.02 | 0.05 | 0.04 |
| 257 | 91,437 | 12,139 | 0.949 | 0.02 | 0.02 | 0.02 | 0.04 | 0.03 |
| 258 | 92,817 | 12,130 | 0.939 | 0.02 | 0.03 | 0.02 | 0.04 | 0.04 |
| 259 | 62,549 | 8383 | 1.671 | 0.04 | 0.04 | 0.03 | 0.07 | 0.06 |
| 260 | 75,731 | 9277 | 0.923 | 0.02 | 0.02 | 0.02 | 0.03 | 0.03 |
| 264 | 120,005 | 13,663 | 0.844 | 0.02 | 0.02 | 0.01 | 0.03 | 0.03 |
| DOY | ||||||||
|---|---|---|---|---|---|---|---|---|
| 255 | ||||||||
| 256 | ||||||||
| 257 | ||||||||
| 258 | ||||||||
| 259 | ||||||||
| 260 | ||||||||
| 264 |
| DOY | ||||||||
|---|---|---|---|---|---|---|---|---|
| 255 | ||||||||
| 258 | ||||||||
| 260 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lösler, M.; Eschelbach, C.; Klügel, T.; Riepl, S. ILRS Reference Point Determination Using Close Range Photogrammetry. Appl. Sci. 2021, 11, 2785. https://doi.org/10.3390/app11062785
Lösler M, Eschelbach C, Klügel T, Riepl S. ILRS Reference Point Determination Using Close Range Photogrammetry. Applied Sciences. 2021; 11(6):2785. https://doi.org/10.3390/app11062785
Chicago/Turabian StyleLösler, Michael, Cornelia Eschelbach, Thomas Klügel, and Stefan Riepl. 2021. "ILRS Reference Point Determination Using Close Range Photogrammetry" Applied Sciences 11, no. 6: 2785. https://doi.org/10.3390/app11062785
APA StyleLösler, M., Eschelbach, C., Klügel, T., & Riepl, S. (2021). ILRS Reference Point Determination Using Close Range Photogrammetry. Applied Sciences, 11(6), 2785. https://doi.org/10.3390/app11062785






