Numerical Analysis of Pressure Profiles and Energy Dissipation across Stepped Spillways Having Curved Risers
Abstract
:1. Introduction
- (1)
- To estimate the total energy dissipation, energy dissipation distribution, and pressure profiles along the curved surface of the steps of the stepped spillway models (with curved risers) and along the vertical surface of steps for simple stepped spillways models under the skimming flow regime of flow.
2. Materials and Methods
2.1. Model Validation
2.2. Modelling of the Spillways
2.3. Properties of Model
2.4. Boundary Conditions
2.5. Flow 3D FEA Numerical Modelling
2.5.1. Mass Continuity Equation
2.5.2. Momentum Equation
2.5.3. RNG k-ε Turbulence Model
2.5.4. VOF Model
2.5.5. Air Entrainment Model
2.5.6. Density Evaluation Model
2.5.7. Drift Flux Model
2.6. General Simulation Setup
2.7. Methodology Limitation
3. Results
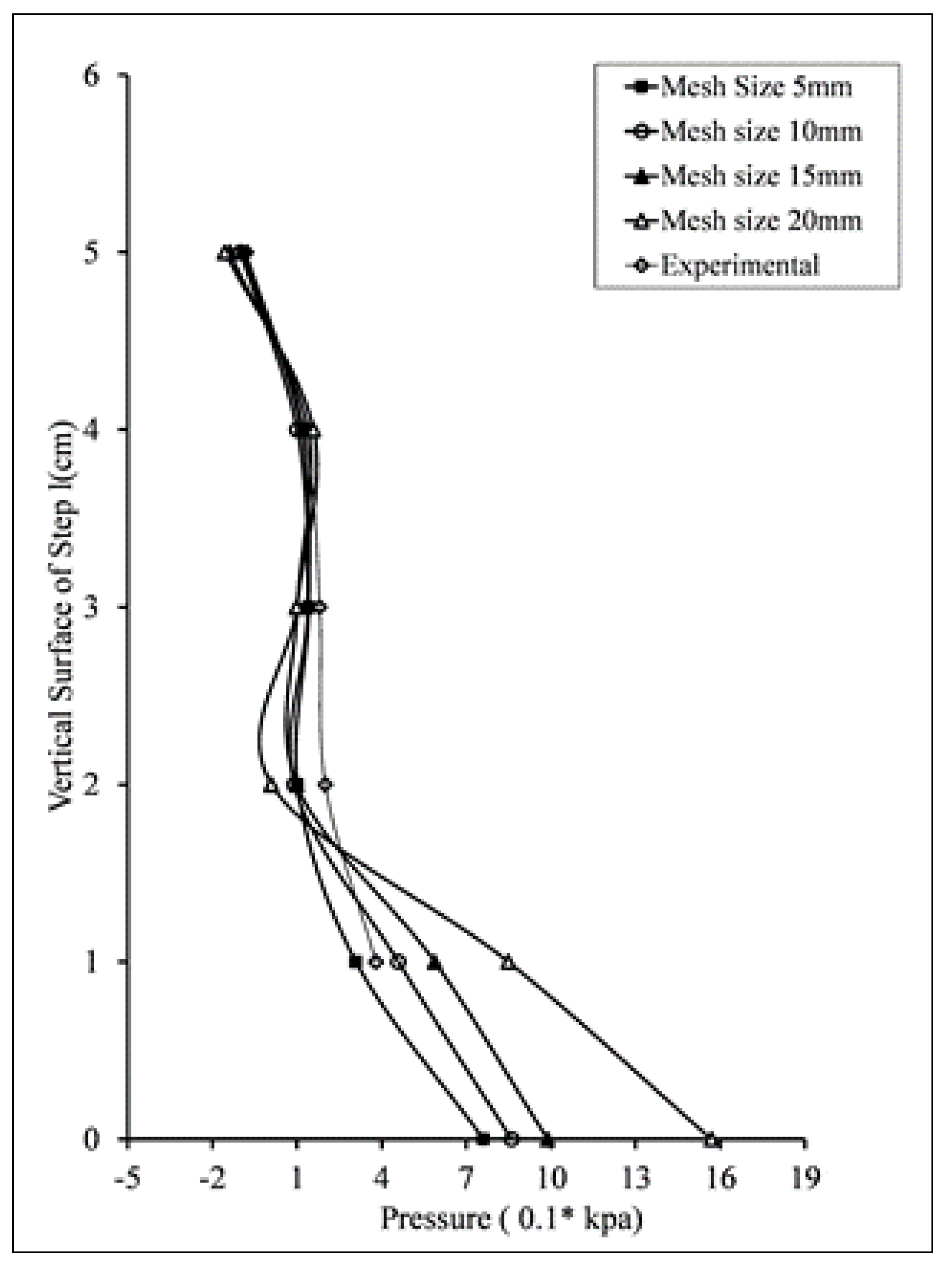
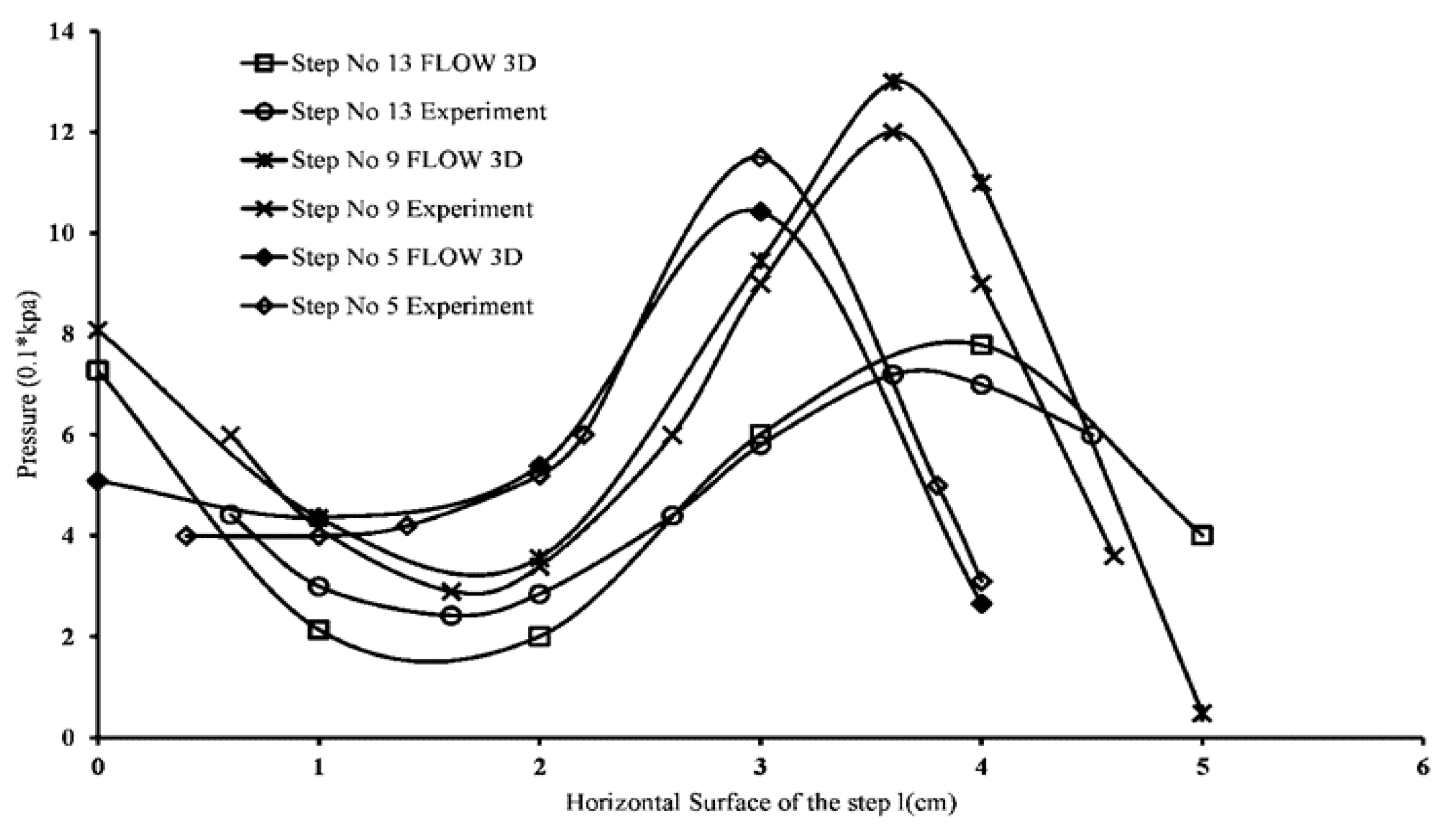
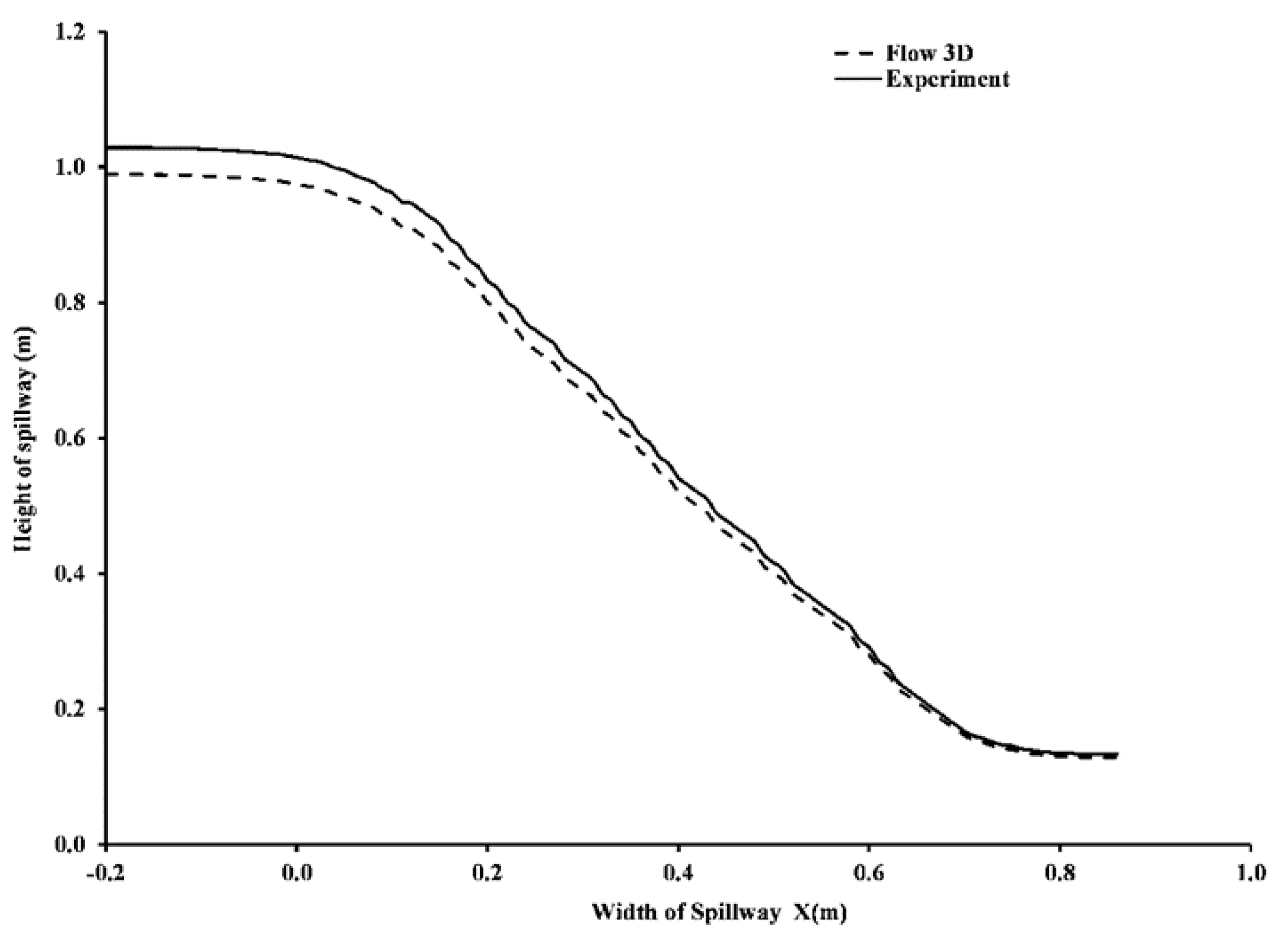
3.1. Model Validation
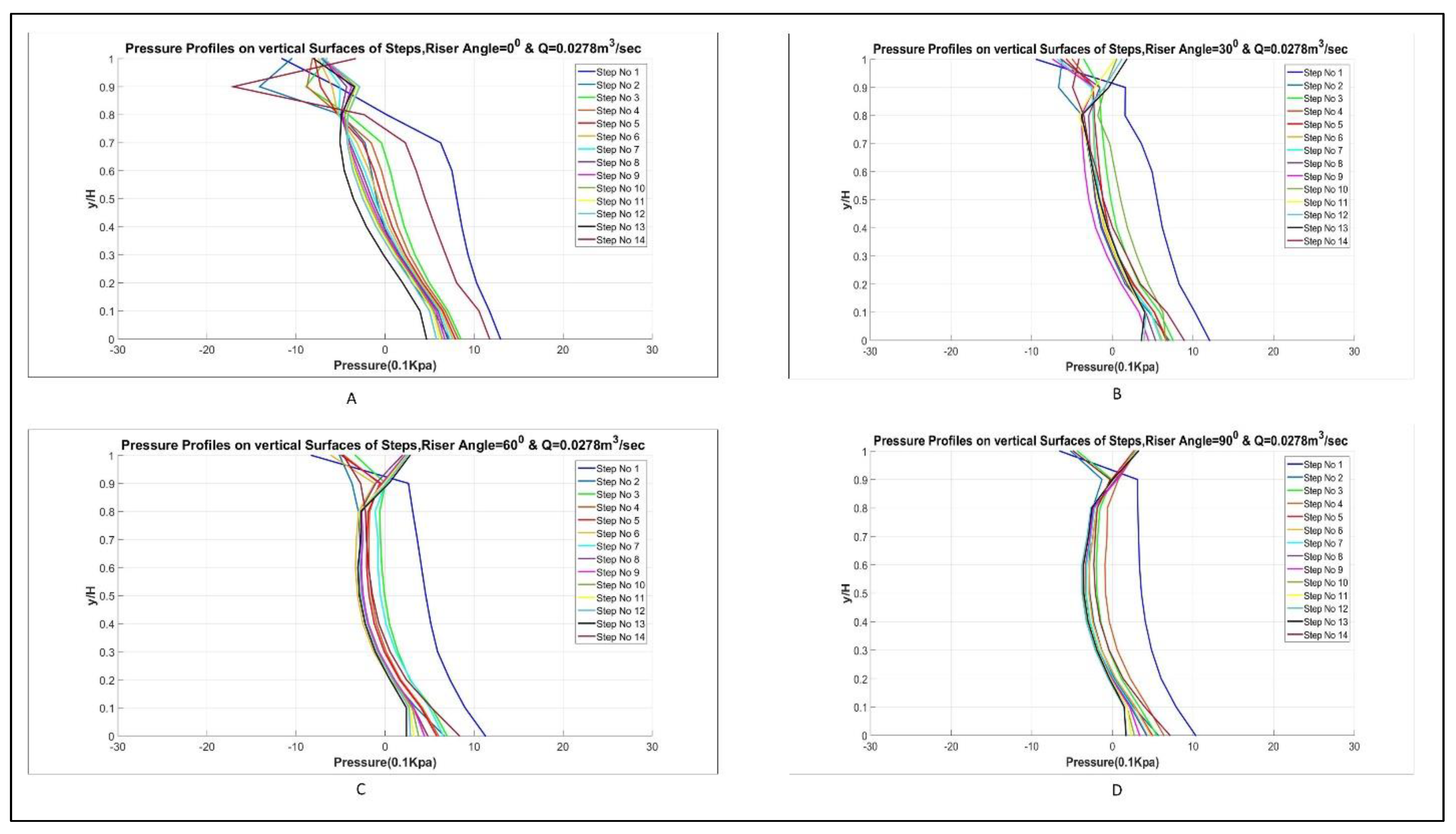
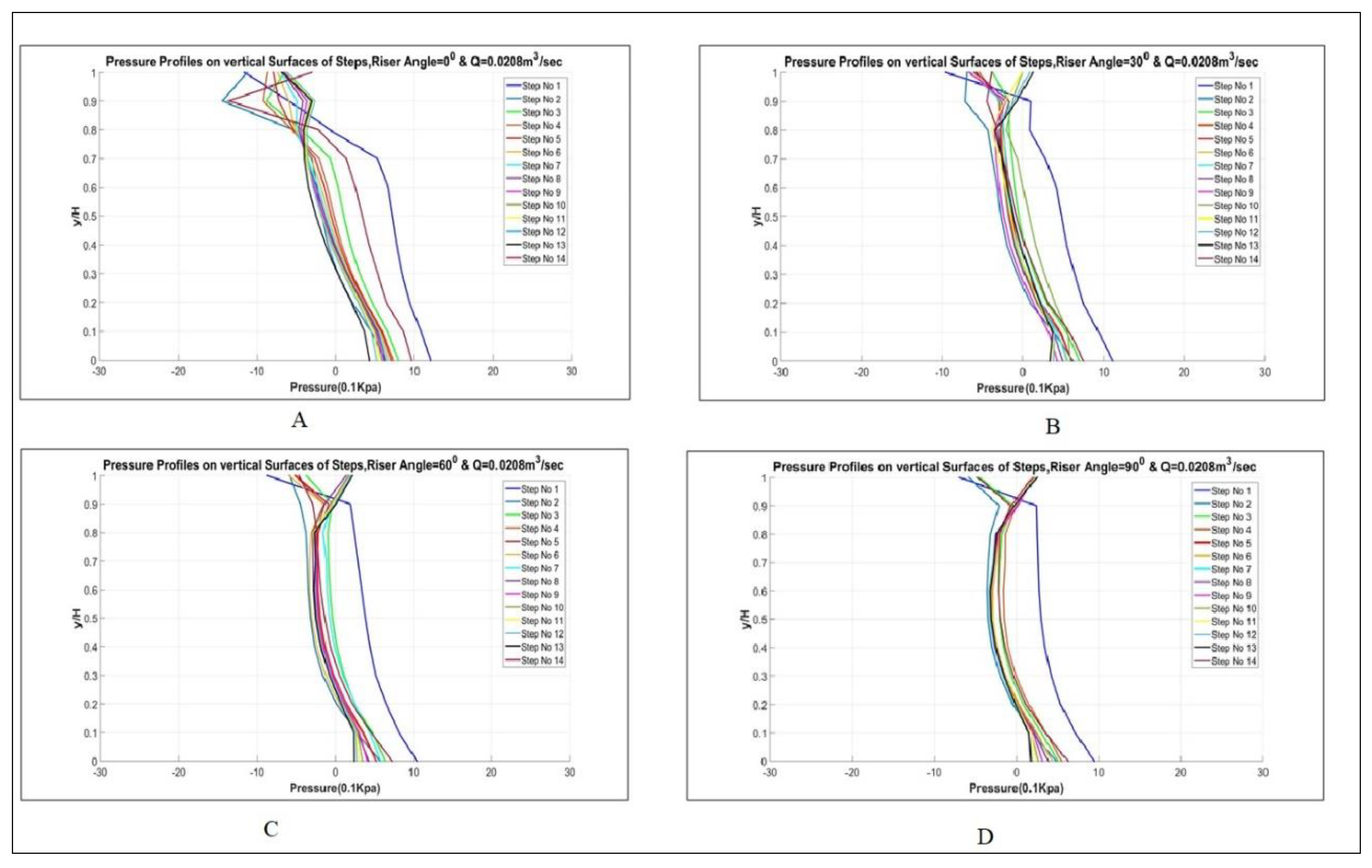
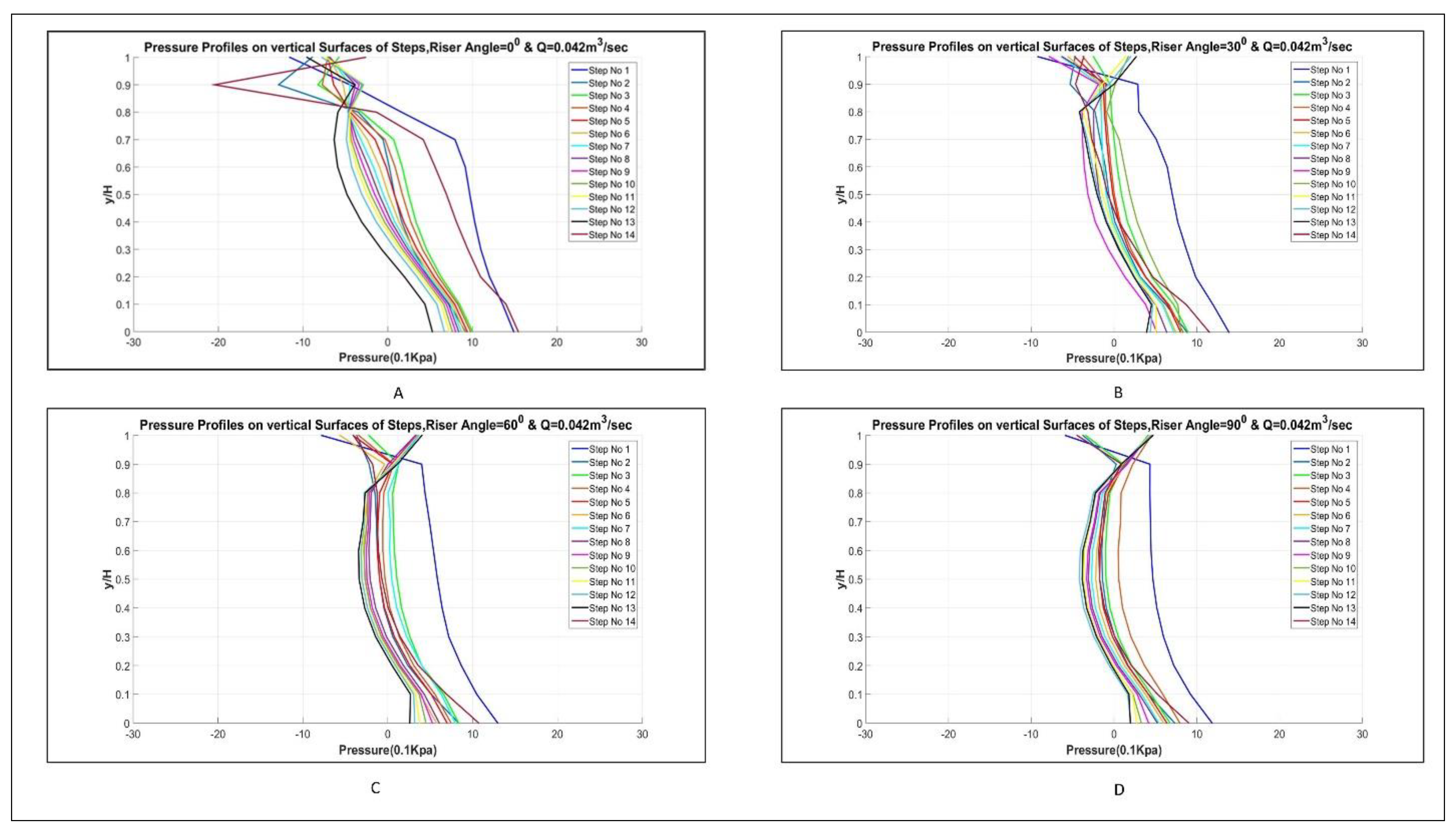
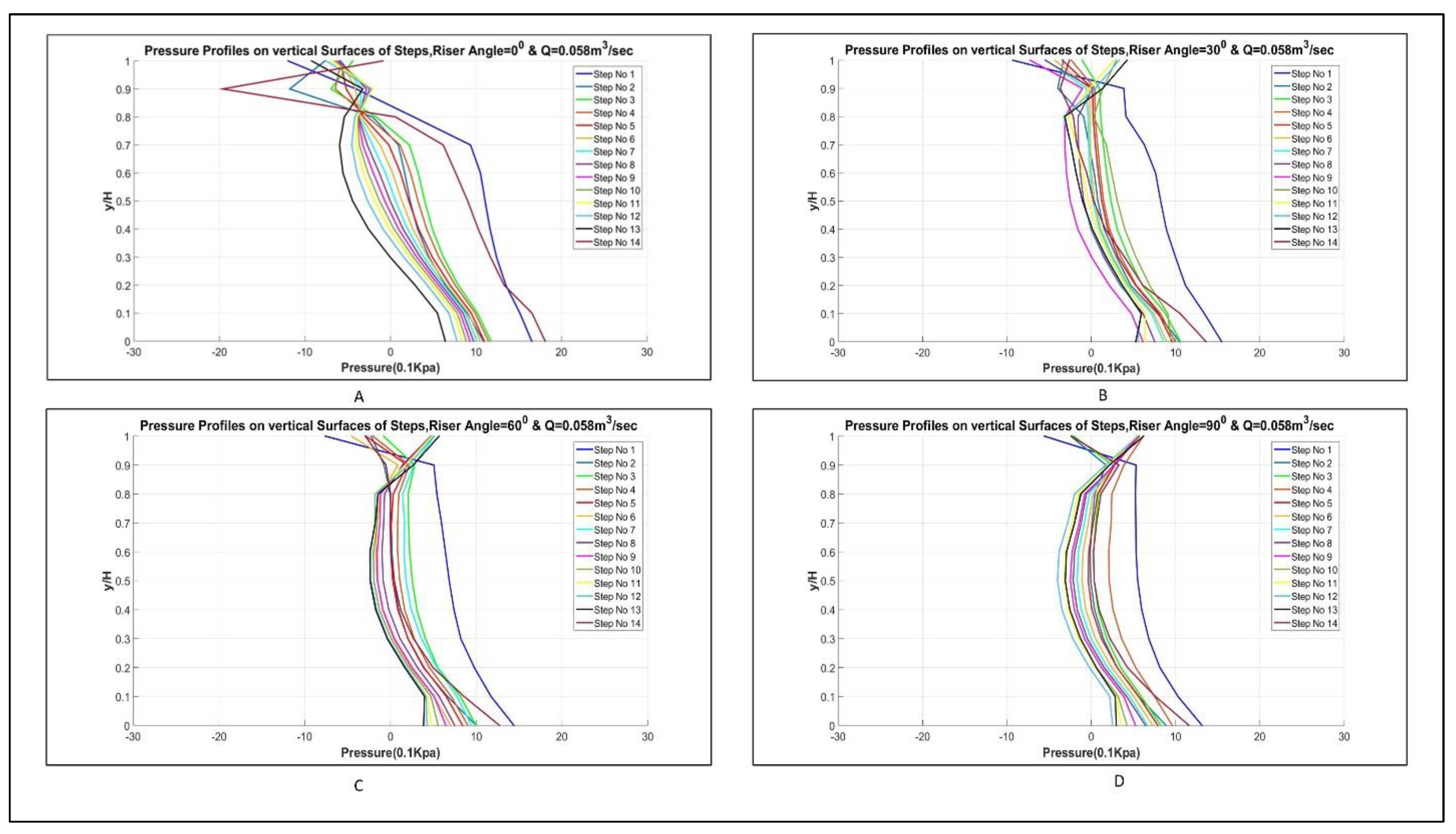
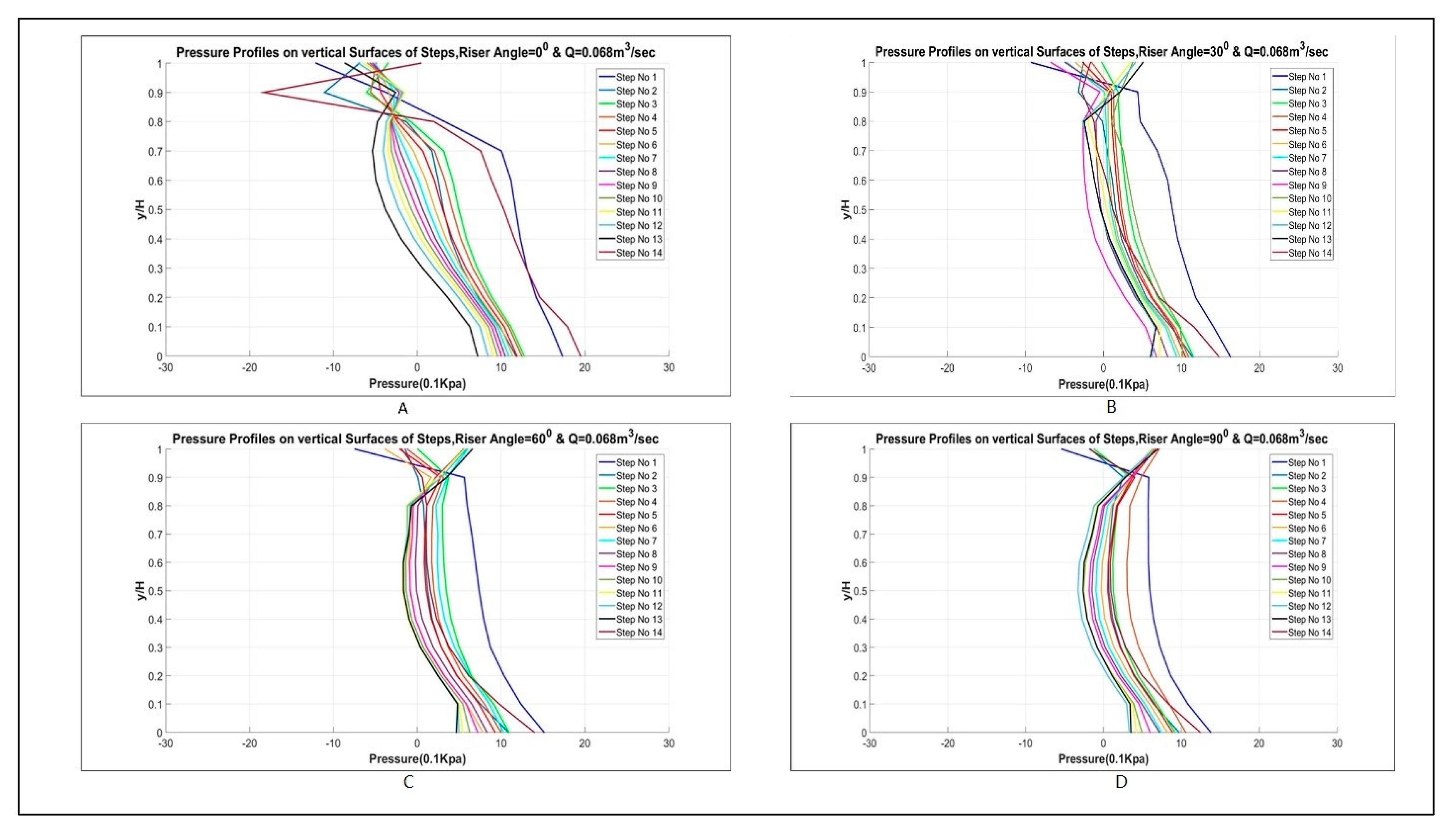
3.1.1. Pressure Profiles
3.1.2. Free Surface Elevation
3.1.3. Pressure Profiles
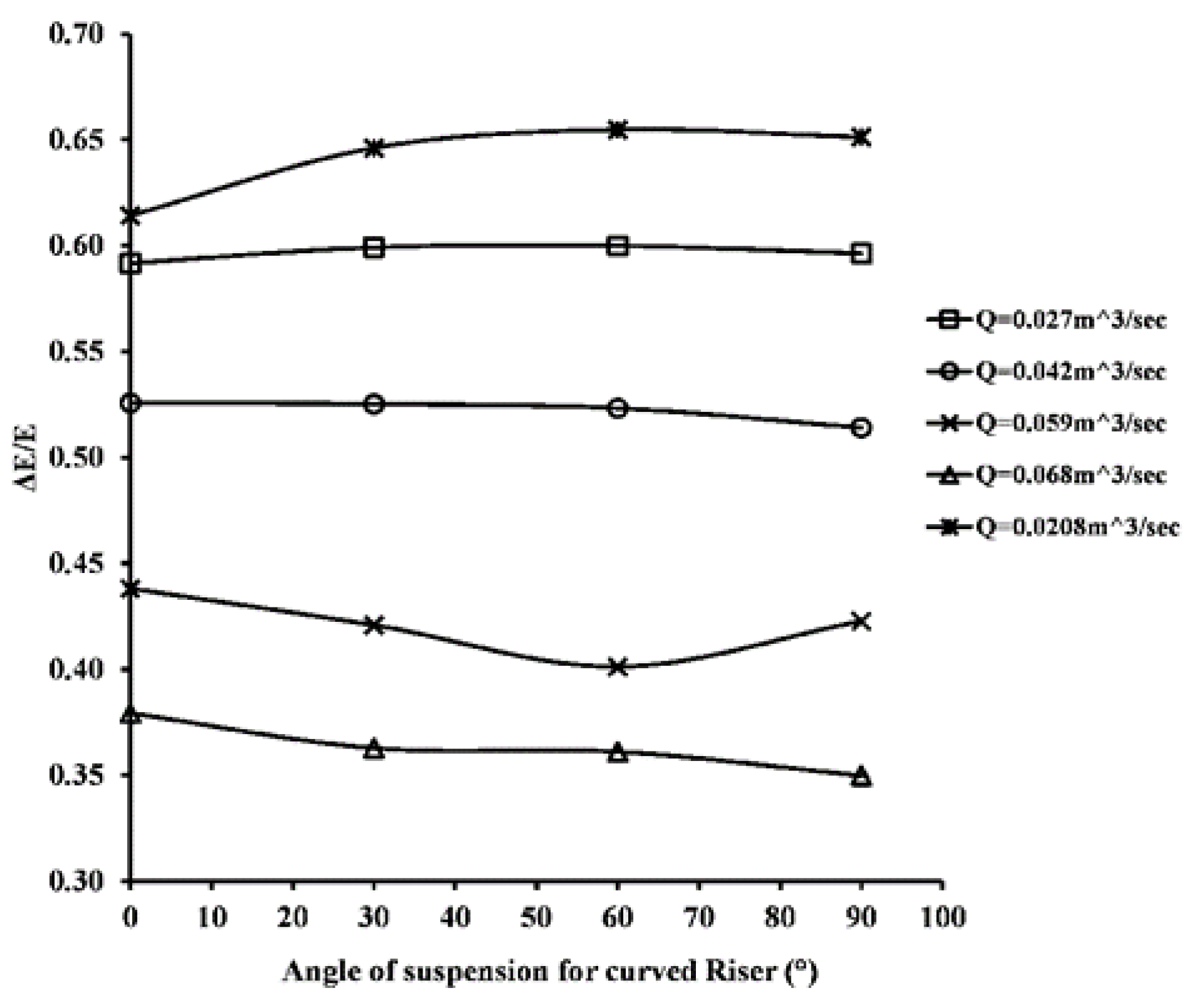
3.2. Total Energy Dissipation
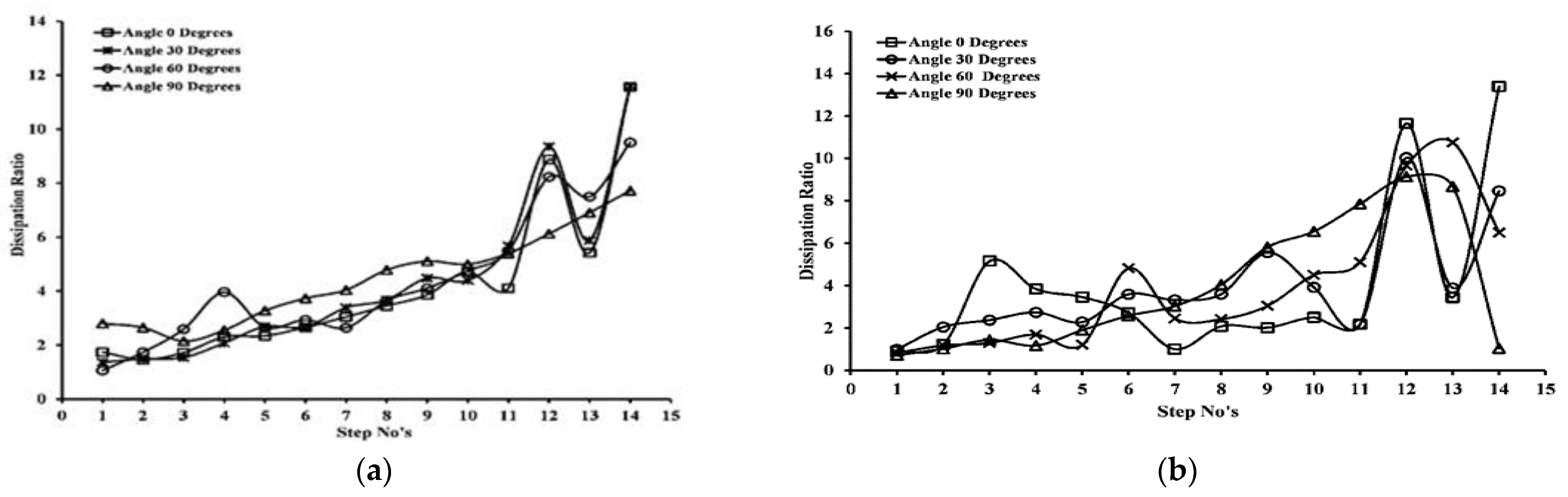
3.3. Energy Dissipation Distribution
4. Conclusions
- Total energy dissipations and pressure profiles were calculated for each of simulation. No increment in energy dissipation was obtained expect for lower flow rates like 0.020 m3/s where it increased to three percent in curved risers stepped spillways as compared to simple stepped spillways.
- In a simple stepped spillway, the lower steps, such as step nos. 12, 13, and 14 were dissipating more energy as compared to higher steps. Moreover, this shifted to higher steps in the case of stepped spillways having curved risers.
- Pressure profiles, along the vertical surface of steps in the case of simple stepped spillways, and along curved risers in the case of curved stepped spillways, showed that simple stepped spillway possess more negative pressure values as compared to curved stepped spillways. This can cause more cavitation in the case of simple stepped spillways as compared to curved stepped spillways, when the local pressure across the step face decreases less than the vapor pressure of water.
Police Recommendations
- This research was conducted through the latest CFD commercial code FLOW3D. Though the calibration process provides enough accuracy through the use of fine grids, there is still possibility for inaccuracies. The finer meshing and the use of second-order advection options can be utilized in FLOW3D to find inaccuracies.
- Spillway models with curved risers demonstrate an increment of three percent energy dissipation for lower flow rates. These types of spillways can be utilized for dams that are subject to continuous low emissions. Compared with a simple stepped spillway, the curved risers efficiently diminish material costs and allow more energy dissipation.
- Spillways with curved risers do not significantly increase energy dissipation for high flow rates. Compared with simple stepped spillways, they dissipate almost the same amount of energy. It is important to use these types of flow rates instead of simple stepped spillways because they can save material costs, but can dissipate the same amount of energy.
- Cavitation is an important parameter studied by dam spillway researchers. The spillways of many dams have been damaged by cavitation. Current research has identified that simple stepped spillways have more negative pressure than spillways with curved risers. The practical use of stepped spillways with curved risers can reduce the possibility of cavitation because they dissipate the same amount of energy.
- The present research shows that the steps that consume more energy are experiencing more negative pressure. Thus, specific steps that dissipate more energy are more susceptible to cavitation. The energy dissipation distribution of the steps can be used to analyze the number of steps that consume more energy and endure more negative pressure. This fact is very useful in a practical sense because more resources can be utilized to build steps that dissipate more energy and are more prone to cavitation than steps that dissipate less energy and are less likely to occur. This can help the design of the spillway in the future, and can stimulate the life of the spillway.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tabari, M.M.R.; Tavakoli, S. Effects of Stepped Spillway Geometry on Flow Pattern and Energy Dissipation. Arab. J. Sci. Eng. 2016, 41, 1215–1224. [Google Scholar] [CrossRef]
- Morovati, K.; Eghbalzadeh, A. Stepped Spillway Performance Study of the Pressure and Turbulent Kinetic Energy versus Discharge and Slope. J. Water Sci. Res. 2016, 8, 63–77. [Google Scholar]
- Bung, D.B.; Valero, D. Re-aeration on stepped spillways with special consideration of entrained and entrapped air. Geosciences 2018, 8, 333. [Google Scholar] [CrossRef] [Green Version]
- Christodoulou, G.C. Energy Dissipation on Stepped Spillways. J. Hydraul. Eng. 1993, 119, 644–650. [Google Scholar] [CrossRef]
- Sarfaraz, M.; Attari, J.; Pfister, M. Numerical Computation of Inception Point Location for Steeply Sloping Stepped Spillways. In Proceedings of the 9th International Congress of Civil Engineering, Isfahan, Iran, 8–10 May 2012; pp. 2–9. [Google Scholar]
- Chanson, H. Stepped spillway flows and air entrainment. Can. J. Civ. Eng. 1993, 20, 422–435. [Google Scholar] [CrossRef] [Green Version]
- Felder, S.; Chanson, H. Energy dissipation, flow resistance and gas-liquid interfacial area in skimming flows on moderate-slope stepped spillways. Environ. Fluid Mech. 2009, 9, 427–441. [Google Scholar] [CrossRef]
- Felder, S.; Chanson, H. Energy dissipation down a stepped spillway with nonuniform step heights. J. Hydraul. Eng. 2011, 137, 1543–1548. [Google Scholar] [CrossRef] [Green Version]
- Chen, Q.; Dai, G.; Liu, H. Volume of fluid model for turbulence numerical simulation of stepped spillway overflow. J. Hydraul. Eng. 2002, 128, 683–688. [Google Scholar] [CrossRef]
- Sorensen, R.M. Stepped spillway hydraulic model investigation. J. Hydraul. Eng. 1985, 111, 1461–1472. [Google Scholar] [CrossRef]
- Rice, C.E.; Kadavy, K.C. Model study of a roller compacted concrete stepped spillway. J. Hydraul. Eng. 1996, 122, 292–297. [Google Scholar] [CrossRef]
- Juny, M.S.; Pomares, J.; Dolz, J. Pressure field in skimming flow over a stepped spillway. Hydraul. Stepped Spillways 2020, 8, 137–145. Available online: http://www.flumen.upc.edu/admin/files/63.pdf (accessed on 15 November 2021).
- Yasuda, Y.; Takahashi, M.; Ohtsu, I. Discussion of “Volume of Fluid Model for Turbulence Numerical Simulation of Stepped Spillway Overflow” by Qun Chen, Guangqing Dai, and Haowu Liu. J. Hydraul. Eng. 2004, 130, 170–172. [Google Scholar] [CrossRef]
- Tabbara, M.; Chatila, J.; Awwad, R. Computational simulation of flow over stepped spillways. Comput. Struct. 2005, 83, 2215–2224. [Google Scholar] [CrossRef]
- Baylar, A.; Emiroglu, M.E.; Bagatur, T. An experimental investigation of aeration performance in stepped spillways. Water Environ. J. 2006, 20, 35–42. [Google Scholar] [CrossRef]
- Gonzalez, C.A.; Chanson, H. Hydraulic design of stepped spillways and downstream energy dissipators for embankment dams. Dam Eng. 2007, 17, 223–244. [Google Scholar]
- Amador, A.; Sánchez-Juny, M.; Dolz, J. Developing flow region and pressure fluctuations on steeply sloping stepped spillways. J. Hydraul. Eng. 2009, 135, 1092–1100. [Google Scholar] [CrossRef]
- Felder, S.; Chanson, H. Energy dissipation and residual energy on embankment dam stepped spillways. In Proceedings of the 19th Canadian Hydrotechnical Conference, Vancouver, BC, Canada, 9–14 August 2009; pp. 1940–1947. [Google Scholar]
- Chamani, M.R.; Rajaratnam, N.; Beirami, M.K. Turbulent jet energy dissipation at vertical drops. J. Hydraul. Eng. 2008, 134, 1532–1535. [Google Scholar] [CrossRef]
- Frizell, K.W.; Renna, F.M.; Matos, J. Cavitation potential of flow on stepped spillways. J. Hydraul. Eng. 2013, 139, 630–636. [Google Scholar] [CrossRef]
- Hamedi, A.; Mansoori, A.; Shamsai, A.; Amirahmadian, S. Effects of end sill and step slope on stepped spillway energy dissipation. J. Water Sci. Res. 2014, 6, 1–5. [Google Scholar]
- Husain, S.M.; Muhammed, J.R.; Karunarathna, H.U.; Reeve, D.E. Investigation of pressure variations over stepped spillways using smooth particle hydrodynamics. Adv. Water Resour. 2014, 6, 52–69. [Google Scholar] [CrossRef]
- Salmasi, F.; Özger, M. Neuro-fuzzy approach for estimating energy dissipation in skimming flow over stepped spillways. Arab. J. Sci. Eng. 2014, 39, 6099–6108. [Google Scholar] [CrossRef] [Green Version]
- Mansoori, A.; Erfanian, S.; Moghadam, F.K.; Mansoori, A.; Erfanian, S.; Moghadam, F.K. A Study of the Conditions of Energy Dissipation in Stepped Spillways with Λ-shaped step Using FLOW-3D. Civ. Eng. J. 2017, 3, 856–867. [Google Scholar] [CrossRef] [Green Version]
- Yusuf, F.; Micovic, Z. Prototype-scale investigation of spillway cavitation damage and numerical modeling of mitigation options. J. Hydraul. Eng. 2020, 146, 100–120. [Google Scholar] [CrossRef]
- Flow Science. User Manual: FLOW-3D® Cast 3.2. 2009, 1–49. Available online: http://www.easysimulation.com/public/flow3dcast/documentation/FLOW-3D_Cast_3.2_Manual.pdf (accessed on 15 November 2021).
- Rajaei, S.H.; Khodashenas, S.R.; Esmaili, K. Comparative evaluation of energy dissipation over short stepped gabion and rigid spillways. J. Hydraul. Res. 2020, 58, 262–273. [Google Scholar] [CrossRef]
- Bentalha, C.; Habi, M. Inception Point and Air-Water Flow Characteristics Over Stepped Spillway: Numerical Study. Larhyss J. 2020, 31, 91–105. [Google Scholar]
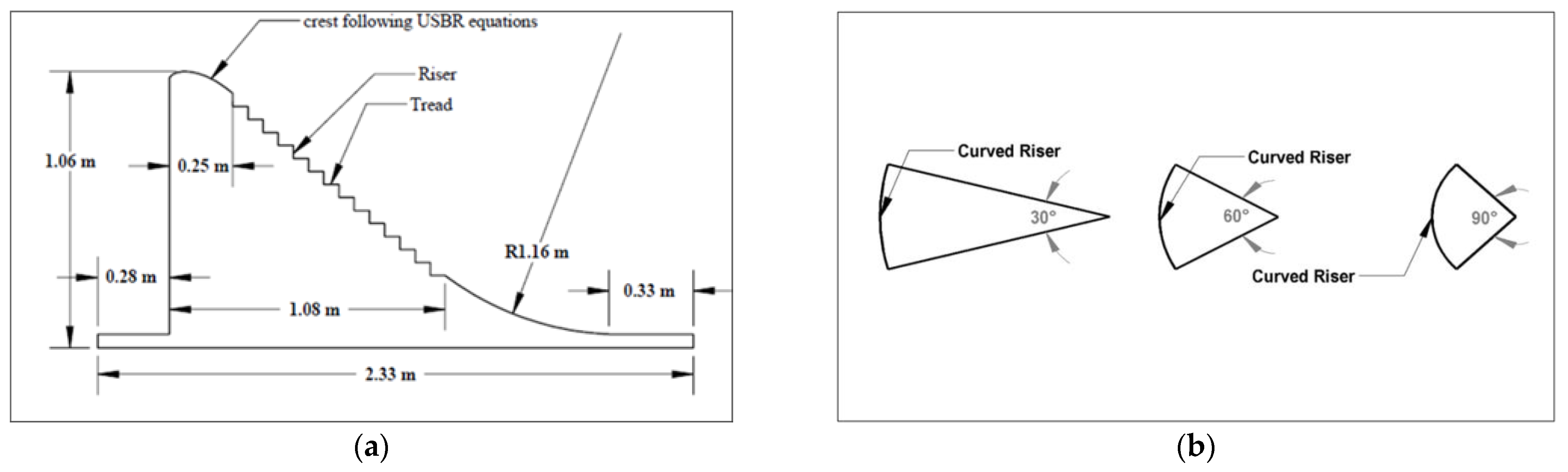
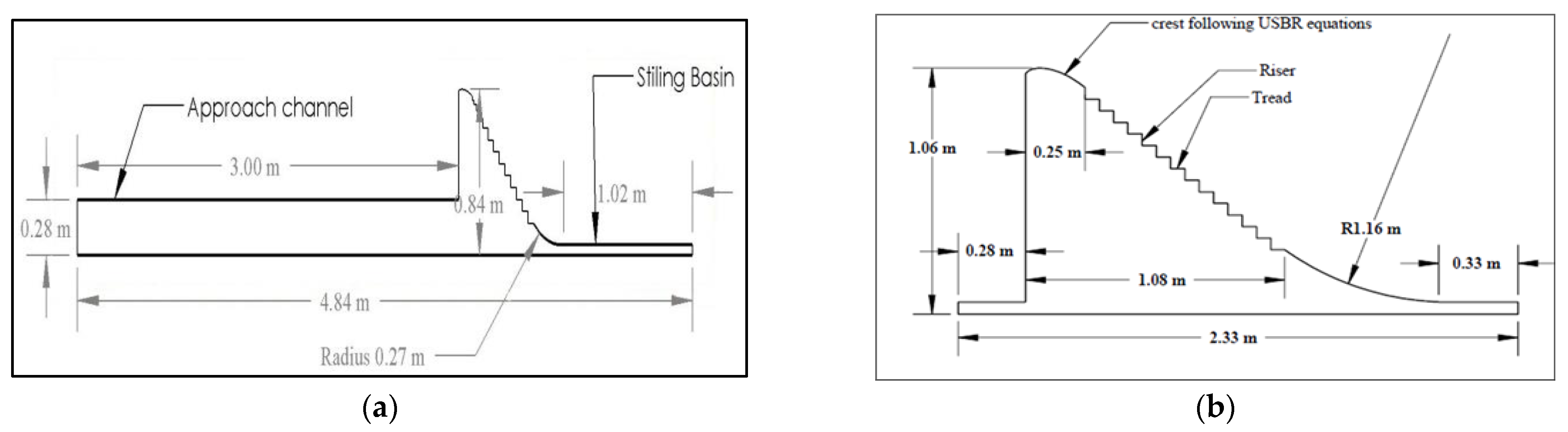




| Reference | Author(s) | Country | Type of Spillway Model | Pressure Profiles | Total Energy Dissipation | Energy Dissipation Distribution | Methodology |
|---|---|---|---|---|---|---|---|
| 28 | [1] | Iran | Vertical risers and Horizontal tread | × | √ | × | Physical Modelling/Laboratory setting |
| 29 | [2] | Algeria | Vertical and Horizontal Tread | × | × | × | Numerical Modelling/Ansys Fluent |
| 30 | [3] | China | Sky Jump/Vertical risers and Horizontal/Inclined Treads | × | √ | × | Physical Modelling/Laboratory setting |
| 31 | [4] | Vietnam | Vertical Risers and Horizontal Tread | × | × | × | Physical Modelling/Laboratory Settings. |
| 32 | [5] | Iran | Sloping steps, Separation between steps | × | √ | × | Physical Modelling & Numerical Modelling. |
| 27 | [6] | Canada | Smooth and Rough Vertical and Horizontal Treads/Steps with edges | √ | × | × | Numerical Modelling FLOW 3D |
| 33 | [7] | Thailand | Vertical Risers and horizontal treads | × | √ | × | Numerical Modelling/Physical Laboratory Model. |
| 34 | [8] | China | Vertical Risers and horizontal treads. | √ | × | × | Numerical Modelling/Physical Laboratory Model. |
| 35 | [9] | Sweden | V type of steps with vertical and horizontal risers. | √ | √ | × | Numerical Modelling FLOW 3D |
| 36 | [10] | China | V type of steps with vertical and horizontal risers. | × | √ | × | Numerical Modelling FLOW 3D |
| 37 | [11] | Iran | Vertical Risers and Pooled horizontal treads. | × | × | × | Numerical Modelling |
| 38 | [12] | Iran | Vertical Risers and Horizontal Treads. | × | × | × | Artificial Neural Network, Support Vector Machine |
| 39 | [13] | Germany | Vertical Risers and Horizontal Treads. | × | × | × | Laboratory Experimentation |
| 40 | [14] | United Kingdom | Vertical Risers and Horizontal Treads. | × | √ | × | Laboratory Experimentation |
| This Research Work | Pakistan | Curved Risers and Horizontal Treads with different angle. | √ | √ | √ | Numerical Modelling FLOW 3D, ArcGis |
| Items | Description |
|---|---|
| Location | Taxila on Haro River (Pakistan) |
| Catchment Area | 308 square miles |
| Design Flood discharge | 166,000 cusecs |
| Main Dam type | earth and Rock fill |
| Maximum dam height | 167 ft |
| No. of spillways | 5 |
| Items | Description |
|---|---|
| Type of spillway | Stepped |
| No of steps | 14 |
| Maximum design head | 213 mm |
| Maximum design discharge | 0.064 m3/s |
| Sr No | Part | Model 2 | Model 1 (Validation Model) |
|---|---|---|---|
| 1 | Total Height | 1.06 m | 78.9 cm |
| 2 | Total Width | 30 cm | 30 cm |
| 3 | No of Steps | 14 | 13 |
| 4 | Riser Height | 6 cm | First 5 steps (2, 2.4, 3, 4, and 5 cm) others 6 cm |
| 5 | Tread length | 5 cm | First 5 steps (1.5, 1.8, 2.25, 3, and 3.75 cm) others 6 cm |
| 6 | Equation of Crest | y = 2.632x1.64 | y = 3.632x1.85 |
| 7 | Tread Shape | Horizontal | Horizontal |
| 8 | Riser Shape | Vertical/Curved | Vertical |
| 9 | Slope of spillway | 1 V:0.80 H | 1 V: 0.75 H |
| 10 | Radius of Bottom Curve | 116 mm | 28 cm |
| Sr No | Property | Value |
|---|---|---|
| 1 | Source Material | acrylic |
| 2 | Specific Gravity | 1.18 |
| 3 | Refractive index | 1.49 |
| 4 | Modulus of Elasticity | 450,000 psi |
| Riser Type | Q = 0.027 | Q = 0.042 | Q = 0.059 | Q = 0.068 | Q = 0.0208 |
|---|---|---|---|---|---|
| Vertical | 59.17% | 52.58% | 43.81% | 37.93% | 61.42% |
| 30° | 59.91% | 52.53% | 42.08% | 36.28% | 64.61% |
| 60° | 59.99% | 52.33% | 40.12% | 36.11% | 65.48% |
| 90° | 59.63% | 51.4% | 42.27% | 34.8% | 65.12% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Saqib, N.u.; Akbar, M.; Pan, H.; Ou, G.; Mohsin, M.; Ali, A.; Amin, A. Numerical Analysis of Pressure Profiles and Energy Dissipation across Stepped Spillways Having Curved Risers. Appl. Sci. 2022, 12, 448. https://doi.org/10.3390/app12010448
Saqib Nu, Akbar M, Pan H, Ou G, Mohsin M, Ali A, Amin A. Numerical Analysis of Pressure Profiles and Energy Dissipation across Stepped Spillways Having Curved Risers. Applied Sciences. 2022; 12(1):448. https://doi.org/10.3390/app12010448
Chicago/Turabian StyleSaqib, Najam us, Muhammad Akbar, Huali Pan, Guoqiang Ou, Muhammad Mohsin, Assad Ali, and Azka Amin. 2022. "Numerical Analysis of Pressure Profiles and Energy Dissipation across Stepped Spillways Having Curved Risers" Applied Sciences 12, no. 1: 448. https://doi.org/10.3390/app12010448
APA StyleSaqib, N. u., Akbar, M., Pan, H., Ou, G., Mohsin, M., Ali, A., & Amin, A. (2022). Numerical Analysis of Pressure Profiles and Energy Dissipation across Stepped Spillways Having Curved Risers. Applied Sciences, 12(1), 448. https://doi.org/10.3390/app12010448





