Influence of Fastener Failure on Dynamic Performance of Subway Vehicle
Abstract
:1. Introduction
2. Model of Vehicle-Structure System
2.1. System Model
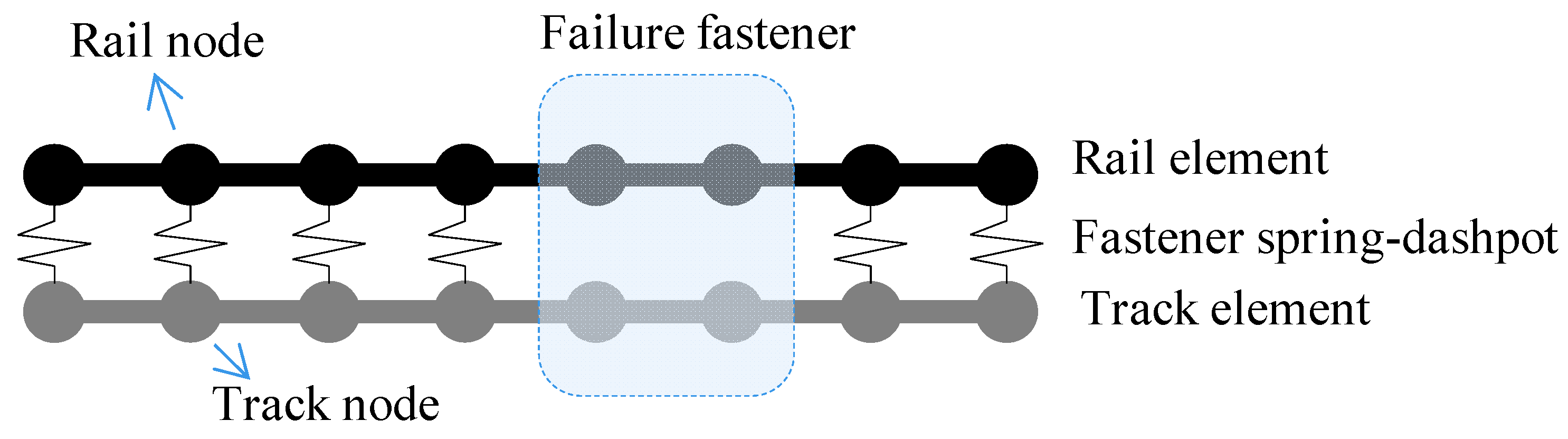
2.1.1. Model of Track
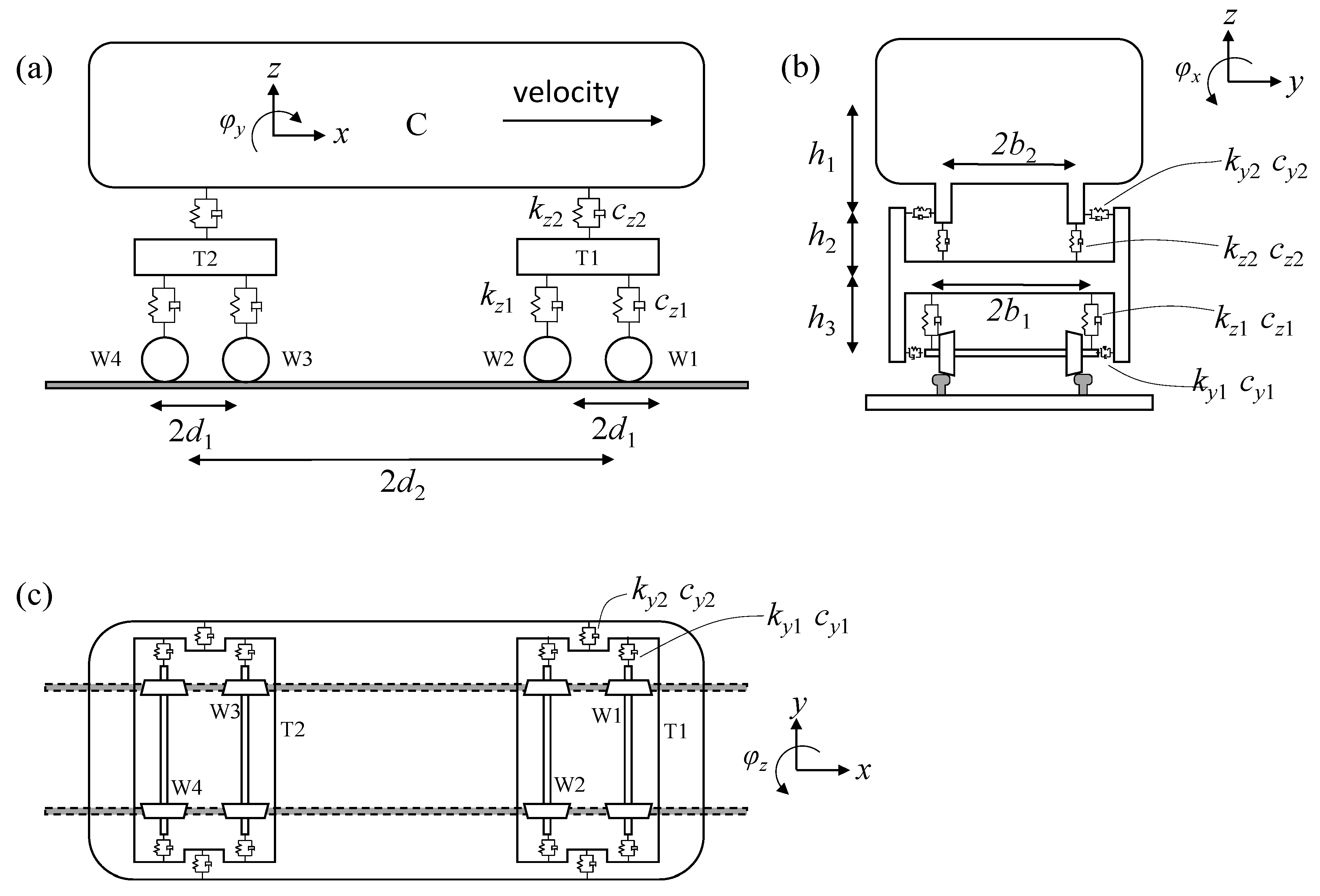
2.1.2. Model of Vehicle
2.1.3. Model of Coupled System
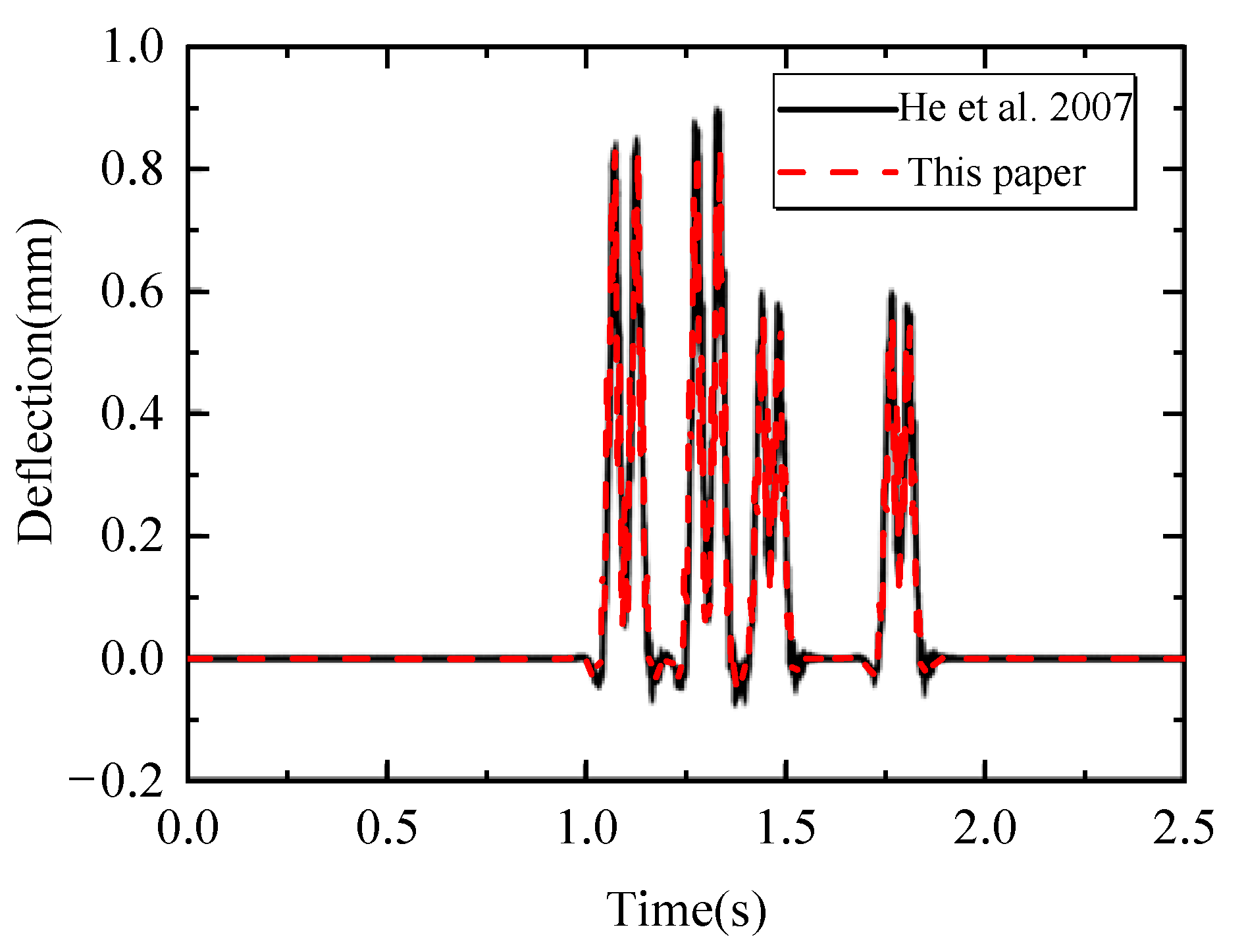
2.2. Model Validation
3. Numerical Analysis
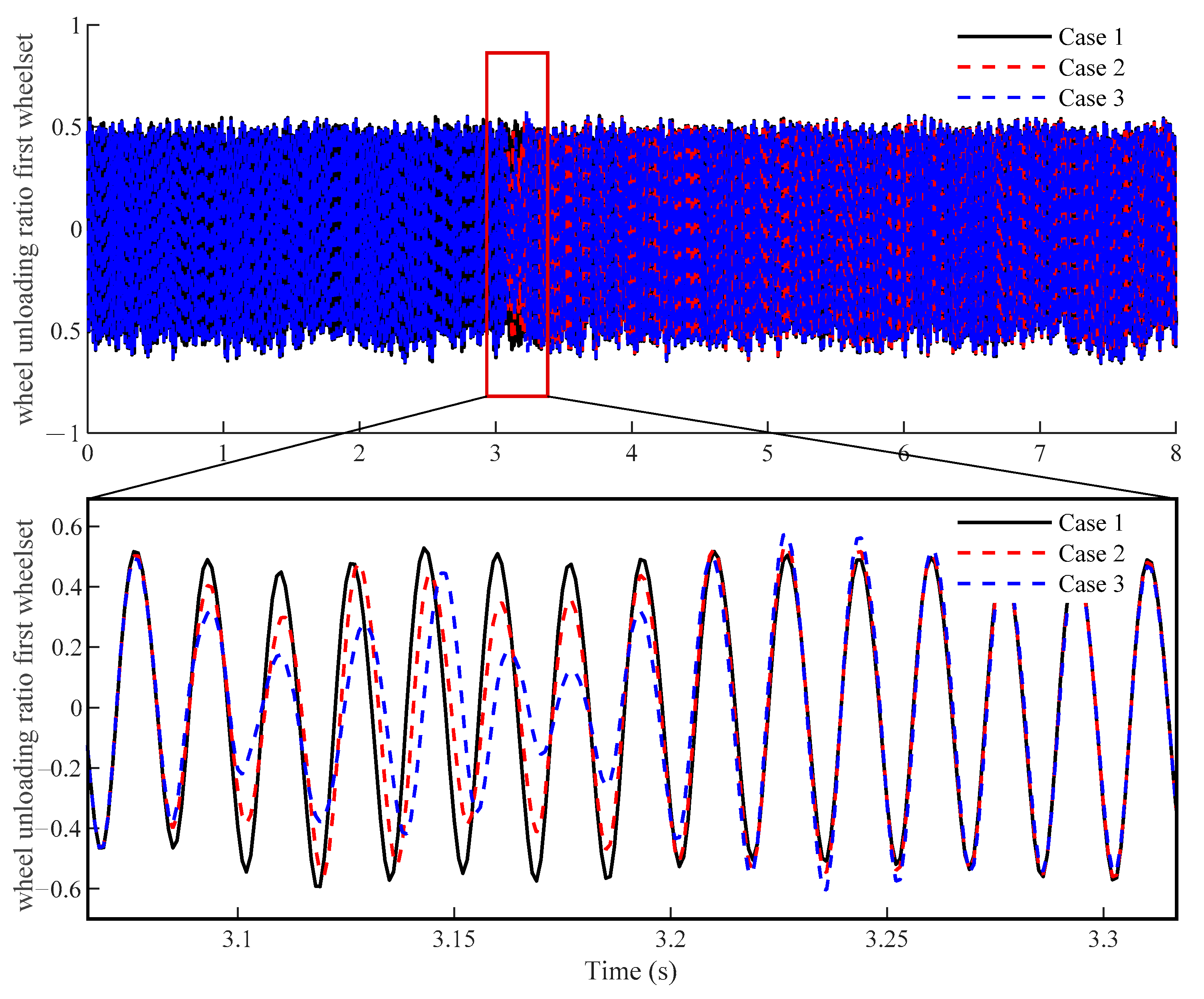
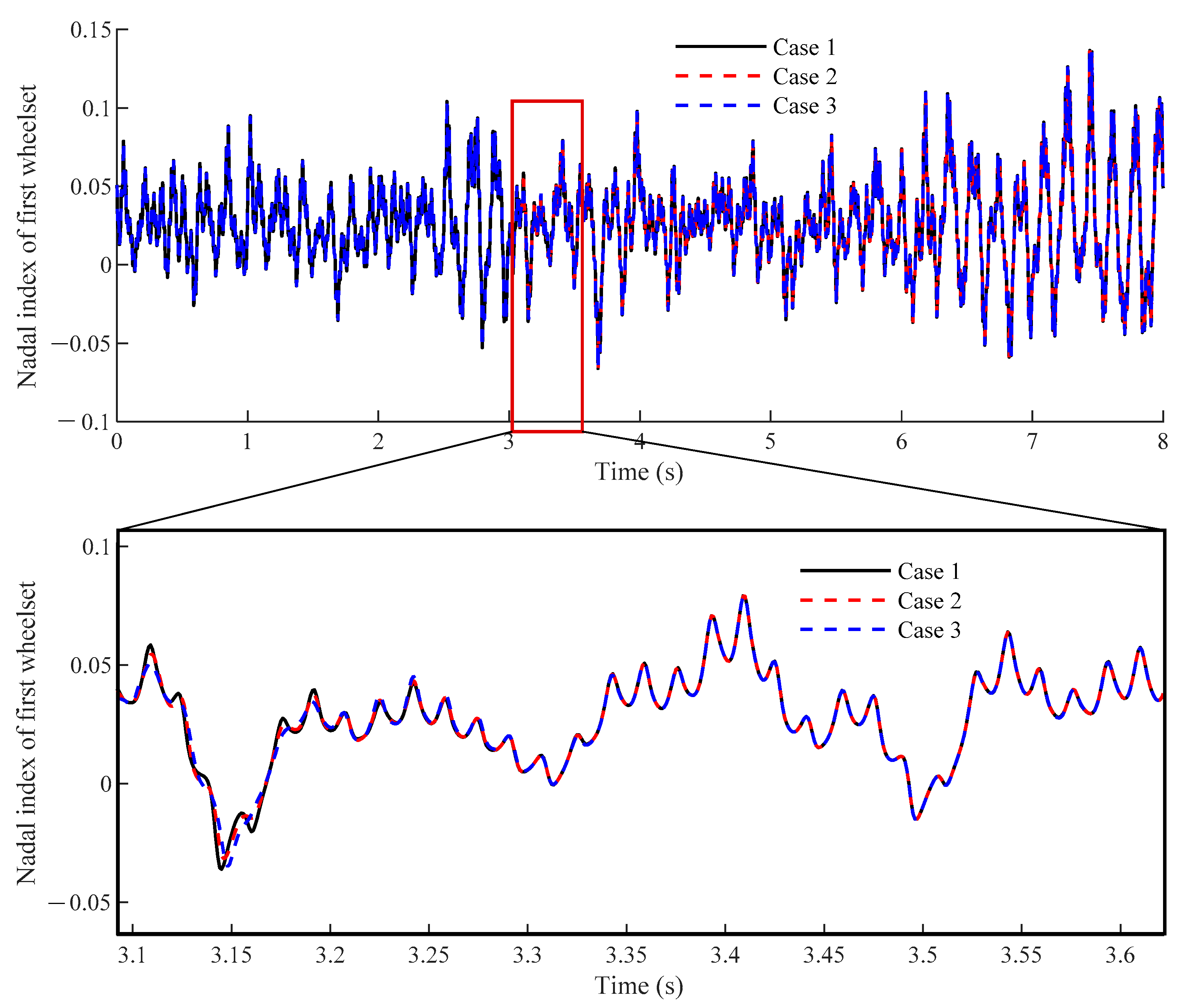
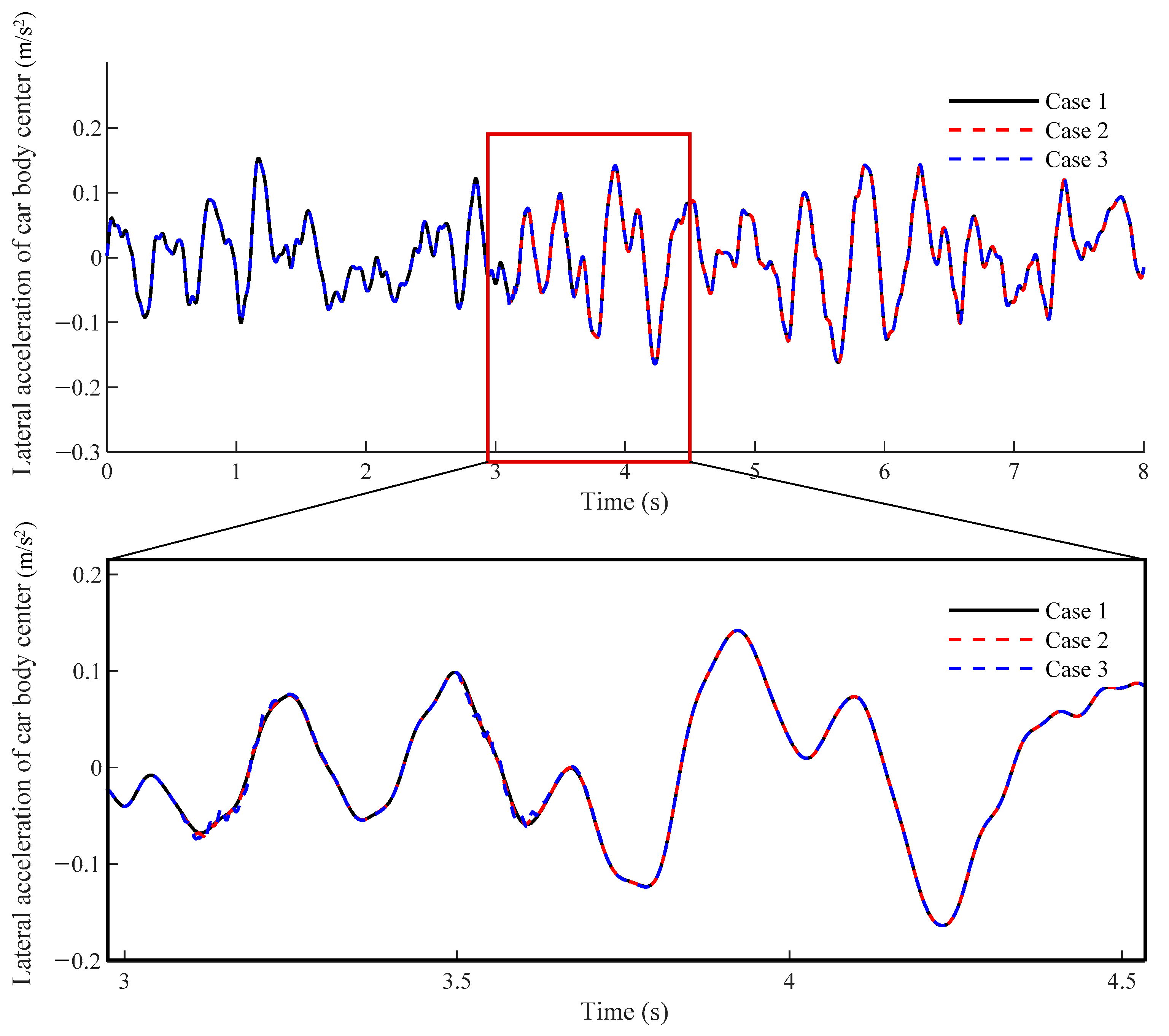
3.1. Time History Analysis
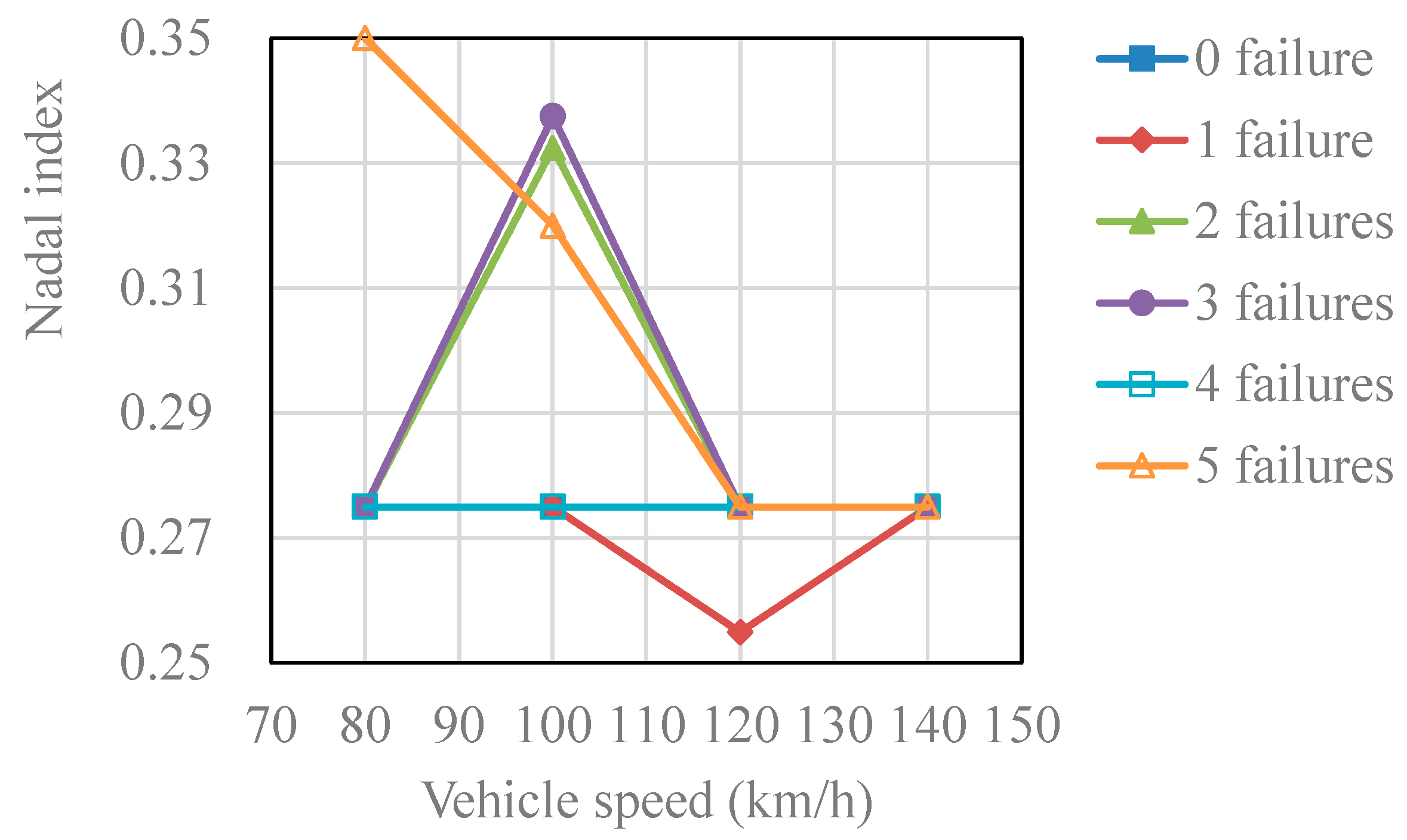
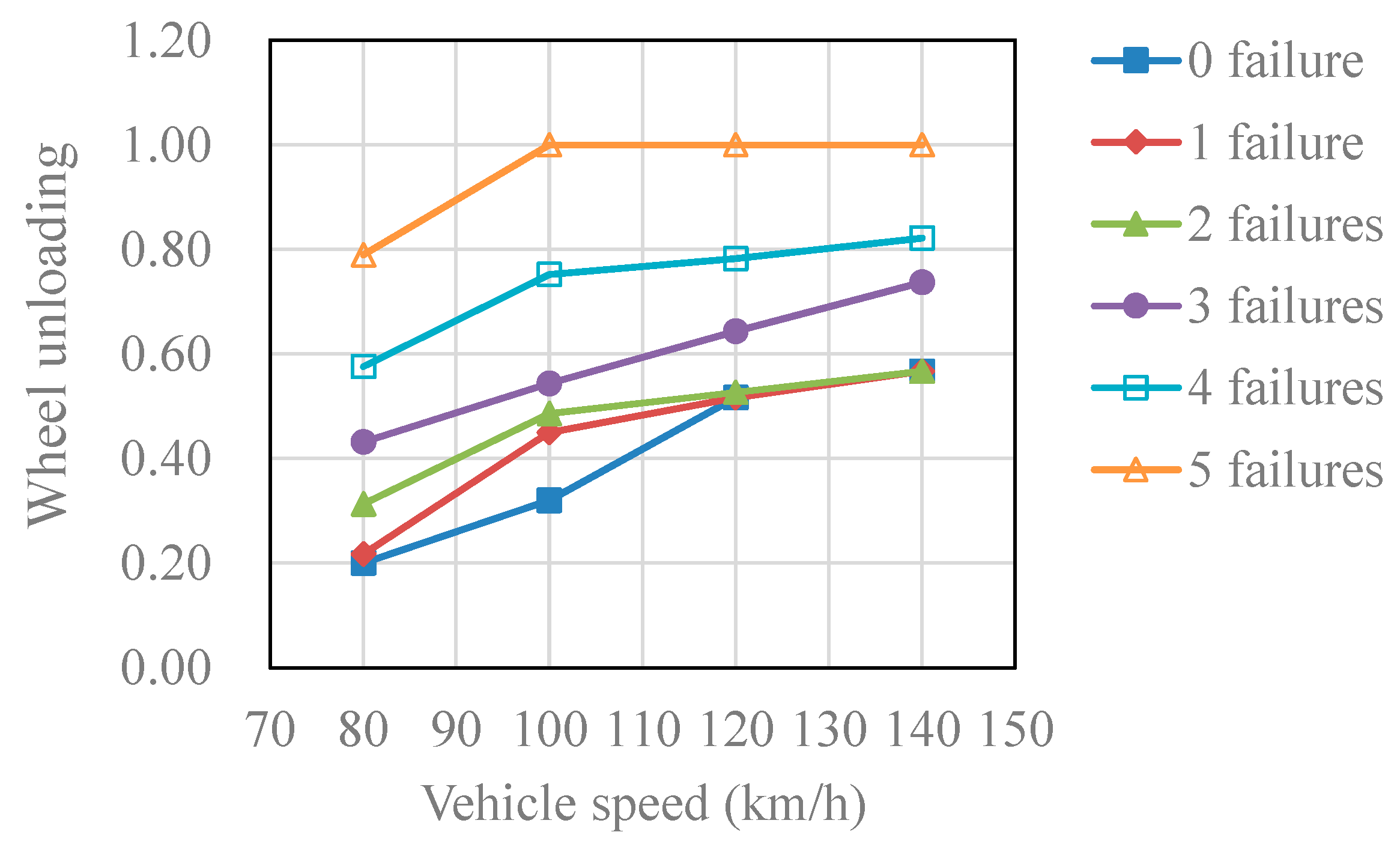
3.2. Parameter Analysis
4. Conclusions
- (1)
- Through the comparison of time history response, it is found that the impact of fastener failure on the structural response is greater than that of vehicle response. Among them, the structural displacement response is more sensitive to structural failure than the acceleration response.
- (2)
- At different vehicle speeds, the number of fastener failures has little effect on the acceleration response of vehicle operation.
- (3)
- At different vehicle speeds, the number of fastener failures has an impact on the Nadal index of the vehicle, but the law is not obvious. In general, the more fastener failures, the more unsafe it is.
- (4)
- Under different vehicle speeds, the number of fastener failures has a significant impact on the wheel load reduction rate of the vehicle, and the wheel load reduction rate increases with the increase in vehicle speed and with the increase in the number of fastener failures. For the engineering background, when only less than three fasteners fail, the vehicle can run safely at the design speed; when four fasteners fail, the safe allowable speed of the vehicle is 130 km/h; when five fasteners fail, the safe allowable speed of the vehicle is 80 km/h.
- (5)
- The vehicle acceleration is not sensitive to the failure of the fastener but the failure of the fastener may endanger the running safety so it is necessary to use manual or other technical means for regular detection of fasteners. According to the normal vehicle operation speed, it is suggested that when more than three continuous fasteners are likely to fail or have become loose, they must be maintained.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ren, J.; Li, X.; Yang, R.; Wang, P.; Xie, P. Criteria for repairing damages of CA mortar for prefabricated framework-type slab track. Constr. Build. Mater. 2016, 110, 300–311. [Google Scholar] [CrossRef]
- Zeng, Z.; Wang, J.; Shen, S.; Li, P.; Abdulmumin Ahmed, S.; Wang, W. Experimental study on evolution of mechanical properties of CRTS III ballastless slab track under fatigue load. Constr. Build. Mater. 2019, 210, 639–649. [Google Scholar] [CrossRef]
- Feng, Y.; Jiang, L.; Zhou, W.; Lai, Z.; Chai, X. An analytical solution to the mapping relationship between bridge structures vertical deformation and rail deformation of high-speed railway. Steel Compos. Struct. 2019, 33, 209–224. [Google Scholar] [CrossRef]
- Feng, Y.; Jiang, L.; Zhou, W.; Chen, M. Post-earthquake track irregularity spectrum of high-speed railways continuous girder bridge. Steel Compos. Struct. 2021, 40, 323–338. [Google Scholar] [CrossRef]
- Yan, Q.; Sun, M.; Qing, S.; Deng, Z.; Dong, W. Numerical investigation on the damage and cracking characteristics of the shield tunnel caused by derailed high-speed train. Eng. Fail. Anal. 2020, 108, 104205. [Google Scholar] [CrossRef]
- Zhu, Z.; Wang, L.; Costa, P.A.; Bai, Y.; Yu, Z. An efficient approach for prediction of subway train-induced ground vibrations considering random track unevenness. J. Sound Vib. 2019, 455, 359–379. [Google Scholar] [CrossRef]
- Hung, H.H.; Chen, G.H.; Yang, Y.B. Effect of railway roughness on soil vibrations due to moving trains by 2.5D finite/infinite element approach. Eng. Struct. 2013, 57, 254–266. [Google Scholar] [CrossRef]
- Yang, Y.B.; Liang, X.; Hung, H.-H.; Wu, Y. Comparative study of 2D and 2.5D responses of long underground tunnels to moving train loads. Soil Dyn. Earthq. Eng. 2017, 97, 86–100. [Google Scholar] [CrossRef]
- Jin, Q.; Thompson, D.J.; Lurcock, D.E.J.; Toward, M.G.R.; Ntotsios, E. A 2.5D finite element and boundary element model for the ground vibration from trains in tunnels and validation using measurement data. J. Sound Vib. 2018, 422, 373–389. [Google Scholar] [CrossRef] [Green Version]
- Liu, X.; Xiang, P.; Jiang, L.; Lai, Z.; Zhou, T.; Chen, Y. Stochastic Analysis of Train–Bridge System Using the Karhunen–Loéve Expansion and the Point Estimate Method. Int. J. Str. Stab. Dyn. 2020, 20, 2050025. [Google Scholar] [CrossRef]
- Liu, X.; Jiang, L.; Lai, Z.; Xiang, P.; Chen, Y. Sensitivity and dynamic analysis of train-bridge coupled system with multiple random factors. Eng. Struct. 2020, 221, 111083. [Google Scholar] [CrossRef]
- Liu, X.; Jiang, L.; Xiang, P.; Lai, Z.; Feng, Y.; Cao, S. Dynamic response limit of high-speed railway bridge under earthquake considering running safety performance of train. J. Cent. South Univ. 2021, 28, 968–980. [Google Scholar] [CrossRef]
- Liu, X.; Jiang, L.; Xiang, P.; Jiang, L.; Lai, Z. Safety and comfort assessment of a train passing over an earthquake-damaged bridge based on a probability model. Struct. Infrastruct. Eng. 2021, 1–12. [Google Scholar] [CrossRef]
- Lai, Z.; Jiang, L.; Zhou, W.; Yu, J.; Zhang, Y.; Liu, X.; Zhou, W. Lateral girder displacement effect on the safety and comfortability of the high-speed rail train operation. Veh. Syst. Dyn. 2021, 1–25. [Google Scholar] [CrossRef]
- Zhu, S.; Cai, C.; Zhai, W. Interface damage assessment of railway slab track based on reliability techniques and vehicle-track interactions. J. Transp. Eng. 2016, 142, 04016041. [Google Scholar] [CrossRef]
- Xu, L.; Liu, H.; Yu, Z. A coupled model for investigating the interfacial and fatigue damage evolution of slab tracks in vehicle-track interaction. Appl. Math. Model. 2022, 101, 772–790. [Google Scholar] [CrossRef]
- Wang, C.; Zhang, J.; Tu, Y.; Sabourova, N.; Grip, N.; Blanksvärd, T.; Elfgren, L. Fatigue assessment of a reinforced concrete railway bridge based on a coupled dynamic system. Struct. Infrastruct. Eng. 2020, 16, 861–879. [Google Scholar] [CrossRef]
- Zhao, L.; Zhou, L.; Yu, Z.; Mahunon, A.D.; Peng, X.; Zhang, Y. Experimental study on CRTS II ballastless track-bridge structural system mechanical fatigue performance. Eng. Struct. 2021, 244, 112784. [Google Scholar] [CrossRef]
- Xu, L.; Liu, X. Matrix coupled model for the vehicle–track interaction analysis featured to the railway crossing. Mech. Syst. Signal Process. 2021, 152, 107485. [Google Scholar] [CrossRef]
- Li, Y.; Chen, J.; Wang, J.; Shi, X.; Chen, L. Analysis of damage of joints in CRTSII Slab track under temperature and vehicle loads. KSCE J. Civ. Eng. 2020, 24, 1209–1218. [Google Scholar] [CrossRef]
- Yuan, Z.; Zhu, S.; Yuan, X.; Zhai, W. Vibration-based damage detection of rail fastener clip using convolutional neural network: Experiment and simulation. Eng. Fail. Anal. 2021, 119, 104906. [Google Scholar] [CrossRef]
- Kang, C.; Schneider, S.; Wenner, M.; Marx, S. Experimental investigation on rail fatigue resistance of track/bridge interaction. Eng. Struct. 2020, 216, 110747. [Google Scholar] [CrossRef]
- Kang, C.; Wenner, M.; Marx, S. Experimental investigation on the rail residual stress distribution and its influence on the bending fatigue resistance of rails. Constr. Build. Mater. 2021, 284, 122856. [Google Scholar] [CrossRef]
- Masoudi Nejad, R.; Liu, Z.; Ma, W.; Berto, F. Fatigue reliability assessment of a pearlitic Grade 900A rail steel subjected to multiple cracks. Eng. Fail. Anal. 2021, 128, 105625. [Google Scholar] [CrossRef]
- Zeng, Q.; Dimitrakopoulos, E.G. Vehicle–bridge interaction analysis modeling derailment during earthquakes. Nonlinear Dyn. 2018, 93, 2315–2337. [Google Scholar] [CrossRef]
- Liu, X.; Jiang, L.; Xiang, P.; Feng, Y.; Lai, Z.; Sun, X. Evaluation of optimal ground motion intensity measures of high-speed railway train running safety on bridges during earthquakes. Struct. Eng. Mech. 2022, 81, 219–230. [Google Scholar]
- Mao, J.; Yu, Z.; Xiao, Y.; Jin, C.; Bai, Y. Random dynamic analysis of a train-bridge coupled system involving random system parameters based on probability density evolution method. Probabilistic Eng. Mech. 2016, 46, 48–61. [Google Scholar] [CrossRef]
- Zeng, Z.-P.; Zhao, Y.-G.; Xu, W.-T.; Yu, Z.-W.; Chen, L.-K.; Lou, P. Random vibration analysis of train–bridge under track irregularities and traveling seismic waves using train–slab track–bridge interaction model. J. Sound Vib. 2015, 342, 22–43. [Google Scholar] [CrossRef]
- Lou, P.; Zeng, Q. Formulation of equations of motion of finite element form for vehicle-track-bridge interaction system with two types of vehicle model. Int. J. Numer. Meth. Engng. 2005, 62, 435–474. [Google Scholar] [CrossRef]
- Zhai, W. Vehicle-Track Coupled Dynamic, 4th ed.; Science Press: Beijing, China, 2017. (In Chinese) [Google Scholar]
- Dinh, V.N.; Kim, K.D.; Warnitchai, P. Dynamic analysis of three-dimensional bridge–high-speed train interactions using a wheel–rail contact model. Eng. Struct. 2009, 31, 3090–3106. [Google Scholar] [CrossRef]
- Liu, X.; Jiang, L.; Xiang, P.; Lai, Z.; Liu, L.; Cao, S.; Zhou, W. Probability analysis of train-bridge coupled system considering track irregularities and parameter uncertainty. Mech. Based Des. Struct. Mach. 2021, 1–18. [Google Scholar] [CrossRef]
- He, D.; Xiang, J.; Zeng, Q. A new method for dynamics modeling of ballastless track. J. Cent. South Univ. 2007, 38, 1206–1211. (In Chinese) [Google Scholar]


| Parameter | Symbol | Unit | Value |
|---|---|---|---|
| Mass of car body | mc | kg | 48,800 |
| Mass of bogie | mt | kg | 2310 |
| Mass of wheel set | mw | kg | 2080 |
| Moment of inertia of vehicle body around X-axis | Jcx | kg·m2 | 159,300 |
| Moment of inertia of vehicle body around Y-axis | Jcy | kg·m2 | 2,352,100 |
| Moment of inertia of vehicle body around Z-axis | Jcz | kg·m2 | 2,033,900 |
| Moment of inertia of bogie around X-axis | Jtx | kg·m2 | 2080 |
| Moment of inertia of bogie around Y-axis | Jty | kg·m2 | 1405 |
| Moment of inertia of bogie around Z-axis | Jtz | kg·m2 | 2450 |
| Moment of inertia of wheel set around X-axis | Jwx | kg·m2 | 749 |
| Moment of inertia of wheel set around Y-axis | Jwy | kg·m2 | 81 |
| Moment of inertia of wheel set around Z-axis | Jwz | kg·m2 | 1026 |
| Distance between two wheel sets of the same bogie | 2d1 | m | 2.50 |
| Distance between two bogies of the same carriage | 2d2 | m | 15.7 |
| Vertical distance between vehicle body and secondary suspension | h1 | m | 0.569 |
| Vertical distance between secondary suspension and bogie | h2 | m | 0.39 |
| Vertical distance between primary suspension and wheel set | h3 | m | 0.075 |
| Horizontal distance between vertical springs of secondary suspension | 2b1 | m | 2.10 |
| Horizontal distance between vertical springs of primary suspension | 2b2 | m | 1.90 |
| Lateral stiffness of primary suspension spring | ky1 | N/m | 980,000 |
| Vertical stiffness of primary suspension spring | kz1 | N/m | 1,176,000 |
| Lateral stiffness of secondary suspension spring | ky2 | N/m | 165,000 |
| Vertical stiffness of secondary suspension spring | kz2 | N/m·s | 373,000 |
| Lateral stiffness of primary suspension damping | cy1 | N/m·s | 0 |
| Vertical stiffness of primary suspension damping | cz1 | N/m·s | 10,000 |
| Lateral stiffness of secondary suspension damping | cy2 | N/m·s | 25,000 |
| Vertical stiffness of secondary suspension damping | cz2 | N/m·s | 30,000 |
| Number | Case |
|---|---|
| Case 1 | Perfect |
| Case 2 | Single failure |
| Case 3 | Two consecutive failures |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhan, T.; Yao, Y.; Li, J.; Liu, X.; Feng, Y. Influence of Fastener Failure on Dynamic Performance of Subway Vehicle. Appl. Sci. 2022, 12, 6769. https://doi.org/10.3390/app12136769
Zhan T, Yao Y, Li J, Liu X, Feng Y. Influence of Fastener Failure on Dynamic Performance of Subway Vehicle. Applied Sciences. 2022; 12(13):6769. https://doi.org/10.3390/app12136769
Chicago/Turabian StyleZhan, Tao, Yuan Yao, Jinping Li, Xiang Liu, and Yulin Feng. 2022. "Influence of Fastener Failure on Dynamic Performance of Subway Vehicle" Applied Sciences 12, no. 13: 6769. https://doi.org/10.3390/app12136769
APA StyleZhan, T., Yao, Y., Li, J., Liu, X., & Feng, Y. (2022). Influence of Fastener Failure on Dynamic Performance of Subway Vehicle. Applied Sciences, 12(13), 6769. https://doi.org/10.3390/app12136769






