Reinforced Concrete Building with IED Detonation: Test and Simulation
Abstract
:1. Introduction
2. Test Description and Instrumentation
3. Numerical Model
3.1. Finite Element Model
3.2. Blast Implementation
3.3. Materials
4. Results and Discussion
4.1. High-Speed Camera
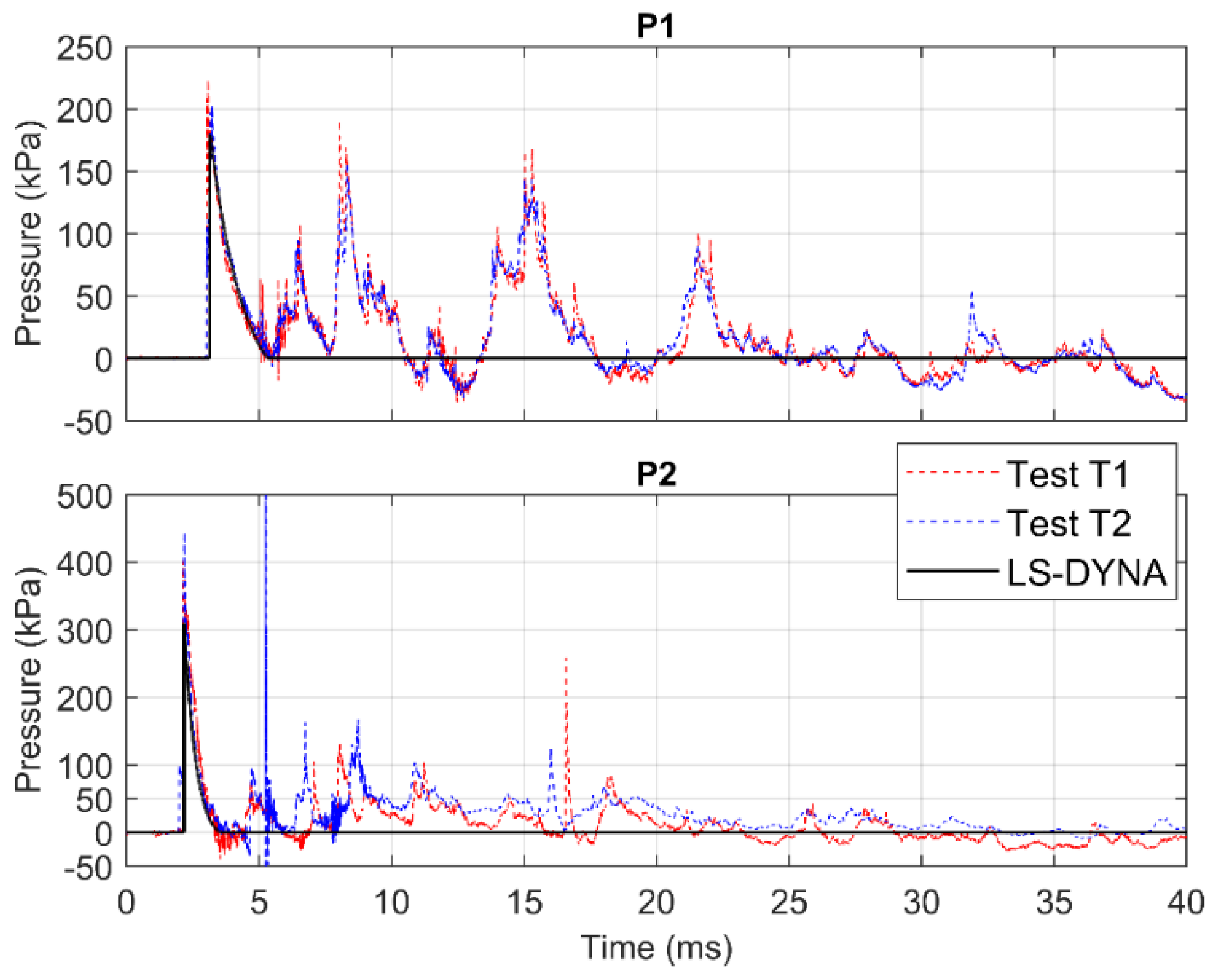
4.2. Pressure Signals
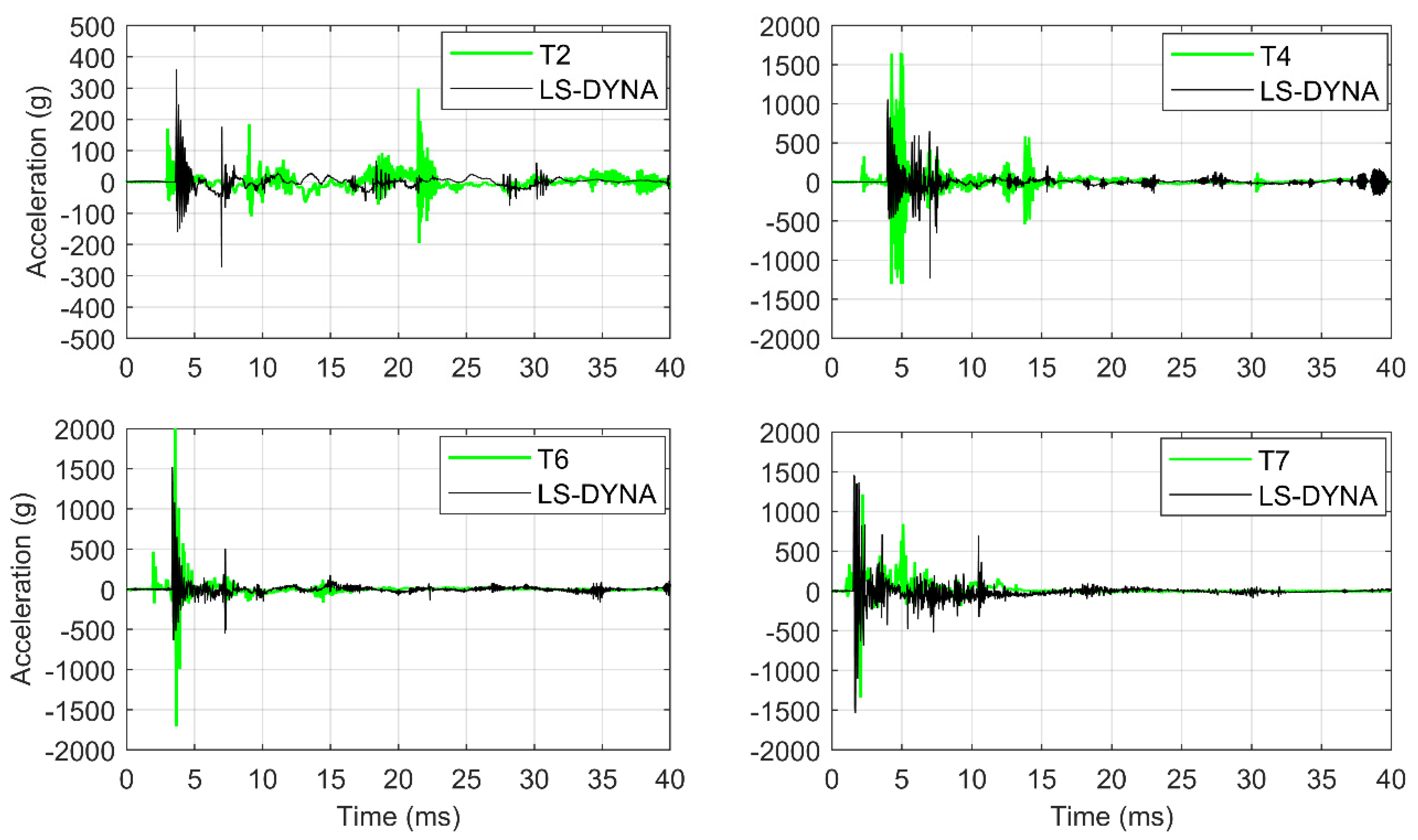
4.3. Acceleration Signals
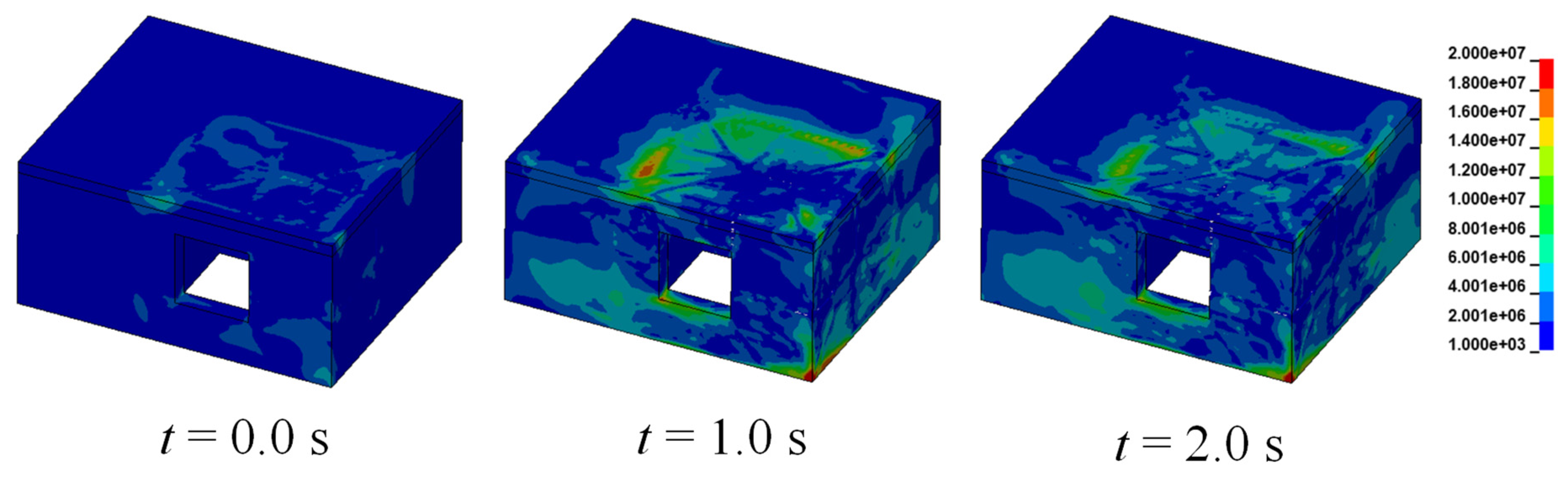
4.4. Final Test E8
4.5. Effects of the IED Type
5. Conclusions
- The high-speed camera images allow us to see the correct detonation of the explosive, while the pressure log allows us to validate the model input data.
- The acceleration recorded at the roof of the structure decreases as more tests are performed due to the loss of stiffness of the structure.
- IEDs of relatively low power (with homemade explosives or low-TNT equivalent), although they cause significant accelerations in the structure, do not compromise its structural stability, while more powerful IEDs (plastic explosives), although with similar accelerations, do put the structural stability of the building at risk.
- A solid element model using LBE offers, even in complex cases such as this one, a reasonable reproduction of the behavior of a structure reducing testing costs by being able to reproduce with some certainty different scenarios.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kamel, H. Review of design techniques of armored vehicles for protection against blast from improvised explosive devices. In Proceedings of the International Mechanical Engineering Congress and Exposition, Salt Lake City, UT, USA, 11–14 November 2019; Volume 10227, pp. 1–18. [Google Scholar]
- Fan, W.; Zhou, N.; Jiao, Q.; Shi, J.; Tang, K. Investigation on the explosive characteristics and damage mode of cylindrical improvised explosive devices. J. Appl. Mech. Tech. Phys. 2020, 61, 1024–1032. [Google Scholar] [CrossRef]
- Liu, H.; Huang, G.; Guo, Z.; Feng, S. Fragments Velocity Distribution and Estimating Method of Thin-Walled Cylindrical Improvised Explosive Devices with Different Length-to-Diameter Ratios. Thin-Walled Struct. 2022, 175, 109212. [Google Scholar] [CrossRef]
- Williams, D.S. Enhancing the Applicability of Blast Modelling and Advice. Int. J. Prot. Struct. 2015, 6, 701–710. [Google Scholar] [CrossRef]
- Price, M.A.; Nguyen, V.; Hassan, O.; Morgan, K. An Approach to Modeling Blast and Fragment Risks from Improvised Explosive Devices. Appl. Math. Model. 2017, 50, 715–731. [Google Scholar] [CrossRef] [Green Version]
- Sielicki, P.W.; Stewart, M.G.; Gajewski, T.; Peksa, P.; Al-rifaie, H.; Studzi, R. Field Test and Probabilistic Analysis of Irregular Steel Debris Casualty Risks from a Person-Borne Improvised Explosive Device. Def. Technol. 2021, 17, 1852–1863. [Google Scholar] [CrossRef]
- Draganić, H.; Gazić, G.; Varevac, D. Experimental Investigation of Design and Retrofit Methods for Blast Load Mitigation—A State-of-the-Art Review. Eng. Struct. 2019, 190, 189–209. [Google Scholar] [CrossRef]
- Goswami, A.; Adhikary, S. Das Retrofitting Materials for Enhanced Blast Performance of Structures: Recent Advancement and Challenges Ahead. Constr. Build. Mater. 2019, 204, 224–243. [Google Scholar] [CrossRef]
- Tai, Y.S.; Chu, T.L.; Hu, H.T.; Wu, J.Y. Dynamic Response of a Reinforced Concrete Slab Subjected to Air Blast Load. Theor. Appl. Fract. Mech. 2011, 56, 140–147. [Google Scholar] [CrossRef]
- Zhao, C.F.; Chen, J.Y.; Wang, Y.; Lu, S.J. Damage Mechanism and Response of Reinforced Concrete Containment Structure under Internal Blast Loading. Theor. Appl. Fract. Mech. 2012, 61, 12–20. [Google Scholar] [CrossRef]
- Lin, X.; Zhang, Y.X.; Hazell, P.J. Modelling the Response of Reinforced Concrete Panels under Blast Loading. J. Mater. 2014, 56, 620–628. [Google Scholar] [CrossRef]
- Jayasooriya, R.; Thambiratnam, D.P.; Perera, N.J.; Kosse, V. Blast and Residual Capacity Analysis of Reinforced Concrete Framed Buildings. Eng. Struct. 2011, 33, 3483–3495. [Google Scholar] [CrossRef]
- Zhang, D.; Yao, S.J.; Lu, F.; Chen, X.G.; Lin, G.; Wang, W.; Lin, Y. Experimental Study on Scaling of RC Beams under Close-in Blast Loading. Eng. Fail. Anal. 2013, 33, 497–504. [Google Scholar] [CrossRef]
- Codina, R.; Ambrosini, D.; Borbón, F. De Alternatives to Prevent the Failure of RC Members under Close-in Blast Loadings. EFA 2016, 60, 96–106. [Google Scholar] [CrossRef]
- Hajek, R.; Fladr, J.; Pachman, J.; Stoller, J.; Foglar, M. An Experimental Evaluation of the Blast Resistance of Heterogeneous Concrete-Based Composite Bridge Decks. Eng. Struct. 2019, 179, 204–210. [Google Scholar] [CrossRef]
- Zhao, C.; Lu, X.; Wang, Q.; Gautam, A.; Wang, J.; Mo, Y.L. Experimental and Numerical Investigation of Steel-Concrete (SC) Slabs under Contact Blast Loading. Eng. Struct. 2019, 196, 109337. [Google Scholar] [CrossRef]
- Reifarth, C.; Castedo, R.; Santos, A.P.; Chiquito, M.; López, L.M.; Pérez-Caldentey, A.; Martínez-Almajano, S.; Alañon, A. Numerical and experimental study of externally reinforced RC slabs using FRPs subjected to close-in blast loads. Int. J. Impact Eng. 2021, 156, 103939. [Google Scholar] [CrossRef]
- Shi, Y.; Li, Z.; Hao, H. A New Method for Progressive Collapse Analysis of RC Frames under Blast Loading. Eng. Struct. 2010, 32, 1691–1703. [Google Scholar] [CrossRef]
- Bermejo, M.; Santos, A.P.; Goicolea, J.M. Development of Practical Finite Element Models for Collapse of Reinforced Concrete Structures and Experimental Validation. Shock. Vib. 2017, 2017, 4636381. [Google Scholar] [CrossRef] [Green Version]
- Alshaikh, I.M.H.; Bakar, B.H.A.; Alwesabi, E.A.H.; Akil, H. Experimental Investigation of the Progressive Collapse of Reinforced Concrete Structures: An Overview. Structures 2020, 25, 881–900. [Google Scholar] [CrossRef]
- Kernicky, T.P.; Whelan, M.J.; Weggel, D.C.; Rice, C.D. Structural Identification and Damage Characterization of a Masonry Infill Wall in a Full-Scale Building Subjected to Internal Blast Load. J. Struct. Eng. 2015, 141, D4014013. [Google Scholar] [CrossRef]
- Xiao, W.; Andrae, M.; Steyerer, M.; Gebbeken, N. Investigations of Blast Loads on a Two-Storeyed Building with a Gable Roof: Full-Scale Experiments and Numerical Study. J. Build. Eng. 2021, 43, 103111. [Google Scholar] [CrossRef]
- Stewart, M.G.; Mueller, J. Terrorism Risks, Chasing Ghosts and Infrastructure Resilience. Sustain. Resilient Infrastruct. 2020, 5, 78–89. [Google Scholar] [CrossRef]
- Dante, D.; William, Y. Primary Blast Injuries—An Updated Concise Review. World J. Surg. 2012, 36, 966–972. [Google Scholar] [CrossRef]
- Mathews, Z.R.; Koyfman, A. Blast Injuries. J. Emerg. Med. 2015, 49, 573–587. [Google Scholar] [CrossRef]
- EN 1992-1-1; Eurocode 2: Design of Concrete Structures—Part 1-1: General Rules and Rules for Buildings. European Committee for Standardization: Brussels, Belgium, 2004.
- EN 1998-2; Eurocode 8: Design of Structures for Earthquake Resistance—Part 2: Bridges. European Committee for Standardization: Brussels, Belgium, 2005.
- Livermore Software Technology Corporation (LSTC). LS-DYNA Keyword User´s Manual—R11; Livermore Software Technology Corporation: Livermore, CA, USA, 2018; p. 3186. [Google Scholar]
- Castedo, R.; Segarra, P.; Alañon, A.; Lopez, L.M.; Santos, A.P.; Sanchidrian, J.A. Air Blast Resistance of Full-Scale Slabs with Different Compositions: Numerical Modeling and Field Validation. Int. J. Impact Eng. 2015, 86, 145–156. [Google Scholar] [CrossRef]
- Castedo, R.; Santos, A.P.; Alañón, A.; Reifarth, C.; Chiquito, M.; López, L.M.; Martínez-Almajano, S.; Pérez-Caldentey, A. Numerical Study and Experimental Tests on Full-Scale RC Slabs under Close-in Explosions. Eng. Struct. 2021, 231, 111774. [Google Scholar] [CrossRef]
- Alañón, A.; Cerro-Prada, E.; Vázquez-Gallo, M.J.; Santos, A.P. Mesh Size Effect on Finite-Element Modeling of Blast-Loaded Reinforced Concrete Slab. Eng. Comput. 2018, 34, 649–658. [Google Scholar] [CrossRef]
- Feng, W.; Chen, B.; Yang, F.; Liu, F.; Li, L.; Jing, L.; Li, H. Numerical Study on Blast Responses of Rubberized Concrete Slabs Using the Karagozian and Case Concrete Model. J. Build. Eng. 2021, 33, 101610. [Google Scholar] [CrossRef]
- Abedini, M.; Zhang, C.; Mehrmashhadi, J.; Akhlaghi, E. Comparison of ALE, LBE and Pressure Time History Methods to Evaluate Extreme Loading Effects in RC Column. Structures 2020, 28, 456–466. [Google Scholar] [CrossRef]
- Suhaimi, K.; Sohaimi, R.M.; Knight, V.F.; Sheng, T.K.; Ahmad, M.M.H.M.; Isa, M.F.M.; Sohaimi, A.S.M.; Noordin, M.N.H.; Syaharani, A. Simulation of Hybrid-III Dummy Response Using Three LS-DYNA Blast Methods. Def. S T Tech. Bull. 2017, 10, 111–120. [Google Scholar]
- Hilding, D. Methods for Modelling Air Blast on Structures in LS-DYNA. In Proceedings of the Nordic LS-DYNA Users’ Conference, Gothenburg, Sweden, 13–14 October 2016; p. 65. [Google Scholar]
- Hyde, D.W. Microcomputer Programs CONWEP and FUNPRO, Applications of TM 5-855-1, ’Fundamentals of Protective Design for Conventional Weapons’(User’s Guide); No. WES/IR; Army Engineer Waterways Experiment Station Vicksburg MS Structures Lab.: Vicksburg, MS, USA, 1988. [Google Scholar]
- Abedini, M.; Zhang, C. Performance Assessment of Concrete and Steel Material Models in LS-DYNA for Enhanced Numerical Simulation, A State of the Art Review. Arch. Comput. Methods Eng. 2021, 28, 2921–2942. [Google Scholar] [CrossRef]
- Tabatabaei, Z.S.; Volz, J.S.; Baird, J.; Gliha, B.P.; Keener, D.I. Experimental and Numerical Analyses of Long Carbon Fiber Reinforced Concrete Panels Exposed to Blast Loading. Int. J. Impact Eng. 2013, 57, 70–80. [Google Scholar] [CrossRef]
- Brad, D.; Michael, O.; Joseph, M. Modeling Reinforced Concrete Protective Construction for Impact Scenarios. In Proceedings of the International Explosives Safety Symposium and Exhibition, San Diego, CA, USA, 6–9 August 2018; p. 298. [Google Scholar]
- Murray, Y.D. Users Manual for LS-DYNA Concrete Material Model 159; Federal Highway Administration: Washington, DC, USA, 2007. [Google Scholar]
- Brannon, R.M.; Leelavanichkul, S. Survey of Four Damage Models for Concrete; Sandia National Laboratories: Albuquerque, NM, USA, 2009. [Google Scholar]
- Cadoni, E.; Dotta, M.; Forni, D.; Tesio, N. High Strain Rate Behaviour in Tension of Steel B500A Reinforcing Bar. Mater. Struct. Constr. 2015, 48, 1803–1813. [Google Scholar] [CrossRef]
- Chiquito, M.; Castedo, R.; Lopez, L.M.; Santos, A.P.; Mancilla, J.M.; Yenes, J.I. Blast Wave Characteristics and TNT Equivalent of Improvised Explosive Device at Small Scaled Distances. Def. Sci. J. 2019, 69, 328–335. [Google Scholar] [CrossRef] [Green Version]











| Test | Day | Explosive Type | Charge (kg) | PETN (g) | TNT Equivalent Mass (kg) | Confinement |
|---|---|---|---|---|---|---|
| T0 | 18 September 2018 | PG-2 | 0.10 | 0 | 0.14 | -- |
| T1 | 18 September 2018 | Black Powder | 3.37 | 10.5 | 0.79 | Steel tubes |
| T2 | 18 September 2018 | Black Powder | 3.27 | 10.5 | 0.77 | Steel tubes |
| T3 | 18 September 2018 | ANFO | 2.29 | 10.5 | 1.48 | Steel tubes |
| T4 | 19 September 2018 | ANFO | 2.20 | 10.5 | 1.42 | Steel tubes |
| T5 | 19 September 2018 | AN/AL | 2.16 | 10.5 | 1.88 | Steel tubes |
| T6 | 19 September 2018 | AN/AL | 2.25 | 10.5 | 1.95 | Steel tubes |
| T7 | 20 September 2018 | PG-2 | 7.00 | 55 | 9.87 | Vest |
| T8 | 20 September 2018 | PG-2 | 8.20 | 55 | 14.21 | Vest |
| #Sensor | Model | Measurement Range (g) | Test | Height (m) |
|---|---|---|---|---|
| A1 | 350C23 | ±10,000 | T0–T4 | 1.375 |
| A1 | 350C04 | ±5000 | T5–T7 | 1.375 |
| A2 | 350C04 | ±5000 | T0–T7 | 1.370 |
| A3 | 350C23 | ±10,000 | T0–T7 | 1.395 |
| A4 | 350B04 | ±5000 | T0–T7 | 1.370 |
| A5 | 350B04 | ±5000 | T0–T7 | 3.300 |
| Property | Concrete | Steel |
|---|---|---|
| Density (kg/m3) | 2300 | 7850 |
| Uniaxial compressive strength (MPa) | 40 | - |
| Maximum aggregate size (m) | 0.02 | - |
| Young modulus (MPa) | - | 2 × 105 |
| Poisson’s ratio | - | 0.3 |
| Yield stress (MPa) | - | 5 × 102 |
| Tangent modulus (MPa) | - | 2 × 104 |
| Test | Explosive | Sensor | Pr (kPa) | Pr (LS-DYNA) (kPa) | Relative Dif. (%) |
|---|---|---|---|---|---|
| T0 | PG-2 | P1 | 88.72 | 99.67 | −12.34 |
| T0 | PG-2 | P2 | 129.80 | 136.02 | −4.79 |
| T1 | Black Powder | P1 | 193.11 | 181.21 | 6.71 |
| T2 | Black Powder | P1 | 195.38 | ||
| T1 | Black Powder | P2 | 372.27 | 309.64 | 17.93 |
| T2 | Black Powder | P2 | 382.36 |
| Test | Explosive | #Sensor | Measured Acceleration (g) | Model Acceleration (g) | Difference (%) |
|---|---|---|---|---|---|
| T0 | PG2 | A2 | 44.4 | 26.3 | 40.77 |
| A3 | 77.1 | 57.8 | 25.03 | ||
| A4 | 29.6 | 30.2 | −1.94 | ||
| T1 | BP | A1 | 207.2 | 152.8 | 26.25 |
| A3 | 699.3 | 416.5 | 40.44 | ||
| A4 | 69.2 | 79.9 | −15.49 | ||
| T2 | BP | A1 | 105.2 | 75.4 | 28.30 |
| A3 | 430.3 | 403.6 | 6.21 | ||
| A5 | 298.8 | 354.8 | −18.76 | ||
| T3 | ANFO | A1 | 1048.9 | 852.4 | 18.73 |
| T4 | ANFO | A1 | 1113.6 | 928.3 | 16.64 |
| A3 | 1048.2 | 809.9 | 22.74 | ||
| A4 | 259.8 | 349.4 | −34.50 | ||
| A5 | 1646.4 | 1056.0 | 35.86 | ||
| T5 | AN/AL | A1 | 998.9 | 1115.2 | −11.64 |
| A3 | 3786.5 | 4192.0 | −10.71 | ||
| A4 | 4685.5 | 4896.0 | −4.49 | ||
| A5 | 2683.10 | 1691.5 | 36.96 | ||
| T6 | AN/AL | A1 | 903.4 | 1126.5 | −28.68 |
| A3 | 5600.6 | 4305.0 | 23.13 | ||
| A4 | 4828.6 | 5094.0 | −5.50 | ||
| A5 | 1996.25 | 1518.4 | 23.94 | ||
| T7 | PG2 | A1 | 902.7 | 652.0 | 27.77 |
| A3 | 1507.90 | 1521.8 | 3.12 | ||
| A4 | 5171.8 | 3464.4 | 33.01 | ||
| A5 | 1342.3 | 1505.0 | −12.12 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Santos, A.P.; Castedo, R.; López, L.M.; Chiquito, M.; Yenes, J.I.; Alañón, A.; Costamagna, E.; Martínez-Almajano, S. Reinforced Concrete Building with IED Detonation: Test and Simulation. Appl. Sci. 2022, 12, 7803. https://doi.org/10.3390/app12157803
Santos AP, Castedo R, López LM, Chiquito M, Yenes JI, Alañón A, Costamagna E, Martínez-Almajano S. Reinforced Concrete Building with IED Detonation: Test and Simulation. Applied Sciences. 2022; 12(15):7803. https://doi.org/10.3390/app12157803
Chicago/Turabian StyleSantos, Anastasio P., Ricardo Castedo, Lina M. López, María Chiquito, José I. Yenes, Alejandro Alañón, Elisa Costamagna, and Santiago Martínez-Almajano. 2022. "Reinforced Concrete Building with IED Detonation: Test and Simulation" Applied Sciences 12, no. 15: 7803. https://doi.org/10.3390/app12157803
APA StyleSantos, A. P., Castedo, R., López, L. M., Chiquito, M., Yenes, J. I., Alañón, A., Costamagna, E., & Martínez-Almajano, S. (2022). Reinforced Concrete Building with IED Detonation: Test and Simulation. Applied Sciences, 12(15), 7803. https://doi.org/10.3390/app12157803










