Analysis of Resonance Transition Periodic Orbits in the Circular Restricted Three-Body Problem
Abstract
:1. Introduction
2. Model Framework
2.1. The CRTBP Model
2.2. Periodic Orbits in the CRTBP Model
3. The First-Order Resonant Periodic Families
3.1. The Unperturbed Two-Body Model:
3.2. The CRTBP Model:
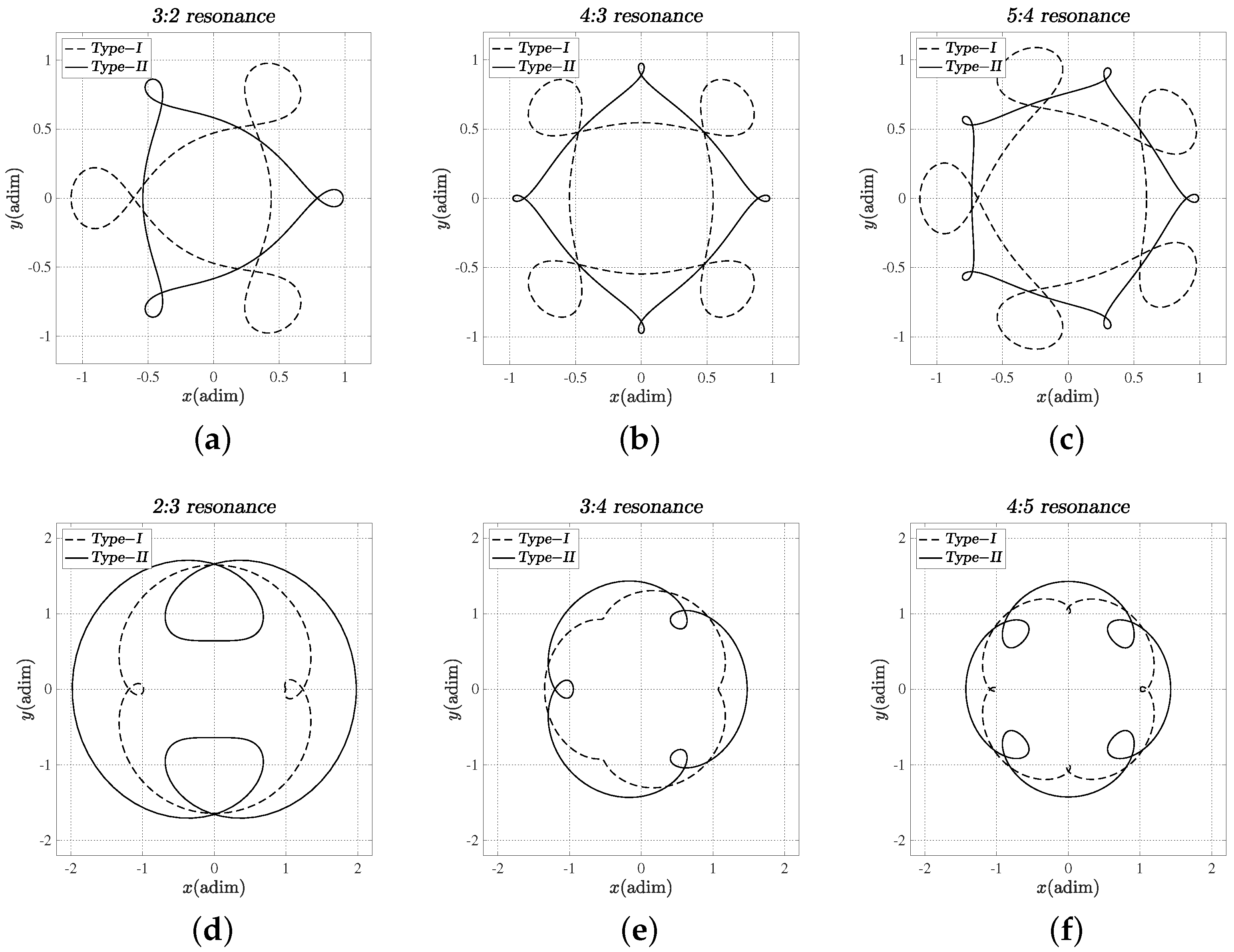
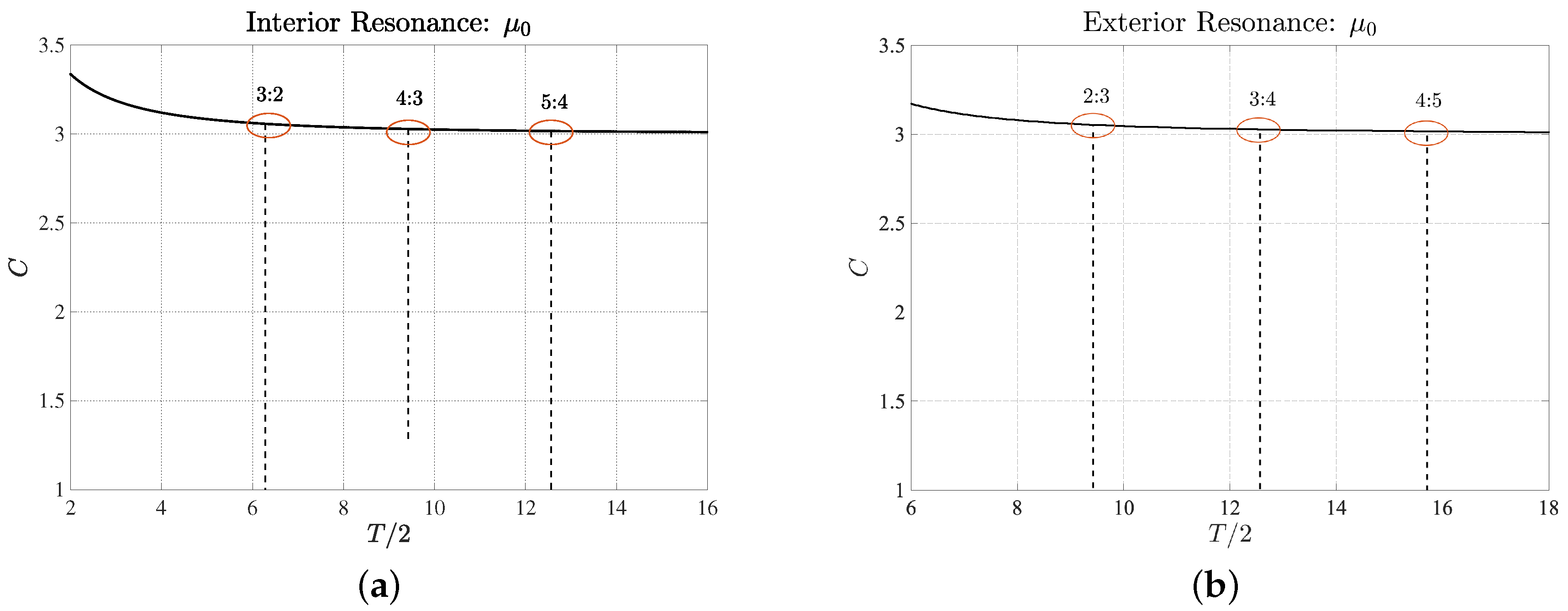
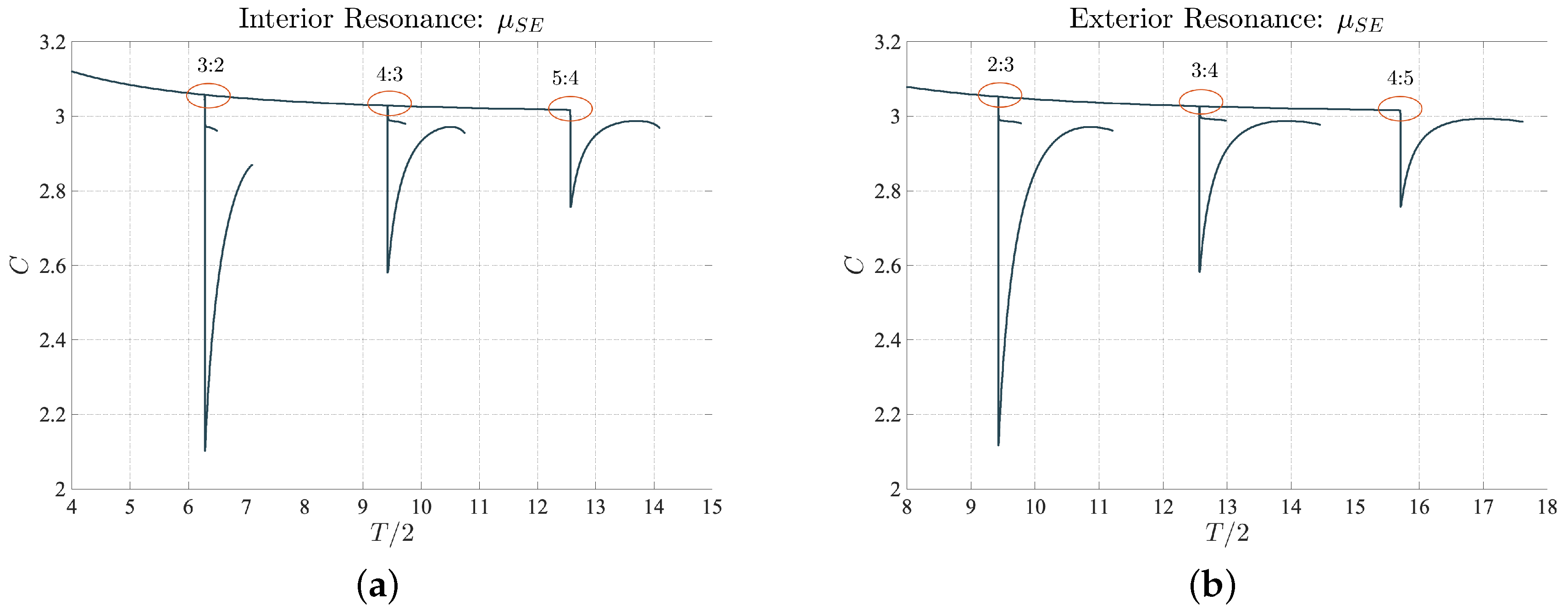
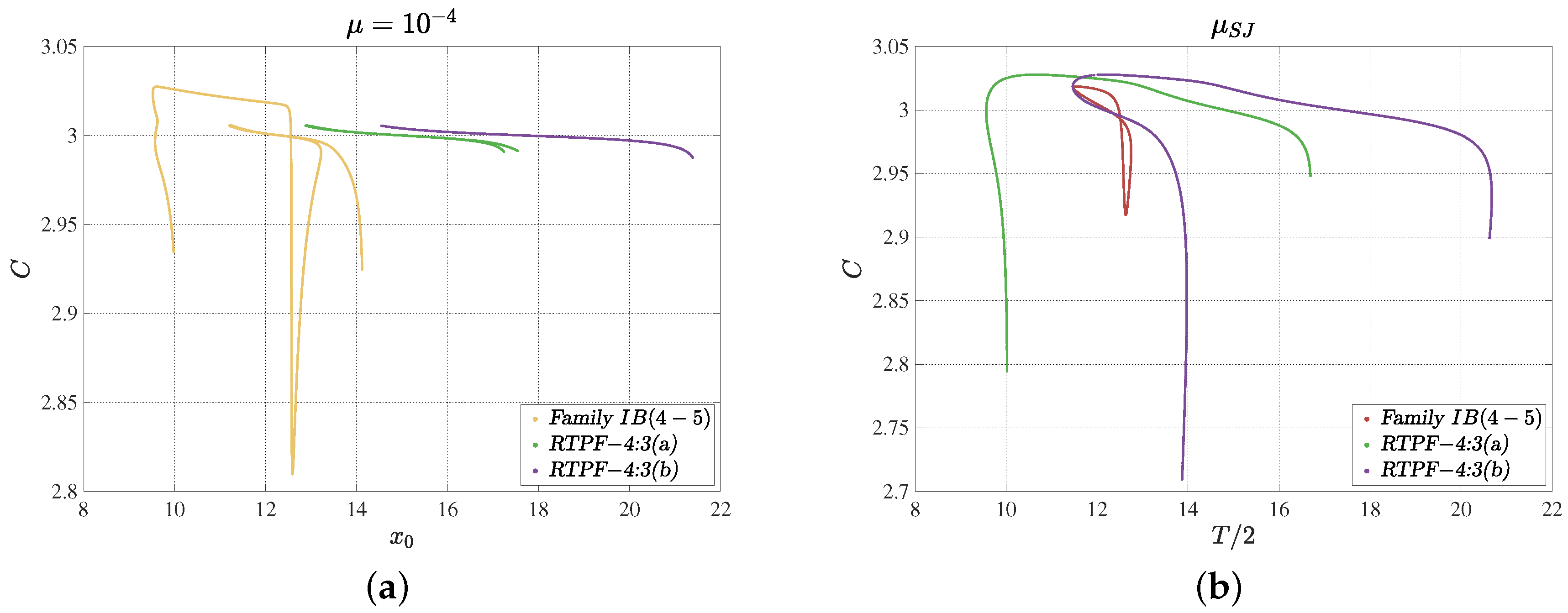
4. Resonance Transition Periodic Families in the CRTBP Model
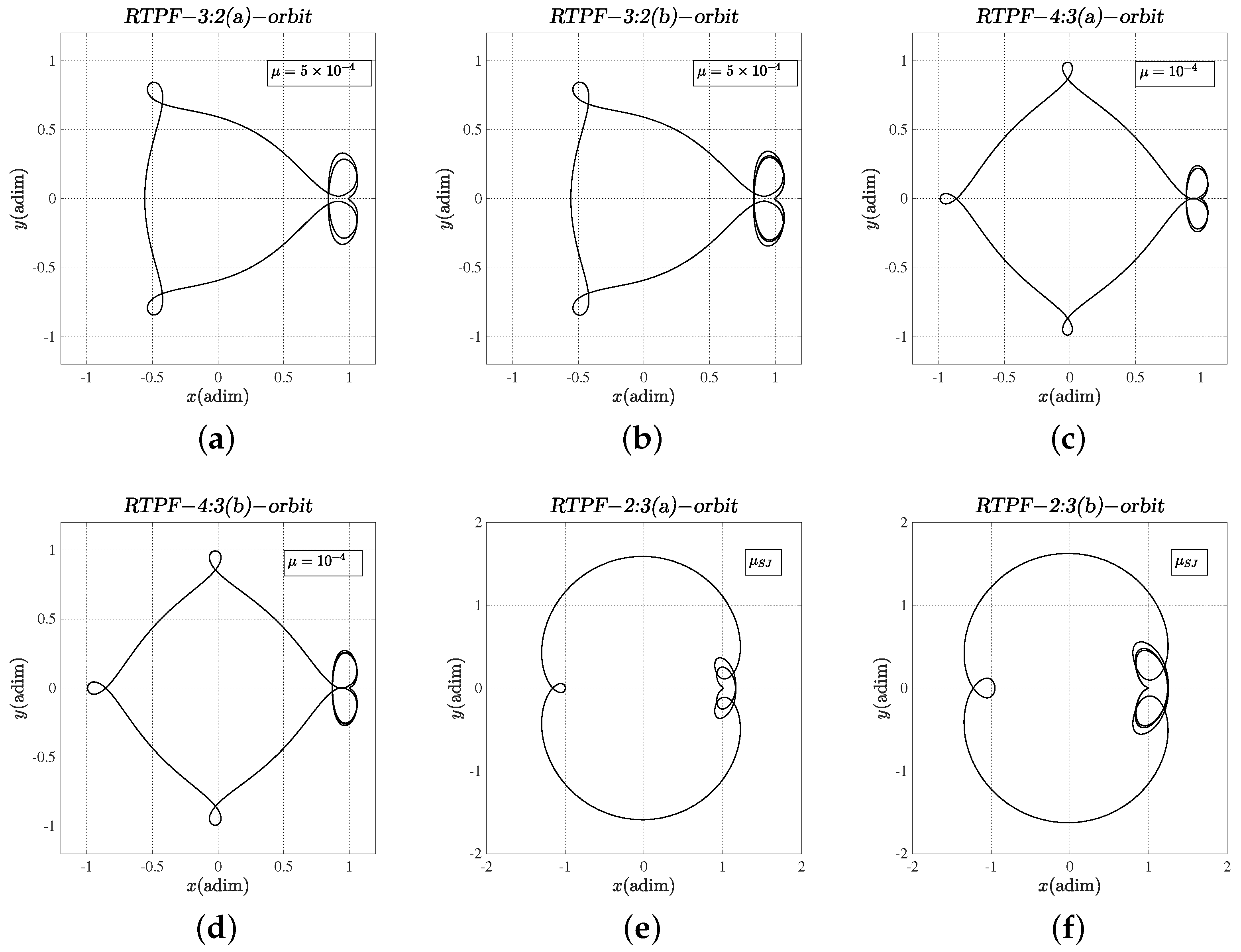
4.1. The RTPFs Related with the 3:2 Resonance
4.2. The RTPFs Related with the 4:3 Resonance
4.3. The RTPFs Related with the 2:3 Resonance
4.4. Summation
5. Computation of the Symmetric RTPOs
5.1. Methodology
- Firstly, we restrict the starting position on the Lyapunov orbit around the collinear or point in the Sun–Jupiter system and extend the method in the previous study [35] to the Sun–Jupiter system. The amplitude of the Lyapunov orbit is defined as the distance between its right intersection point (see the yellow dot in Figure 12) with the x-axis and the or the point. In our work, it is fixed at 0.05 (dimensionless unit) of the Sun–Jupiter distance. One remark is that a different value can be chosen. Initial states of the planar Lyapunov orbit are denoted as . The Lyapunov orbit is symmetric about the x-axis. The initial position is placed on the positive x-axis and the initial velocity is exactly along the positive y-direction. Therefore, the initial state of the Lyapunov orbit is ;
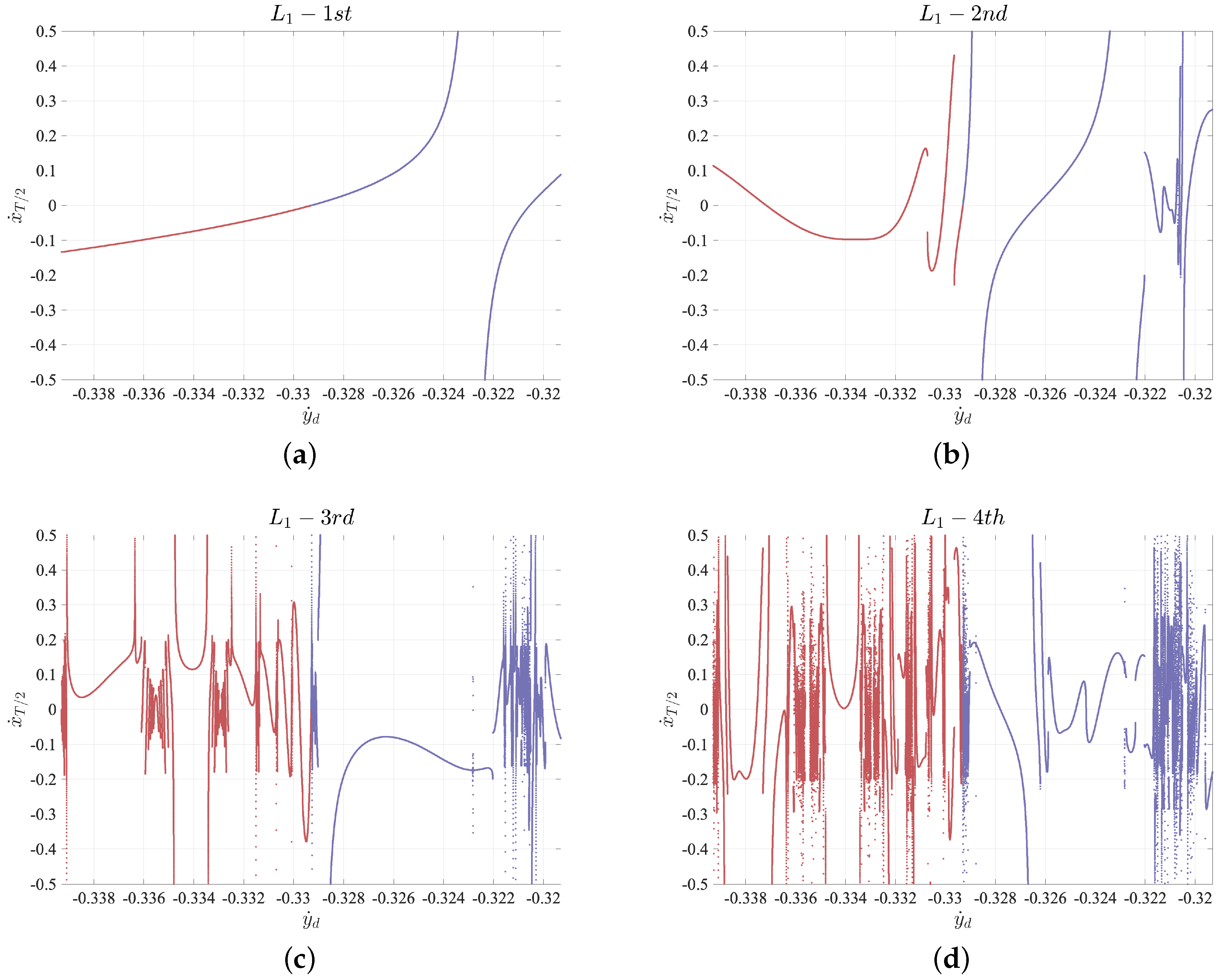
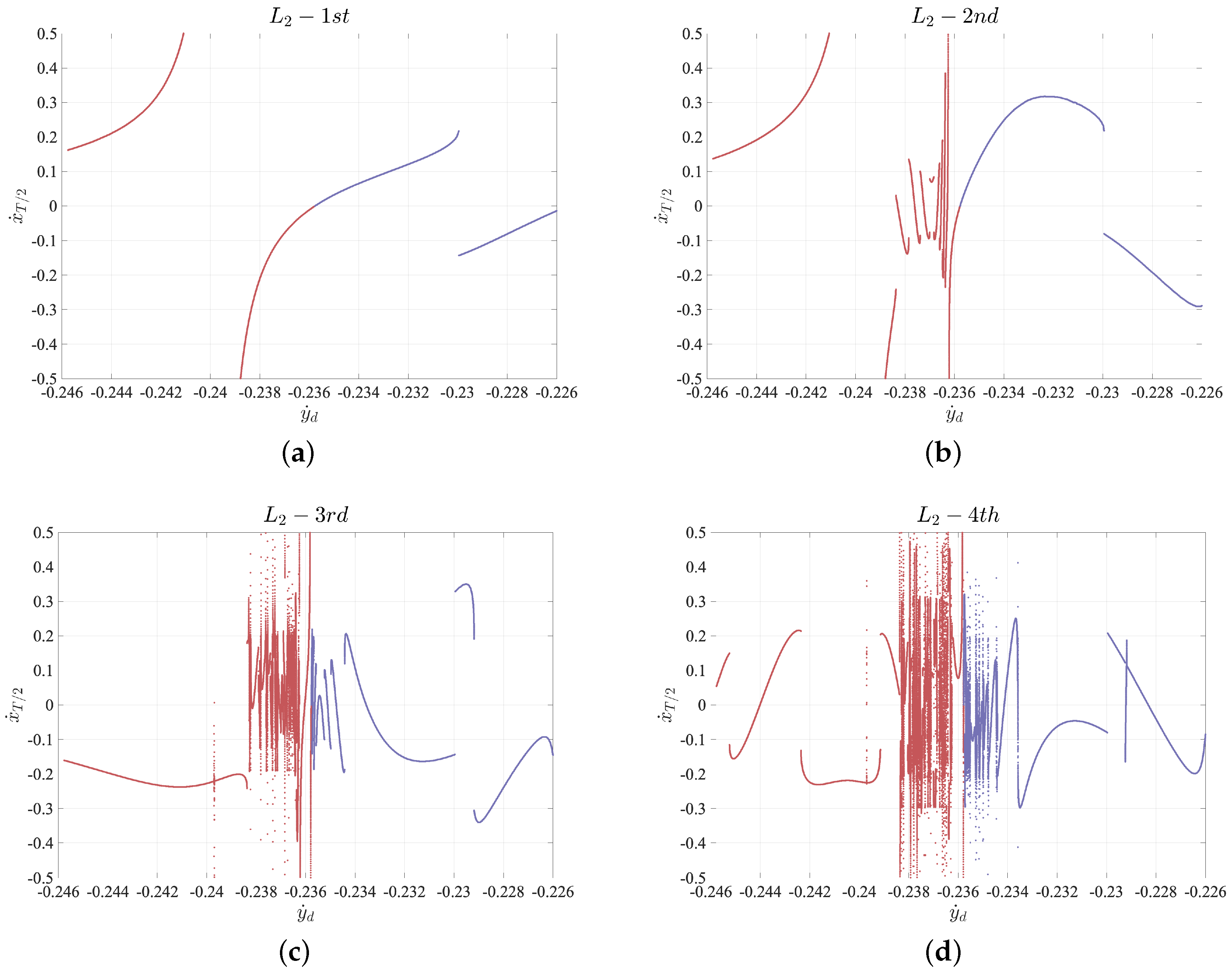
- We add a tiny perturbation along the direction of initial velocity on the Lyapunov orbit. The velocity change is in the range of [−0.01, 0.01] in this study, i.e., the departure velocity at the initial position is restricted to be within [−0.01 + , 0.01 + ], where 0.01 is a dimensionless velocity in the Sun–Jupiter system. Due to the linear stability of the collinear libration point, the orbit can leave the vicinity of the collinear libration point with this tiny perturbation to the initial velocity. The schematic diagram of generating initials of the RTPOs is displayed in Figure 12;
- By integration, the trajectory leaves the initial position on the x-axis. If the initial velocity is appropriate, it crosses the x-axis again one or more times after some integration time. Generally, at the new intersection point (see the green point in Figure 12), the velocity is not perpendicular to the x-axis. We record the x component of the velocity at the new intersection point and denote it as . For a different value of the departure velocity , the value of is different. By varying the values of and the times of the trajectory intersects with the x-axis after departure, the relationships between and are obtained;
- The relationships between and are presented in Figure 13 (cases for ) and Figure 14 (cases for ). According to the well-known symmetry property of the CRTBP model [26], if , the trajectory from to is half of a periodic orbit. From Figure 13 and Figure 14, we can see that there exist some dots satisfying within a certain tolerance, which means that we can use these initial states where to generate the periodic orbits, especially for the RTPOs. After finding these initial states , we can use the Newton iteration method to find the exact initial velocity that makes . The same algorithm has been used to generate the symmetric horseshoe periodic orbits in the CRTBP model [33,36].
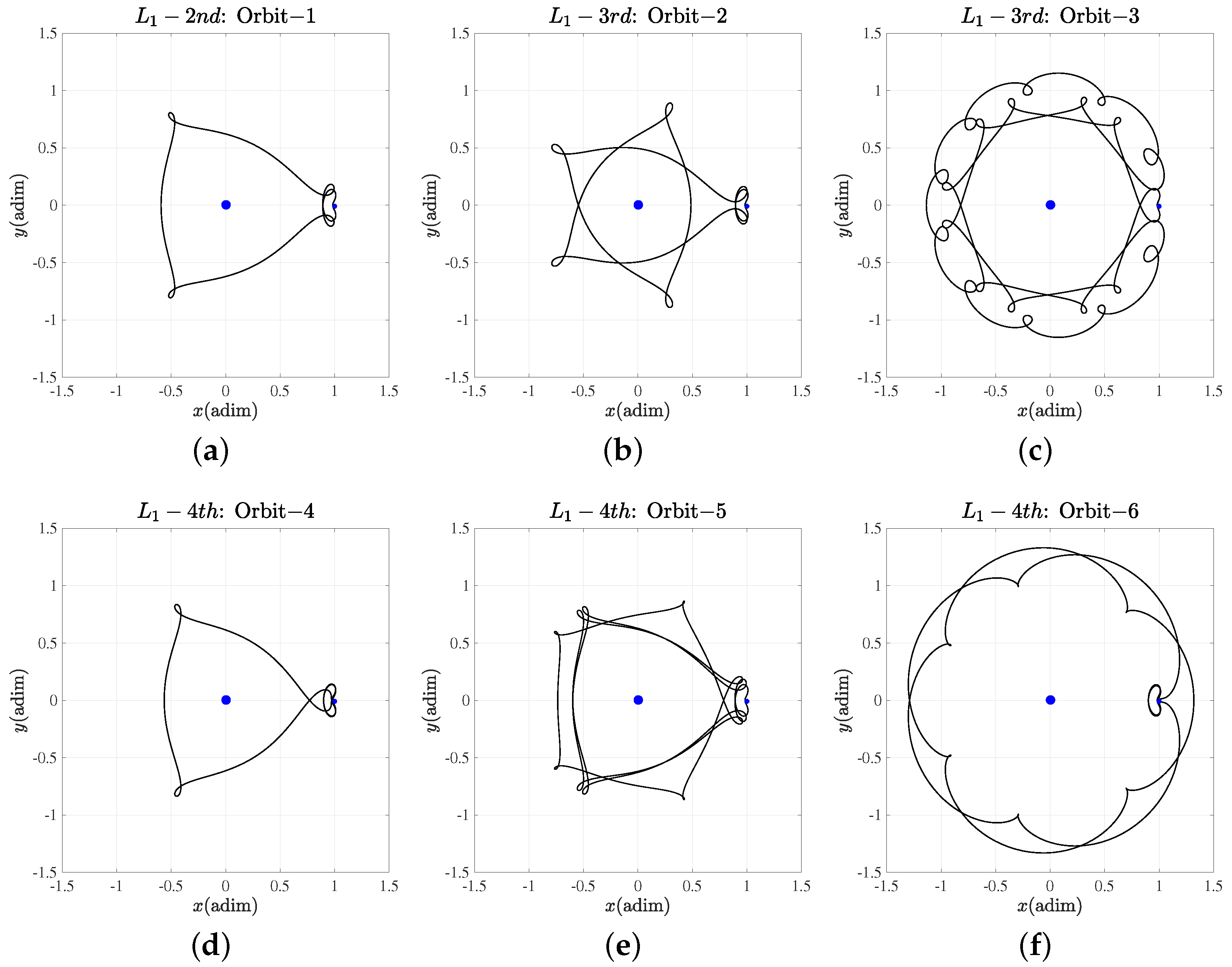
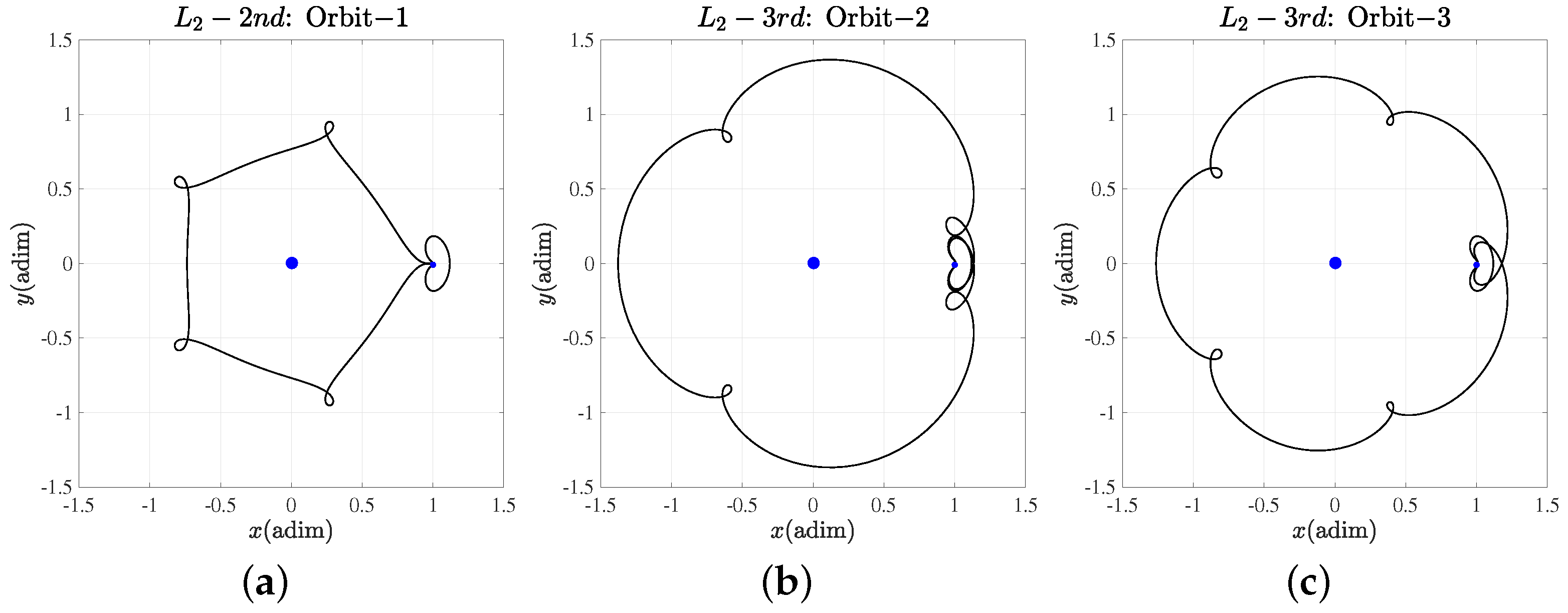
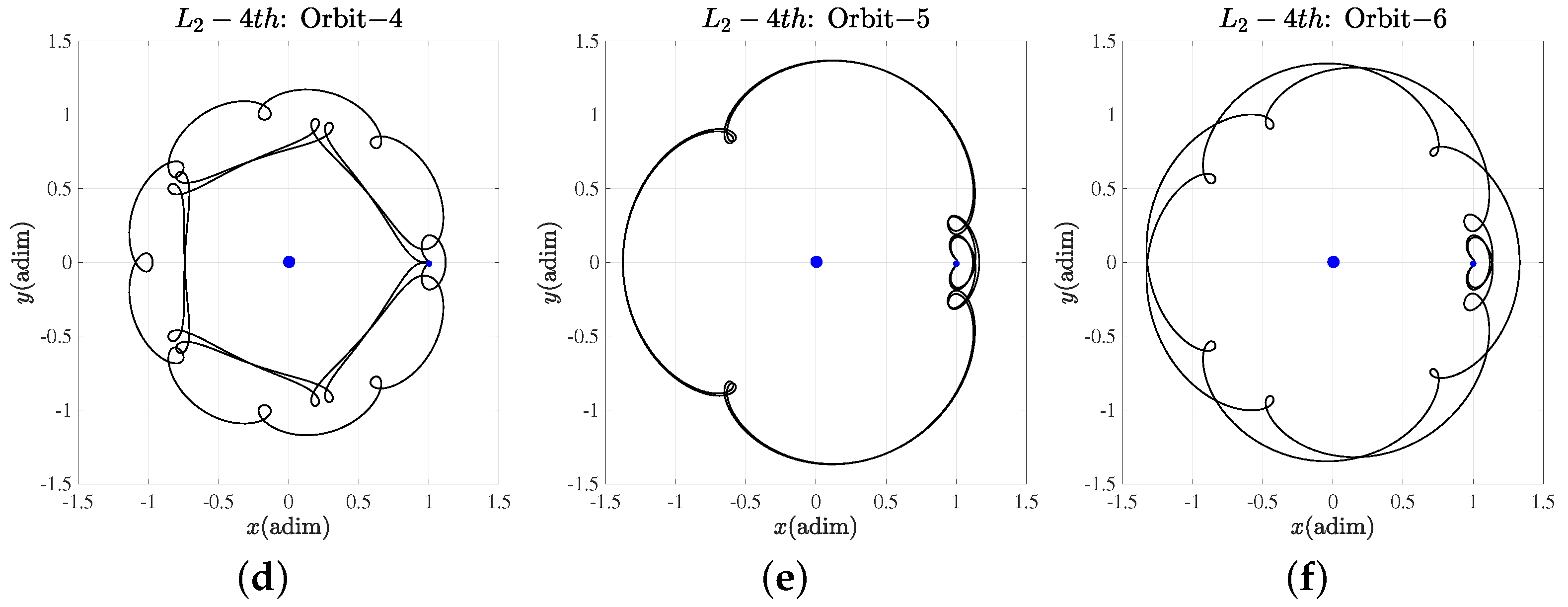
5.2. Results
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Rivera, E.J.; Laughlin, G.; Butler, R.P.; Vogt, S.S.; Haghighipour, N.; Meschiari, S. The Lick-Carnegie Exoplanet Survey: A Uranus-Mass Fourth Planet for GJ 876 in an Extrasolar Laplace Configuration. Astrophys. J. 2010, 719, 890–899. [Google Scholar] [CrossRef]
- MacDonald, M.G.; Ragozzine, D.; Fabrycky, D.C.; Ford, E.B.; Holman, M.J.; Isaacson, H.T.; Lissauer, J.J.; Lopez, E.D.; Mazeh, T.; Rogers, L.; et al. A Dynamical Analysis of the Kepler-80 System of Five Transiting Planets. Astron. J. 2016, 152, 105. [Google Scholar] [CrossRef]
- Hadjidemetriou, J.D. Periodic Orbits and Stability; IAU Colloq. 96: The Few Body Problem; Valtonen, M.J., Ed.; Springer: Berlin/Heidelberg, Germany, 1988; p. 31. [Google Scholar] [CrossRef]
- Pan, S.; Hou, X. Review Article: Resonant families of periodic orbits in the restricted three-body problem. Res. Astron. Astrophys. 2022, 22, 072002. [Google Scholar] [CrossRef]
- Belbruno, E.; Marsden, B.G. Resonance Hopping in Comets. Astron. J. 1997, 113, 1433. [Google Scholar] [CrossRef]
- Howell, K.; Marchand, B.; Lo, M. Temporary Satellite Capture of Short-Period Jupiter Family Comets from the Perspective of Dynamical Systems. J. Astronaut. Sci. 2000, 49. [Google Scholar] [CrossRef]
- Koon, W.S.; Lo, M.W.; Marsden, J.E.; Ross, S.D. Heteroclinic connections between periodic orbits and resonance transitions in celestial mechanics. Chaos 2000, 10, 427–469. [Google Scholar] [CrossRef]
- Koon, W.S.; Lo, M.W.; Marsden, J.E.; Ross, S.D. Resonance and Capture of Jupiter Comets. Celest. Mech. Dyn. Astron. 2001, 81, 27–38. [Google Scholar] [CrossRef]
- Belbruno, E.; Topputo, F.; Gidea, M. Resonance transitions associated to weak capture in the restricted three-body problem. Adv. Space Res. 2008, 42, 1330–1351. [Google Scholar] [CrossRef]
- Khain, T.; Becker, J.; Adams, F. The Resonance Hopping Effect in the Neptune-planet Nine System. Publ. Astron. Soc. Pac. 2020, 132, 124401. [Google Scholar] [CrossRef]
- Malhotra, R.; Zhang, N. On the divergence of first-order resonance widths at low eccentricities. Mon. Not. R. Astron. Soc. 2020, 496, 3152–3160. [Google Scholar] [CrossRef]
- Malhotra, R. New results on orbital resonances. IAU Symp. 2022, 364, 85–101. [Google Scholar] [CrossRef] [PubMed]
- Antoniadou, K.I.; Libert, A.S. Bridges and gaps at low-eccentricity first-order resonances. Mon. Not. R. Astron. Soc. 2021, 506, 3010–3017. [Google Scholar] [CrossRef]
- Lo, M.; Parker, J. Unstable Resonant Orbits near Earth and Their Applications in Planetary Missions. In Proceedings of the AIAA Guidance, Navigation, and Control Conference and Exhibit, Providence, RI, USA, 16–19 August 2004. [Google Scholar] [CrossRef]
- Anderson, R.L. Tour Design Using Resonant-Orbit Invariant Manifolds in Patched Circular Restricted Three-Body Problems. J. Guid. Control Dyn. 2021, 44, 106–119. [Google Scholar] [CrossRef]
- Vaquero, M.; Howell, K.C. Leveraging Resonant-Orbit Manifolds to Design Transfers Between Libration-Point Orbits. J. Guid. Control Dyn. 2014, 37, 1143–1157. [Google Scholar] [CrossRef]
- Canales, D.; Gupta, M.; Park, B.; Howell, K.C. A transfer trajectory framework for the exploration of Phobos and Deimos leveraging resonant orbits. Acta Astronaut. 2022, 194, 263–276. [Google Scholar] [CrossRef]
- Lei, H.; Xu, B. Resonance transition periodic orbits in the circular restricted three-body problem. Astrophys. Space Sci. 2018, 363, 70. [Google Scholar] [CrossRef]
- Lei, H. Dynamical models for secular evolution of navigation satellites. Astrodynamics 2019, 4, 57–73. [Google Scholar] [CrossRef]
- McCarthy, B.; Howell, K. Leveraging quasi-periodic orbits for trajectory design in cislunar space. Astrodynamics 2021, 5, 139–165. [Google Scholar] [CrossRef]
- Lian, Y.; Huo, Z.; Cheng, Y. On the dynamics and control of the Sun-Earth L2 tetrahedral formation. Astrodynamics 2021, 5, 331–346. [Google Scholar] [CrossRef]
- Mccomas, D.; Carrico, J.; Hautamaki, B.; Intelisano, M.; Lebois, R.; Loucks, M.; Policastri, L.; Reno, M.; Scherrer, J.; Schwadron, N.; et al. A new class of long-term stable lunar resonance orbits: Space weather applications and the Interstellar Boundary Explorer. Space Weather 2011, 9, 11002. [Google Scholar] [CrossRef]
- Dichmann, D.; Lebois, R.; Carrico, J. Dynamics of Orbits Near 3:1 Resonance in the Earth-Moon System. J. Astronaut. Sci. 2014, 60, 51–86. [Google Scholar] [CrossRef]
- Gangestad, J.W.; Henning, G.A.; Persinger, R.R.; Ricker, G.R. A High Earth, Lunar Resonant Orbit for Lower Cost Space Science Missions. arXiv 2013, arXiv:1306.5333. [Google Scholar]
- Ricker, G.R. The Transiting Exoplanet Survey Satellite (TESS): Discovering New Earths and Super-Earths in the Solar Neighborhood. In AAS/Division for Extreme Solar Systems Abstracts; American Astronomical Society: Washington, DC, USA, 2015. [Google Scholar]
- Szebehely, V. Theory of Orbits: The Restricted Problem of Three Bodies. Am. J. Phys. 1968, 36, 375. [Google Scholar] [CrossRef]
- Lara, M.; Peláez, J. On the numerical continuation of periodic orbits. An intrinsic, 3-dimensional, differential, predictor-corrector algorithm. Astron. Astrophys. 2002, 389, 692–701. [Google Scholar] [CrossRef]
- Murray, C.D.; Dermott, S.F. Solar System Dynamics; Cambridge University Press: Cambridge, UK, 1999. [Google Scholar] [CrossRef]
- Hadjidemetriou, J.D. Resonant Motion in the Restricted Three Body Problem. Celest. Mech. Dyn. Astron. 1993, 56, 201–219. [Google Scholar] [CrossRef]
- Hou, X.; Xin, X.; Feng, J. Genealogy and stability of periodic orbit families around uniformly rotating asteroids. Commun. Nonlinear Sci. Numer. Simul. 2018, 56, 93–114. [Google Scholar] [CrossRef]
- Hadjidemetriou, J.D.; Ichtiaroglou, S. A qualitative study of the Kirkwood gaps in the asteroids. Astron. Astrophys. 1984, 131, 20–32. [Google Scholar]
- Hadjidemetriou, J.D. Periodic Orbits. Celest. Mech. 1984, 34, 379–393. [Google Scholar] [CrossRef]
- Hou, X.H.; Liu, L. The Symmetric Horseshoe Periodic Families and the Lyapunov Planar Family Around L 3. Astron. J. 2008, 136, 67. [Google Scholar] [CrossRef]
- Colombo, G.; Franklin, F.A.; Munford, C.M. Saturn’s Rings. Astron. J. 1968, 73, 111. [Google Scholar] [CrossRef]
- Zhang, H.; Li, Y.; Zhang, K. A novel method of periodic orbit computation in circular restricted three-body problem. Sci. China E Technol. Sci. 2011, 54, 2197–2203. [Google Scholar] [CrossRef]
- Schanzle, A.F. Horseshoe-shaped orbits in the Jupiter-sun restricted problem. Astron. J. 1967, 72, 149. [Google Scholar] [CrossRef]

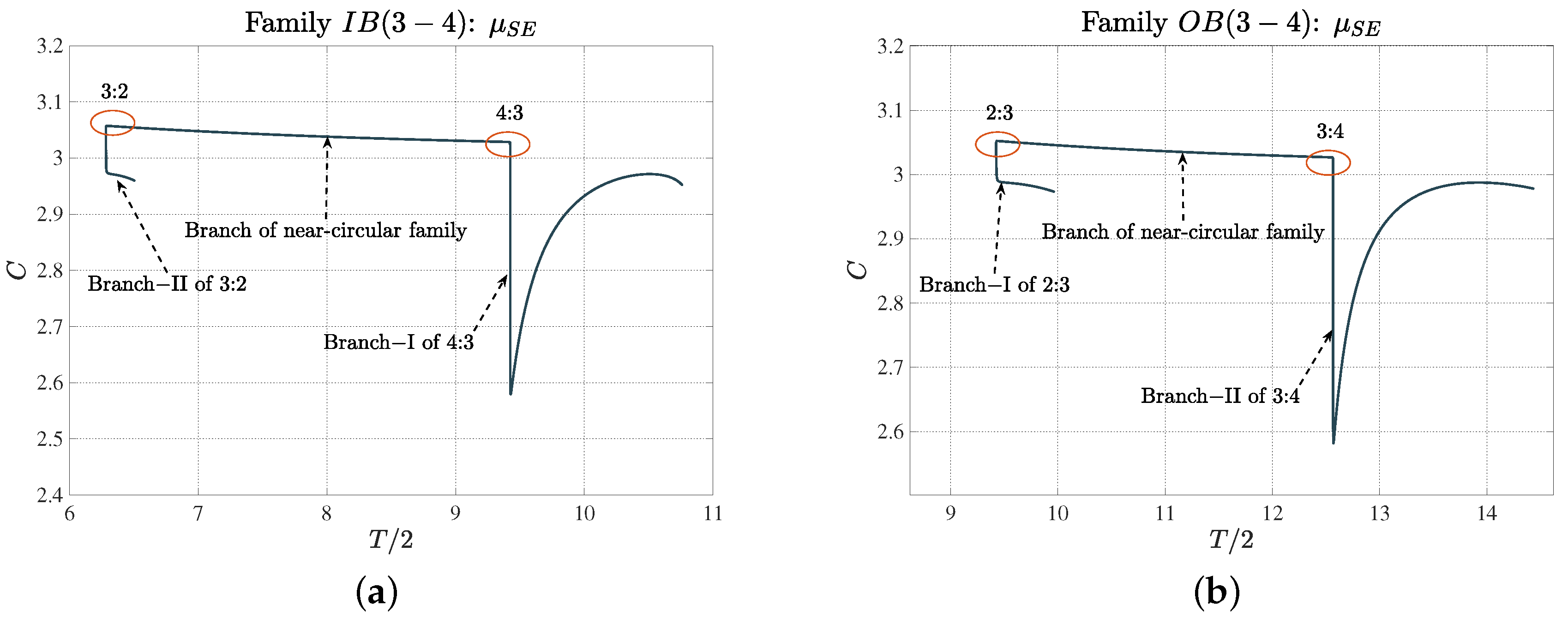





| System/Name | Values |
|---|---|
| Two-body system () | 0 |
| Sun–Earth system () | 3.003480575402412 |
| Sun–Jupiter system () | 9.538811803631013 |
| Orbit/Name | C | |||
|---|---|---|---|---|
| RTPF-3:2(a)-orbit () | 0.996693105698827 | −0.606721682695370 | 13.572632053631988 | 2.986678114083724 |
| RTPF-3:2(b)-orbit () | 0.839807356007294 | 0.324698985902574 | 17.371772692048165 | 2.985500211312612 |
| RTPF-4:3(a)-orbit () | 0.999071125547079 | −0.498067952701473 | 17.147473111884469 | 2.992921442199951 |
| RTPF-4:3(b)-orbit () | 0.872399628274439 | 0.253293221227850 | 21.274174849052791 | 2.990627628838839 |
| RTPF-2:3(a)-orbit () | 1.003548207343015 | 0.656988112184208 | 16.124846254286862 | 2.989316084875661 |
| RTPF-2:3(b)-orbit () | 1.240062798333267 | −0.432604173138644 | 21.223783359411804 | 2.969522450671411 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pan, S.; Hou, X. Analysis of Resonance Transition Periodic Orbits in the Circular Restricted Three-Body Problem. Appl. Sci. 2022, 12, 8952. https://doi.org/10.3390/app12188952
Pan S, Hou X. Analysis of Resonance Transition Periodic Orbits in the Circular Restricted Three-Body Problem. Applied Sciences. 2022; 12(18):8952. https://doi.org/10.3390/app12188952
Chicago/Turabian StylePan, Shanshan, and Xiyun Hou. 2022. "Analysis of Resonance Transition Periodic Orbits in the Circular Restricted Three-Body Problem" Applied Sciences 12, no. 18: 8952. https://doi.org/10.3390/app12188952
APA StylePan, S., & Hou, X. (2022). Analysis of Resonance Transition Periodic Orbits in the Circular Restricted Three-Body Problem. Applied Sciences, 12(18), 8952. https://doi.org/10.3390/app12188952




