Effect of Soil Damping on the Soil–Pile–Structure Interaction Analyses in Cohesionless Soils
Abstract
:1. Introduction
2. The Method
2.1. Soil Models
2.2. Verification Analyses Using Hyperbolic and MC Constitutive Models
2.3. Numerical Analyses Using Hyperbolic and MC Constitutive Models
3. Analysis Results
3.1. Verification Analysis
3.1.1. Non-Liquefiable Soil (Case 1)
3.1.2. Liquefiable Soil (Case 2)
3.2. Numerical Analyses
4. Conclusions
- The nonlinear soil behavior can be modeled using the modulus degradation curves in numerical analyses of soil–pile–structure problems. According to the verification analyses, if the soil damping is integrated into the model accurately, the use of modulus degradation can represent the response even for liquefiable soils.
- The constitutive models without a cut-off for G/Gmax might cause significantly higher damping ratios. To overcome the higher damping ratio problem, the minimum G/Gmax value was set to 0.05, and closer results were obtained in the verification analyses. Thus, the cut-off must be applied to eliminate higher damping ratios at large strains. Generally, the agreement between the 3D analysis results and centrifuge test outputs was reasonably good.
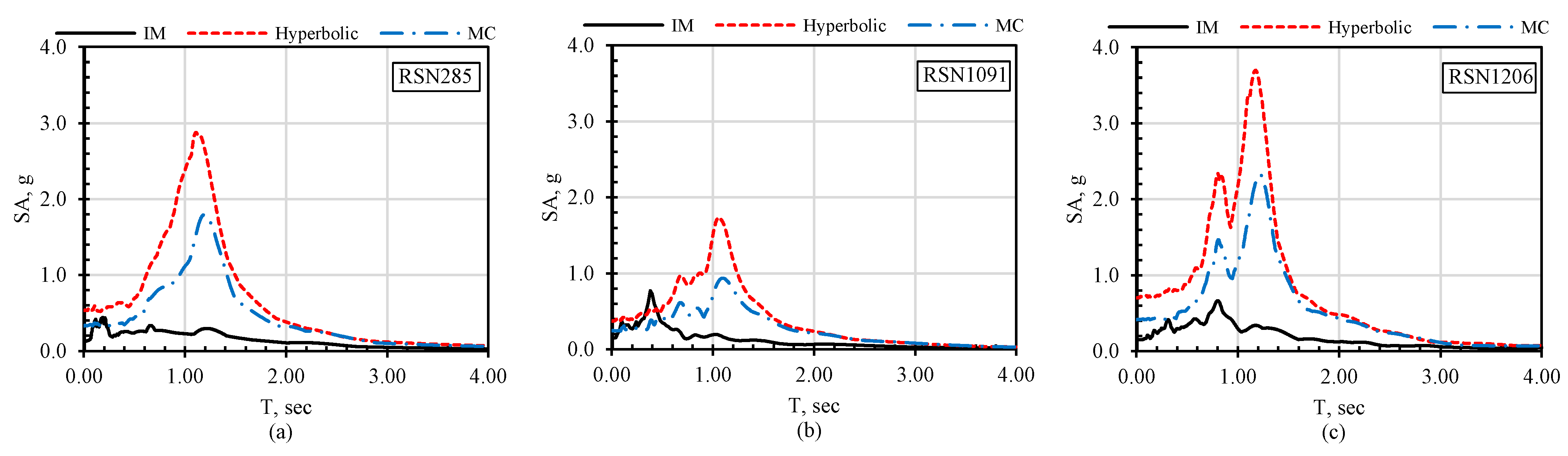
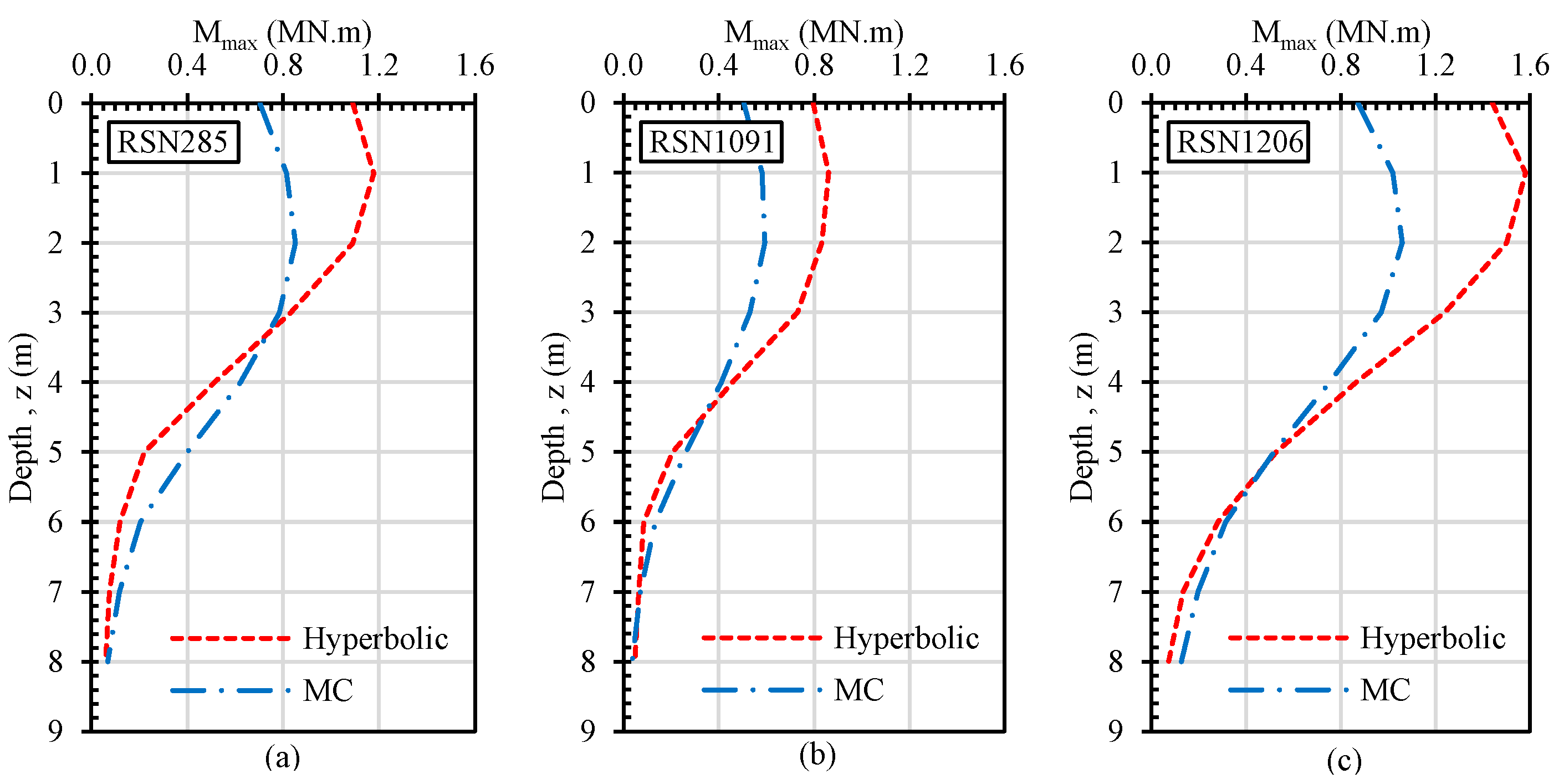
- Dynamic single pile–soil–structure analyses performed with the additional earthquake records selected from the PEER database have confirmed the effect of soil damping on the pile and structure response. Peak structure accelerations, peak spectral accelerations, and bending moments along the pile were compared for hyperbolic and MC models. The peak structure accelerations and the peak bending moments for all earthquake records were greater in the hyperbolic model. The difference between the hyperbolic and MC models varies between 27 and 63%, depending on the applied earthquake record. The results have indicated that the soil damping ratio in the MC model could be considerably high, especially in the shallow depths, since the lower confining stresses cause the shear strength to be significantly low.
- Apart from the damping effect, the analyses have shown that the selection of earthquake records significantly affects the structure and pile response. In particular, although the selected earthquakes have the same peak ground acceleration, as much as three times the difference might occur in the resulting structure accelerations (RSN 1613 and RSN 1206).
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Brown, D.A.; Shie, C.-F. Some numerical experiments with a three dimensional finite element model of a laterally loaded pile. Comput. Geotech. 1991, 12, 149–162. [Google Scholar] [CrossRef]
- Luo, C.; Yang, X.; Zhan, C.; Jin, X.; Ding, Z. Nonlinear 3D finite element analysis of soil–pile–structure interaction system subjected to horizontal earthquake excitation. Soil Dyn. Earthq. Eng. 2016, 84, 145–156. [Google Scholar] [CrossRef]
- Giannakos, S.; Gerolymos, N.; Gazetas, G. Cyclic lateral response of piles in dry sand: Finite element modeling and validation. Comput. Geotech. 2012, 44, 116–131. [Google Scholar] [CrossRef]
- Bathe, K.-J. Finite Element Procedures; Klaus-Jurgen Bathe: Watertown, MA, USA, 2006. [Google Scholar]
- Wang, S.; Kutter, B.L.; Chacko, M.J.; Wilson, D.W.; Boulanger, R.W.; Abghari, A. Nonlinear seismic soil-pile structure interaction. Earthq. Spectra 1998, 14, 377–396. [Google Scholar] [CrossRef]
- Nogami, T.; Otani, J.; Konagai, K.; Chen, H.-L. Nonlinear soil-pile interaction model for dynamic lateral motion. J. Geotech. Eng. 1992, 118, 89–106. [Google Scholar] [CrossRef]
- Allotey, N.; El Naggar, M.H. A numerical study into lateral cyclic nonlinear soil–pile response. Can. Geotech. J. 2008, 45, 1268–1281. [Google Scholar] [CrossRef]
- Poulos, H.G. Behavior of laterally loaded piles: I-single piles. J. Soil Mech. Found. Div. 1971, 97, 711–731. [Google Scholar] [CrossRef]
- Banerjee, P.; Davies, T. The behaviour of axially and laterally loaded single piles embedded in nonhomogeneous soils. Geotechnique 1978, 28, 309–326. [Google Scholar] [CrossRef]
- Randolph, M.F. The response of flexible piles to lateral loading. Geotechnique 1981, 31, 247–259. [Google Scholar] [CrossRef]
- Boulanger, R.W.; Curras, C.J.; Kutter, B.L.; Wilson, D.W.; Abghari, A. Seismic soil-pile-structure interaction experiments and analyses. J. Geotech. Geoenviron. Eng. 1999, 125, 750–759. [Google Scholar] [CrossRef]
- Finn, W. A study of piles during earthquakes: Issues of design and analysis. Bull. Earthq. Eng. 2005, 3, 141–234. [Google Scholar] [CrossRef]
- Rahmani, A.; Taiebat, M.; Finn, W.L.; Ventura, C.E. Evaluation of p-y springs for nonlinear static and seismic soil-pile interaction analysis under lateral loading. Soil Dyn. Earthq. Eng. 2018, 115, 438–447. [Google Scholar] [CrossRef]
- Darendeli, M.B. Development of a New Family of Normalized Modulus Reduction and Material Damping Curves; The University of Texas at Austin: Austin, TX, USA, 2001. [Google Scholar]
- Ishibashi, I.; Zhang, X. Unified dynamic shear moduli and damping ratios of sand and clay. Soils Found. 1993, 33, 182–191. [Google Scholar] [CrossRef]
- Seed, H.B.; Idriss, I.M. Soil Moduli and Damping Factors for Dynamic Response Analysis; EERC: Grand Forks, ND, USA, 1970. [Google Scholar]
- Matasović, N.; Vucetic, M. Cyclic characterization of liquefiable sands. J. Geotech. Eng. 1993, 119, 1805–1822. [Google Scholar] [CrossRef]
- Vucetic, M.; Dobry, R. Effect of soil plasticity on cyclic response. J. Geotech. Eng. 1991, 117, 89–107. [Google Scholar] [CrossRef]
- Schnabel, P.; Seed, H.B.; Lysmer, J. Modification of seismograph records for effects of local soil conditions. Bull. Seismol. Soc. Am. 1972, 62, 1649–1664. [Google Scholar] [CrossRef]
- Groholski, D.R.; Hashash, Y.M.; Kim, B.; Musgrove, M.; Harmon, J.; Stewart, J.P. Simplified model for small-strain nonlinearity and strength in 1D seismic site response analysis. J. Geotech. Geoenviron. Eng. 2016, 142, 04016042. [Google Scholar] [CrossRef]
- Phillips, C.; Hashash, Y.M. Damping formulation for nonlinear 1D site response analyses. Soil Dyn. Earthq. Eng. 2009, 29, 1143–1158. [Google Scholar] [CrossRef]
- Park, D.; Hashash, Y.M. Soil damping formulation in nonlinear time domain site response analysis. J. Earthq. Eng. 2004, 8, 249–274. [Google Scholar] [CrossRef]
- Régnier, J.; Bonilla, L.F.; Bard, P.Y.; Bertrand, E.; Hollender, F.; Kawase, H.; Sicilia, D.; Arduino, P.; Amorosi, A.; Asimaki, D.; et al. PRENOLIN: International Benchmark on 1D Nonlinear Site-Response Analysis—Validation Phase Exercise. Bull. Seismol. Soc. Am. 2018, 108, 876–900. [Google Scholar] [CrossRef]
- Beaty, M.H.; Byrne, P.M. UBCSAND Constitutive Model, Version 904aR; Itasca UDM: Minneapolis, MN, USA, 2011; p. 69. [Google Scholar]
- Boulanger, R.; Ziotopoulou, K. PM4Sand (Version 3): A Sand Plasticity Model for Earthquake Engineering Applications. In Center for Geotechnical Modeling Report No. UCD/CGM-15/01; Department of Civil and Environmental Engineering, University of California: Davis, CA, USA, 2015. [Google Scholar]
- Cheng, Z. Application of SANISAND Dafalias-Manzari model in FLAC 3D. In Continuum and Distinct Element Numerical Modeling in Geomechanics, (October 2013); Itasca International Inc.: Minneapolis, MN, USA, 2013. [Google Scholar]
- Hokmabadi, A.S.; Fatahi, B.; Samali, B. Assessment of soil–pile–structure interaction influencing seismic response of mid-rise buildings sitting on floating pile foundations. Comput. Geotech. 2014, 55, 172–186. [Google Scholar] [CrossRef]
- Maheshwari, B.K.; Truman, K.; El Naggar, M.; Gould, P. Three-dimensional nonlinear analysis for seismic soil–pile-structure interaction. Soil Dyn. Earthq. Eng. 2004, 24, 343–356. [Google Scholar] [CrossRef]
- Guin, J.; Banerjee, P. Coupled soil-pile-structure interaction analysis under seismic excitation. J. Struct. Eng. 1998, 124, 434–444. [Google Scholar] [CrossRef]
- Gohl, W.B. Response of Pile Foundations to Simulated Earthquake Loading: Experimental and Analytical Results Volume I. Ph.D. Thesis, University of British Columbia, Vancouver, BC, Canada, 1991. [Google Scholar]
- Wilson, D.W. Soil-Pile-Superstructure Interaction in Liquefying Sand and Soft Clay; Citeseer: University Park, PA, USA, 1998; Volume 1998. [Google Scholar]
- Itasca Consulting Group, Inc. FLAC3D—Fast Lagrangian Analysis of Continua in Three-Dimensions, Version 7.0; Itasca: Minneapolis, MN, USA, 2019. [Google Scholar]
- Thavaraj, T.; Liam Finn, W.; Wu, G. Seismic response analysis of pile foundations. Geotech. Geol. Eng. 2010, 28, 275–286. [Google Scholar] [CrossRef]
- Kwon, S.Y.; Yoo, M. Study on the dynamic soil-pile-structure interactive behavior in liquefiable sand by 3D numerical simulation. Appl. Sci. 2020, 10, 2723. [Google Scholar] [CrossRef]
- Masing, G. Eigenspannungen und Verfestigung beim Messing [Fundamental Stresses and Strengthening with Brass]; International Congress of Applied Mechanich: Zurich, Switzerland, 1926. [Google Scholar]
- Kuhlemeyer, R.L.; Lysmer, J. Finite element method accuracy for wave propagation problems. J. Soil Mech. Found. Div. 1973, 99, 421–427. [Google Scholar] [CrossRef]
- Finn, W.; Fujita, N. Piles in liquefiable soils: Seismic analysis and design issues. Soil Dyn. Earthq. Eng. 2002, 22, 731–742. [Google Scholar] [CrossRef]
- The Pacific Earthquake Engineering Research Center (PEER). PEER Strong Motion Database on Line. Available online: https://peer.berkeley.edu/peer-strong-ground-motion-databases (accessed on 5 April 2022).


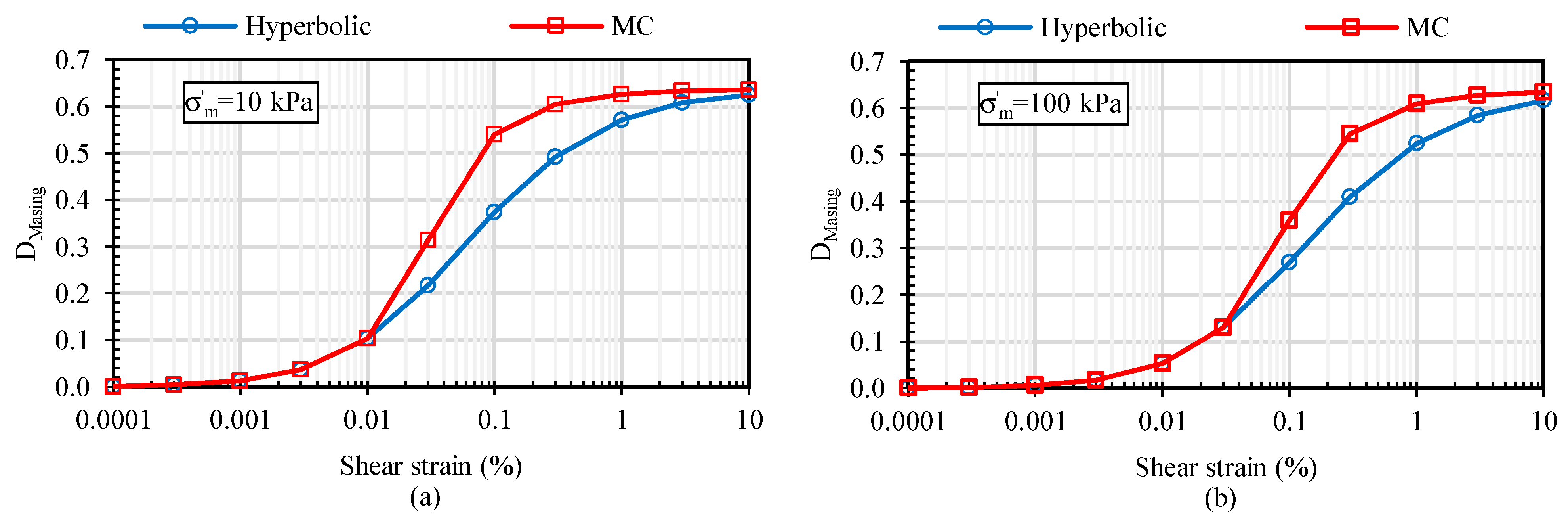




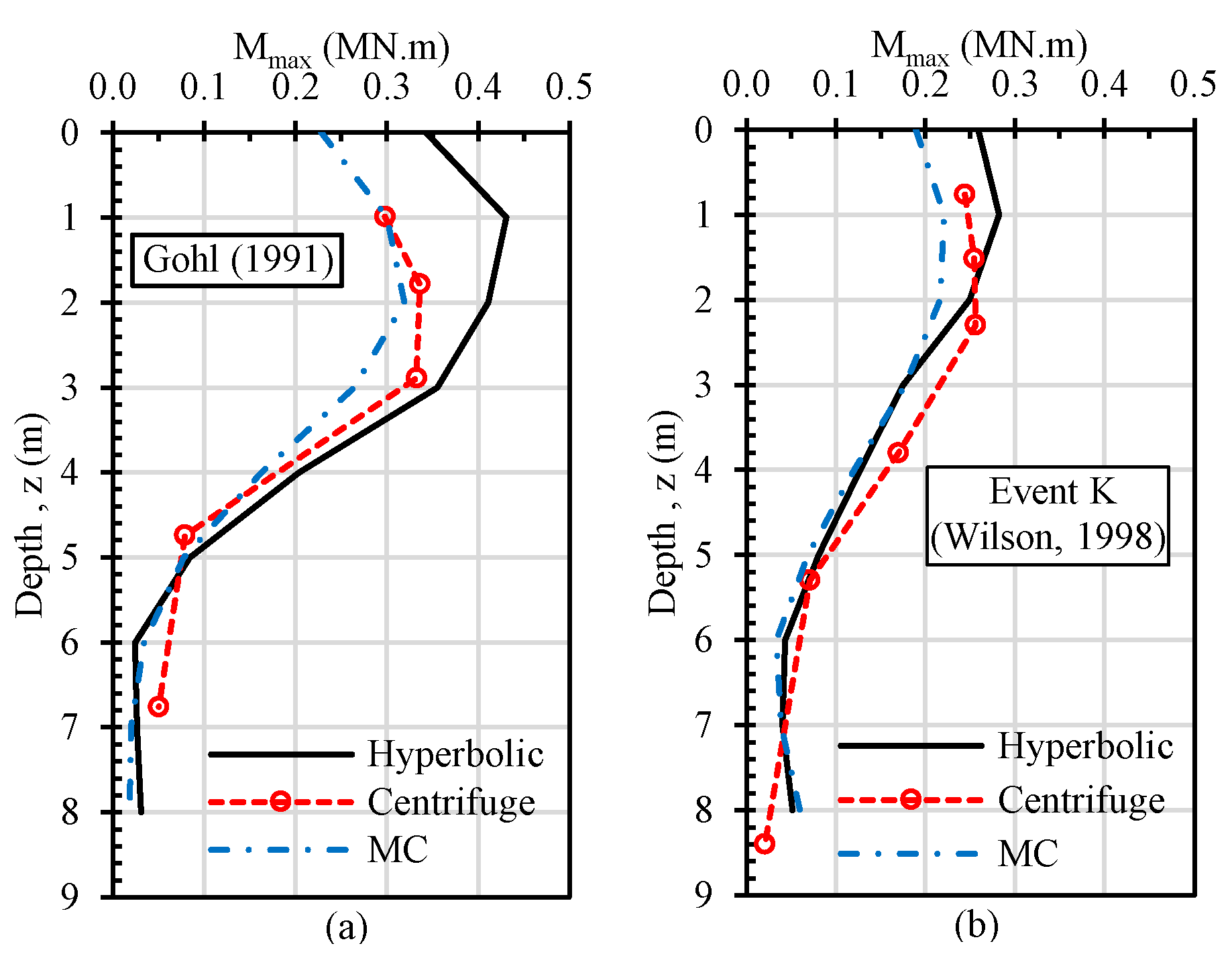
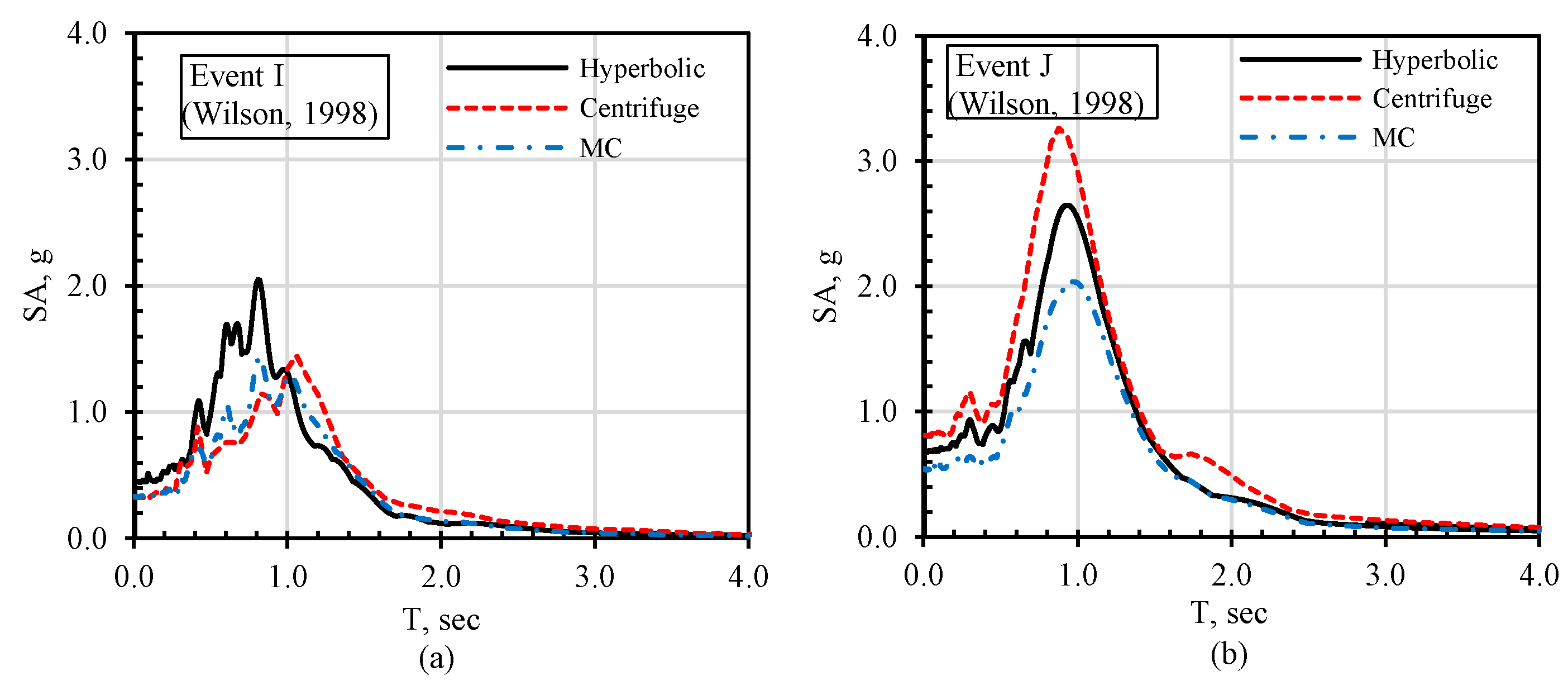
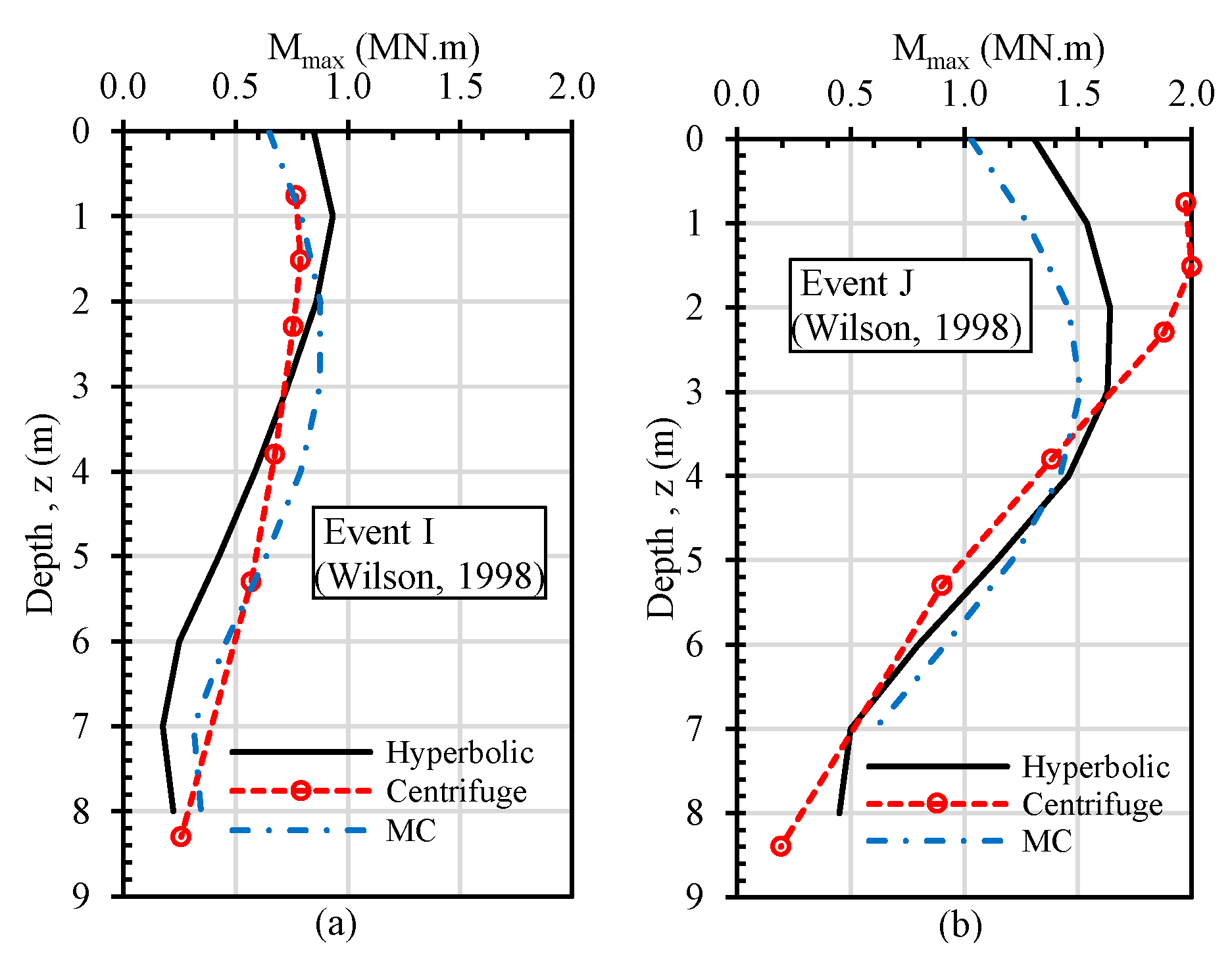




| Wilson, 1998 | Gohl, 1991 | ||
|---|---|---|---|
| Layer | Layer 1 | Layer 2 | Single Layer |
| Effective unit weight, γ′ (kN/m3) | 9.5 | 9.9 | 15.1 |
| Relative density (%) | 55 | 80 | 40 |
| Friction angle, φ′ (°) | 36 | 40 | 34 |
| Dilation angle ψ (°) | 4 | 8 | 2 |
| Poisson’s ratio, ν | 0.45 | 0.45 | 0.30 |
| Structure (Wilson, 1998) | Structure (Gohl, 1991) | ||||||
|---|---|---|---|---|---|---|---|
| Flexural Stiffness EI (MN·m2) | Height (m) | Mass (Mg) | Tfixed (s) | Flexural Stiffness EI (MN·m2) | Height (m) | Mass (Mg) | Tfixed (s) |
| 427 | 3.8 | 49 | 0.3 | 172 | 2.0 | 52.2 | 0.3 |
| Layer | Single Layer |
|---|---|
| Effective unit weight, γ′ (kN/m3) | 18 |
| Relative density (%) | 55 |
| Friction angle, φ′ (°) | 36 |
| Dilation angle ψ (°) | 4 |
| Poisson’s ratio, ν | 0.30 |
| Pile | Structure | ||||||
|---|---|---|---|---|---|---|---|
| Diameter (m) | Length (m) | E (MN/m2) | I (m4) | Mass (Mg) | Flexural Stiffness EI (MN·m2) | H (m) | Tfixed (s) |
| 0.65 | 16 | 30,000 | 0.00876 | 40 | 262.8 | 5.0 | 0.5 |
| PEER Code | Earthquake | Year | Mw | Station | Fault | Rrup (km) | (Vs)30 (m/s) | Pga (g) | SF |
|---|---|---|---|---|---|---|---|---|---|
| RSN143 | Tabas, Iran | 1978 | 7.35 | Tabas | Reverse | 2.05 | 766 | 0.14 | 0.17 |
| RSN285 | Irpinia, Italy-01 | 1980 | 6.90 | Bagnoli Irpinio | Normal | 8.18 | 650 | 0.13 | 1.0 |
| RSN572 | Taiwan SMART1 (45) | 1986 | 7.30 | SMART1 E02 | Reverse | 51.35 | 672 | 0.14 | 1.0 |
| RSN1091 | Northridge-01 | 1994 | 6.69 | Vasquez Rocks Park | Reverse | 23.64 | 996 | 0.15 | 1.0 |
| RSN1206 | Chi-Chi, Taiwan | 1992 | 7.62 | CHY042 | Reverse Oblique | 28.17 | 665 | 0.15 | 1.5 |
| RSN1613 | Duzce, Turkey | 1999 | 7.14 | Lamont 1060 | Strike Slip | 25.88 | 782 | 0.16 | 3.0 |
| RSN | 143 | 572 | 1613 | 285 | 1091 | 1206 | |
|---|---|---|---|---|---|---|---|
| PA (g) | Hyperbolic MC | 0.24 | 0.31 | 0.24 | 0.53 | 0.37 | 0.70 |
| 0.18 | 0.19 | 0.15 | 0.33 | 0.24 | 0.41 | ||
| PSA (g) | Hyperbolic MC | 1.27 | 1.43 | 0.94 | 2.87 | 1.73 | 3.69 |
| 0.95 | 1.02 | 0.62 | 1.79 | 0.94 | 2.32 | ||
| Mmax (kN·m) | Hyperbolic MC | 533 | 701 | 543 | 1180 | 862 | 1580 |
| 419 | 451 | 333 | 853 | 593 | 1060 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alver, O.; Eseller-Bayat, E.E. Effect of Soil Damping on the Soil–Pile–Structure Interaction Analyses in Cohesionless Soils. Appl. Sci. 2022, 12, 9002. https://doi.org/10.3390/app12189002
Alver O, Eseller-Bayat EE. Effect of Soil Damping on the Soil–Pile–Structure Interaction Analyses in Cohesionless Soils. Applied Sciences. 2022; 12(18):9002. https://doi.org/10.3390/app12189002
Chicago/Turabian StyleAlver, Ozan, and Esra Ece Eseller-Bayat. 2022. "Effect of Soil Damping on the Soil–Pile–Structure Interaction Analyses in Cohesionless Soils" Applied Sciences 12, no. 18: 9002. https://doi.org/10.3390/app12189002
APA StyleAlver, O., & Eseller-Bayat, E. E. (2022). Effect of Soil Damping on the Soil–Pile–Structure Interaction Analyses in Cohesionless Soils. Applied Sciences, 12(18), 9002. https://doi.org/10.3390/app12189002









