Comparing the Measured and Thermodynamically Predicted AFm Phases in a Hydrating Cement
Abstract
:1. Introduction
2. Materials and Methods
2.1. Specimen Preparation
2.2. XRD and TGA/DTG Analysis
2.3. Isothermal Calorimetry Analysis
3. Experimental Results
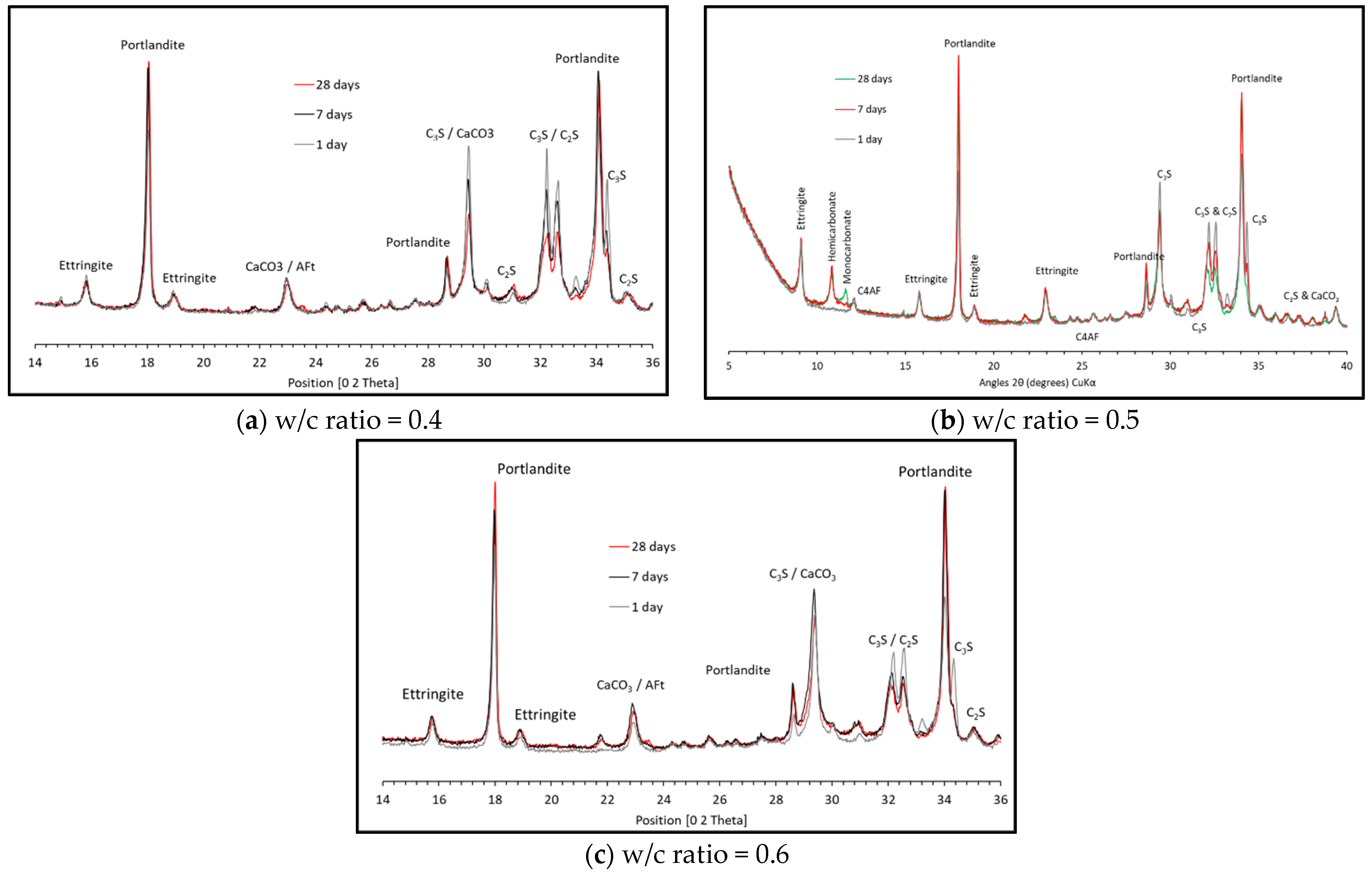
3.1. Solid Phases XRD
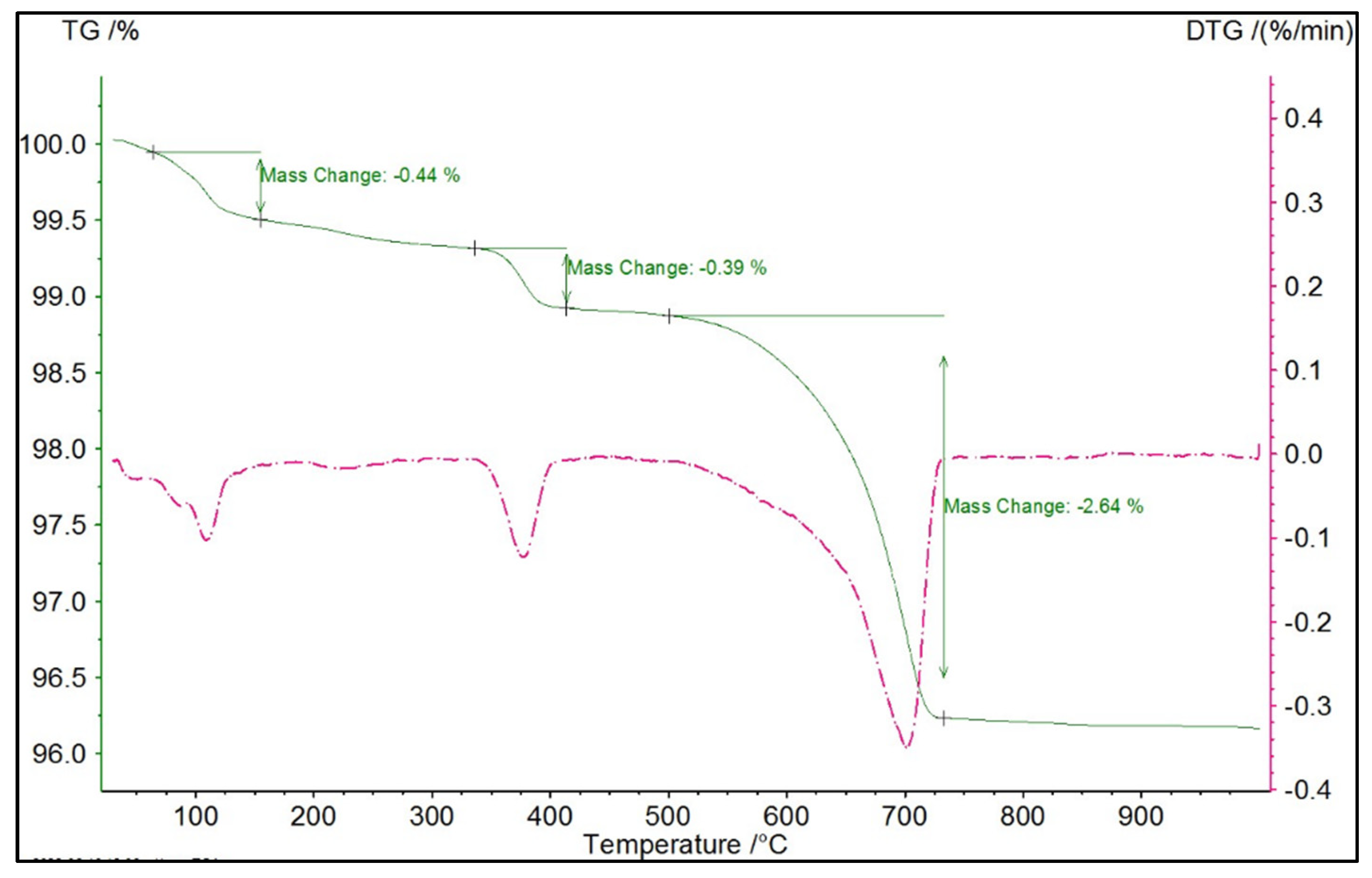
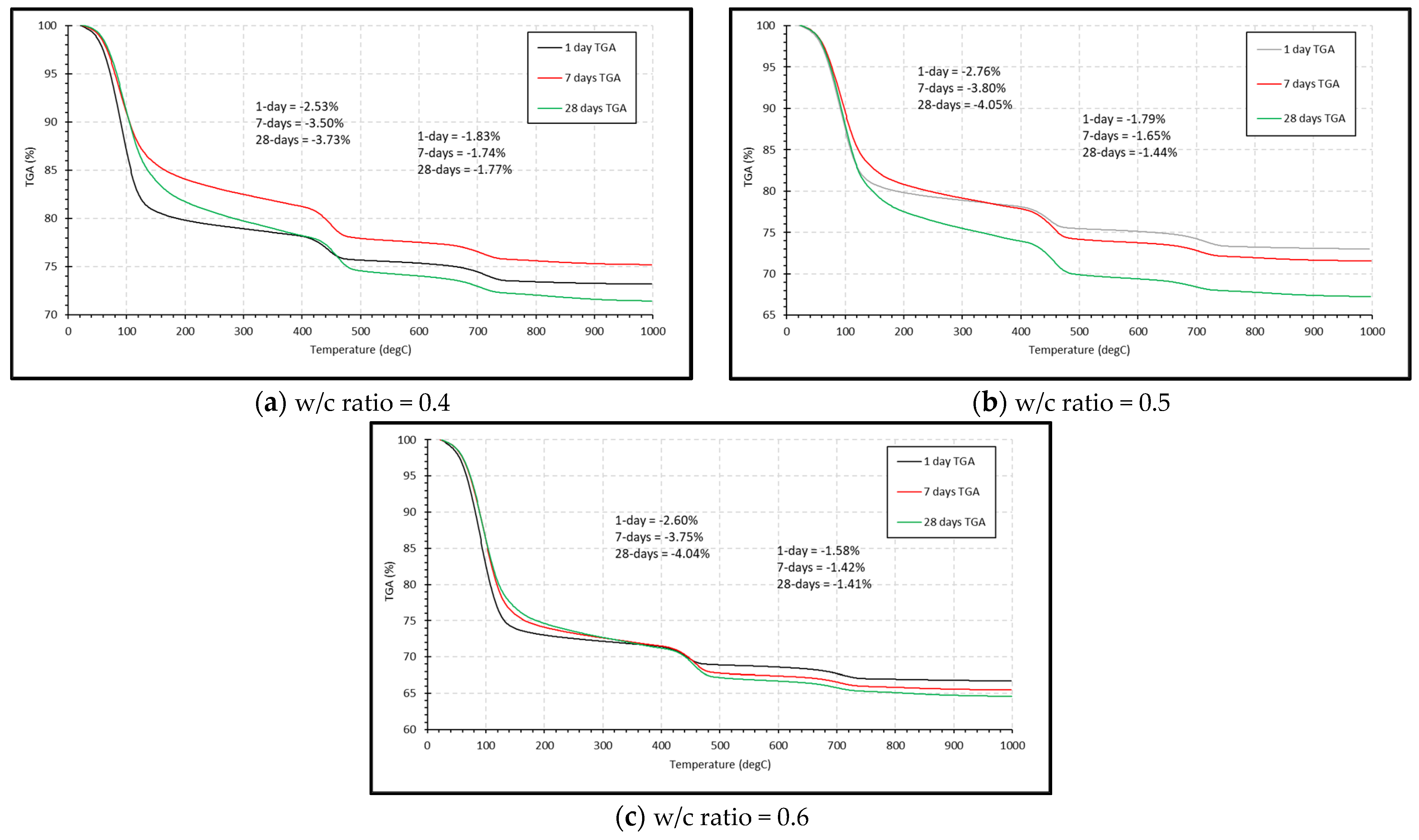
3.2. Thermogravimetric Analysis
3.3. Heat of Hydration Analysis
4. Thermodynamic Modelling of Cement Hydration
4.1. C-S-H Gel
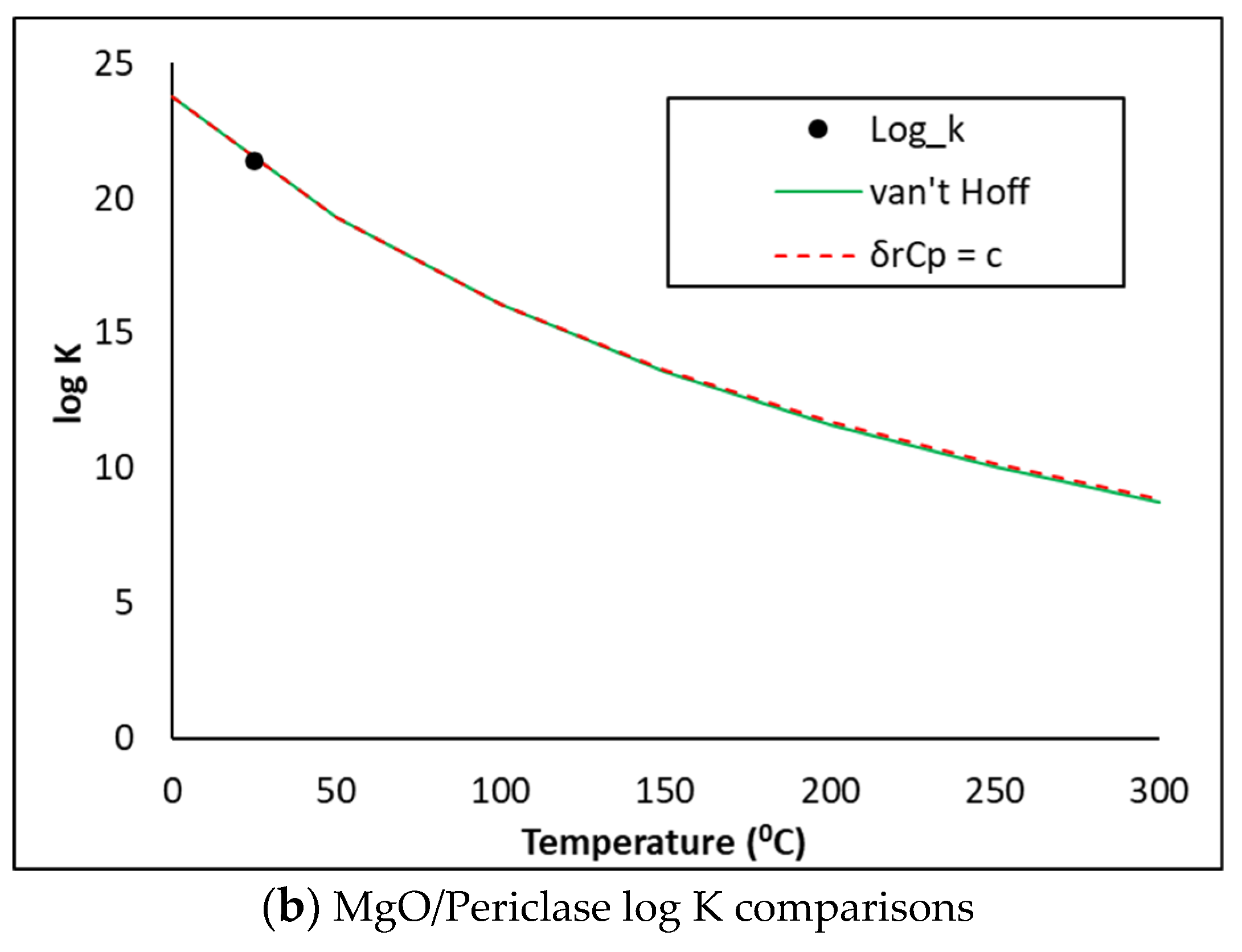
4.2. Thermodynamic Descriptions of SO3, MgO and Periclase
5. Analysis Output
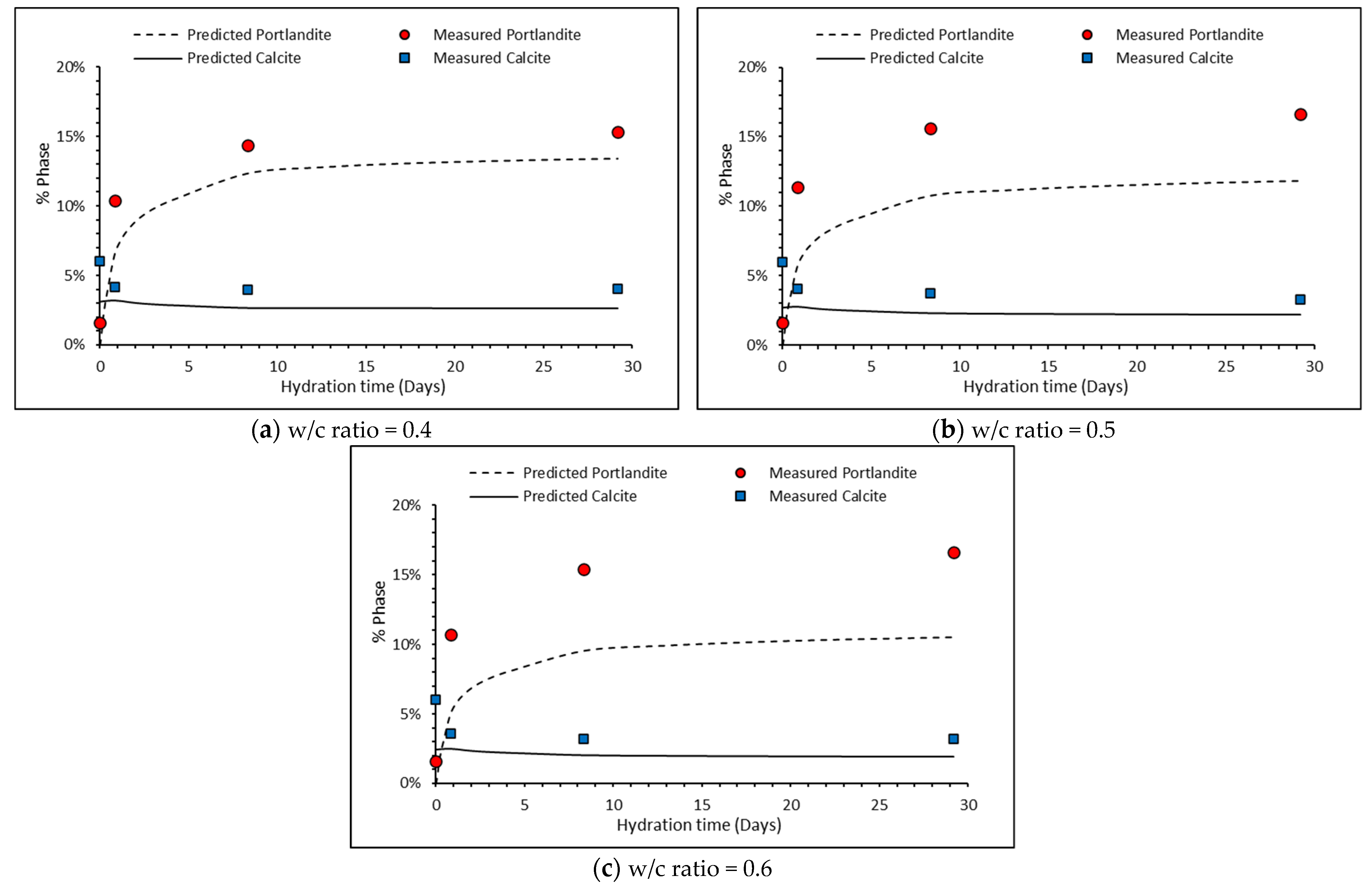
5.1. Comparison between Measured and Predicted Portlandite and Calcite Contents
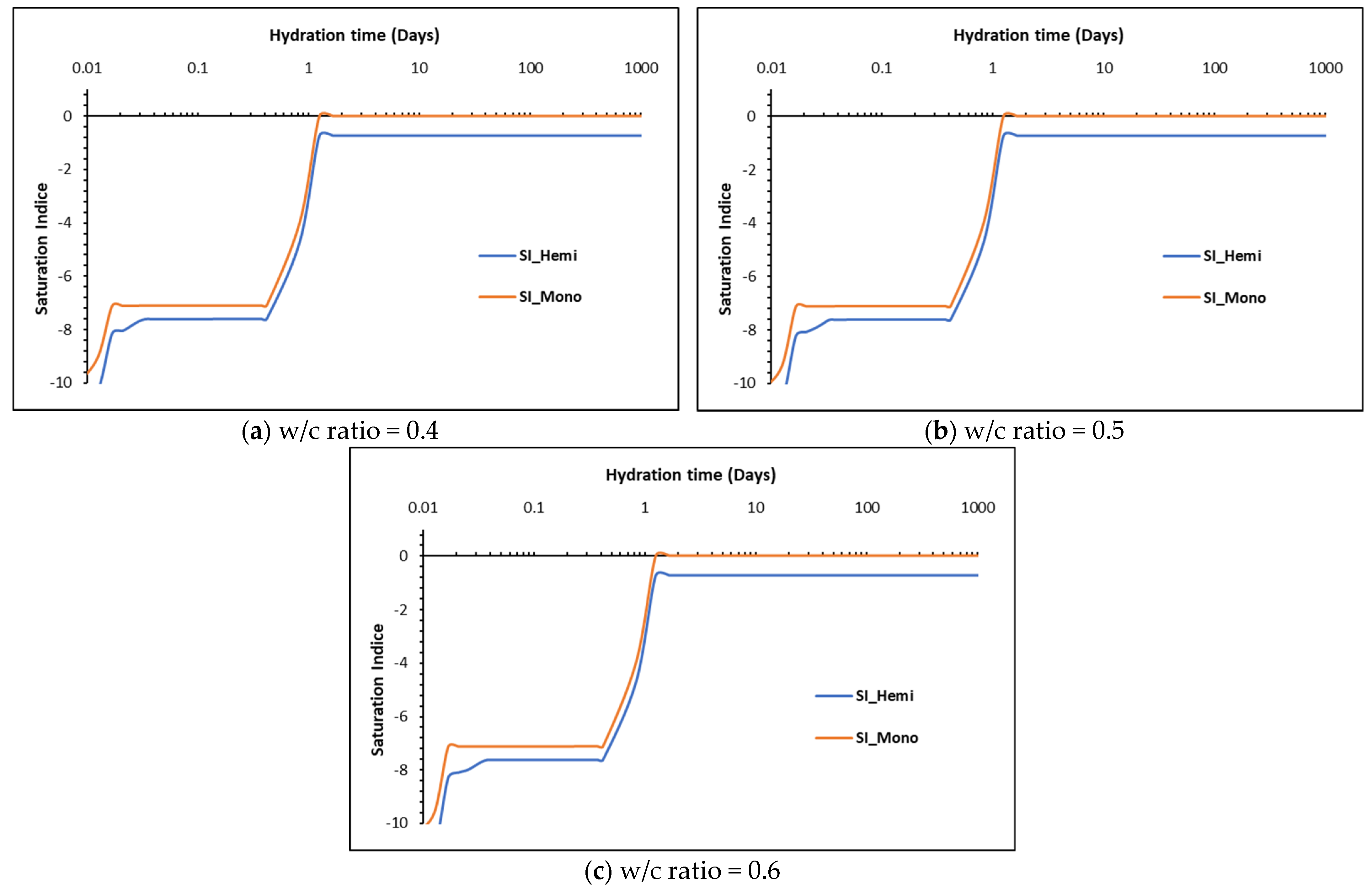
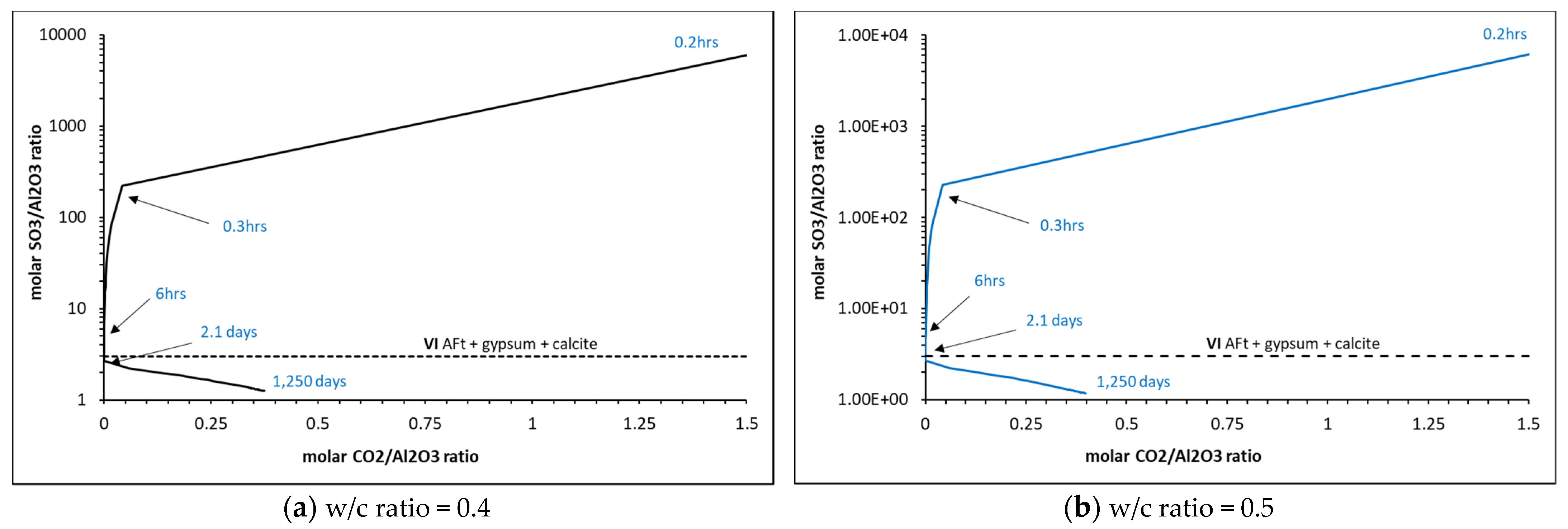
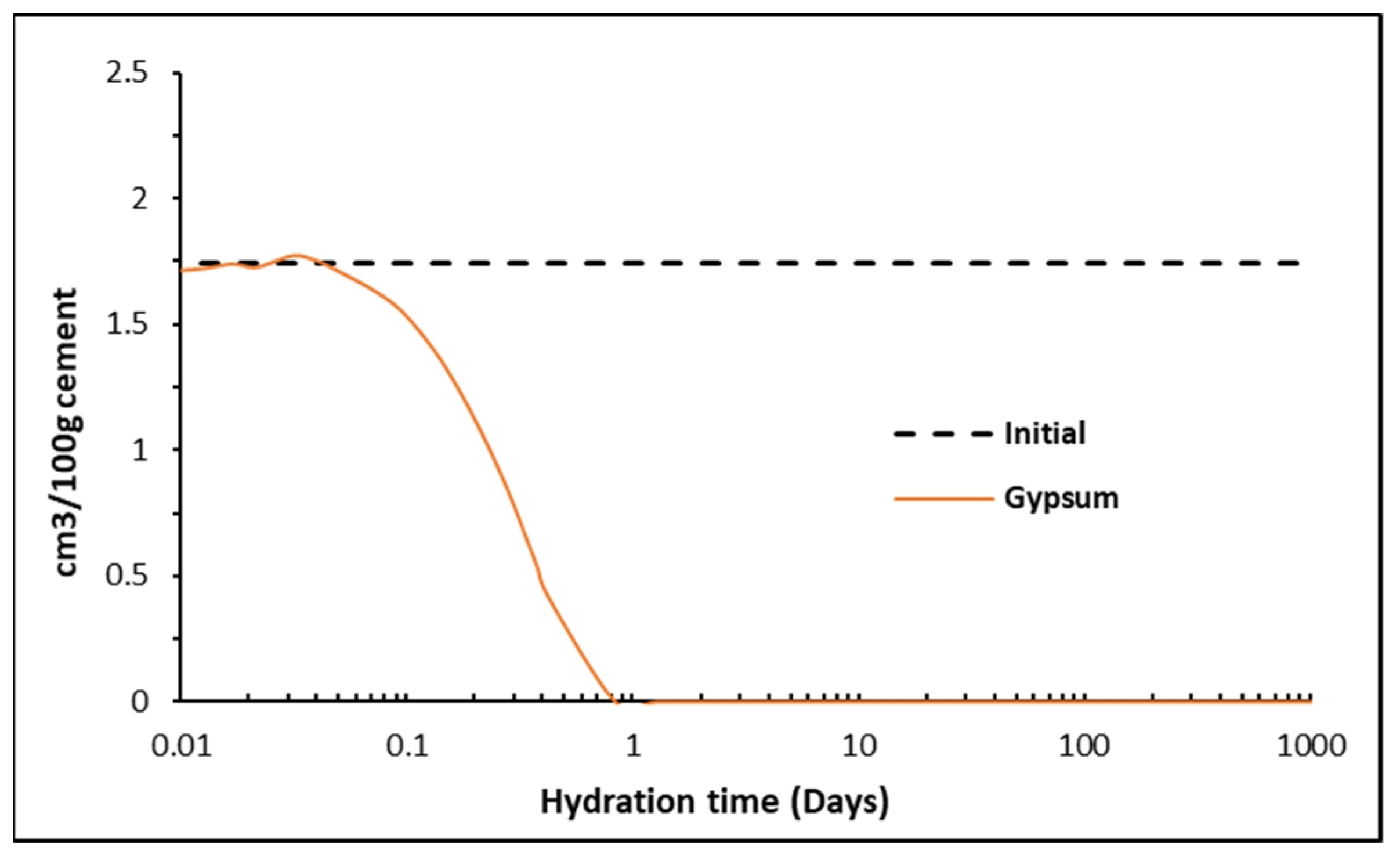
5.2. Phase Assemblages
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Rothstein, D.; Thomas, J.J.; Christensen, B.J.; Jennings, H.M. Solubility behavior of Ca-, S-, Al-, and Si-bearing solid phases in Portland cement pore solutions as a function of hydration time. Cem. Concr. Res. 2002, 32, 1663–1671. [Google Scholar] [CrossRef]
- Lothenbach, B.; Saout, G.L.; Gallucci, E.; Scrivener, K. Influence of limestone on the hydration of Portland cements. Cem. Concr. Res. 2008, 38, 848–860. [Google Scholar] [CrossRef]
- Lothenbach, B.; Winnefeld, F.; Alder, C.; Wieland, E.; Lunk, P. Effect of temperature on the pore solution, microstructure and hydration products of Portland cement pastes. Cem. Concr. Res. 2007, 37, 483–491. [Google Scholar] [CrossRef]
- Lothenbach, B.; Winnefeld, F. Thermodynamic modelling of the hydration of Portland cement. Cem. Concr. Res. 2006, 36, 209–226. [Google Scholar] [CrossRef]
- Holmes, N.; Tyrer, M.; West, R.P.; Lowe, A.; Kelliher, D. Using PHREEQC to model cement hydration. Constr. Build. Mater. 2022, 319, 126–129. [Google Scholar] [CrossRef]
- Holmes, N.; Kelliher, D.; Tyrer, M. Thermodynamic cement hydration modelling using HYDCEM. In Proceedings of the Civil Engineering Research in Ireland 2020, Online Conference, 27 August 2020. [Google Scholar]
- Parkhurst, D.J.; Appelo, C.A.J. Description of Input and Examples for PHREEQC Version 3—A Computer Program for Speciation, Batch-reaction, One-dimensional Transport and Inverse Geochemical Calculations. US Geol. Surv. Tech. Methods 2013, 6, 497. [Google Scholar]
- Matschei, T.; Lothenbach, B.; Glasser, F.P. The AFm phase in Portland cement. Cem. Concr. Res. 2007, 37, 118–130. [Google Scholar] [CrossRef]
- Baquerizo, L.G.; Matschei, T.; Scrivener, K.; Saeidpour, M.; Wadsö, L. Hydration states of AFm cement phases. Cem. Concr. Res. 2015, 73, 143–157. [Google Scholar] [CrossRef]
- Matschei, T.; Lothenbach, B.; Glasser, F.P. The role of calcium carbonate in cement hydration. Cem. Concr. Res. 2007, 37, 551–558. [Google Scholar] [CrossRef]
- Georget, F.; Lothenbach, B.; Wilson, W.; Zunino, F.; Scrivener, K.L. Stability of hemicarbonate under cement paste-like conditions. Cem. Concr. Res. 2022, 153, 106692. [Google Scholar] [CrossRef]
- Kuzel, H.-J.; Pöllmann, H. Hydration of C3A in the presence of Ca(OH)2, CaSO4·2H2O and CaCO3. Cem. Concr. Res. 1991, 21, 885–895. [Google Scholar] [CrossRef]
- Damidot, D.; Stronach, S.; Kindness, A.; Atkins, M.; Glasser, F.P. Thermodynamic investigation of the CaO-Al2O3-CaCO3-H2O closed system at 25 °C and the influence of Na2O. Cem. Concr. Res. 1994, 24, 563–572. [Google Scholar] [CrossRef]
- Hobbs, M.Y. Solubilities and Ion Exchange Properties of Solid Solutions between the OH, Cl and CO3 end Members of the Monocalcium Aluminate Hydrates. Ph.D. Thesis, University of Waterloo, Waterloo, ON, Canada, 2001. [Google Scholar]
- Chou, L.; Garrels, R.M.; Wollast, R. Comparative study of the kinetics and mechanisms of dissolution of carbonate minerals. Chem. Geol. 1989, 78, 269–282. [Google Scholar] [CrossRef]
- British Standards Institute. BS EN 197-1 Cement: Composition, Specification and Conformity Criteria for Common Cements; British Standards Institute: London, UK, 2000. [Google Scholar]
- Hesse, C.; Goetz-Neunhoeffer, F.; Neubauer, J. A new approach in quantitative in-situ XRD of cement pastes: Correlation of heat flow curves with early hydration reactions. Cem. Concr. Res. 2011, 41, 123–128. [Google Scholar] [CrossRef]
- Jensen, D.; Neubauer, J.; Goetz-Neunhoeffer, F.; Haerzschel, R.; Hergeth, W.D. Change in reaction kinetics of a Portland cement caused by a superplasticizer—Calculation of heat flow curves from XRD data. Cem. Concr. Res. 2012, 42, 327–332. [Google Scholar] [CrossRef]
- Holmes, N.; Walker, C.; Tyrer, M.; Kelliher, D. Predicting Chemical Shrinkage in hydrating cements. Polym. Spec. Issue Entitled Cem. Based Compos. Des. Synth. Prop. Rev. 2022. under review. [Google Scholar]
- Holmes, N.; Walker, C.; Tyrer, M.; Kelliher, D. Deriving discrete solid phases from CSH-3T and CSHQ end-members to model cement hydration in PHREEQC. In Proceedings of the Civil Engineering Research in Ireland (CERI) Conference, Dublin, Ireland, 25–26 August 2022; Holmes, N., de Paor, C., West, R., Eds.; CERI/ITRN: Dublin, Ireland, 2022; pp. 28–33. [Google Scholar]
- Lothenbach, B.; Kulik, D.A.; Matschei, T.; Balonis, M.; Baquerizo, L.; Dilnesa, B.; Miron, G.D.; Myers, R.J. Cemdata18: A chemical thermodynamic database for hydrated Portland cements and alkali-activated materials. Cem. Concr. Res. 2019, 115, 472–506. [Google Scholar] [CrossRef] [Green Version]
- Walker, C.; Sutou, S.; Oda, C.; Mihara, M.; Honda, A. Calcium silicate hydrate (C-S-H) gel solubility data and a discrete solid phase model at 25 °C based on two binary non-ideal solid solutions. Cem. Concr. Res. 2016, 79, 1–30. [Google Scholar] [CrossRef]
- Grenthe, I.; Puigdomenech, I. Modelling in Aquatic Chemistry; OECD Publishing: Paris, France, 1997. [Google Scholar]
- Lothenbach, B.; Matschei, T.; Glasser, G.; Möschner, F. Thermodynamic modelling of the effect of temperature on the hydration and porosity of Portland cement. Cem. Concr. Res. 2008, 38, 1–18. [Google Scholar] [CrossRef]






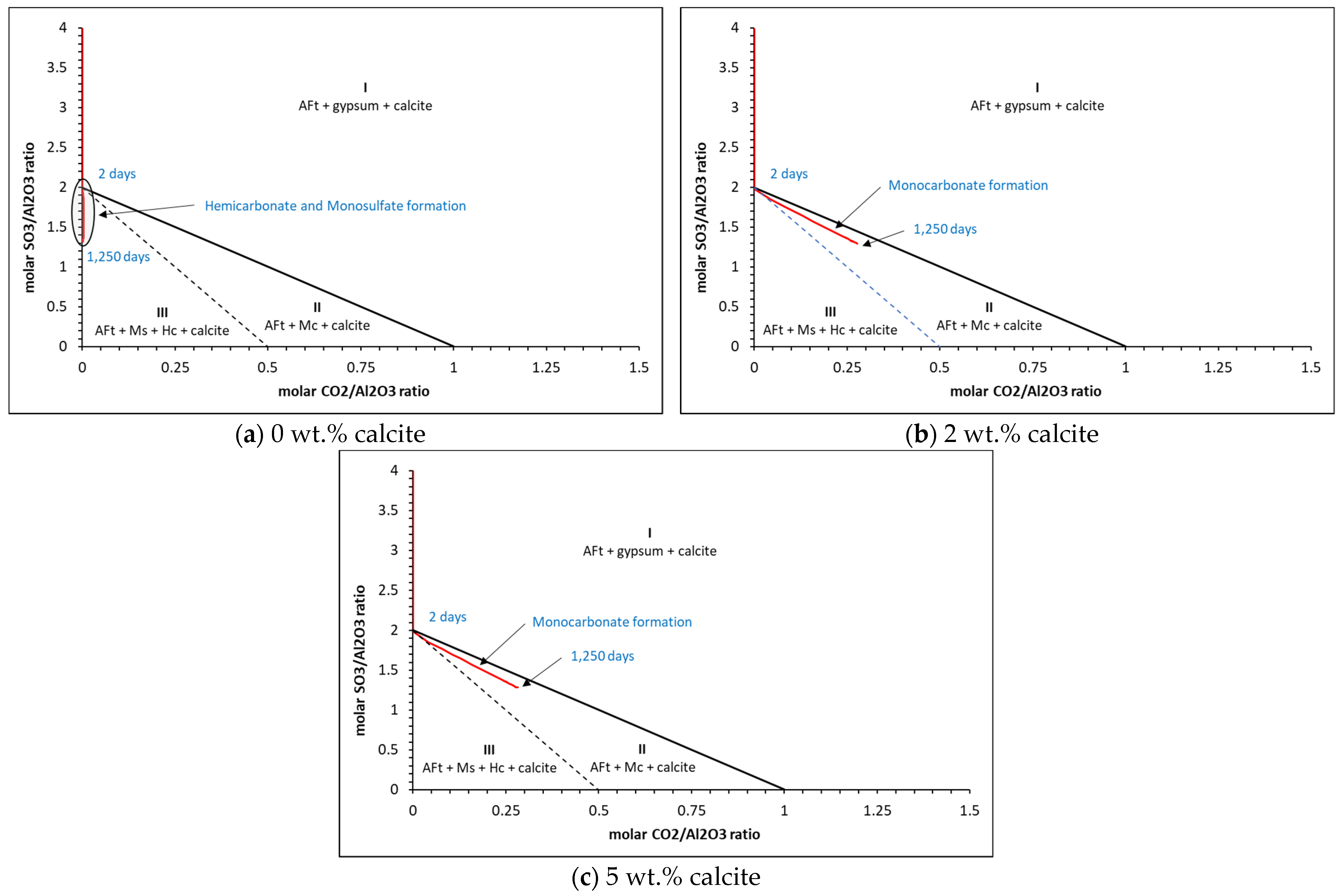
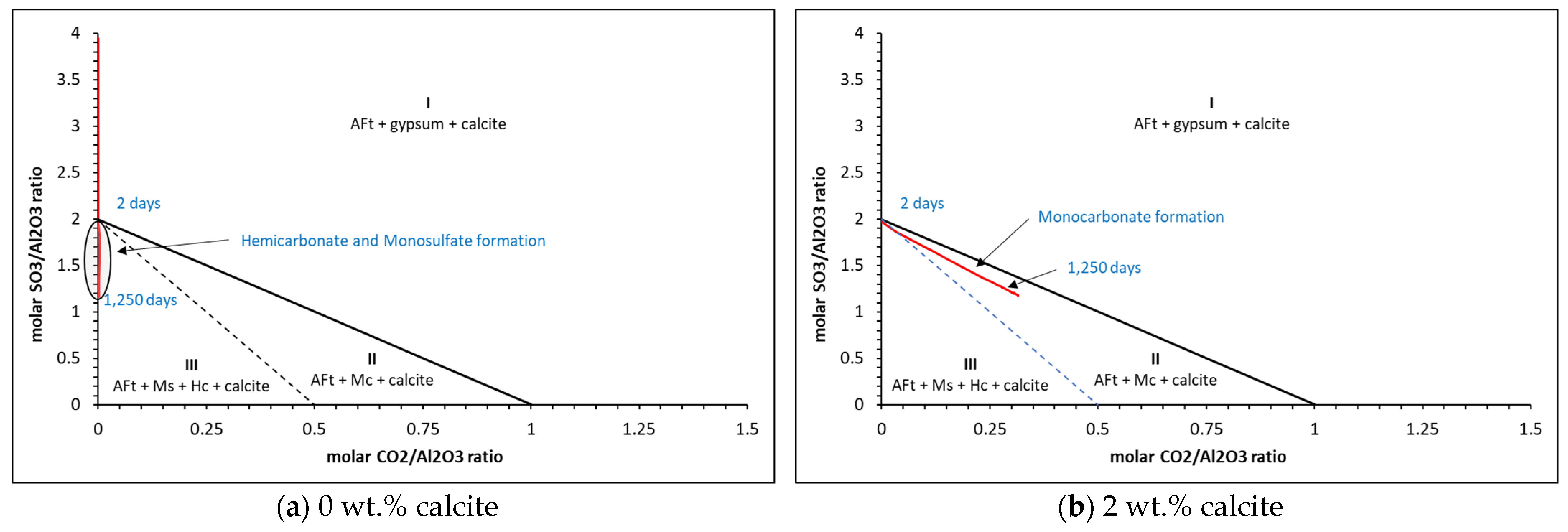
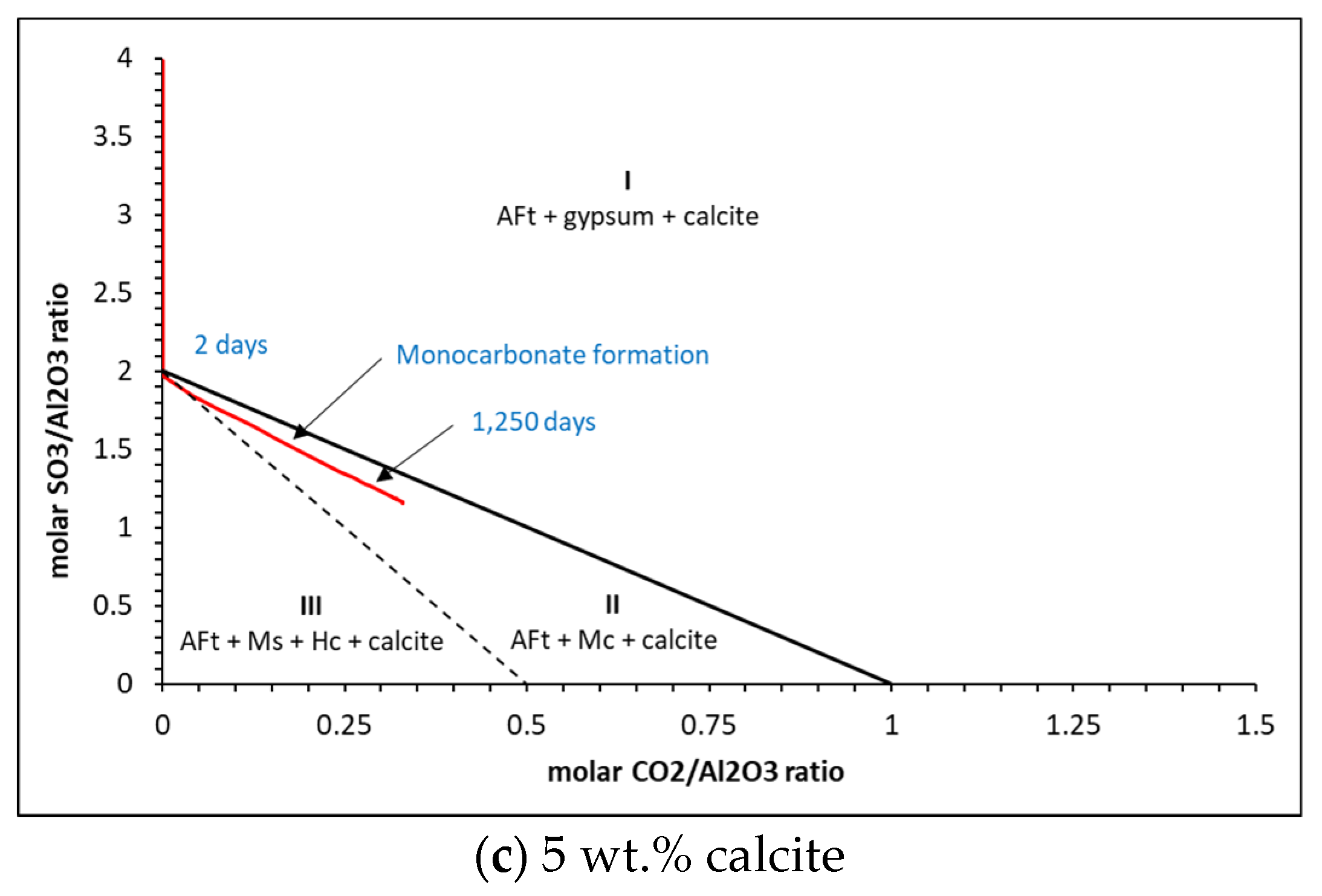
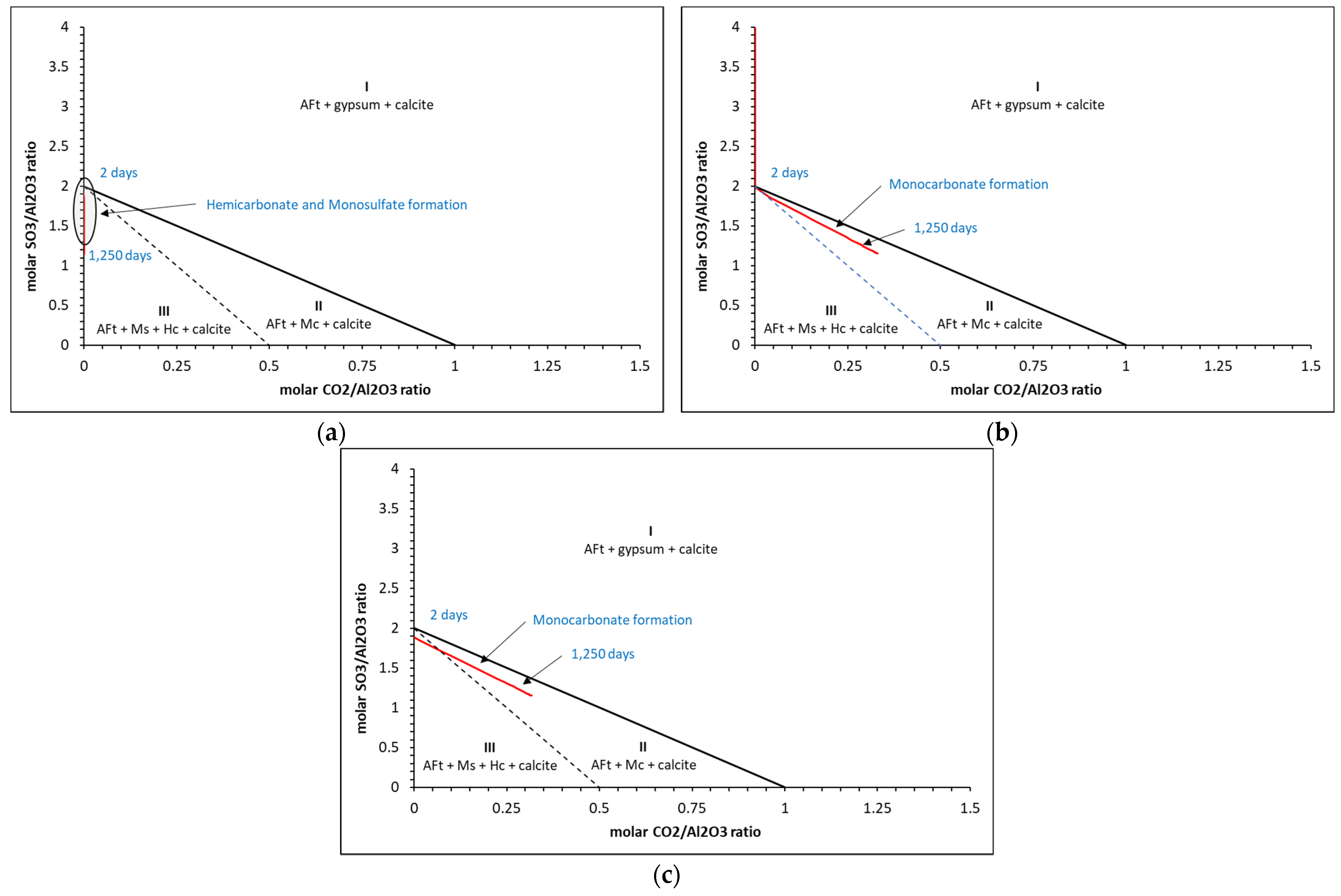

| Oxide | g/100 g | Phase | g/100 g | Moles |
|---|---|---|---|---|
| SiO2 | 20.19 | C3S | 56.62 | 0.2305 |
| Al2O3 | 4.79 | C2S | 16.59 | 0.0963 |
| Fe2O3 | 2.86 | C3A | 7.64 | 0.0283 |
| CaO | 64.59 | C4AF | 8.46 | 0.0174 |
| MgO | 1.68 | Calcite | 5.84 | 0.0583 |
| Na2O | 0.16 | Gypsum | 4.00 | 0.0233 |
| K2O | 0.63 | K2SO4 | 1.02 | 0.0059 |
| CO2 * | 2.64 | Na2SO4 | 0.16 | 0.0011 |
| SO3 | 2.49 | K2O | 0.06 | 0.0007 |
| Blaine fineness (m2/kg) | 419 | Na2O | 0.09 | 0.0014 |
| Loss on Ignition (LOI) | 2.72 | MgO | 1.63 | 0.0405 |
| SO3 | 1.86 | 0.0233 |
| w/c Ratio | Age | Portlandite (%) c.450 °C | Calcite (%) c.700 °C |
|---|---|---|---|
| Unhydrated Clinker | 1.60% | 6.00% | |
| 0.4 | 1-day | 10.40 | 4.16 |
| 7 days | 14.39 | 3.95 | |
| 28 days | 15.34 | 4.02 | |
| 0.5 | 1-day | 11.35 | 4.07 |
| 7 days | 15.62 | 3.75 | |
| 28 days | 16.65 | 3.27 | |
| 0.6 | 1-day | 10.68 | 3.59 |
| 7 days | 15.42 | 3.23 | |
| 28 days | 16.61 | 3.21 |
| Mineral Name [Composition] | Dissolution Reaction | |||||||
|---|---|---|---|---|---|---|---|---|
| SO3 [SO3] | vi | Component | ∆fGom (J/mol) | ∆fHom (J/mol) | Som (J/K/mol) | Cpom (J/K/mol) | RMM (g/mol) | Z (-) |
| −1 | SO3 | −374,141 | −451,663 | 79.496 | No data | 80.0642 | 0 | |
| −1 | H2O | −237,181.38 | −285,837.3 | 69.92418 | 75.360,526 | 18.01528 | 0 | |
| 2 | H+ | 0 | 0 | 0 | 0 | 1.00794 | 1 | |
| 1 | SO4−2 | −744,459 | −909,697 | 18.82801 | −266.09072 | 96.0636 | −2 | |
| ∆rΞom | −133,136.62 | −172,196.7 | −130.59217 | NAN | --- | 0 | ||
| Phase description for PHREEQC PHASE data block | ||||||||
| SO3 + 1 H2O = + 2 H+ + 1 SO4−2 | ||||||||
| log_k 23.3244 | ||||||||
| delta_h −172.197 kJ/mol | ||||||||
| analytical_expression | −6.821263 | 0 | 8994.406 | 0 | 0 | |||
| MgO [MgO] | vi | Component | ∆fGom (J/mol) | ∆fHom (J/mol) | Som (J/K/mol) | Cpom (J/K/mol) | RMM (g/mol) | Z (-) |
| −1 | MgO | −569,314 | −601,600 | 26.95 | 37.237 | 40.3044 | 0 | |
| −2 | H+ | 0 | 0 | 0 | 0 | 1.00794 | 1 | |
| 1 | Mg+2 | −453,985 | −465,929 | −138.072 | −21.662091 | 24.305 | 2 | |
| 1 | H2O | −237,181.38 | −285,837.3 | 69.92418 | 75.360526 | 18.01528 | 0 | |
| ∆rΞom | −121,852.7454 | −150,166.3 | −95.09782 | 16.461435 | --- | 0 | ||
| Phase description for PHREEQC PHASE data block | ||||||||
| MgO + 2 H+ = + 1 Mg+2 + 1 H2O | ||||||||
| log_k 21.3476 | ||||||||
| delta_h−150.166 kJ/mol | ||||||||
| analytical_expression | −10.72611 | 0 | 8100.045 | 1.979844 | 0 | |||
| Periclase [MgO] | vi | Component | ∆fGom (J/mol) | ∆fHom (J/mol) | Som (J/K/mol) | Cpom (J/K/mol) | RMM (g/mol) | Z (-) |
| −1 | MgO | −569,314 | −601,600 | 26.95 | 37.237 | 40.3044 | 0 | |
| −2 | H+ | 0 | 0 | 0 | 0 | 1.00794 | 1 | |
| 1 | Mg+2 | −453,985 | −465,929 | −138.072 | −21.662091 | 24.305 | 2 | |
| 1 | H2O | −237,181.38 | −285,837.3 | 69.92418 | 75.360526 | 18.01528 | 0 | |
| ∆rΞom | −121,852.7454 | −150,166.3 | −95.09782 | 16.461435 | --- | 0 | ||
| Phase description for PHREEQC PHASE data block | ||||||||
| MgO + 2H+ = + 1Mg+2 + 1H2O | ||||||||
| log_k 21.3476 | ||||||||
| delta_h −150.166 kJ/mol | ||||||||
| analytical_expression | −10.72611 | 0 | 8100.045 | 1.979844 | 0 | |||
| w/c Ratio | Time (Days) | Portlandite | Calcite | ||
|---|---|---|---|---|---|
| Measured (%) | Predicted (%) | Measured (%) | Predicted (%) | ||
| 0.4 | 1 day | 10.41 | 6.44 | 4.16 | 3.19 |
| 7 days | 14.39 | 12.34 | 3.95 | 2.67 | |
| 28 days | 15.34 | 13.41 | 4.02 | 2.65 | |
| 0.5 | 1 day | 11.35 | 5.59 | 4.07 | 2.78 |
| 7 days | 15.62 | 10.77 | 3.75 | 2.32 | |
| 28 days | 16.65 | 11.85 | 3.27 | 3.27 | |
| 0.6 | 1 day | 10.68 | 4.94 | 3.59 | 2.46 |
| 7 days | 15.42 | 9.52 | 3.23 | 2.05 | |
| 28 days | 16.61 | 10.50 | 3.21 | 1.95 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Holmes, N.; Russell, M.; Davis, G.; Tyrer, M. Comparing the Measured and Thermodynamically Predicted AFm Phases in a Hydrating Cement. Appl. Sci. 2022, 12, 10147. https://doi.org/10.3390/app121910147
Holmes N, Russell M, Davis G, Tyrer M. Comparing the Measured and Thermodynamically Predicted AFm Phases in a Hydrating Cement. Applied Sciences. 2022; 12(19):10147. https://doi.org/10.3390/app121910147
Chicago/Turabian StyleHolmes, Niall, Mark Russell, Geoff Davis, and Mark Tyrer. 2022. "Comparing the Measured and Thermodynamically Predicted AFm Phases in a Hydrating Cement" Applied Sciences 12, no. 19: 10147. https://doi.org/10.3390/app121910147
APA StyleHolmes, N., Russell, M., Davis, G., & Tyrer, M. (2022). Comparing the Measured and Thermodynamically Predicted AFm Phases in a Hydrating Cement. Applied Sciences, 12(19), 10147. https://doi.org/10.3390/app121910147








