Comparison between Different Re-Entry Technologies for Debris Mitigation in LEO
Abstract
:1. Introduction
2. Deorbiting Technologies
3. Materials and Methods
4. Results
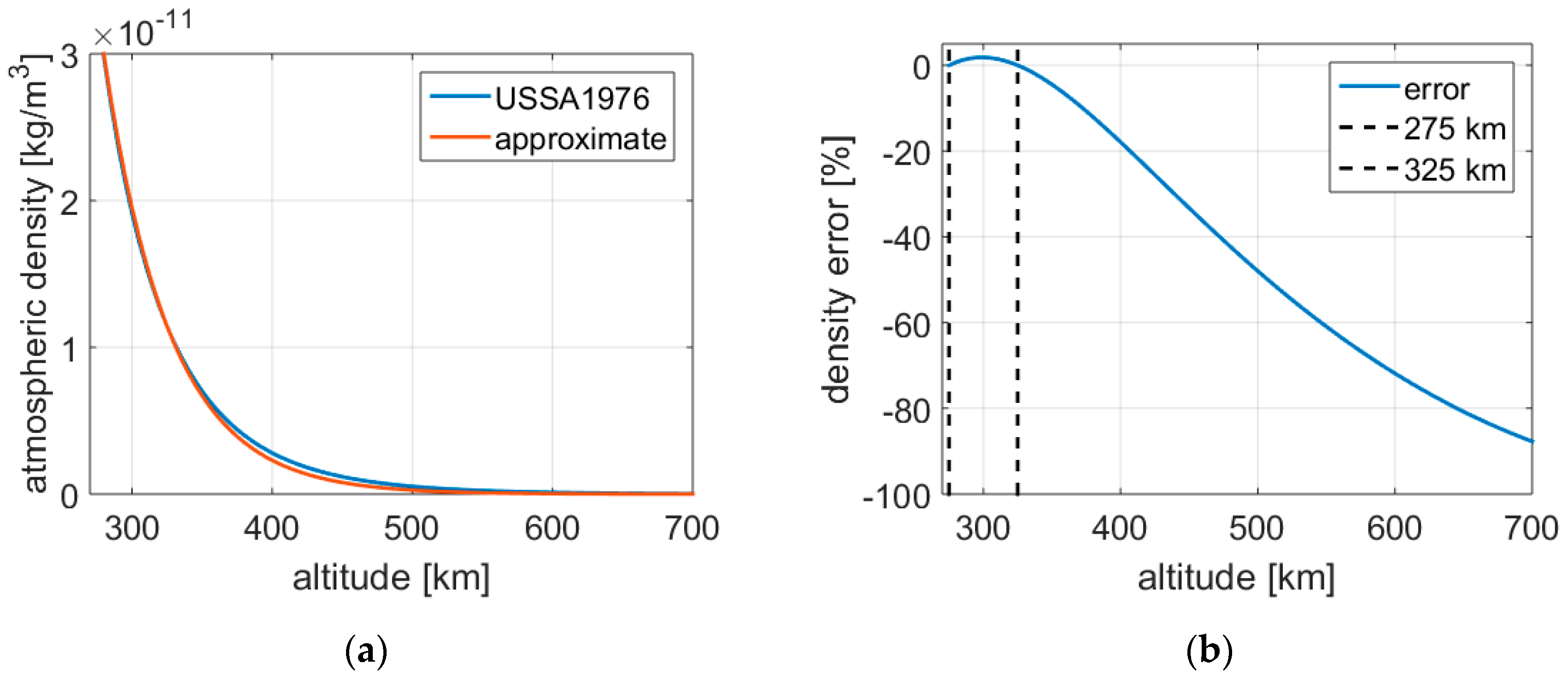
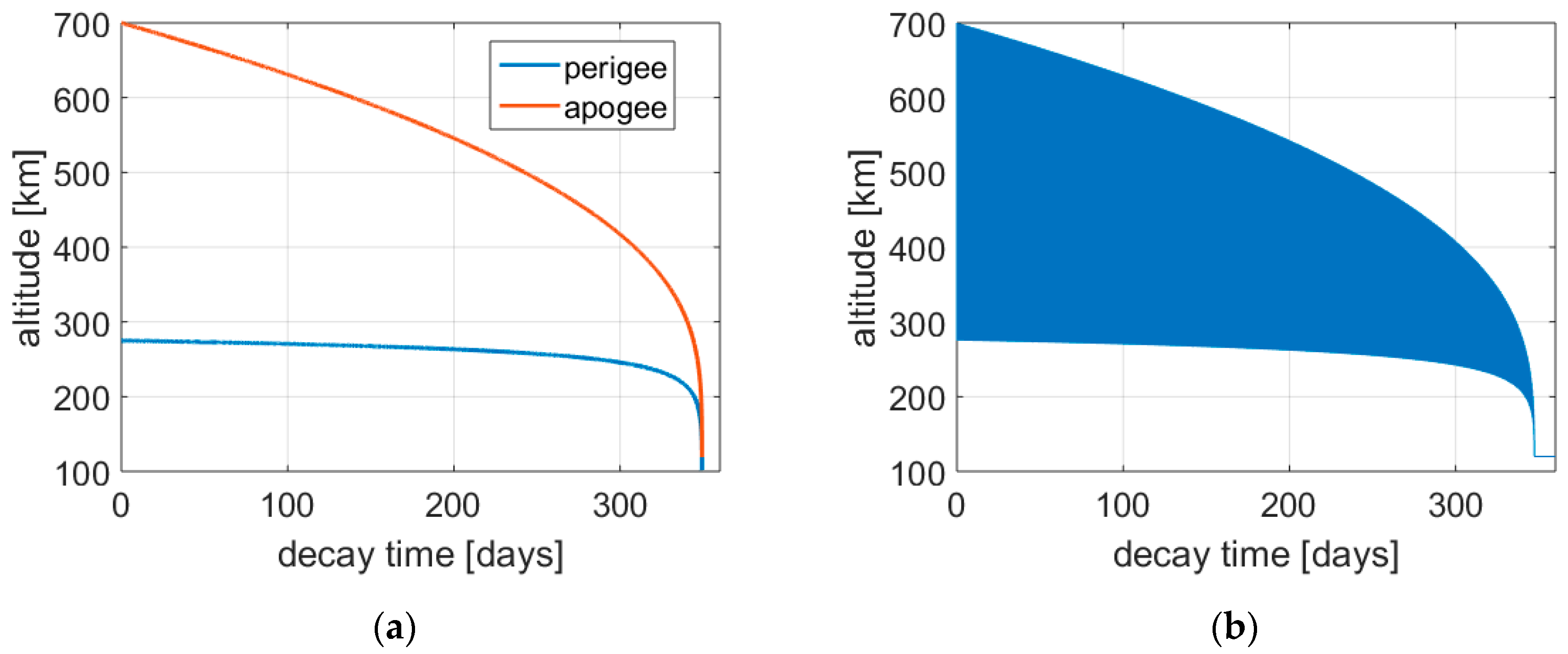
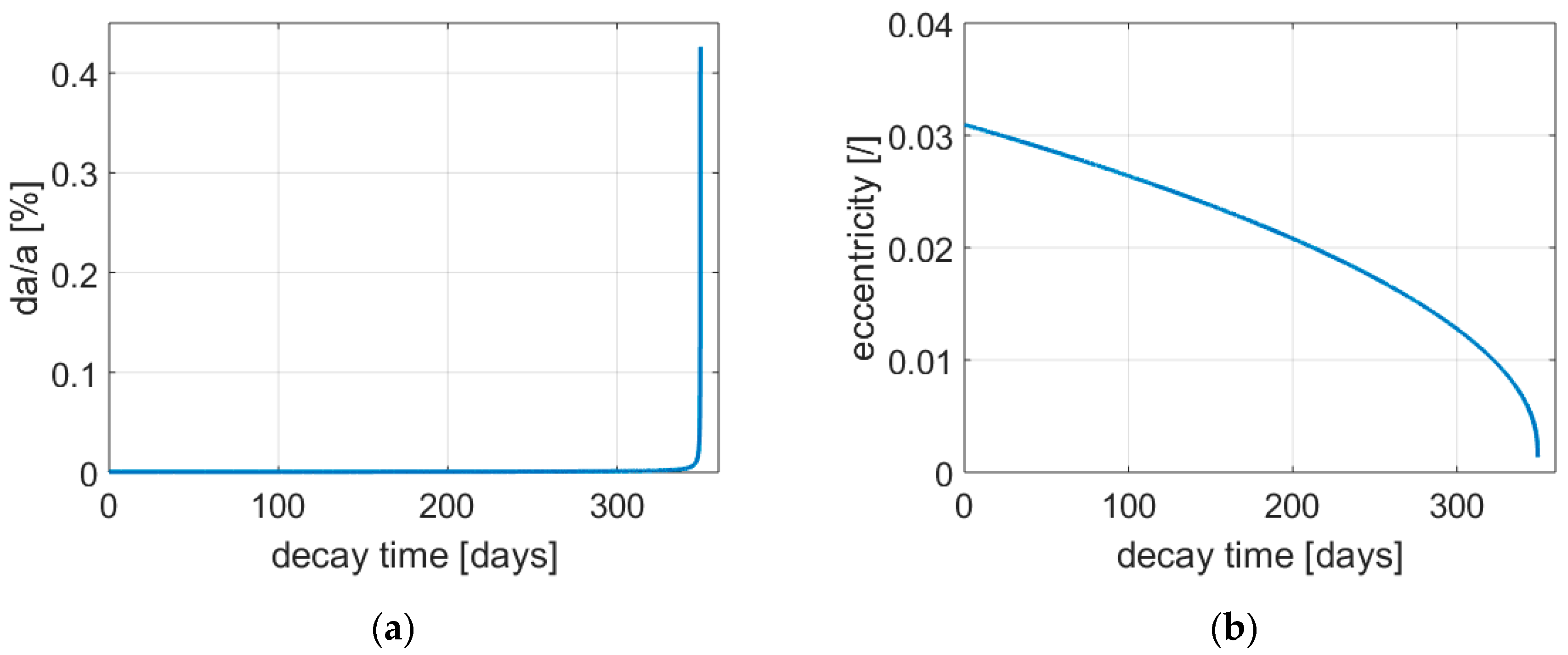
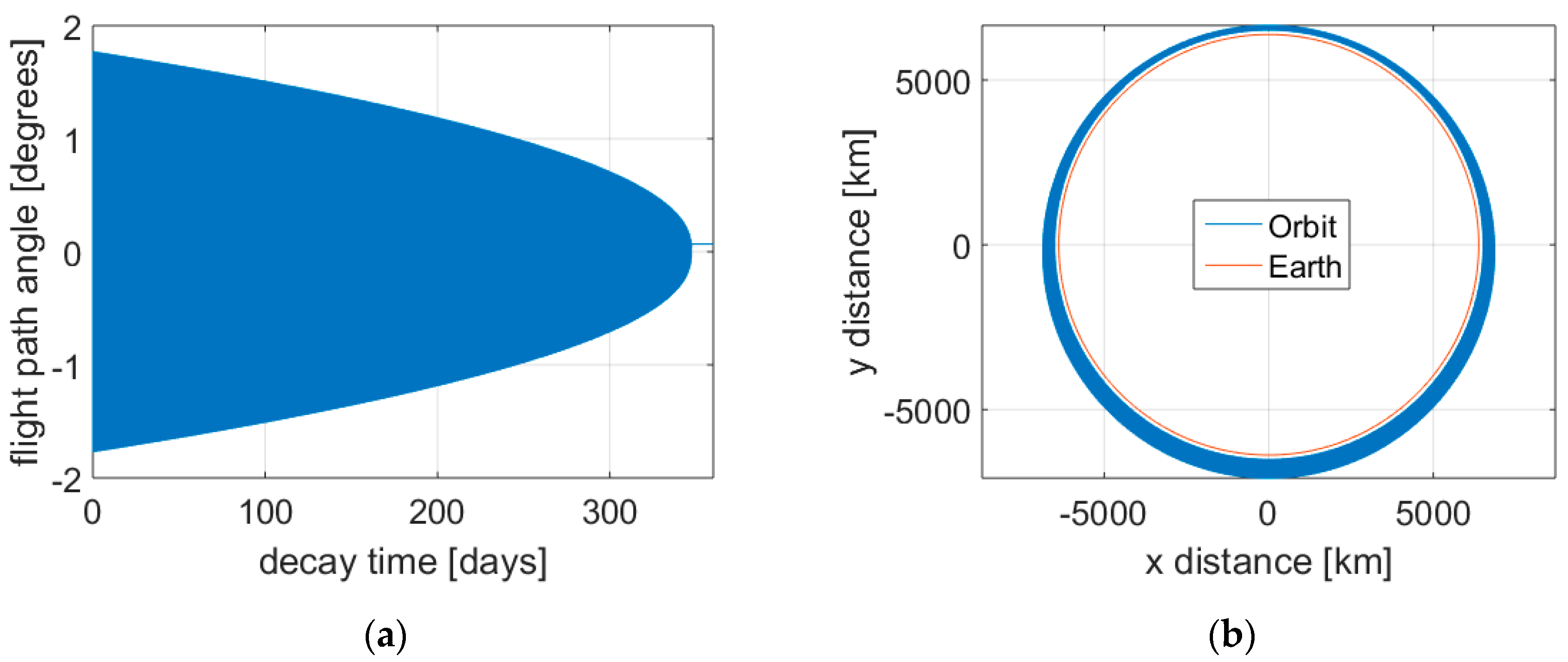
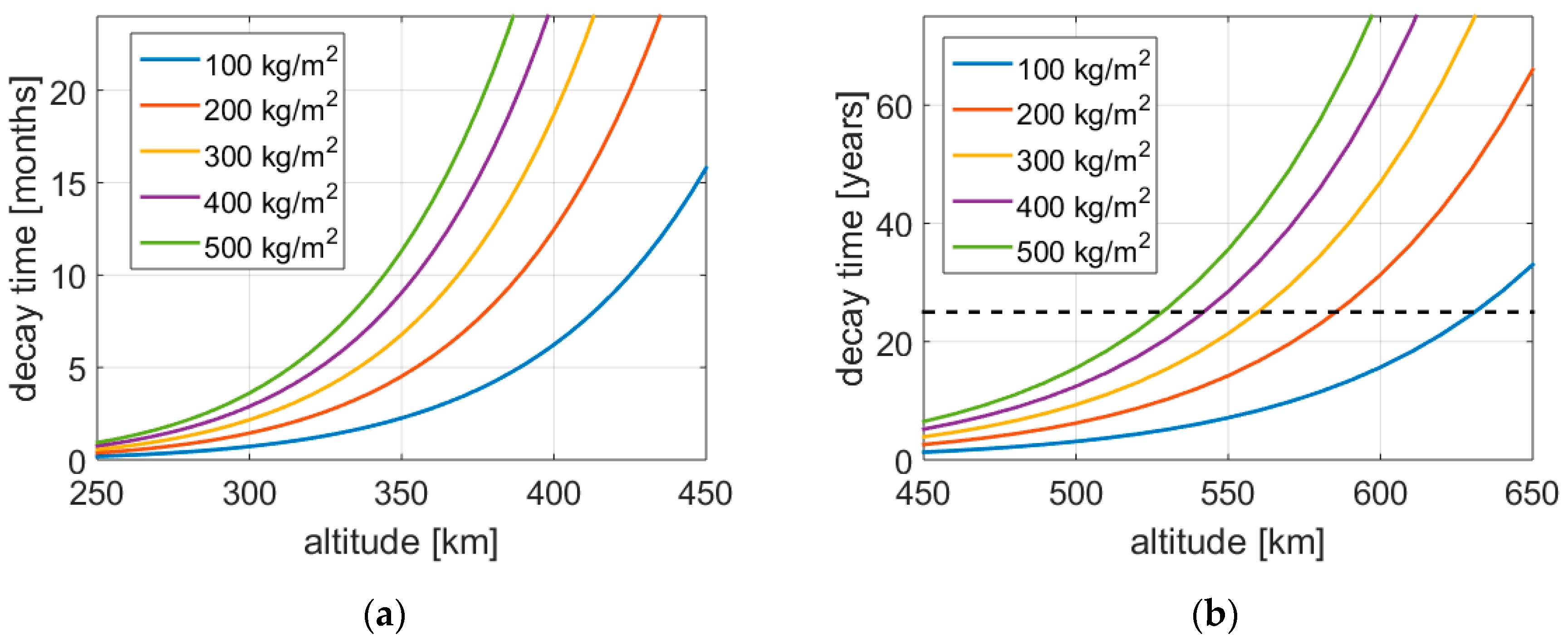
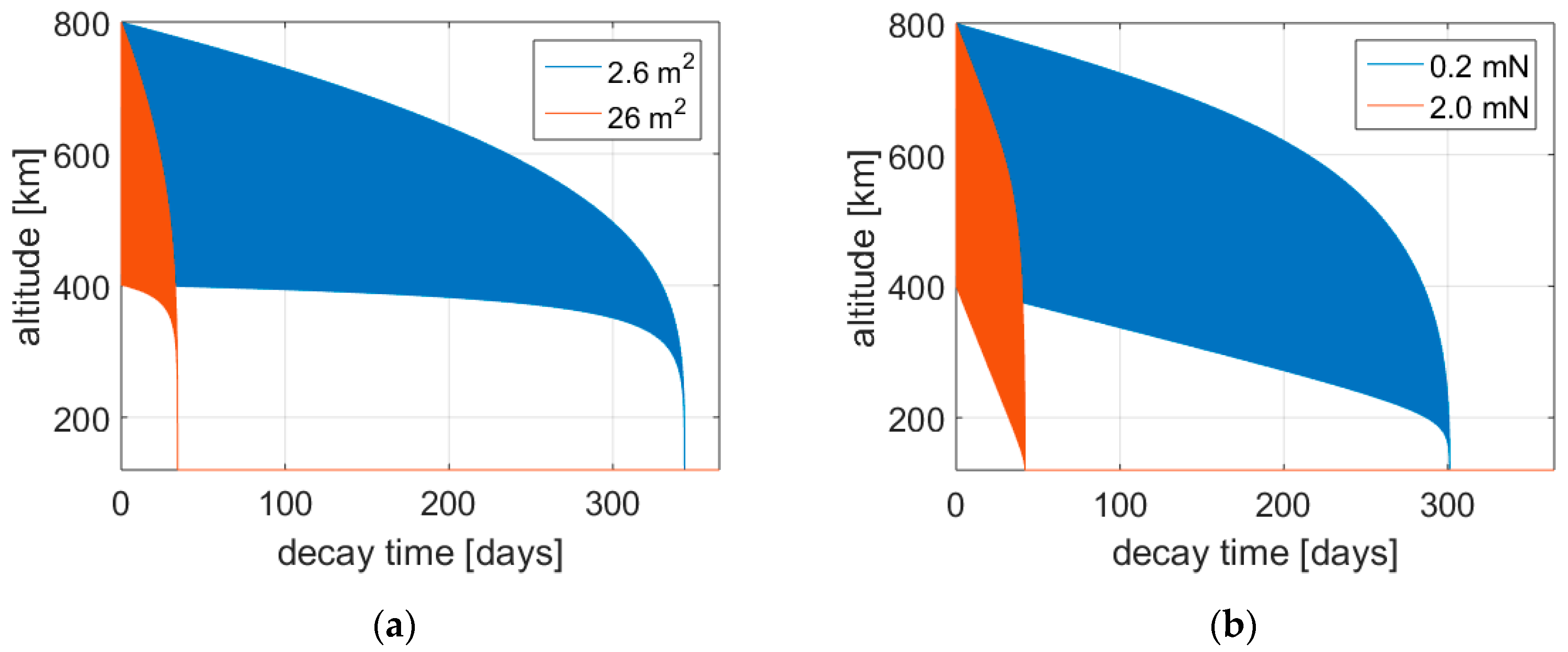
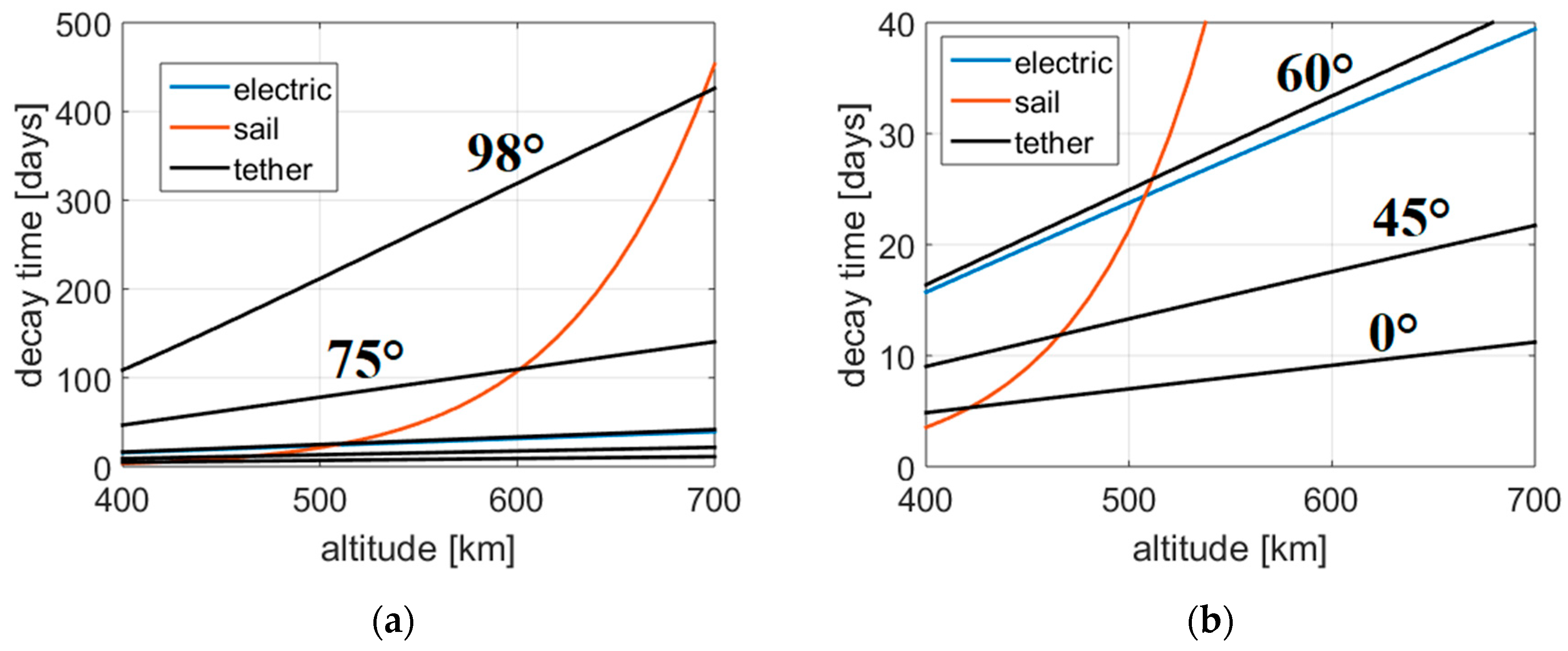
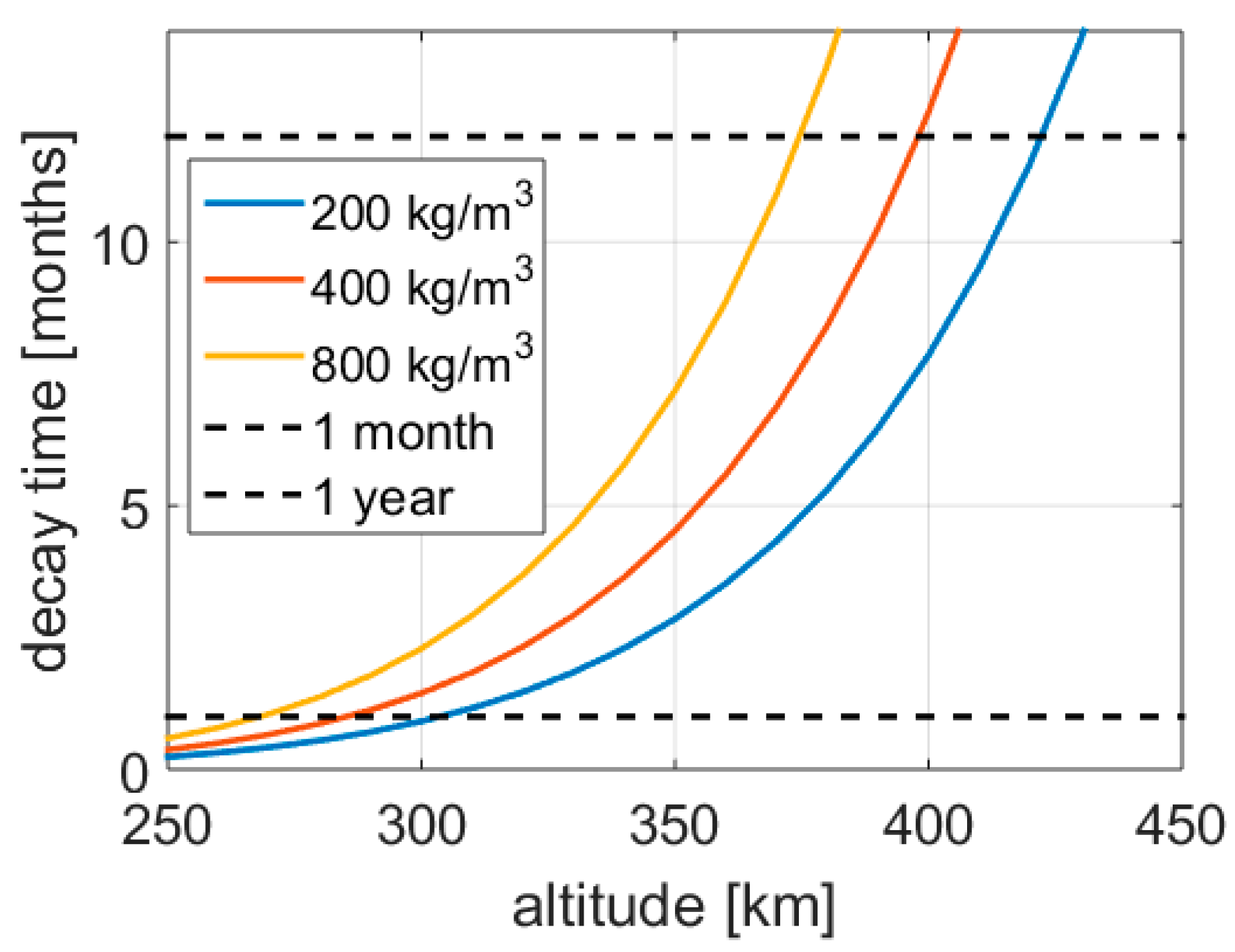
4.1. Natural Decay
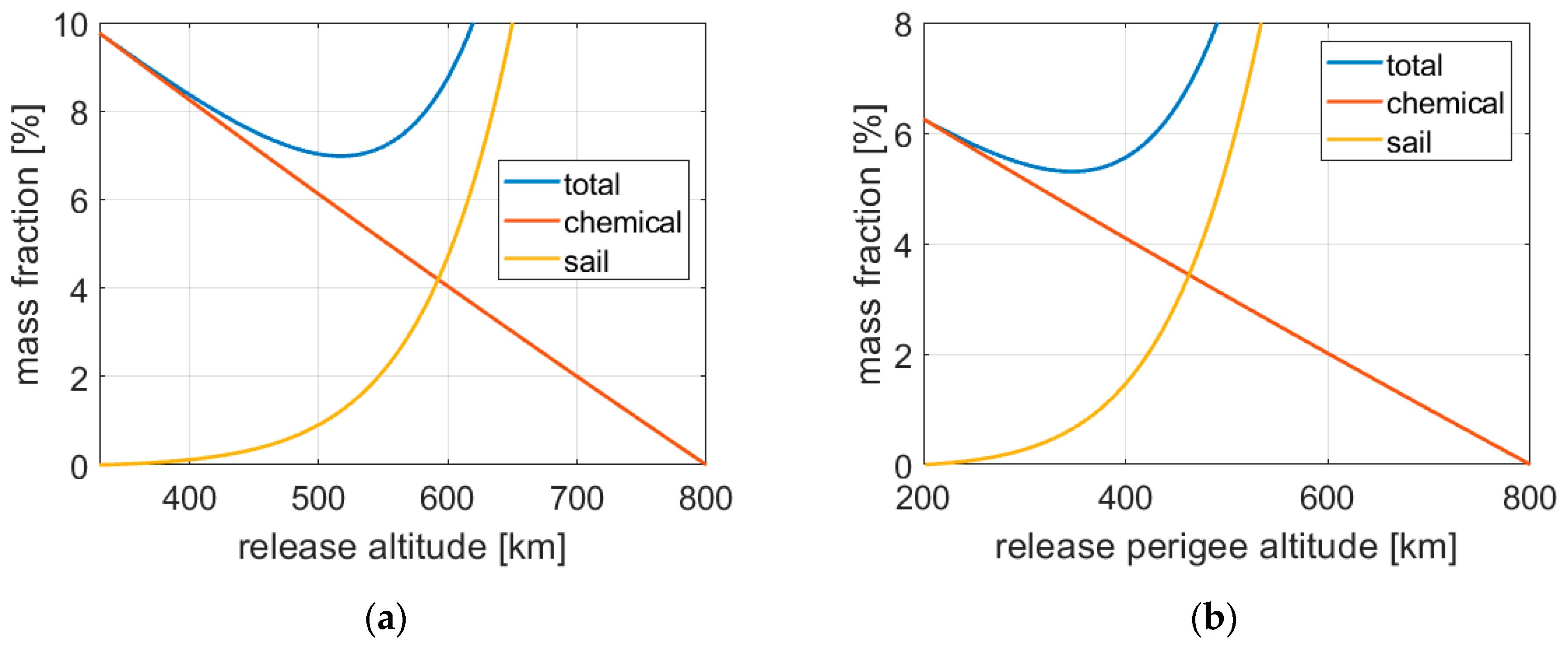
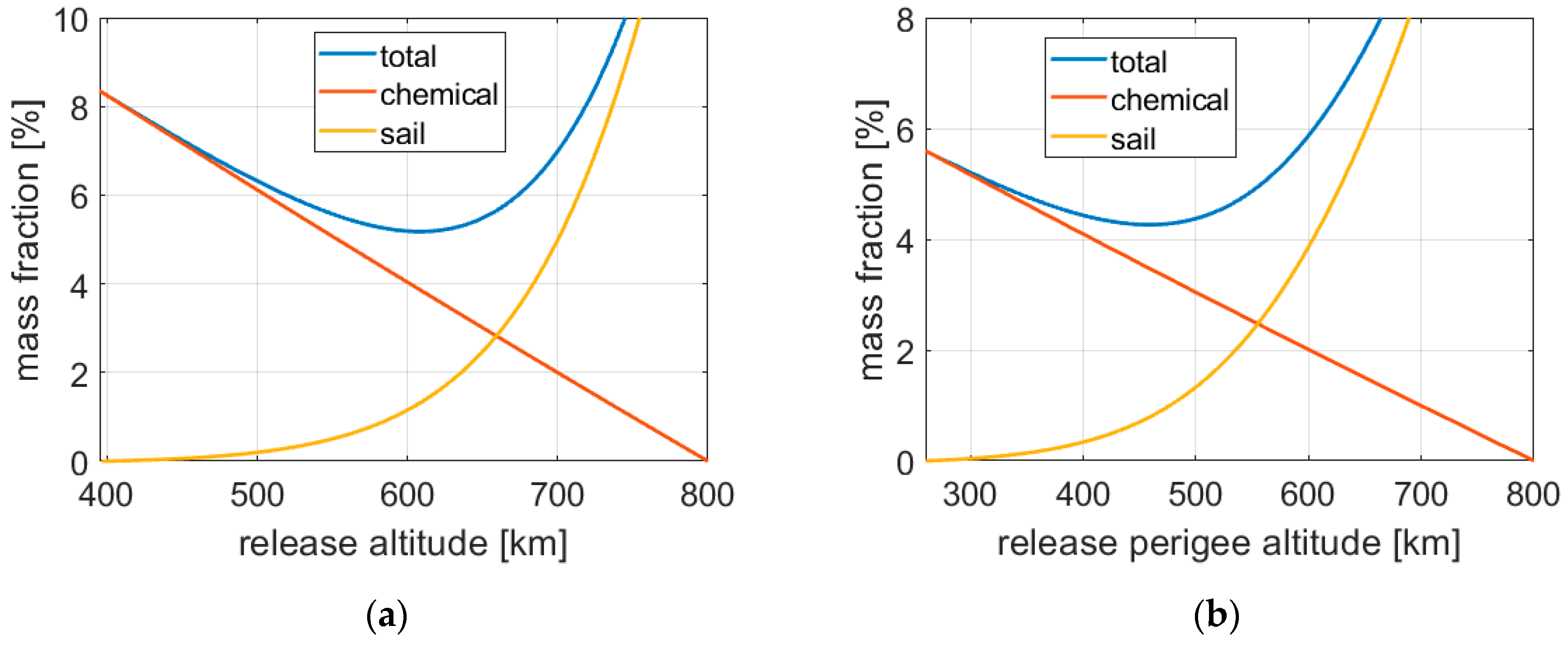
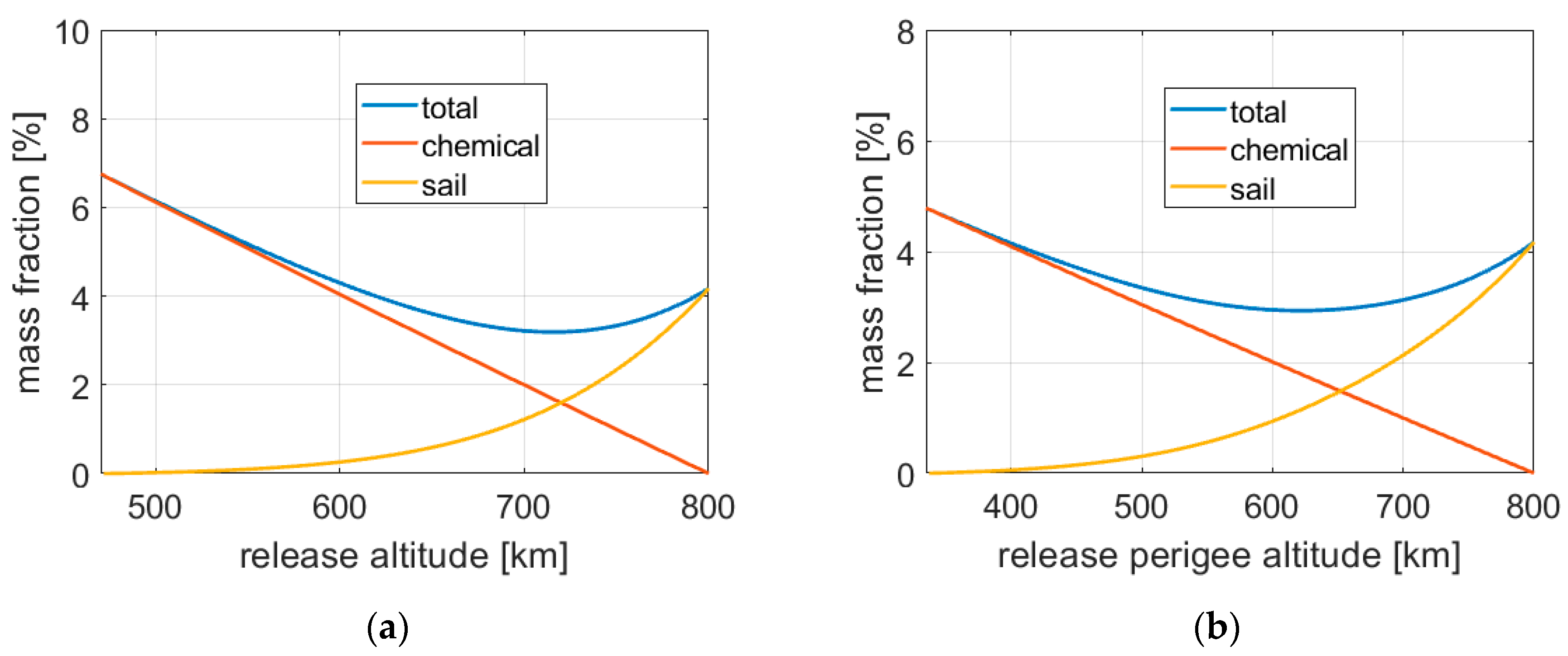
4.2. Drag Sail
- The impact of small debris with the sail has probably fewer effects and consequences than an impact with the satellite;
- In case of cooperative systems, a collision avoidance process can occur [49]. This maneuver is based on an uncertainty area of impact that is much larger than the satellite itself and is thus not a linear (but rather sublinear) function of the satellite area. So, while the probability of passive impact remains constant, the probability of a collision avoidance maneuver by a third party is definitely reduced as the falling time is shortened;
- It is much easier and cheaper to track a large falling satellite for a shorter time (with the sail) than its original, way smaller, counterpart for a much longer time.
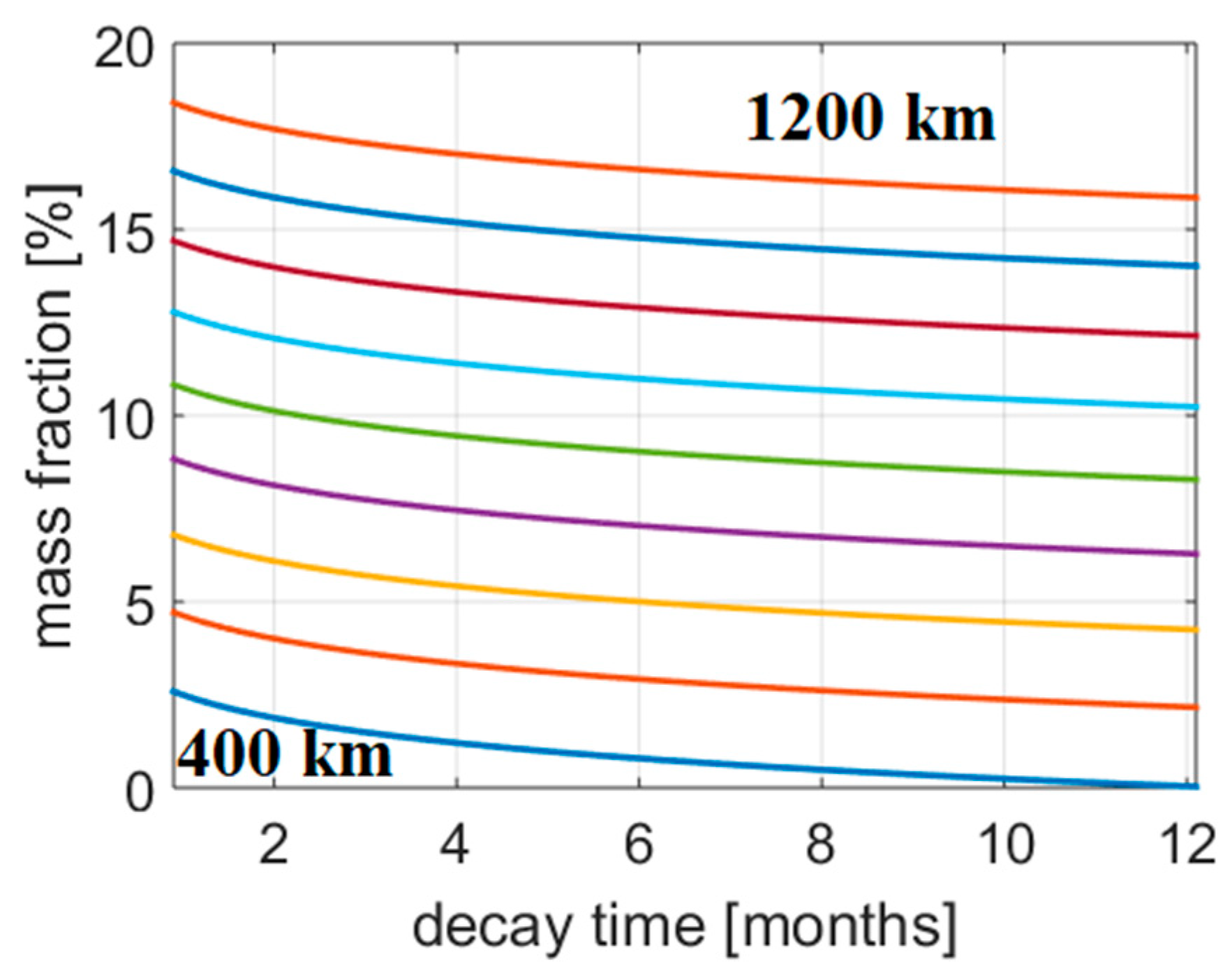
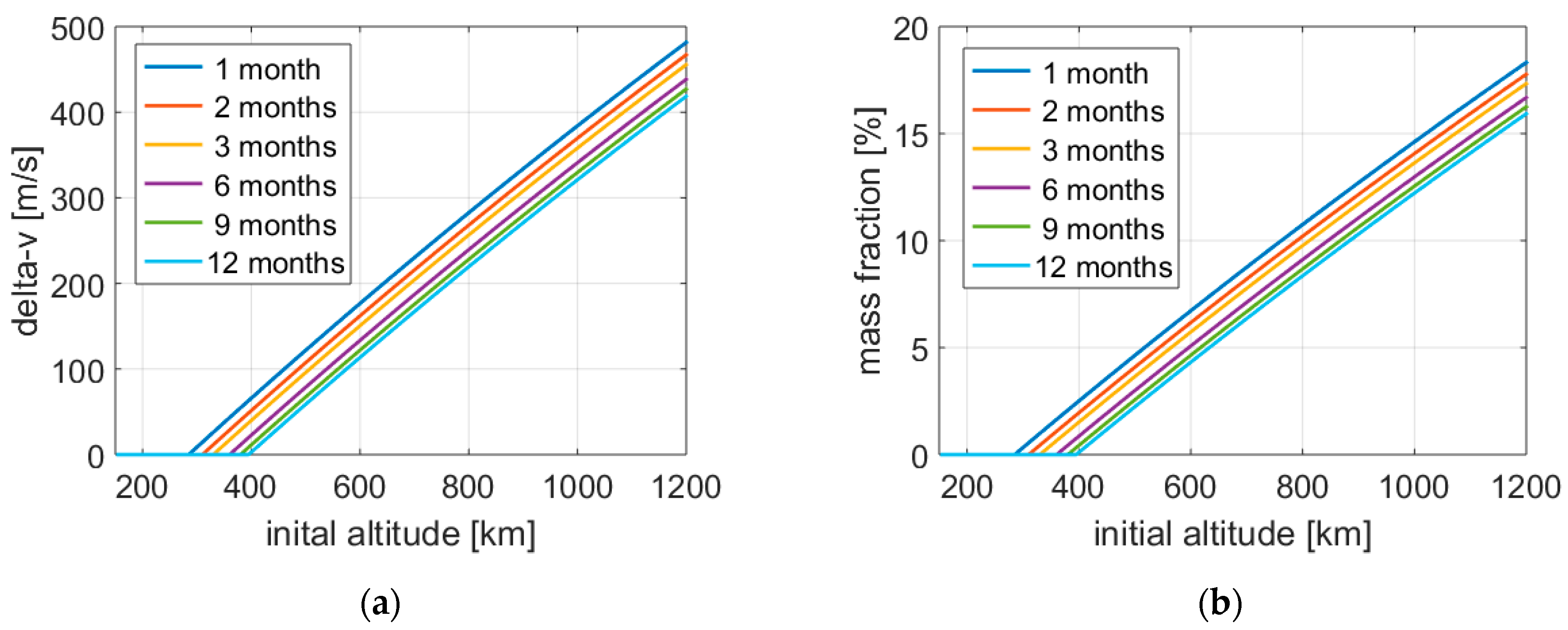
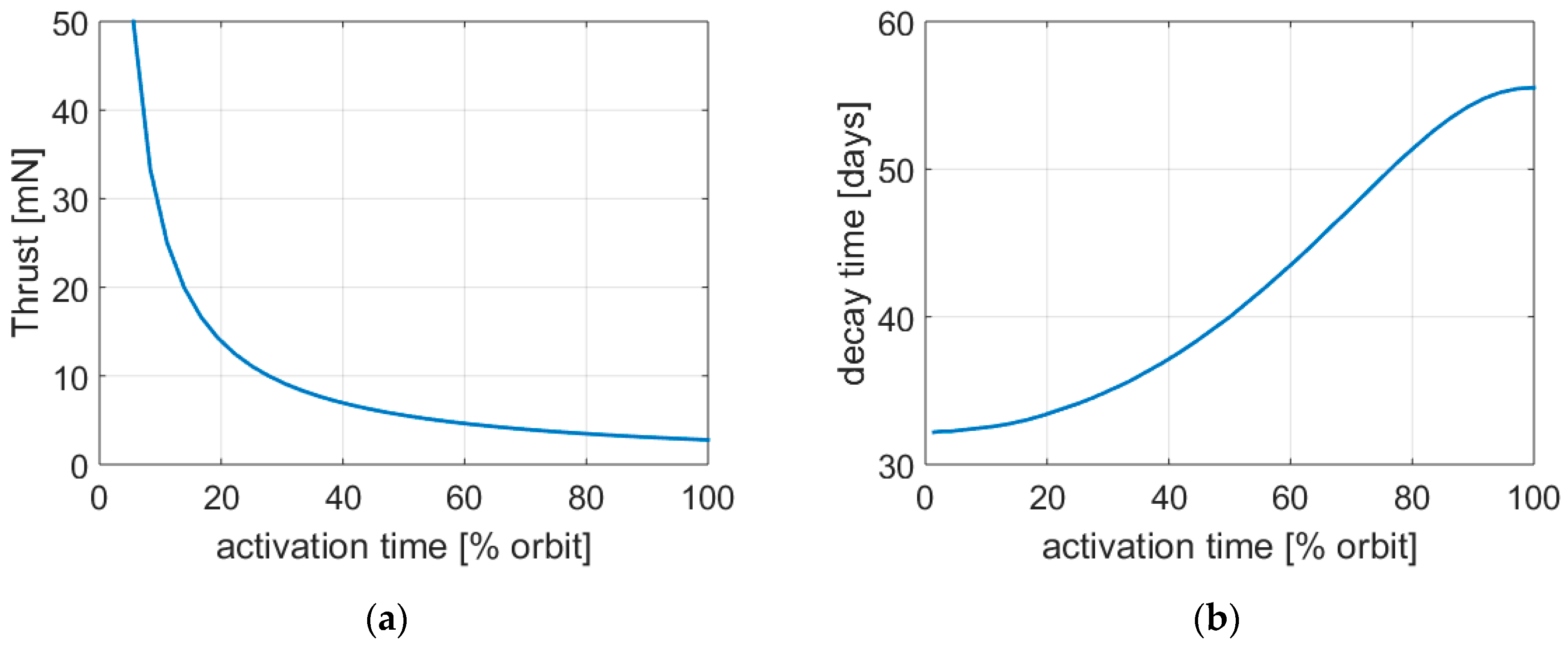
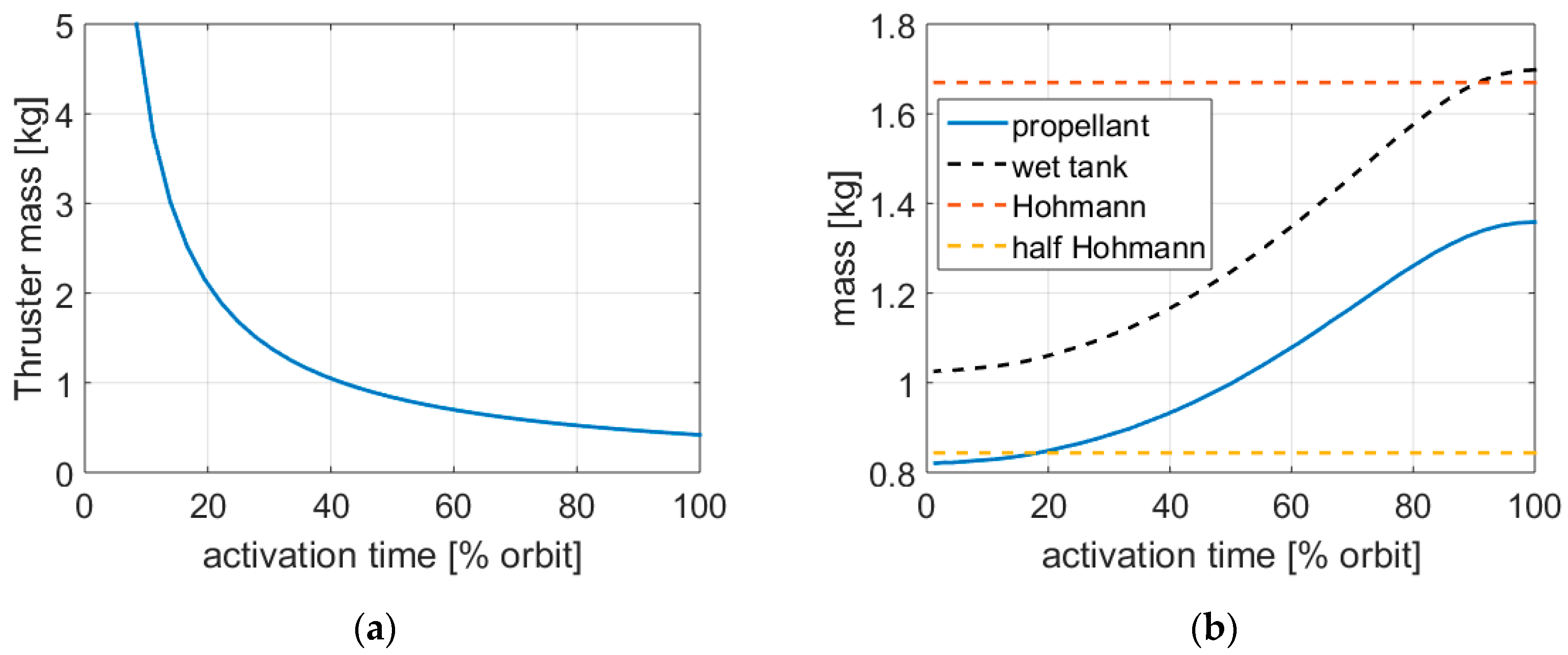
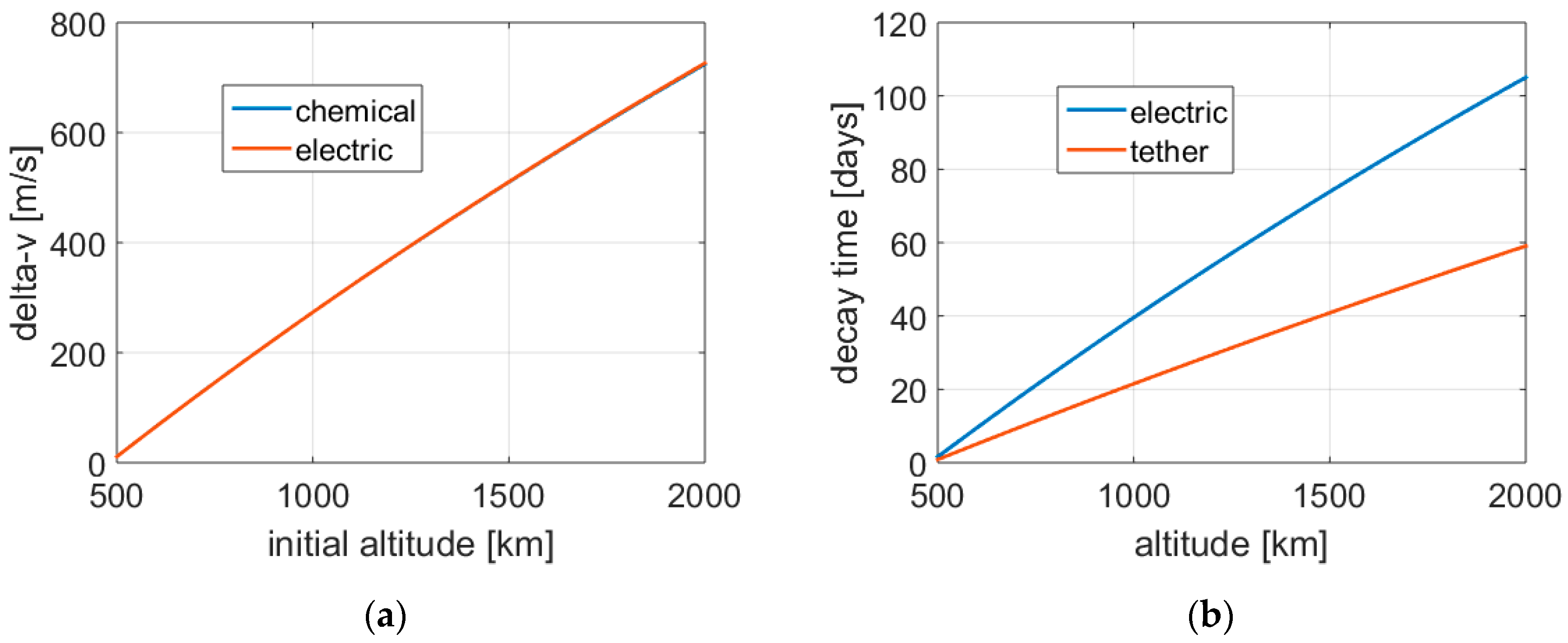
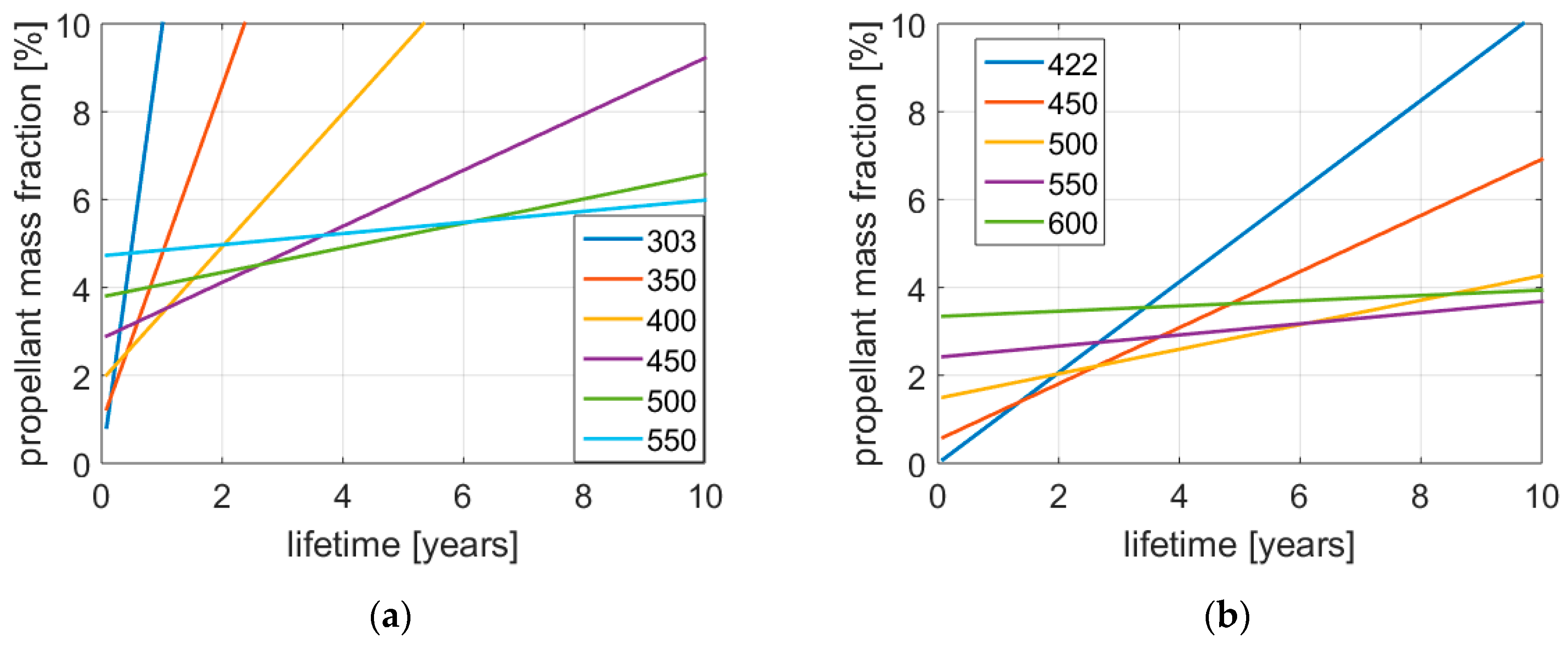
4.3. Chemical Propulsion
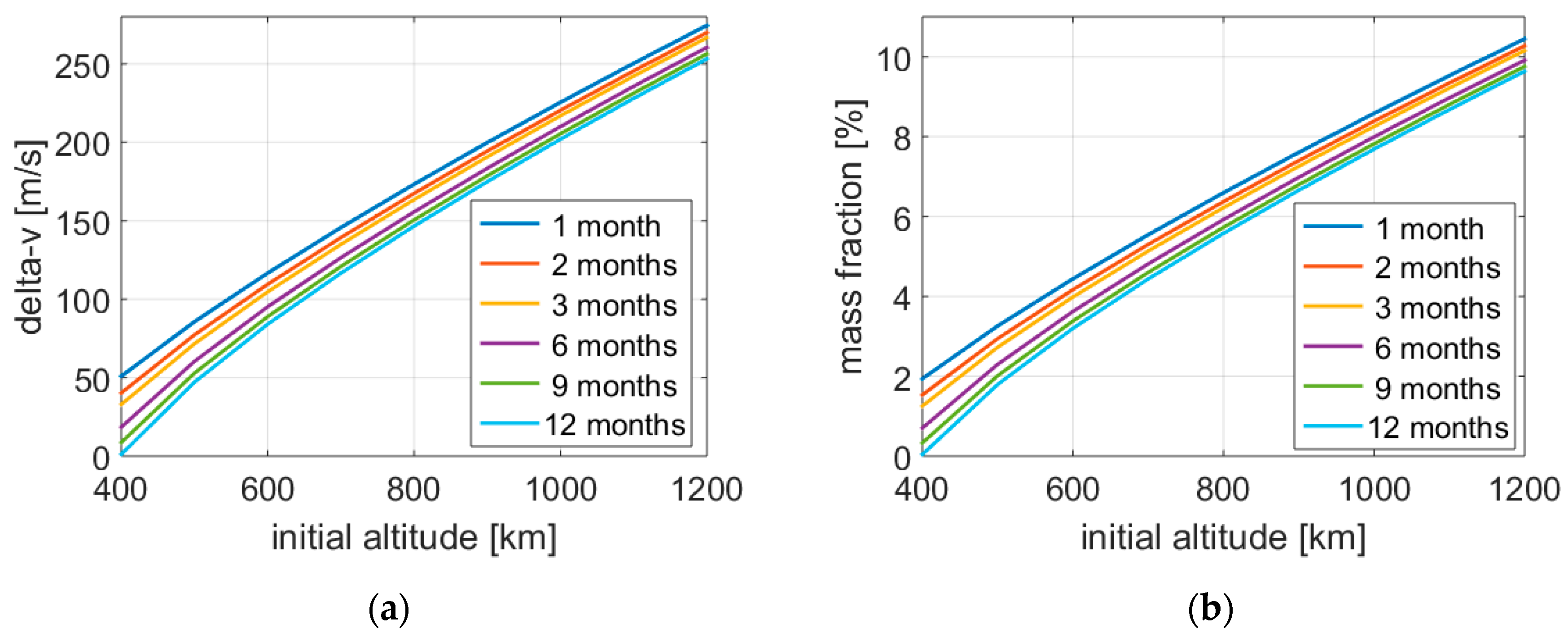
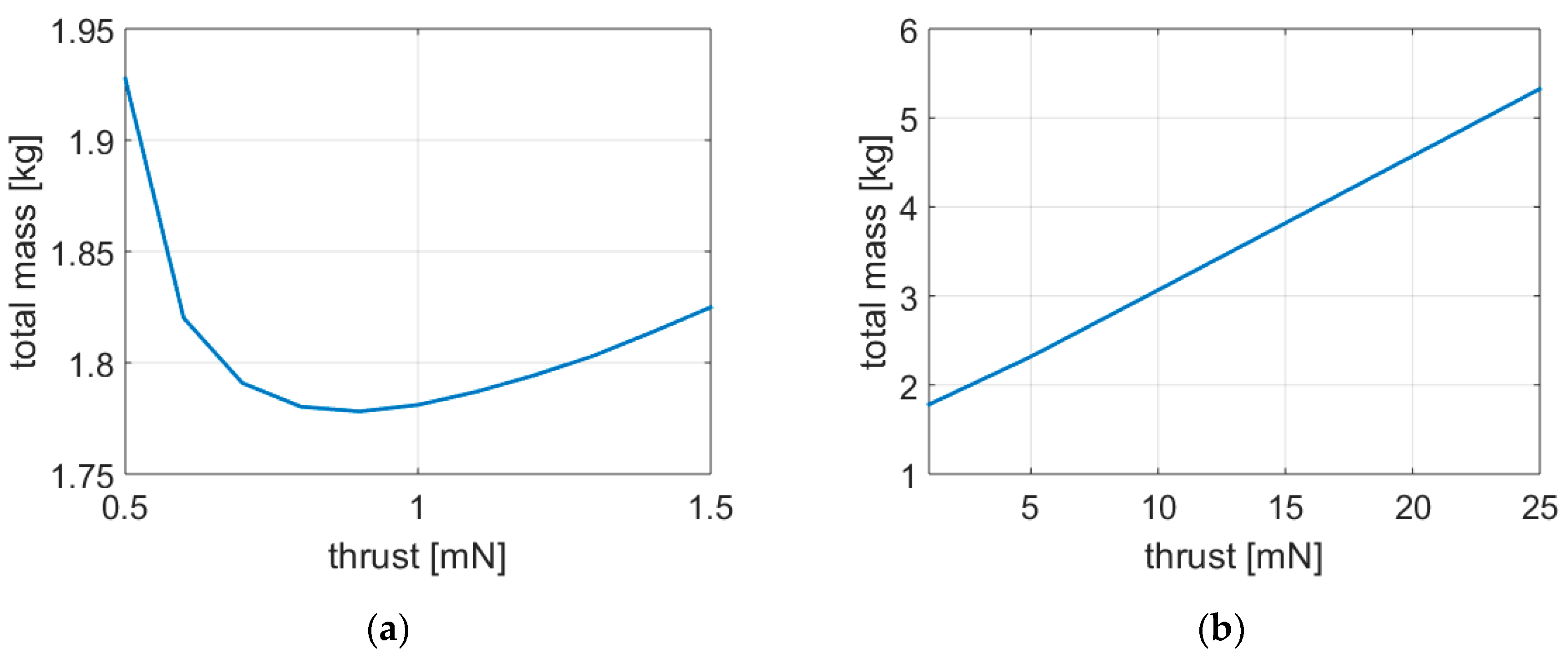
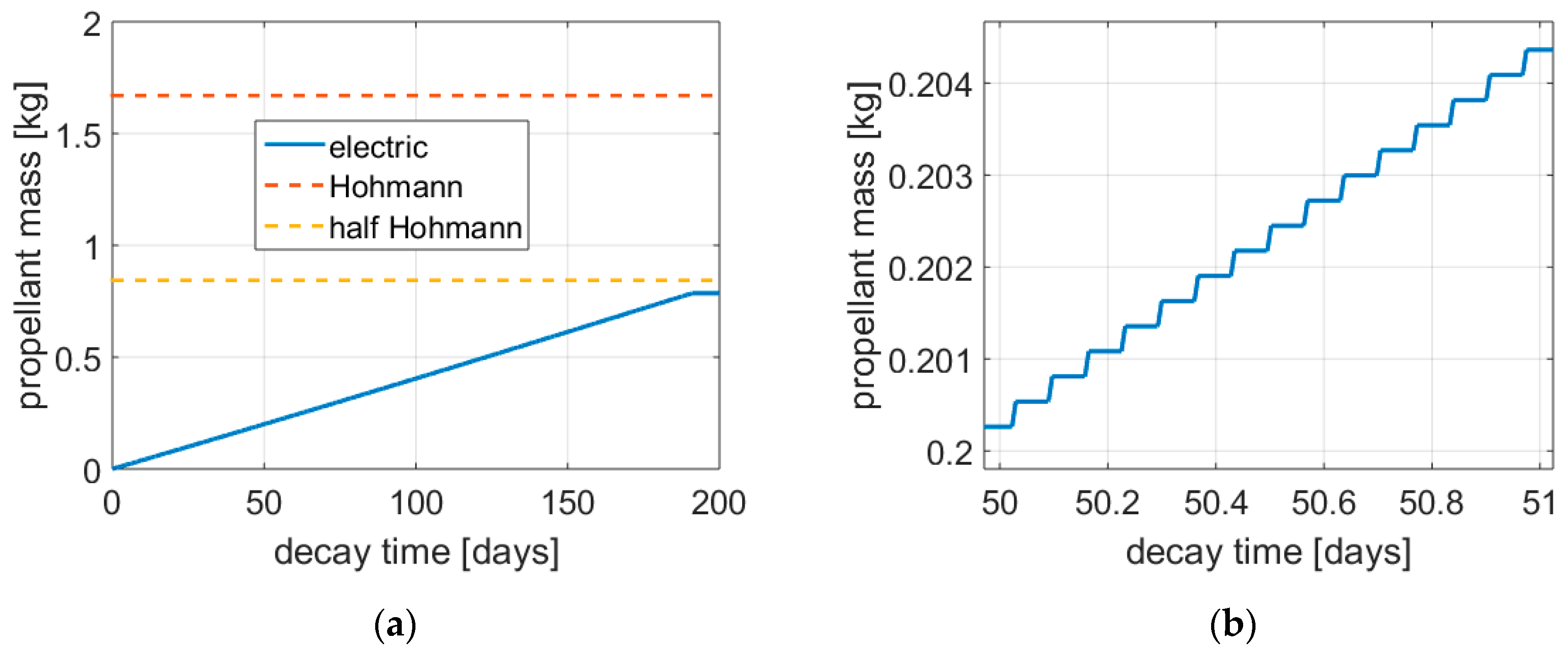
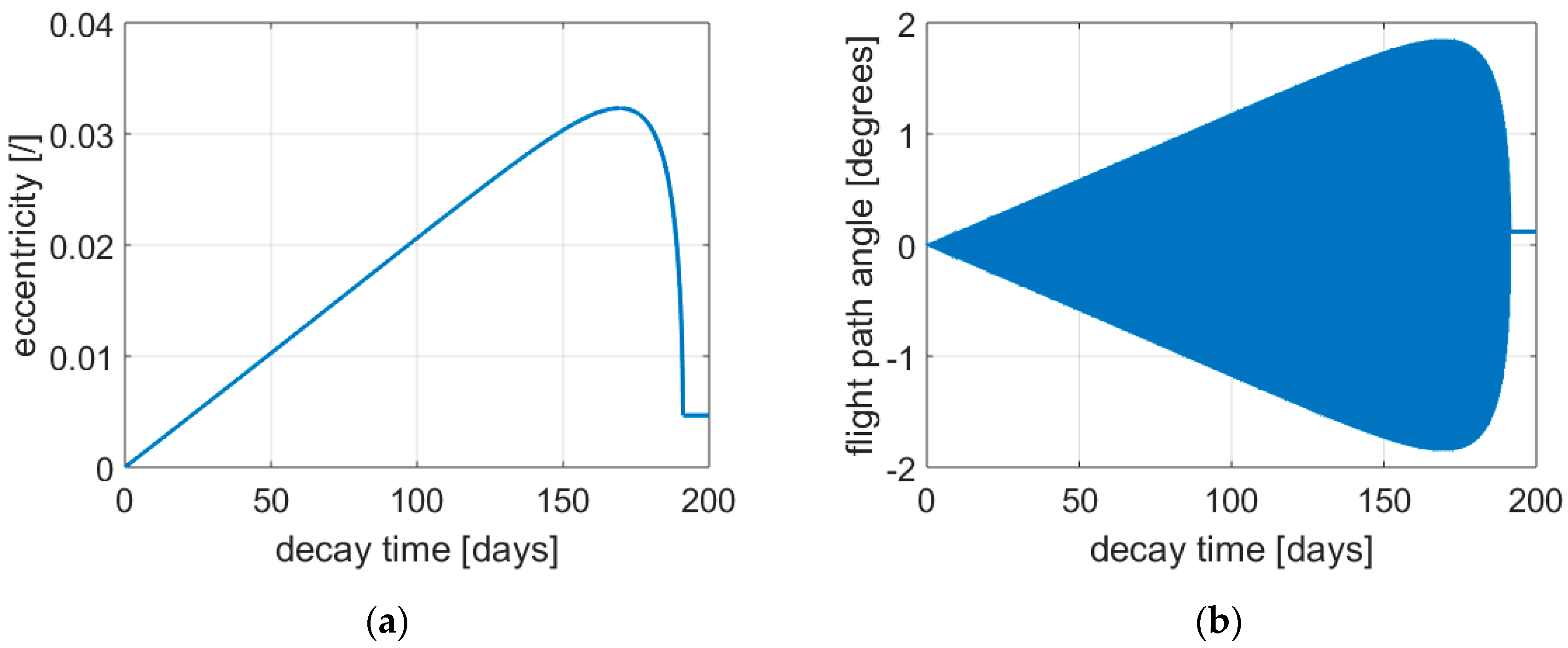
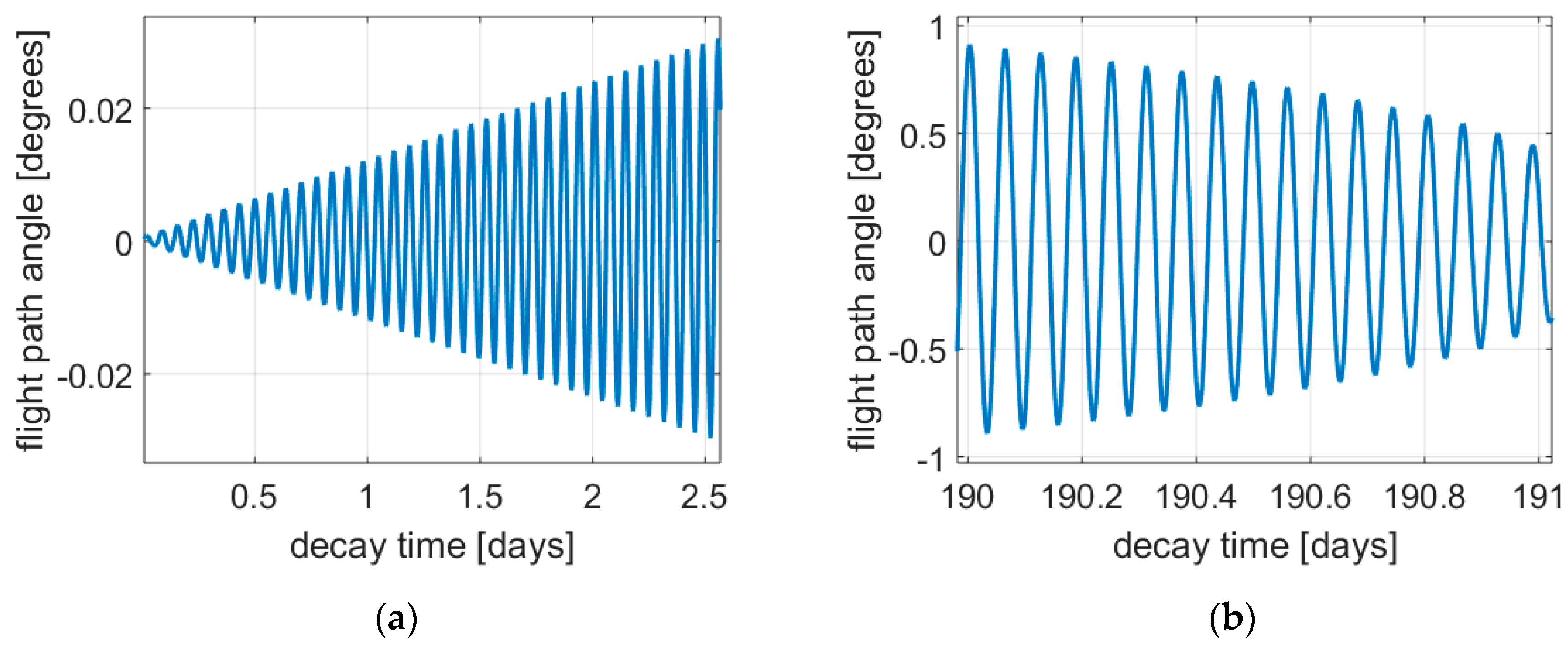
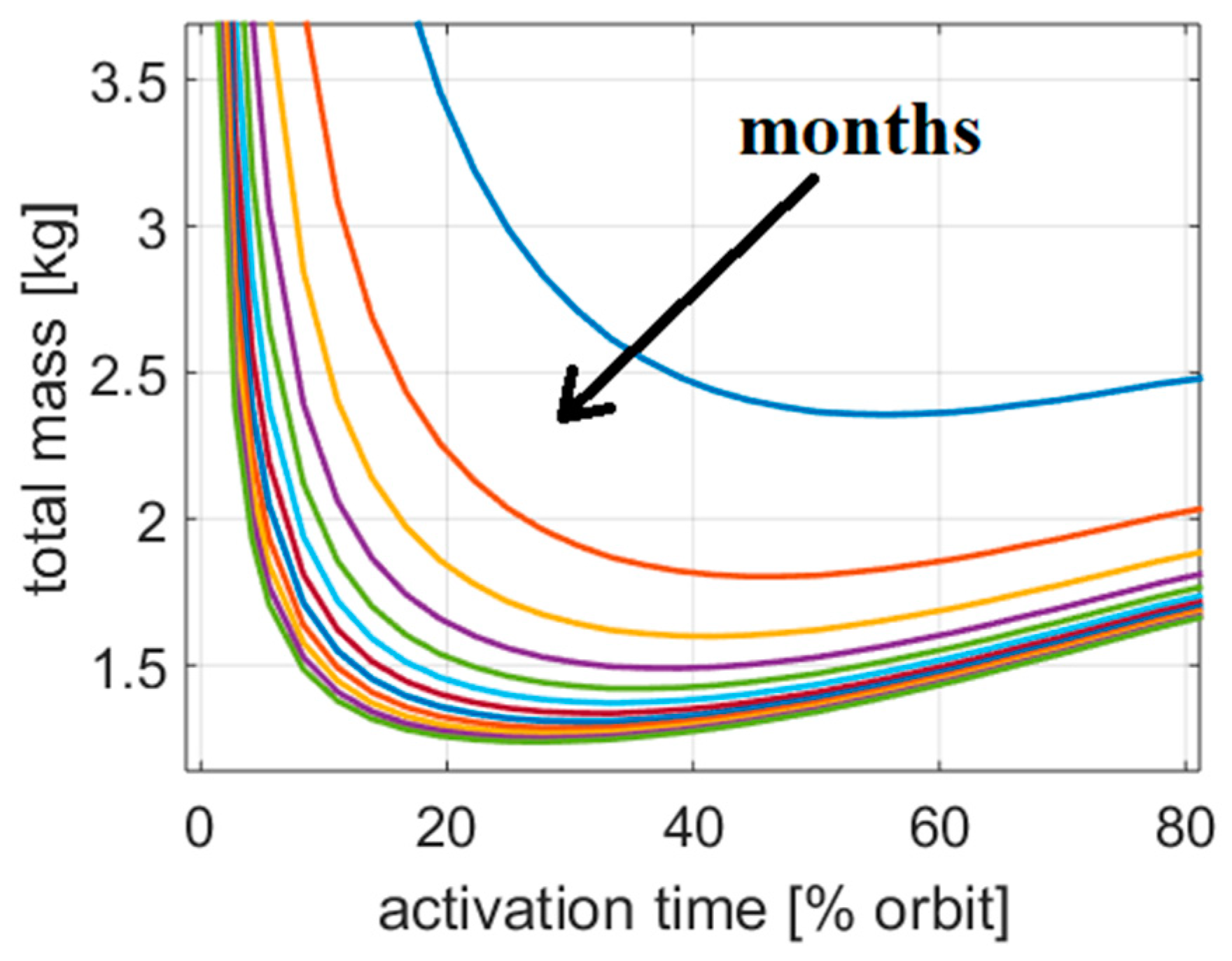
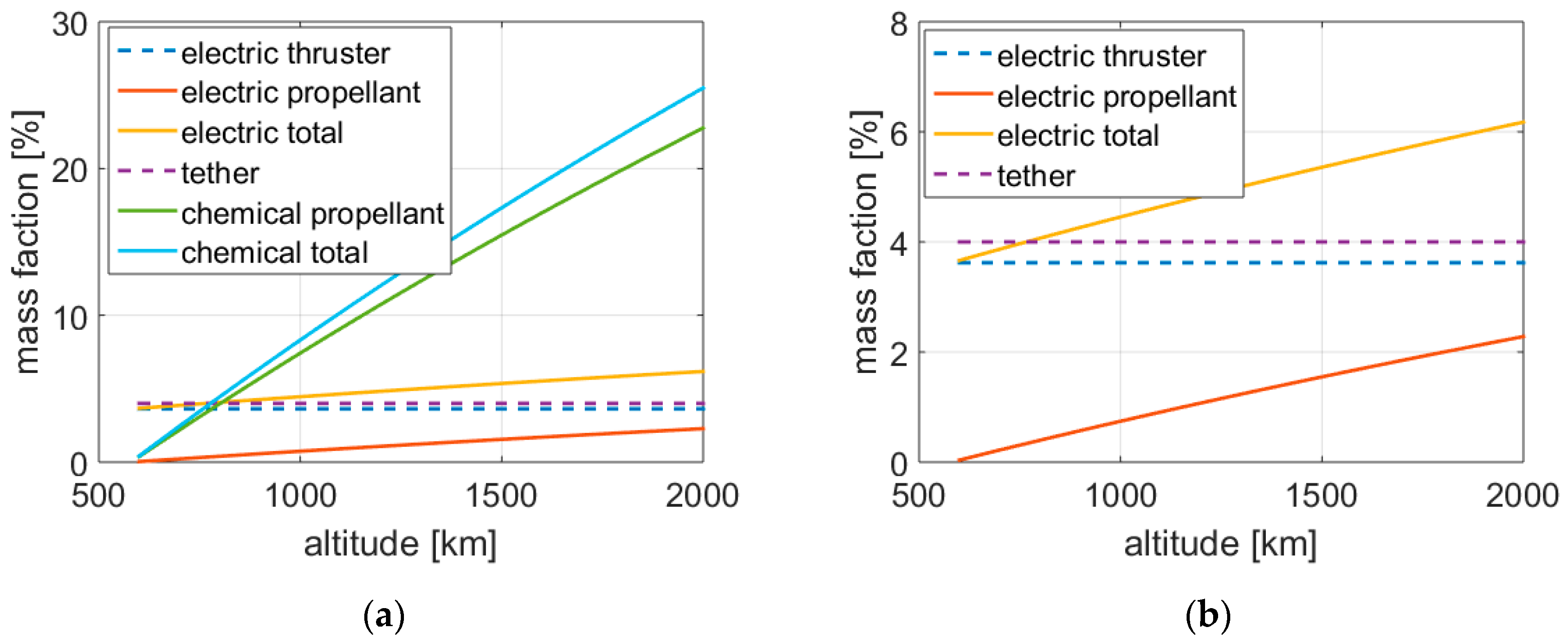
4.4. Electric Propulsion
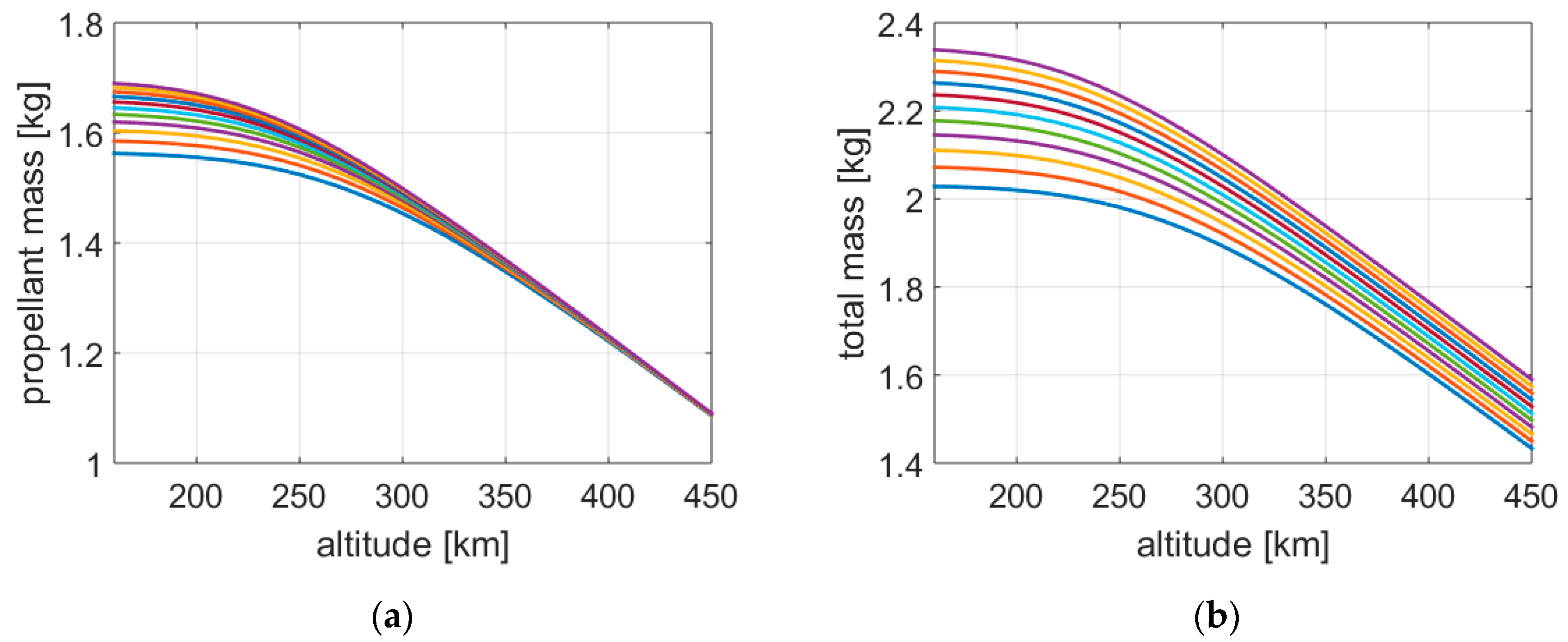
4.5. Electrodynamic Tether
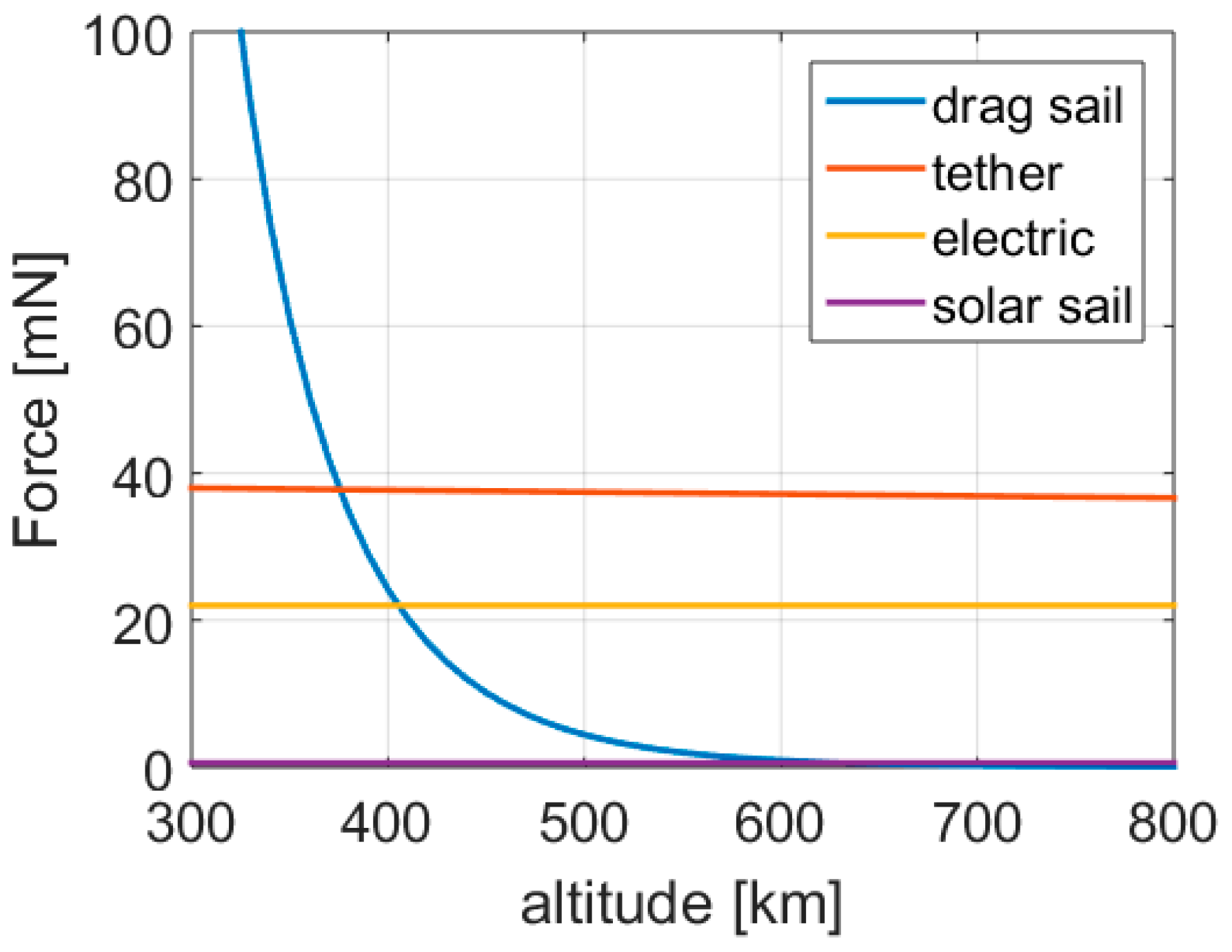
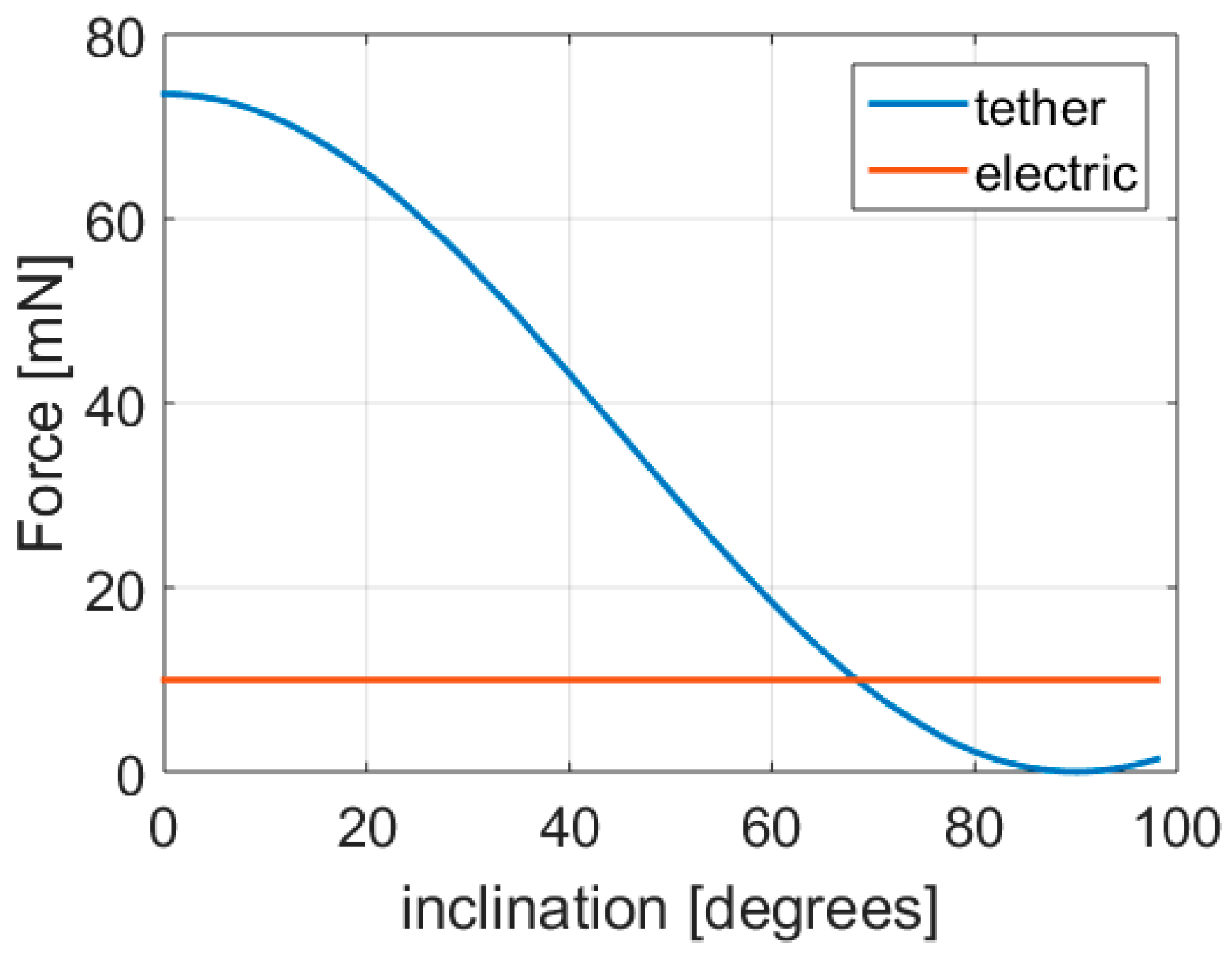
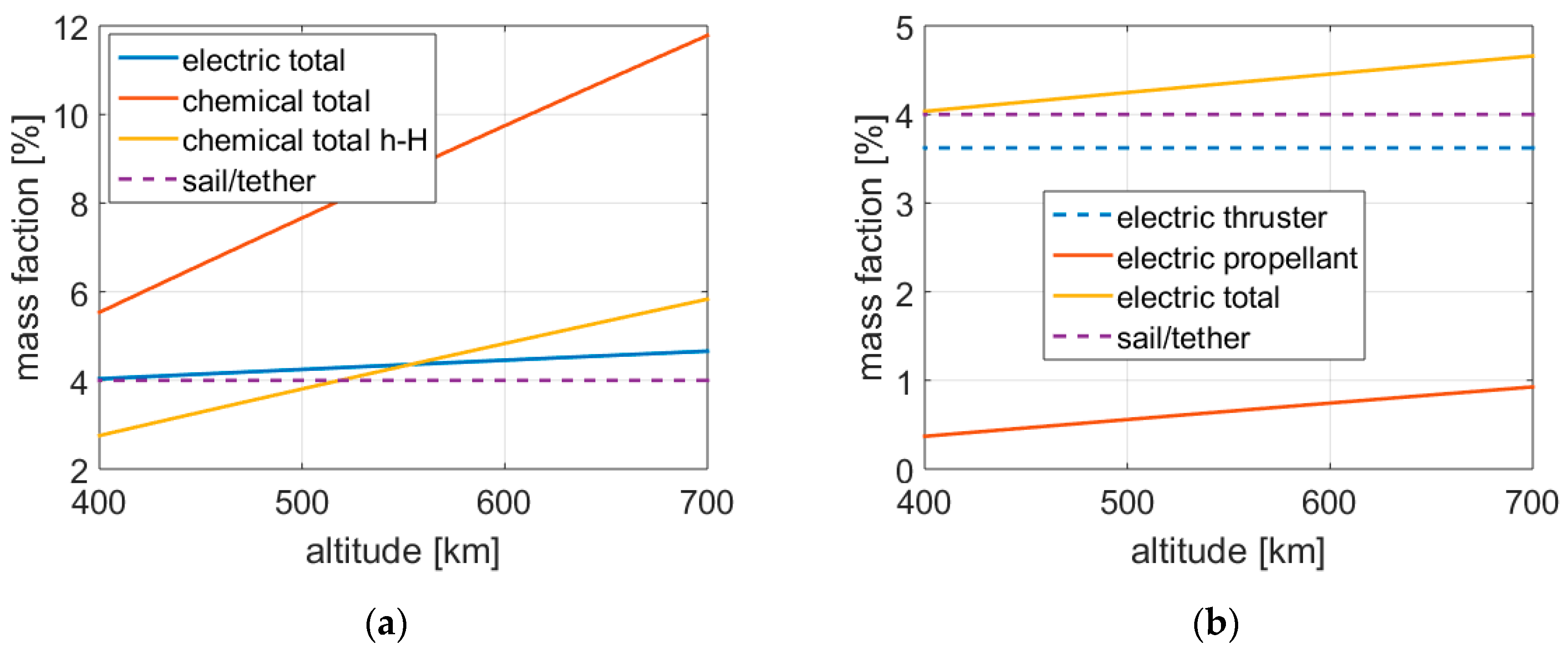
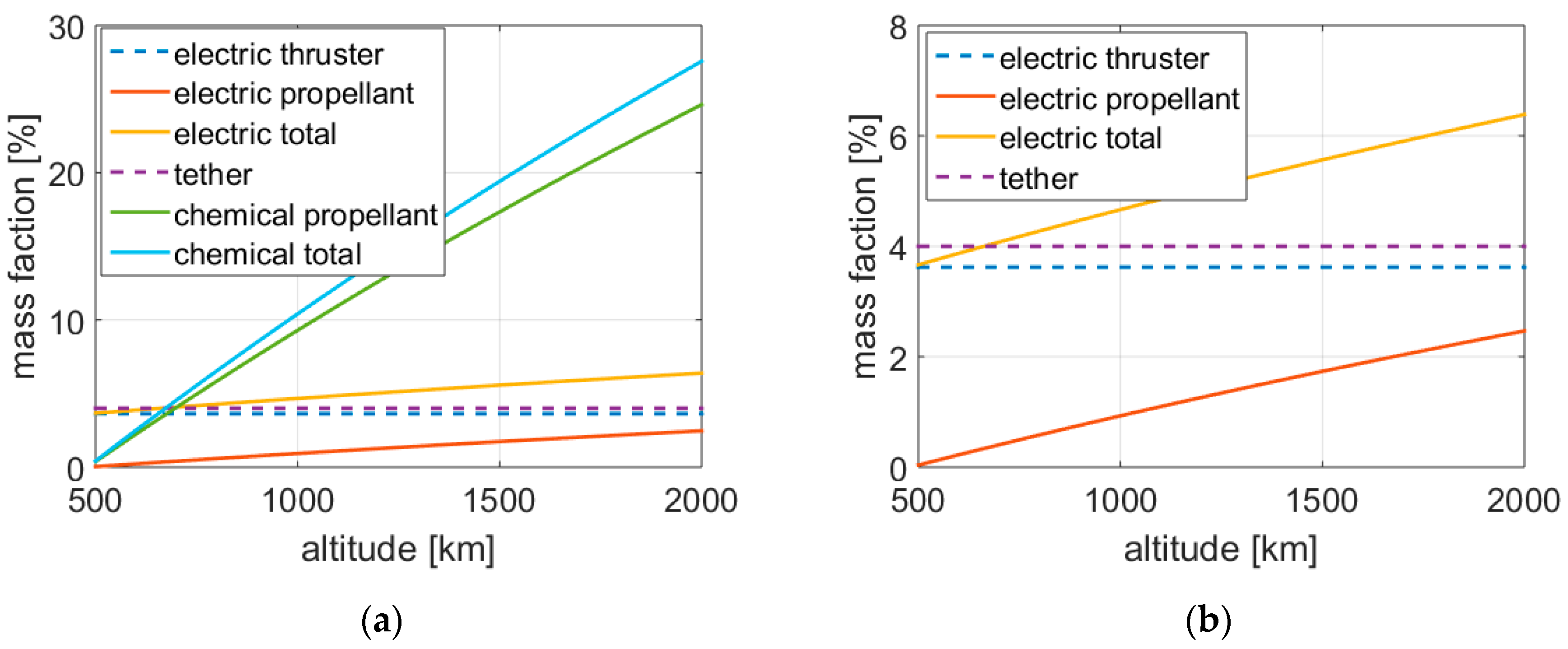
4.6. Comparison
4.7. Combinations
- The tether in drag mode is able to generate power [40] that can be potentially used by the electric thruster;
- The two systems can potentially be integrated, sharing some components like the cathode and the power management system, thus providing a synergistic effect;
- A combined off-the-shelves system could be used at any orbital inclination with near-optimal performance. This is particularly interesting for constellations of satellites deployed in different orbital inclinations, where choosing a single combined commercial unit (instead of different ones, tether vs. electric, optimized for different inclinations) is advisable for mass production and integration.
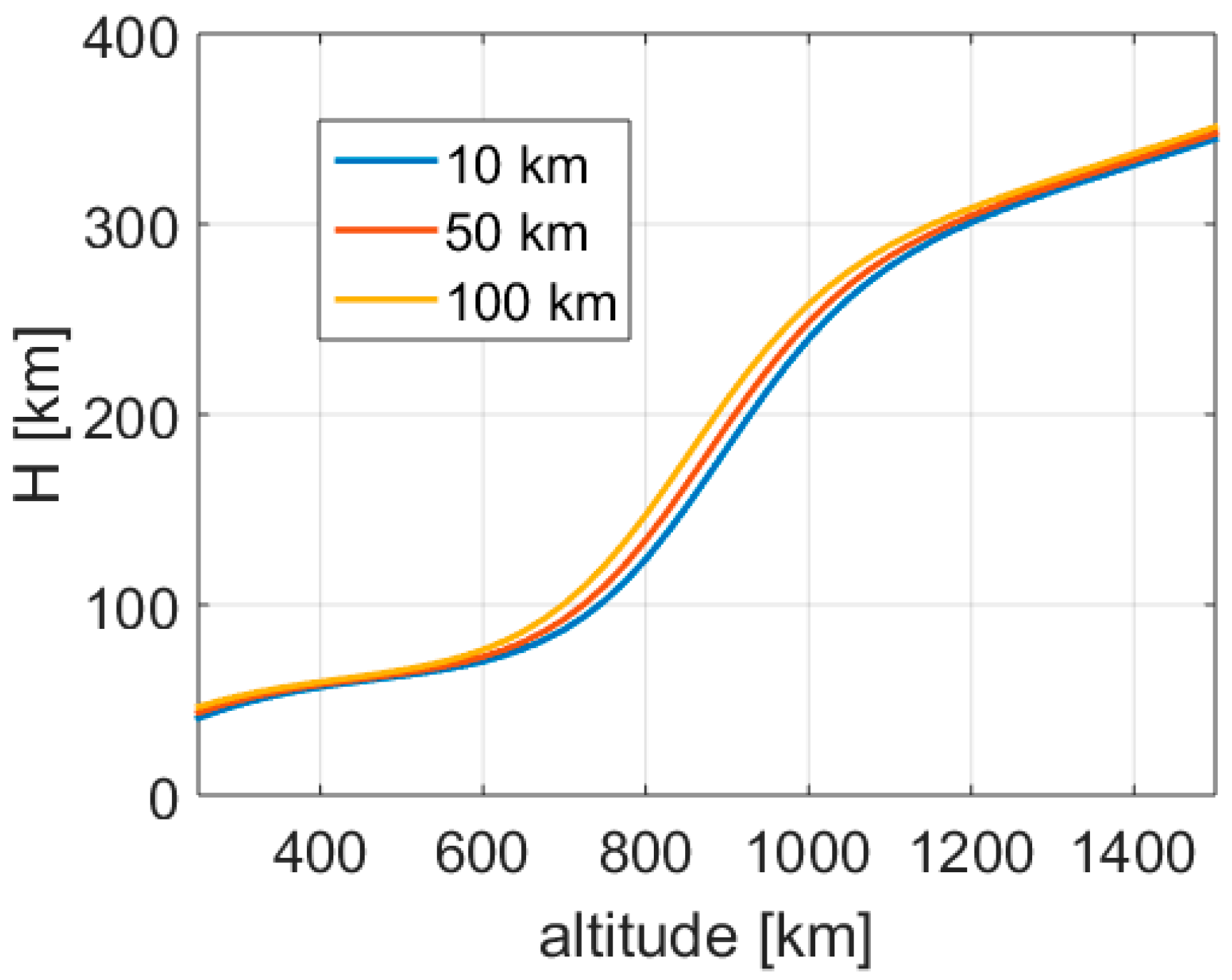
4.8. Drag Compensation
5. Other Topics
5.1. Integrated vs. Independent System
5.2. Active Debris Removal and Life Extension
5.3. Type of Re-Entry
6. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Space.com. Available online: https://www.space.com/spacex-starlink-satellite-collision-alerts-on-the-rise (accessed on 19 August 2022).
- Parabolic Arc. Available online: http://parabolicarc.com/2022/07/17/swarm-dodges-collision-during-climb-to-escape-suns-wrath/#more-87593 (accessed on 19 August 2022).
- Ars Technica. Available online: https://arstechnica.com/information-technology/2017/10/spacex-and-oneweb-broadband-satellites-raise-fears-about-space-debris/ (accessed on 19 August 2022).
- Parabolic Arc. Available online: http://parabolicarc.com/2022/03/26/space-situational-assessment-2021-the-growing-menace-of-space-debris/ (accessed on 19 August 2022).
- Kessler, D.J.; Cour-Palais, B.G. Collision frequency of artificial satellites: The creation of a debris belt. J. Geophys. Res. Space Phys. 1978, 83, 2637–2646. [Google Scholar] [CrossRef]
- Karacalioglu, A.G.; Stupl, J. The Impact of New Trends in Satellite Launches on the Orbital Debris Environment. In Proceedings of the NASA: 8th IAASS Conference, Safety first, Safety for All, Melbourne, FL, USA, 18–20 May 2016. [Google Scholar]
- ESA Space Debris Office. ESA’s Annual Space Environment Report; GEN-DB-LOG-00288-OPS-SD; ESA: Darmstadt, Germany, 2020. [Google Scholar]
- Wittig, M. Space Debris and De-Orbiting. MEW Aerospace. In TU Symposium and Workshop on Small Satellite Regulation and Communication Systems; Czech Republic: Prague, Czech, 2–4 March 2015. [Google Scholar]
- SpaceX. Available online: https://www.spacex.com/rideshare/ (accessed on 19 August 2022).
- ESA. Available online: https://www.esa.int/Enabling_Support/Operations/Space_debris_mitigation_the_case_for_a_code_of_conduct (accessed on 19 August 2022).
- Hull, S.M. NASA Disposal Guidelines. 2013. Available online: https://ntrs.nasa.gov/citations/20130000278 (accessed on 19 August 2022).
- IADC Steering Group and Working Group 4. IADC Space Debris Mitigation Guidelines. 2020. Available online: https://www.google.com/url?sa=t&rct=j&q=&esrc=s&source=web&cd=&ved=2ahUKEwjzwbyA7df6AhUL3KQKHQgHDDsQFnoECAwQAQ&url=https%3A%2F%2Fwww.iadc-home.org%2Fdocuments_public%2Ffile_down%2Fid%2F5249&usg=AOvVaw3Y9m5766xNnRyXuPdNPtW5 (accessed on 19 August 2022).
- Parabolic Arc. Available online: http://parabolicarc.com/2022/02/24/spacexs-approach-to-space-sustainability-and-safety/ (accessed on 19 August 2022).
- Parabolic Arc. Available online: http://www.parabolicarc.com/2022/07/20/neuraspace-creates-smart-traffic-management-solution-for-satellite-constellations/ (accessed on 19 August 2022).
- Janovsky, R. End-Of-Life De-Orbiting Strategies for Satellites. In Proceedings of the 54th International Astronautical Congress of the International Astronautical Federation, the International Academy of Astronautics, and the International Institute of Space Law, Bremen, Germany, 29 September–3 October 2003. [Google Scholar] [CrossRef]
- Cornara, S.; Beech, T.; Belló-Mora, M.; Martinez de Aragon, A. Satellite Constellation Launch, Deployment, Replacement, and End-Of-Life Strategies. SSC99-X-1. In Proceedings of the 13th Annual AIAA/USU Conference on Small Satellites, Logan, Utah, 23–26 August 1999. [Google Scholar]
- Sanchez-Arriaga, G.; Sanmartin, J.R.; Lorenzini, E.C. Comparison of technologies for deorbiting spacecraft from low-earth-orbit at end of mission. Acta Astronaut. 2017, 138, 536–542. [Google Scholar] [CrossRef] [Green Version]
- Steyn, W.H. De-Orbiting Strategies; Stellenbosch University: Stellenbosch, South Africa.
- Mostafa, A.; El-Saftawy, M.I.; Abouelmagd, E.I.; López, M.A. Controlling the perturbations of solar radiation pressure on the Lorentz spacecraft. Symmetry 2020, 12, 1423. [Google Scholar] [CrossRef]
- Abouelmagd, E.I.; Mortari, D.; Selim, H.H. Analytical study of periodic solutions on perturbed equatorial two-body problem. Int. J. Bifurc. Chaos 2015, 25, 1540040. [Google Scholar] [CrossRef]
- Abouelmagd, E.I. Periodic solution of the two–body problem by KB averaging method within frame of the modified Newtonian potential. J. Astronaut. Sci. 2018, 65, 291–306. [Google Scholar] [CrossRef]
- U.S. Standard Atmosphere; U.S. Government Printing Office: Washington, DC, USA, 1976.
- Picone, J.M.; Hedin, A.E.; Drob, D.P.; Aikin, A.C. NRLMSISE-00 empirical model of the atmosphere: Statistical comparisons and scientific issues. J. Geophys. Res. Space Phys. 2002, 107, 1468. [Google Scholar] [CrossRef]
- Parabolic Arc. Available online: http://parabolicarc.com/2022/06/14/space-flight-laboratory-announces-successful-deorbiting-of-nanosatellite-with-drag-sail-technology/ (accessed on 19 August 2022).
- Black, A.; Spencer, D.A. DragSail systems for satellite deorbit and targeted reentry. J. Space Saf. Eng. 2020, 7, 397–403. [Google Scholar] [CrossRef]
- Long, A.C.; Spencer, D.A. A Scalable Drag Sail for the Deorbit of Small Satellites. J. Small Satell. 2018, 7, 773–788. [Google Scholar]
- Taylor, B.; Fellowes, S.; Dyer, B.; Viquerat, A.; Aglietti, G. A modular drag-deorbiting sail for large satellites in low Earth orbit. In Proceedings of the SciTech 2020, Orlando, FL, USA, 6–10 January 2020. [Google Scholar]
- Sikes, J.D.; Ledbetter, W.; Sood, R.; Medina, K.; Turse, D. Keeping Low Earth Orbit Clean: Deorbit Analysis for an Articulating Boom Drag Sail. Adv. Astronaut. Sci. 2020, 175, 1–15. [Google Scholar]
- Faber, D.; Overlack, A.; Welland, W.; van Vliet, L.; Wieling, W.; Tata Nardini, F. Nanosatellite deorbit motor. SSC13-I-9. In Proceedings of the 27th annual AIAA/USU Conference on Small Satellite, Logan, Utah, 12–15 August 2013. [Google Scholar]
- Schonenborg, R.A.C.; Schöyer, H.F.R. Solid Propulsion De-Orbiting and Re-Orbiting. In Proceedings of the 5th European Conference on Space Debris, Darmstadt, Germany, 30 March–2 April 2009. [Google Scholar]
- Schonenborg, R.A.C. Solid propellant de-orbiting for constellation satellites. In Proceedings of the 4th International Spacecraft Propulsion Conference, Chia Laguna, Italy, 2–9 June 2004. [Google Scholar]
- Barato, F. Challenges of Ablatively Cooled Hybrid Rockets for Satellites or Upper Stages. Aerospace 2021, 8, 190. [Google Scholar] [CrossRef]
- Barato, F.; Paccagnella, E.; Pavarin, D. Explicit Analytical Equations for Single Port Hybrid Rocket Combustion Chamber Sizing. J. Propuls. Power 2020, 36, 869–886. [Google Scholar] [CrossRef]
- Barato, F.; Bellomo, N.; Pavarin, D. Integrated approach for hybrid rocket technology development. Acta Astronaut. 2016, 128, 257–261. [Google Scholar] [CrossRef]
- Barato, F.; Grosse, M.; Bettella, A. Hybrid Rocket Residuals—An Overlooked Topic. AIAA 2014-3753. In Proceedings of the 50th AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Cleveland, OH, USA, 28–30 July 2014. [Google Scholar] [CrossRef]
- Tadini, P. Hybrid Rocket Propulsion for Active Removal of Large Abandoned Objects. Ph.D. Thesis, Politecnico di Milano, Milan, Italy, 2014. [Google Scholar]
- Tonetti, S.; Cornara, S.; Faenza, M.; Verberne, O.; Langener, T. Feasibility Study of Active Debris Removal Using Hybrid Propulsion Solutions. In Proceedings of the Stardust Global Virtual Workshop II, Southampton, UK, 19–22 January 2016. [Google Scholar]
- MIT OpenCourseWare 16.522 Space Propulsion; Springer: Cambridge, MA, USA, 2015; Available online: http://ocw.mit.edu (accessed on 19 August 2022).
- Sarego, G.; Olivieri, L.; Valmorbida, A.; Bettanini, C.; Colombatti, G.; Pertile, M.; Lorenzini, E.C. Deorbiting Performance of Electrodynamic Tethers to Mitigate Space Debris. World Academy of Science, Engineering and Technology. Int. J. Aerosp. Mech. Eng. 2021, 15, 185–191. [Google Scholar]
- Bilen, S.; McTernan, J.; Gilchrist, B.; Bell, I.; Voronka, N.; Hoyt, R. Electrodynamic Tethers for Energy Harvesting and Propulsion on Space Platforms. AIAA 2010-8844. In Proceedings of the AIAA SPACE 2010 Conference & Exposition, Anaheim, CA, USA, 30 August–2 September 2010. [Google Scholar]
- Olivieri, L.; Mantellato, R.; Francesconi, A. A Tethered Space Tug Concept Demonstration for Active Debris Removal Missions. In Proceedings of the ESA GNC 2017, Salzburg, Austria, 29 May–June 2017. [Google Scholar]
- Thethers Unlimited CubeSat Terminator Tape Brochure. Available online: https://www.tethers.com/ (accessed on 19 August 2022).
- Thethers Unlimited NanoSat Terminator Tape Brochure. Available online: https://www.tethers.com/ (accessed on 19 August 2022).
- Aurora Propulsion Technologies APB-S Brochure. Available online: https://www.aurorapt.fi (accessed on 19 August 2022).
- Montenbruck, O.; Gill, E. Satellite Orbits: Models, Methods and Applications; Springer: Berlin, Heidelberg, 2001. [Google Scholar] [CrossRef]
- Chobotov, V.A. Orbital Mechanics, 3rd ed.; AIAA Education Series; American Institute of Aeronautics and Astronautics, Inc.: Reston, VA, USA, 2008. [Google Scholar]
- Vallado, D.A. Fundamental of Astrodynamics and Applications, 2nd ed.; Microcosm Press: El Segundo, CA, USA; Kluwer Academic Publishers: Boston, MA, USA, 2001. [Google Scholar]
- GOCE ESA’s Gravity Mission. Available online: https://www.esa.int/Applications/Observing_the_Earth/FutureEO/GOCE (accessed on 19 August 2022).
- Klinkrad, H.; Sanchez-Ortiz, N. Collision Avoidance for Operational ESA Satellites. ESA SP-587. In Proceedings of the 4th European Conference on Space Debris, Darmstadt, Germany, 18–20 April 2005. [Google Scholar]
- Barato, F.; Trezzolani, F.; Manente, M.; Pavarin, D.; Andreussi, T.; Andrenucci, M. Electric Propulsion Technology for Low Earth Mission of Micro/Nano-Satellites. In Proceedings of the International Astronautic Conference 2015, Jerusalem, Israel, 12–16 October 2015. [Google Scholar]
- Bertolucci, G.; Barato, F.; Toson, E.; Pavarin, D. Impact of propulsion system characteristics on the potential for cost reduction of earth observation missions at very low altitudes. Acta Astronaut. 2020, 176, 173–191. [Google Scholar] [CrossRef]
- Rossettini, L. Technical Day on “Deorbiting Strategies”. Available online: https://www.dorbit.space/ (accessed on 1 December 2015).
- Innocenti, L. Clean Space; ESA Clean Space Office: Darmstadt, Germany, 2019. [Google Scholar]
- Astromaterials Research & Exploration Science NASA Orbital Debris Program Office. Available online: https://orbitaldebris.jsc.nasa.gov/reentry/ (accessed on 19 August 2022).
- Eggen, N.; Tiago Soares, T.; Innocenti, L. Containment Methods for The Atmospheric Reentry of Satellites. In Proceedings of the First Int’l. Orbital Debris Conference, Sugar Land, TX, USA, 9–12 December 2019. [Google Scholar]
- Trisolini, M.; Lewis, H.G.; Colombo, C. On the Demisability and Survivability of Modern Spacecraft. In Proceedings of the 7th European Conference on Space Debris, Darmstadt, Germany, 18–21 April 2017; Flohrer, T., Schmitz, F., Eds.; The ESA Space Debris Office: Darmstadt, Germany, 2017. Available online: http://spacedebris2017.sdo.esoc.esa.int (accessed on 1 June 2017).
- Waswa, M.B.P. Spacecraft Design-for-Demise Strategy, Analysis and Impact on Low Earth Orbit Space Missions. Master’s Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 2009. [Google Scholar]




















Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Barato, F. Comparison between Different Re-Entry Technologies for Debris Mitigation in LEO. Appl. Sci. 2022, 12, 9961. https://doi.org/10.3390/app12199961
Barato F. Comparison between Different Re-Entry Technologies for Debris Mitigation in LEO. Applied Sciences. 2022; 12(19):9961. https://doi.org/10.3390/app12199961
Chicago/Turabian StyleBarato, Francesco. 2022. "Comparison between Different Re-Entry Technologies for Debris Mitigation in LEO" Applied Sciences 12, no. 19: 9961. https://doi.org/10.3390/app12199961
APA StyleBarato, F. (2022). Comparison between Different Re-Entry Technologies for Debris Mitigation in LEO. Applied Sciences, 12(19), 9961. https://doi.org/10.3390/app12199961





