An Anisotropic Damage Model of Quasi-Brittle Materials and Its Application to the Fracture Process Simulation
Abstract
:1. Introduction
2. Anisotropic Damage Model
2.1. Failure Criterion
2.2. Stiffness Degradation Model
2.3. Damage Evolution Equations
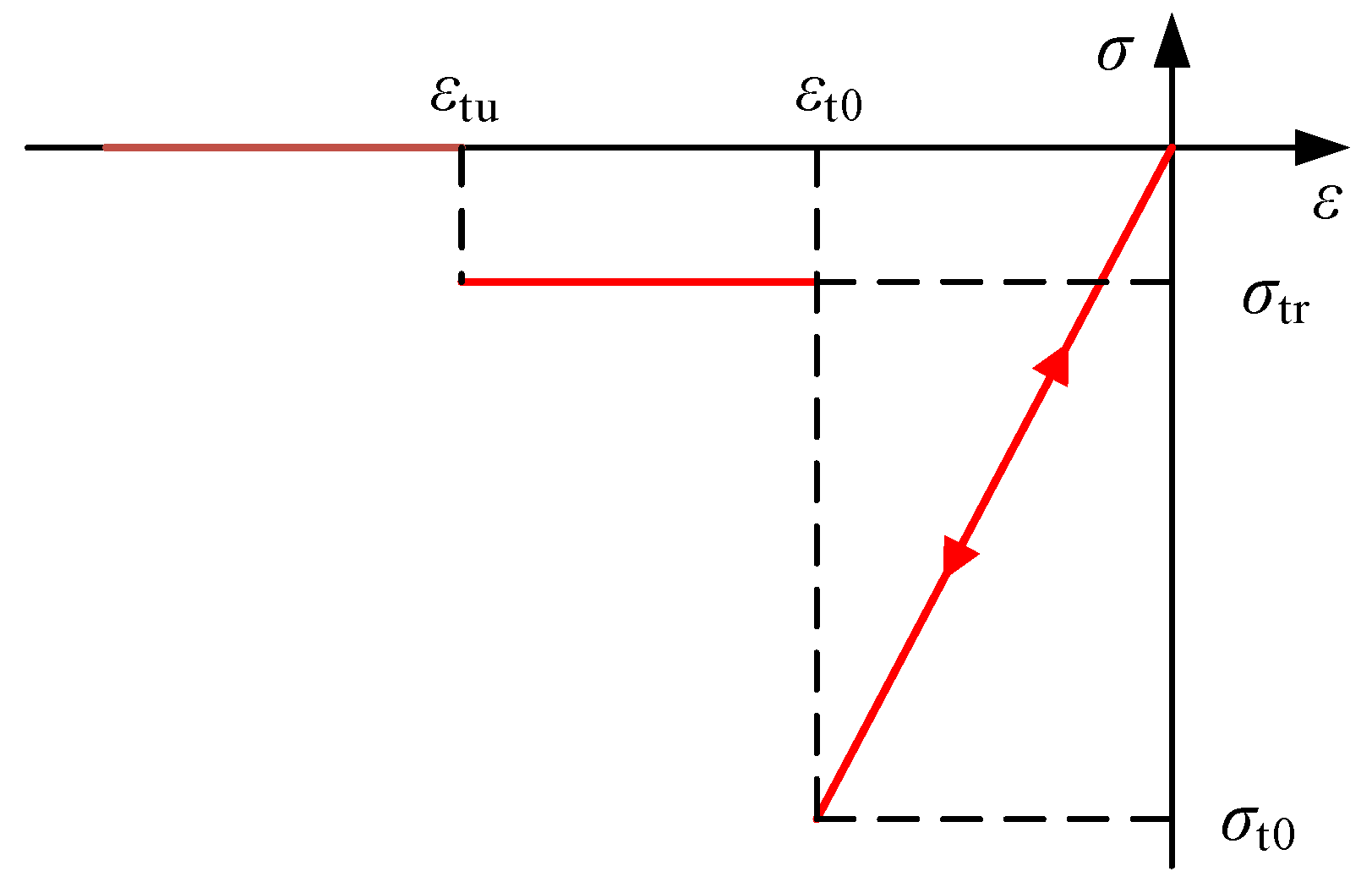
2.3.1. Tension Damage
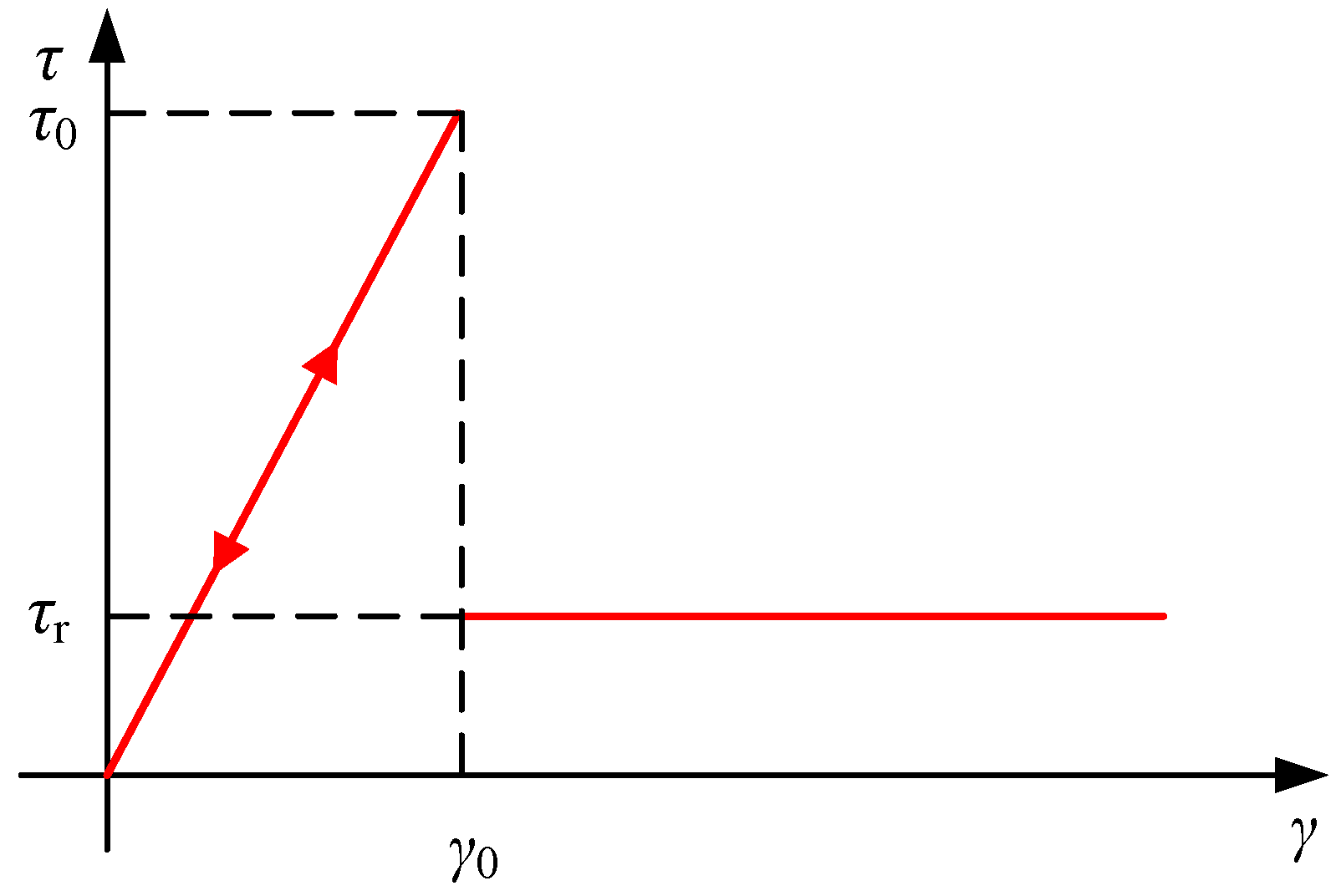
2.3.2. Shear Damage
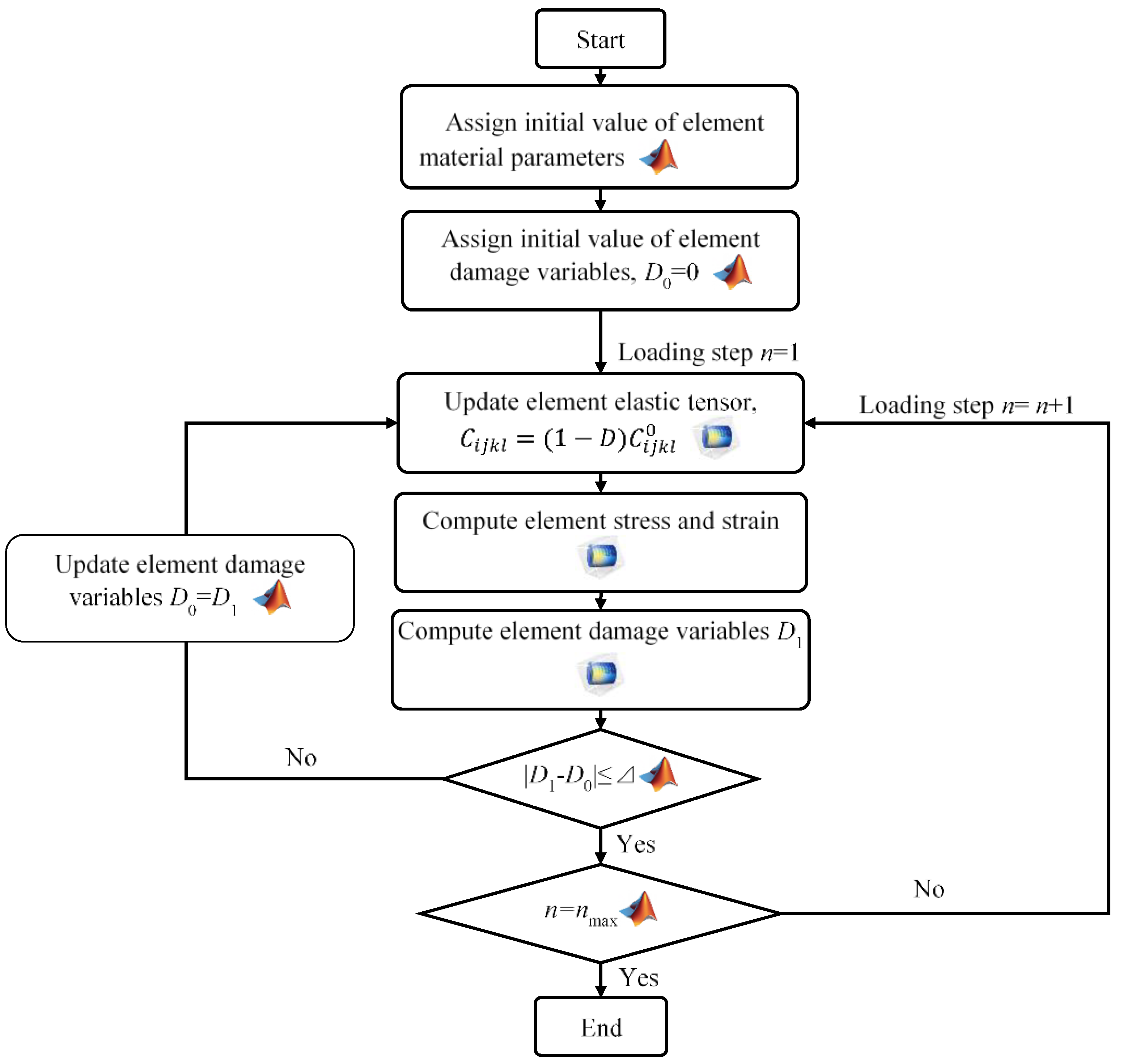
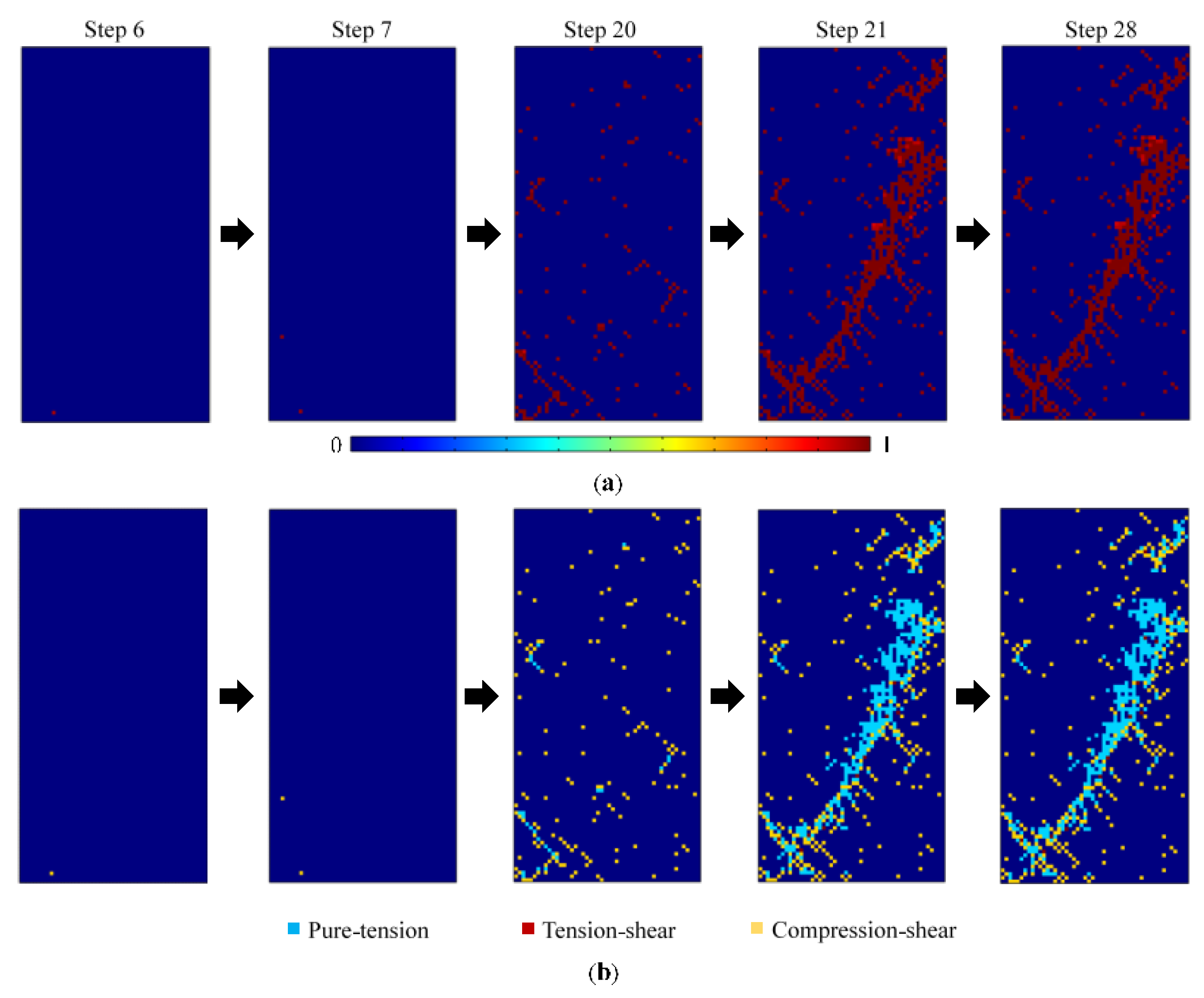
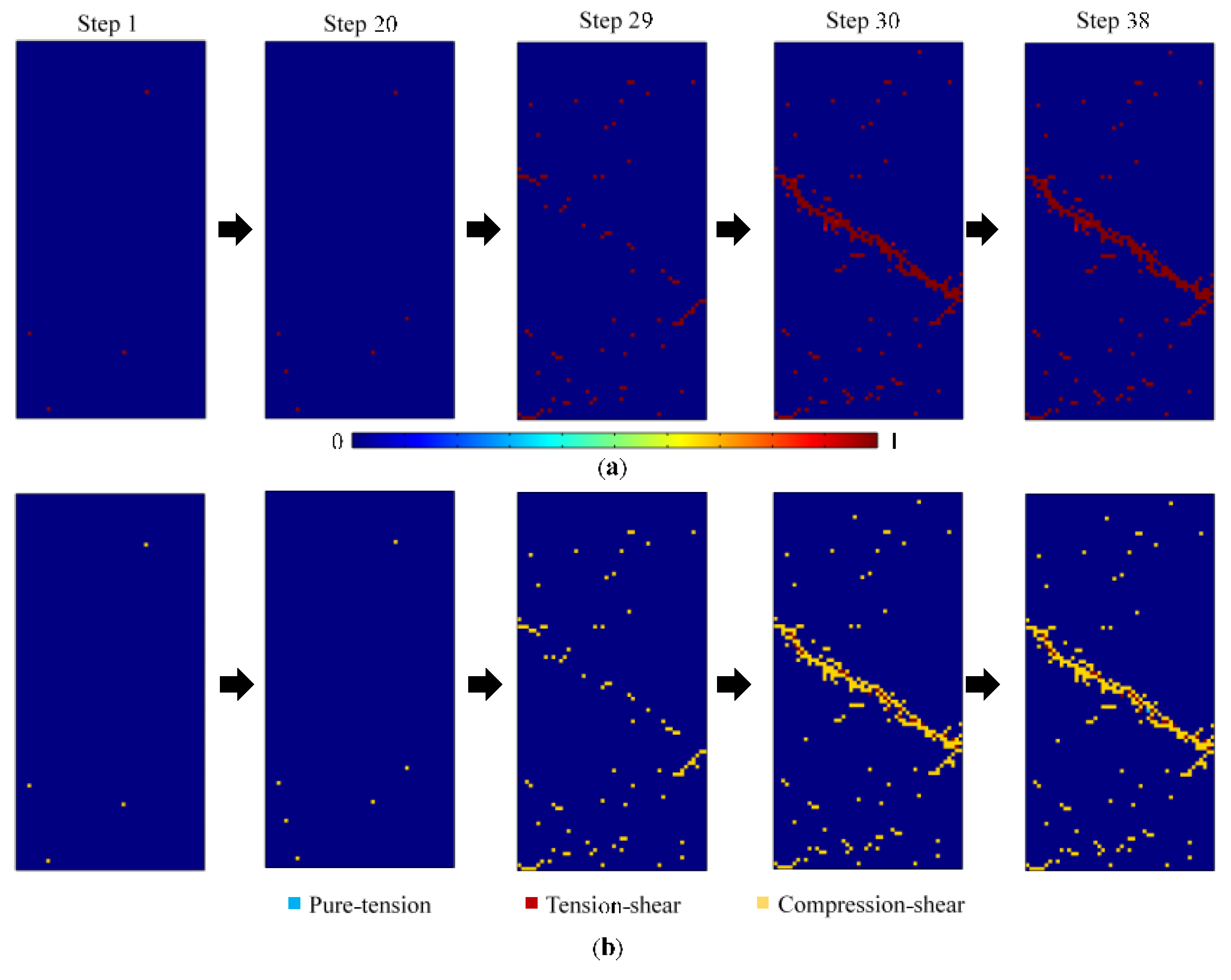
3. Simulation of the Fracture Process
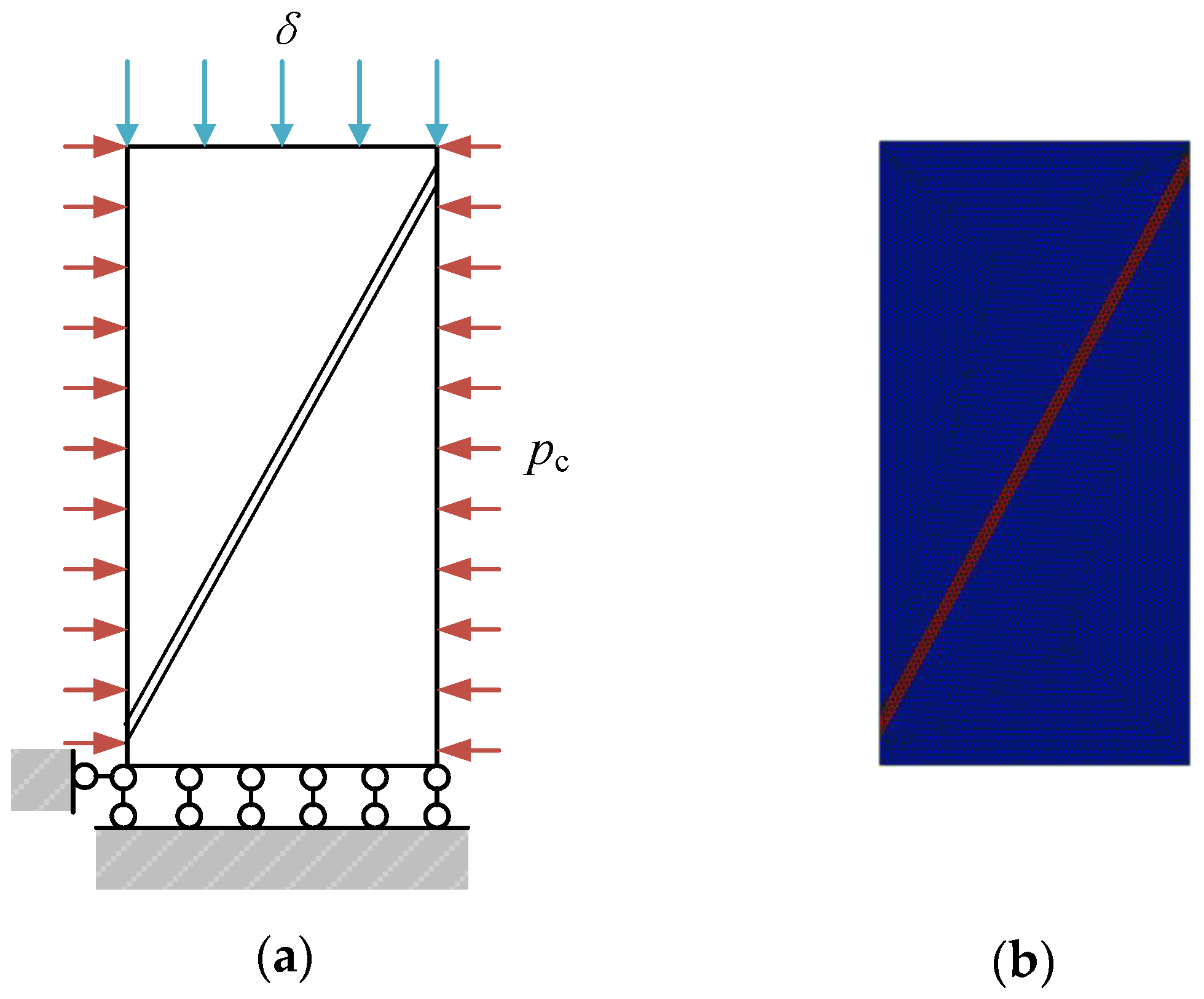
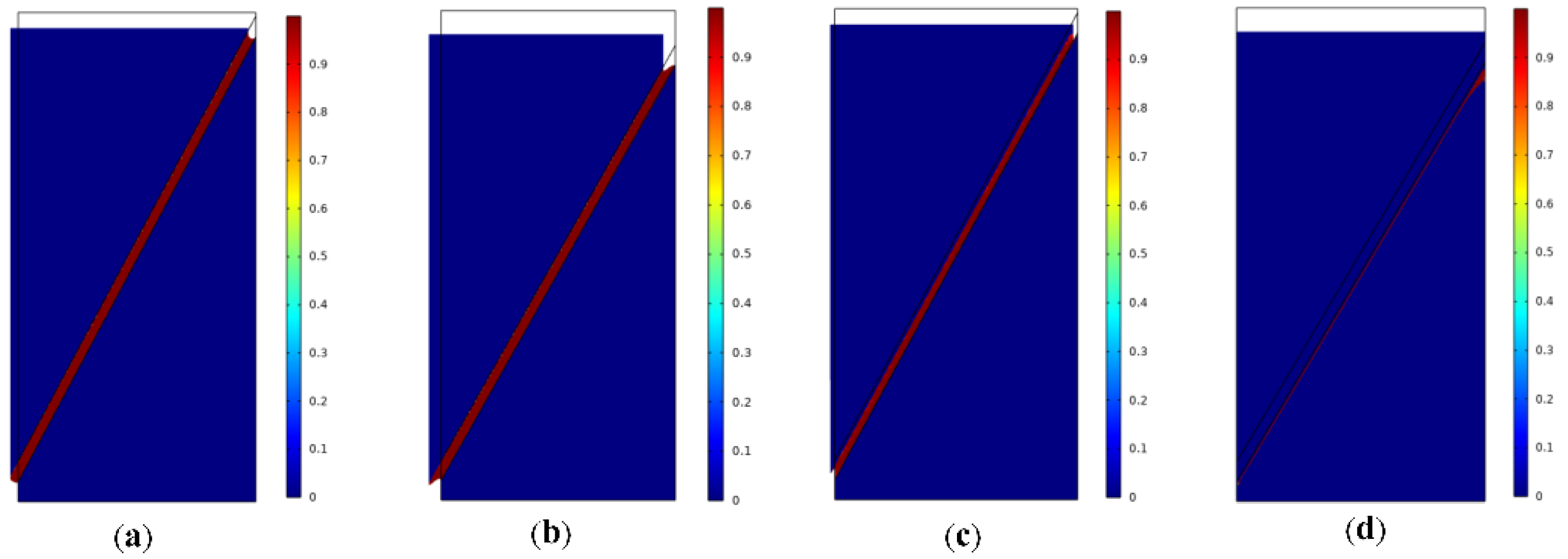
4. Test of the Anisotropic Damage Model
5. Uniaxial and Biaxial Compression Tests
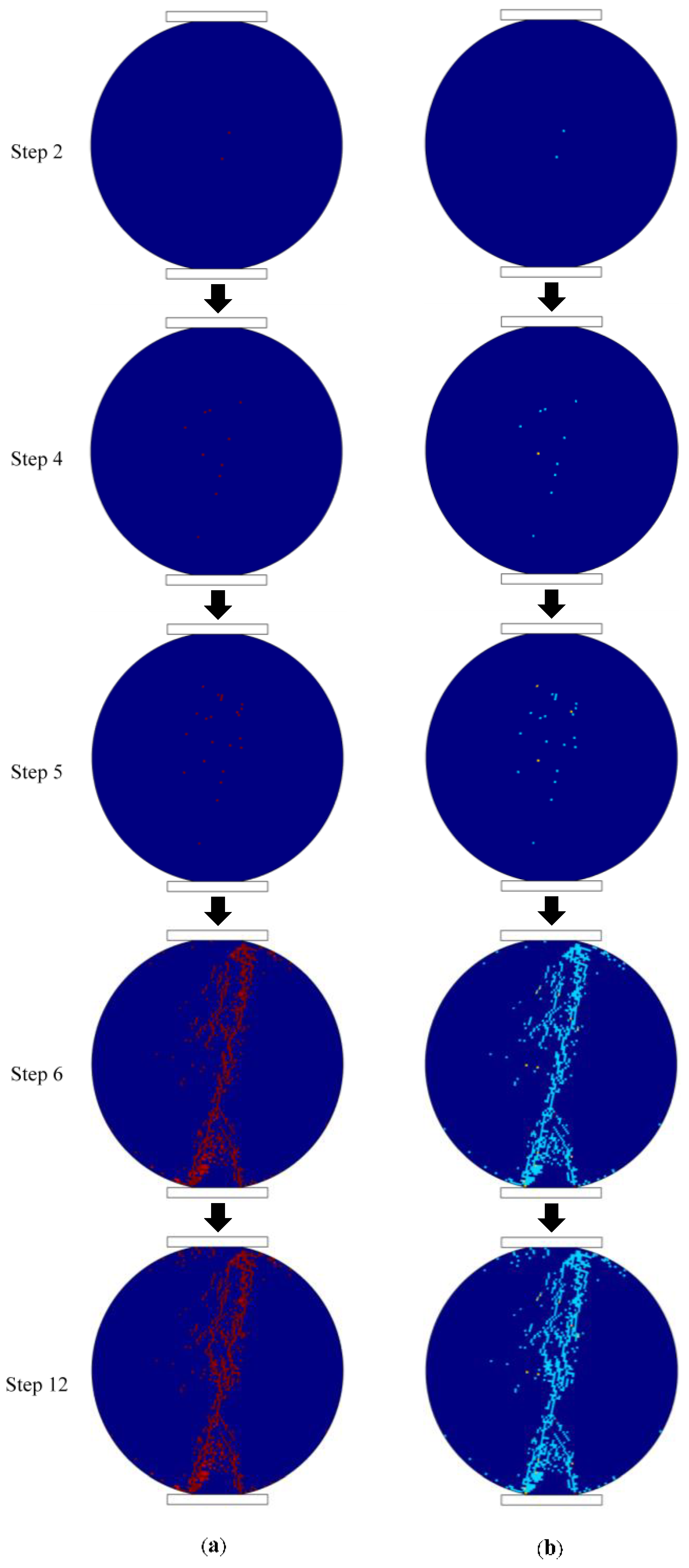
5.1. Damage Evolution
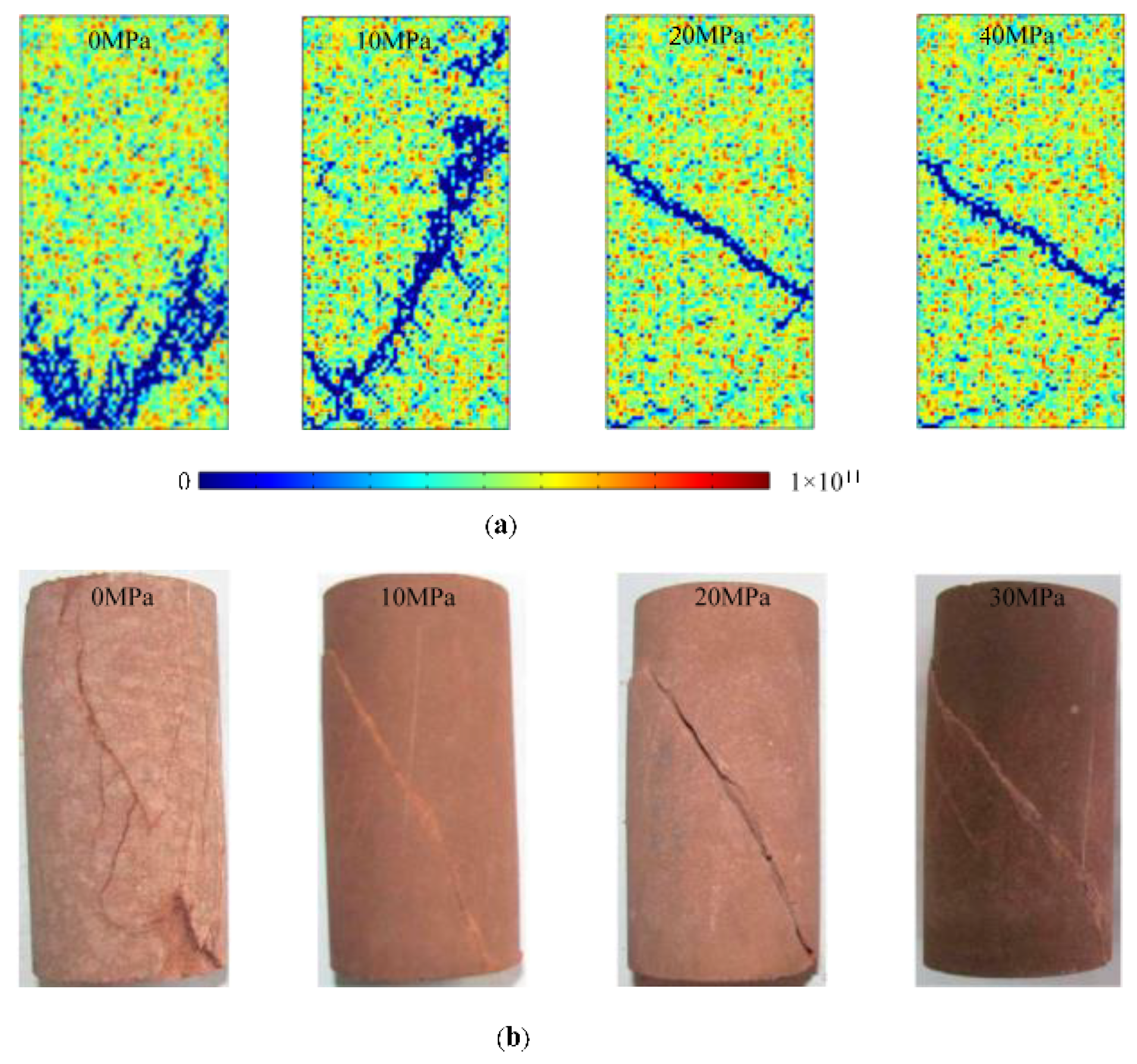
5.2. Fracture Modes
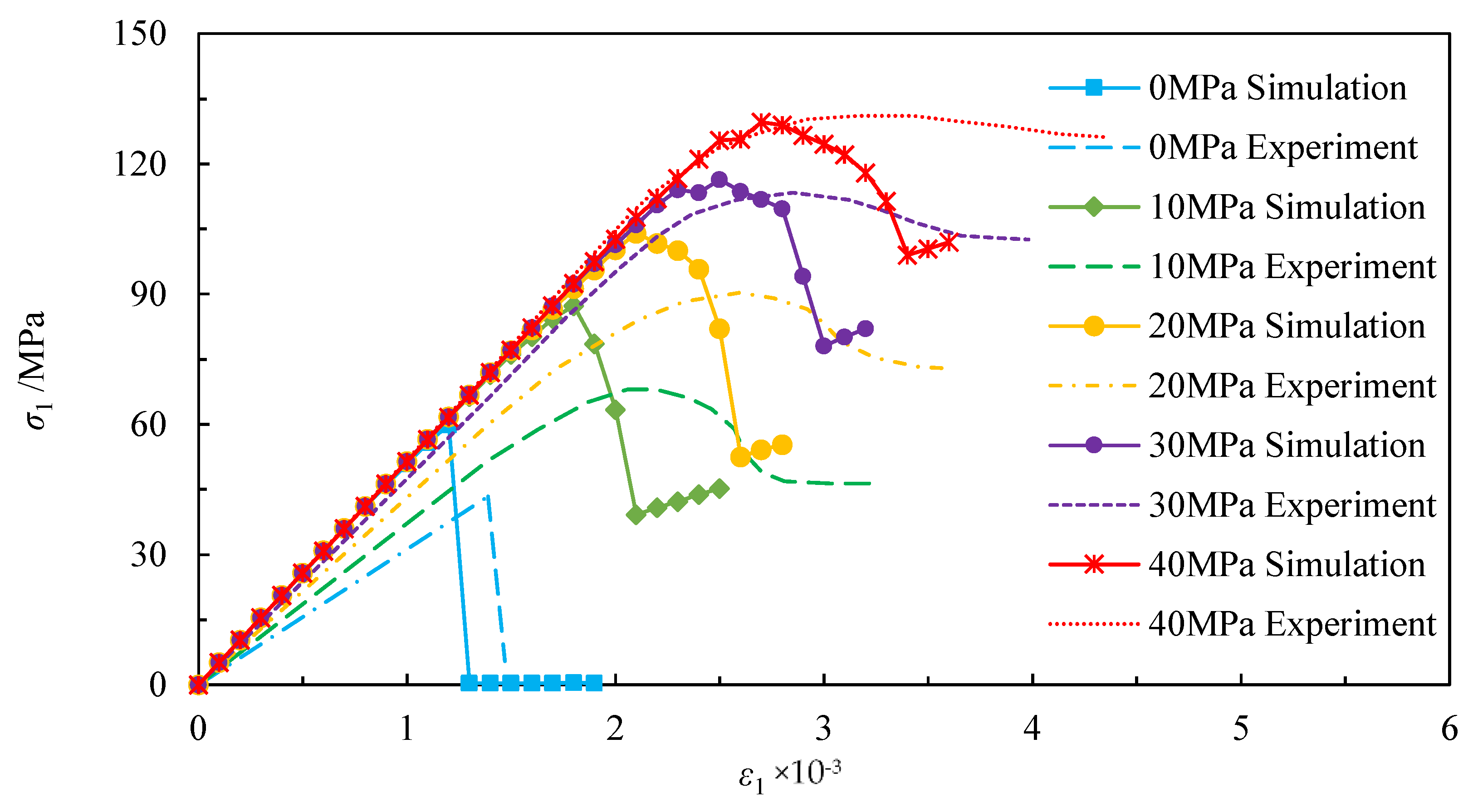
5.3. Stress–Strain Curves
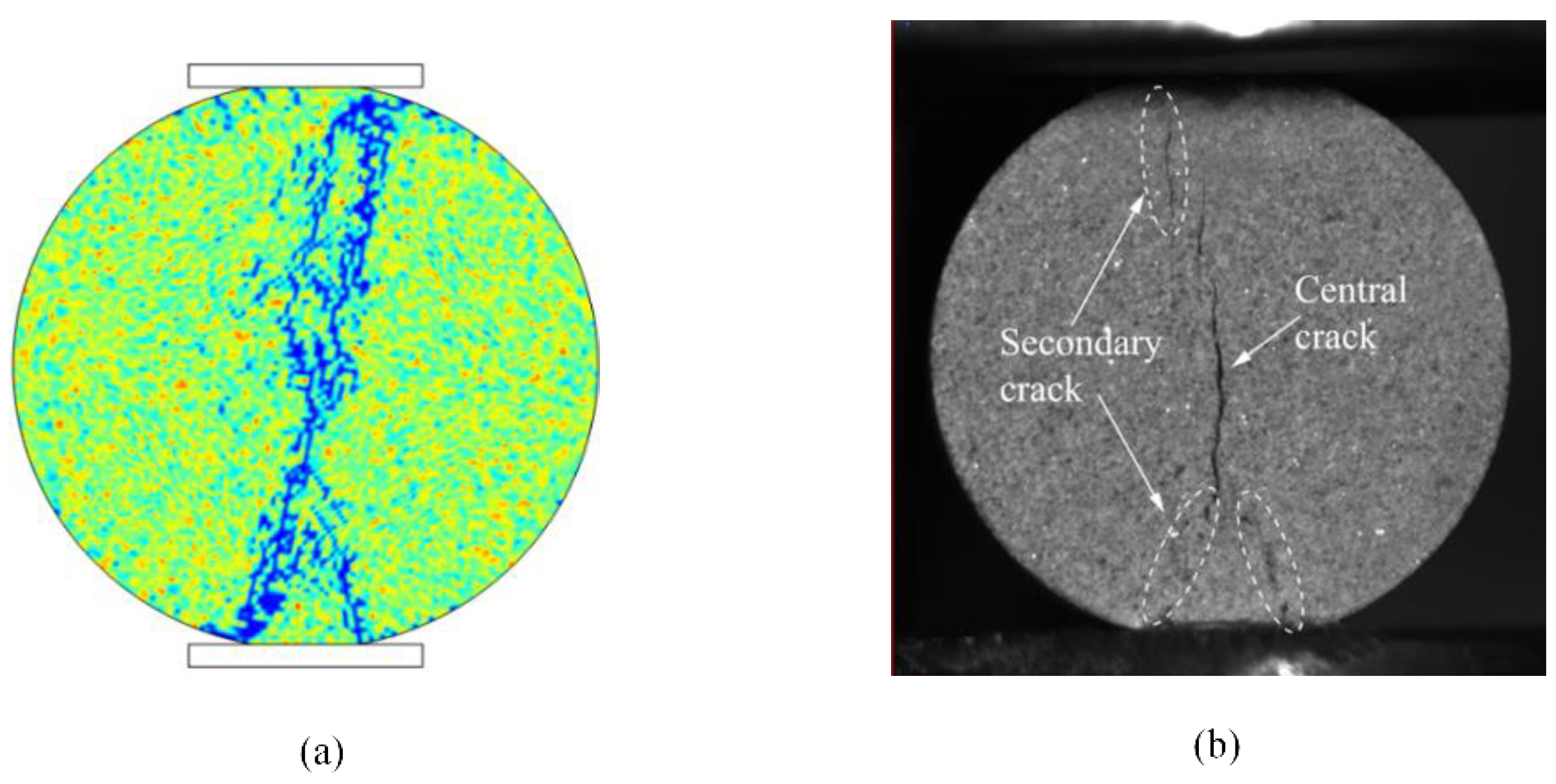
6. Brazilian Splitting Test
7. Conclusions
- A novel anisotropic damage model is proposed to improve the conventional isotropic damage model. It avoids the interpenetration of crack surfaces and unifies three forms of damage, which include pure tension, tension–shear, and compression–shear, as one smooth criterion. In addition, the shear damage evolution equation considers the equivalent shear strain on the failure face as the independent variable, which has a clearer physical meaning.
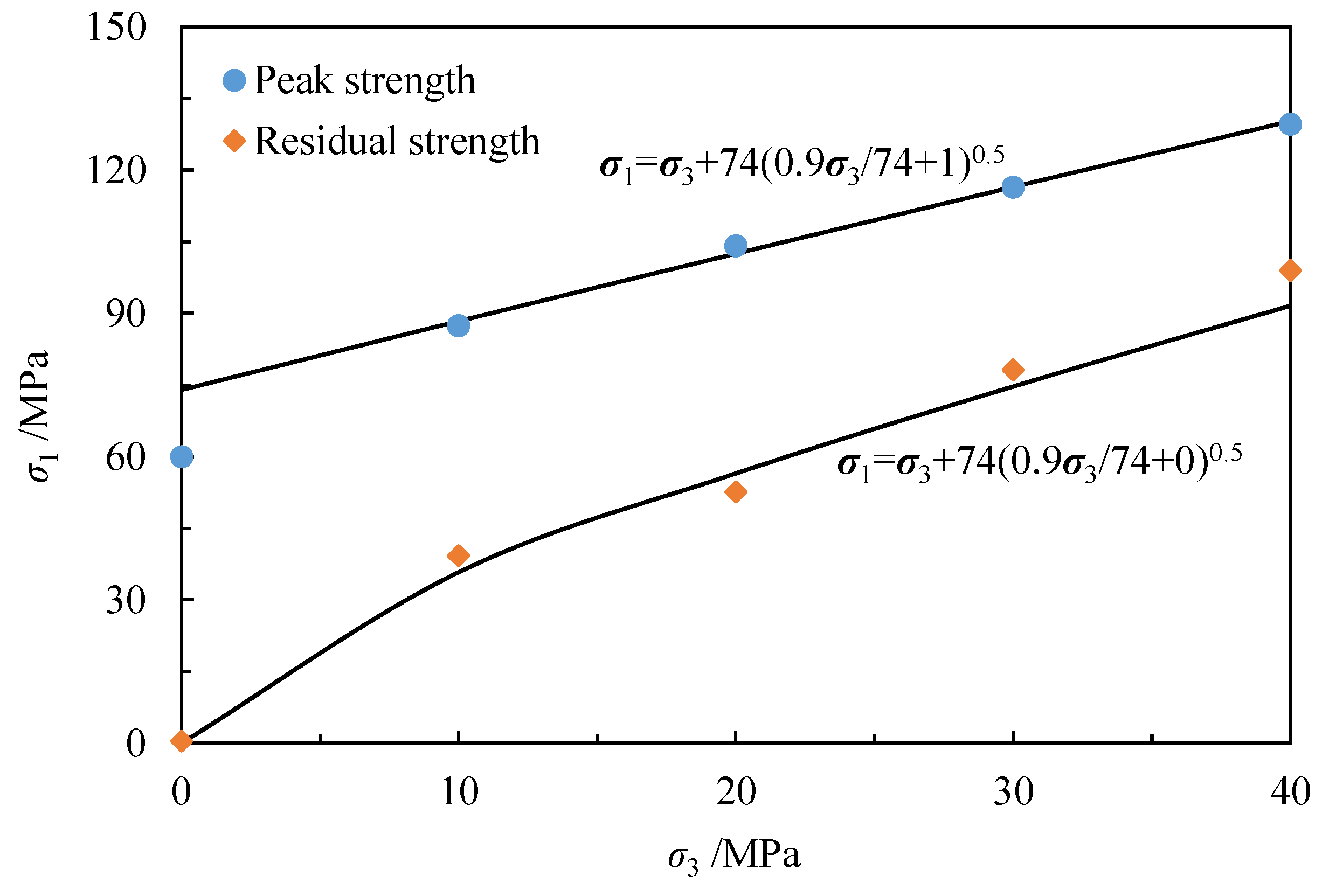
- The proposed anisotropic damage model is able to simulate the failure behavior of quasi-brittle materials more effectively. It can effectively simulate not only the mesoscale pure tension, tension shear, and compression shear damages but also the macroscale fracture mode and its evolution. It can also simulate the strength characteristics, including the peak and residual strengths, approximately.
- The simplified two-dimensional geometries were used to verify the proposed anisotropic damage model by comparison with a conventional damage model. However, the proposed anisotropic damage model and finite element implementation strategy proposed in this study also apply to three-dimensional, multi-scale, and multi-field coupling problems.
- Numerical tests of the failure behavior of quasi-brittle materials have the advantages of strong universality, convenience, flexibility, and repeatability compared with laboratory tests. In addition, they can provide mesoscale mechanical information that is difficult to observe in laboratory tests. However, owing to the limitations of the nonlinear solution method, numerical tests will take longer if there are more loading steps. The combination of numerical simulation and machine learning algorithms will help improve the efficiency of numerical tests better to serve the scientific research and teaching of quasi-brittle materials, which is also a follow-up research goal of the authors.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Khosravani, M.R.; Silani, M.; Weinberg, K. Fracture studies of ultra-high performance concrete using dynamic Brazilian tests. Theor. Appl. Fract. Mech. 2018, 93, 302–310. [Google Scholar] [CrossRef]
- Yu, J.; Shang, X. Analysis of the influence of boundary pressure and friction on determining fracture toughness of shale using cracked Brazilian disc test. Eng. Fract. Mech. 2019, 212, 57–69. [Google Scholar] [CrossRef]
- Hokka, M.; Black, J.; Tkalich, D.; Fourmeau, M.; Kane, A.; Hoang, N.-H.; Li, C.C.; Chen, W.W.; Kuokkala, V.-T. Effects of strain rate and confining pressure on the compressive behavior of Kuru granite. Int. J. Impact Eng. 2016, 91, 183–193. [Google Scholar] [CrossRef]
- Ge, X.R.; Ren, J.X.; Pu, Y.B.; Ma, W. Real-in time CT test of the rock meso-damage propagation law. Sci. China Ser. E 2001, 44, 328–336. [Google Scholar] [CrossRef]
- Li, Y.Y.; Cui, H.Q.; Zhang, P.; Wang, D.K.; Wei, J.P. Three-dimensional visualization and quantitative characterization of coal fracture dynamic evolution under uniaxial and triaxial compression based on μCT scanning. Fuel 2020, 262, 116568. [Google Scholar] [CrossRef]
- Liu, Z.L.; Ma, C.D.; Wei, X.A.; Xie, W.B. Experimental study on mechanical properties and failure modes of pre-existing cracks in sandstone during uniaxial tension/compression testing. Eng. Fract. Mech. 2021, 255, 107966. [Google Scholar] [CrossRef]
- Yang, S.Q.; Huang, Y.H.; Tang, J.Z. Mechanical, acoustic, and fracture behaviors of yellow sandstone specimens under triaxial monotonic and cyclic loading. Int. J. Rock Mech. Min. 2020, 130, 104268. [Google Scholar] [CrossRef]
- Zhu, Q.Q.; Li, X.B.; Li, D.Y.; Ma, C.D. Experimental investigations of static mechanical properties and failure characteristics of damaged diorite after dynamic triaxial compression. Int. J. Rock Mech. Min. 2022, 153, 105106. [Google Scholar] [CrossRef]
- Valliappan, S.; Murti, V.; Zhang, W.H. Finite element analysis of anisotropic damage mechanics problems. Eng. Fract. Mech. 1990, 35, 1061–1071. [Google Scholar] [CrossRef]
- Xu, Y.; Yao, W.; Xia, K.W. Numerical study on tensile failures of heterogeneous rocks. J. Rock Mech. Geotech. 2020, 12, 54–62. [Google Scholar] [CrossRef]
- Zhang, W.; Guo, T.K.; Qu, Z.Q.; Wang, Z.Y. Research of fracture initiation and propagation in HDR fracturing under thermal stress from meso-damage perspective. Energy 2019, 178, 508–521. [Google Scholar] [CrossRef]
- Zhou, S.W.; Zhuang, X.Y.; Zhu, H.H.; Rabczuk, T. Phase field modelling of crack propagation, branching and coalescence in rocks. Theor. Appl. Fract. Mech. 2018, 96, 174–192. [Google Scholar] [CrossRef] [Green Version]
- Lu, X.X.; Cheng, L.; Tie, Y.; Hou, Y.L.; Zhang, C.Z. Crack propagation simulation in brittle elastic materials by a phase field method. Theor. Appl. Mech. Lett. 2019, 9, 339–352. [Google Scholar] [CrossRef]
- Fakhimi, A.; Villegas, T. Application of dimensional analysis in calibration of a discrete element model for rock deformation and fracture. Rock Mech. Rock Eng. 2007, 40, 193–211. [Google Scholar] [CrossRef]
- Qiu, J.D.; Li, D.Y.; Li, X.B.; Zhou, Z.L. Dynamic fracturing behavior of layered rock with different inclination angles in SHPB tests. Shock Vib. 2017, 2017, 7687802. [Google Scholar] [CrossRef] [Green Version]
- Ergenzinger, C.; Seifried, R.; Eberhard, P. A discrete element model to describe failure of strong rock in uniaxial compression. Granul. Matter 2011, 13, 341–364. [Google Scholar] [CrossRef]
- Shang, J.; Duan, K.; Gui, Y.; Zhao, Z. Numerical investigation of the direct tensile behavior of laminated and transversely isotropic rocks containing incipient bedding planes with different strengths. Comput. Geotech. 2017, 104, 373–388. [Google Scholar] [CrossRef] [Green Version]
- Li, K.H.; Yin, Z.Y.; Cheng, Y.M.; Cao, P.; Meng, J.J. Three-dimensional discrete element simulation of indirect tensile behaviour of a transversely isotropic rock. Int. J. Numer. Anal. Methods Geomech. 2020, 44, 1812–1832. [Google Scholar] [CrossRef]
- Zhang, X.D.; Bui, T.Q. A fictitious crack XFEM with two new solution algorithms for cohesive crack growth modeling in concrete structures. Eng. Comput. 2015, 32, 473–497. [Google Scholar] [CrossRef]
- Wu, J.Y.; Qiu, J.F.; Nguyen, V.P.; Mandal, T.K.; Zhang, L.J. Computational modeling of localized failure in solids: XFEM vs PF-CZM. Comput. Methods Appl. Mech. Eng. 2019, 345, 618–643. [Google Scholar] [CrossRef]
- Haddad, M.; Sepehrnoori, K. XFEM-Based CZM for the simulation of 3D multiple-cluster hydraulic fracturing in quasi-brittle shale formations. Rock Mech. Rock Eng. 2016, 49, 4731–4748. [Google Scholar] [CrossRef]
- Peixoto, R.G.; Ribeiro, G.O.; Pitangueira, R.L.S. A boundary element method formulation for quasi-brittle material fracture analysis using the continuum strong discontinuity approach. Eng. Fract. Mech. 2018, 202, 47–74. [Google Scholar] [CrossRef]
- Ooi, E.T.; Natarajan, S.; Song, C.; Ooi, E.H. Crack propagation modelling in concrete using the scaled boundary finite element method with hybrid polygon-quadtree meshes. Int. J. Fract. 2017, 203, 135–157. [Google Scholar] [CrossRef]
- Chen, C.S.; Pan, E.; Amadei, B. Fracture mechanics analysis of cracked discs of aniso-tropic rock using the boundary element method. Int. J. Rock Mech. Min. 1998, 35, 195–218. [Google Scholar] [CrossRef]
- Crouch, S.L. Analysis of Stresses and Displacements around Underground Excavations: An Application of the Displacement Discontinuity Method, 2nd Printing; Geomechanics Report; University of Minnesota: Minneapolis, MN, USA, 1980. [Google Scholar]
- Kuriyama, K.; Mizuta, Y. Three-dimensional elastic analysis by the displacement discontinuity method with boundary division into triangular leaf elements. Int. J. Rock Mech. Min. 1993, 30, 111–123. [Google Scholar] [CrossRef]
- Marji, M.F. Numerical analysis of quasi-static crack branching in brittle solids by a modified displacement discontinuity method. Int. J. Solids Struct. 2014, 51, 1716–1736. [Google Scholar] [CrossRef] [Green Version]
- Abdollahipour, A.; Marji, M.F.; Bafghi, A.Y.; Gholamnejad, J. Time-dependent crack propagation in a poroelastic medium using a fully coupled hydromechanical displacement discontinuity method. Int. J. Fract. 2016, 199, 71–87. [Google Scholar] [CrossRef]
- Xiroudakis, G.; Stavropoulou, M.; Exadaktylos, G. Three-dimensional elastic analysis of cracks with the g2 constant displacement discontinuity method. Int. J. Numer. Anal. Methods Geomech. 2019, 43, 2355–2382. [Google Scholar] [CrossRef]
- Tang, C.A.; Tang, S.B. Applications of rock failure process analysis (RFPA) method. J. Rock Mech. Geotech. 2011, 3, 352–372. [Google Scholar] [CrossRef]
- Li, G.; Tang, C.A. A statistical meso-damage mechanical method for modeling trans-scale progressive failure process of rock. Int. J. Rock Mech. Min. 2015, 74, 133–150. [Google Scholar] [CrossRef]
- Sun, C.; Zheng, H.; Liu, W.D.; Lu, W.T. Numerical investigation of complex fracture network creation by cyclic pumping. Eng. Fract. Mech. 2020, 233, 107103. [Google Scholar] [CrossRef]
- Sun, B.; Liu, X.J.; Xu, Z.D. A novel physical continuum damage model for the finite element simulation of crack growth mechanism in quasi-brittle geomaterials. Theor. Appl. Fract. Mech. 2021, 114, 103030. [Google Scholar] [CrossRef]
- Miehe, C.; Hofacker, M.; Schänzel, L.-M.; Aldakheel, F. Phase field modeling of fracture in multi-physics problems. Part II. Coupledbrittle-to-ductile failure criteria and crack propagation in thermo-elastic–plastic solids. Comput. Methods Appl. Mech. Eng. 2015, 294, 486–522. [Google Scholar] [CrossRef]
- Staroselsky, A.; Acharya, R.; Cassenti, B. Phase field modeling of fracture and crack growth. Eng. Fract. Mech. 2019, 205, 268–284. [Google Scholar] [CrossRef]
- Noll, T.; Kuhn, C.; Olesch, D.; Müller, R. 3D phase field simulations of ductile fracture. GAMM-Mitt. 2020, 43, e202000008. [Google Scholar] [CrossRef] [Green Version]
- Wei, C.H.; Zhu, W.C.; Chen, S.K.; Ranjith, P.G. A coupled thermal–hydrological–mechanical damage model and its numerical simulations of damage evolution in APSE. Materials 2016, 9, 841. [Google Scholar] [CrossRef] [Green Version]
- Wang, Z. A Modified Mohr Coulomb Criterion for Rocks with Smooth Tension Cut-off. IOP Conf. Ser. Earth Environ. Sci. 2020, 525, 012027. [Google Scholar] [CrossRef]
- Zhou, G.L.; Xu, T.; Heap, M.J.; Meredith, P.G.; Mitchell, T.M.; Sesnic, A.S.Y.; Yuan, Y. A three-dimensional numerical meso-approach to modeling time- independent deformation and fracturing of brittle rocks. Comput Geotech. 2020, 117, 103274. [Google Scholar] [CrossRef]
- She, C.X.; Liu, J. Two-parameter parabolic-type yield criterion based on Mohr strength theory. Eng. J. Wuhan Univ. 2008, 41, 33–36. [Google Scholar]
- Gill, S. A damage model for the frictional shear failure of brittle materials in compression. Comput. Methods Appl. Mech. Eng. 2021, 385, 114048. [Google Scholar] [CrossRef]
- Murakami, S. Continuum Damage Mechanics: A Continuum Mechanics Approach to the Analysis of Damage and Fracture; Springer Science & Business Media: Nagoya, Japan, 2012; pp. 19–20. [Google Scholar]
- Tang, S.; Zhang, H.; Tang, C.; Liu, H. Numerical model for the cracking behavior of heterogeneous brittle solids subjected to thermal shock. Int. J. Solids Struct. 2016, 80, 520–531. [Google Scholar] [CrossRef]
- Chen, Z.H.; Tham, L.G.; Yeung, M.R.; Xie, H. Confinement effects for damage and failure of brittle rocks. Int. J. Rock Mech. Min. 2006, 43, 1262–1269. [Google Scholar] [CrossRef]
- Guo, T.K.; Du, Z.M. Meso-numerical simulation of rock triaxial compression based on the damage model. Conf. Ser. Earth Environ. Sci. 2021, 687, 012134. [Google Scholar] [CrossRef]
- Zong, Y.J.; Han, L.J.; Wei, J.J.; Wen, S.Y. Mechanical and damage evolution properties of sandstone under triaxial compression. Int. J. Min. Sci. Technol. 2016, 26, 601–607. [Google Scholar] [CrossRef]
- Yao, M.D.; Rong, G.; Zhou, C.B.; Peng, J. Effects of Thermal Damage and Confining Pressure on the Mechanical Properties of Coarse Marble. Rock Mech. Rock Eng. 2016, 49, 2043–2054. [Google Scholar] [CrossRef]
- Zhang, J.C.; Lin, Z.N.; Dong, B.; Guo, R.X. Triaxial Compression Testing at Constant and Reducing Confining Pressure for the Mechanical Characterization of a Specific Type of Sandstone. Rock Mech. Rock Eng. 2021, 54, 1999–2012. [Google Scholar] [CrossRef]
- Benz, T.; Schwab, R.; Kauther, R.A.; Vermeer, P.A. A Hoek–Brown criterion with intrinsic material strength factorization. Int. J. Rock Mech. Min. 2008, 45, 210–222. [Google Scholar] [CrossRef]
- Khosravani, M.R.; Friebertshäuser, K.; Weinberg, K. On the use of peridynamics in fracture of ultra-high performance concrete. Mech. Res. Commun. 2022, 123, 103899. [Google Scholar] [CrossRef]
- You, M.; Su, C. Experimental study on split test with flattened disk and tensile strength of rock. Chin. J. Rock Mech. Eng. 2004, 23, 3106–3112. [Google Scholar]
- Yan, Z.L.; Dai, F.; Liu, Y.; Wei, M.D.; You, W. New insights into the fracture mechanism of flattened Brazilian disc specimen using digital image correlation. Eng. Fract. Mech. 2021, 252, 107810. [Google Scholar] [CrossRef]
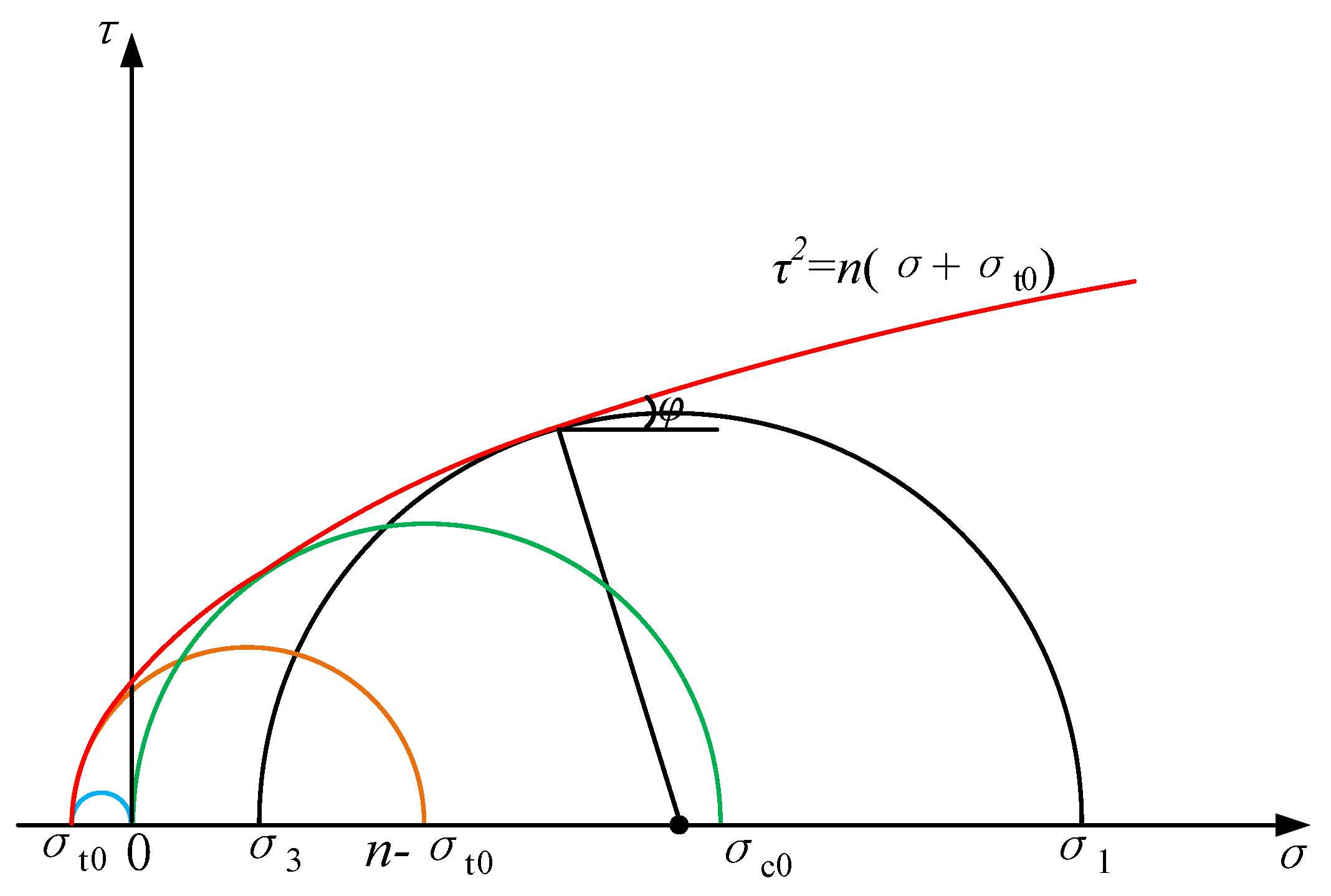





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, H.; Zhou, B.; Xue, S.; Deng, X.; Jia, P.; Zhu, X. An Anisotropic Damage Model of Quasi-Brittle Materials and Its Application to the Fracture Process Simulation. Appl. Sci. 2022, 12, 12073. https://doi.org/10.3390/app122312073
Wang H, Zhou B, Xue S, Deng X, Jia P, Zhu X. An Anisotropic Damage Model of Quasi-Brittle Materials and Its Application to the Fracture Process Simulation. Applied Sciences. 2022; 12(23):12073. https://doi.org/10.3390/app122312073
Chicago/Turabian StyleWang, Haijing, Bo Zhou, Shifeng Xue, Xuejing Deng, Peng Jia, and Xiuxing Zhu. 2022. "An Anisotropic Damage Model of Quasi-Brittle Materials and Its Application to the Fracture Process Simulation" Applied Sciences 12, no. 23: 12073. https://doi.org/10.3390/app122312073
APA StyleWang, H., Zhou, B., Xue, S., Deng, X., Jia, P., & Zhu, X. (2022). An Anisotropic Damage Model of Quasi-Brittle Materials and Its Application to the Fracture Process Simulation. Applied Sciences, 12(23), 12073. https://doi.org/10.3390/app122312073








