Creep Characteristics of Soil in the Sliding Zone of Huangtupo Landslide
Abstract
1. Introduction
2. Materials and Methods
2.1. Test Materials
2.2. Test Apparatus and Procedures
3. Results of the Creep Tests
3.1. Compressibility of Remolded Samples
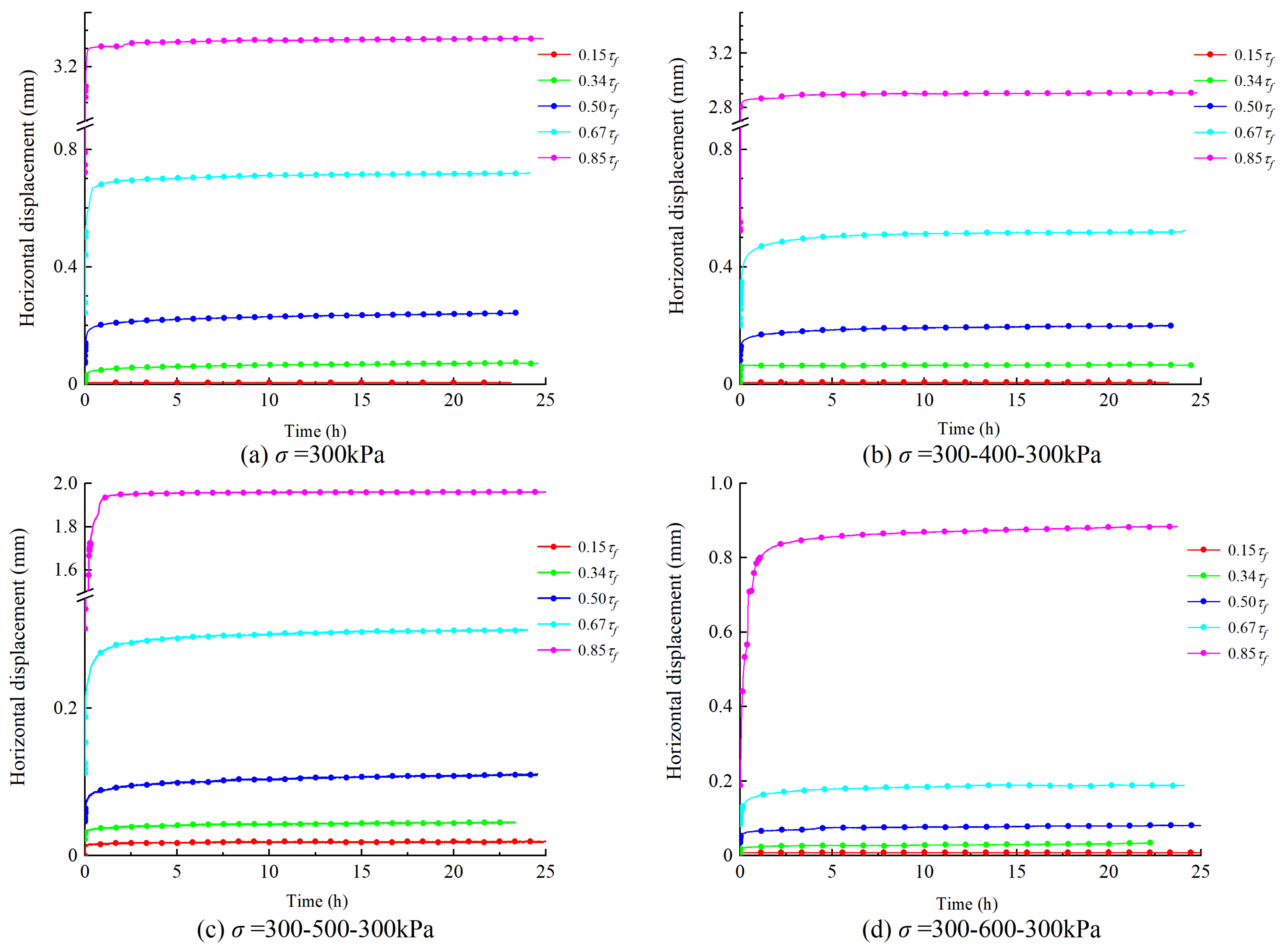
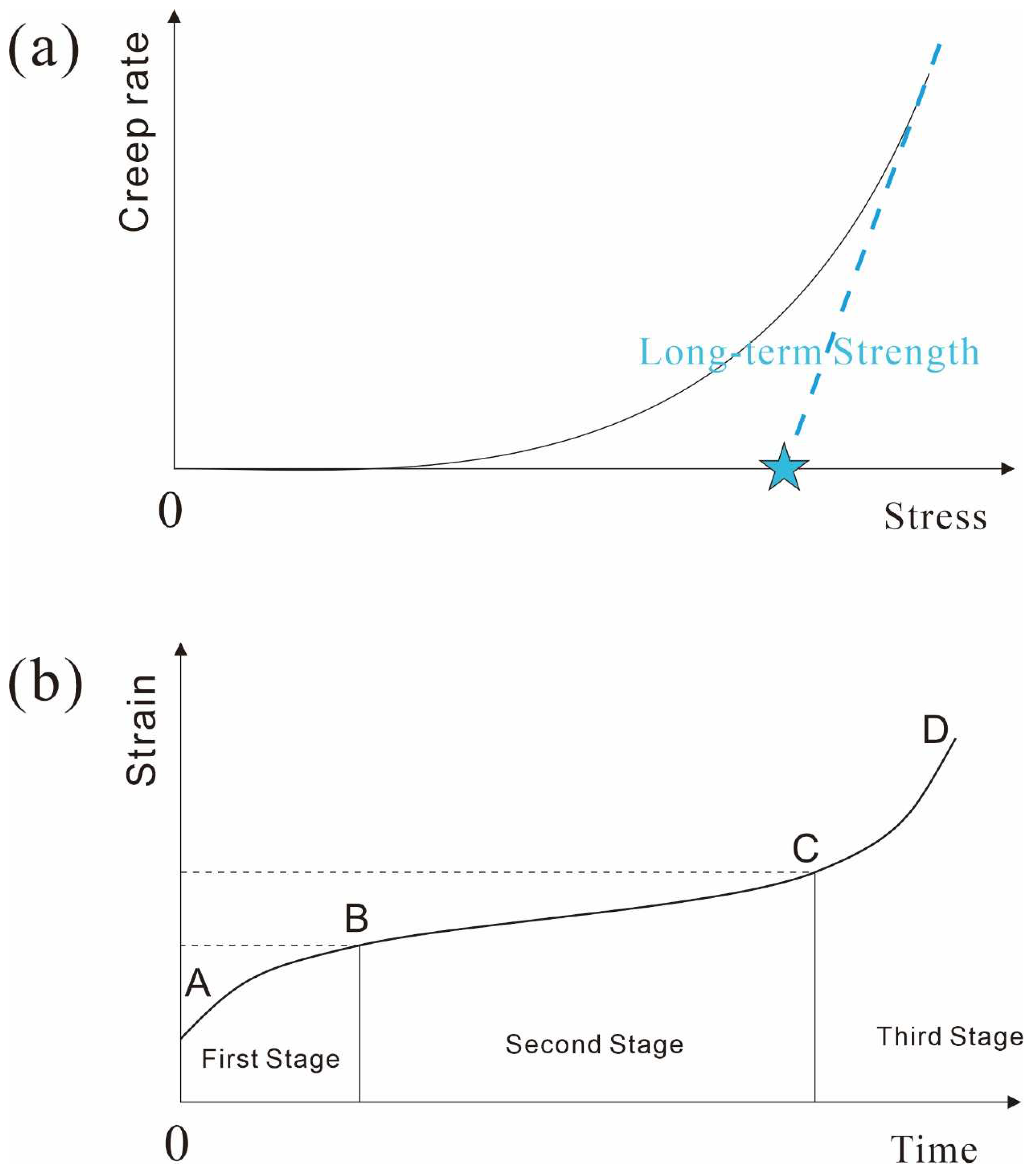
3.2. Analysis of Creep Displacement–Time Relationship
3.3. Analysis of Isochronous Creep Characteristics
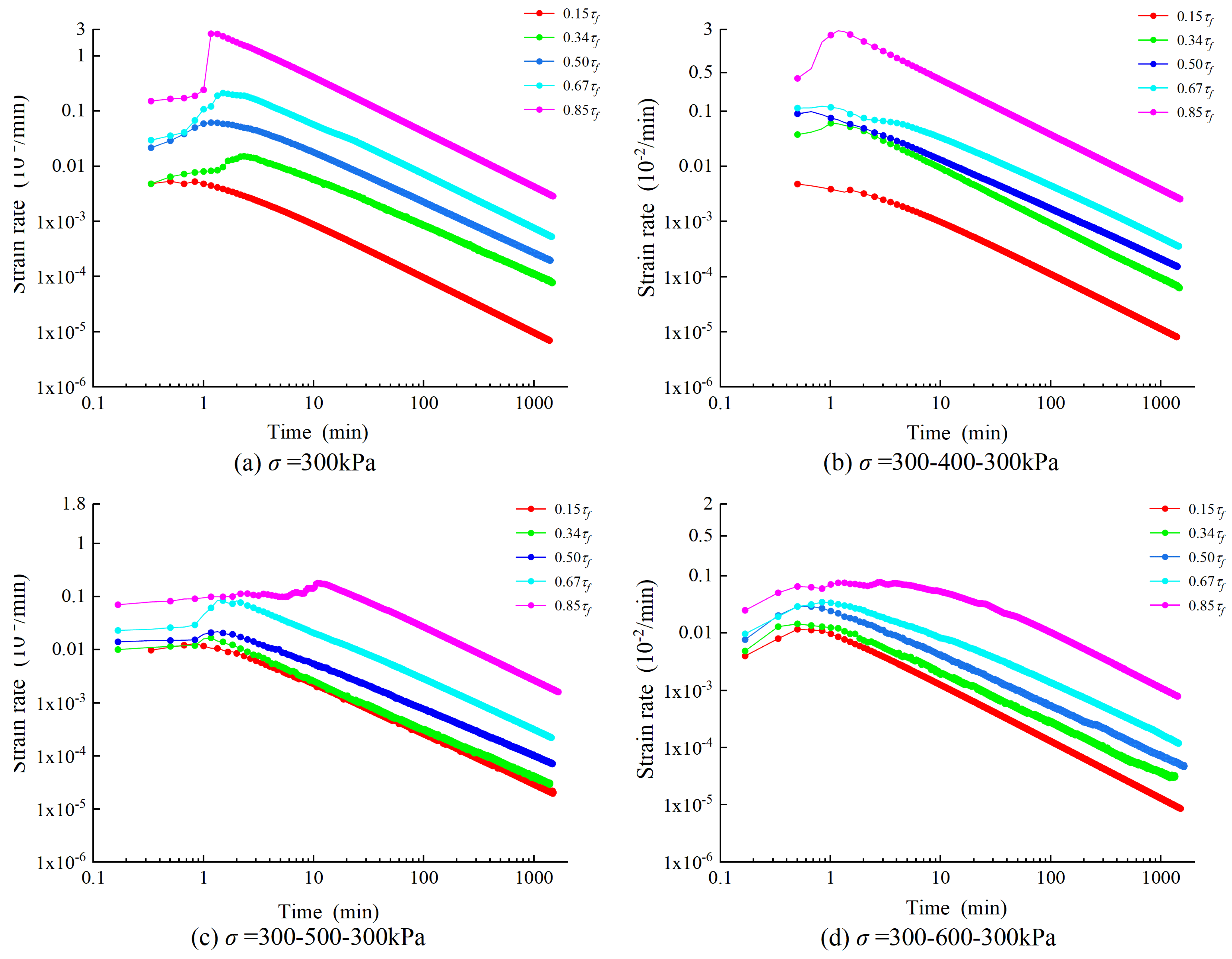
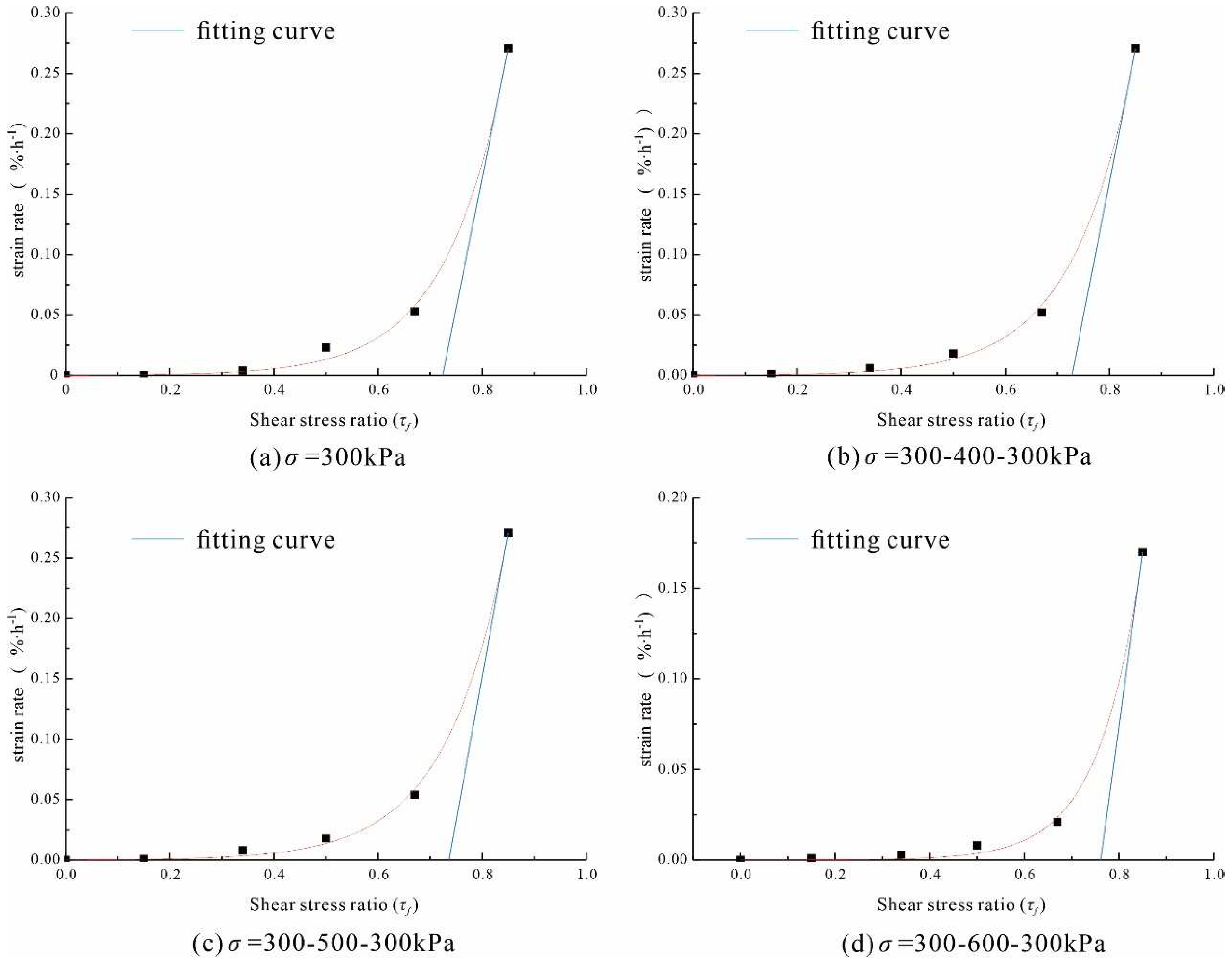
3.4. Analysis of Creep Rate
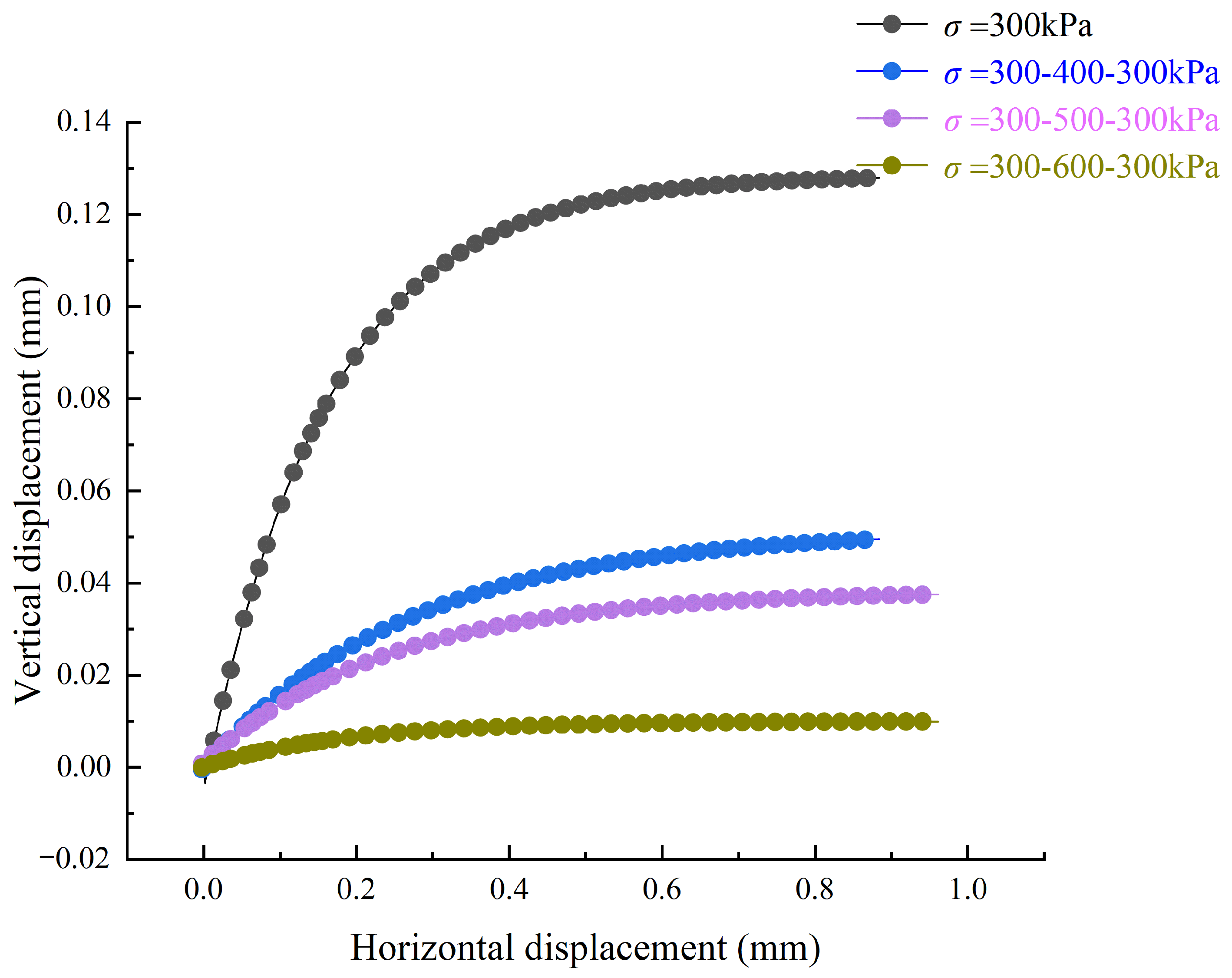
3.5. Analysis of Dilatancy
4. Shear Creep Model and Verification
4.1. Construction of Burgers Constitutive Model
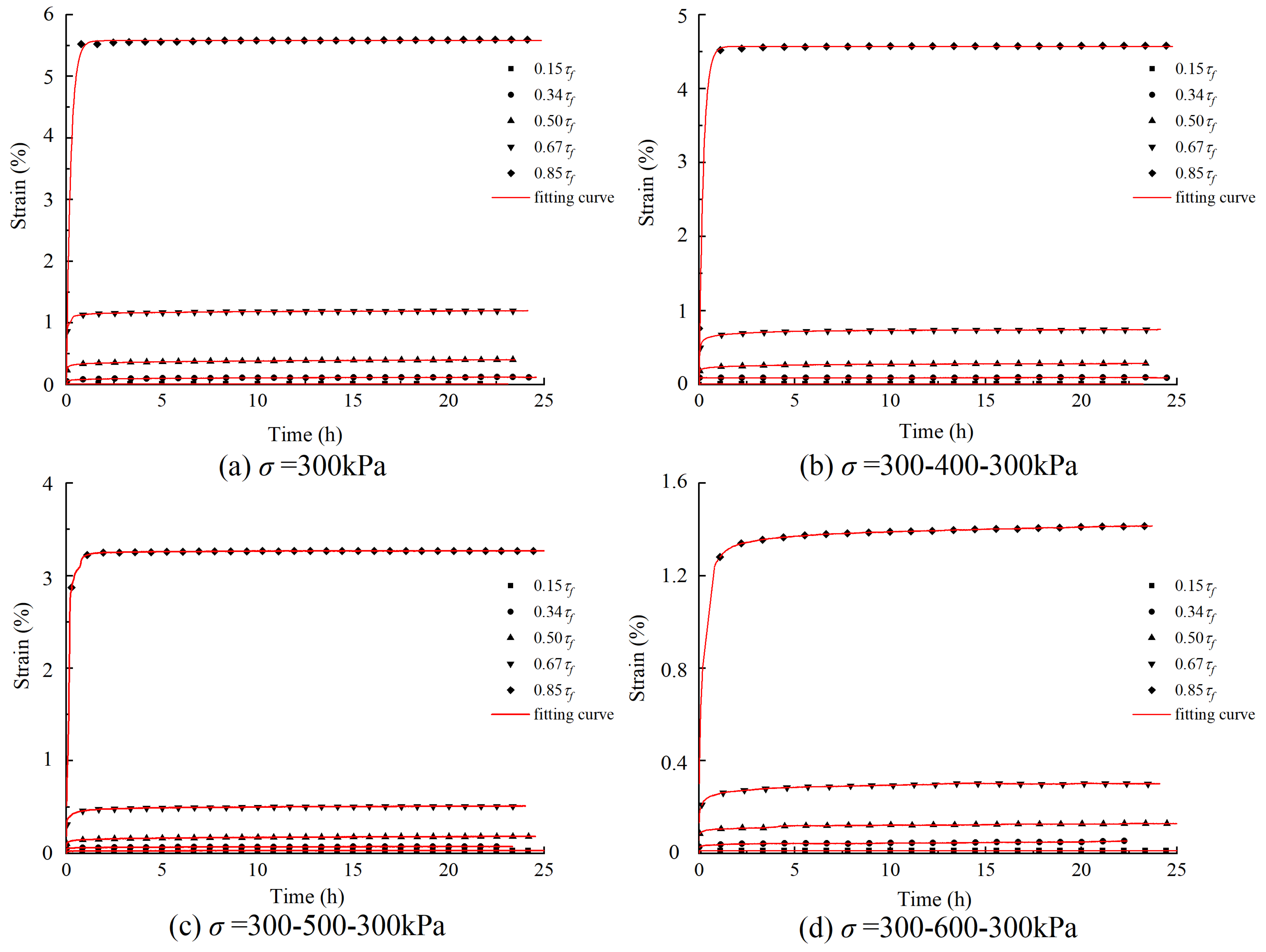
4.2. The Comparison of Fitted Curves and Experimental Curves
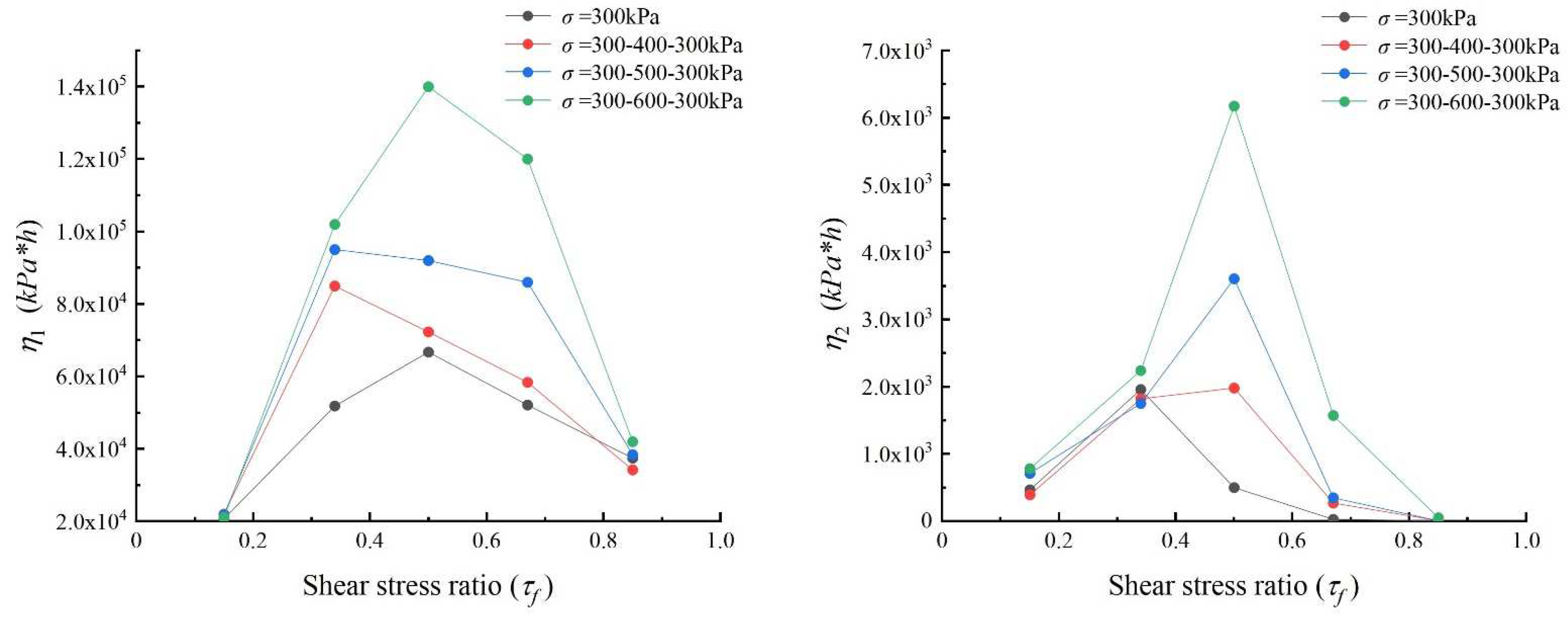
4.3. Analysis of the Parametric Properties of the Burgers Model
5. Discussion
6. Conclusions
- The direct shear creep of the sliding zone soil was mainly divided into the attenuation creep stage and the steady creep stage; the accelerated creep stage was not observed. At the moment of the application of shear stress, the sliding zone soil produced instantaneous deformation, and the degree of deformation was positively correlated with the shear stress. The sliding zone soil was in the attenuation creep stage, and the creep rate decayed rapidly to zero with the low shear stress; the sliding zone soil was in the steady-state creep stage, the creep rate decayed to a constant value, and the visco-plastic creep characteristics were more obvious with the higher shear stress.
- With the increase in the pre-consolidation pressure applied to the sliding zone soil, the creep displacement was correspondingly reduced. When the level of loading stress was high, the shear strain of the sliding soil was also at a high level. Additionally, the strain rate of the sliding zone soil was positively correlated with shear stress and negatively correlated with the previous consolidation pressure. In this study, shear shrinkage occurred during the shear process.
- We found that the Burgers model can accurately fit the creep process of sliding zone soil. The shear modulus of the Burgers model was positively correlated with the applied shear stress. When the applied shear stress increased, the viscosity coefficient increased first and then decreased. The long-term strength value of the sliding zone soil was obtained using the steady-state creep rate method, and the value was about 72% of the peak strength. The long-term strength also increased with the increase in consolidation stress.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wang, S.; Wu, W.; Wang, J.; Yin, Z.; Cui, D.S.; Xiang, W. Residual-state creep of clastic soil in a reactivated slow-moving landslide in the Three Gorges Reservoir Region, China. Landslides 2018, 15, 2413–2422. [Google Scholar] [CrossRef]
- Tan, Q.W.; Tang, H.M.; Fan, L.; Xiong, C.R.; Fan, Z.Q.; Zhao, M.; Li, C.; Wang, D.J.; Zou, Z.X. In situ triaxial creep test for investigating deformational properties of gravelly sliding zone soil: Example of the Huangtupo 1# landslide, China. Landslides 2018, 15, 2499–2508. [Google Scholar]
- Miao, H.; Wang, G. Effects of clay content on the shear behaviors of sliding zone soil originating from muddy interlayers in the Three Gorges Reservoir, China. Eng. Geol. 2021, 294, 106380. [Google Scholar] [CrossRef]
- Zou, L.; Wang, S.; Lai, X. Creep model for unsaturated soils in sliding zone of Qianjiangping landslide. J. Rock Mech. Geotech. Eng. 2013, 5, 162–167. [Google Scholar] [CrossRef]
- Luo, Q.; Chen, X. Experimental Research on Creep Characteristics of Nansha Soft Soil. Sci. World J. 2014, 2014, 968738. [Google Scholar] [CrossRef]
- Lian, B.Q.; Wang, X.G.; Zhan, H.B.; Wang, J.D.; Peng, J.B.; Gu, T.F.; Zhu, R.S. Creep mechanical and microstructural insights into the failure mechanism of loess landslides induced by dry-wet cycles in the Heifangtai platform, China. Eng. Geol. 2022, 300, 106589. [Google Scholar] [CrossRef]
- Xie, X.; Qi, S.W.; Zhao, F.S.; Wang, D.H. Creep behavior and the microstructural evolution of loess-like soil from Xi’an area, China. Eng. Geol. 2018, 236, 43–59. [Google Scholar] [CrossRef]
- Feng, X. Experiment and Numerical Simulation on Creep Mechanical Behaviors of Mudstone under Unloading Condition. Geofluids 2022, 2022, 7175774. [Google Scholar] [CrossRef]
- Wang, S.; Wang, J.E.; Wu, W.; Cui, D.S.; Su, A.J.; Xiang, W. Creep properties of clastic soil in a reactivated slow-moving landslide in the Three Gorges Reservoir Region, China. Eng. Geol. 2020, 267, 105493. [Google Scholar] [CrossRef]
- Long, J.H.; Guo, W.B.; Li, P.; Li, T.L. Creep property of soil in sliding zone of loess landslide. Yantu Gongcheng Xuebao/Chin. J. Geotech. Eng. 2010, 32, 1023–1028. [Google Scholar]
- Qin, Z.; Fu, H.L.; Chen, X.X. A study on altered granite meso-damage mechanisms due to water invasion-water loss cycles. Environ. Earth Sci. 2019, 78, 10. [Google Scholar] [CrossRef]
- Sun, M.J.; Tang, H.M.; Wang, X.H.; Hu, X.L.; Wang, M.Y.; Ni, W.D. Creep properties of sliding-zone soil from a creeping landslide. Rock Soil Mech. 2017, 38, 385–391. [Google Scholar]
- Nazer, N.S.M.; Tarantino, A. Creep response in shear of clayey geo-materials under saturated and unsaturated conditions. In Proceedings of the 3rd European Conference on Unsaturated Soils (E-UNSAT), Paris, France, 12–14 September 2016. [Google Scholar]
- Zhu, F.; Duan, Z.Y.; Wu, Z.Y.; Wu, Y.Q.; Li, T.L.; Cai, Y.D. Experimental Study on Direct Shear Creep Characteristics and Long-term Strength of Red Layer Sliding Zone Soil in Southern Hunan. In Proceedings of the International Conference on Materials, Mechanical and Manufacturing Engineering (IC3ME 2013), Guilin, China, 15 August 2014. [Google Scholar]
- Jiang, X.Z.; Wen, B.P. Creep behavior of slip zone of reactivated slow-moving landslide and its characteristic strength. Rock Soil Mech. 2015, 36, 495–501, 549. [Google Scholar]
- Mahinroosta, R.; Poorjafar, A. Effect of stress state and particle-size distribution on the stress reduction of sandy soils during saturation. Constr. Build. Mater. 2017, 150, 1–13. [Google Scholar] [CrossRef]
- Li, J.J.; Kong, L.W. Creep Properties of Expansive Soils under Triaxial Drained Conditions and its Nonlinear Constitutive Model. Period. Polytech. Civ. Eng. 2021, 65, 1269–1278. [Google Scholar] [CrossRef]
- Kutergin, V.N.; Kal’bergenov, R.G.; Karpenko, F.S.; Leonov, A.R.; Merzlyakov, V.P. Determination of Rheological Properties of Clayey Soils by the Relaxation Method. Soil Mech. Found. Eng. 2013, 50, 1–6. [Google Scholar] [CrossRef]
- Xiao, B.; Hu, M.Y.; Zhou, P.Y.; Lu, Y.K.; Zhang, Y. Creep Behavior of Saturated Clay in Triaxial Test and a Hyperbolic Model. Geofluids 2021, 2021, 7882046. [Google Scholar] [CrossRef]
- Gu, R.G.; Zou, Y.; Fang, Y.G.; Hu, Y.G. Rheological model of soft soils using nonlinear instantaneous elastic modulus. Rock Soil Mech. 2018, 39, 237–241. [Google Scholar]
- Ma, B.N.; Xie, X.Y.; Liu, K.F. Rheological catastrophic model for soft clays. J. Cent. South Univ. 2012, 19, 2316–2322. [Google Scholar] [CrossRef]
- Hu, H.; Zhang, B.W.; Yu, W.; Lu, L.F.; Zhang, C.M.; Niu, T.X. Rheological Behaviors of Structural Soft Soil in Dongting Lake Area. Geotech. Geol. Eng. 2020, 38, 4995–5003. [Google Scholar] [CrossRef]
- Zhu, Y.B.; Yu, H.M. An improved Mesri creep model for unsaturated weak intercalated soils. J. Cent. South Univ. 2014, 21, 4677–4681. [Google Scholar] [CrossRef]
- Wang, P.; Liu, E.L.; Song, B.T.; Liu, X.Y.; Zhang, G.; Zhang, D. Binary medium creep constitutive model for frozen soils based on homogenization theory. Cold Reg. Sci. Technol. 2019, 162, 35–42. [Google Scholar] [CrossRef]
- Li, L.L.; Guan, J.F.; Xiao, M.L.; Liu, H.C.; Tang, K.D. A creep constitutive model for transversely isotropic rocks. Rock Soil Mech. 2020, 41, 2922–2930. [Google Scholar]
- Okuka, A.S.; Zorica, D. Fractional Burgers models in creep and stress relaxation tests. Appl. Math. Model. 2020, 77, 1894–1935. [Google Scholar] [CrossRef]
- Zhao, T.B.; Zhang, Y.B.; Zhang, Q.Q.; Tan, Y.L. Analysis on the creep response of bolted rock using bolted Burgers model. Geomech. Eng. 2018, 14, 141–149. [Google Scholar]
- Zhao, Y.L.; Liu, Q.; Tang, L.M.; Xie, S.L. The double Burgers model of fractured rock masses considering creep fracture damage. J. Vibroeng. 2019, 21, 974–987. [Google Scholar] [CrossRef]
- Chang, Z.L.; Gao, H.X.; Huang, F.M.; Chen, J.W.; Huang, J.S.; Guo, Z.Z. Study on the creep behaviours and the improved Burgers model of a loess landslide considering matric suction. Nat. Hazards 2020, 103, 1479–1497. [Google Scholar] [CrossRef]
- Tang, H.M.; Li, C.D.; Hu, X.L.; Wang, L.Q.; Criss, R.; Su, A.J.; Wu, Y.P.; Xiong, C.R. Deformation response of the Huangtupo landslide to rainfall and the changing levels of the Three Gorges Reservoir. Bull. Eng. Geol. Environ. 2014, 74, 933–942. [Google Scholar] [CrossRef]
- Cao, Z.E.; Tang, J.; Zhao, X.E.; Zhang, Y.G.; Wang, B.; Li, L.C.; Guo, F. Failure Mechanism of Colluvial Landslide Influenced by the Water Level Change in the Three Gorges Reservoir Area. Geofluids 2021, 2021, 6865129. [Google Scholar] [CrossRef]
- Tang, H.M.; Li, C.D.; Hu, X.L.; Su, A.J.; Wang, L.Q.; Wu, Y.P.; Criss, R.; Xiong, C.R.; Li, Y.A. Evolution characteristics of the Huangtupo landslide based on in situ tunnelling and monitoring. Landslides 2014, 12, 511–521. [Google Scholar] [CrossRef]
- Wang, J.B.; Zhang, Q.; Song, Z.P.; Zhang, Y.W. Experimental study on creep properties of salt rock under long-period cyclic loading. Int. J. Fatigue 2021, 143, 10. [Google Scholar] [CrossRef]
- Kolarik, J.; Pegoretti, A. Proposal of the Boltzmann-like superposition principle for nonlinear tensile creep of thermoplastics. Polym. Test. 2008, 27, 596–606. [Google Scholar] [CrossRef]
- Li, C.; Tang, H.M.; Han, D.W.; Zou, Z.X. Exploration of the creep properties of undisturbed shear zone soil of the Huangtupo landslide. Bull. Eng. Geol. Environ. 2017, 78, 1237–1248. [Google Scholar] [CrossRef]
- MWR (Ministry of Water Resources). GB/T 50123-2019; Standard for Geotechnical Testing Method. MWR: Beijing, China, 2019.
- Dafalias, Y.F. An anisotropic critical state clay plasticity model. Mech. Res. Commun. 1986, 13, 341–347. [Google Scholar] [CrossRef]
- Majid, T.; Nourm, A. Significance of sail dilatancy in slope analysis. J. Geotech. Environ. Eng. ASCE 2000, 26, 75–80. [Google Scholar]
- Zhao, C.F.; Wu, Y.; Zhao, C.; Liu, F.M.; Liu, F. Effect of unloading on shear behaviour of interface between sand and concrete. Chin. J. Rock Mech. Eng. 2018, 37, 1020–1029. [Google Scholar]
- Chen, Q.; Cui, D.S.; Wang, J.E.; Liu, Q.B. An experimental study of creep characteristics of sliding zone soil of Huangtupo landslide under different consolidation stresses. Rock Soil Mech. 2020, 41, 1635–1642. [Google Scholar]
- Zhang, W.B.; Ma, J.Z.; Tang, L. Experimental study on shear strength characteristics of sulfate saline soil in Ningxia region under long-term freeze-thaw cycles. Cold Reg. Sci. Technol. 2019, 160, 48–57. [Google Scholar] [CrossRef]
- Chang, C.R.; Wang, G.H. Creep of clayey soil induced by elevated pore-water pressure: Implication for forecasting the time of failure of rainfall-triggered landslides. Eng. Geol. 2022, 296, 106461. [Google Scholar] [CrossRef]
- Chen, Z.J. The mechanical problems for the long-term stability of ground galleries. Chin. J. Rock Me-Chanics Eng. 1982, 1, 1–20. [Google Scholar]
- Vyalov, S.S.; Meschyan, S.R. Creep and Long-Term Strength of Soils Subjected to Variable Load. In Proceedings of the Soil Mechanics and Foundation Engineering Mexico, Mexico City, Mexico, 1 January 1969; pp. 423–431. [Google Scholar]
- Shibata, T.; Karube, K. Creep Rate and Creep Strength of Clays. In Proceedings of the 7th International Conference on Soil Mechanics and Foundation Engineering, Mexico City, Mexico, 1 January 1969. [Google Scholar]
- Zhu, R.S.; Xie, W.L.; Liu, Q.Q.; Yang, H.; Wang, Q.Y. Shear behavior of sliding zone soil of loess landslides via ring shear tests in the South Jingyang Plateau. Bull. Eng. Geol. Environ. 2022, 81, 244. [Google Scholar] [CrossRef]
- Munkholm, L.J.; Heck, R.J.; Deen, B.; Zidar, T. Relationship between soil aggregate strength, shape and porosity for soils under different long-term management. Geoderma 2016, 268, 52–59. [Google Scholar] [CrossRef]


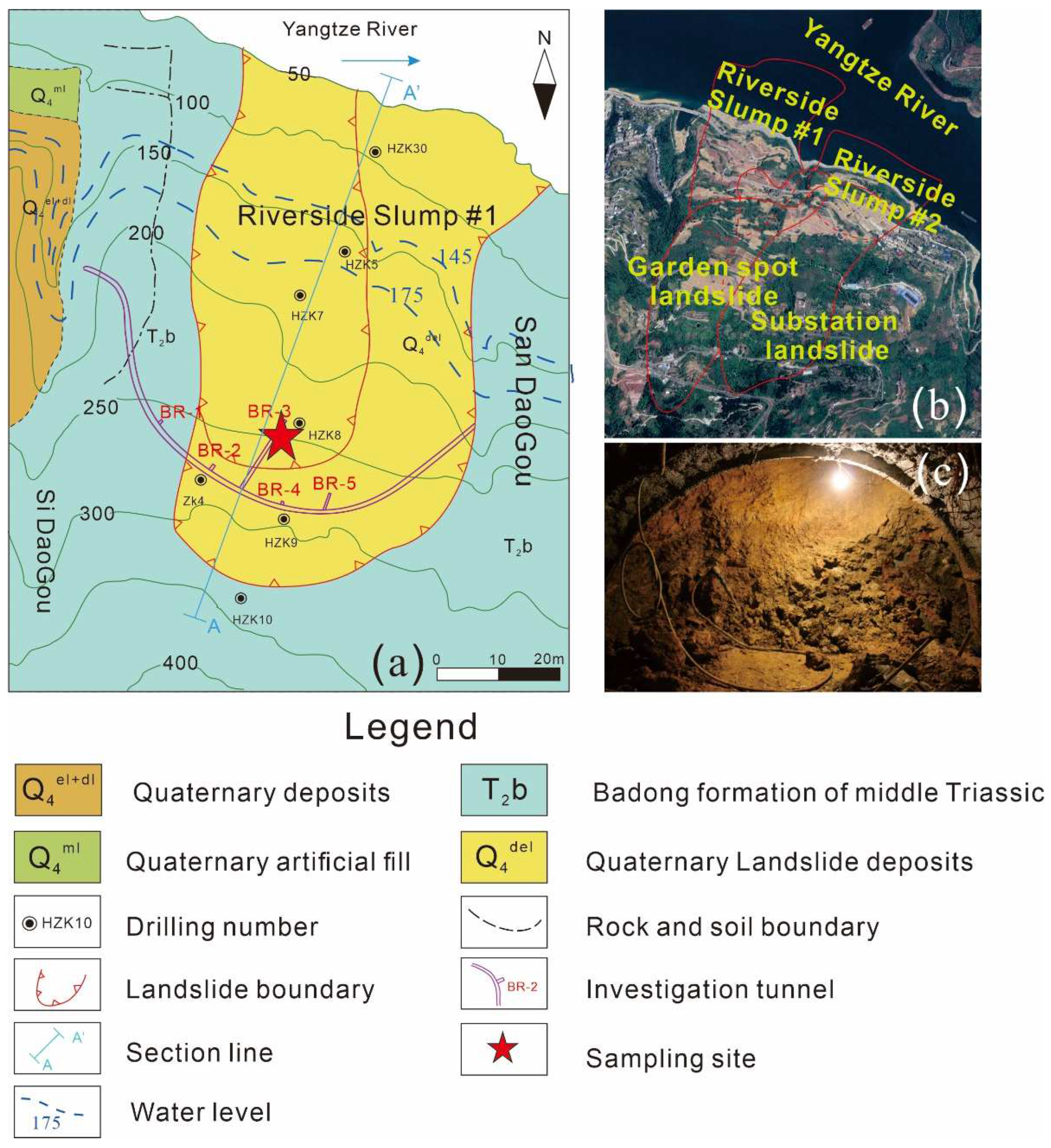
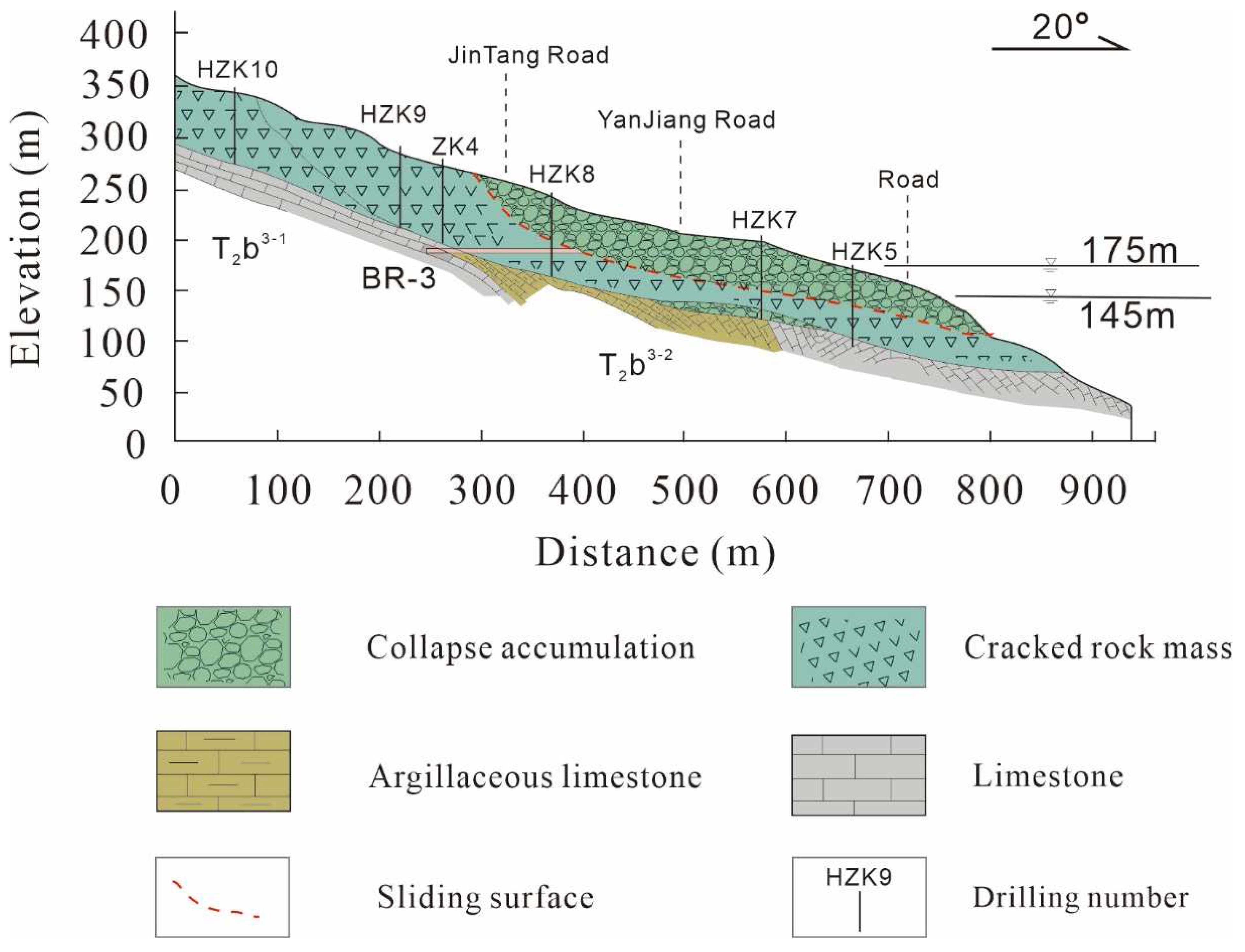
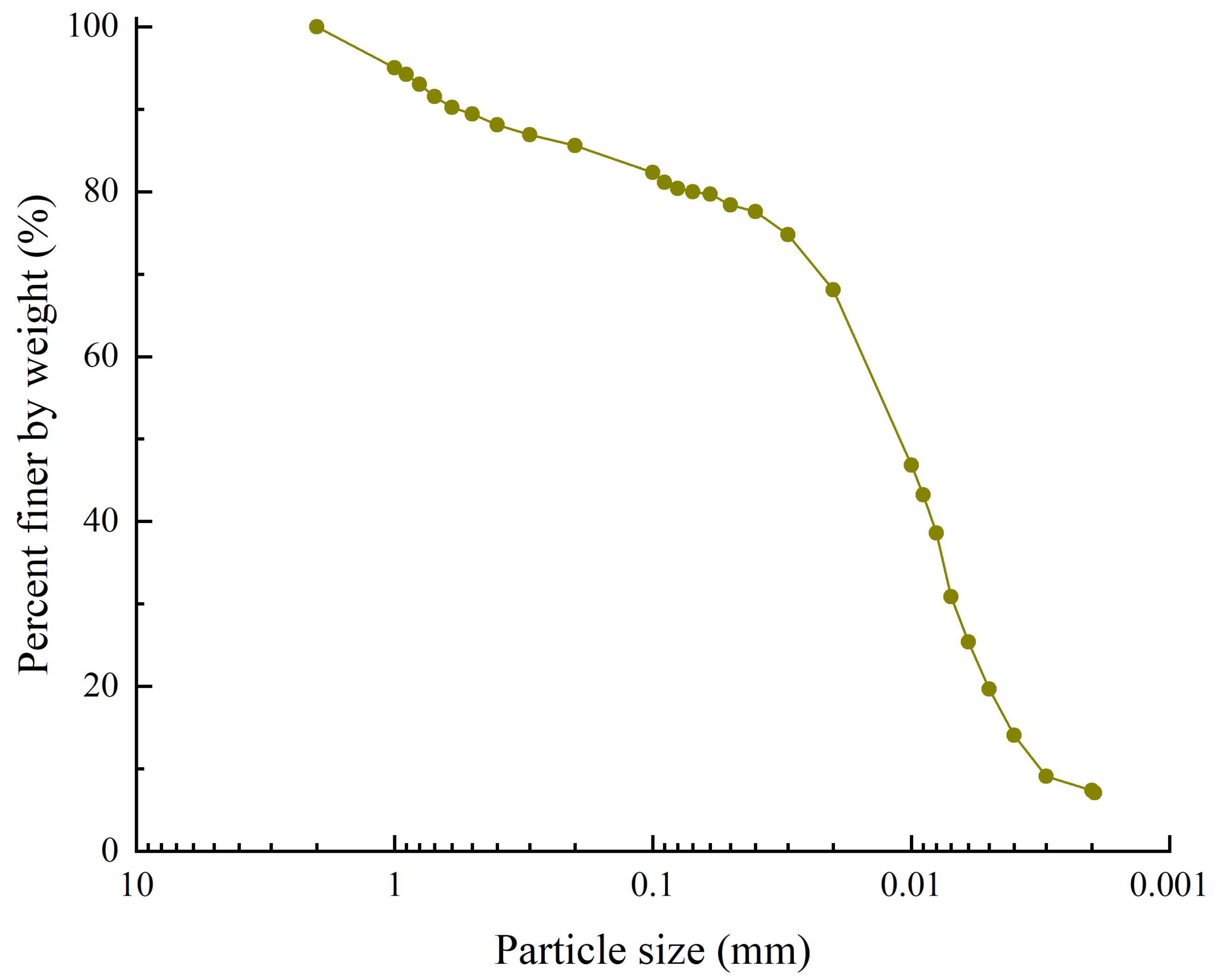

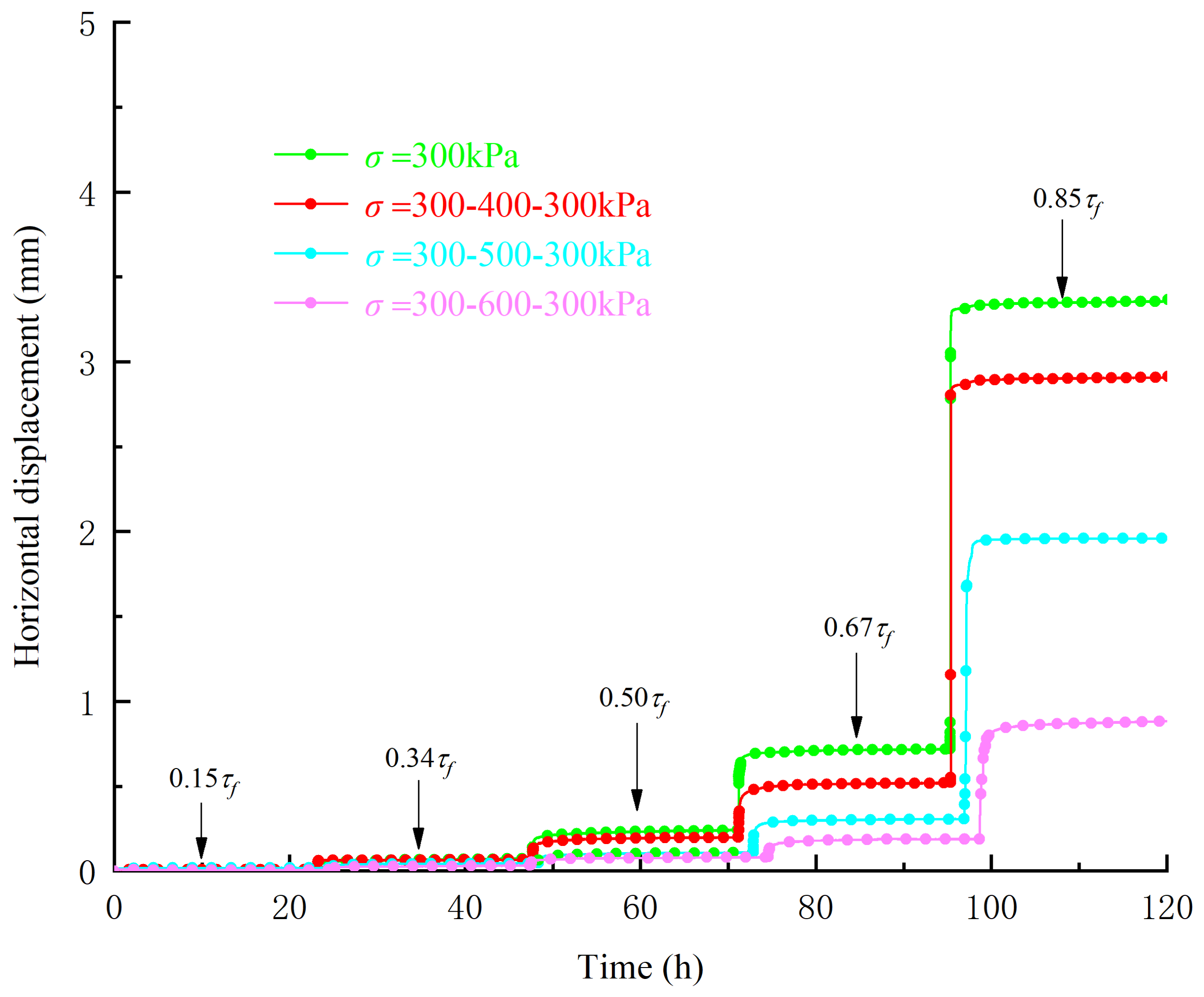




| Sample Number | Loading–Unloading Amplitude R (kPa) | Consolidation Path | Shear Stress (kPa) |
|---|---|---|---|
| HD-1 | 0 | 300 | 17.85→40.47→59.51→79.74→101.17 |
| HD-2 | 100 | 300-400-300 | 22.15→50.21→73.85→98.95→125.54 |
| HD-3 | 200 | 300-500-300 | 26.46→59.97→88.19→118.17→149.91 |
| HD-4 | 300 | 300-600-300 | 30.76→69.71→102.52→137.38→174.28 |
| Sample Number | τ (kPa) | G1 (kPa) | G2 (kPa) | (kPa h) | (kPa h) | R2 |
|---|---|---|---|---|---|---|
| HD-1 | 17.85 | 1358 | 3198 | 2.07 × 104 | 468 | 0.885 |
| 40.47 | 759 | 1501 | 5.18 × 104 | 1960 | 0.964 | |
| 59.51 | 293.4 | 807.4 | 6.67 × 104 | 501.2 | 0.94 | |
| 79.74 | 160.9 | 198.8 | 5.21 × 104 | 28.8 | 0.91 | |
| 101.17 | 23.29 | 50.6 | 3.74 × 104 | 6.78 | 0.88 | |
| HD-2 | 22.15 | 1567 | 3215 | 2.20 × 104 | 394 | 0.896 |
| 50.21 | 803.6 | 1824 | 8.50 × 104 | 1820 | 0.895 | |
| 73.85 | 318.6 | 1158 | 7.23 × 104 | 1982 | 0.967 | |
| 98.95 | 175.4 | 423.9 | 5.84 × 104 | 269.7 | 0.905 | |
| 125.54 | 30.56 | 60.8 | 3.42 × 104 | 10.21 | 0.971 | |
| HD-3 | 26.46 | 1711 | 4054 | 2.18 × 104 | 714 | 0.919 |
| 59.97 | 903.2 | 3982 | 9.50 × 104 | 1750 | 0.956 | |
| 88.19 | 600.2 | 2169 | 9.20 × 104 | 3605 | 0.966 | |
| 118.17 | 301.3 | 709.7 | 8.60 × 104 | 348.8 | 0.926 | |
| 149.91 | 3.84 | 39.6 | 3.84 × 104 | 6.31 | 0.971 | |
| HD-4 | 30.76 | 2048 | 5312 | 2.10 × 104 | 783 | 0.901 |
| 69.71 | 1699 | 4839.9 | 1.02 × 105 | 2239 | 0.9522 | |
| 102.52 | 749.3 | 3158.4 | 1.40 × 105 | 6172 | 0.9646 | |
| 137.38 | 474.4 | 1273.9 | 1.20 × 105 | 1573 | 0.9454 | |
| 174.28 | 300.2 | 131.24 | 4.20 × 104 | 53.26 | 0.987 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liao, M.; Cui, D.; Bao, X.; Qiao, Z.; Zhao, C. Creep Characteristics of Soil in the Sliding Zone of Huangtupo Landslide. Appl. Sci. 2022, 12, 12439. https://doi.org/10.3390/app122312439
Liao M, Cui D, Bao X, Qiao Z, Zhao C. Creep Characteristics of Soil in the Sliding Zone of Huangtupo Landslide. Applied Sciences. 2022; 12(23):12439. https://doi.org/10.3390/app122312439
Chicago/Turabian StyleLiao, Mingke, Deshan Cui, Xun Bao, Zhuo Qiao, and Chenxi Zhao. 2022. "Creep Characteristics of Soil in the Sliding Zone of Huangtupo Landslide" Applied Sciences 12, no. 23: 12439. https://doi.org/10.3390/app122312439
APA StyleLiao, M., Cui, D., Bao, X., Qiao, Z., & Zhao, C. (2022). Creep Characteristics of Soil in the Sliding Zone of Huangtupo Landslide. Applied Sciences, 12(23), 12439. https://doi.org/10.3390/app122312439





