Characteristics of Local Geomagnetic Field Variations and the Tectonic Stress Field Adjacent to the 21 May 2021, Ms 6.4 Yangbi Earthquake, Yunnan, China
Abstract
:Featured Application
Abstract
1. Introduction
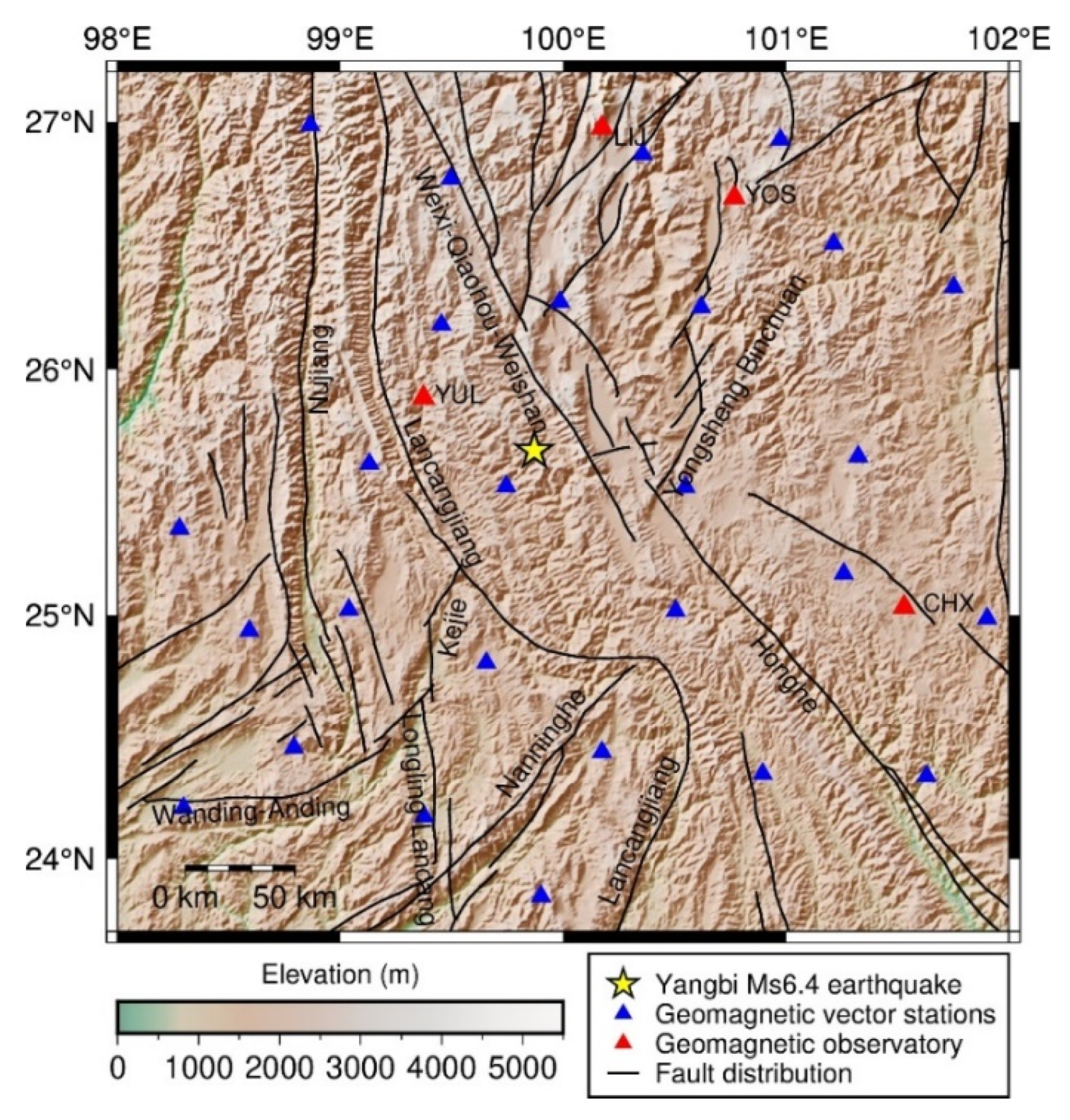
2. Data and Methods
2.1. Yangbi Ms 6.4 Earthquake
2.2. Inversion and Modeling Data
2.2.1. FMS Data
2.2.2. Geomagnetic Measurement Data
2.3. Inversion and Modeling Methods
2.3.1. TSF Inversion Method
2.3.2. LGF Modeling Method
3. Results
3.1. TSF Characteristics
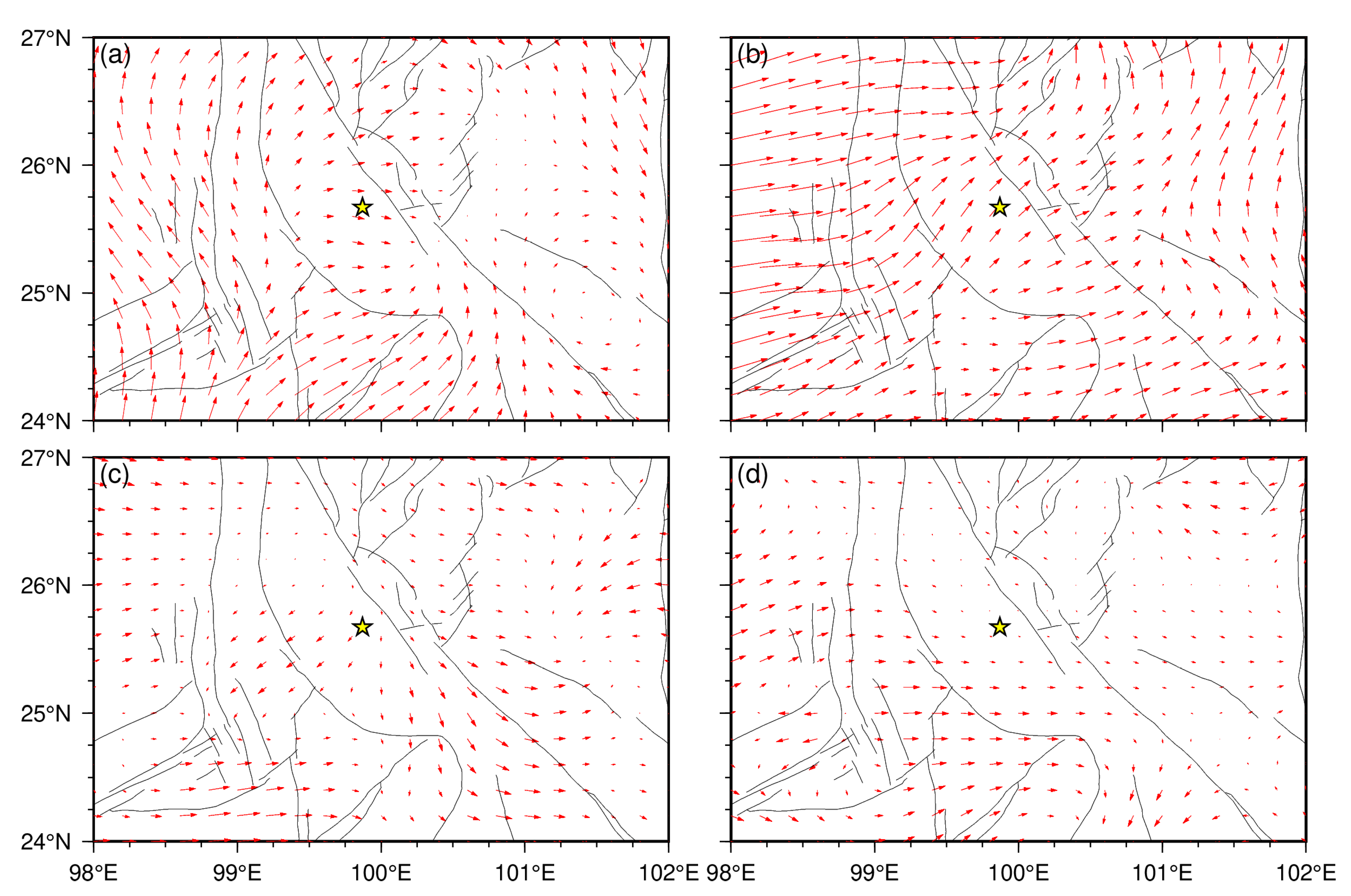
3.2. Variations in Regional LGF
4. Discussion
4.1. Analysis of Geomagnetic Field Changes Based on the Meta-Instability Theory
4.2. Seismomagnetic Mechanism
5. Conclusions
- (1)
- The azimuth of the maximum principal compressive stress axis of the TSF in the epicentral area was deflected nearly 180 degrees from before to after the Yangbi earthquake.
- (2)
- The epicenter was not located in the area with large values of annual anomalous variations in the geomagnetic field but near the area with zero variation and its adjacent area.
- (3)
- The area was found to have entered the meta-instability stage one year before the earthquake.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Mao, Z.; Chen, C.H.; Zhang, S.; Yisimayili, A.; Yu, H.; Yu, C.; Liu, J.Y. Locating Seismo-Conductivity Anomaly before the 2017 Mw 6.5 Jiuzhaigou Earthquake in China Using Far Magnetic Stations. Remote Sens. 2020, 12, 1777. [Google Scholar] [CrossRef]
- Sarlis, N.V. Statistical Significance of Earth’s Electric and Magnetic Field Variations Preceding Earthquakes in Greece and Japan Revisited. Entropy 2018, 20, 561. [Google Scholar] [CrossRef] [Green Version]
- Sasmal, S.; Chowdhury, S.; Kundu, S.; Politis, D.Z.; Potirakis, S.M.; Balasis, G.; Hayakawa, M.; Chakrabarti, S.K. Pre-Seismic Irregularities during the 2020 Samos (Greece) Earthquake (M = 6.9) as Investigated from Multi-Parameter Approach by Ground and Space-Based Techniques. Atmosphere 2021, 12, 1059. [Google Scholar] [CrossRef]
- Wang, Z.; Yuan, J.; Chen, B.; Chen, S.; Wang, C.; Mao, F. Local magnetic field changes during gas injection and extraction in an underground gas storage. Geophys. J. Int. 2019, 217, 271–279. [Google Scholar] [CrossRef]
- Lin, J.; Stein, R.S. Stress triggering in thrust and subduction earthquakes and stress interaction between the southern San Andreas and nearby thrust and strike-slip faults. J. Geophys. Res. Solid Earth 2004, 109, B02303. [Google Scholar] [CrossRef] [Green Version]
- Ellsworth, W.L.; Xu, Z.H. Determination of the stress tensor from focal mechanism data. EOS Trans. Am. Geophys. Union 1980, 61, 1117. [Google Scholar]
- Gephart, J.W.; Forsyth, D.W. An improved method for determining the regional stress tensor using earthquake focal mechanism data: Application to the San Fernando earthquake sequence. J. Geophys. Res. Solid Earth 1984, 89, 9305–9320. [Google Scholar] [CrossRef]
- Lund, B.; Townend, J. Calculating horizontal stress orientations with full or partial knowledge of the tectonic stress tensor. Geophys. J. Int. 2007, 170, 1328–1335. [Google Scholar] [CrossRef] [Green Version]
- Michael, A.J. Use of focal mechanisms to determine stress: A control study. J. Geophys. Res. 1987, 92, 357–368. [Google Scholar] [CrossRef]
- Sun, Y.; Zhao, X.; Huang, Y.; Yang, H.; Li, F. Characteristic of focal mechanisms and stress field of Yunnan area. Seismol. Geol. 2017, 39, 390–407. (In Chinese) [Google Scholar]
- Blakely, R.J. Geomagnetism and Paleomagnetism. Rev. Geophys. 1987, 25, 895–899. [Google Scholar] [CrossRef]
- Witze, A. Earth’s magnetic field is acting up and geologists don’t know why. Nature 2019, 565, 143–144. [Google Scholar] [CrossRef]
- Qiu, Y.; Wang, Z.; Jiang, W.; Zhang, B.; Li, F.; Guo, F. Combining CHAMP and Swarm Satellite Data to Invert the Lithospheric Magnetic Field in the Tibetan Plateau. Sensors 2017, 17, 238. [Google Scholar] [CrossRef] [Green Version]
- Thébault, E.; Purucker, M.; Whaler, K.A.; Langlais, B.; Sabaka, T.J. The Magnetic Field of the Earth’s Lithosphere. Space Sci. Rev. 2010, 155, 95–127. [Google Scholar] [CrossRef]
- Gao, Y.; Harris, J.M.; Wen, J.; Huang, Y.; Twardzik, C.; Chen, X.; Hu, H. Modeling of the coseismic electromagnetic fields observed during the 2004 Mw 6.0 Parkfield earthquake. Geophys. Res. Lett. 2016, 43, 620–627. [Google Scholar] [CrossRef] [Green Version]
- Liu, X.; Hattori, K.; Han, P.; Chen, H.; Chie, Y.; Zhao, X. Possible anomalous changes in solar quiet daily geomagnetic variation (Sq) related to the 2011 off the Pacific coast of Tohoku Earthquake (Mw 9.0). Pure Appl. Geophys. 2020, 177, 333–346. [Google Scholar] [CrossRef]
- Marchetti, D.; De Santis, A.; Campuzano, S.A.; Soldani, M.; Piscini, A.; Sabbagh, D.; Cianchini, G.; Perrone, L.; Orlando, M. Swarm Satellite Magnetic Field Data Analysis Prior to 2019 Mw = 7.1 Ridgecrest (California, USA) Earthquake. Geosciences 2020, 10, 502. [Google Scholar] [CrossRef]
- Johnston, M.J.S. Review of magnetic and electric field effects near active faults and volcanoes in the USA. Phys. Earth Planet. Inter. 1989, 57, 47–63. [Google Scholar] [CrossRef]
- McGarr, A.; Zoback, M.D.; Hanks, T.C. Implications of an elastic analysis of in situ stress measurements near the San Andreas fault. J. Geophys. Res. 1982, 87, 7797–7806. [Google Scholar] [CrossRef] [Green Version]
- Hao, J.; Hastie, L.M.; Stacey, F.D. Theory of the seismomagnetic effect a reassessment. Phys. Earth Planet. Inter. 1982, 28, 129–140. [Google Scholar] [CrossRef]
- Florio, G.; Fedi, M.; Rapolla, A.; Fountain, D.M.; Shive, P.N. Anisotropic magnetic susceptibility in the continental lower crust and its implications for the shape of magnetic anomalies. Geophys. Res. Lett. 1993, 20, 2623–2626. [Google Scholar] [CrossRef]
- Gorev, R.V.; Udalov, O.G. Micromagnetic simulation of the magnetoelastic effect in submicron structures. Phys. Solid State 2019, 61, 1563–1571. [Google Scholar] [CrossRef]
- Breiner, S. Piezomagnetic effect at the time of local earthquakes. Nature 1964, 202, 790–791. [Google Scholar] [CrossRef]
- Roskosz, M.; Rusin, A.; Bieniek, M. Analysis of relationships between residual magnetic field and residual stress. Meccanica 2013, 48, 45–55. [Google Scholar] [CrossRef] [Green Version]
- Wang, Z.; Chen, B.; Yuan, J.; Yang, F.; Jia, L.; Wang, C. Localized geomagnetic field anomalies in an underground gas storage. Phys. Earth Planet. Inter. 2018, 283, 92–97. [Google Scholar] [CrossRef]
- Chen, C.H.; Liu, J.Y.; Lin, P.Y.; Yen, H.Y.; Hattori, K.; Liang, W.T.; Chen, Y.I.; Yeh, Y.H.; Zeng, X. Pre-seismic geomagnetic anomaly and earthquake location. Tectonophysics 2010, 489, 240–247. [Google Scholar] [CrossRef]
- Han, P.; Zhuang, J.; Hattori, K.; Chen, C.-H.; Febriani, F.; Chen, H.; Yoshino, C.; Yoshida, S. Assessing the Potential Earthquake Precursory Information in ULF Magnetic Data Recorded in Kanto, Japan during 2000–2010: Distance and Magnitude Dependences. Entropy 2020, 22, 859. [Google Scholar] [CrossRef] [PubMed]
- Gilder, S.A.; LeGoff, M.; Peyronneau, J.; Chervin, J.C. Novel high pressure magnetic measurements with applications to magnetite. Geophys. Res. Lett. 2002, 29, 1392. [Google Scholar] [CrossRef] [Green Version]
- Nagata, T. Anisotropic magnetic susceptibility of rocks under mechanical stresses. Pure Appl. Geophys. 1970, 78, 110–122. [Google Scholar] [CrossRef]
- Stacey, F.D.; Johnston, M.J.S. Theory of the piezomagnetic effect in titanomagnetite-bearing. Pure Appl. Geophys. 1972, 97, 146–155. [Google Scholar] [CrossRef] [Green Version]
- Yamazaki, K. Temporal variations in magnetic signals generated by the piezomagnetic effect for dislocation sources in a uniform medium. Geophys. J. Int. 2016, 206, 130–141. [Google Scholar] [CrossRef]
- Zhan, Z. Investigations of tectonomagnetic phenomena in China. Phys. Earth Planet. Inter. 1989, 57, 11–22. [Google Scholar]
- Lei, X.L.; Wang, Z.W.; Ma, S.L.; He, C.R. A preliminary study on the characteristics and mechanism of the May 2021 MS6.4 Yangbi earthquake sequence, Yunnan, China. Acta Seismol. Sin. 2021, 43, 261–286. (In Chinese) [Google Scholar]
- Ma, J. On “whether earthquake precursors help for prediction do exist”. Chin. Sci. Bull. 2016, 61, 409–414. (In Chinese) [Google Scholar] [CrossRef] [Green Version]
- Long, F.; Qi, Y.; Yi, G.; Wu, W.; Wang, G.; Zhao, X.; Peng, G. Relocation of the Ms 6.4 Yangbi earthquake sequence on May 21, 2021 in Yunnan Province and its seismogenic structure analysis. Chin. J. Geophys. 2021, 64, 2631–2646. (In Chinese) [Google Scholar]
- Deng, Q.D.; Zhang, P.Z.; Ran, Y.K.; Yang, X.P.; Min, W.; Chen, L.C. Active tectonics and earthquake activities in China. Earth Sci. Front. 2003, 10, 66–73. (In Chinese) [Google Scholar]
- Dziewonski, A.M.; Chou, T.A.; Woodhouse, J.H. Determination of earthquake source parameters from waveform data for studies of global and regional seismicity. J. Geophys. Res. 1981, 86, 2825–2852. [Google Scholar] [CrossRef]
- Ekström, G.; Nettles, M.; Dziewonski, A.M. The global CMT project 2004-2010: Centroid-moment tensors for 13,017 earthquakes. Phys. Earth Planet. Inter. 2012, 200–201, 1–9. [Google Scholar] [CrossRef]
- Gu, Z.; Zhan, Z.; Gao, J.; Han, W.; An, Z.; Yao, T.; Chen, B. Geomagnetic survey and geomagnetic model research in China. Earth Planets Space 2006, 58, 741–750. [Google Scholar] [CrossRef] [Green Version]
- Menke, W. Nonlinear Inverse Problems. In Geophysical Data Analysis: Discrete Inverse Theory, 3rd ed.; Academic Press: San Diego, CA, USA, 2012; pp. 163–188. [Google Scholar]
- Hardebeck, J.L.; Michael, A.J. Damped regional-scale stress inversions: Methodology and examples for southern California and the Coalinga aftershock sequence. J. Geophys. Res. Solid Earth 2006, 111, B11310. [Google Scholar] [CrossRef]
- Martínez-Garzón, P.; Kwiatek, G.; Ickrath, M.; Bohnhoff, M. MSATSI: A MATLAB© package for stress inversion combining solid classic methodology, a new simplified user-handling and a visualization tool. Seismol. Res. Lett. 2014, 85, 896–904. [Google Scholar] [CrossRef] [Green Version]
- Gao, Q.; Cheng, D.; Wang, Y.; Li, S.; Wang, M.; Yue, L.; Zhao, J. Compensation method for diurnal variation in three-component magnetic survey. Appl. Sci. 2020, 10, 986. [Google Scholar] [CrossRef] [Green Version]
- Wang, M.; Shen, Z.K. Present-day crustal deformation of continental China derived from GPS and its tectonic implications. J. Geophys. Res. Solid Earth 2020, 125, e2019JB018774. [Google Scholar] [CrossRef] [Green Version]
- Xu, Y.; Koper, K.D.; Burlacu, R.; Herrmann, R.B.; Li, D.N. A New Uniform Moment Tensor Catalog for Yunnan, China, from January 2000 through December 2014. Seismol. Res. Lett. 2020, 91, 891–900. [Google Scholar] [CrossRef]
- Song, C.; Chen, Z.; Zhou, S.; Xu, Y.; Chen, B. Geomagnetic field change before and after 2021 Yangbi Ms 6.4 earthquake. Seismol. Geol. 2021, 43, 958–971. (In Chinese) [Google Scholar] [CrossRef]
- Wessel, P.; Luis, J.F.; Uieda, L.; Scharroo, R.; Wobbe, F.; Smith, W.H.F.; Tian, D. The Generic Mapping Tools version 6. Geochem. Geophys. Geosyst. 2019, 20, 5556–5564. [Google Scholar] [CrossRef] [Green Version]


| Earthquake Time | Epicenter Location | Magnitude | Fault-Plane I | Fault-Plane II | |||||
|---|---|---|---|---|---|---|---|---|---|
| Longitude (°) | Latitude (°) | Ms | Strike | Dip | Rake | Strike | Dip | Rake | |
| 2021.5.18 21:39 | 99.93 | 25.65 | 4.2 | 41 | 78 | 8 | 309 | 82 | 168 |
| 2021.5.19 20:05 | 99.91 | 25.65 | 4.4 | 229 | 90 | −15 | 319 | 75 | 180 |
| 2021.5.21 20:56 | 99.93 | 25.64 | 4.2 | 159 | 45 | −115 | 12 | 50 | −67 |
| 2021.5.21 21:21 | 99.93 | 25.65 | 5.6 | 217 | 86 | −12 | 308 | 78 | −176 |
| 2021.5.21 21:48 | 99.88 | 25.69 | 6.4 | 135 | 75 | −168 | 42 | 78 | −15 |
| 2021.5.21 21:56 | 99.95 | 25.64 | 4.9 | 220 | 84 | 20 | 128 | 70 | 174 |
| 2021.5.21 22.15 | 99.97 | 25.60 | 4.0 | 128 | 80 | 160 | 222 | 70 | 11 |
| 2021.5.21 22.21 | 99.98 | 25.60 | 5.2 | 146 | 48 | −161 | 43 | 76 | −44 |
| 2021.5.21 23:23 | 99.98 | 25.60 | 4.5 | 127 | 87 | 177 | 217 | 87 | 3 |
| 2021.5.22 00:51 | 99. 87 | 25.69 | 4.0 | 35 | 85 | −9 | 126 | 81 | −175 |
| 2021.5.22 09:48 | 99.88 | 25.67 | 4.0 | 313 | 84 | −176 | 223 | 86 | −6 |
| 2021.5.22 20:14 | 99.93 | 25.61 | 4.4 | 325 | 44 | −140 | 204 | 63 | −54 |
| 2021.5.27 19:52 | 99.95 | 25.74 | 4.1 | 194 | 90 | −5 | 284 | 85 | 180 |
| Location | σ1 | σ2 | σ3 | R Value | |||||
|---|---|---|---|---|---|---|---|---|---|
| Azimuth | Plunge | Azimuth | Plunge | Azimuth | Plunge | R Best | R Min | R Max | |
| (98° E, 25° N) | −159.2 | 10.3 | 56.2 | 77.4 | −67.9 | 7.1 | 0.45 | 0.00 | 0.72 |
| (99° E, 24° N) | −169.7 | 4.2 | 21.2 | 85.7 | −79.6 | 0.8 | 0.35 | 0.01 | 0.67 |
| (99° E, 25° N) | −170.2 | 4.9 | 21.7 | 85.0 | −80.1 | 1.0 | 0.34 | 0.01 | 0.69 |
| (99° E, 26° N) | −177.1 | 1.3 | −8.7 | 88.7 | 92.9 | 0.3 | 0.22 | 0.00 | 0.35 |
| (100° E, 25° N) | 1.0 | 2.5 | 169.0 | 87.4 | −89.1 | 0.5 | 0.21 | 0.01 | 0.38 |
| (100° E, 26° N) | −4.4 | 2.7 | −175.2 | 87.3 | 85.6 | 0.4 | 0.13 | 0.00 | 0.30 |
| (100° E, 27° N) | −8.0 | 21.7 | 174.1 | 68.3 | 82.3 | 0.7 | 0.07 | 0.00 | 0.23 |
| (101° E, 25° N) | 174.3 | 1.4 | 45.6 | 87.7 | −95.7 | 1.8 | 0.21 | 0.00 | 0.42 |
| (101° E, 26° N) | 170.5 | 6.2 | −16.8 | 83.7 | 80.4 | 0.8 | 0.24 | 0.01 | 0.49 |
| (101° E, 27° N) | −11.2 | 24.0 | 176.2 | 65.8 | 80.0 | 2.8 | 0.07 | 0.01 | 0.27 |
| (102° E, 26° N) | −10.6 | 1.7 | −128.5 | 86.4 | 79.5 | 3.1 | 0.31 | 0.01 | 0.53 |
| Components | Different Annual Variation Cycles | |||
|---|---|---|---|---|
| May 2018 to May 2019 | May 2019 to May 2020 | May 2020 to May 2021 | May–June 2021 | |
| Declination (′) | 0.86 | 0.91 | −0.20 | 0.07 |
| 0.18~0.96 | 0.65~1.31 | −0.67~0.51 | −0.37~0.78 | |
| Inclination (′) | 0.54 | −1.73 | 0.29 | −0.12 |
| −0.25~0.65 | −2.43~−1.03 | −0.26~0.67 | −0.18~0.27 | |
| Total intensity (nT) | 3.8 | −7.3 | −1.7 | −1.9 |
| 1.9~5.0 | −11.2~−4.7 | −7.6~1.1 | −3.0~1.5 | |
| North component (nT) | −1.7 | 10.1 | −3.9 | −0.4 |
| −2.4~4.7 | 4.3~14.2 | −5.8~−1.2 | −1.4~1.7 | |
| East component (nT) | 9.2 | 9.6 | −2.0 | 0.7 |
| 1.9~10.1 | 6.9~13.8 | −7.1~5.6 | −4.0~8.5 | |
| Horizontal intensity (nT) | −1.9 | 9.9 | −3.8 | −0.4 |
| −2.6~4.7 | 4.1~14.0 | −6.5~−0.5 | −1.5~1.8 | |
| Vertical intensity (nT) | 8.3 | −23.2 | 2.0 | −2.5 |
| −1.4~9.6 | −15.5~−33.1 | −7.9~7.0 | −3.9~3.6 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Z.; Ni, Z.; Chen, S.; Su, S.; Yuan, J. Characteristics of Local Geomagnetic Field Variations and the Tectonic Stress Field Adjacent to the 21 May 2021, Ms 6.4 Yangbi Earthquake, Yunnan, China. Appl. Sci. 2022, 12, 1005. https://doi.org/10.3390/app12031005
Wang Z, Ni Z, Chen S, Su S, Yuan J. Characteristics of Local Geomagnetic Field Variations and the Tectonic Stress Field Adjacent to the 21 May 2021, Ms 6.4 Yangbi Earthquake, Yunnan, China. Applied Sciences. 2022; 12(3):1005. https://doi.org/10.3390/app12031005
Chicago/Turabian StyleWang, Zhendong, Zhe Ni, Shuanggui Chen, Shupeng Su, and Jiehao Yuan. 2022. "Characteristics of Local Geomagnetic Field Variations and the Tectonic Stress Field Adjacent to the 21 May 2021, Ms 6.4 Yangbi Earthquake, Yunnan, China" Applied Sciences 12, no. 3: 1005. https://doi.org/10.3390/app12031005
APA StyleWang, Z., Ni, Z., Chen, S., Su, S., & Yuan, J. (2022). Characteristics of Local Geomagnetic Field Variations and the Tectonic Stress Field Adjacent to the 21 May 2021, Ms 6.4 Yangbi Earthquake, Yunnan, China. Applied Sciences, 12(3), 1005. https://doi.org/10.3390/app12031005






