Detection of Geomagnetic Signals as Precursors to Some Earthquakes in China
Abstract
:1. Introduction
2. Polarization Analysis
3. Polarization Results and Earthquakes
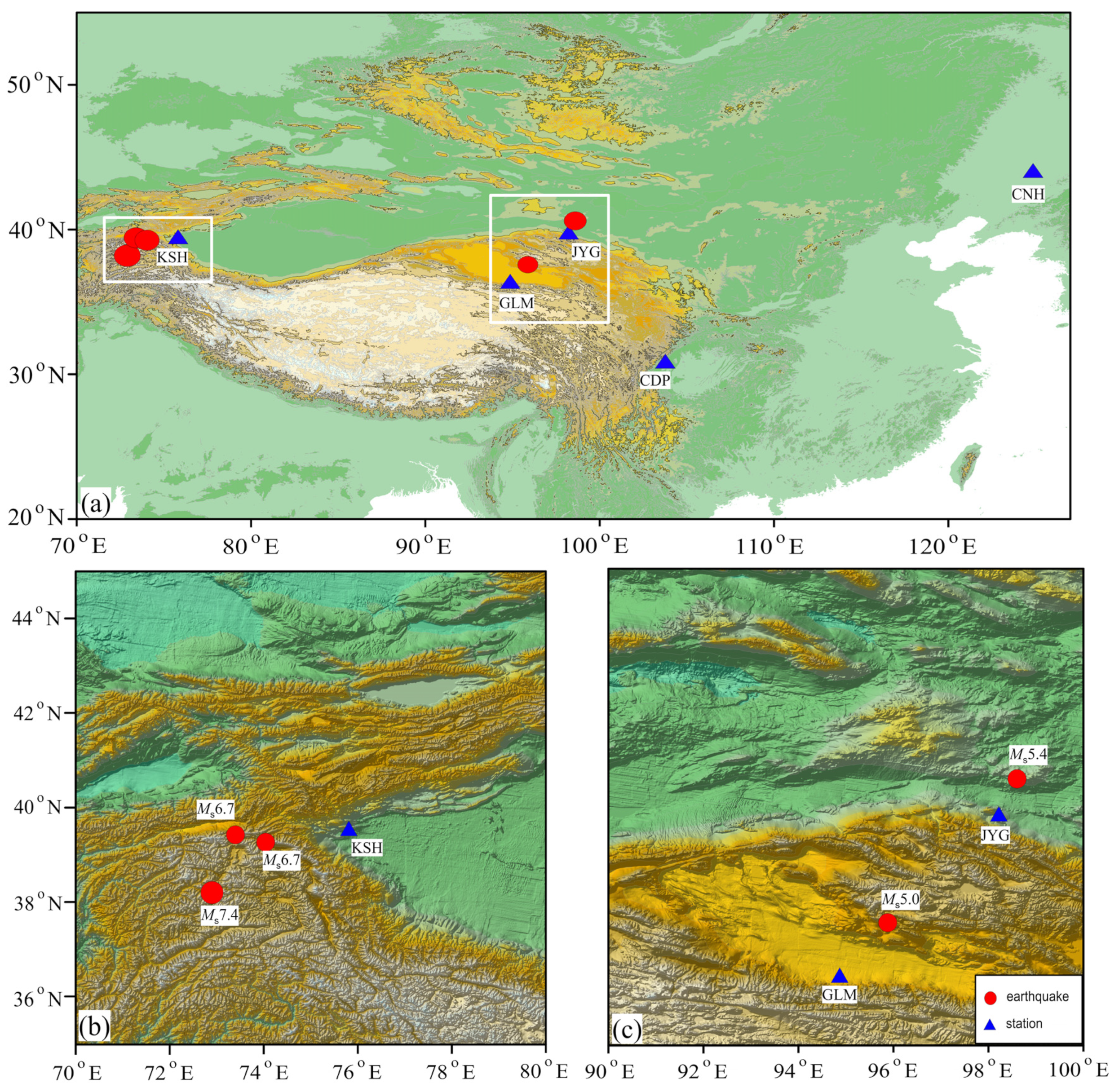
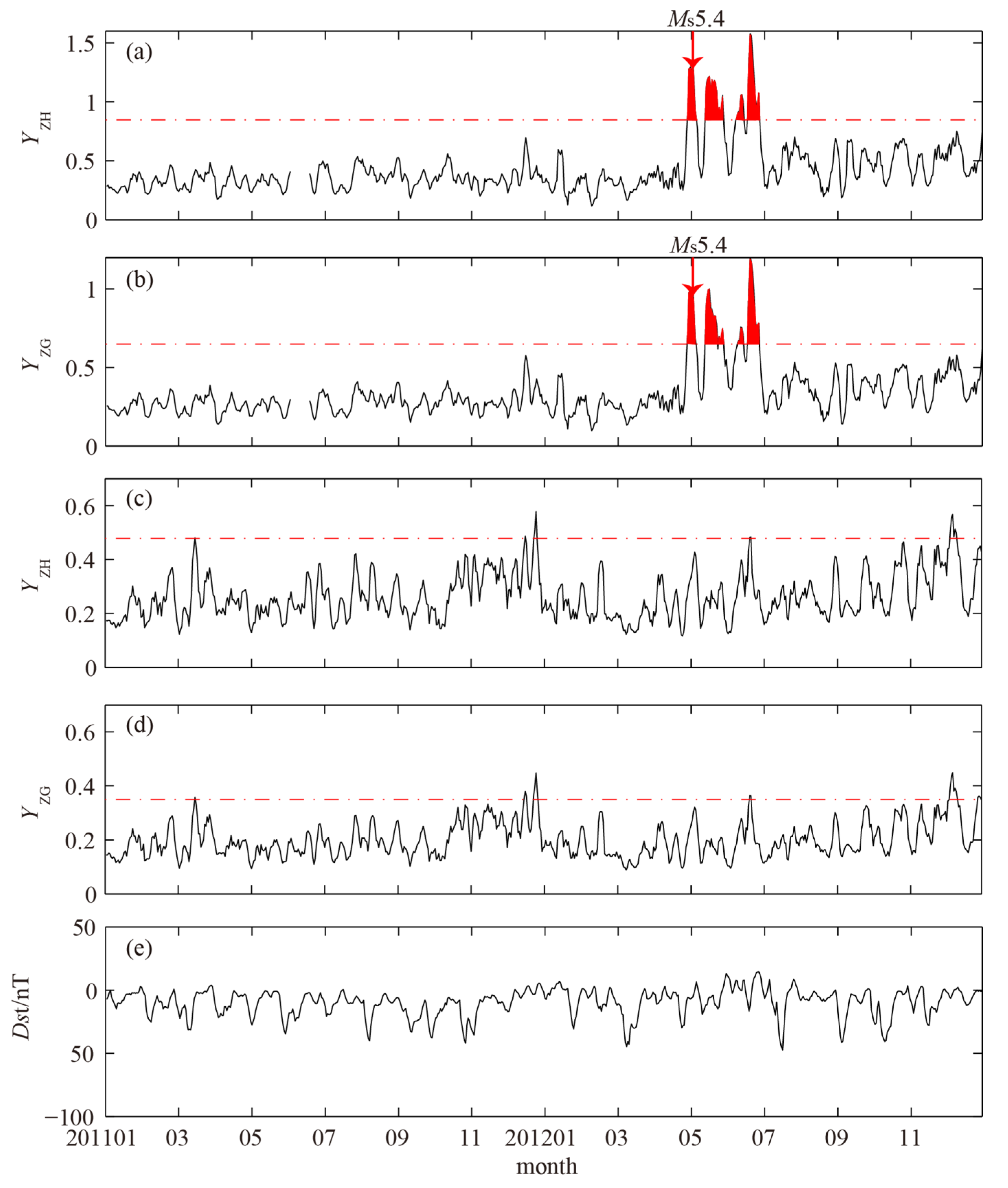
3.1. Polarization Results of the Jinta Earthquake
3.2. Polarization Results of Haixi Earthquake
3.3. Polarization Results of the KSH Station
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Nagao, T.; Enomoto, Y.; Fujinawa, Y.; Hata, M.; Hayakawa, M.; Huang, Q.; Izutsu, J.; Kushida, Y.; Maeda, K.; Oike, K.; et al. Electromagnetic anomalies associated with 1995 Kobe earthquake. J. Geodyn. 2002, 33, 401. [Google Scholar] [CrossRef]
- Akinaga, K.; Tanaka, H. Electric and magnetic phenomena observed before the volcano-seismic activity in 2000 in the Izu island region, Japan. Proc. Natl. Acad. Sci. USA 2002, 99, 7352–7355. [Google Scholar]
- Hattori, K. ULF geomagnetic changes associated with large earthquakes. Terr. Atmos. Ocean. Sci. 2004, 15, 329–360. [Google Scholar] [CrossRef] [Green Version]
- Hattori, K.; Serita, A.; Gotoh, K.; Yoshinoa, C.; Haradab, M.; Isezakid, N.; Hayakawa, M. ULF geomagnetic anomaly associated with 2000 Izu islands earthquakes swarm, Japan. Phys. Chem. Earth 2004, 29, 425–435. [Google Scholar] [CrossRef]
- Li, Q.; Zhu, P.Y.; Alimjian, M.; Xu, G.X. Detection of ULF electromagnetic emissions as a precursor to two earthquakes in China. Earthq. Sci. 2011, 24, 601–607. [Google Scholar] [CrossRef] [Green Version]
- Pride, S.R. Governing equations for the coupled electromagnetics and acoustics of porous-media. Phys. Rev. B 1994, 50, 15678–15696. [Google Scholar] [CrossRef]
- Merzer, M.; Klemperer, S.L. Modeling low-frequency magnetic-field precursors to the Loma Prietaearthquzke with a precursory increase in fault-zone conductivity. Pure Appl. Geophys. 1997, 150, 217–248. [Google Scholar] [CrossRef]
- Hunt, A.G. Comment on “Modeling low-frequency magnetic-field precursors to the Loma Prietaearthquzke with a precursory increase in fault-zone conductivity” by M. Merzer and S. L. Klemperer. Pure Appl. Geophys. 2005, 162, 2573–2575. [Google Scholar] [CrossRef]
- Ren, H.X.; Wen, J.; Huang, Q.H.; Chen, X.F. Electrokinetic effect combined with surface-charge assumption: A possible generation mechanism of coseismic EM signals. Geophys. J. Int. 2015, 200, 835–848. [Google Scholar] [CrossRef] [Green Version]
- Thomas, J.N.; Love, J.J.; Johnston, M.J.S.; Yumoto, K. On the reported magnetic precursor of the 1993 Guam earthquake. Geophys. Res. Lett. 2009, 36, L16301. [Google Scholar]
- Masci, F. On the seismogenic increase of the ratio of the ULF geomagnetic field components. Phys. Earth Planet. Inter. 2011, 187, 19–32. [Google Scholar] [CrossRef] [Green Version]
- Hayakawa, M.; Kawate, R.; Molchanov, O.A.; Yumoto, K. Results of ultra-low-frequency magnetic field measurements during the Guam earthquake of 8 August 1993. Geophys. Res. Lett. 1996, 23, 241–244. [Google Scholar] [CrossRef]
- Gladychev, V.; Baransky, L.; Schekotov, A. Study of electromagnetic emissions associated with seismic activity in Kamchatka region. Hazards Earth Syst. Sci. 2001, 1, 127–136. [Google Scholar] [CrossRef] [Green Version]
- Molchanov, O.; Schekotov, A.; Fedorov, E.; Belyaev, G.; Gordeev, E. Preseismic ULF electromagnetic effect from observation at Kamchatka. Nat. Hazards Earth Syst. Sci. 2003, 3, 203–209. [Google Scholar] [CrossRef]
- Kopytenko, Y.; Ismagilov, V.; Hayakawa, M.; Smirnova, N.; Troyan, V.; Peterson, T. Investigation of the ULFelectromagnetic phenomena related toearthquakes: Contemporary achievementsand the perspectives. Ann. Geophys. 2009, 44, 325–334. [Google Scholar] [CrossRef]
- Prattes, G.; Schwingenschuh, K.; Eichelberger, H.U.; Magnes, W.; Boudjada, M.; Stachel, M.; Vellante, M.; Wesztergom, V.; Nenovski, P. Multi-point ground-based ULF magnetic field observations in Europe during seismic active periods in 2004 and 2005. Nat. Hazards Earth Syst. Sci. 2008, 8, 501–507. [Google Scholar] [CrossRef] [Green Version]
- Rawat, G.; Chauhan, V.; Dhamodharan, S. Fractal dimension variability in ULF magnetic field with reference to local earthquake at MPGO, Ghuttu. Geomat. Nat. Hazards Risk 2016, 7, 1937–1947. [Google Scholar] [CrossRef] [Green Version]
- Dewi, C.N.; Febriani, F.; Anggono, T.; Syuhada; Soedjatmiko, B.; Prasetio, A.D.; Ahadi, S. The ULF geomagnetic anomalous signal associated with Nias earthquake M 5.3 North Sumatra Indonesia on 6 September 2018. J. Phys. Conf. Ser. 2020, 1568, 012027. [Google Scholar] [CrossRef]
- Yusof, K.A.; Abdul, H.N.S.; Abdullah, M.; Ahadi, S.; Yoshikawa, A. Assessment of signal processing methods for geomagnetic precursor of the 2012 M6.9 Visayas, Philippines earthquake. Acta Geophys. 2019, 67, 1297–1306. [Google Scholar] [CrossRef]
- Ida, Y.; Yang, D.; Li, Q.; Sun, H.; Hayakawa, M. Detection of ULF electromagnetic emissions as a precursor to an earthquake in China with an improved polarization analysis. Nat. Hazards Earth Syst. Sci. 2008, 8, 775–777. [Google Scholar] [CrossRef]
- Yusof, K.A.; Abdullah, M.; Hamid, N.S.A.; Ahadi, S.; Yoshikawa, A. Correlations between Earthquake Properties and Characteristics of Possible ULF Geomagnetic Precursor over Multiple Earthquakes. Universe 2021, 7, 20. [Google Scholar] [CrossRef]
- Haines, C.; Owens, M.J.; Barnard, L.; Lockwood, M.; Ruffenach, A. The Variation of Geomagnetic Storm Duration with Intensity. Sol. Phys. 2021, 29, 154. [Google Scholar] [CrossRef] [Green Version]
- Heyns, M.J.; Lotz, S.I.; Gaunt, C.T. Geomagnetic Pulsations Driving Geomagnetically Induced Currents. Space Weather 2021, 19, 1–42. [Google Scholar] [CrossRef]
- Kotsarenko, A.; Enríquez, R.P.; Cruz-Abeyro, J.A.L.; Koshevaya, S.; Grimalsky, V.; Zúñiga, F.R. Analysis of the ULF electromagnetic emission related to seismic activity, Teoloyucan geomagnetic station, 1998–2001. Nat. Hazards Earth Syst. Sci. 2004, 4, 679–684. [Google Scholar] [CrossRef] [Green Version]
- Yao, X.Y.; Yang, D.M.; Chen, H.R.; He, Y.F.; Gao, D.P. Analysis on temporal-spatial distribution of the short-period geomagnetic activities. Chin. J. Geophys. 2012, 55, 2660–2668. [Google Scholar] [CrossRef]
- Hayakawa, M.; Itoh, T.; Hattori, K.; Yumoto, K. ULF electromagnetic precursors for an earthquake at Biak, Indonesia on 17 February 1996. Geophys. Res. Lett. 2000, 27, 1531–1534. [Google Scholar] [CrossRef]
- Hattori, K.; Han, P.; Yoshino, C.; Febriani, F.; Yamaguchi, H.; Chen, C.H. Investigation of ULF seismo-magnetic phenomena in Kanto, Japan during 2000-2010: Case studies and statistical studies. Surv. Geophys. 2013, 34, 293–316. [Google Scholar] [CrossRef]
- Surkov, V.V.; Molchanov, O.A.; Hayakawa, M. Pre-earthquake ULF electromagnetic perturbations as a result of inductive seismomagnetic phenomena during microfracturing. J. Atmos. Sol.-Terr. Phys. 2003, 65, 31–46. [Google Scholar] [CrossRef]
- Dey, C.; Baruah, S.; Rawat, G.; Chetia, T.; Baruah, S.; Sharma, S. Appraisal of contemporaneous application of polarization ratio and fractal analysis for studying possible seismo-electromagnetic emissions during an intense phase of seismicity in and around Assam Valley and the Eastern Himalayas, India. Phys. Earth Planet. Inter. 2021, 318, 106759. [Google Scholar] [CrossRef]
- Hayakawa, M.; Hattori, K.; Ohta, K. Monitoring of ULF (ultra-low-frequency) Geomagnetic Variations Associated with Earthquakes. Sensors 2007, 7, 1108–1122. [Google Scholar] [CrossRef] [Green Version]
- Robert, J.J.; Duba, A.G.; Mathez, E.A.; Shankland, T.J.; Kinzler, R. Carbon-enhanced Electrical Conductivity During Fracture of Rocks. J. Geophys. Res. 1999, 104, 737–747. [Google Scholar] [CrossRef]




| Date | Lon/o | Lat/o | Epicenter | Magnitude/Ms | Depth/km | Station | Distance/km |
|---|---|---|---|---|---|---|---|
| 18 June 2008 | 91.20 | 35.30 | Zhiduo | 5.4 | 10 | GLM | 360 |
| 13 October 2008 | 73.90 | 39.50 | Tajikistan | 5.3 | 8 | KSH | 164 |
| 10 November 2008 | 95.90 | 37.60 | Haixi | 6.3 | 10 | GLM | 140 |
| 20 February 2009 | 78.60 | 40.80 | Aheqi | 5.2 | 13 | KSH | 249 |
| 19 April 2009 | 78.30 | 41.30 | Aheqi | 5.4 | 30 | KSH | 290 |
| 22 April 2009 | 77.30 | 40.10 | Artush | 5.0 | 25 | KSH | 140 |
| 28 August 2009 | 95.90 | 37.60 | Haixi | 6.6 | 10 | GLM | 160 |
| 7 September 2010 | 73.90 | 39.50 | Uqia | 5.5 | 30 | KSH | 300 |
| 11 August 2011 | 77.20 | 39.90 | Artush | 5.8 | 10 | KSH | 127 |
| 1 December 2011 | 77.00 | 38.40 | Shache | 5.0 | 30 | KSH | 160 |
| 9 March 2012 | 81.40 | 39.50 | Luopu | 5.9 | 30 | KSH | 480 |
| 3 May 2012 | 98.60 | 40.60 | Jinta | 5.4 | 8 | JYG | 100 |
| 31 May 2012 | 78.82 | 43.52 | Kazakhstan | 5.9 | 8 | KSH | 520 |
| 1 June 2012 | 75.10 | 39.90 | Uqia | 5.0 | 7 | KSH | 75 |
| 11 August 2012 | 78.20 | 40.00 | Artush | 5.2 | 9 | KSH | 210 |
| 29 January 2013 | 79.70 | 42.60 | Kazakhstan | 6.1 | 20 | KSH | 480 |
| 30 January 2013 | 94.70 | 32.90 | Zaduo | 5.1 | 20 | GLM | 390 |
| 12 February 2013 | 92.40 | 38.50 | Haixi | 5.1 | 10 | GLM | 320 |
| 11 March 2013 | 77.50 | 40.20 | Artush | 5.2 | 8 | KSH | 165 |
| 5 June 2013 | 95.88 | 37.58 | Haixi | 5.0 | 4 | GLM | 155 |
| 30 June 2013 | 73.41 | 39.22 | Tajikistan | 5.0 | 10 | KSH | 209 |
| 23 November 2013 | 75.71 | 42.53 | Kazakhstan | 5.2 | 9 | KSH | 340 |
| 21 October 2013 | 76.90 | 35.30 | Kashmir | 5.5 | 30 | KSH | 470 |
| 23 November 2013 | 75.71 | 42.53 | Kyrgyzstan | 5.2 | 9 | KSH | 340 |
| 1 December 2013 | 78.98 | 40.26 | Keping | 5.3 | 9 | KSH | 280 |
| 9 July 2014 | 78.27 | 39.31 | Maigaiti | 5.1 | 8 | KSH | 220 |
| 14 November 2014 | 77.27 | 42.16 | Kyrgyzstan | 5.7 | 10 | KSH | 330 |
| 10 January 2015 | 77.27 | 40.21 | Artush | 5.0 | 10 | KSH | 150 |
| 3 July 2015 | 78.20 | 37.60 | Pishan | 6.5 | 10 | KSH | 300 |
| 18 November 2015 | 73.04 | 40.38 | Kyrgyzstan | 6.0 | 7 | KSH | 256 |
| 7 December 2015 | 72.90 | 38.20 | Tajikistan | 7.4 | 30 | KSH | 291 |
| 26 June 2016 | 73.40 | 39.43 | Kyrgyzstan | 6.7 | 10 | KSH | 207 |
| 17 October 2016 | 94.93 | 32.81 | Zaduo | 6.2 | 9 | GLM | 400 |
| 25 November 2016 | 74.04 | 39.27 | Aketao | 6.7 | 10 | KSH | 155 |
| 3 May 2017 | 71.58 | 39.42 | Tajikistan | 6.2 | 30 | KSH | 380 |
| 5 May 2017 | 71.50 | 39.45 | Tajikistan | 6.0 | 20 | KSH | 380 |
| 11 May 2017 | 75.25 | 37.58 | Taxkorgan | 5.5 | 8 | KSH | 220 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yao, X.; Wang, W.; Teng, Y. Detection of Geomagnetic Signals as Precursors to Some Earthquakes in China. Appl. Sci. 2022, 12, 1680. https://doi.org/10.3390/app12031680
Yao X, Wang W, Teng Y. Detection of Geomagnetic Signals as Precursors to Some Earthquakes in China. Applied Sciences. 2022; 12(3):1680. https://doi.org/10.3390/app12031680
Chicago/Turabian StyleYao, Xiuyi, Wanqing Wang, and Yuntian Teng. 2022. "Detection of Geomagnetic Signals as Precursors to Some Earthquakes in China" Applied Sciences 12, no. 3: 1680. https://doi.org/10.3390/app12031680
APA StyleYao, X., Wang, W., & Teng, Y. (2022). Detection of Geomagnetic Signals as Precursors to Some Earthquakes in China. Applied Sciences, 12(3), 1680. https://doi.org/10.3390/app12031680





