1. Introduction
About 4000 people died in 2019 in the European Union as a direct result of moped and motorcycle crashes, accounting for 18% of the total motor vehicle fatalities [
1]. Motorcyclists have an increased risk of injury in case of collision, which is particularly relevant in the case of head injuries [
2]. The use of helmets is the most effective way of preventing motorcyclists’ head injuries [
3], and improving the impact performance of helmets leads to reduce the risk of head injury and fatalities. Most helmets are developed and designed according to the requirements prescribed in the relevant helmet standards. There are numerous motorcycle helmet safety standards around the world: ECE-22.05/06 in Europe [
4,
5], DOT and Snell in USA [
6,
7], and JIS-T in Japan [
8] are among some of them. The objective of a motorcycle helmet standard is to ensure a minimum level of head protection under some specific test conditions. However, methods and requirements vary from one standard to another and, therefore, the performance against impact of motorcyclist helmets is influenced by the requirements included in each standard [
9,
10].
One of these requirements, which has been controversial over the last decades, is the need of a penetration test. The penetration test measures the resistance of the helmet shell to impacts against sharp objects. In these tests, the helmet is positioned on a headform or a spherical device support. Then, a conical striker is dropped to hit the outer surface of the static helmet shell. The required performance criterion consists of ensuring that there is no contact between the striker tip and the headform or spherical support.
Over the years, some research has pointed out that the penetration test was either not necessary or that it could negatively influence helmet performance in more common real-life crash scenarios. In a statistical study, Otte et al. found that the frequency of motorcycle accidents involving penetrating objects was extremely small [
11]. Shuaeib et al. stated that the penetration test is the main parameter that would determine the thickness of the helmet shell, leading to a thicker shell that would account for about 50% of the weight of the helmet [
12]. Furthermore, some researchers stated that the penetration test causes helmets to be designed with a stiffer shell that could result in an increased risk of head injury in impacts against rigid flat surfaces [
13,
14]. These concerns resulted in the elimination of the penetration tests from some standards, while others continue demanding this requirement. In Europe, a penetration test is not required in the current regulation ECE-22.05/06 [
4,
5], while several other standards and regulations do require this procedure as part of the helmet assessment program [
6,
7,
8,
15].
However, the link between increased helmet shell stiffness and a higher acceleration headform response in case of impact has been addressed on the basis of simplified models of the helmet behavior that, for instance, do not take into account the effects on the helmet behavior of different impact velocities [
16] and other contributing factors to impact energy management such as the role of the shell in producing a proper load distribution over a greater liner area [
17] and the variation of protective padding density at different helmet locations [
18,
19]. The aim of this study was to empirically demonstrate if the inclusion of a penetration test in motorcyclist helmet testing standards results in an increased risk of head injury for the motorcyclists in a set of commercially available helmets. More specifically, the goal of this paper was to assess the influence of the shell resistance to the penetration test on the impact performance of helmets at two different impact velocities.
2. Materials and Methods
The experimental method was designed to study if the shell stiffness assessed by the penetration test influences the impact performance of motorcycle helmets. First, 20 full-face motorcycle helmet models were exposed to a penetration test. Then, on the basis of the observed results from the penetration tests, we classified four helmet models as hard shell helmets and six as soft shell helmets. The 10 remaining helmet models were unclassified and then not further considered in the study. Only the 10 classified helmet models were selected to be drop tested at two different velocities. A new helmet sample was used for each velocity, and therefore 20 helmets were drop tested. Thus, a total of 40 helmet samples were used in this study.
All the helmets were composed of composite shell and the protective padding was made of expanded polystyrene (EPS). The retention system of the helmets was based on the double D-ring buckle. All the helmets complied with the European regulation [
4]. The tests were performed at the Impact Laboratory of the University of Zaragoza.
2.1. Penetration Test
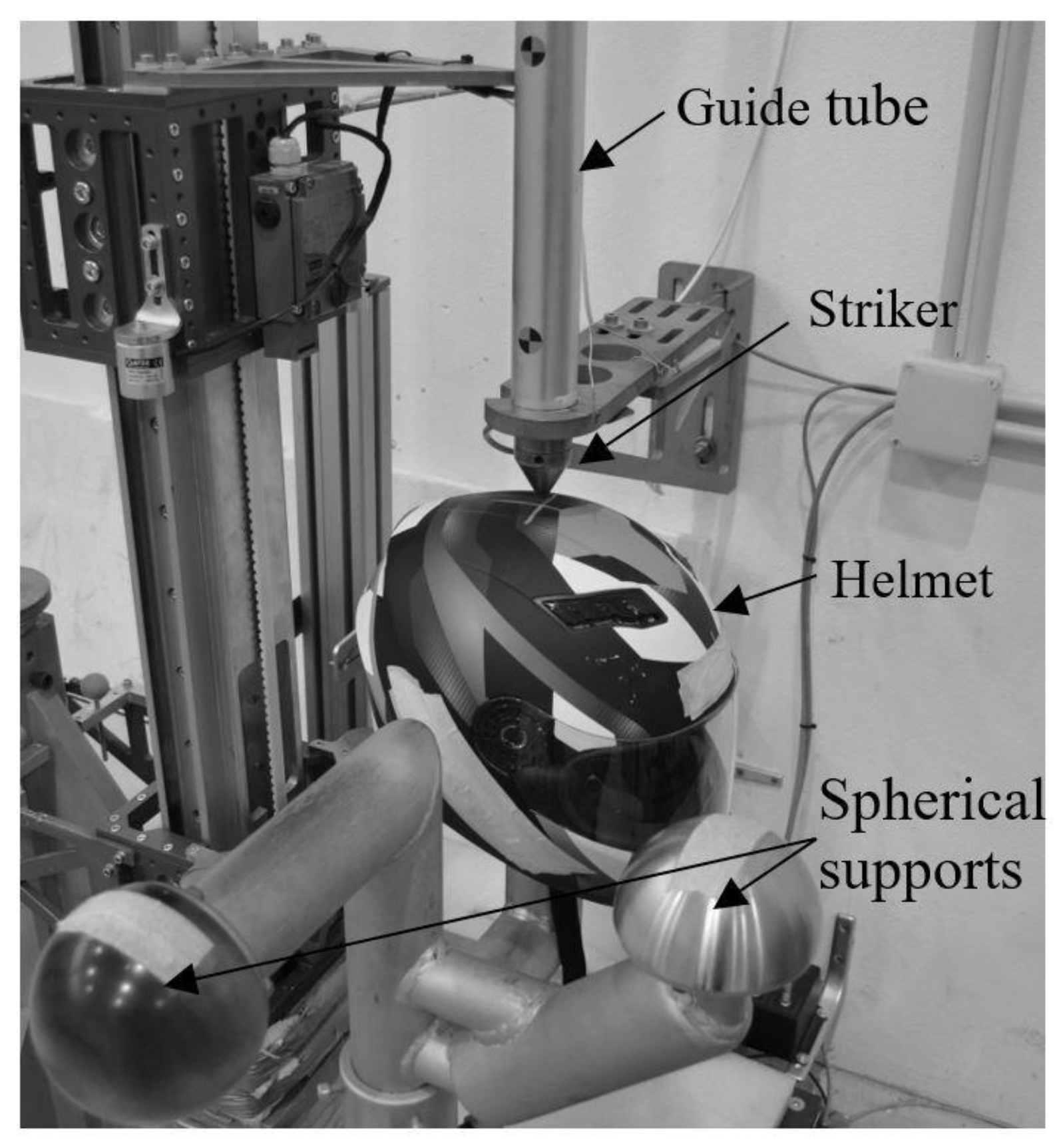
A conventional penetration test was conducted on one sample of each helmet model (see
Figure 1). The striker mass was 3 kg with a 60° conical head, and it was dropped from a height of 2 m above the surface of the helmet shell [
15]. Between 2 and 4 points were randomly tested on each sample. Typical impacted areas were the front, top, lateral, and rear of the helmet shell on or above the test line, as defined by Snell [
7]. Impacts on vent openings were not performed. The locations for the impact points as well as the order in which they were tested were randomly selected for each helmet as prescribed in the test procedure [
6,
7,
8,
15]. The intrusion of the conical tip of the striker into the helmet was measured after each impact. Then, the average and the standard deviation values of the intrusion measurements were calculated for each helmet and used as an indicator of the shell stiffness to classify the helmets. Only helmets with an average intrusion higher than 15 mm (soft shell helmets) and lower than 10 mm (hard shell helmets) were selected for the impact performance comparison and were exposed to the drop test. The rest of the helmets that resulted in intermediate values of intrusion were not further considered in the study.
2.2. Impact Absorption Tests (Drop Tests)
The test matrix consisted of 60 impacts onto a flat anvil. After the selection process based on the penetration test results, a new sample of each selected helmet model was drop tested at 5 m/s and another sample at 8.2 m/s. Each helmet was tested on the front, lateral, and top areas (three impacts per helmet sample at each impact speed). The selected impact areas corresponded with the points B, X (right), and P, as described in the European regulation [
5] and shown in
Figure 2.
A free fall guided impact machine (Model: Quebrantahuesos 6.0, +D, Pozuelo de Alarcón, Spain) was used for the impact absorption tests (see
Figure 3). As the helmets tested were not of the same size, three metallic headform sizes were used (Model: 100_04_FMH, Cadex Inc., Saint-Jean-sur-Richelieu, QC, Canada) to ensure an appropriate fitting of the headform for each helmet size, as prescribed in the regulation [
4,
5]. Four helmet types were tested with the 535 mm headform circumference, three with the 575 mm headform, and three with the 605 mm headform [
20]. The corresponding headform masses were 4.1 kg, 4.7 kg, and 5.6 kg respectively. The headforms were positioned inside the helmets according to the requirements of Annex 5 of ECE-22.06 [
5], and the retention system was adjusted under the chin of the headforms and tightened to a tension of 75 N [
15]. Before each impact, the headform was re-positioned, and the retention system re-tensioned.
A wireless system (Model: iCONO, +D, Pozuelo de Alarcón, Spain) was used to measure the linear acceleration at the center of gravity of the headforms. The wireless system incorporates three orthogonal uniaxial accelerometers (Model: 64C-2000, MEAS, Nanshan District Shenzhen, China) and an acquisition system (Model: SLICE NANO, DTS, Seal Beach, CA, USA). Data were recorded at 10 kHz, filtered using a low-pass filter CFC-1000, and post-processed using a validated and developed in-house script of Matlab (Matlab R2013b, MathWorks, Natick, MA, USA).
2.3. Statistical Hypothesis Testing
The objective of the main statistical hypothesis testing was to assess the influence of the shell stiffness on the impact performance of the helmets against head injuries. For that reason, the helmet models were classified into two groups (soft and hard shell groups) depending on the result of the penetration test. As aforementioned, 10 out of the 20 penetration tested helmets were selected for the impact performance comparison. Within the selected group, four helmet models were grouped into the hard shell group, while the remaining six helmet models were included in the soft shell group. Both groups had helmets of three different sizes. The hard shell group was composed of two helmets that were tested with the 535 headform, one with the 575 and one with the 605. The soft shell group was composed of two helmets tested with the 535 headform, two with the 575, and two with the 605.
The peak resultant linear acceleration (PLA) and the head injury criterion (HIC) measured at the center of gravity of the headform were the selected metrics to determine the impact performance of the helmets because they are the usual parameters included in helmet standards to assess head protection [
5].
Since three different headform sizes were used in this study, a preliminary statistical hypothesis testing was carried out to rule out any possible influence of the headform size on the PLA or HIC variables. A non-parametric Kruskal–Wallis H test with a significance level of 0.05 was performed to analyze whether the size of the headform (three different sizes) was significantly related to the values of either PLA or HIC. The Kruskal–Wallis test is an extension of the two sample hypotheses testing to more than two independent samples and it replaces the ANOVA test when sample sizes are small. The results of this analysis are included in the
Appendix A.
After ensuring the independence of the PLA and HIC variables from the helmet size, we carried out the main statistical analysis for the comparison of the impact performance between the two shell groups. A non-parametric test, the Mann–Whitney U test for independent samples with a significance level of 0.05, was used for this analysis due to the limited sample size. Statistical analyses were performed using the Real Statistics Resource Pack add-in in Excel (Excel 2016, Microsoft, Redmond, WA, USA).
4. Discussion
The objective of this study was to provide insight into the effects of including a penetration test, which is the main driver that determines helmet shell thickness and therefore of its stiffness, in order to improve the protective performance of helmets. To that end, the impact performance of 10 helmet models, which were sorted into either hard or soft shell groups, were compared at two impact speeds. PLA and HIC variables were selected to determine the protection capability of the helmets.
Since three headform sizes were used in the impact absorption tests, the influence of the headform size on the PLA or HIC variables was analyzed prior to carry out the main statistical analysis of this study. In
Appendix A,
Table A1 and
Table A2 include the PLA and HIC mean and the SD for each headform group, together with the
p-value for each impact point tested at 5 m/s and 8.2 m/s, respectively. The preliminary statistical hypothesis testing could not find any significant influence of the headform size on the PLA or HIC values at neither of the tested speeds. This result was expected because normally, the requirements of the helmet standards are the same for all headform sizes and therefore helmet manufacturers individually adjust the performance of each helmet size.
Regarding the impact performance comparison, the main statistical hypothesis testing showed no significant differences between the hard shell group and the soft shell group on the results of the impact absorption tests at 5 m/s. Similar results were observed in the tests at 8.2 m/s, except for the impacts on the helmet P point, which, showing contrary results to what had been suggested in previous research [
13,
14], resulted in significantly higher PLA for the soft shell group (
p-value = 0.038), even if the HIC value was not significantly different (
p-value = 0.352). These findings seem to be contradictory with the statement that including a penetration test in regulations causes helmets to be designed with a stiffer shell that behave very rigidly when striking flat surfaces [
13,
14]. While the above statement is correct for helmets in which only the shell thickness or helmet stiffness is increased [
21], it does not hold for actual helmets in which both the shell and the protective padding can be varied jointly. Indeed, the impact performance of a motorcycle helmet depends both on the material and dimensions of the shell and on the characteristics of the protective padding or liner, and then there is a combination of the characteristics of the shell and liner that makes it possible to improve the helmet impact performance [
22]. During an impact, a stiffer shell distributes the impact load over a greater area of the helmet, reducing the crushed volume of the liner and, therefore, decreasing the energy absorption, which may result in an increase of the linear acceleration. However, this effect can be compensated using a lower density of protective padding as long as its thickness is enough to prevent a bottom out effect. This practice is very common in current helmet design to compensate shell stiffness caused by shell geometry. For example, the higher shell stiffness due to the concavity form of the top part of full-face helmets is compensated with lower density or grooved shape liner at the top part [
23]. This attempts to make the helmet impact response site-independent; however, other limitations such as liner thickness, especially at the side of the helmet, makes this point site-dependent because higher liner densities must be used at this location in order to prevent a bottom out effect of a liner. The site-dependent impact response could explain the contradictory results observed in the X point impacts (side impact) of this study. Therefore, a stiffer shell does not necessarily mean that the helmet will exhibit a global stiff mechanical behavior, but that the characteristics of the liner will be chosen to balance the effects of the stiffness of the shell, which depends on the material, thickness, and external geometry. Therefore, if a helmet stiffness increase caused by a stiffer shell can be compensated with the characteristics of the liner, the next question is: which type of stiff shell or soft shell improves the protection capabilities of the helmets?
Although no general trend was observed in the results of this study to provide a convincing answer to the above question, some particular results such as the significantly higher PLA for the soft shell group for the point P at 8.2 m/s and the extremely high acceleration peak in the impact on the B point of one helmet within the soft shell group also at 8.2 m/s suggest that hard shell helmets would provide better protection at higher impact speeds. These results are in line with a simulation study that stated that the impact speed is an important parameter in helmet design and concluded that for high impact speeds, the helmet should be designed with a stiffer shell and denser protective padding than for low speeds [
16]. Furthermore, the importance of the impact speed in helmet impact performance can also be appreciated by comparing the impact absorption test results between both impact speeds for each impact location. If the PLA and HIC values within the hard shell group were lower at 5 m/s for the X point than the values of the soft shell group, then the results of the hard shell group were higher at 8.2 m/s for the X point and vice versa for the B and P points. These results also highlight that shell stiffness has an important influence in the overall dynamic performance of the helmets. While helmets with stiffer shells tend to absorb energy by liner deformation from the inside, where the load distribution is determined by the compatibility of the liner dimensions and headform shape, helmets with softer shells tend to absorb energy predominantly from the outside, where the load distribution is determined by the geometry of the object hit. As a result of the higher load distribution capacity of helmets with stiffer shells, helmets with softer shells tend to bottom out sooner compared to helmets with stiffer shells [
17]. In addition, hard shell helmets would provide better protection when striking objects with a greater variety of shapes, especially during concentrated impacts on small or sharp objects [
24].
The results of our study suggest that hard shell helmets, even if they can be strongly influenced by the penetration test, would provide better protection at higher impact speeds without harming the helmet performance at lower impact speeds. In addition, another effect of the penetration test is the control of the size of the vent openings of the helmet, which could result in a decrease load distribution capacity on those areas if the size of the openings was large enough. However, the energy of the penetration test must be chosen carefully because high energy penetration tests could lead composite shells to do not delaminate for impacts into real-life crash scenarios [
25], and delamination is an additional energy absorbing mechanism of composite shells that improves helmet impact performance [
26]. On the negative side, hard shell helmets result in heavier helmets that may negatively impact rider’s comfort. In this study, the hard shell helmets were around 200 g heaviest when compared with soft shell helmets of the same size.
A potential limitation of this study is the focus only on linear injury metrics (PLA and HIC) to assess the protection performance of the helmets. It is well known that these metrics do not consider the rotational kinematics of the head, which are proposed as the main mechanism of brain diffuse injuries [
27]. In this regard, the project COST 327 carried out oblique tests at different impact speeds with two almost identical helmets that differed only in mass and shell stiffness, concluding that neither the helmet mass nor the shell stiffness seems to significantly affect the rotational accelerations and tangential forces in oblique impacts with composite shell helmets [
28]. In addition, although rotational kinematics are being included in several recently proposed testing programs and only in oblique impacts [
5,
15], most existing mandatory helmet regulations only consider linear injury metrics to date [
4,
6,
7,
8].