Tensiometric and Thermodynamic Study of Aliphatic and Aromatic Amine in Aqueous D-Glucose Solutions: A Comparative Study
Abstract
:1. Introduction
2. Experimental Section
2.1. Materials
2.2. Method
2.3. Surface Parameters along Thermodynamic Physicochemical Interpretations of Molecular Interactions by Various Mathematical Equations
2.3.1. Gibbs Adsorption Isotherm Equations of Interfacial Parameters
2.3.2. Linear Regression Equations of Thermodynamic Parameters
2.3.3. Interfacial Adsorption Equations of Aggregation
3. Results and Discussion
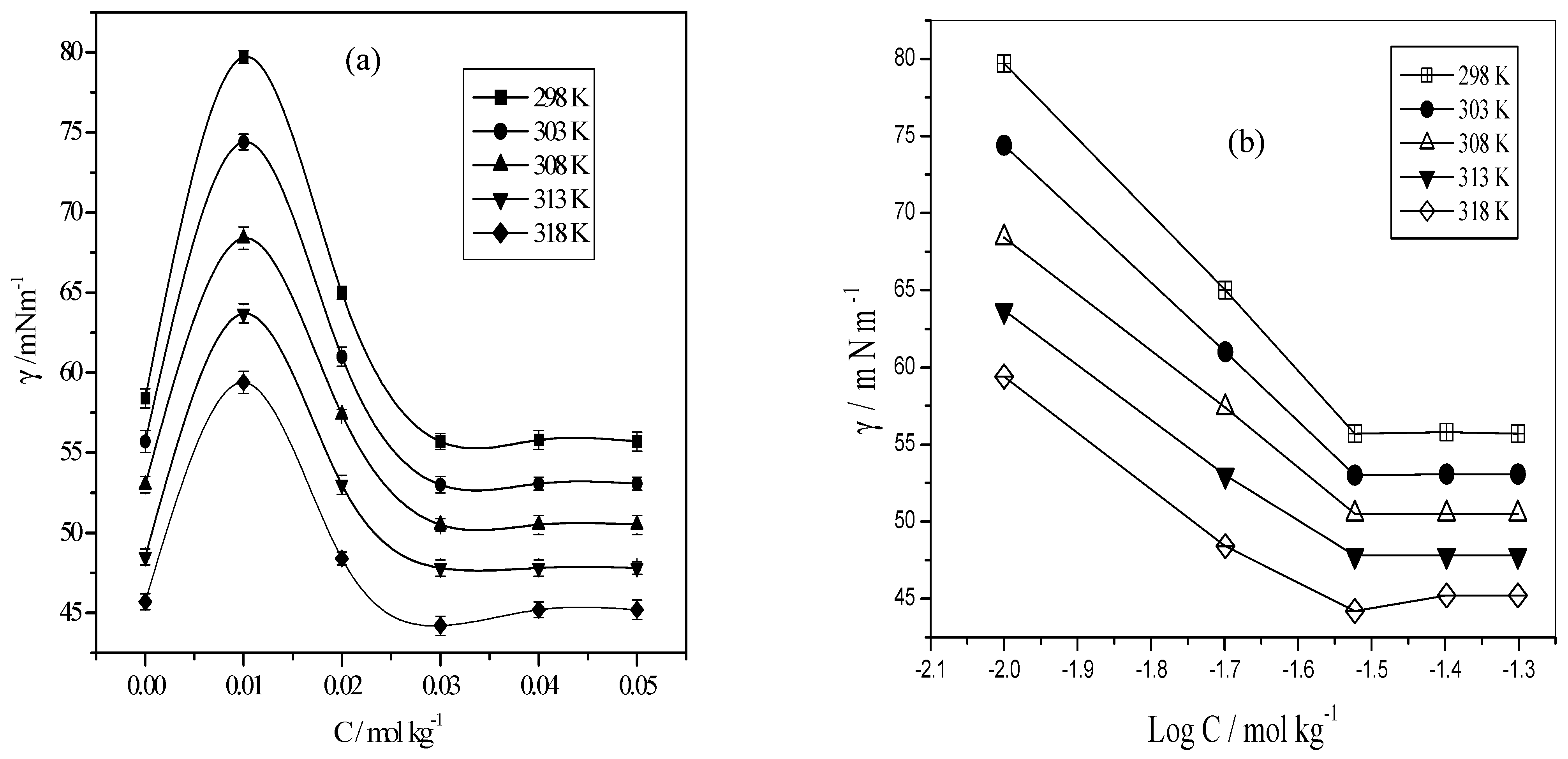
3.1. Self-Aggregation, i.e., Critical Micelle Concentration (CMC)
3.2. Influence of Aliphatic Amines on the Surface Tension Data
3.3. Influence of Aromatic Amines on the Surface Tension Data, Formation of cac, and CMC
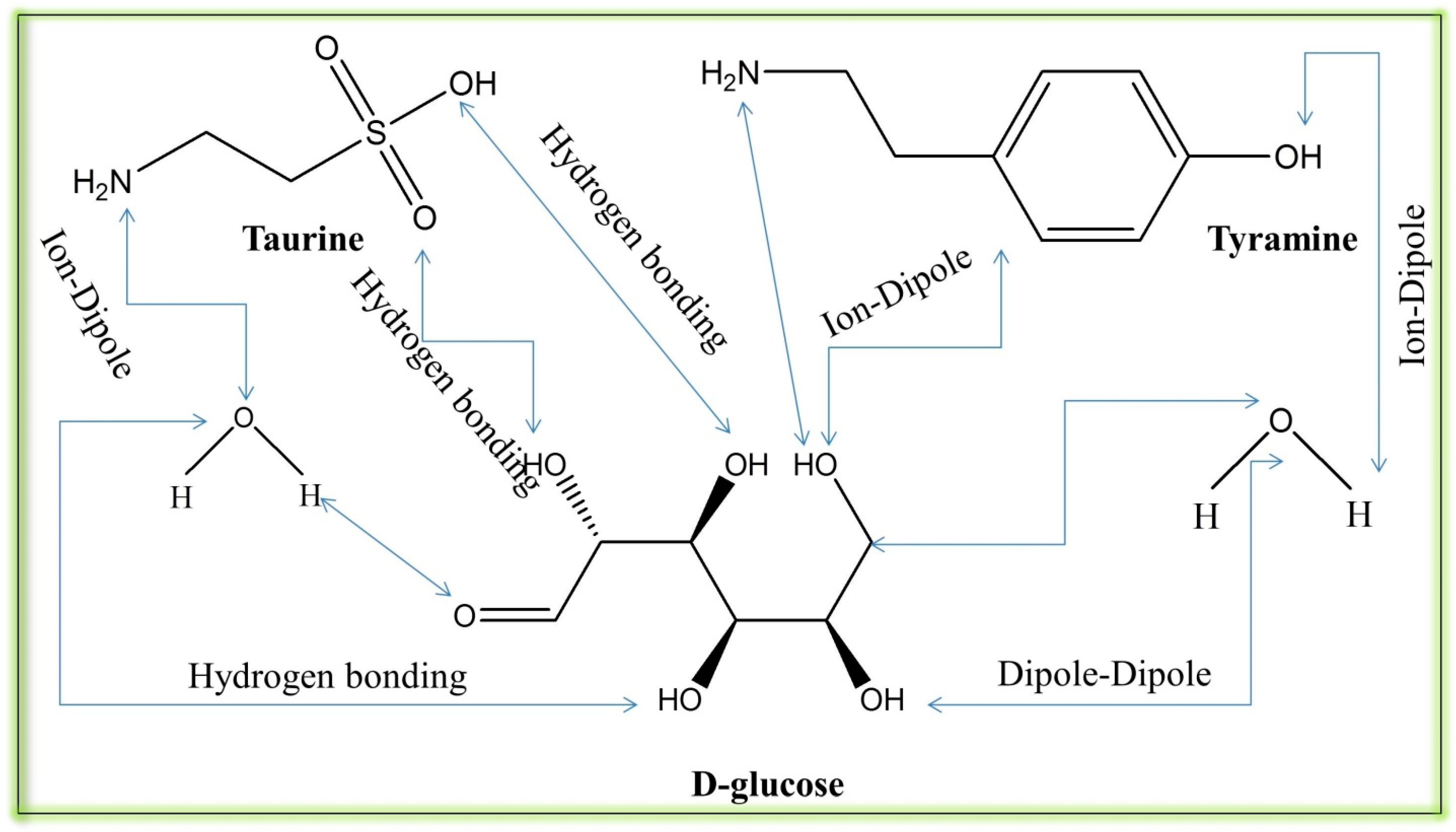
3.4. Counterion Effects on the Micellization Process
3.5. Interfacial Parameters and Gibbs Adsorption Isotherm
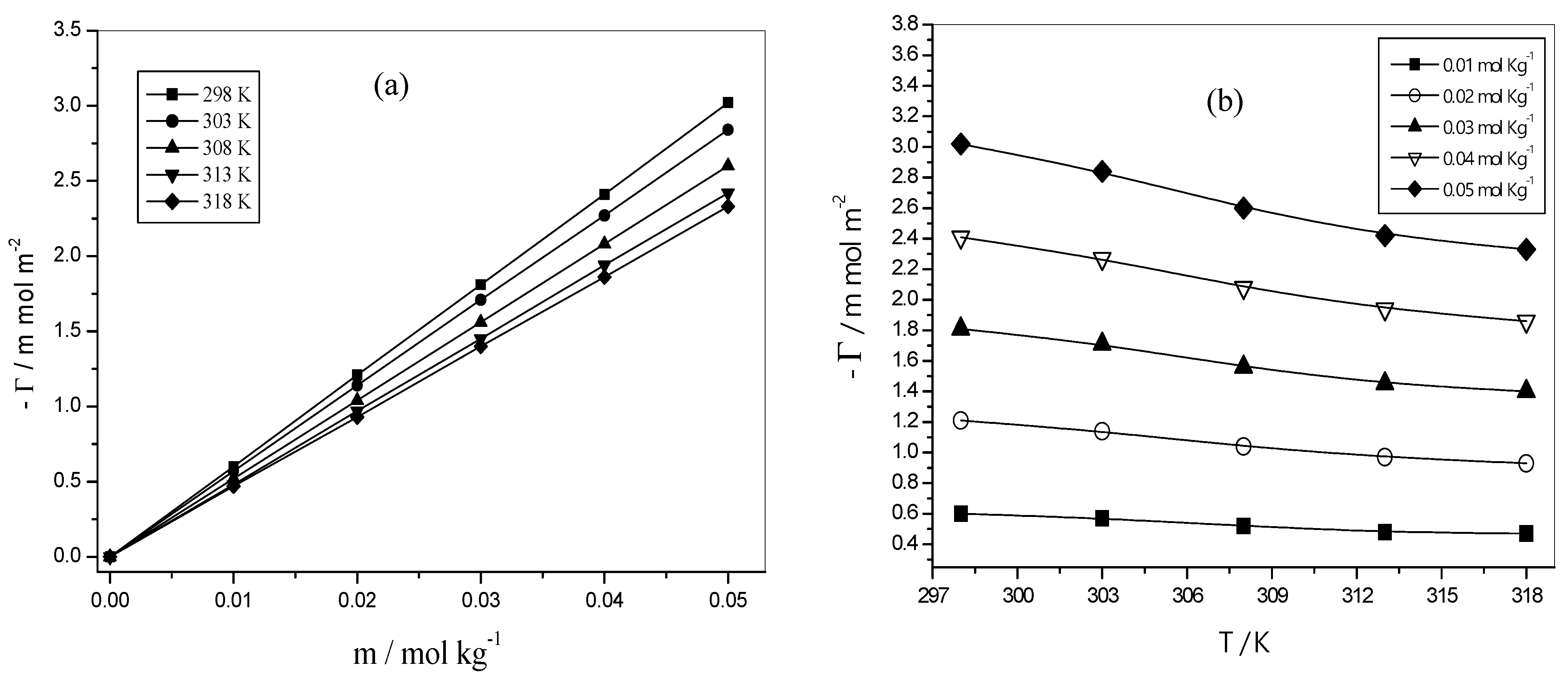
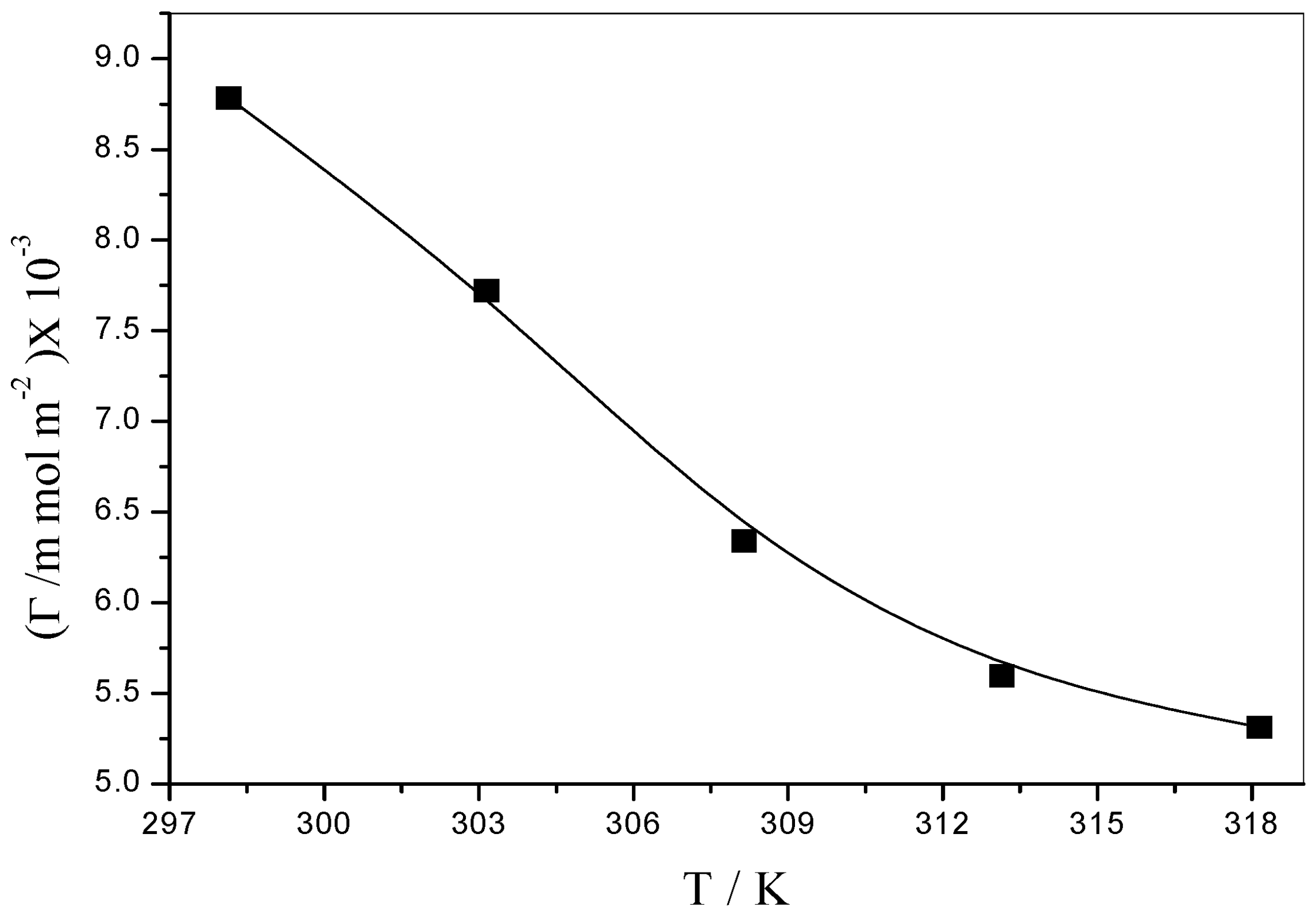
3.5.1. Surface Excess Concentration (Γ)
3.5.2. The Minimum Surface Area per Molecule (Am)
3.5.3. Surface Pressure ()
3.6. Thermodynamic Physiochemical Parameters of Surface Formation
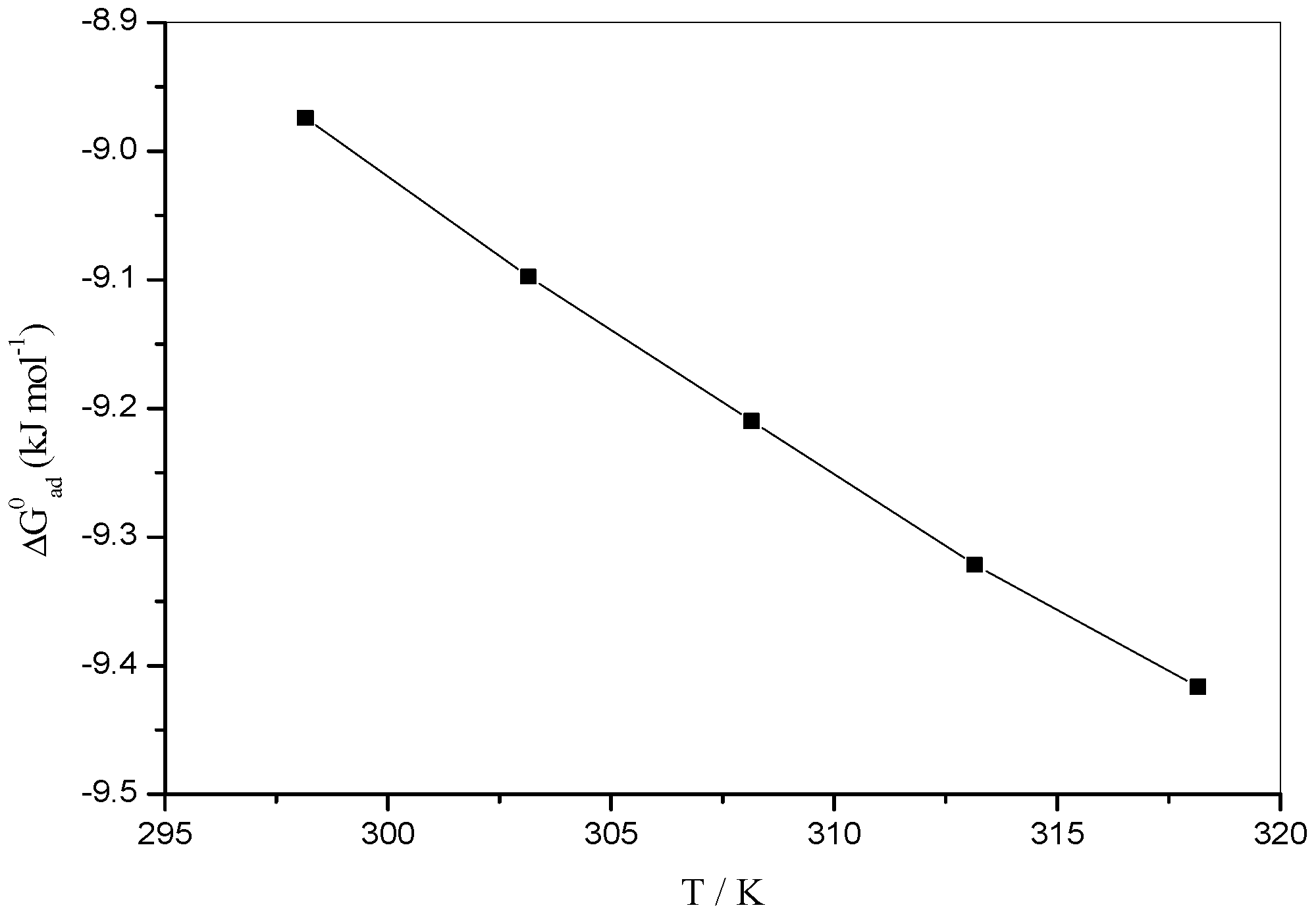
3.7. Thermodynamic Parameter of Adsorption
4. Conclusions
Funding
Institutional Review Board Statement
Acknowledgments
Conflicts of Interest
References
- Coleman, W.; Chung, H. Pyrolys is GC-MS analysis of Amadori compounds derived from selected amino acids with glucose and rhamnose. J. Anal. Appl. Pyrolysis 2002, 63, 349–366. [Google Scholar] [CrossRef]
- Zhuo, K.; Liu, Q.; Wang, Y.; Ren, Q.; Wang, J. Volumetric and Viscosity Properties of Monosaccharides in Aqueous Amino Acid Solutions at 298.15 K. J. Chem. Eng. Data 2006, 51, 919–927. [Google Scholar] [CrossRef]
- Huyghues-Despointes, A.; Yaylayan, V.A.; Keyhani, A. Pyrolysis/GC/MS Analysis of 1-[(2′-carboxyl)pyrrolidinyl]-1-deoxy-D-fructose (Proline Amadori Compound). J. Agric. Food Chem. 1994, 42, 2519–2524. [Google Scholar] [CrossRef]
- Visentin, V.; Marq, P.; Bour, S.; Subra, C.; Prévot, D.; Morin, N.; Valet, P.; Monje, M.-C.; Nepveu, F.; Carpéné, C. Effect of prolonged treatment with tyramine on glucose tolerance in streptozotocin-induced diabetic rats. J. Physiol. Biochem. 2003, 59, 225–232. [Google Scholar] [CrossRef]
- Belitz, H.-D.; Grosch, W.; Schieberle, P. Food Chemistry; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Kulikova, G.A.; Parfenyuk, E.V. Influence of Side Chain of L-α-amino Acids on Their Interaction with D-glucose in Dilute Aqueous Solutions. J. Solut. Chem. 2008, 37, 835–840. [Google Scholar] [CrossRef]
- Pal, A.; Kumar, S. Volumetric properties of l-alanine, and l-valine in aqueous sucrose solutions at T = (288.15 and 308.15) K. J. Chem. Thermodyn. 2005, 37, 1085–1092. [Google Scholar] [CrossRef]
- Magdassi, S. Surface Activity of Proteins: Chemical and Physicochemical Modifications; CRC Press: Boca Raton, FL, USA, 1996. [Google Scholar]
- Romero, C.M.; Albis, A. Influence of Polyols and Glucose on the Surface Tension of Bovine α-Lactalbumin in Aqueous Solution. J. Solut. Chem. 2010, 39, 1865–1876. [Google Scholar] [CrossRef]
- Sengupta, T.; Razumovsky, L.; Damodaran, S. Energetics of Protein−Interface Interactions and Its Effect on Protein Adsorption. Langmuir 1999, 15, 6991–7001. [Google Scholar] [CrossRef]
- Kaushik, J.K.; Bhat, R. Thermal Stability of Proteins in Aqueous Polyol Solutions: Role of the Surface Tension of Water in the Stabilizing Effect of Polyols. J. Phys. Chem. B 1998, 102, 7058–7066. [Google Scholar] [CrossRef]
- Yampolskaya, G.; Platikanov, D. Proteins at fluid interfaces: Adsorption layers and thin liquid films. Adv. Colloid Interface Sci. 2006, 128–130, 159–183. [Google Scholar] [CrossRef]
- Valderrama, J.M. Caracterización Interfacial de Proteínas y Tensioactivos: Aplicación a Dispersiones Alimentarias. Ph.D. Thesis, Universidad de Granada, Granada, Spain, 2006. [Google Scholar]
- Mehr, H.M.; Koocheki, A. Physicochemical properties of Grass pea (Lathyrus sativus L.) protein nanoparticles fabricated by cold atmospheric-pressure plasma. Food Hydrocoll. 2021, 112, 106328. [Google Scholar] [CrossRef]
- Oladzadabbasabadi, N.; Nafchi, A.M.; Ariffin, F.; Wijekoon, M.J.O.; Al-Hassan, A.; Dheyab, M.A.; Ghasemlou, M. Recent advances in extraction, modification, and application of chitosan in packaging industry. Carbohydr. Polym. 2022, 277, 118876. [Google Scholar] [CrossRef] [PubMed]
- Zamani, Z.; Razavi, S.M. Physicochemical, rheological and functional properties of Nettle seed (Urtica pilulifera) gum. Food Hydrocoll. 2021, 112, 106304. [Google Scholar] [CrossRef]
- Huxtable, R.J. Physiological actions of taurine. Physiol. Rev. 1992, 72, 101–163. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Carver, J.D. Advances in nutritional modifications of infant formulas. Am. J. Clin. Nutr. 2003, 77, 1550S–1554S. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ben, X.-M. Nutritional management of newborn infants: Practical guidelines. World J. Gastroenterol. WJG 2008, 14, 6133–6139. [Google Scholar] [CrossRef]
- Roysommuti, S.; Wyss, J.M. Perinatal taurine exposure affects adult arterial pressure control. Amino Acids 2014, 46, 57–72. [Google Scholar] [CrossRef] [Green Version]
- Suwanich, A.; Wyss, J.M.; Roysommuti, S. Taurine supplementation in spontaneously hypertensive rats: Advantages and limitations for human applications. World J. Cardiol. 2013, 5, 404. [Google Scholar] [CrossRef]
- Yamori, Y.; Taguchi, T.; Hamada, A.; Kunimasa, K.; Mori, H.; Mori, M. Taurine in health and diseases: Consistent evidence from experimental and epidemiological studies. J. Biomed. Sci. 2010, 17, S6. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ames, J.M. The Maillard Reaction, Biochemistry of Food Proteins; Springer: Berlin/Heidelberg, Germany, 1992; pp. 99–153. [Google Scholar]
- Sajid, I.; Khaliq, S.; Tabassum, S.; Anis, L.; Ahmed, S.; Haider, S. A dose related study on the effects of taurine administration on recognition and spatial memory functions and depression like symptoms in rats. Int. J. Adv. Res. 2013, 1, 364–371. [Google Scholar]
- Morin, N.; Visentin, V.; Calise, D.; Marti, L.; Zorzano, A.; Testar, X.; Valet, P.; Fischer, Y.; Carpéné, C. Tyramine stimulates glucose uptake in insulin-sensitive tissues in vitro and in vivo via its oxidation by amine oxidases. J. Pharmacol. Exp. Ther. 2002, 303, 1238–1247. [Google Scholar] [CrossRef] [Green Version]
- Ali, K.; Shah, A.-U.A.; Bilal, S. Surface tensions and thermodynamic parameters of surface formation of aqueous salt solutions: III. Aqueous solution of KCl, KBr and KI. Colloids Surfaces A Physicochem. Eng. Asp. 2009, 337, 194–199. [Google Scholar] [CrossRef]
- Nabi, A.; Tasneem, S.; Jesudason, C.G.; Lee, V.S.; Zain, S.B.M. Study of interaction between cationic surfactant (CTAB) and paracetamol by electrical conductivity, tensiometric and spectroscopic methods. J. Mol. Liq. 2018, 256, 100–107. [Google Scholar] [CrossRef]
- Nabi, A.; Jesudason, C.G.; Malik, M.A.; Nabi, F. Prediction of viscosities and COSMO-RS analyses in binary mixtures of N,N-dimethylformamide with acetone. Phys. Chem. Liq. 2017, 55, 264–275. [Google Scholar] [CrossRef]
- Ali, K.; Haq, A.U.; Bilal, S.; Siddiqi, S. Concentration and temperature dependence of surface parameters of some aqueous salt solutions. Colloids Surfaces A Physicochem. Eng. Asp. 2006, 272, 105–110. [Google Scholar] [CrossRef]
- Castro, E.; Taboada, P.; Mosquera, V. Cosolvent Effects on the Micellization of Oxyphenyl(copoly)ethylene Oxide Copolymers in Aqueous Solution. J. Phys. Chem. B 2006, 110, 13113–13123. [Google Scholar] [CrossRef]
- Belton, G. Langmuir adsorption, the Gibbs adsorption isotherm, and interfacial kinetics in liquid metal systems. Metall. Mater. Trans. B 1976, 7, 35–42. [Google Scholar] [CrossRef]
- Naess, S.N.; Elgsaeter, A.; Foss, B.J.; Li, B.; Sliwka, H.-R.; Partali, V.; Melø, T.B.; Naqvi, K.R. Hydrophilic Carotenoids: Surface Properties and Aggregation of Crocin as a Biosurfactant. Helv. Chim. Acta 2006, 89, 45–53. [Google Scholar] [CrossRef]
- Chauhan, S.; Sharma, P.; Rana, D. Thermodynamics and micellization of cetyltrimethyl ammonium bromide in the presence of lysozyme. J. Mol. Liq. 2013, 187, 1–6. [Google Scholar] [CrossRef]
- Rao, K.S.; Singh, T.; Trivedi, T.J.; Kumar, A. Aggregation Behavior of Amino Acid Ionic Liquid Surfactants in Aqueous Media. J. Phys. Chem. B 2011, 115, 13847–13853. [Google Scholar] [CrossRef] [PubMed]
- Abraham, M.; Abraham, M.C. Ziogas, Surface tension of liquids from molten nitrate mixtures to water. J. Am. Chem. Soc. 1991, 113, 8583–8590. [Google Scholar] [CrossRef]
- Maeda, H. A Simple Thermodynamic Analysis of the Stability of Ionic/Nonionic Mixed Micelles. J. Colloid Interface Sci. 1995, 172, 98–105. [Google Scholar] [CrossRef]
- Shah, A.-U.A.; Ali, K.; Bilal, S. Surface tension, surface excess concentration, enthalpy and entropy of surface formation of aqueous salt solutions. Colloids Surfaces A Physicochem. Eng. Asp. 2013, 417, 183–190. [Google Scholar] [CrossRef]
- Soni, S.S.; Sastry, N.V.; Aswal, V.K.; Goyal, P.S. Micellar Structure of Silicone Surfactants in Water from Surface Activity, SANS and Viscosity Studies. J. Phys. Chem. B 2002, 106, 2606–2617. [Google Scholar] [CrossRef]
- Sínanoĝlu, O.; Abdulnur, S. Hydrophobic stacking of bases and the solvent denaturation of DNA. Photochem. Photobiol. 1964, 3, 333–342. [Google Scholar] [CrossRef]
- Noudeh, G.D.; Housaindok, M.; Bazzaz, B.S.F. The Effect of Temperature on Thermodynamic Parameters of Micellization of Some Surfactants. J. Appl. Sci. 2007, 7, 47–52. [Google Scholar] [CrossRef] [Green Version]
- Ikeda, N.; Aratono, M.; Motomura, K. Thermodynamic study on the adsorption of sodium chloride at the water/hexane interface. J. Colloid Interface Sci. 1992, 149, 208–215. [Google Scholar] [CrossRef]
- Zhao, C.; Ma, P.; Li, J. Partial molar volumes and viscosity B-coefficients of arginine in aqueous glucose, sucrose and l-ascorbic acid solutions at T = 298.15 K. J. Chem. Thermodyn. 2005, 37, 37–42. [Google Scholar] [CrossRef]
- Banipal, T.S.; Kaur, J.; Banipal, P.K.; Singh, K. Study of Interactions between Amino Acids and Zinc Chloride in Aqueous Solutions through Volumetric Measurements at T = (288.15 to 318.15) K. J. Chem. Eng. Data 2008, 53, 1803–1816. [Google Scholar] [CrossRef]
- Hamid, M.K.; Rüter, A.; Kuczera, S.; Olsson, U. Slow Dissolution Kinetics of Model Peptide Fibrils. Int. J. Mol. Sci. 2020, 21, 7671. [Google Scholar] [CrossRef]
- Chandra, A.; Patidar, V.; Singh, M.; Kale, R. Physicochemical and friccohesity study of glycine, l-alanine and l-phenylalanine with aqueous methyltrioctylammonium and cetylpyridinium chloride from T = (293.15 to 308.15)K. J. Chem. Thermodyn. 2013, 65, 18–28. [Google Scholar] [CrossRef]
- Niraula, T.P.; Shah, S.K.; Chatterjee, S.K.; Bhattarai, A. Effect of methanol on the surface tension and viscosity of sodiumdodecyl sulfate (SDS) in aqueous medium at 298.15–323.15 K. Karbala Int. J. Mod. Sci. 2018, 4, 26–34. [Google Scholar] [CrossRef]
- Matubayasi, N.; Yoshikawa, R. Thermodynamic quantities of surface formation of aqueous electrolyte solutions: VII. Aqueous solution of alkali metal nitrates LiNO3, NaNO3, and KNO3. J. Colloid Interface Sci. 2007, 315, 597–600. [Google Scholar] [CrossRef] [PubMed]
- Matubayasi, N.; Matsuo, H.; Yamamoto, K.; Yamaguchi, S.-I.; Matuzawa, A. Thermodynamic Quantities of Surface Formation of Aqueous Electrolyte Solutions: I. aqueous solutions of NaCl, MgCl2, and LaCl3. J. Colloid Interface Sci. 1999, 209, 398–402. [Google Scholar] [CrossRef]
- Matubayasi, N.; Tsunetomo, K.; Sato, I.; Akizuki, R.; Morishita, T.; Matuzawa, A.; Natsukari, Y. Thermodynamic quantities of surface formation of aqueous electrolyte solutions: IV. Sodium halides, anion mixtures, and sea water. J. Colloid Interface Sci. 2001, 243, 444–456. [Google Scholar] [CrossRef]
- Khan, A.; Siddiq, M. Surface activity and micellar behavior of dimethylamino- and trimethylammonium- tipped oxyethylene-oxybutylene diblock copolymers in aqueous media. J. Appl. Polym. Sci. 2010, 118, 3324–3332. [Google Scholar] [CrossRef]


| Parameters T/(K) | γCMC (mNm−1) | (mNm−1) | CMC (mol·kg−1) | ∂γ/∂log10 C | −Γ (103 mol·m−2) | Am (10−5 nm2) |
|---|---|---|---|---|---|---|
| 298.15 | 55.95 | 2.45 | 0.02998 | 50.14 | 8.7831 ± 0.098 | 1.8903 |
| 303.15 | 53.33 | 2.37 | 0.03019 | 44.81 | 7.7199 ± 0.091 | 2.1507 |
| 308.15 | 50.59 | 2.41 | 0.03035 | 37.41 | 6.3405 ± 0.092 | 2.6186 |
| 313.15 | 47.70 | 0.80 | 0.03048 | 33.56 | 5.5971 ± 0.097 | 2.9663 |
| 318.15 | 44.81 | 0.89 | 0.03062 | 32.36 | 5.3122 ± 0.094 | 3.1255 |
| m (mol·kg−1) | T/(K) | ||||
|---|---|---|---|---|---|
| 298.15 | 303.15 | 308.15 | 313.15 | 318.15 | |
| Glucose + aq. Taurine | Surface excess concentration (Γ × 10−4/mol m−2) | ||||
| 0.01 | 6.0341 ± 0.023 | 5.6845 ± 0.066 | 5.1978 ± 0.011 | 4.8457 ± 0.051 | 4.6561 ± 0.038 |
| 0.02 | 12.0683 ± 0.022 | 11.3689 ± 0.069 | 10.3956 ± 0.020 | 9.6915 ± 0.084 | 9.3122 ± 0.093 |
| 0.03 | 18.1024 ± 0.024 | 17.0534 ± 0.036 | 15.5931 ± 0.051 | 14.5372 ± 0.067 | 13.9683 ±0.056 |
| 0.04 | 24.1365 ± 0.024 | 22.7379 ± 0.030 | 20.7911 ± 0.035 | 19.3829 ± 0.030 | 18.6243 ± 0.090 |
| 0.05 | 30.1707 ± 0.033 | 28.4224 ± 0.091 | 25.9889 ± 0.029 | 24.2287 ±0.061 | 23.2804 ± 0.036 |
| m (mol·kg−1) | T/(K) | ||||
|---|---|---|---|---|---|
| 298.15 | 303.15 | 308.15 | 313.15 | 318.15 | |
| Glucose + aq. Taurine | Surface pressure (π/mNm−2) | ||||
| 0.01 | 2.00 | 2.07 | 2.03 | 2.06 | 2.06 |
| 0.02 | 3.70 | 3.80 | 3.63 | 3.50 | 3.53 |
| 0.03 | 5.30 | 5.30 | 5.03 | 4.79 | 4.76 |
| 0.04 | 6.70 | 6.57 | 6.16 | 5.90 | 5.75 |
| 0.05 | 7.33 | 7.03 | 6.56 | 6.27 | 6.16 |
| m (mol·kg−1) | T/(K) | ||||
|---|---|---|---|---|---|
| 298.15 | 303.15 | 308.15 | 313.15 | 318.15 | |
| Glucose. aq. Taurine | Enthalpies, ΔH (kJ/mol) | ||||
| 0 | 13.26 ± 0.036 | 11.185 ± 0.043 | 8.94 ± 0.021 | 6.735 ± 0.096 | 4.723 ±0.113 |
| 0.01 | 10.664 ± 0.033 | 9.594 ± 0.069 | 8.464 ± 0.094 | 7.304 ± 0.089 | 6.364 ± 0.119 |
| 0.02 | 13.434 ± 0.040 | 12.334 ± 0.035 | 11.334 ± 0.083 | 10.334 ± 0.084 | 9.364 ± 0.125 |
| 0.03 | 17.496 ± 0.082 | 16.496 ± 0.044 | 15.596 ± 0.087 | 14.706 ± 0.087 | 13.796 ± 0.106 |
| 0.04 | 21.758 ± 0.069 | 20.888 ± 0.062 | 20.128 ± 0.061 | 19.258 ± 0.023 | 18.468 ± 0.121 |
| 0.05 | 15.466 ± 0.061 | 14.766 ± 0.064 | 14.066 ± 0.091 | 13.226 ± 0.031 | 12.396 ± 0.109 |
| Glucose + aq. Taurine | ||
|---|---|---|
| m (mol·kg−1) | ΔS/(kJ·mol−1 K−1) | R2 |
| 0 | 0.215 ± 0.0046 | 0.99826 |
| 0.01 | 0.217 ± 0.0050 | 0.99862 |
| 0.02 | 0.202 ± 0.0044 | 0.99928 |
| 0.03 | 0.183 ± 0.0079 | 0.99947 |
| 0.04 | 0.164 ± 0.0045 | 0.99715 |
| T/K | /(kJ·mol−1) | /(kJ·mol−1) | /(kJ·mol−1) | /(kJ·mol−1 K−1) |
|---|---|---|---|---|
| 298.15 | −8.6953 ± 0.041 | −8.9743 ± 0.020 | 15.5336 ± 0.024 | 0.022 ± 0.103 |
| 303.15 | −8.8230 ± 0.033 | −9.0976 ± 0.016 | 15.7669 ± 0.024 | 0.022 ± 0.103 |
| 308.15 | −8.9559 ± 0.033 | −9.2098 ± 0.019 | 15.9891 ± 0.027 | 0.022 ± 0.103 |
| 313.15 | −9.0894 ± 0.031 | −9.3217 ± 0.018 | 16.2110 ± 0.023 | 0.022 ± 0.103 |
| 318.15 | −9.2225 ± 0.044 | −9.4164 ± 0.015 | 16.4157 ± 0.029 | 0.022 ± 0.103 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tasneem, S. Tensiometric and Thermodynamic Study of Aliphatic and Aromatic Amine in Aqueous D-Glucose Solutions: A Comparative Study. Appl. Sci. 2023, 13, 7012. https://doi.org/10.3390/app13127012
Tasneem S. Tensiometric and Thermodynamic Study of Aliphatic and Aromatic Amine in Aqueous D-Glucose Solutions: A Comparative Study. Applied Sciences. 2023; 13(12):7012. https://doi.org/10.3390/app13127012
Chicago/Turabian StyleTasneem, Shadma. 2023. "Tensiometric and Thermodynamic Study of Aliphatic and Aromatic Amine in Aqueous D-Glucose Solutions: A Comparative Study" Applied Sciences 13, no. 12: 7012. https://doi.org/10.3390/app13127012
APA StyleTasneem, S. (2023). Tensiometric and Thermodynamic Study of Aliphatic and Aromatic Amine in Aqueous D-Glucose Solutions: A Comparative Study. Applied Sciences, 13(12), 7012. https://doi.org/10.3390/app13127012






