Study on Low Temperature Drying Characteristics of Chicken Manure in a Humid Environment
Abstract
:1. Introduction
2. Materials and Methods
2.1. Experimental Material
2.2. Experimental Apparatus
2.3. Experimental Uncertainty
2.4. Experiments Procedure
2.5. Calculation of Drying Parameters
3. Results and Discussion
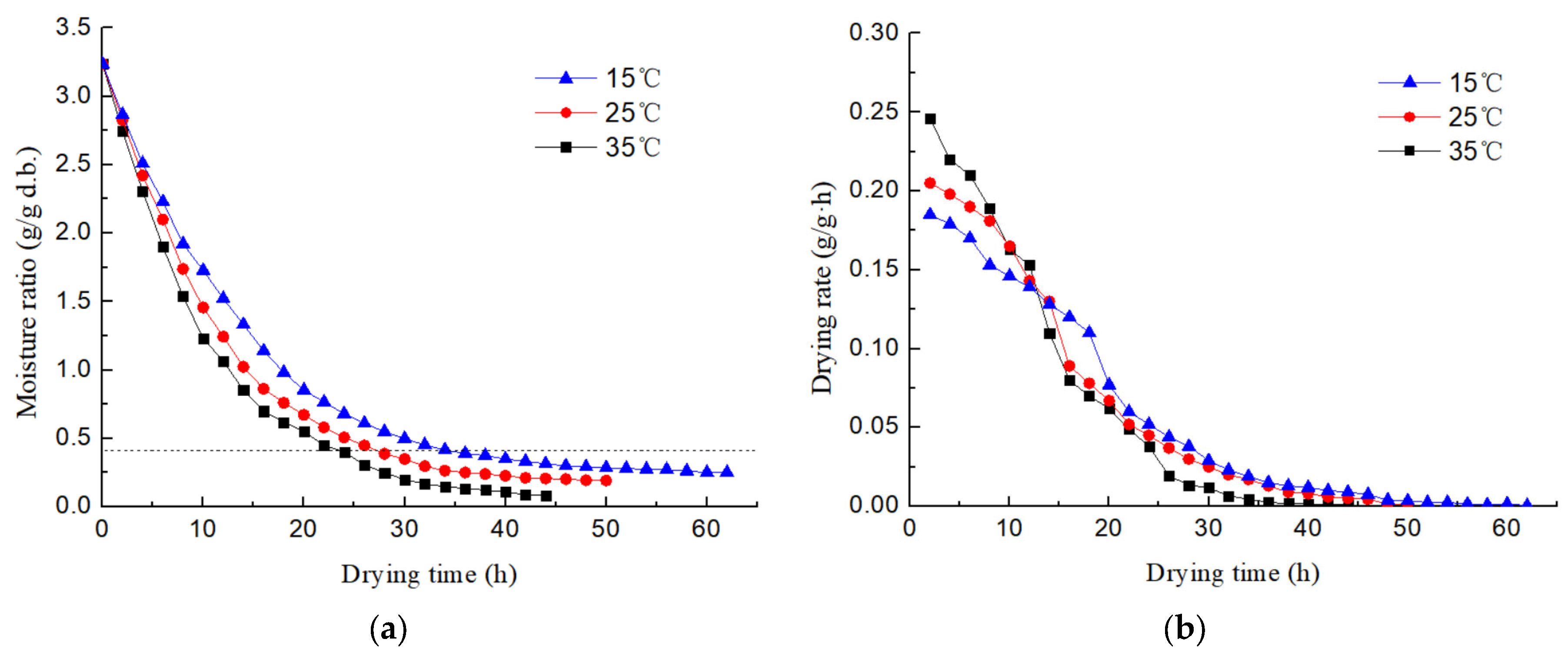
3.1. Drying Characteristic at Different Drying Temperatures
3.2. Drying Characteristics at Different Air Velocities
3.3. Drying Characteristics at Different Depths of Manure Layer
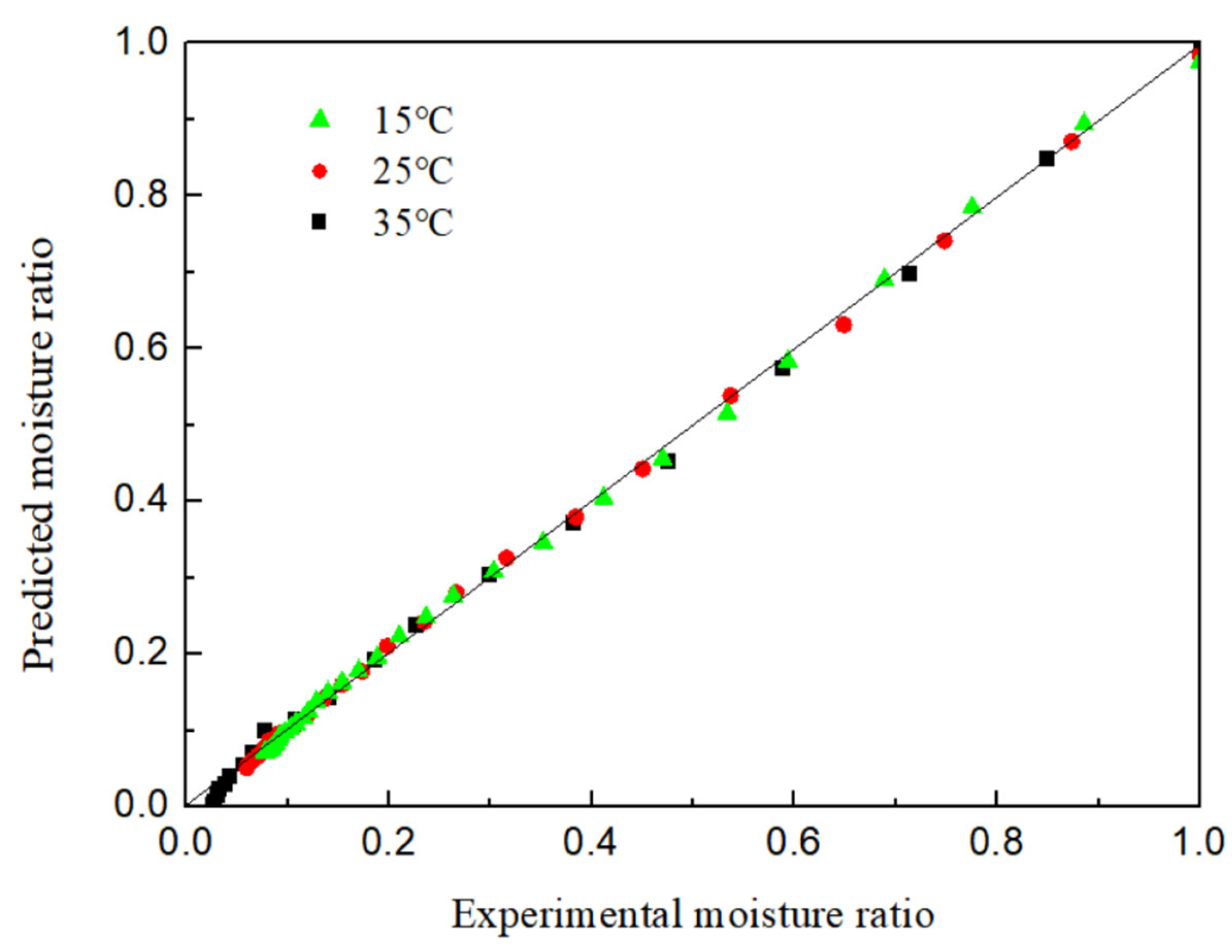
3.4. Fitting of the Drying Curves
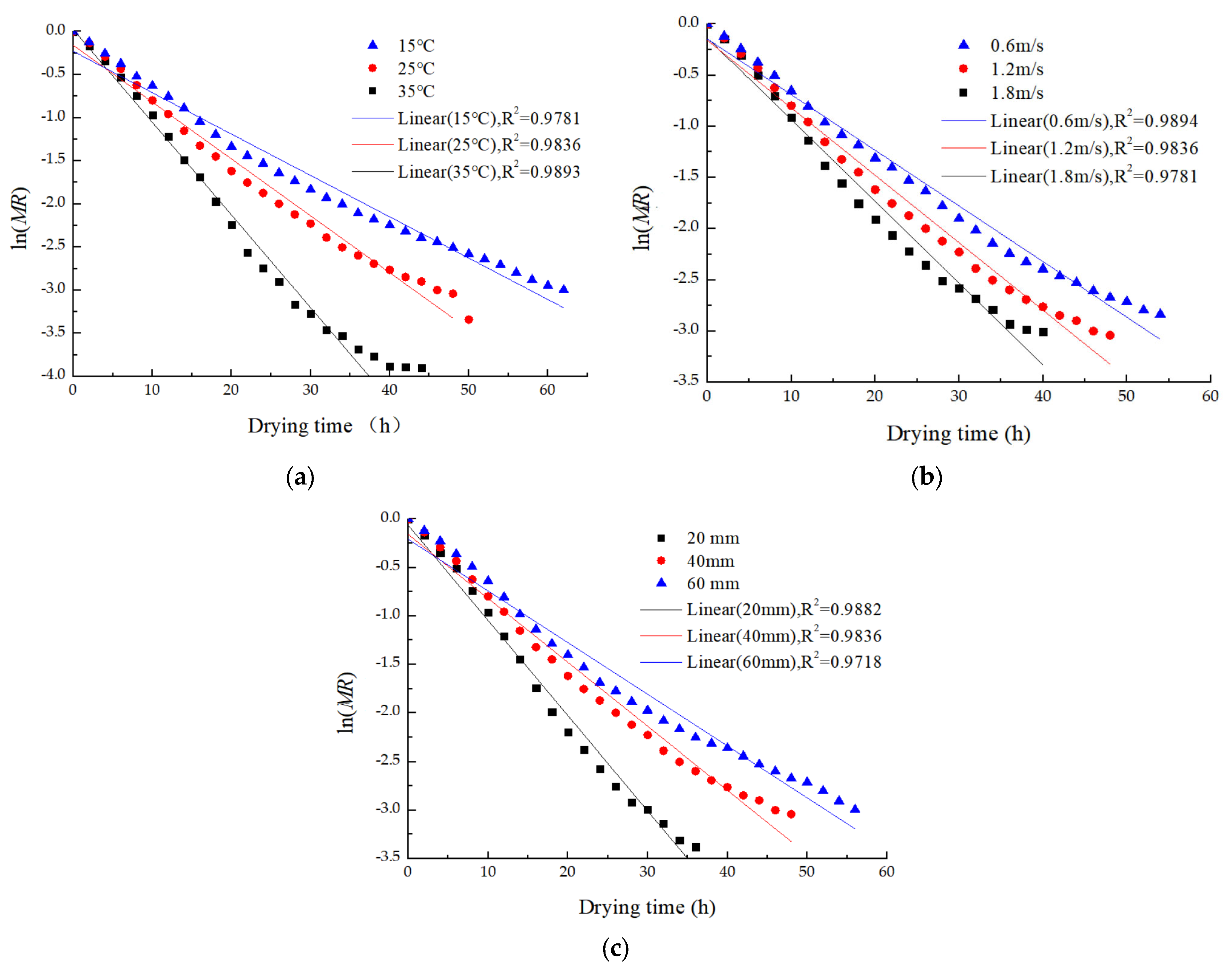
3.5. Calculation of Effective Diffusivity
4. Conclusions
- (1)
- The drying effect was proportional to the temperature and air velocity, and was inversely proportional to the depth of the manure layer. The drying time of the low- temperature drying process in a humid environment was more than 24 h, which will increase the disposal cycle of chicken manure. Therefore, it was necessary to increase the drying temperature and air velocity or reduce the thickness of the manure layer to shorten the drying time to within 24 h.
- (2)
- The low-temperature drying process of chicken manure in a humid environment had only a falling rate period, with no accelerated or constant rate period, and there was a turning point in the falling rate period. Furthermore, with increasing temperature and air velocity and decreasing manure depth, the turning points of the drying rate curves appeared earlier.
- (3)
- The Exponential model was considered to be more suitable for describing the low-temperature drying process of chicken manure in a humid environment, which can be used to predict the drying rate and select reasonable drying parameters in actual production. The effective diffusivity of a low-temperature drying process of chicken manure in a humid environment changed between 6.37 × 10−7 and 2.17 × 10−6 m2/h, and the values were proportional to the temperature and air velocity.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kotir, J.H. Climate change and variability in Sub-Saharan Africa: A review of current and future trends and impacts on agriculture and food security. Environ. Dev. Sustain. 2011, 13, 587–605. [Google Scholar] [CrossRef]
- Loyon, L. Overview of manure treatment in France. Waste Manag. 2017, 61, 516–520. [Google Scholar] [CrossRef] [PubMed]
- Manogaran, M.D.; Shamsuddin, R.; Yusoff, M.H. A Review on Treatment Processes of Chicken Manure. Clean. Circ. Bioeconomy 2022, 2, 100013. [Google Scholar] [CrossRef]
- Wilkinson, K.G.; Tee, E.; Tomkins, R.B.; Hepworth, G.; Premier, R. Effect of heating and aging of poultry litter on the persistence of enteric bacteria. Poult. Sci. 2011, 90, 10–18. [Google Scholar] [CrossRef]
- Lin, X.; Zhang, R.; Jiang, S.; Mashad, H.; Xin, H. Emissions of ammonia, carbon dioxide and particulate matter from cage-free layer houses in California. Atmos. Environ. 2017, 152, 246–255. [Google Scholar] [CrossRef] [Green Version]
- Aboltins, A.; Kic, P. Forced convection in drying of poultry manure. Agron. Res. 2015, 13, 215–222. [Google Scholar]
- Liska, R.; Kic, P. Drying process of poultry manure at various temperatures. In Proceedings of the 4th International Conference TAE, Prague, Czech Republic, 7–10 September 2010. [Google Scholar]
- Ghaly, A.E.; Macdonald, K.N. Kinetics of thin layer drying of poultry manure. Am. J. Biochem. Biotechnol. 2012, 8, 128–142. [Google Scholar] [CrossRef] [Green Version]
- Zheng, W.; Xiong, Y.; Gates, R. Air temperature, carbon dioxide, and ammonia assessment inside a commercial cage layer barn with manure-drying tunnels. Poult. Sci. 2020, 99, 3885–3896. [Google Scholar] [CrossRef]
- Li, X.; Li, B.; Zheng, W.; Wang, Q.; Tong, Q. Drying performance of manure drying system with waste heat of poultry house in summer. Trans. Chin. Soc. Agric. Eng. 2019, 35, 188–194. [Google Scholar] [CrossRef]
- Winkel, A.; Mosquera, J.; Aarnink, A.J.A.; Koerkamp, P.W.G.G.; Ogink, N.W.M. Evaluation of manure drying tunnels to serve as dust filters in the exhaust of laying hen houses: Emissions of particulate matter, ammonia, and odour. Biosyst. Eng. 2017, 162, 81–98. [Google Scholar] [CrossRef]
- Li, X.; Zheng, W.; Li, B.; Tong, Q. Optimization of low-temperature drying of laying-hen manure using response surface methodology. J. Air Waste Manag. Assoc. 2020, 70, 206–218. [Google Scholar] [CrossRef] [PubMed]
- Winkel, A.; Losada, J.M.; Ellen, H.H. Dust filtering properties and ammonia emission of on-farm drying systems for poultry manure. Q. J. Med. 2012, 25, 527–539. [Google Scholar]
- Li, X.; Li, B.; Tong, Q. The effect of drying temperature on nitrogen loss and pathogen removal in laying hen manure. Sustainability 2020, 12, 403. [Google Scholar] [CrossRef] [Green Version]
- Keiichi, M.; Masayuki, H.; Takuya, K. Increased total nitrogen content of poultry manure by decreasing water content through composting processes. Soil Sci. Plant Nutr. 2011, 57, 705–709. [Google Scholar] [CrossRef]
- Rosa, E.; Arriaga, H.; Merino, P. Ammonia emission from a manure-belt laying hen facility equipped with an external manure drying tunnel. J. Clean. Prod. 2019, 251, 119591. [Google Scholar] [CrossRef]
- Chepete, J.H.; Hongwei, X.; Hong, L. Ammonia Emissions of Laying-Hen Manure as Affected by Accumulation Time. J. Poult. Sci. 2011, 48, 133–138. [Google Scholar] [CrossRef] [Green Version]
- Majdi, H.J.A.; Esfahan, M. Optimization of convective drying by response surface methodology. Comput. Electron. Agric. 2019, 156, 574–584. [Google Scholar] [CrossRef]
- Ghaly, A.; Alhattab, M. Drying poultry manure for pollution potential reduction and production of organic fertilizer. Am. J. Environ. Sci. 2013, 9, 88–102. [Google Scholar] [CrossRef] [Green Version]
- Aboltins, A.; Kic, P. Comparison of two methods of poultry manure drying. Eng. Rural. Dev. 2014, 30, 143–149. [Google Scholar]
- Wu, Y.; Liu, Y.; Han, J. Study on convective drying characteristics of dredged sludge from Dian Lake. Sep. Sci. Technol. 2015, 50, 1731–1739. [Google Scholar] [CrossRef]
- Shekarau, B.L.; Zakka, R.; Tsokwa, T. Mathematical modelling of thin layer drying kinetics of cashew apple pomace in hot air oven dryer. Acta Period. Technol. 2020, 51, 119–136. [Google Scholar] [CrossRef]
- Siewkian, C.; Chunglim, L.; Supramaniam, C.V. Thin-layer drying characteristics and quality evaluation of air-dried Ganoderma tsugae Murrill. Dry. Technol. 2009, 27, 975–984. [Google Scholar] [CrossRef]
- Ameri, B.; Hanini, S.; Boumahdi, M. Influence of drying methods on the thermodynamic parameters, effective moisture diffusion and drying rate of wastewater sewage sludge. Renew. Energy 2020, 147, 1107–1119. [Google Scholar] [CrossRef]
- Wang, Z.; Xu, L.; Liu, D. Effects of Air Temperature and Humidity on the Kinetics of Sludge Drying at Low Temperatures. Energies 2021, 14, 7722. [Google Scholar] [CrossRef]
- Guine, R.; Lima, M.J.; Pereira, R. Study of the drying kinetics and calculation of mass transfer properties in hot air drying of Cynara cardunculus. Open Agric. 2020, 5, 740–750. [Google Scholar] [CrossRef]




| Samples | Moisture (%) | Ash (%) | Volatiles (%) | pH |
|---|---|---|---|---|
| Chicken manure | 78.4 | 27.0 | 71.5 | 7.3 |
| Parameter | Uncertainty |
|---|---|
| Temperature via temperature sensor | ±0.1 (°C) |
| Humidity via humidity sensor | ±2.5 (%) |
| Air velocity | ±0.1 (m/s) |
| Manure weight | ±0.1(g) |
| Group | Air Velocity (m/s) | Thickness (mm) | Temperature (°C) |
|---|---|---|---|
| 1 | 1.2 | 40 | 15 |
| 2 | 1.2 | 40 | 25 |
| 3 | 1.2 | 40 | 35 |
| 4 | 1.2 | 20 | 25 |
| 5 | 1.2 | 60 | 25 |
| 6 | 0.6 | 40 | 25 |
| 7 | 1.8 | 40 | 25 |
| Drying Model | Model Expression |
|---|---|
| Lewis | MR = exp(−Kt) |
| Page | MR = exp(−Ktn) |
| Wang and singh | MR = 1 + At + Bt2 |
| Henderson and Pabis model | MR = Aexp(−Kt) |
| Exponential | MR = Aexp(−Kt) + C |
| Group | Model | Model Coefficients | R2 | χ2 | RMSE |
|---|---|---|---|---|---|
| 15 °C | Lewis | A = 0.0613 | 0.9873 | 8.57 × 10−4 | 0.0288 |
| Page | K = 0.0829, n = 0.8978 | 0.9910 | 6.06 × 10−4 | 0.0238 | |
| 1.2 m/s | Wang and Singh | A = −0.0435, B = 7.72 × 10−4 | 0.9495 | 3.41 × 10−3 | 0.0567 |
| Henderson and Pabis | A = 0.9788, K = 0.0599 | 0.9841 | 8.49 × 10−4 | 0.0282 | |
| 40 mm | Exponential | C = 0.0608, A = 0.9587, K = 0.0745 | 0.9986 | 9.39 × 10−5 | 0.0092 |
| 25 °C | Lewis | A = 0.0772 | 0.9939 | 3.37 × 10−4 | 0.0180 |
| Page | K = 0.08205, n = 0.9776 | 0.9945 | 3.38 × 10−4 | 0.0176 | |
| 1.2 m/s | Wang and Singh | A = −0.05182, B = 7.72 × 10−4 | 0.9662 | 2.59 × 10−3 | 0.0488 |
| Henderson and Pabis | A = 1.0053, K = 0.0776 | 0.9954 | 3.49 × 10−4 | 0.0179 | |
| 40 mm | Exponential | C = 0.0357, A = 0.9898, K = 0.0873 | 0.9985 | 1.13 × 10−4 | 0.0099 |
| 35 °C | Lewis | A = 0.1008 | 0.9943 | 5.21 × 10−4 | 0.0223 |
| Page | K = 0.0682, n = 1.1550 | 0.9933 | 6.44 × 10−5 | 0.0076 | |
| 1.2 m/s | Wang and Singh | A = −0.0694, B = 0.0012 | 0.9849 | 1.37 × 10−3 | 0.0351 |
| Henderson and Pabis | A = 1.0396, K = 0.1046 | 0.9957 | 3.91 × 10−4 | 0.0188 | |
| 40 mm | Exponential | C = −0.0209, A = 1.0515, K = 0.0985 | 0.9964 | 3.26 × 10−4 | 0.0166 |
| 25 °C | Lewis | A = 0.1002 | 0.9920 | 7.28 × 10−4 | 0.0263 |
| Page | K = 0.0694, n = 1.1479 | 0.9913 | 3.27 × 10−4 | 0.0171 | |
| 1.2 m/s | Wang and Singh | A = −0.0717, B = 0.0013 | 0.9903 | 8.87 × 10−4 | 0.0282 |
| Henderson and Pabis | A = 1.0373, K = 0.1038 | 0.9930 | 6.44 × 10−4 | 0.0236 | |
| 20 mm | Exponential | C = −0.0097, A = 1.0430, K = 0.1009 | 0.9932 | 6.23 × 10−4 | 0.0233 |
| 25 °C | Lewis | A = 0.0657 | 0.9880 | 8.84 × 10−4 | 0.0292 |
| Page | K = 0.0721, n = 0.9674 | 0.9879 | 8.87 × 10−4 | 0.0287 | |
| 1.2 m/s | Wang and Singh | A = −0.0476, B = 5.87 × 10−4 | 0.9654 | 5.86 × 10−4 | 0.0234 |
| Henderson and Pabis | A = 1.0079, K = 0.0662 | 0.9876 | 9.12 × 10−4 | 0.0292 | |
| 60 mm | Exponential | C = 0.17328, A = 3.1951, K = 0.0793 | 0.9954 | 3.43 × 10−4 | 0.0175 |
| 25 °C | Lewis | A = 0.0636 | 0.9968 | 2.38 × 10−4 | 0.0152 |
| Page | K = 0.0716, n = 0.9595 | 0.9421 | 2.01 × 10−4 | 0.0137 | |
| 0.6 m/s | Wang and Singh | A = −0.0466, B = 5.70 × 10−4 | 0.9714 | 2.12 × 10−3 | 0.0444 |
| Henderson and Pabis | A = 0.9963, K = 0.0633 | 0.9908 | 2.46 × 10−4 | 0.0151 | |
| 40 mm | Exponential | C = 0.0360, A = 0.9804, K = 0.0712 | 0.9992 | 5.68 × 10−5 | 0.0071 |
| 25 °C | Lewis | A = 0.0905 | 0.9948 | 4.34 × 10−4 | 0.0206 |
| Page | K = 0.0798, n = 1.0492 | 0.9921 | 4.06 × 10−4 | 0.0192 | |
| 1.8 m/s | Wang and Singh | A = −0.0649, B = 0.0011 | 0.9778 | 1.86 × 10−3 | 0.0409 |
| Henderson and Pabis | A = 1.0249, K = 0.0928 | 0.9953 | 3.949 × 10−4 | 0.0189 | |
| 40 mm | Exponential | C = 0.0225, A = 1.0135, K = 0.0994 | 0.9962 | 3.17 × 10−4 | 0.0165 |
| Air Velocity (m/s) | Thickness (mm) | Temperature/(°C) | Slope | D/(m2·h−1) |
|---|---|---|---|---|
| 1.2 | 40 | 15 | −0.04795 | 8.31 × 10−7 |
| 1.2 | 40 | 25 | −0.06591 | 1.31 × 10−6 |
| 1.2 | 40 | 35 | −0.10742 | 2.13 × 10−6 |
| 1.2 | 20 | 25 | −0.09809 | 6.37 × 10−7 |
| 1.2 | 60 | 25 | −0.05328 | 2.17 × 10−6 |
| 0.6 | 40 | 25 | −0.05435 | 1.08 × 10−6 |
| 1.8 | 40 | 25 | −0.07983 | 1.59 × 10−6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, X.; Kang, X.; Xi, L.; Dou, Q.; Shi, Z. Study on Low Temperature Drying Characteristics of Chicken Manure in a Humid Environment. Appl. Sci. 2023, 13, 7957. https://doi.org/10.3390/app13137957
Li X, Kang X, Xi L, Dou Q, Shi Z. Study on Low Temperature Drying Characteristics of Chicken Manure in a Humid Environment. Applied Sciences. 2023; 13(13):7957. https://doi.org/10.3390/app13137957
Chicago/Turabian StyleLi, Xuanyang, Xiangtao Kang, Lei Xi, Qi Dou, and Zhifang Shi. 2023. "Study on Low Temperature Drying Characteristics of Chicken Manure in a Humid Environment" Applied Sciences 13, no. 13: 7957. https://doi.org/10.3390/app13137957
APA StyleLi, X., Kang, X., Xi, L., Dou, Q., & Shi, Z. (2023). Study on Low Temperature Drying Characteristics of Chicken Manure in a Humid Environment. Applied Sciences, 13(13), 7957. https://doi.org/10.3390/app13137957







