Effect of Pole Head Profile on Torque Performance of a Disc-Type Multipole Magnetorheological Brake

Abstract

A magnetorheological brake (MRB) works on the shearing effect of MR fluid in the magnetic field to develop resistance between the stator and the rotor, which are relative moving surfaces. Researchers achieved high torque volume ratios in magnetorheological fluid braking systems by adding multiple layers and using multipole approaches. Even though several designs already exist, there is a need for innovative ideas and advanced structures of MRBs for practical engineering applications. The number of magnetic poles, pole geometry and placement have a significant effect on the performance of multipole MR brakes. This research focused on the effect of the pole head geometry in a multipole dual disc compact MR brake to understand the relationship between the geometry and its magnetic flux flow. The magnetic field generated by the electromagnetic coil’s orthogonal travel through pole heads, rotor and MR fluid layers results in friction to fulfill the action of braking that achieves maximum brake torque. This flow depends on the magnetic pole head area and corresponding core dimensions. This study considered polygon, square, hexagon, trapezoid and ellipse pole head profiles in place of the conventional circular pole and the best possible core dimensions were used for each geometry. To study the brake’s performance, COMSOL Multiphysics software (version 5.5) commonly used for 3D electromagnetic modeling was used to simulate and examine the magnetic flux intensity for various pole head configurations. Results indicate an improvement compared to previous works, considering the same dimensional limitations and the flux leakage between the adjacent poles being much less. The hexagonal and elliptical pole heads had better magnetic field area, magnetic intensity distribution and higher magnetic flux lines piercing through the MR fluid perpendicularly than the circular and square pole heads. An increment of 3.88% for braking torque performance was obtained, and the braking torque was significantly enhanced.

1. Introduction

Magnetorheological fluids are intelligent smart materials that are controllable, adjustable and vary in their rheological properties (elasticity, viscosity or plasticity) in the application of a magnetic field supplied by a coil current. These fluids can also exhibit fast, strong and reversible changes [1]. Magnetorheological fluids are suspensions of miniature particles, made up of carbonyl iron or powdered iron or iron–cobalt alloy, that are transformed into a solid gel and line up to form chain-like structures in the direction of the magnetic flux paths after a few milliseconds when the magnetic field is applied [2]. Magnetorheological fluids have characteristic properties that enable a quick response time and stiffness, which are directly proportional to the applied magnetic field and also can achieve a wide range of apparent viscosity, which is controllable since the strength of the magnetic field can be varied with the help of an input current to the electromagnet [3,4]. MR fluid holds great potential in electromechanical interfaces such as brakes, where the operative function is in direct shear mode. These smart fluids are confined in a small gap between a rotor and a stator, which are perpendicular to the rotational axis [5]. The magnetic field lines are radially directed to penetrate the MR fluid gap to generate controllable shear stress that causes friction on the rotating disk surfaces, thus generating a retarding torque [6].Giving priority to ensuring magnetorheological brake rapid response, a wide range of research has been carried out by many researchers over the last decades to enhance output braking torque when the increased magnetic field is limited to magnetic saturation in the working area of the fluid.

The magnetorheological brake was initially designed in 2003 by W. H. Li and H. Du with a single disc configuration model, indicating a progressive increase in brake torque with an increase in magnetic field strength [7]. The torque produced in a disc-type brake depends on various parameters such as disc radius, disc thickness and the composition of MR fluid. These parameters affect the shear stress generated that sources friction in the surfaces of the rotating disk while braking due to the change in viscosity under the applied magnetic field [8]. A dual disc design was proposed to achieve high torque density when fluid was inserted between the rotating and fixed discs. Attia et al. studies revealed that MR fluid gains fast braking responses due to exerted shear force acting perpendicularly to the dual rotating discs [9]. Daoming et al. experimented and proved that the multidisc concept can satisfy the requirement of maximum braking torque by showing a good linear relationship under the condition of magnetic saturation [10]. Further, these studies were extended to single pole multiple discs concept in which a model proposed by Huang et al. exhibited advantages of rapid response, magnetic medium characteristics and arrangement structure of the magnetic flux flow [11].

To increase the active area of the MR fluid, the multiple electromagnetic poles concept was introduced in MR brakes after a single electromagnetic pole reached the limits of torque enhancement due to the restriction of the magnetic field’s strength. In 2013, Shiao et al. proposed this novel design and outcomes that explain the increased magnetic flux flow through pole heads [12]. Later on, Shiao et al. introduced a model of six electromagnetic poles with bilayer MR fluid by merging concepts of the multipole and multilayering to study the magnetic field superposition’s influence on the braking torque, and this study revealed that, due to geometric dimensional parameters at each pole head, the working area of the fluid increases and exhibits great enhancement in braking torque and TVR [13]. Wu et al. studied the multipole and dual-gap magnetorheological brake to find the impact of individual input currents on the active energizing surface areas on the rotor disk where the concentrated magnetic flux is generated [14,15].

Axial and radial magnetic flux can influence braking output torque by increasing either the axial length or radial dimension of the rotating disc. Shiao et al. explained that by varying pole head radial distance, the flow of magnetic flux increased effectively and improved brake performance [16]. Structured models of brakes such as single-disk, dual disk, drum and hybrid types by maximizing the braking torque with the torque ratio higher than desired and by minimizing the mass of the brake were carried out by Zhou et al. [17]. With the compactness of the magnetorheological brake facilitating maximum braking torque, Shiao et al. proposed a design with a small radial dimension that results in large torque transmission capacity [18]. Magnets with varying shapes, coil winding gaps and shank dimensions can influence the magnetic flux captured by the stator [19]. In addition to the compact design, Song et al. studied the impact of shaft dimensions that intensify more efficient areas of magnetic flux across the MR fluid [20]. Li et al. discussed the new concept of axial flux in permanent magnets, which are mechanically activated to determine the magnetic field distribution at the midpoint of the shank, and Binyet et al. proposed a modification with built-in permanent magnets that increase the internal magnetic field intensity [21].

Nguyen et al. studied the effect of the shape of the brake envelope such as rectangular, pentagonal and spline shapes to understand the flux flow [22]. Sheth et al. investigated special geometric shapes to the pole heads to gain the maximum torque and also investigated the effect of these pole shapes on the motor [23]. Mehmet et al. explained that different geometric shapes of the pole heads have an impact on motor performance [24]. Until now, none of the studies have focused on the effect of magnetic pole head geometry on an MR brake’s performance. Therefore, this work focuses on investigating the influence of pole geometries such as the shape, area, radial position of the pole head and corresponding shank dimensions on the MR brake’s torque. Conventional pole heads such as circle or square have to be changed to trapezoid, pentagon and hexagonal pole head profiles to increase the working area of the brake. The ellipse pole head profile covers the maximum radial distance on the surface of the brake with a larger pole head area. These shapes also influence the flow of magnetic flux, which simultaneously affects braking torque.

2. Concept of the MR Brake

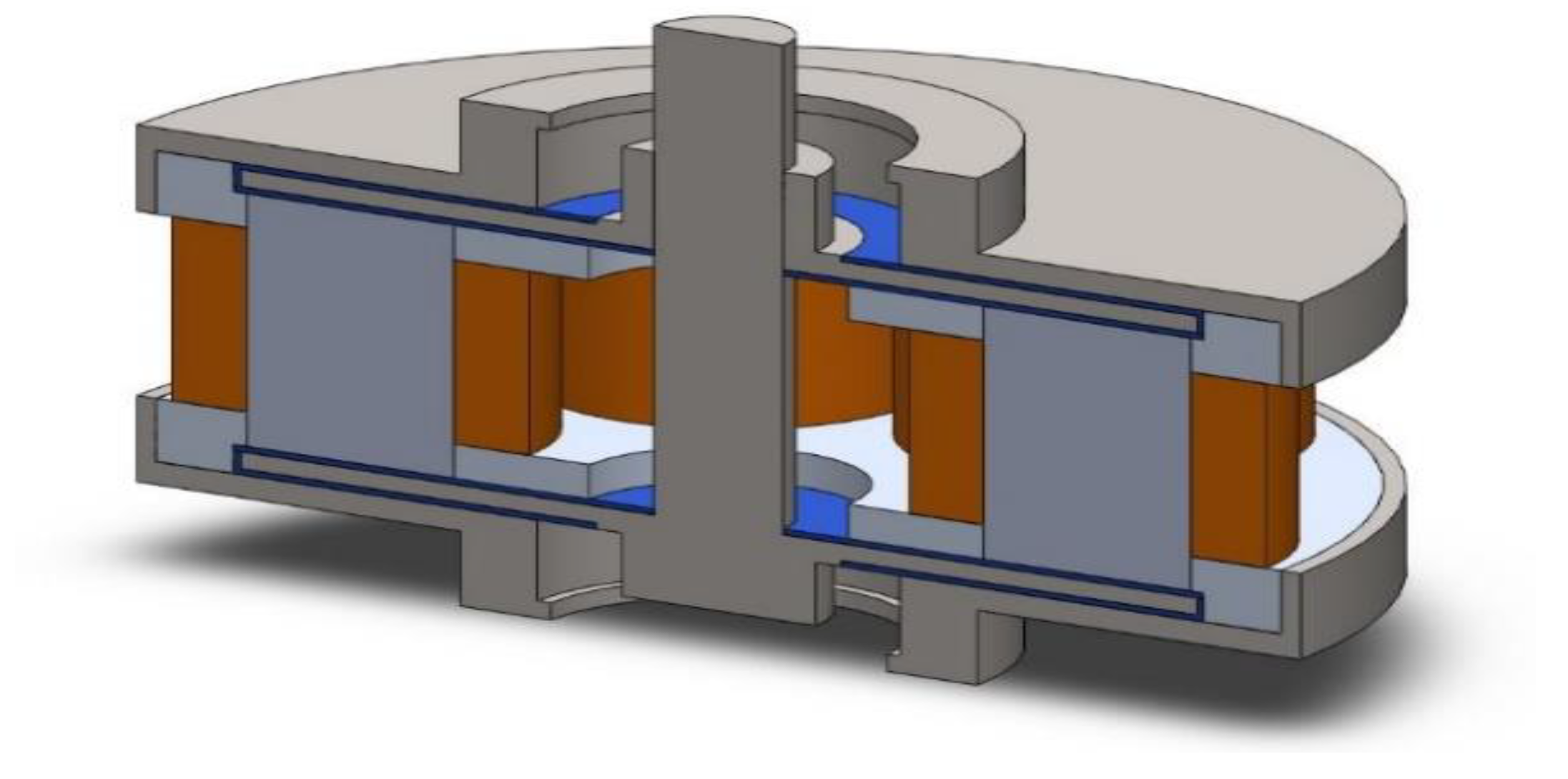
A magnetorheological brake that has a symmetrical structure with a dual disc configuration comprising four magnetorheological fluid layers and six magnetic poles, as shown in Figure 1. The main components include stator cases, rotor discs, pole heads, fluid layers, shank/core, stator caps and outer covers. Optimizing the geometry based on the parameters, referred to in

Table 1. Dimensions of the brake design parameters.

Parameters	Range
Number of poles	6
The gap between the poles	10–14 mm
Pole head radius	7.5–8.5 mm
Shank radius	6.5–8.5 mm
Number of turns of the coil winding	220–290 turns

, influences the output torque. The materials of the components have to be selected based on thermal, structural and magnetic properties.

The working concept of an MR brake is that a larger working area of fluid results in higher total braking torque due to increased shear stress in the MR fluid. To achieve maximum stress, the selection of MR fluid is crucial, and it should have the largest scale of saturation. So, high-quality MR fluid (MRF-140CG) produced by the LORD corporation is used in this magnetorheological brake.

Table 2. Properties of magnetorheological fluid (MRF-140CG).

Properties	MRF-140CG
Viscosity at 40 °C	0.280 Pa-s
Density	3.64 g/cm3
Thermal conductivity	0.28–1.28 W/m°C
Operating temperature	−40 to +130 °C
Yield strength at 200 kA/m	57 kPa

represents properties such as the viscosity, density, conductivity, operating temperatures and yield strength of MR fluid. The larger working area of MR fluid is dependent on the pole head area, which is helpful for generating larger magnetic flux. However, a larger pole head area reduces the coil-winding space and also makes magnetic flux potentially bypass from one pole to its adjacent pole via the MRF. The nonmagnetic stator case was designed for the insertion of magnetic poles in a circular array with an alloy of aluminum.

Six magnetic poles with magnetically permeable cylindrical cores/shanks, as shown in Figure 2a, were used to create a multipole magnetic field. Magnetic cores contain pole heads that are inserted into the holes of the stator case and shank that create the flux cycle between two adjacent cores, as shown in Figure 2b. A higher-pole MR brake does not always achieve higher brake torque because if the gap between the two pole heads is too close, then the available winding space between poles decreases with the increased number of poles. Magnetically permeable rotor discs were placed parallel to the surface of the stator cases and pole heads.

3. Pole Head Geometry

The pole head geometry should be improved to obtain maximum shear stress, which helps in increasing braking torque. The magnetic field induced by the coil winding flows through the shank and reaches the pole head assembled inside the nonmagnetic stator case in the form of flux lines. The pole head can be divided into three regions: the central region, where the pole is responsible for passing the maximum flux; the high field region, where the pole head experiences the maximum flux flow after the central region; and the low field region, which occurs at the edges of the poles where the flux flow is less. A smaller magnetic field allows the flow to drop more rapidly at the edge of the pole heads.

Pole head profiles have to be changed appropriately to enhance the braking torque in compact magnetorheological brake configurations. The effective working area of MR fluid depends on the pole head area: if the area increases, then shear stress developed in the MR fluid increases, resulting in higher braking torque. So, square and trapezoid pole head shapes were considered in this study for larger pole head areas. Magnetic flux flow is another factor that is influenced by the pole head’s area. If the gap between poles is smaller, the flux flow from one pole to the adjacent pole might be bypassed. Considering this aspect, the hexagon and pentagon pole head shapes with larger areas are arranged in a symmetrically linear configuration. The radial distance between the center of the pole to the center of the brake affects the final torque. An ellipse pole head profile is considered to achieve a larger radial distance and also a larger gap between the poles. To obtain higher torque and overcome the bypass of flux flow in this compact design, different geometric pole head profiles—as shown in Figure 3—such as square, trapezoid, pentagon, ellipse, hexagon and circle were analyzed.

For the simulation study, electromagnetic analysis was carried out to calculate the amount of magnetic field generated. It is a flexible platform that allows users to model all relevant physical aspects of any type of design and is used to support the optimization process of torque enhancement. In this analysis, the resulting magnetic field intensity distribution within the magnetorheological brake configuration was analyzed.

The magnetorheological brake can be modeled and evaluated using finite element analysis software like COMSOL, which is commonly used for electromagnetic modeling. To develop a 3D model of a magnetorheological fluid brake, Solid Works was used, and COMSOL Multiphysics was used to calculate magnetic flux with changing pole head shape. One of the benefits of utilizing COMSOL is the material definition feature, which allows us to define the nonlinear connection between magnetic flux and magnetic field, known as the B–H curve.

The surface integration tool helped us to compute the magnetic flux on the core surface and MR fluid, which led us to use a 2D graph that illustrates the magnetic flux density on the disks. The simulation was performed with various numbers of turns in a coil and also by varying the current. The load in the present problem is the current flowing through the coil, responsible for the magnetic flux. Another benefit of COMSOL for accuracy in the output is the ability to modify the size of the elements in the mesh section. After the mesh was generated, as shown in Figure 4, the finite element model (FEM) was solved using a parametric nonlinear solver and the magnetic field’s distribution onto the MR fluid. Once the results of magnetic field distribution were known, the shear stress value was obtained to evaluate the performance of the brake in terms of output torque.

4. Initial Validation of the Results

The proposed multipole magnetorheological brake three-dimensional computer model was validated against the experimental results of the optimized MRB presented previously by Shiao et al. [25]. To compare the performance of the proposed MR brake, the relationship between braking torque and the number of turns of the core’s winding at different input currents was studied. The optimized dimensions of the brake were set accordingly. The number of poles was six, and the gap between the centers of the poles was set to 28.25 mm using 3 mm pole head thickness; the radii of pole head radius and shank radius were 8.5 mm and 8.0 mm, respectively.

Brake torque increases gradually by increasing the number of turns at a maximum input current of 2 Amp. Overall, the experimental results were comparatively similar to the simulation results of the proposed model, whereas the graph, as shown in Figure 5, had slightly lower values in the simulation. The difference observed is due to the viscosity as well as the friction, which was not accurately accounted for in the simulation model.

Since the results are similar comparatively, this research can be further investigated using different pole head geometries to understand their effect on brake torque. So, these results prove that the proposed pole head shapes have different effects. Hence, the results of validation prove that the proposed pole head geometry variation in an MR brake can be investigated for further analysis.

5. Results

Simulation results of the magnetic flux density of a magnetorheological brake were analyzed. Considering the optimized brake parameter range, the present study on varied pole head geometries observes their effect on braking torque. The simulation process for different types of pole head profiles primarily focuses on the best values for pole head radius (varied from 7.5 mm to 8.5 mm), shank dimensions (varied from 7.0 mm to 8.0 mm) and the number of wire turns around the coil that affect output shear force.

As shown in Figure 6, the braking torque for all pole head profiles at 270 wire turns and center of pole radii of 28.5 mm were discussed. The circle profile obtained the best output value of 15.655 N-m at an 8.5 mm pole head radius and 8.0 mm shank radius. The trapezoid profile obtained the best output value of 14.02 N-m using an 8.0 mm pole head radius and 8.0 mm shank radius. The square profile obtained the best output value of 13.45 N-m using an 8.0 mm pole head radius and 8.0 mm shank radius. But, for the 8.5 mm pole head radii, the square shape had a drawback in that it overlapped with the other pole head, since the dimension of the magnetorheological brake studied had a fixed parameter of 38 mm rotor radius. The polygon profile obtained the best output value of 15.36 N-m using an 8.0 mm pole head radius and 8.0 mm shank radius. Similar to the square profile, the polygon pole head of 8.5 mm radii also had a drawback in that it overlapped with the other pole head, since the dimension of the magnetorheological brake studied had a fixed parameter of 38 mm rotor radius. The hexagon profile obtained the best output value of 16.27 N-m at an 8.0 mm pole head radius and 8.0 mm shank radius. In the ellipse profile, due to its shape, the possible pole head radius was 6.3 mm, the shank radius was 6.5 mm, the center of the pole radius was 27 mm and the output braking torque value was 16.09 N-m. Therefore, the obtained results for different pole head profiles were influenced based on different parameters such as effective area of MR fluid, maximum magnetic flux flow, radial dimensions and shank radius.

5.1. Effective Area of MR Fluid

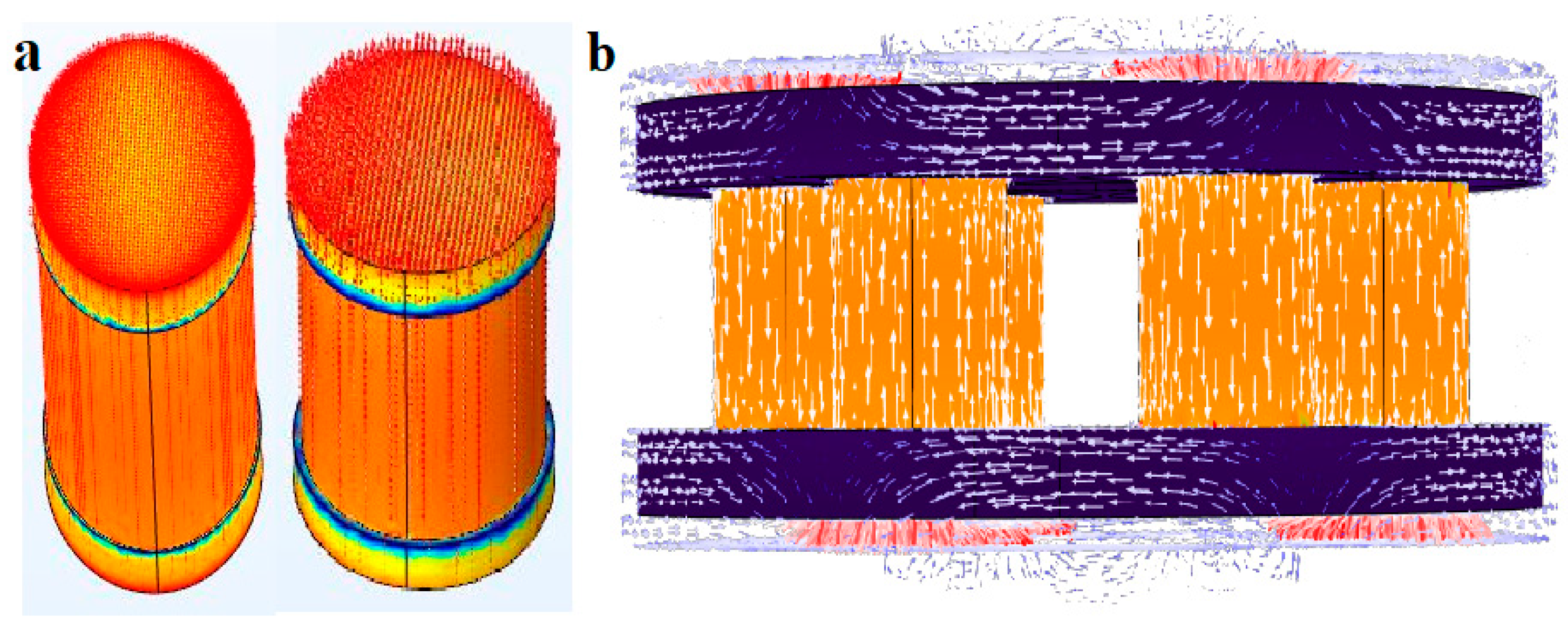
Shear stress developed in the MR fluid depends on the pole head area. The maximum yield shear stress of the fluid was in a controllable active state and it increased on the disc surface, simultaneously increasing the braking torque. The inner layers of the brake, as shown in Figure 7a, had higher stress, which resulted in increased magnetic field strength, and when it passed through the rotor and reached the outer layer (Figure 7b), the stress was significantly less and the flux also dispersed to the surroundings, as shown in the figure. In the upshot of this study, the hexagonal pole head benefitted from higher yield stress compared to other pole head configurations.

5.2. Maximum Magnetic Flux Flow

Poles are the main source of magnetic flux generated in the MRF effective area, which is between the core and rotor disk surface. On the pole head surface area, the produced magnetic flux penetrates orthogonally across the circular disc surface and the stator, which results in creating a yield resistance to produce field torque for the brake. Magnetic flow presents in the form of a loop from one pole to the adjacent pole through the MR gap and stator, and then reverts through the MR gap to the adjacent pole. To increase this flux, more poles are required, but the pole radius has to be reduced due to compactness.

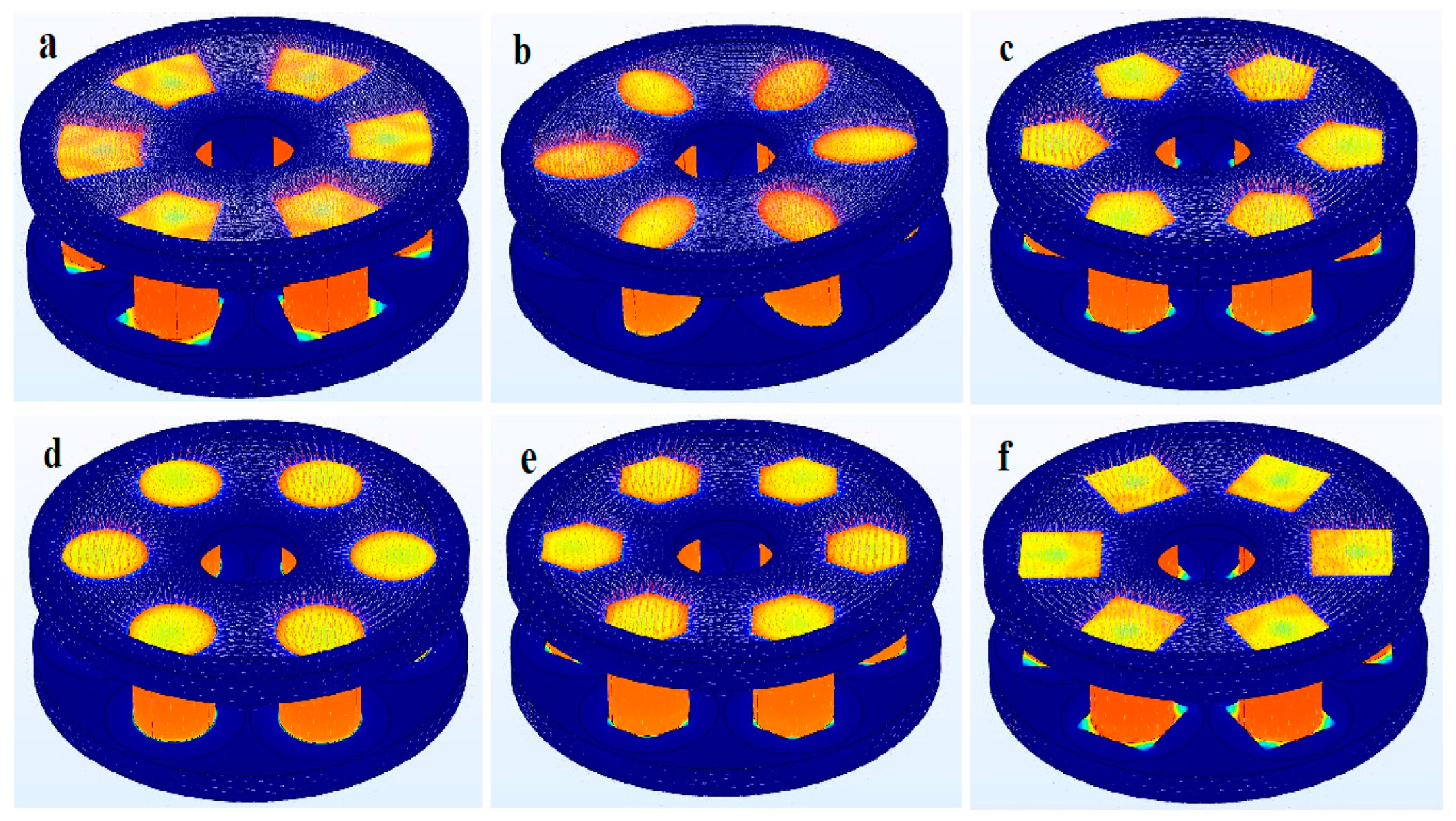
As shown in Figure 8, observations of this study for all pole head profiles explain that at different pole head radii, the density of the flux is distributed equally, indicated by color contours. The red color arrows on the pole head area indicate flux concentration. Higher density flux occurs at the middle distance between the two effective areas. The gaps between those magnetic coils were optimized to prevent magnetic flux leakages as well as to avoid magnetic saturation. It can be seen that the density of the flux is distributed equally, and the white color occurs on the sides of a pole, indicating the effective area that indicates the flux concentration transferring to the adjacent pole. The 90° corner angle of the square reduces the flux flow due to its shape configuration; the distance between the center of the pole head area and the vertex is high, so the concentration of flux varies. The major drawback of using the circle pole head profile is that the shank radius and pole head radius cannot be of the same dimension. The ellipse pole head shape is similar to a circle shape, but it is placed in the radial direction. It is also feasible for manufacturing and also able to flow at a comparatively higher maximum flux than other profiles. At the 120° higher corner angle of the hexagonal shape, the flux flow can travel in more directions from the six vertices. The trapezoid pole head shape assembled in the MRB obtained inferior braking due to its configuration. The distance between the center of the shank and the edges of the pole head was greater, so in this area the flow of flux was less, which simultaneously affected the torque.

5.3. Effect of Coil/Shank Shape and Radius

Brake performance is directly affected by the resistance of the coil based on parameters such as the number of wire turns, applied current and coil/shank dimensions. Increasing the number of wire turns and amount of input current influences the yield stress, and the saturation limits depend on the magnitude of the yield’s shear stress. An approximate number of wire turns is anticipated by the quotient of the coil area and the axial width of the coil. The greater axial width of the coil yields a greater number of turns and thus achieves a higher braking torque. The wire is to be wound precisely so that each strand effectively occupies a total cross-sectional area, which improves braking torque. Torque increment is extremely low once the selected coil material reaches the magnetic saturation point.

Ellipse pole head geometry was designed with an elliptical shank to gain higher yield stress, and for all other geometries, a circular coil was used for analysis. Shank radii varied from 6.5 mm to 8.5 mm, and pole head radii based on the saturation condition from 7.0 to 8.0 mm were preferred; the number of wire turns was calculated based on the axial width of the coil; the gauge of the wire was 0.57 mm at a constant applied current of 2 amperes. From Figure 9, we can observe three magnetic conditions namely, fixed concentration flux, fixed dispersive flux, and fixed total flux, which relate to electric and magnetic fields acting on elliptical and circular coils that are important for understanding the behavior of electromagnetic fields. In Figure 9, (i) represents the fixed concentration flux, where the applied current is 2 A and the lower number of turns results in a lower amount of flux flow; (ii) represents the fixed dispersive flux condition, where the coil current is 2A and the average number of turns, which results in equally distributed flux flow through all the sides of pole head profiles; (iii) is the saturated condition of a coil with 2A coil current and a maximum number of turns, which shows an impact on the flux flow. Higher braking performance was obtained at an optimum coil saturation condition using an 8.0 mm shank radius for the square, trapezoid, circle, pentagon and hexagonal pole heads and using a 6.5 mm shank radius for the ellipse pole head.

5.4. Effect of Radial Geometric Dimension

A magnetorheological brake utilizes both axial and radial magnetic flux to generate braking torque. The rotor of the brake in the proposed work is a dual disc configuration and the MR fluid acts as the force on the disc in a shear mode. In this disc brake, the torque is easily affected by the centrifugal force in a superficial direction. In particular, radial dimensions affect the magnetic field that acts on the disc surface and a very weak magnetic field is exerted axially to both sides of the rotor. Additionally, torque enhancement can be achieved by increasing either the axial or the radial dimensions of the rotor. The extended rotor’s radial dimensions create a greater active area of the fluid, thus producing higher shear stress and, subsequently, higher braking torque.

Design configurations for different pole head geometries varied due to the compactness of the brake. Analysis for each pole head shape was conducted from 27.0 mm to 29.0 mm, which is the maximum radial width to the midpoint of the pole head. As shown in Figure 10, for all pole head configurations, the obtained torque (16.09 N-m) is maximum at a radial width of 27.0 mm, and at 27.5 mm, the torque is (15.73 N-m). For a 28.0 mm radius to the midpoint of the pole head, the obtained torque is (15.34 N-m), which is the maximum. In all the pole head profiles, the maximum output torque (16.27 N-m) was obtained at 28.5 mm. At a higher radial dimension of 29.0 mm, the torque (15.98 N-m) slightly decreased due to the active chain area of MR fluid penetrating the magnetic flux to the outward direction of the brake.

5.5. Comparison of Various Pole Head Profiles for Obtained Braking Torque

Six different pole head profiles of a magnetorheological brake were compared to the shear force value obtained in the analysis to evaluate which profile achieved higher and more effective braking torque. Here, based on the observations, the highest braking torque was obtained using hexagonal pole head geometry and the lowest was obtained using square pole head geometry. An increment of 3.88% for braking torque performance was obtained. The simulation results of the multipole dual disc design show the advantage of making it a high-yield area that achieves high shear force. For different pole head geometries, electromagnetic analysis was carried out to prevent the occurrence of magnetic saturation near the coil, and it can be seen that the magnetic flow direction in all the pole head profiles is tangible as a loop from one pole through the MR gap across the rotor. It is also noteworthy that the elliptical shape shank where the core winding can be wound directly is also convenient in manufacturing. The torque increases nearly linearly with the increase in the number of turns (N) and applied current (I), significantly determining the magnitude of the magnetic field strength.

The square pole head geometry obtained 13.45 N-m, the circle pole head profile obtained 15.65 N-m, the pentagon pole head profile obtained 15.36 N-m, the ellipse pole head profile obtained 16.09 N-m, and the trapezoid pole head profile obtained 14.02 N-m. Comparing all pole head profiles, the output braking torque is depicted in Figure 11, showing that a larger corner angle (hexagon) can produce high magnetic flux density, which helps in torque improvement. The obtained braking torque was 16.27 N-m for a hexagonal profile, which is an increment when compared with other pole head geometries.

6. Conclusions

This research paper reported the electromagnetic simulation outcomes on compact multipole dual disc magnetorheological brakes by varying pole head profiles. From the analysis, it can be concluded that the maximum shear stress of the fluid is in the controllable active state for the hexagonal pole head and benefits from the increased magnetic field strength compared to other pole head configurations. The magnetic flux flow for all the pole head profiles provides good correlation with the torque generated. The magnetorheological fluid effective area of a hexagonal shape form as a loop of magnetic flux from one pole to the adjacent pole and a higher rate of flux flow can travel in all directions of a pole. Torque enhancement was achieved at 28.5 mm radial dimensions that create a greater active area of the fluid. The fixed dispersive flux condition affects the resistance of the coil based on parameters such as greater axial width of the coil and a greater number of turns, resulting in equally distributed flux flow and higher braking performance at optimum coil saturation. Six different pole head geometries (pentagon, square, hexagonal, circle, ellipse and trapezoid) were analyzed, wherein the hexagon profile resulted in a high shear force value, and the square profile had the lowest output. An increment of 3.88% for braking torque performance was obtained using the hexagonal pole head profile and the braking torque was significantly enhanced.