Construction and Application of Fuzzy Comprehensive Evaluation Model for Rockburst Based on Microseismic Monitoring

Abstract

Based on the relationship between rockburst and microseismic event indicators, this study proposes that the risk of rockburst in mine working faces, roadways, and even the entire mine should be studied through the “double high” risk evaluation of microseismic events. The 43 evaluation indexes of rockburst were optimized into eight indexes by using the expert scoring method. Considering the eight indexes as the basic events and the “double high” risk of microseismic events as the top event, the “double high” accident tree of microseismic events was established. According to the qualitative analysis results of the accident tree, the microseismic activity evaluation index was determined, and the “double high” risk evaluation index system was constructed for microseismic events. The system included three first-level indicators and eight second-level indicators. The fuzzy hierarchical comprehensive evaluation model was used to evaluate the “double high” risk of microseismic events in the Yanbei Coal Mine. In this paper, a microseismic monitoring and evaluation index model is constructed to simplify the existing evaluation system, which is convenient to effectively establish the connection between microseismic monitoring data and rockburst index and provide important theoretical support for underground monitoring and rockburst prevention.

1. Introduction

Underground impact pressure has become one of the main disasters restricting mine production and safety in China [1]. Therefore, it is of great importance to research underground shock pressure prediction, risk evaluation, and prevention and control measures to ensure the safe mining of coal resources [2,3,4]. Scholars in China and abroad have conducted more research on the risk assessment of rockbursts [5,6,7,8].

Prediction is the key to the prevention and control of underground shock pressure. At present, the main forecasting methods include the rock mechanics method represented by the drilling cuttings method, geophysical methods represented by microseismic and electromagnetic radiation and acoustic emission, the empirical analogy method represented by the comprehensive index method, and the dynamic method of surrounding rock represented by the dynamic method of roof [9,10,11]. The microseismic monitoring system is a regional monitoring method, which can automatically record the microseismic activity and provide a basis for rockburst risk evaluation by real-time calculation of microseismic position and energy [12,13]. The principle is to utilize the different times to reach the starting point of the P wave received by the underground seismic substation to perform two-dimensional and three-dimensional positioning. The other aims are to determine the location, energy, and magnitude of the vibration damage, mark it on the excavation engineering plan, and quickly report it to the production command system. Microseismic monitoring has become an important means of forecasting rockbursts, and it has great development prospects [14]. Currently, countries such as Poland, South Africa, and Canada have established national mine vibration monitoring networks, which are broadly employed in the prediction of rockbursts [15,16].

In safety evaluation, also known as risk evaluation, the principles and methods of safety system engineering are used to comprehensively evaluate and predict the possible risks and possible consequences of the proposed or existing projects and systems. The evaluation is performed based on the size of the mine impact accident risk and the proposed safety countermeasures to achieve the goal of engineering and system safety [17,18,19,20]. At present, coal mine safety evaluation methods used outside China mainly include evaluation technology based on mine risk evaluation, the safety index method, the coal mine operation safety evaluation method, and the human-machine based safety evaluation method [21,22,23,24].

In the early 1980s, safety system engineering was introduced in China, and safety evaluation was highly valued by many enterprises and management departments [25]. By applying the principles of foreign safety evaluation methods, relevant companies in the machinery, aviation, aerospace, metallurgy, and other industries have developed many safety evaluation methods [26,27] such as safety checklist (SCA), fault tree analysis (FTA), event tree analysis (ETA), fault type and impact analysis (FMEA), hazard and operability study (HAZOP), pre-hazard analysis (PHA), and the work environment hazard assessment method (ELC) [28]. In 1988, the former Ministry of Machinery and Electronics promulgated China’s first safety evaluation standard named “Safety Evaluation Standard for Machinery Plants”, which has since been revised and applied to more than 1000 enterprises in China. Subsequently, China’s pharmaceutical industry, aerospace industry, the conventional weapons industry, petrochemical enterprises, electronic enterprises, and other departments have put forward their own safety evaluation standards to strictly regulate enterprises to carry out safety evaluation.

In recent years, with the continuous promotion and application of safety system engineering in coal systems, the research on the safety evaluation of coal mines has attracted the attention of more and more Chinese scholars, and many research results have been achieved. In this regard, the three-step coal mine accident safety evaluation method (which is a branch of the index method), the accident tree method and safety countermeasures, the comprehensive evaluation index method of coal mine safety production status, and the gray system method were put forward successively. These achievements greatly promoted the application of safety evaluation in the coal industry. However, Chinese scholars have mainly focused on the rockburst mechanism, monitoring and forecasting methods, and prevention and control measures. There is little discussion on the risk of rockburst, and a systematic safety evaluation method of rockburst has not been formed so far [29,30,31,32]. In the past, some scholars put forward experience category analysis, methods of drilling cuttings, and methods of determination of moisture content to perform the relevant evaluation. These methods have attained some applications; however, they are often only static index reflecting methods that cannot dynamically reflect the influence of the various indicators of evaluation results of percussive ground pressure. Thus, the results of these methods are accompanied by certain errors, affecting the scientific decisions of coal enterprises [33]. The mechanism of rockburst is very complicated, and there are many influencing factors. It is necessary to choose a reasonable evaluation method to make an effective comprehensive evaluation of the various factors of shock hazard. This is needed for correct measuring of the degree of shock hazard and for providing a reference for the formulation of prevention and relief measures [34,35].

In this paper, an innovative and reasonable evaluation method is proposed to solve the problem of the imperfect evaluation method of coal mine safety, especially in the aspect of rockburst risk evaluation, aiming at the effective comprehensive evaluation of various factors of impact hazards. This method introduces the concept of dynamic evaluation and reflects the changing trend of rockburst risk in time by establishing a dynamic evaluation system, so as to improve the accuracy and comprehensiveness of the evaluation method. Compared with the traditional evaluation method, which can only reflect the method statically, this method can comprehensively consider the influence of various factors on the evaluation results of rockburst, making the evaluation results more reliable. Based on the concept of shock pressure, this paper discusses the relationship between microseismic events and their energy and frequency, and introduces the “double high” phenomenon as the top event. Each evaluation index was considered as the basic or intermediate events. Furthermore, the accident tree, the analytic hierarchy process, and the fuzzy comprehensive evaluation model were adopted to determine the weight of each evaluation index. The fuzzy comprehensive evaluation method was used to evaluate each index comprehensively, to divide the danger level, to put forward the safety response measures, and to evaluate the effectiveness of prevention and control measures.

The existing impact risk evaluation methods mostly include the empirical analogy analysis method, method of drilling cuttings, and acoustic emission method [36]. The common disadvantage of these methods is that a single index is employed as the impact risk evaluation index, which may bring significant errors to the impact risk evaluation results and thus can affect the safety decisions of coal mining enterprises [37]. Therefore, it is necessary to comprehensively consider the impact factors of rockburst and to apply the relevant theories of a comprehensive evaluation to establish a comprehensive evaluation model for rockburst risk. Through the evaluation of the risk during which the energy and frequency of microseismic monitoring increased at the same time, this research analyzed the factors that led to the occurrence of rockburst. These factors are used as the basis for the design of rockburst prevention measures to eliminate or reduce the risk of rockburst and to ensure the safety of mine production [38].

2. Evaluation Index System of Rockburst Based on Microseismic Events

2.1. Principles for the Establishment of Index System

The complexity and variety of influencing factors of microearthquakes necessitate a comprehensive consideration in hazard assessment. To evaluate the risk of “double high” energy and frequency of microseismic events and quantify the hazardous degree of rock breakup, an appropriate safety assessment method must be selected [39]. This requires the establishment of an evaluation index system based on several principles. Firstly, the system should reflect the objective reality and essence of microseismicity changes scientifically and objectively. Subjective and objective factors need to be considered, ensuring a balance between theory and experience. Secondly, the evaluation method and system should form a harmonious whole, providing reliable and comprehensive data and information. This includes considering objectivity, integrity, hierarchical structure, correlation, practicability, and comprehensiveness [40]. Thirdly, wherever possible, factors should be quantified to reveal the underlying causes of microseismic activity changes. Moreover, the inclusion of stable factors and exclusion of those heavily influenced by chance is necessary to maintain the stability of the evaluation index system. Additionally, the system should be designed with accessibility in mind, allowing for the examination and evaluation of the dangerous situation of rockburst and the implementation of effective risk elimination measures [41,42]. Lastly, the evaluation factor system should be feasible, facilitating data collection, simplifying procedures, and avoiding unnecessary complexity, thus ensuring easy acceptance and implementation by enterprises. Through adherence to these principles, a robust and practical evaluation index system can be established.

2.2. Determination of Evaluation Indexes

Accident tree analysis (ATA), derived from fault tree analysis (FTA), is the most widely used analysis method in safety system engineering. This paper analyzes the risk of rockburst accidents caused by rockburst in the process of microseismic monitoring. According to the principle of microseismic monitoring, various factors related to rockburst are considered comprehensively, and the rockburst events involving 3 intermediate events and 8 basic events are determined according to the experts’ qualitative evaluation.

Table 1. Event sheet of coal mine rockburst accident tree.

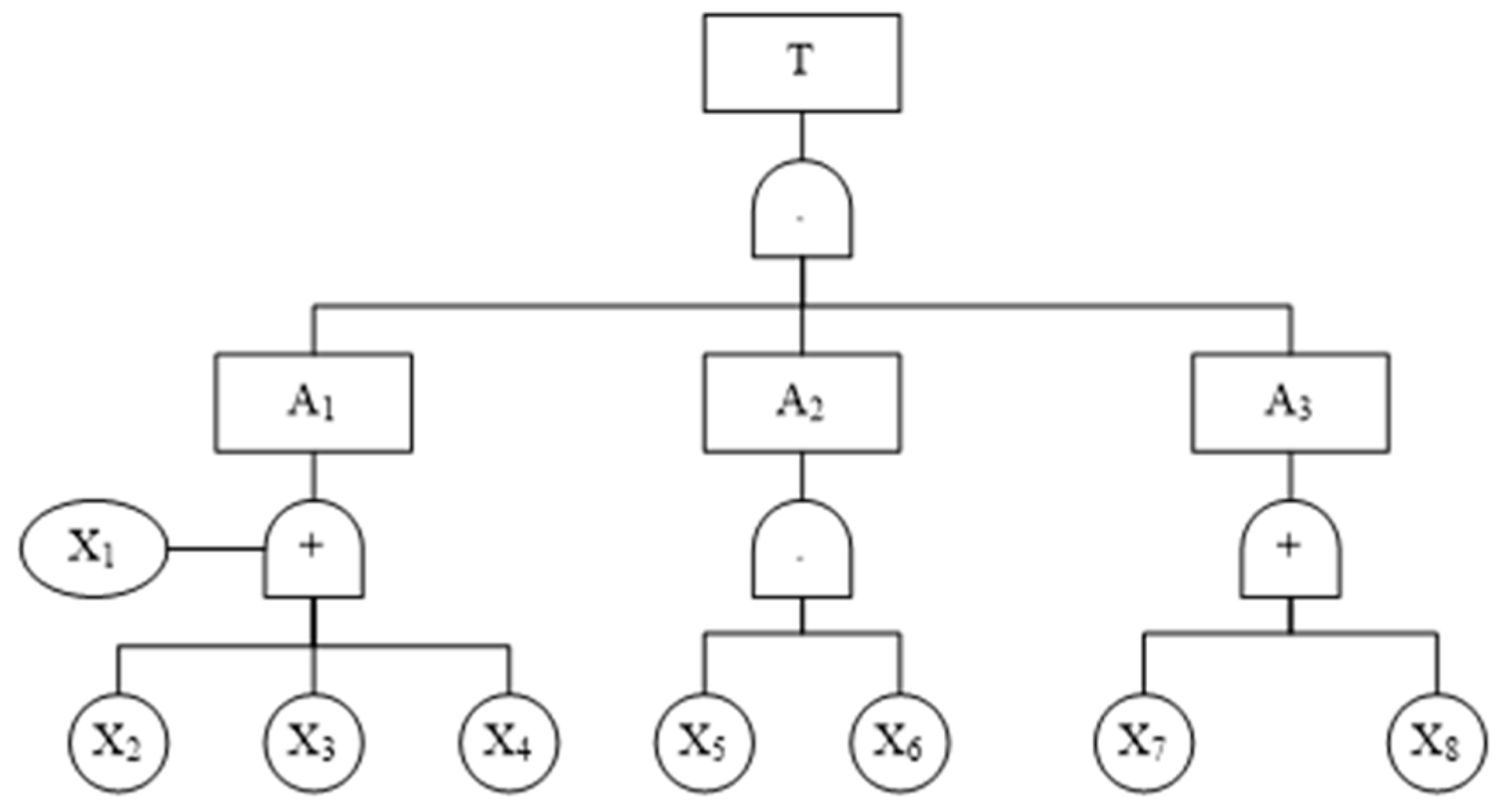
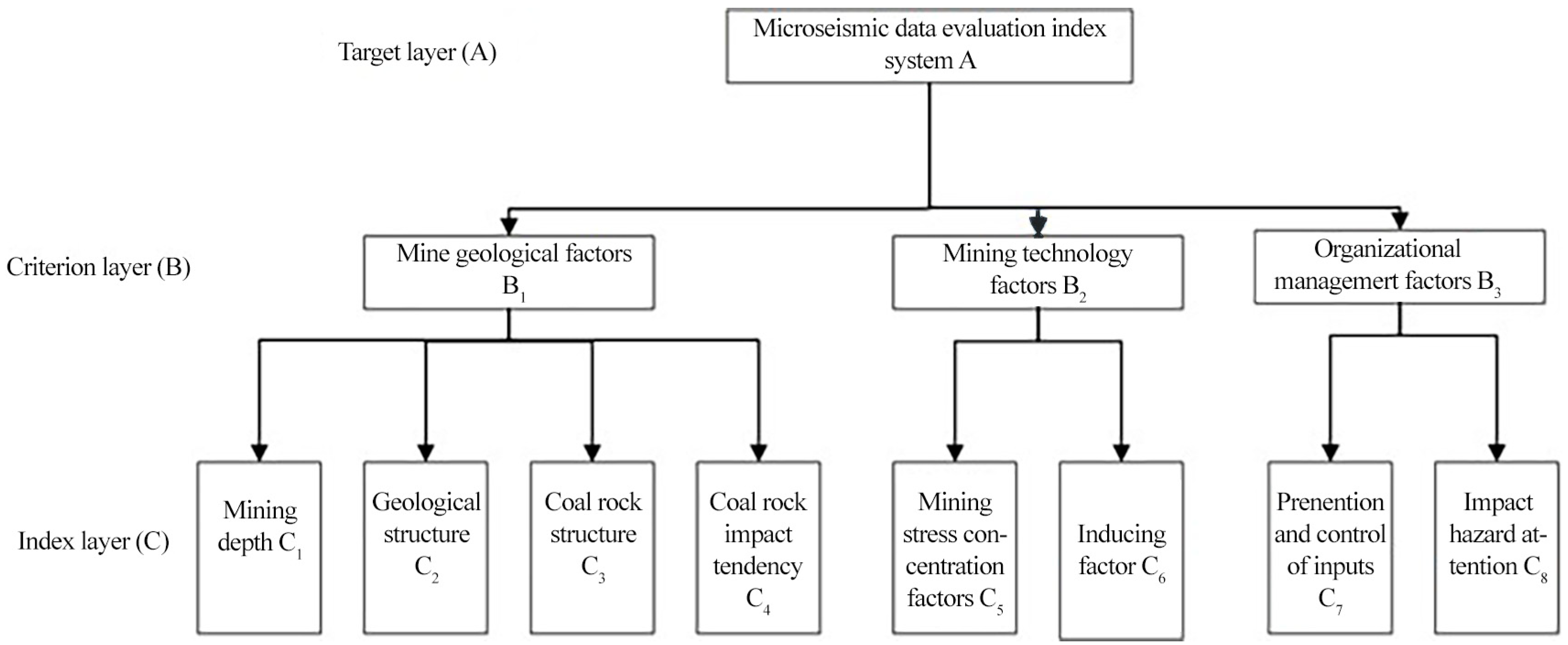
Label	Event	Label	Event
T	“Double high” energy and frequency of microseismic events	X3	Geological structure
A1	Geological factor of mine	X4	Coal and rock structure
A2	Technical factor of production	X5	Mining stress concentration factor
A3	Organizational management factor	X6	Inducing factors
X1	The bursting liability of coal rock	X7	Control input factor
X2	Mining depth	X8	Impact risk attention

shows the relevant code and name comparisons. An accident tree model with rockburst accident T as the top-level event is established.

To evaluate rockburst risk, identifying its impact factors or evaluation indexes is crucial. In Section 2.1, we analyzed these factors and summarized the evaluation indexes. Considering their distinct attributes, the indexes were categorized into three groups: mine geological factors, mining technology factors, and organization and management factors. Numerous indicators influence rockburst, with multiple indicators falling into each category. However, directly evaluating rockburst with these indicators would be overly complex, as some indicators may have similar meanings, explaining the same issue from different perspectives. For instance, indicators like the bursting energy index, elastic energy index, dynamic failure time, and unidirectional compressive strength of coal are all utilized to illustrate the susceptibility of coal rock to bursting [43].

Taking the “double high” risk of microseismic event as the top event, and the event leading to increased microseismicity (i.e., ground burst evaluation index) as the intermediate event and the basic event (Table 1), the “double high” accident tree of microseismic event energy and frequency was established (Figure 1).

(1) Expression of accident tree structure function

The minimum cut set reflects the danger of the system in the accident tree, and each minimum cut set is the set that causes the top event to occur. The more the minimum cut sets of the accident tree, the more ways the top accident occurs, and the greater the risk of the system accident. According to Figure 1, an accident tree model with the “double high” energy and frequency of microseismic events as the top event is established. Then, the structure function expression of the accident tree is as follows:

T = A₁·A₂·A₃ = X₁·(X₂ + X₃ + X₄)·(X₅·X₆)·(X₇ + X₈) = X₁·X₂·X₅·X₆·X₇ + X₁·X₃·X₅·X₆·X₇ + X₁·X₄·X₅·X₆·X₇ + X₁·X₂·X₅·X₆·X₈ + X₁·X₃·X₅·X₆·X₈ + X₁·X₄·X₅·X₆·X₈

(1)

(2) Determination of the minimum cut set

According to Equation (1), six kinds of minimum cut sets of the accident tree of injury accidents introduced by the “double high” energy and frequency change of microseismic events are calculated, that is, there are six kinds of possibilities leading to the occurrence of the top accident. The Boolean algebraic calculation result of the minimum cut set (K_i) of the accident tree is as follows:

K₁ = {X₁, X₂, X₅, X₆, X₇}; K₂ = {X₁, X₃, X₅, X₆, X₇}; K₃ = {X₁, X₄, X₅, X₆, X₇}; K₄ = {X₁, X₂, X₅, X₆, X₈}; K₅ = {X₁, X₃, X₅, X₆, X₈}; K₆ = {X₁, X₄, X₅, X₆, X₈}

(2)

(3) Analysis of structural importance

The importance degree of each basic event in the accident tree structure is called structural importance. The influence degree of basic events on top events can be analyzed through the structural importance degree, which provides important information for enhancing system security.

Using the minimum cut set to solve the structural importance coefficient can be approximated by the following three formulas:

$$I_{\varphi (i)}=\frac{1}{K}\cdot {\displaystyle \sum }_{j=1}^K\frac{1}{n_j}(j\in K_j)$$

(3)

$$I_{\varphi (i)}={\displaystyle \sum }_{x_i\in K_j}\frac{1}{2^{n_j-1}}$$

(4)

$$\begin{array}{r}\hfill l_{\mathsf{\Phi }(i)}=1-{\displaystyle {\displaystyle \prod }_{x_i\in K_j}}\left(1-\frac{1}{2^{n_j-1}}\right)\end{array}$$

(5)

In this formula, $I_{\mathsf{\Phi }\left(i\right)}$ = the i-th fundamental event structure importance coefficient, the larger the $I_{\mathsf{\Phi }\left(i\right)}$ value, the greater the importance. K = minimum total number of cut sets and n_j = the number of fundamental events in the j-th minimum cut set P_j.

Formula (5) has the highest accuracy and is calculated. The approximate value of the importance coefficient of each basic event structure in the minimum cut set is as follows:

The basic events X₁, X₅, and X₆ are all in K₁–K₅, then:

$$\begin{array}{r}\hfill I(1)=I(5)=I(6)=1-\left(1-\frac{1}{2^4}\right)\left(1-\frac{1}{2^4}\right)\left(1-\frac{1}{2^4}\right)\left(1-\frac{1}{2^4}\right)\left(1-\frac{1}{2^4}\right)=0.2758\end{array}$$

The basic events X₂ in K₁, K₄, then:

$$\begin{array}{r}\hfill I(2)=1-\left(1-\frac{1}{2^4}\right)\left(1-\frac{1}{2^4}\right)=0.1211\end{array}$$

The basic events X₃ in K₂, K₅, then:

$$I(3)=1-\left(1-\frac{1}{2^4}\right)\left(1-\frac{1}{2^4}\right)=0.1211$$

The basic events X₄ in K₃, K₆, then:

$$I(4)=1-\left(1-\frac{1}{2^4}\right)\left(1-\frac{1}{2^4}\right)=0.1211$$

The basic events X₇ in K₁, K₂, K₃, then:

$$I(7)=1-\left(1-\frac{1}{2^4}\right)\left(1-\frac{1}{2^4}\right)\left(1-\frac{1}{2^4}\right)=0.1760$$

The basic events X₈ in K₄, K₅, K₆, then:

$$\begin{array}{r}\hfill I(8)=1-\left(1-\frac{1}{2^4}\right)\left(1-\frac{1}{2^4}\right)\left(1-\frac{1}{2^4}\right)=0.1760\end{array}$$

According to the criterion of structural importance degree, the order of the importance degree of the cut set of basic events can be expressed as follows:

$$I(1)=I(5)=I(6)>I(7)=I(8)>I(2)=I(3)=I(4)$$

As can be observed from the “double high” accident tree diagram of microseismic events, there were 24 logic gates in the accident tree, including 2 logic or gates and 22 logic and gates. Moreover, each basic event exists easily; thus, the “double high” microseismic events occur easily in coal mines. Furthermore, according to the calculation results of the minimum cut set, there were six minimum cut sets, which were evenly distributed, and each minimum cut set had five basic events. From the perspective of structural importance, the bursting liability of coal rock, mining stress concentration factor, and inductive factor were the largest, indicating that these factors had the greatest influence on the “double high” microseismic event accident. The second structural importance was the attention to the prevention and control of input and impact risk, suggesting that the organization and management factors were indispensable factors for the phenomenon of “double high” energy and frequency of microseismic events. Ultimately, the three geological factors of the mining depth, geological structure, and coal rock structure were also very important.

2.3. Establishment of Evaluation Index System

According to the above analysis, the first layer structure of the microseismic “double high” risk evaluation index system included mine geological factors, mining technology factors, and organization and management factors (Figure 2). In addition, according to the established principles of the evaluation system in Section 2.2, the method of combining system analysis and analytic hierarchy process was employed to refine each major category of factors, and all qualitative factors and quantitative factors were described to meet the information and data processing requirements required by the follow-up evaluation model. According to this principle, each category was divided, and the second layer structure of microseismic “double high” risk evaluation index system was established. The detailed evaluation indexes are shown in Figure 2.

3. Fuzzy Hierarchical Comprehensive Evaluation Model of Rockburst

3.1. Establishment of Analytic Hierarchy Process and Weight Calculation

From the evaluation index system determined by the accident tree, the meanings and manifestations of indexes were different, and there was no comparability and comprehensibility among indexes. Therefore, quantitative indexes must be dimensionless, that is, indexes with different properties and dimensions must be transformed into a relative number (quantitative value) that can be integrated [44]. The essence of dimensionless index processing in the fuzzy mathematics method is to solve the fuzzy membership function in a certain interval. Correct determination of membership function is the basis of applying fuzzy set theory to solve practical problems. The membership function is a quantitative description of a fuzzy concept, which directly affects the quality of evaluation results and determines the scientific nature and accuracy of evaluation results [45].

The determination of index weight is the basis of the “double high” evaluation of microseismic events. The index weight measures the relative importance of the evaluation index in the evaluation system, and the index weight has a very important influence on the final evaluation result. The establishment of a scientific and effective weight system of the evaluation index is an extremely critical step in the evaluation and an important guarantee for the scientific evaluation of the “double high” risk of microseismic events [46]. This study utilized the analytic hierarchy process to determine the weight of the index.

By comparing the indicators of the “double high” risk evaluation system of microseismic events established in Section 2.3, a judgment matrix A = (AIJ) was constructed, and then the weight of each indicator was calculated. The methods for calculating weight vector and characteristic root include the root method and the sum-product method. In this research, the sum-product method was chosen to calculate the weight and characteristic vector of the matrix. The calculation process included the following four steps [47]:

(1) The judgment matrix was normalized according to columns:

$$\overline{b_{ij}}=\frac{b_{ij}}{{{\displaystyle \sum }}_{i=1}^n{b}_{ij}}(\mathrm{i},\mathrm{j}=1,2,\dots ,\mathrm{n})$$

(6)

(2) The normalized judgment matrix was added according to rows:

$$W_i={\displaystyle {\displaystyle \sum }_{j=1}^n}\overline{b_{ij}}(\mathrm{i},\mathrm{j}=1,2,\dots ,\mathrm{n})$$

(7)

(3) Vector normalization was performed using the following equation:

$$W_i={\displaystyle {\displaystyle \sum }_{j=1}^n}\overline{b_{ij}}(\mathrm{i},\mathrm{j}=1,2,\dots ,\mathrm{n})$$

(8)

$W={(W_1,W_2,\cdots \hspace{0.17em},W_n)}^T$ is the eigenvector.

(4) The maximum eigen root was calculated through the following equation:

$${\lambda }_{\max }={\displaystyle \sum }_{i=1}^n\frac{{(AW)}_i}{n{W}_i}$$

(9)

${\left(AW\right)}_i$ is the itch element of the vector AW.

Thus, once we have the equation of ${\lambda }_{\max }$, the consistency test is needed to maintain the consistency of evaluators’ thinking logic on multi-factor evaluation and to avoid contradictory results, which is also a necessary condition to ensure the reliability of evaluation conclusions. When the judgments are completely consistent, ${\lambda }_{\max }=\mathrm{n}$, the remaining characteristic roots are zero, and the consistency index (CI) is defined as follows:

$$CI=\frac{{\lambda }_{\max }-n}{n}$$

(10)

When it is inconsistent, the ratio of the consistency index CI of the judgment matrix to the average random consistency index RI of the same order ($CR=\frac{CI}{RI}$) is generally used as the standard to evaluate whether the consistency of the matrix is acceptable.

When the order is 1 and 2, the judgment matrix is always completely consistent. When the order is greater than 2, if CR < 0.1, the consistency of the judgment matrix is considered acceptable; otherwise, it is necessary to adjust the judgment matrix to cause it to have satisfactory consistency [47].

3.2. Mathematical Model of the Fuzzy Level Comprehensive Evaluation

According to different needs, a variety of fuzzy comprehensive evaluation models can be proposed, but all of them are given by different synthetic operations between the weight vector A and the single-factor evaluation matrix R.

Table 2. Mathematical model of the fuzzy comprehensive evaluation.

Serial Number	Type	Meaning
1	Principal determinant type M (∧, ∨)	∧ means taking a small operation, ∨ means taking large operation
2	The main factor is highlighted as Ⅰ type M (·, ∨)	· stands for ordinary multiplication operation, ∨ means taking large operation
3	The main factor is highlighted as Ⅱ type M (∧, ⊕)	∧ means taking a small operation, ⊕ means sum with an upper limit of one, namely: x⊕y = min(1,x + y)
4	Weighted mean type M (·, +)	· is normal multiplication, and + is a normal addition

shows four basic methods of common fuzzy relation synthesis operations [48].

For the same object set, based on the basic algorithm of the fuzzy comprehensive evaluation model, different mathematical models are used to calculate the sorting results that may be different. This is in line with the objective reality, because if we observe and analyze the same thing from different angles, different conclusions may be achieved. Model 4 is more accurate and suitable for a comprehensive evaluation because it considers overall factors, while model 1, model 2, and model 3 are rough and suitable for a comprehensive evaluation that focuses on major factors. Therefore, the weighted average M (·, +) model was selected for the mathematical model between the weight vector and the single-factor evaluation matrix in the risk evaluation of “double high” microseismic events. In this regard, the basic steps were as follows.

Step 1: Determine the evaluation index set and evaluation set. Factor set U = (mine geological factor (U1), mining technology factor (U2), organization and management factor (U3)), U1 = (mining depth (C1), geological structure (C2), coal rock structure (C3), coal rockburst liability (C4)), U2 = (stress concentration (C1), mining induction (C2)), U3 = (insufficient prevention and control input (C1), underestimated impact risk (C2)), and evaluation set V = (no impact risk, medium impact risk, strong impact risk).

Step 2: Set up the weight distribution vector of m evaluation factors, and use the analytic hierarchy process to get the weight of each level factor.

Step 3: Perform a single-factor fuzzy evaluation:

$$\underset{¯}{X}=[\begin{array}{c}{B}_1\\ B_2\\ ⋮ \\ B_n\end{array}]=\left[\begin{array}{cccc}{b}_{11}& b_{12}& \cdots & b_{1m}\\ b_{21}& b_{22}& \cdots & b_{2m}\\ ⋮ & ⋮ & & ⋮ \\ b_{n1}& b_{n2}& \cdots & b_{nm}\end{array}\right]$$

(11)

A comprehensive evaluation matrix R was established for each evaluation object. The R_i = (r_i1, r_i2, r_i3…, r_in) is the single-factor evaluation of the i-th factor u_i; thus, it represents the frequency distribution of the i(1 ≤ i ≤ m) index u_i of the r_ij on the j (1 ≤ j ≤ n) components v_j, which is generally normalized to satisfy ${\displaystyle {\displaystyle \sum }_{j=1}^n}{\mathrm{r}}_{ij}=1$.

Step 4: Carry out a fuzzy comprehensive evaluation. The result of the fuzzy comprehensive evaluation was obtained by compound operation of a single factor:

$$B=A•R=A•\left[\begin{array}{c}{B}_1\\ B_2\\ ⋮\\ B_n\end{array}\right]=(a_{k1},a_{k2},\dots ,a_{kn})•\left[\begin{array}{cccc}{b}_{11}& b_{12}& \cdots & b_{1m}\\ b_{21}& b_{22}& \cdots & b_{2m}\\ ⋮& ⋮& & ⋮\\ b_{n1}& b_{n2}& \cdots & b_{nm}\end{array}\right]=(b_1,b_2,\cdots \hspace{0.17em},b_m)$$

(12)

4. Fuzzy Hierarchical Comprehensive Evaluation of Impact Risk in Yanbei Coal Mine

4.1. Mine Overview

Yanbei Coal Mine is the backbone mine of Gansu Province Pingliang Huating Coal Industry Group, Huating coal power Co., LTDwith an annual output of 6 million tons. The mine field is located in the middle of Huating Coal Field, with geographical coordinates between 106°26′17″–106°39′59″ east longitude and 35°13′16″–35°17′33″ north latitude. The mining line is 8 km long. The tilt width is 0.6–3.5 km, and the mine field area is 12 square kilometers. Geological reserves of the mine are 634 million tons, recoverable reserves are 357 million tons, and the main coal seam is mined in the coal seam No. 5 with a dip angle of 3–51°. The average coal thickness of the mine is 46 m, which belongs to the coexistence of a gently inclined and steep inclined extra-thick coal seam.

The surface elevation of 250204 working face is +1501 m–+1606 m, the working face elevation is +1013.2 m–+1116.6 m, and the mining depth is 440–480 m. The north of the 250204 working face is located in the west wing of the syncline, which is arranged along the strike, with an inclination of 13°–16°. The southern part of the working face is the anticlinal axis, the north is high and the south is low, and the coal seam direction turns to 180°. The seam floor is developed along the strike of the secondary fold, and the floor is undulating. There are no faults, magma intrusions, or other geological structures in the working face. The five layers of coal that are mined in Yanbei Coal Mine have high brittleness and hardness, which can bear large compressive stress and have the basic characteristics of a dangerous coal seam prone to rockburst. The basic roof thickness of the fifth coal layer is 5–18 m, and it is composed of hard siltstone. The basic bottom thickness is 6.5–19 m, and it is composed of hard medium-to-coarse sandstone with strong impact risk [49].

4.2. AHP Calculation of Index Weight in Index Layer

The scaling method of 1–9 and its reciprocal was adopted for the value of the elements of the judgment matrix, and the ratio of the two elements was used [47] as follows:

$$y_{\mathrm{ij}}=\frac{x_i}{x_j}$$

(13)

$x_i$: the weight or score value of the i-th factor (indicator) affecting the energy and frequency of microseismic events; $x_j$: the weight or score value of the j-th factor (index) affecting the energy and frequency of microseismic events; $y_{ij}$: the element value of the judgment matrix.

The element value calculated by Equation (13) is rounded. When rounded, the value is only rounded, but the maximum value cannot exceed 9. If it is expressed by a fraction and its denominator is the reciprocal of $y_{ij}<1$, take the whole, and only enter when taking the whole. According to the above method, the scale weight (scoring value) of three indexes is evaluated, which is used to determine the importance of the evaluation index, and then build the judgment matrix, as shown in

Table 3. Judgment matrix of evaluation index of the criterion layer.

Judgment Matrix	B1	B2	B3
B1	1	2	4
B2	1/2	1	2
B3	1/4	1/2	1

.

The “sum-product method” introduced already was used to calculate the weight of factors, and the weight of factors was obtained using Equations (6)–(10), as follows.

(1) Normalize the evaluation index judgment matrix of the criterion layer according to Equation (6):

$${\displaystyle \sum }_{i=1}^n{b}_{i1}=1+\frac{1}{2}+\frac{1}{4}=1.750\hspace{1em}\hspace{1em}\hspace{1em}{\overline{b}}_{11}=\frac{b_{11}}{{{\displaystyle \sum }}_{i=1}^n{b}_{i1}}=\frac{1}{1.750}=0.571$$

$${\overline{b}}_{21}=\frac{b_{21}}{{{\displaystyle \sum }}_{i=1}^n{b}_{i1}}=\frac{0.500}{1.750}=0.286\hspace{1em}\hspace{1em}\hspace{1em}{\overline{b}}_{31}=\frac{b_{31}}{{{\displaystyle \sum }}_{i=1}^n{b}_{i1}}=\frac{0.250}{1.750}=0.143$$

$${\displaystyle \sum }_{i=1}^n{b}_{i2}=2+1+\frac{1}{2}=3.500\hspace{1em}\hspace{1em}\hspace{1em}{\overline{b}}_{12}=\frac{b_{12}}{{{\displaystyle \sum }}_{i=1}^n{b}_{i2}}=\frac{2}{3.500}=0.571$$

$${\overline{b}}_{22}=\frac{b_{22}}{{{\displaystyle \sum }}_{i=1}^n{b}_{i2}}=\frac{1}{3.500}=0.286\hspace{1em}\hspace{1em}\hspace{1em}{\overline{b}}_{32}=\frac{b_{32}}{{{\displaystyle \sum }}_{i=1}^n{b}_{i2}}=\frac{0.5}{3.500}=0.143$$

$${\displaystyle \sum }_{i=1}^n{b}_{i3}=4+2+1=7\hspace{1em}\hspace{1em}\hspace{1em}\begin{array}{r}\hfill {\overline{b}}_{13}=\frac{b_{13}}{{{\displaystyle \sum }}_{i=1}^n{b}_{i3}}=\frac{4}{7}=0.571\end{array}$$

$$\begin{array}{r}\hfill {\overline{b}}_{23}=\frac{b_{23}}{{{\displaystyle \sum }}_{i=1}^n{b}_{i3}}=\frac{2}{7}=0.286\end{array}\hspace{1em}\hspace{1em}\hspace{1em}\begin{array}{r}\hfill {\overline{b}}_{33}=\frac{b_{33}}{{{\displaystyle \sum }}_{i=1}^n{b}_{i3}}=\frac{1}{7}=0.143\end{array}$$

(2) Construct the judgment matrix after normalization:

$$\left(\begin{array}{ccc}0.571& 0.571& 0.571\\ 0.286& 0.286& 0.286\\ 0.143& 0.143& 0.143\end{array}\right)$$

(3) Add the elements of raws of the normalized judgment matrix:

$${\overline{W}}_1={\displaystyle \sum }_{j=1}^n{b}_{1j}=0.571+0.571+0.571=1.713$$

$${\overline{W}}_2={\displaystyle \sum }_{j=1}^n{b}_{1j}=0.286+0.286+0.286=0.858$$

$$\begin{array}{r}\hfill {\overline{W}}_3={\displaystyle {\displaystyle \sum }_{j=1}^n}{b}_{3j}=0.143+0.143+0.143=0.429\end{array}$$

Then normalize the vector = [1.713, 0.858, 0.429], as follows:

$${\displaystyle \sum }_{j=1}^n{\overline{W}}_j=1.713+0.858+0.429=3.000;\hspace{1em}\hspace{1em}\hspace{1em}\begin{array}{cc}{W}_1=\frac{{\overline{W}}_1}{{{\displaystyle \sum }}_{j=1}^n{\overline{W}}_j}& =\frac{1.713}{3}=0.571\end{array};$$

$$\begin{array}{r}\hfill W_2=\frac{{\overline{W}}_2}{{{\displaystyle \sum }}_{j=1}^n{\overline{W}}_j}=\frac{0.858}{3}=0.286\end{array};\hspace{1em}\hspace{1em}\hspace{1em}\begin{array}{r}\hfill W_3=\frac{{\overline{W}}_3}{{\displaystyle {\displaystyle \sum }_{j=1}^n}{\overline{W}}_j}=\frac{0.429}{3}=0.143\end{array};$$

Thus, the eigenvector = [0.571,0.286,0.143]^T.

(4) Calculate the maximum eigen root ${\lambda }_{\max }$ of the judgment matrix:

$$AW=\left(\begin{array}{ccc}& & \\ 1& 2& 4\\ \frac{1}{2}& 1& 2\\ & & \\ \frac{1}{4}& \frac{1}{2}& 1\end{array}\right)\hspace{0.17em}\left(\begin{array}{c}0.571\\ 0.286\\ 0.143\end{array}\right)$$

$${(AW)}_1=1\times 0.571+2\times 0.286+4\times 0.143=1.715;$$

$${(AW)}_2=\frac{1}{2}\times 0.571+1\times 0.286+2\times 0.143=0.858;$$

$${(AW)}_3=\frac{1}{4}\times 0.571+\frac{1}{2}\times 0.286+1\times 0.143=0.429;$$

$$\begin{gathered} {\lambda }_{\max }={\displaystyle \sum }_{i=1}^n\frac{{(AW)}_i}{n{W}_i}=\frac{{(AW)}_1}{3W_1}+\frac{{(AW)}_2}{3W_2}+\frac{{(AW)}_3}{3W_3} \\ =1.715\times 3\times 0.571+0.858\times 3\times 0.286+0.429\times 3\times 0.143=3.001 \end{gathered}$$

(5) Hierarchical single sorting and consistency test:

$$CI-\frac{{\lambda }_{max}-n}{n-1}=\frac{3.001-3}{3-1}=0.0005; CR=\frac{CI}{RI}=\frac{0.0005}{0.52}=0.001<0.1;$$

These results indicated that the results of the judgment matrix are acceptable, and the obtained weight value matrix = [0.571,0.286,0.143]^T is correct.

4.2.1. Calculation of Weight of Mine Geological Factors

The geological factors of the mine included four indexes: mining depth (C₁), geological structure (C₂), coal rock structure (C₃), and rockburst liability (C₄). According to Section 2.3, the mining depth factor of the 250204 working face in Yanbei Coal Mine was Ⅱ, with medium impact risk, and the index score was 1. The geological structural factors were Ⅲ, with strong impact risk, and the index score was 5. The structural factors of coal and rock were Ⅱ, with medium impact risk, and the index score was 2. The impact liability factor of coal rock was Ⅲ, with strong impact risk, and the index score was 5. Based on the calculation method and steps mentioned in Section 3.1, the importance of the weights of these four indicators was evaluated and the judgment matrix B₁ was constructed accordingly (

Table 4. Judgment matrix of evaluation index of the mine geological factors.

Judgment Matrix B1	C1	C2	C3	C4	W1	λmax	$\frac{\mathit{C}\mathit{I}}{\mathit{R}\mathit{I}}$
C1	1	1/5	1/2	1/5	0.075	4.004	0.001 < 0.1
C2	5	1	3	1	0.393
C3	2	1/3	1	1/3	0.138
C4	5	1	3	1	0.393

).

4.2.2. Calculation of the Weight of Mining Technology Factors

The mining technical factors included two indexes: mining stress concentration factor (C₅) and inducing factor (C₆). According to Section 2.3, the mining stress concentration factor of the 250204 working face in Yanbei Coal Mine was Ⅱ, with a medium impact risk, and the index score was 3. The inductive factors were Ⅲ, with strong impact risk, and the index score was 5. According to the method and calculation steps mentioned in Section 3.1, the importance of the scale weight of these two indicators was evaluated and the judgment matrix B₂ was constructed as a result (

Table 5. Judgment matrix of evaluation index of the mining technology factors.

Judgment Matrix B2	C5	C6	W2	λmax	$\frac{\mathit{C}\mathit{I}}{\mathit{R}\mathit{I}}$
C5	1	1/2	0.333	2.0	0.0
C6	2	1	0.667

).

4.2.3. Calculation of Weight of Organizational Management Factors

The organizational management factors included two indexes: insufficient prevention investment (C₇) and ignoring impact risk (C₈). According to Section 3.2, the input factors for prevention and control of the 250204 working face in Yanbei Coal Mine were Ⅰ, with no impact risk or weak impact risk, and the index score was 1. The inductive factors were Ⅱ, with a medium risk of shock, and the score index was 3. Based on the calculation method and steps mentioned in Section 3.1, the importance of the scale weight of these two indicators was evaluated to construct the judgment matrix B₃ (

Table 6. Judgment matrix of evaluation index of the organizational management factors.

Judgment Matrix B3	C7	C8	W2	λmax	$\frac{\mathit{C}\mathit{I}}{\mathit{R}\mathit{I}}$
C7	1	1/3	0.25	2.0	0.0
C8	3	1	0.75

).

4.3. Single-Factor Membership Degree

According to the classification of the “double high” risk evaluation index system of microseismic events in Section 3, the single-factor membership function adopted the fuzzy expression of “no shock risk, medium shock risk, and strong shock risk.” According to the idea of the expert investigation method of membership function, a safety checklist was chosen as the investigation method [50]. The safety checklist was chiefly qualitative in practical application. Therefore, to make enterprises better apply the safety checklist, the study employed the ratio method to determine the single-factor membership degree. That is, the ratio of the number of each level to the total number of all the evaluation items of the index was utilized as the membership degree of the evaluation index.

To carry out a unified measurement, this paper, based on the current coal mine safety management system and the relevant research results of domestic and foreign experts on rockburst, formulates a safety check list. The assessor only needs to tick “√” in the corresponding column of the assessment score [51]. Combined with the actual situation and expert opinions of Yanbei Coal Mine, the detailed evaluation of the impact risk of Yanbei Coal Mine is shown in

Table 7. Membership statistics of the risk evaluation index.

Factors	Detailed Inspection Items	Evaluation Grade
		1	2	3
Mining depth	Actual working depth		√
	Mining above critical depth			√
Depth of mining membership		0.000	0.500	0.500
Geological structure	Fold (anticline, syncline)			√
	Fault			√
	The coal seam dip angle and thickness change sharply zone		√
	Tectonic change zone and tectonic stress zone			√
Geological structure membership degree		0.000	0.250	0.750
Coal and rock structure	Hard, thick, integrated roof			√
	Hard coal seam floor			√
	Coal with high strength, large elastic modulus, small moisture content, large metamorphic degree, and a large proportion of dark coal			√
Subjection degree of coal structure		0.000	0.000	1.000
The bursting liability of coal rock	Dynamic failure time of coal		√
	The impact energy index of coal			√
	The elastic energy index of coal			√
	Unidirectional compressive strength of coal		√
Subjection degree of coal rockburst liability		0.000	0.500	0.500
Mining stress concentration factor	Mining method (whether long arm or dry mining)	√
	Roof management method		√
	Mining procedures (whether to mine the working face, whether to advance and return to each other, whether to excavate the roadway in the supporting pressure zone)			√
	Face length		√
	Caving ratio		√
	Close to the residual mining area and stop-mining line			√
	Coal pillar			√
	Mined-out area			√
	Mining speed			√
Mining stress concentration factor membership degree		0.111	0.333	0.556
Inducing factors	Blasting			√
	Roof		√
	Initial pressure and periodic pressure			√
	Coal mining (support shifting)		√
Membership degree of inducing factors		0.000	0.500	0.500
Prevention and control of inputs	Support technology equipment is not in place		√
	No effective monitoring and forecasting equipment were selected		√
	Reasonable and effective anti-flushing measures have not been considered			√
Control input membership		0.000	0.667	0.333
Impact risk attention	Weak awareness of anti-impact		√
	The law of rock movement is not grasped			√
	No special administrative body has been established		√
	The knowledge of rockburst has not been studied		√
Attachment degree of impact risk		0.000	0.750	0.250

Note: 1—no impact risk; 2—medium impact risk; 3—strong impact risk.

.

4.4. Results of the Fuzzy Comprehensive Evaluation

The accident tree structure of the fuzzy hierarchical comprehensive evaluation model was as follows.

(1) The second-level fuzzy comprehensive evaluation:

① Mine geological factors: $\underset{¯}{R_1}=\left(\begin{array}{ccc}0& 0.5& 0.5\\ 0& 0.25& 0.75\\ 0& 0& 1\\ 0& 0.5& 0.5\end{array}\right)$

$$\underset{¯}{W_1^{\prime }}=(0.075{\xi }_3,0.393{\xi }_3,0.138{\xi }_3,0.393{\xi }_1)=\left(\begin{array}{cccc}0.075& 0.393& 0.138& 1.179\end{array}\right)$$

After normalization: ${\underset{¯}{W}}_1^{″}=\left(\begin{array}{cccc}0.042& 0.220& 0.077& 0.661\end{array}\right)$

$$\underset{¯}{B^{″}}1=\underset{¯}{W^{″}}1\times R1=\left(\begin{array}{ccc}0.000& 0.406& 0.594\end{array}\right);$$

② Mining technology factors: ${\underset{¯}{R}}_2=\left(\begin{array}{ccc}0.111& 0.333& 0.556\\ 0.000& 0.500& 0.500\end{array}\right)$

$${\underset{¯}{W}}_2^{\prime }=(0.333{\xi }_1,0.667{\xi }_1)=\left(\begin{array}{cc}1& 2\end{array}\right)$$

After normalization: ${\underset{¯}{W}}_2^{″}=(0.333,0.667)$

$${\underset{¯}{B}}_2{}^{″}={\underset{¯}{\mathrm{W}}}_2{}^{″}•{\underset{¯}{R}}_2=\left(\begin{array}{ccc}0.037& 0.444& 0.519\end{array}\right);$$

③ Organizational management factors: ${\underset{¯}{R}}_3=\left(\begin{array}{ccc}0.000& 0.667& 0.333\\ 0.000& 0.750& 0.250\end{array}\right)$

$${\underset{¯}{W}}_3^{\prime }=(0.750{\xi }_2,0.250{\xi }_2)=\left(\begin{array}{cc}1.5& 0.5\end{array}\right)$$

After normalization: ${\underset{¯}{W}}_3{}^{″}=(0.750,0.250)$

$${\underset{¯}{B}}_3{}^{″}=W_3{}^{″}•R_3=\left(0.00,0.688,0.312\right)$$

(2) Fuzzy comprehensive evaluation

Weight vector of the criterion layer: $\underset{¯}{\mathrm{W}}=(0.571,0.286,0.143)$

$$R_{}^{″}=\left[\begin{array}{c}{B}_1^{″}\\ B_2^{″}\\ B_3^{″}\end{array}\right]=\left(\begin{array}{ccc}0.000& 0.406& 0.594\\ 0.037& 0.444& 0.519\\ 0.00& 0.668& 0.312\end{array}\right).$$

Therefore, $B_{}^{″}=W_{}^{″}•R=(\begin{array}{c}0.012,0.455,0.533\end{array})$.

The evaluation results demonstrated that the “double high” risk of microseismic events in the 250204 working face of Yanbei Coal Mine belongs to the membership degree of “no impact risk, medium impact risk, and strong impact risk” of “0.012, 0.455, 0.533”. Based on the principle of maximum membership degree, the “double high” risk of microseismic events in Yanbei Coal Mine belongs to the strong impact risk. That is, the 250204 working face of Yanbei Coal Mine has a strong impact risk. The fuzzy level comprehensive evaluation method represents the qualitative problem and its judgment in the form of quantity and carries out the fuzzy operation, which reduces the subjectivity of people to a certain extent and makes the evaluation more objective and scientific [41].

5. Conclusions

Considering impact ground pressure hazard in the coal mine as the research object, and using the accident tree, analytic hierarchy process, and the fuzzy comprehensive evaluation model of the microseismic events, we put forward a more effective prevention measure through double risk evaluation and analysis of the impact ground pressure hazard of the mine. The following conclusions were drawn from this study.

(1) This study can effectively evaluate the impact of coal mining in coal mine by establishing a “double high” risk index system. The index system is controlled by eight basic layers of the three intermediate layers, forming an accident tree on the top of a double height event. In this study, the paper expounds and establishes the principle of establishing the “double high” risk index system, which makes the evaluation result more accurate and comprehensive. The index system contains several evaluation indicators that can be divided into different levels to assess the impact risks of different levels. This new index system provides a more reliable basis for the assessment of the damage of the coal mine and provides effective support for the formulation of relevant decision-making and prevention measures.

(2) The evaluation index of a “double high” risk of microseismic events in Yanbei Coal Mine was analyzed, and the evaluation indexes were classified. Among them, the four indexes of mining depth, surrounding rock structure, mining stress concentration, and impact risk belonged to level Ⅱ, with a medium impact risk. The three indexes of the geological structure, coal rock impact risk, and inductive factors belonged to level Ⅲ, with a strong impact risk. The input factor of prevention and control belonged to level Ⅰ, without an impact risk (or with a weak impact risk).

(3) The weight of the criterion layer and index layer of the “double high” risk evaluation index system of microseismic events was calculated by the analytic hierarchy process. The fuzzy hierarchical comprehensive evaluation model was employed to evaluate the “double high” risk of microseismic events in the 250204 working face of Yanbei Coal Mine, and the evaluation model was modified based on the qualitative analysis results of the accident tree.