Two-Phase Non-Singular Terminal Sliding Mode Control of Nonlinear Systems
Abstract
:1. Introduction
2. Preliminaries
3. Problem Formulation
4. The NTSM Controller Design
4.1. TSM with FD
4.2. NTSM with FD
4.3. Extensions to MIMO Systems
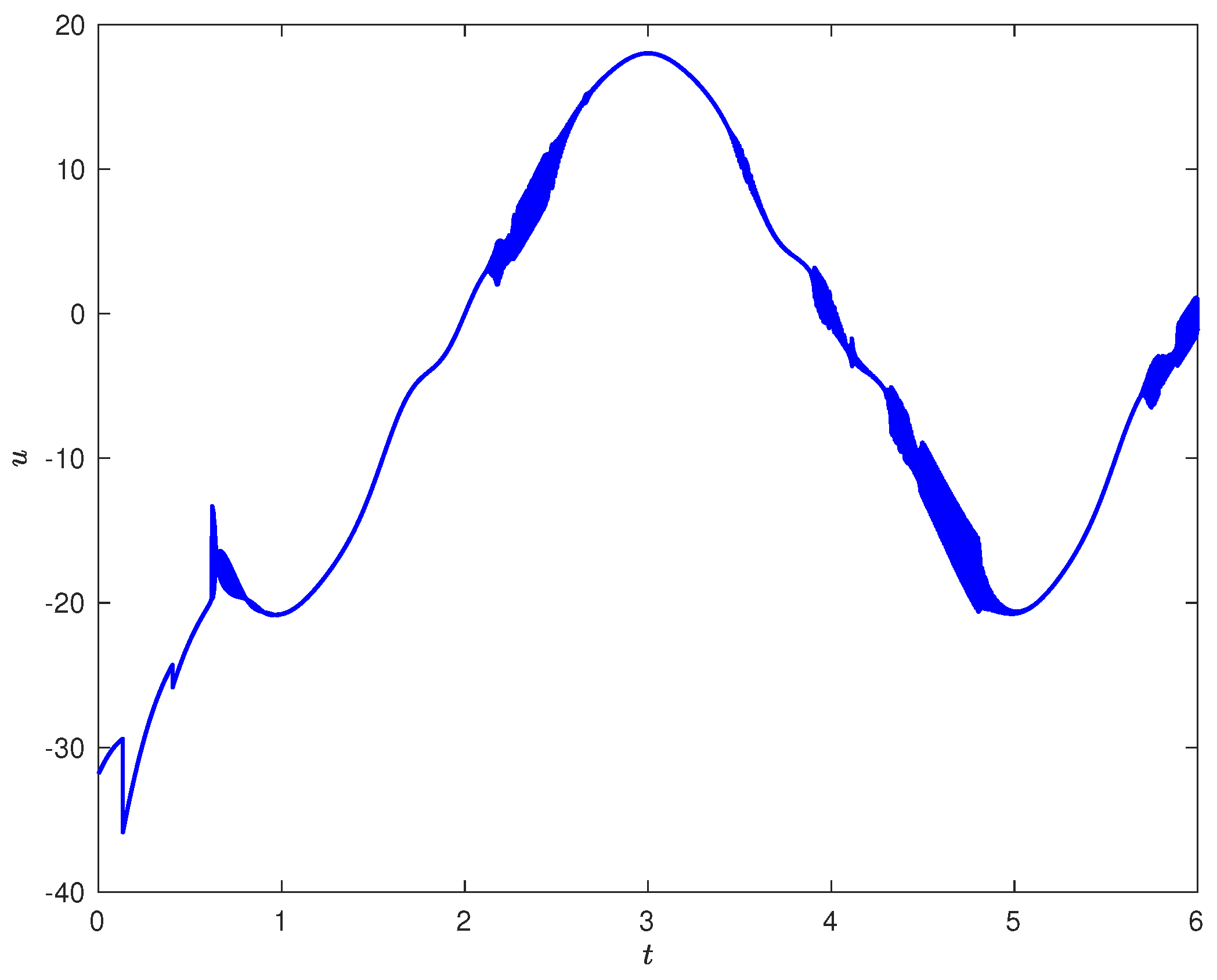
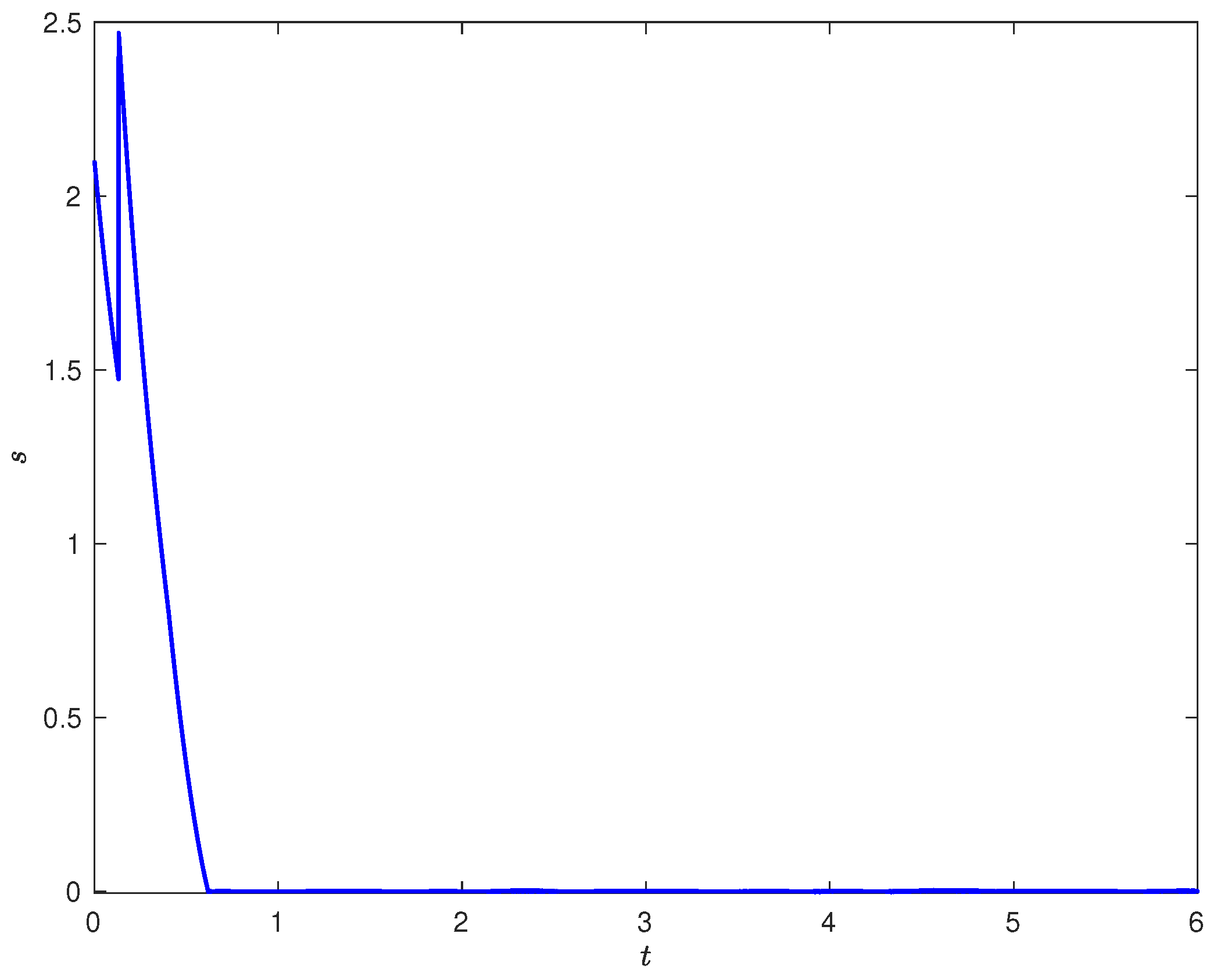
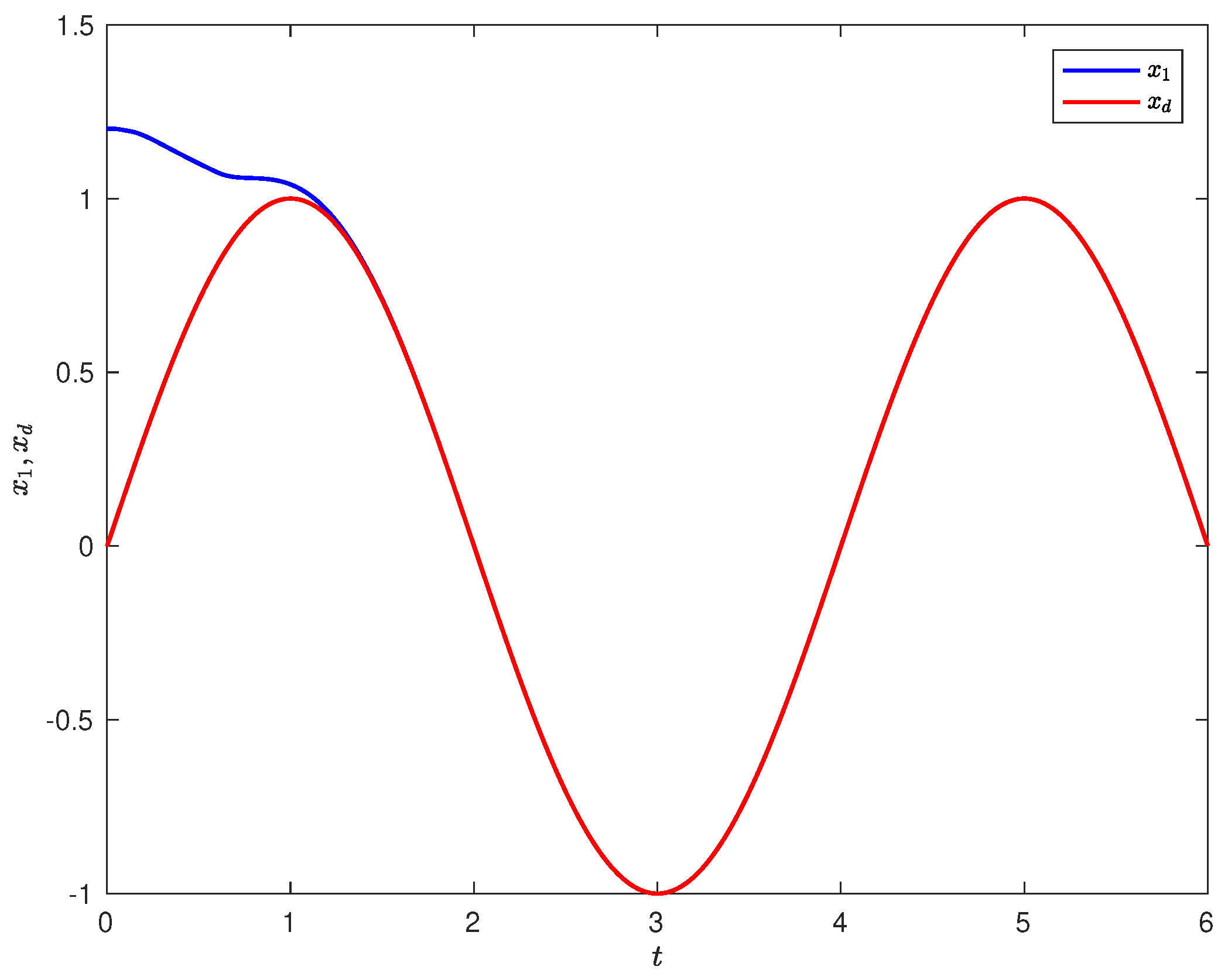
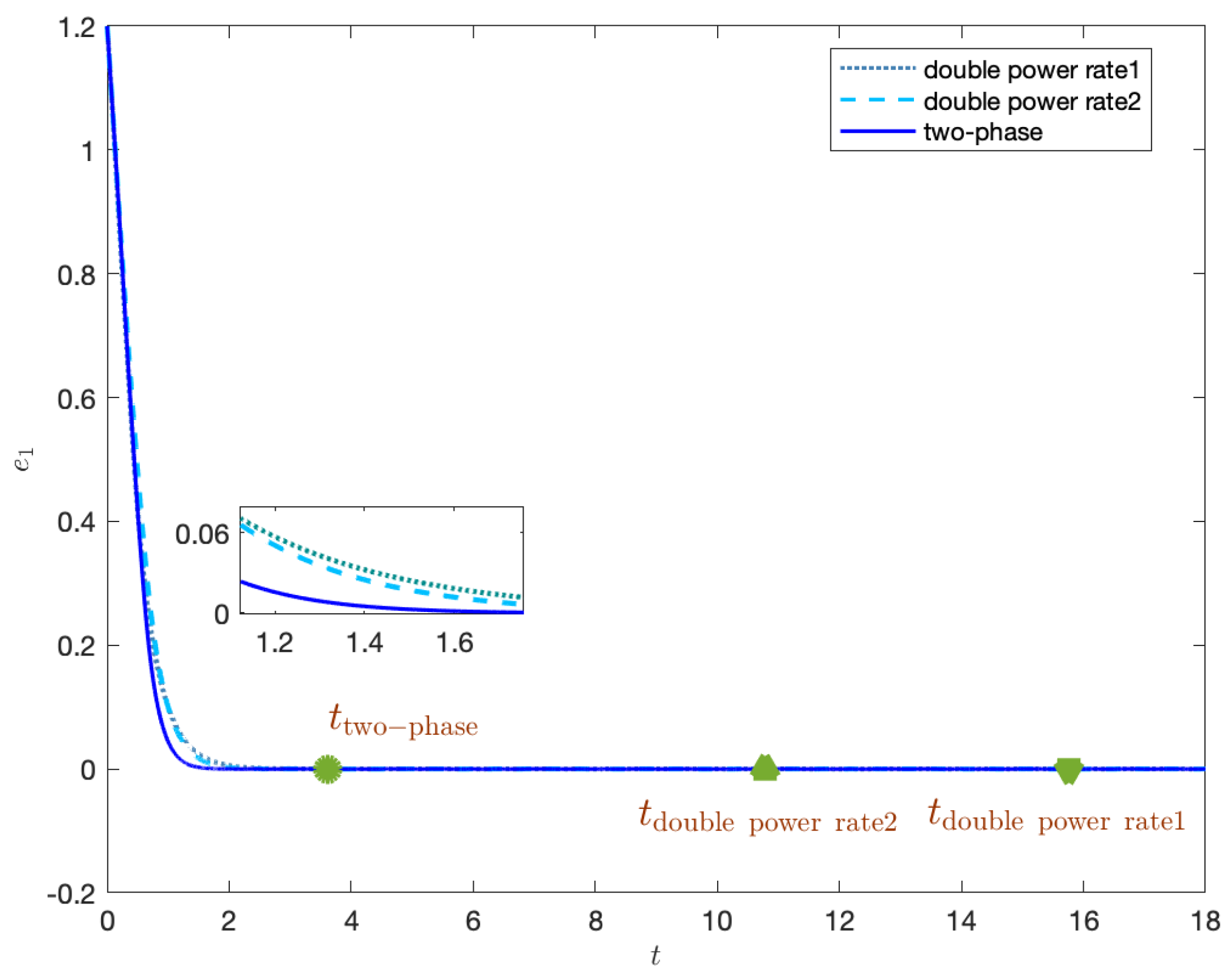
5. Numerical Simulations
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Utkin, V.I. Sliding Modes in Control and Optimization; Springer: Berlin/Heidelberg, Germany, 1992. [Google Scholar]
- Cui, R.; Chen, L.; Yang, C.; Chen, M. Extended state observer-based integral sliding mode control for an underwater robot with unknown disturbances and uncertain nonlinearities. IEEE Trans. Industrial Elect. 2017, 64, 6785–6795. [Google Scholar] [CrossRef]
- Shao, K.; Zheng, J.; Huang, K.; Wang, H.; Man, Z.; Fu, M. Finite-time control of a linear motor positioner using adaptive recursive terminal sliding mode. IEEE Trans. Industrial Elect. 2019, 67, 6659–6668. [Google Scholar] [CrossRef]
- Wang, Z.; Li, S.; Li, Q. Continuous nonsingular terminal sliding mode control of DC–DC boost converters subject to time-varying disturbances. IEEE Trans. Circuits Syst.-II Expre. Brie. 2020, 67, 2552–2556. [Google Scholar] [CrossRef]
- Bartolini, G.; Ferrara, A.; Usai, E. Chattering avoidance by second-order sliding mode control. IEEE Trans. Autom. Contr. 1998, 43, 241–246. [Google Scholar] [CrossRef]
- Dehkordi, N.M.; Sadati, N.; Hamzeh, M. A robust backstepping high-order sliding mode control strategy for grid-connected DG units with harmonic/interharmonic current compensation capability. IEEE Trans. Susta. Ener. 2017, 8, 561–572. [Google Scholar] [CrossRef]
- He, X.; Li, X.; Song, S. Nonsingular terminal sliding-mode control of second-order systems subject to hybrid disturbances. IEEE Trans. Circuits Syst.-II: Expre. Brie. 2022, 69, 5019–5023. [Google Scholar] [CrossRef]
- Kamal, S.; Moreno, J.A.; Chalanga, A.; Bandyopadhyay, B.; Fridman, L.M. Continuous terminal sliding-mode controller. Automatica 2016, 69, 308–314. [Google Scholar] [CrossRef]
- Polyakov, A.; Poznyak, A. Reaching time estimation for super-twisting second-order sliding-mode controller via Lyapunov function designing. IEEE Trans. Autom. Contr. 2009, 54, 1951–1955. [Google Scholar] [CrossRef]
- Moreno, J.A.; Osorio, M. Strict Lyapunov functions for the super-twisting algorithm. IEEE Trans. Autom. Contr. 2012, 57, 1035–1040. [Google Scholar] [CrossRef]
- Utkin, V.I.; Poznyak, A.S. Adaptive sliding mode control with application to super-twist algorithm: Equivalent control method. Automatica 2013, 49, 39–47. [Google Scholar] [CrossRef]
- Yang, J.; Li, S.; Yu, X. Sliding-mode control for systems with mismatched uncertainties via a disturbance observer. IEEE Trans. Indus. Elect. 2013, 60, 160–169. [Google Scholar] [CrossRef]
- Sun, R.; Zhou, Z.; Zhu, X. Finite-time terminal sliding mode attitude control for tailless full-wing configuration UAVs based on extended state observers and auxiliary compensators. ISA Trans. 2023; early access. [Google Scholar] [CrossRef]
- Zak, M. Terminal actuators in neural networks. Neural Netwo. 1989, 2, 259–274. [Google Scholar] [CrossRef]
- Man, Z.; Paplinski, A.P.; Wu, H.R. A robust MIMO terminal sliding mode control scheme for rigid robotic manipulators. IEEE Trans. Autom. Contr. 1994, 39, 2464–2469. [Google Scholar]
- Chen, G.; Song, Y.; Guan, Y. Terminal sliding mode-based con- sensus tracking control for networked uncertain mechanical systems on digraphs. IEEE Trans. Neur. Netwo. Learn. Syst. 2018, 29, 749–756. [Google Scholar] [CrossRef]
- Jin, M.; Lee, J.; Ahn, K.K. Continuous nonsingular terminal sliding-mode control of shape memory alloy actuators using time delay estimation. IEEE/ASME Trans. Mecha. 2015, 20, 899–909. [Google Scholar] [CrossRef]
- Feng, Y.; Yu, X.; Man, Z. Non-singular adaptive terminal sliding mode control of rigid manipulators. Automatica 2002, 38, 2159–2167. [Google Scholar] [CrossRef]
- Yu, S.; Yu, X.; Shirinzadeh, B.; Man, Z. Continuous finite-time control for robotic manipulators with terminal sliding mode. Automatica 2005, 41, 1957–1964. [Google Scholar] [CrossRef]
- Yang, L.; Yang, J. Nonsingular fast terminal sliding-mode control for nonlinear dynamical systems. Int. Jour. Robu. Nonli. Contr. 2011, 21, 1865–1879. [Google Scholar] [CrossRef]
- Xu, S.; Chen, C.; Wu, Z. Study of nonsingular fast terminal sliding-mode fault-tolerant control. IEEE Trans. Indus. Elect. 2015, 62, 3906–3913. [Google Scholar] [CrossRef]
- Sun, Y.; Gao, Y.; Zhao, Y.; Liu, Z.; Wang, J.; Kuang, J.; Yan, F.; Liu, J. Neural network-based tracking control of uncertain robotic systems: Predefined-time nonsingular terminal sliding-mode approach. IEEE Trans. Ind. Electron. 2022, 69, 10510–10520. [Google Scholar] [CrossRef]
- Lian, S.; Meng, W.; Shao, K.; Zheng, J.; Zhu, S.; Li, H. Full attitude control of a quadrotor using fast nonsingular terminal sliding mode with angular velocity planning. IEEE Trans. Indus. Elect. 2023, 70, 3975–3984. [Google Scholar] [CrossRef]
- Feng, Y.; Yu, X.; Han, F. On nonsingular terminal sliding-mode control of nonlinear systems. Automatica 2013, 49, 1715–1722. [Google Scholar] [CrossRef]
- Zuo, Z. Non-singular fixed-time terminal sliding mode control of non-linear systems. IET Contr. Theor. Appl. 2015, 9, 545–552. [Google Scholar] [CrossRef]
- Dong, H.; Yang, X.; Kuang, Z.; Liu, M. On practical terminal sliding-mode control for systems with or without mismatched uncertainty. J. Frankl. Inst. 2022, 359, 8084–8106. [Google Scholar] [CrossRef]
- Bassetto, M.; Niccolai, L.; Boni, L.; Mengali, G.; Quarta, A.A.; Circi, C.; Pizzurro, S.; Pizzarelli, M.; Pellegrini, R.C.; Cavallini, E. Sliding mode control for attitude maneuvers of Helianthus solar sail. Acta Astronaut. 2022, 198, 100–110. [Google Scholar] [CrossRef]
- Nguyen, N.; Oh, H.; Moon, J. Continuous nonsingular terminal sliding-mode control with integral-type sliding surface for disturbed systems: Application to attitude control for quadrotor UAVs under external disturbances. IEEE Trans. Aeros. Elect. Syst. 2022, 58, 5635–5660. [Google Scholar] [CrossRef]
- Zhao, K.; Liu, W.; Zhou, R.; Dai, W.; Wu, S.; Qiu, P.; Yin, Y.; Jia, N.; Yi, J.; Huang, G.; et al. Model-free fast integral terminal sliding-mode control method based on improved fast terminal sliding-mode observer for PMSM with unknown disturbances. ISA Trans. 2023; early access. [Google Scholar] [CrossRef]
- Asadi, D.; Bagherzadeh, S. Nonlinear adaptive sliding mode tracking control of an airplane with wing damage. Proc. Inst. Mech. Eng. Part J. Aerosp. Eng. 2017, 232, 1405–1420. [Google Scholar] [CrossRef]
- Zhu, D.; Huang, P.; Du, B.; Zhu, P. Adaptive nonsingular terminal sliding mode control of robot manipulator based on contour error compensation. Sci. Rep. 2023, 13, 330. [Google Scholar]
- Polyakov, A. Nonlinear feedback design for fixed-time stabilization of linear control systems. IEEE Trans. Autom Contr. 2012, 57, 2106–2110. [Google Scholar] [CrossRef]
- Ni, J.; Liu, C.; Li, S. Fast fixed-time nonsingular terminal sliding mode control and its application to chaos suppression in power system. IEEE Trans. Circu. Sys. II Exp. Bri. 2017, 64, 151–155. [Google Scholar] [CrossRef]
- Ferrara, A.; Incremona, G.P. Predefined-time output stabilization with second order sliding mode generation. IEEE Trans. Autom. Contr. 2021, 66, 1445–1451. [Google Scholar] [CrossRef]
- Moulay, E.; Léchappé, V.; Bernuau, E.; Plestan, F. Robust fixed-time stability: Application to sliding-mode control. IEEE Trans. Autom. Contr. 2022, 67, 1061–1066. [Google Scholar] [CrossRef]
- Sun, M. Two-phase attractors for finite-duration consensus of multiagent systems. IEEE Trans. Syste. Man Cyber. Syst. 2018, 50, 1757–1765. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, H.; Tang, C. Two-Phase Non-Singular Terminal Sliding Mode Control of Nonlinear Systems. Appl. Sci. 2023, 13, 12684. https://doi.org/10.3390/app132312684
Li H, Tang C. Two-Phase Non-Singular Terminal Sliding Mode Control of Nonlinear Systems. Applied Sciences. 2023; 13(23):12684. https://doi.org/10.3390/app132312684
Chicago/Turabian StyleLi, He, and Chengshuang Tang. 2023. "Two-Phase Non-Singular Terminal Sliding Mode Control of Nonlinear Systems" Applied Sciences 13, no. 23: 12684. https://doi.org/10.3390/app132312684
APA StyleLi, H., & Tang, C. (2023). Two-Phase Non-Singular Terminal Sliding Mode Control of Nonlinear Systems. Applied Sciences, 13(23), 12684. https://doi.org/10.3390/app132312684




