Dynamic Behavior of Aluminum Plates Subjected to Sequential Fragment Impact and Blast Loading: An Experimental Study
Abstract
1. Introduction
2. Dynamic Behavior of Thin Aluminum Plates Subjected to the Impact of Fragment-Simulating Projectiles
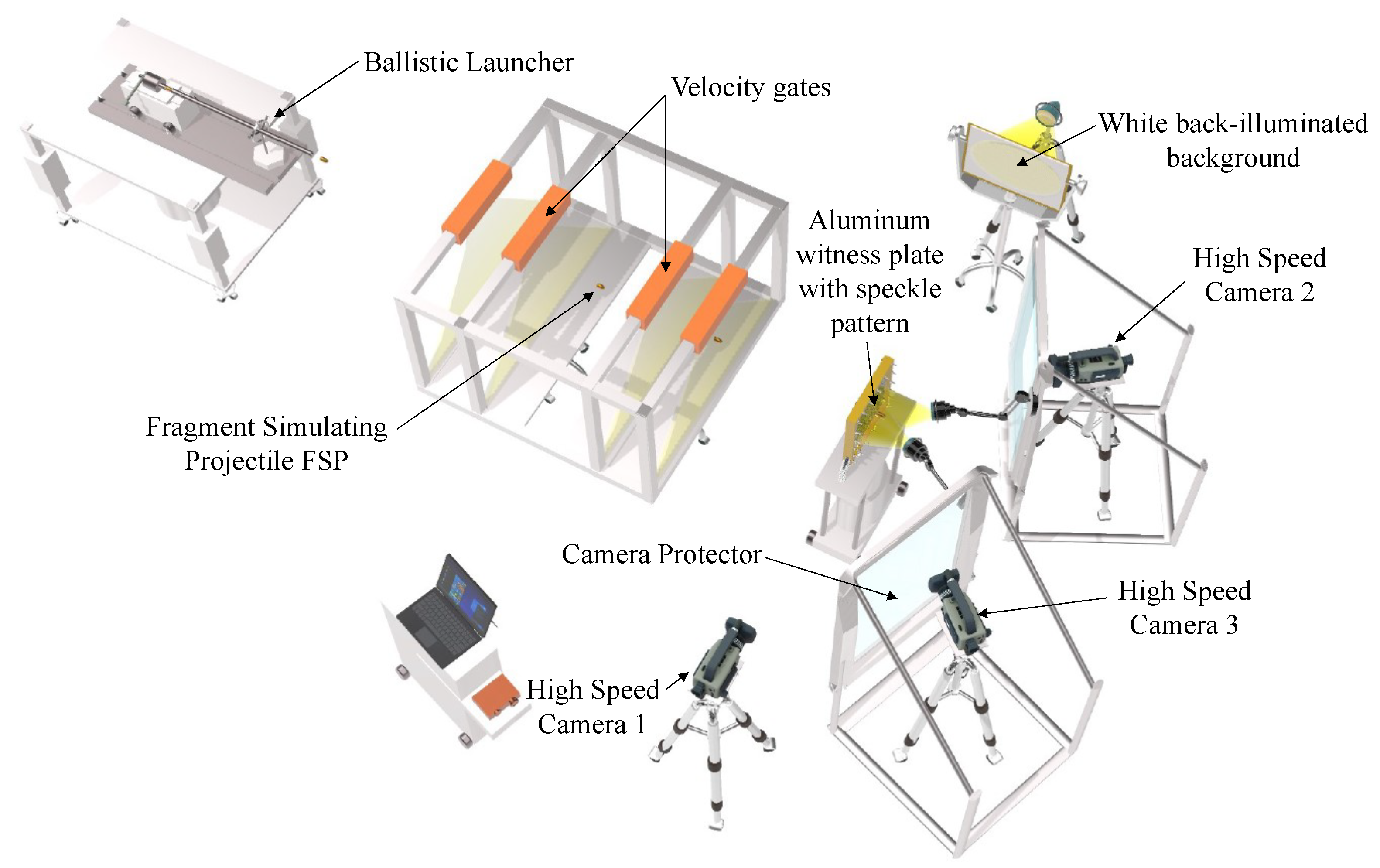
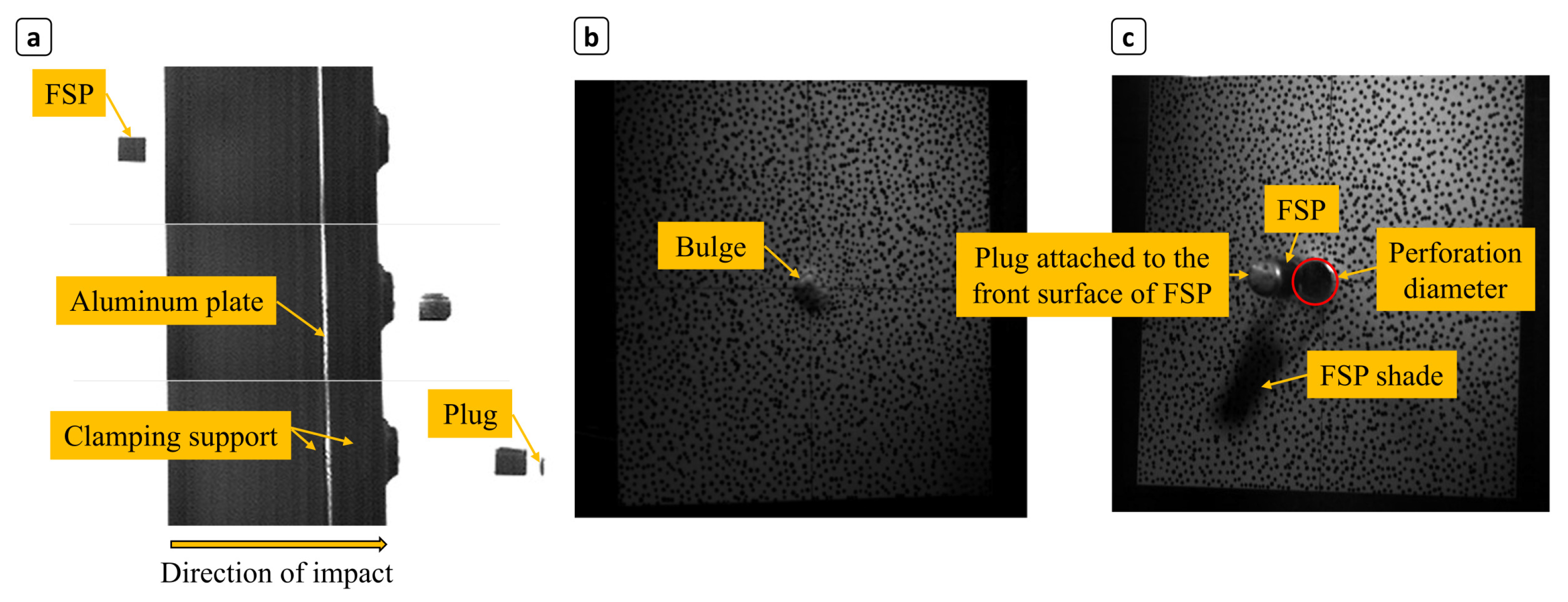
2.1. Experimental Setup
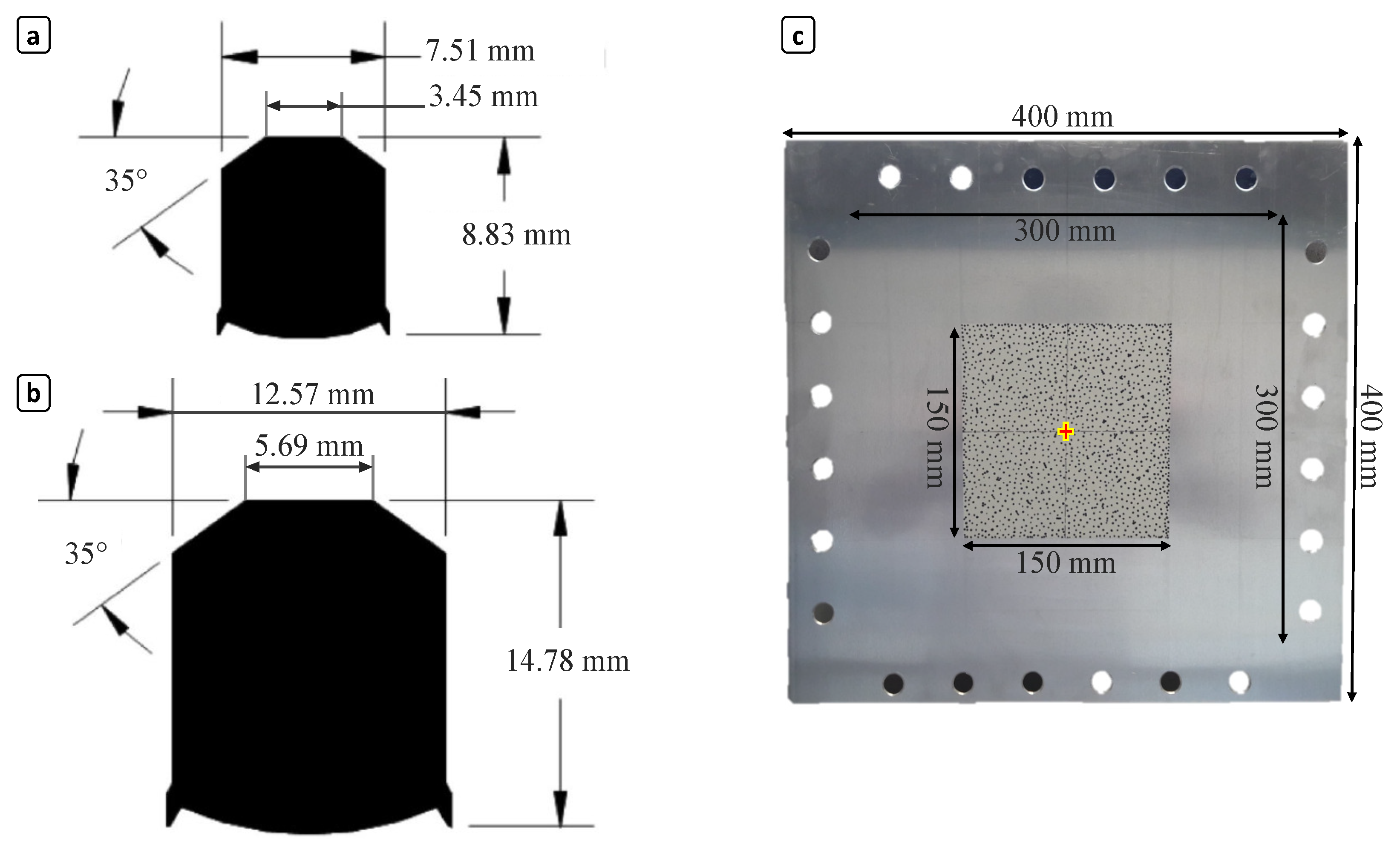
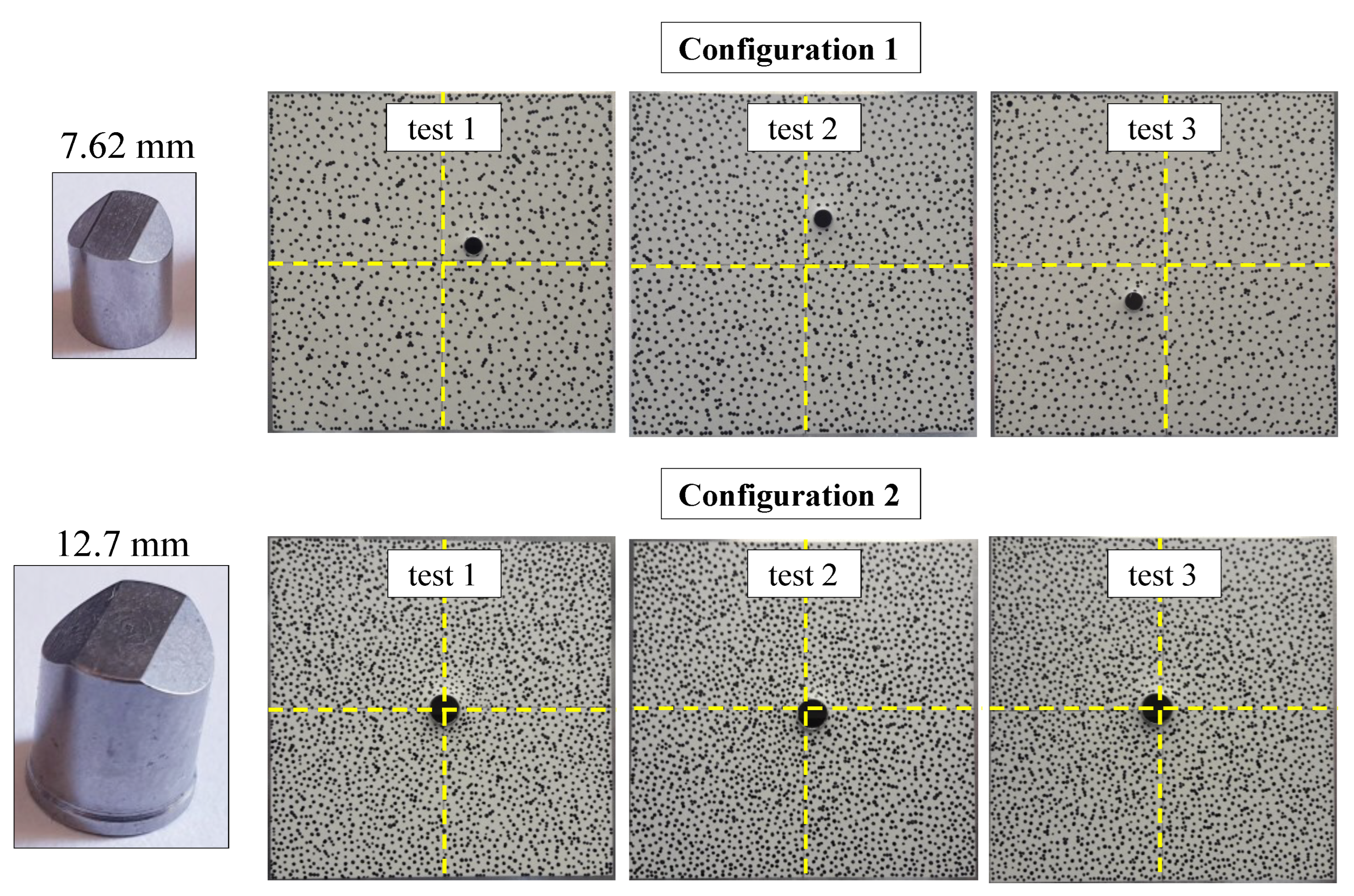
2.2. Experimental Program
2.3. Experimental Results
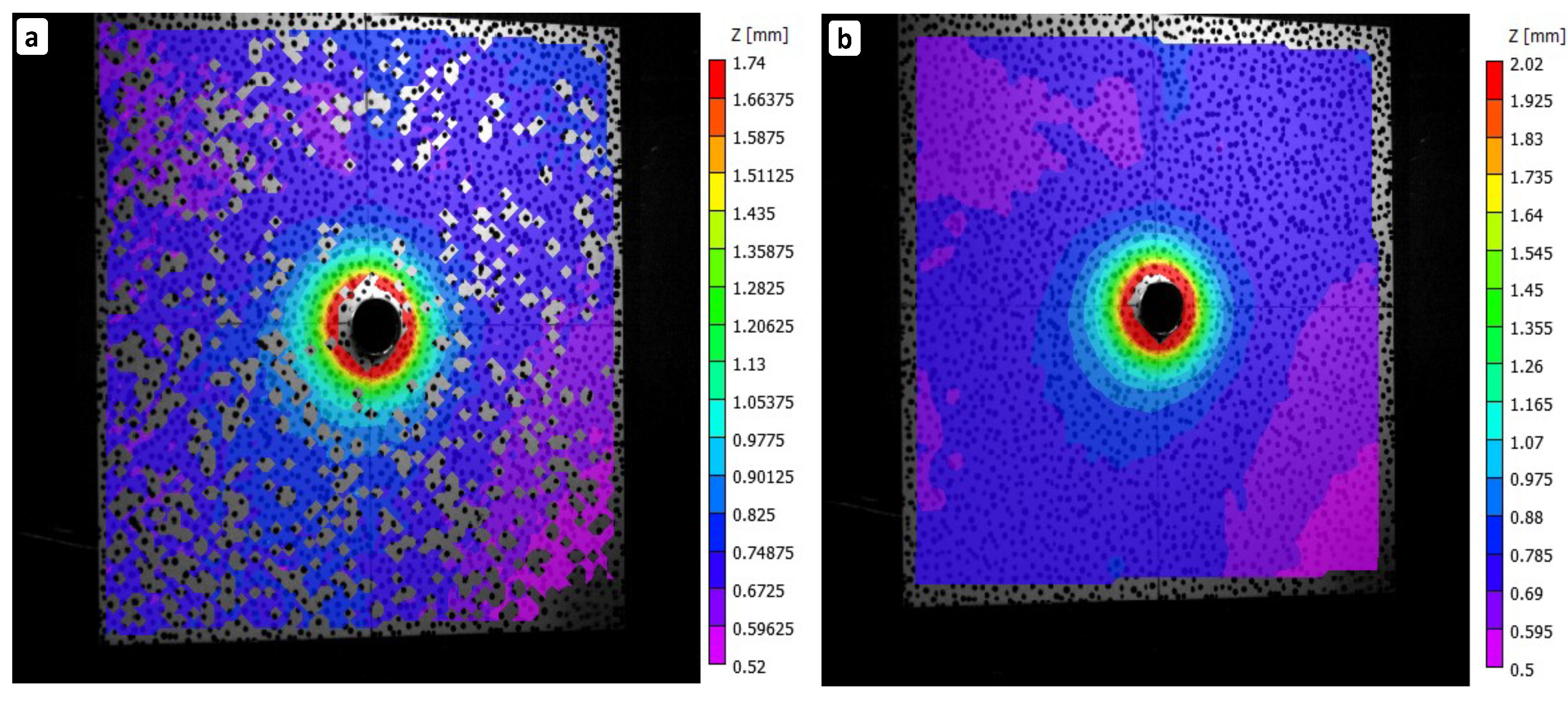
2.3.1. Plates Subjected to a Single FSP Impact
2.3.2. Plates Subjected to Three FSP Impacts
3. Dynamic Behavior of Blast-Loaded Aluminum Plates Pre-Perforated by Ballistic Impact
3.1. Experimental Setup
3.2. Experimental Results
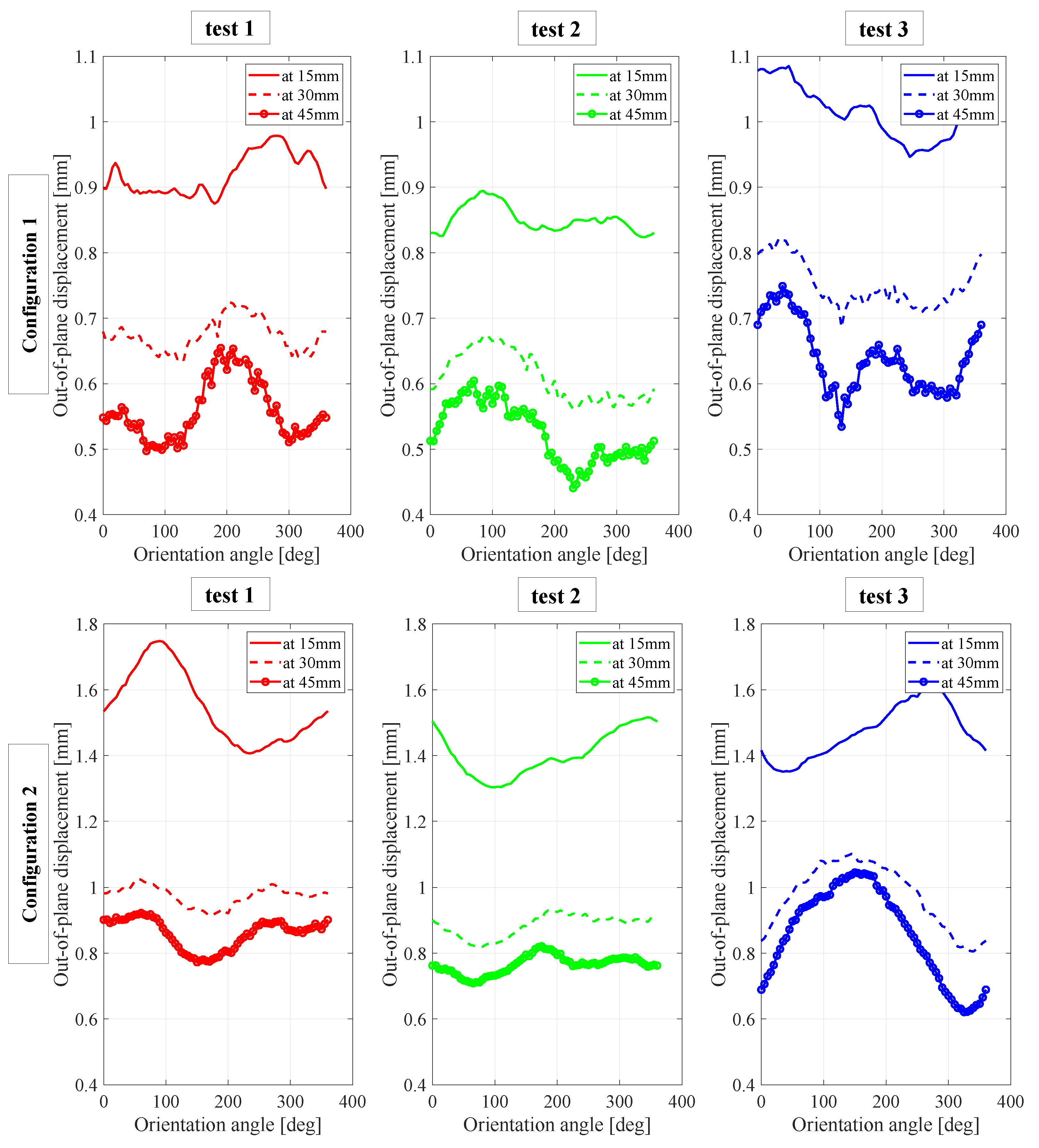
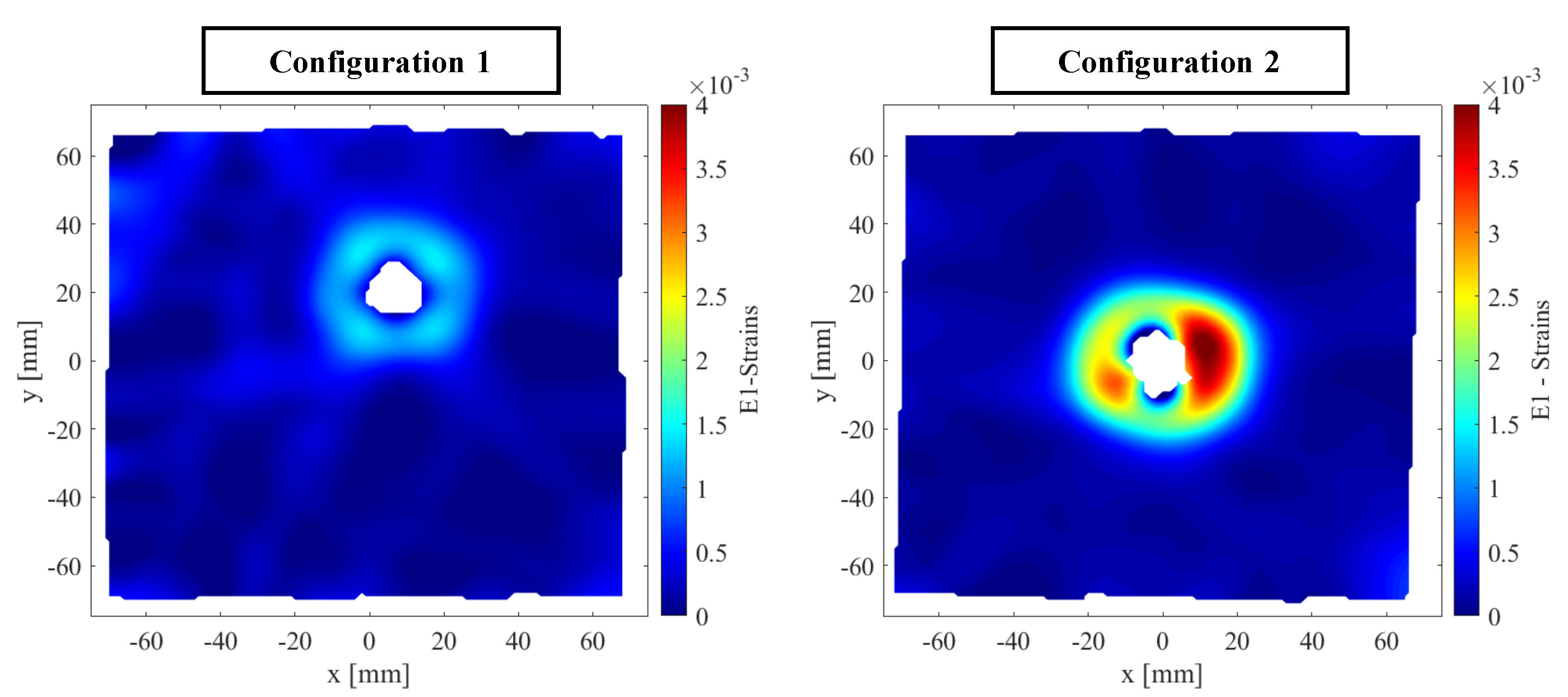
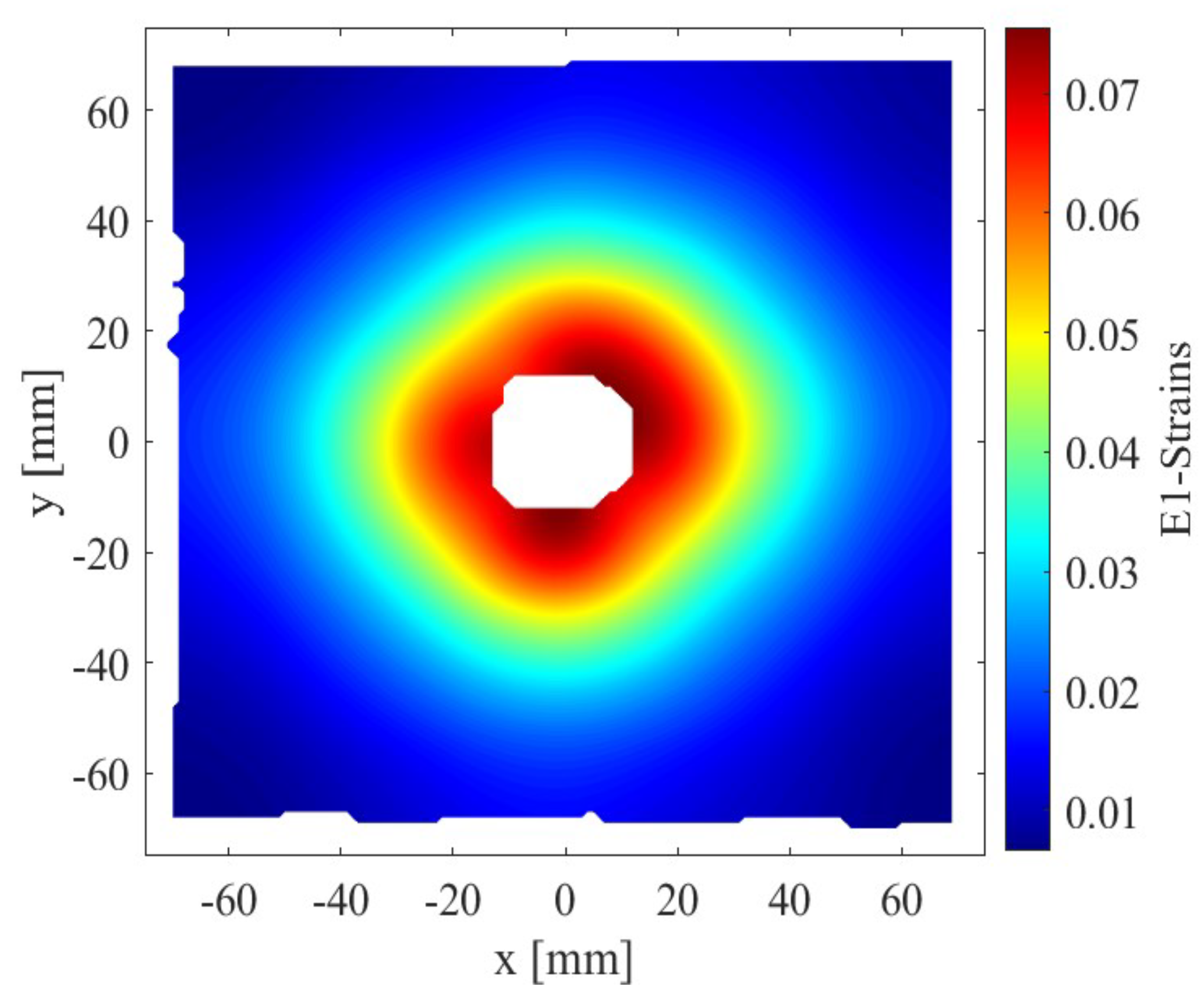
3.2.1. Blast-Loaded Plates with Single FSP Impact
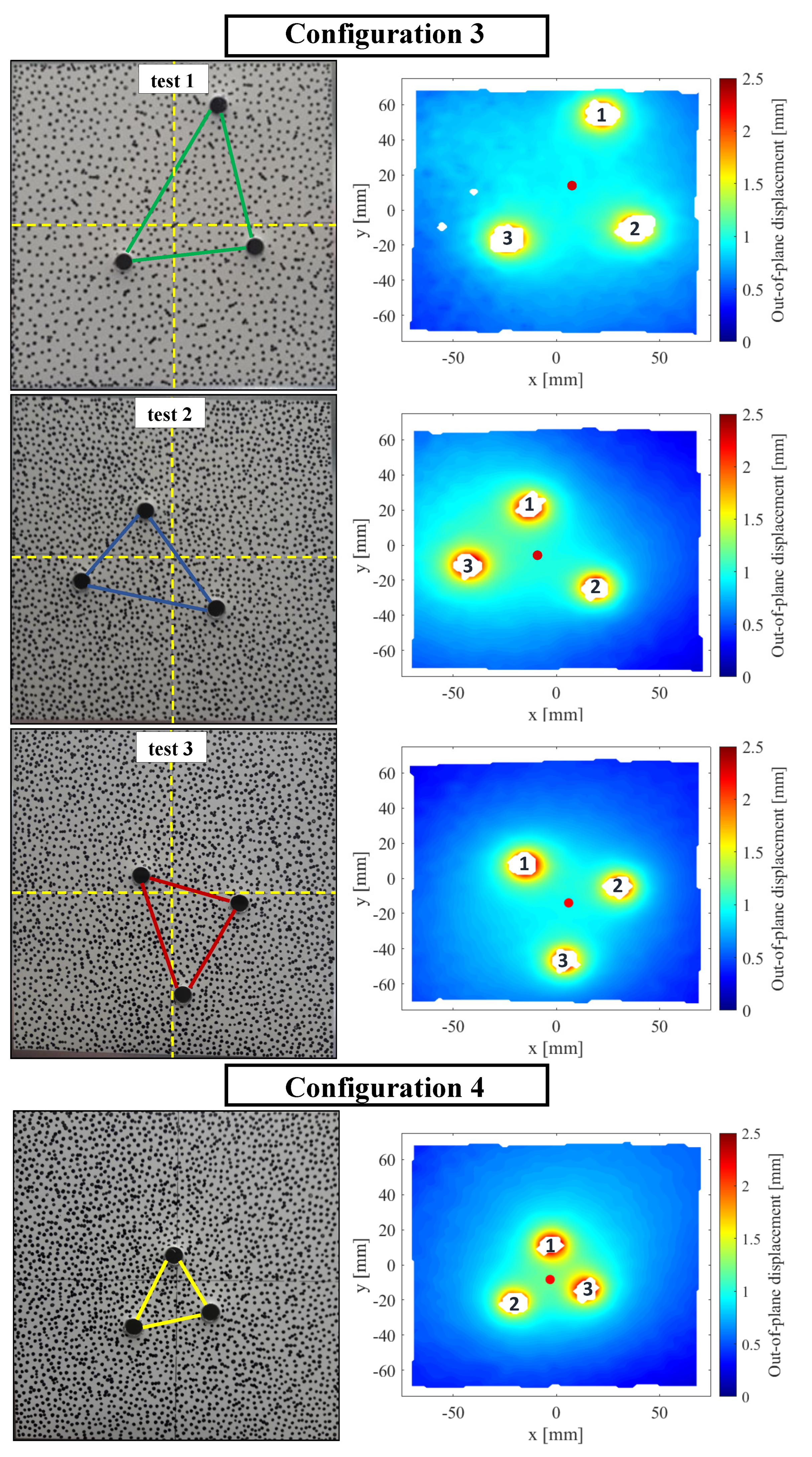
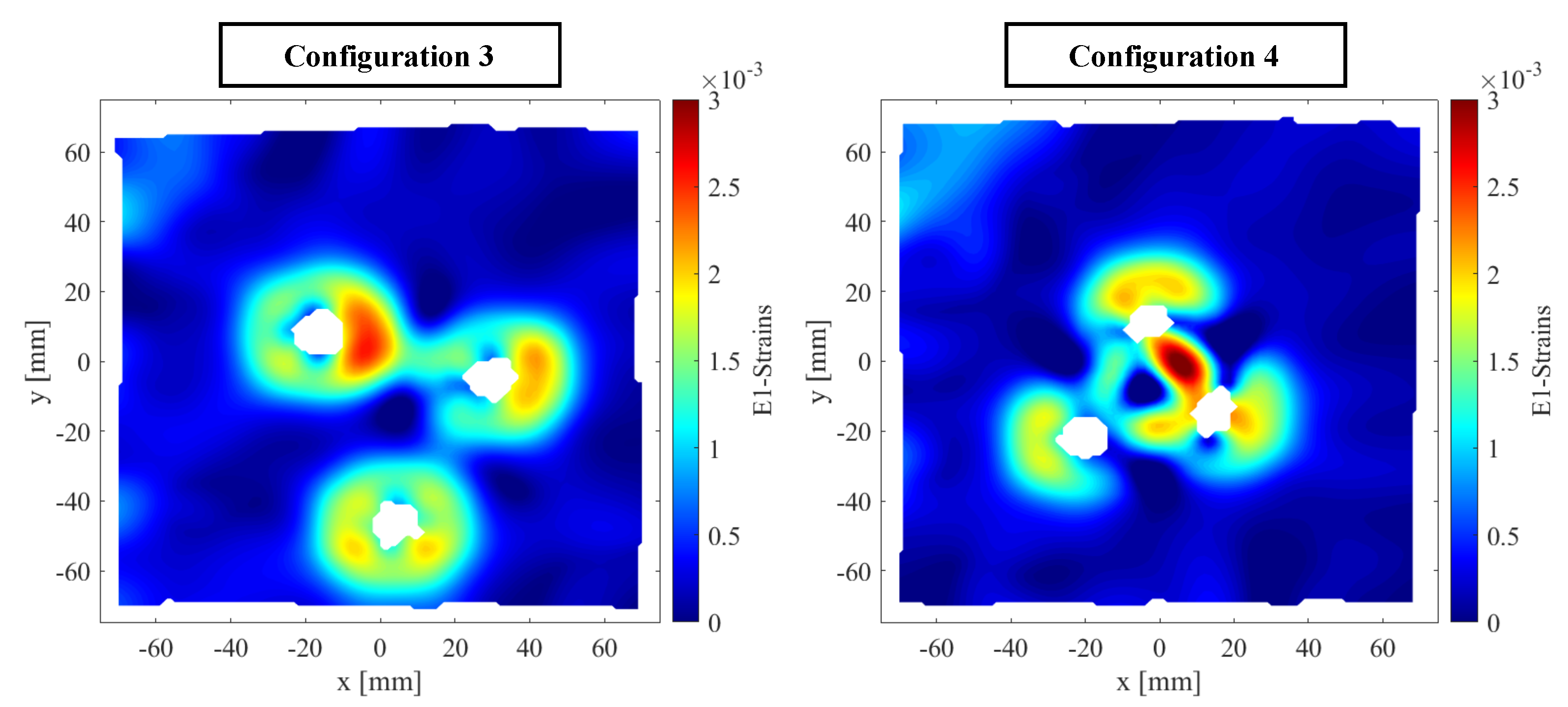
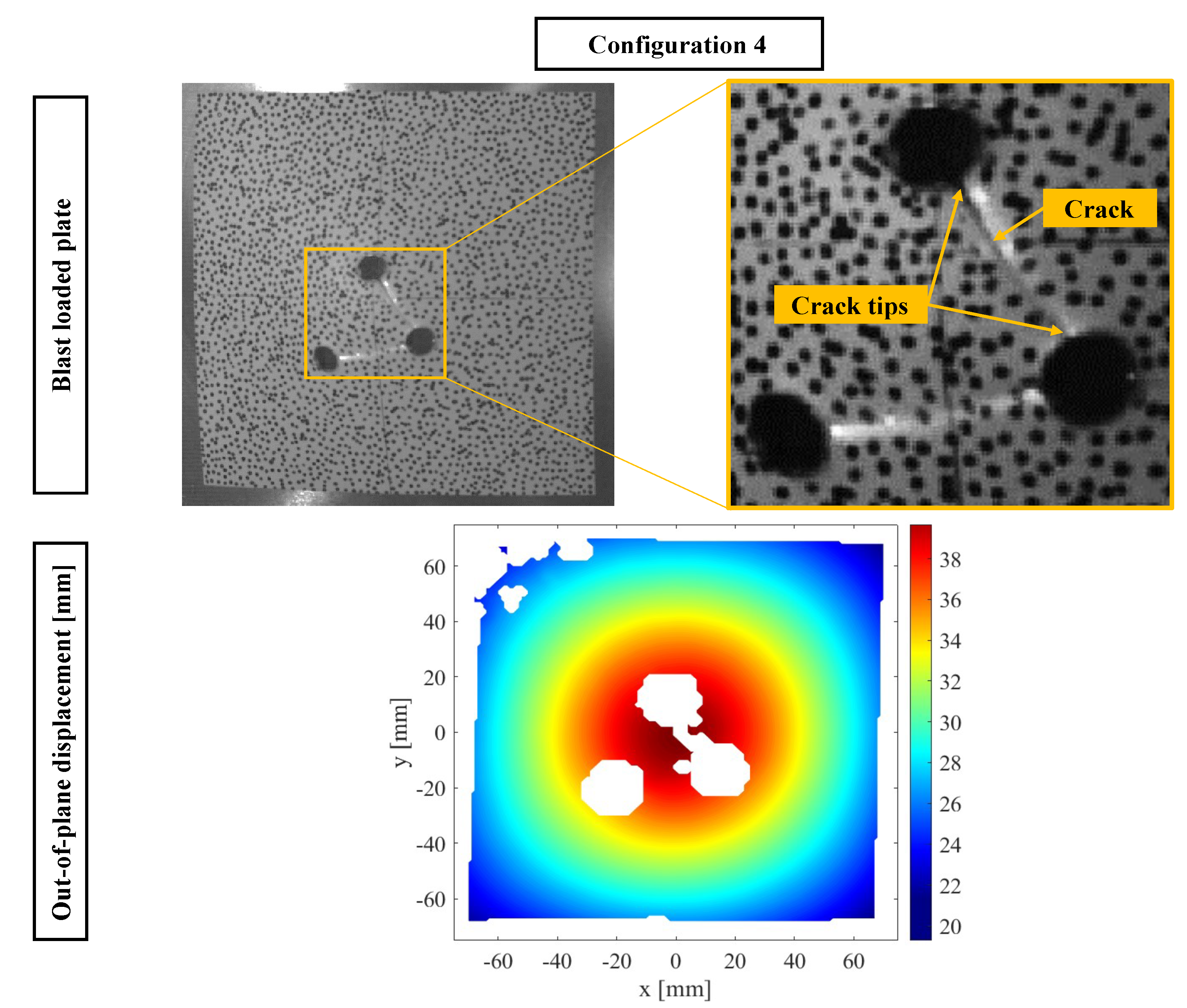
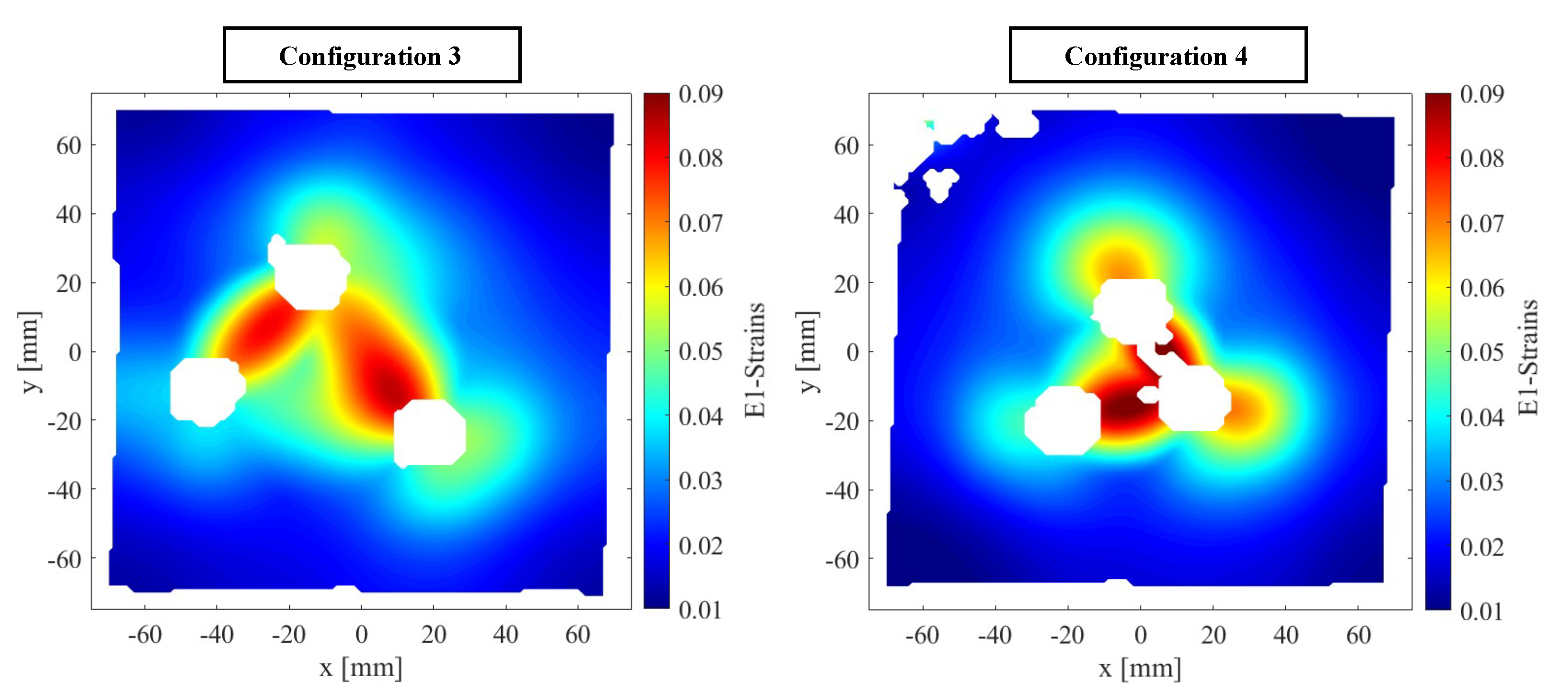
3.2.2. Blast-Loaded Plates with Three FSP Impacts
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Improvised Explosive Device (IED) Monitor 2021. Available online: https://aoav.org.uk/2022/explosive-violence-monitor-2021/ (accessed on 21 February 2023).
- Terrorism Situation and Trend Reports 2022. Available online: https://www.europol.europa.eu/cms/sites/default/files/documents/Tesat_Report_2022_0.pdf (accessed on 21 February 2023).
- Atoui, O.; Kechagiadakis, G.; Moumen, A.; Maazoun, A.; Belkassem, B.; Pyl, L.; Lecompte, D. An explosive driven shock tube-based laboratory scale test for combined blast and fragment impact loading. Appl. Sci. 2022, 12, 6854. [Google Scholar] [CrossRef]
- Grisaro, H.Y.; Dancygier, A.N. Characteristics of combined blast and fragments loading. Int. J. Impact Eng. 2018, 116, 51–64. [Google Scholar] [CrossRef]
- Nyström, U.; Gylltoft, K. Numerical studies of the combined effects of blast and fragment loading. Int. J. Impact Eng. 2009, 36, 995–1005. [Google Scholar] [CrossRef]
- Marchand, K.A.; Vargas, M.; Nixon, J.D. The Synergistic Effects of Combined Blast and Fragment Loadings; Technical Report; Southwest Research Institute: San Antonio, TX, USA, 1992. [Google Scholar]
- Hyde, D.W. Microcomputer Programs CONWEP and FUNPRO: Fundamentals of Protective Design for Conventional Weapons User’s Guide; Technical Report; Army Engineer Waterways Experiment Station Vicksburg MS Structures Lab: Vicksburg, MS, USA, 1988. [Google Scholar]
- Børvik, T.; Dey, S.; Clausen, A. Perforation resistance of five different high-strength steel plates subjected to small-arms projectiles. Int. J. Impact Eng. 2009, 36, 948–964. [Google Scholar] [CrossRef]
- Aune, V.; Fagerholt, E.; Hauge, K.O.; Langseth, M.; Børvik, T. Experimental study on the response of thin aluminium and steel plates subjected to airblast loading. Int. J. Impact Eng. 2016, 90, 106–121. [Google Scholar] [CrossRef]
- Grisaro, H.Y.; Dancygier, A.N. Representation of damage caused by fragmentation impact in design and analysis of reinforced concrete barriers. Eng. Struct. 2019, 197, 109387. [Google Scholar] [CrossRef]
- Osnes, K.; Dey, S.; Hopperstad, O.S.; Børvik, T. On the dynamic response of laminated glass exposed to impact before blast loading. Exp. Mech. 2019, 59, 1033–1046. [Google Scholar] [CrossRef]
- Cai, S.; Liu, J.; Zhang, P.; Li, C.; Cheng, Y.; Chen, C. Experimental study on failure mechanisms of sandwich panels with multi-layered aluminum foam/UHMWPE laminate core under combined blast and fragments loading. Thin-Walled Struct. 2021, 159, 107227. [Google Scholar] [CrossRef]
- Li, L.; Zhang, Q.C.; Zhang, R.; Wang, X.; Zhao, Z.Y.; He, S.Y.; Han, B.; Lu, T.J. A laboratory experimental technique for simulating combined blast and impact loading. Int. J. Impact Eng. 2019, 134, 103382. [Google Scholar] [CrossRef]
- Li, W.; Wang, P.; Feng, G.p.; Lu, Y.g.; Yue, J.z.; Li, H.m. The deformation and failure mechanism of cylindrical shell and square plate with pre-formed holes under blast loading. Def. Technol. 2021, 17, 1143–1159. [Google Scholar] [CrossRef]
- Li, Y.; Wu, W.; Zhu, H.; Wu, Z.; Du, Z. The influence of different pre-formed holes on the dynamic response of square plates under air-blast loading. Eng. Fail. Anal. 2017, 78, 122–133. [Google Scholar] [CrossRef]
- Granum, H.; Aune, V.; Børvik, T.; Hopperstad, O.S. Effect of heat-treatment on the structural response of blast-loaded aluminium plates with pre-cut slits. Int. J. Impact Eng. 2019, 132, 103306. [Google Scholar] [CrossRef]
- Rakvåg, K.G.; Underwood, N.; Schleyer, G.; Børvik, T.; Hopperstad, O.S. Transient pressure loading of clamped metallic plates with pre-formed holes. Int. J. Impact Eng. 2013, 53, 44–55. [Google Scholar] [CrossRef]
- Elveli, B.S.; Berstad, T.; Børvik, T.; Aune, V. Performance of thin blast-loaded steel plates after ballistic impact from small-arms projectiles. Int. J. Impact Eng. 2023, 173, 104437. [Google Scholar] [CrossRef]
- Aune, V.; Fagerholt, E.; Langseth, M.; Børvik, T. A shock tube facility to generate blast loading on structures. Int. J. Prot. Struct. 2016, 7, 340–366. [Google Scholar] [CrossRef]
- Yu, R.; Zhang, Q.; Wei, Z.; Li, L.; Yue, Z.; Wang, X.; Lu, T.J. Dynamic response of fully-clamped steel plate under laboratory-simulated sequential fragment impact and blast loading. Thin-Walled Struct. 2023, 182, 110144. [Google Scholar] [CrossRef]
- Ballistic Equipment For Protective Material Testing 2017. Available online: http://www.prototypa.com/file/2522/prototypa-production-protection-2017-02-reduced-quality.pdf (accessed on 21 February 2023).
- Ballistic Test Method for Personal Armor Materials and Combat Clothing: STANAG 2920 PPS Edition 2. Available online: http://www.englands1.com/site/wp-content/uploads/8_stanag_2920.pdf (accessed on 13 December 2022).
- Moumen, A.; Stirbu, B.; Grossen, J.; Laboureur, D.; Gallant, J.; Hendrick, P. Particle image velocimetry for velocity measurement of muzzle flow: Detailed experimental study. Powder Technol. 2022, 405, 117509. [Google Scholar] [CrossRef]
- Louar, M.A. Estimation of the strain rate hardening model parameters of GFRE using blast loads and inverse modelling. Ph.D. Thesis, Vrije Universiteit Brussel, Brussel, Belgium, 2017. [Google Scholar]
- Sutton, M.A.; Orteu, J.J.; Schreier, H.W. Image Correlation for Shape, Motion and Deformation Measurements: Basic Concepts, Theory and Applications; Springer: Berlin/Heidelberg, Germany, 2009; p. 321. [Google Scholar] [CrossRef]
- Louar, M.A.; Belkassem, B.; Ousji, H.; Spranghers, K.; Kakogiannis, D.; Pyl, L.; Vantomme, J. Explosive driven shock tube loading of aluminium plates: Experimental study. Int. J. Impact Eng. 2015, 86, 111–123. [Google Scholar] [CrossRef]
- Ousji, H.; Belkassem, B.; Louar, M.A.; Kakogiannis, D.; Reymen, B.; Pyl, L.; Vantomme, J. Parametric study of an explosive-driven shock tube as blast loading tool. Exp. Tech. 2016, 40, 1307–1325. [Google Scholar] [CrossRef]
- PCB Dynamic Pressure Sensors for High Frequency Measurements. Available online: https://www.pcb.com/Contentstore/MktgContent/LinkedDocuments/Pressure/TM-PRS-113B-102B_lowres.pdf (accessed on 21 March 2022).









| Material | Composition | Density | Young’s Modulus | Poisson’s Ratio | Plate Dimensions |
|---|---|---|---|---|---|
| [kg/m] | [GPa] | [-] | [mm × mm × mm] | ||
| Al | 1050 A | 2710 | 69 | 0.33 |
| Configuration | Test | Number of FSPs | FSP Caliber | Impact Position |
|---|---|---|---|---|
| [mm] | [mm, mm] | |||
| Test 1 | 1 | 7.62 | [17, 10] | |
| Configuration 1 | Test 2 | 7.62 | [7, 21] | |
| Test 3 | 7.62 | [−15, −17] | ||
| Test 1 | 1 | 12.7 | [−4, 0] | |
| Configuration 2 | Test 2 | 12.7 | [−1, 0] | |
| Test 3 | 12.7 | [3, −1] | ||
| Test 1 | 3 | 7.62 | [20, 55], [38, −11], [−23, −17] | |
| Configuration 3 | Test 2 | 7.62 | [−13, 22], [19, −24], [−43, −11] | |
| Test 3 | 7.62 | [−16, 8], [30, −4], [4, −46] | ||
| Configuration 4 | Test 1 | 3 | 12.7 | [−3, 11], [−21, −22], [15, −14] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Atoui, O.; Maazoun, A.; Aminou, A.; Belkassem, B.; Pyl, L.; Lecompte, D. Dynamic Behavior of Aluminum Plates Subjected to Sequential Fragment Impact and Blast Loading: An Experimental Study. Appl. Sci. 2023, 13, 3542. https://doi.org/10.3390/app13063542
Atoui O, Maazoun A, Aminou A, Belkassem B, Pyl L, Lecompte D. Dynamic Behavior of Aluminum Plates Subjected to Sequential Fragment Impact and Blast Loading: An Experimental Study. Applied Sciences. 2023; 13(6):3542. https://doi.org/10.3390/app13063542
Chicago/Turabian StyleAtoui, Oussama, Azer Maazoun, Aldjabar Aminou, Bachir Belkassem, Lincy Pyl, and David Lecompte. 2023. "Dynamic Behavior of Aluminum Plates Subjected to Sequential Fragment Impact and Blast Loading: An Experimental Study" Applied Sciences 13, no. 6: 3542. https://doi.org/10.3390/app13063542
APA StyleAtoui, O., Maazoun, A., Aminou, A., Belkassem, B., Pyl, L., & Lecompte, D. (2023). Dynamic Behavior of Aluminum Plates Subjected to Sequential Fragment Impact and Blast Loading: An Experimental Study. Applied Sciences, 13(6), 3542. https://doi.org/10.3390/app13063542






